Calcium hydride with aluminium for thermochemical energy storage applications

Abstract

Thermochemical energy storage has the potential to unlock large-scale storage of renewable energy sources by integrating with power production facilities. Metal hydrides have high thermochemical energy storage densities through reversible hydrogenation. Particularly, calcium hydride presents remarkable properties to integrate with high-temperature systems. The addition of aluminium to calcium hydride enables lower operating temperatures below 700 °C. The CaH₂–2Al system reacts through a two-step reaction mechanism, which was verified via in situ powder diffraction analysis. The thermodynamics of dehydrogenation have been determined for both dehydrogenation steps with step 1 having a ΔH_des = 79 ± 3 kJ mol⁻¹ and ΔS_des = 113 ± 4 J mol⁻¹ K⁻¹, while step 2 has a ΔH_des = 99 ± 4 kJ mol⁻¹ and ΔS_des = 128 ± 5 J mol⁻¹ K⁻¹. The reaction kinetics for both steps were determined using the Kissinger method from DSC-TGA data to be 138 ± 12 kJ mol⁻¹ and 98 ± 8 kJ mol⁻¹ for step 1 and 2, respectively. Reversible hydrogenation over step 2, for 66 cycles at 670 °C under 20 bar of H₂, determined the sorption capacity to be stable at 91% of the theoretical maximum of 1.1 wt% H₂. A materials-based cost analysis evaluates the system at 9.2 US$ per kW h_th, with an energy density of 1031 kJ kg⁻¹.

---

Introduction

The global energy landscape must rapidly transition from fossil fuels to alternative systems to reduce the emission of greenhouse gases.¹ Energy storage is key to ensure the decarbonisation of energy production while keeping up with energy demand. The diversification of energy storage systems is necessary to enable the efficient utilisation of renewable resources. For example, thermal energy storage (TES) is appropriate to integrate concentrated solar power (CSP) plants.² Particularly, recent TES studies show that thermochemical energy storage (TCES) materials have the potential to be implemented into thermochemical batteries (TCB) providing energy storage solutions for power production facilities.³

Metal hydrides have been reported to be suitable for diverse applications including fuel cells, hydrogen storage, and solid state batteries but they also show exceptional properties for TCES, with volumetric energy storage densities up to 2423 kW h_th m⁻³ and operating temperatures ranging from 200 to 1000 °C.^4–6 A recent paper by Adams et al. highlighted the advantages of metal hydrides for TCES, and addressed the challenges related to their implementation in large-scale systems in terms of reaction modelling, reactor material selection and design.⁷ Hardy et al. modelled a high-temperature TCES system operating with a metal hydride pair for solar energy storage; of which the outcomes confirmed that appropriate operating conditions and sizing are crucial to ensure the viability of the system and avoid failure due to thermal ratcheting.⁸ Feng et al. proposed an optimal reactor design methodology with heat transfer fluid flowing through a regular helical tube placed in the reactor bed powder to optimise energy storage using a metal hydride.⁹ Experimental TCB prototypes using MgH₂ or Mg₂FeH₆ have been investigated at medium temperatures below 500 °C.^10–14 For example, Poupin et al. built a prototype using 900 g of magnesium iron hydride and a coil heat exchanger flowing supercritical water; the cycling showed promising results at ∼450 °C with an energy storage capacity reaching 87% of the theoretical maximum and a heat extraction efficiency of 80%.¹² Recently, the CaH₂–MgH₂–ZrCl₄ material developed by Singh et al. showed reversible hydrogenation below 400 °C with improved kinetics, which could be used for medium temperature TCES.¹⁵ Although there have been some excellent advances in this field, the development of metal hydrides that operate at ≈700 °C is required to enable efficient heat-to-power engines to be used at their optimal operating temperature.¹⁶

The understanding of the intrinsic physical properties of metal hydrides is crucial to evaluate their potential to integrate TCES system.¹⁷ Recently, Balakrishnan et al. updated the thermodynamics and kinetics of pure calcium hydride at high temperature in the molten and solid state.¹⁸ Calcium hydride is an appealing material because of its high energy storage density (5131 kJ kg⁻¹), but the decomposition temperature of above 900 °C and the sluggish reaction kinetics hinder the utilisation of pure CaH₂ for technological applications.¹⁸ In the past decades, several studies looked at the thermodynamic destabilisation of CaH₂ with additives to reduce the operating temperature and enhance the reaction kinetics.¹⁹ The CaH₂–11Zn system was determined to operate at 1 bar of H₂ and ∼600 °C, where cyclic reversibility of the first reaction step over 10 cycles resulted in the loss of 0.02 wt% sorption of H₂.²⁰ The addition of Si to CaH₂ was shown to cause decomposition via multistep reactions forming Ca_xSi_y compounds with reversibility at 700–800 °C and hydrogen partial pressure of 1–5 bar, which overall, showed potential for TCES applications.²¹ Sofianos et al. destabilised CaH₂ with calcium halides.²² The resulting operating temperature (650 °C) and pressure (1–10 bar) are suitable for TCES for CSP; however, the particle sintering and slow reaction kinetics are important issues compromising the viability of these systems for application.²² Similarly, the addition of Al₂O₃ to CaH₂ (1 : 1 molar ratio) showed sintering resulting in poor cycling capacity, but reducing the initial amount of additives may promote the sorption stability of this system operating at 636 °C and 1 bar of H₂.²³

In 1981, Veleckis investigated Ca–Al alloys and reported the formation of CaAl₄ and CaAl₂ in a two-step reaction process (eqn (1) and (2)), along with the conclusion that the system has potential as a high temperature hydrogen storage medium due to its reversible hydrogenation capabilities at 450–650 °C.²⁴ An absorption enthalpy of −83.1 kJ mol⁻¹ H₂ was also determined for the reaction of CaAl₂ with H₂, giving a theoretical energy density of 865 kJ kg⁻¹ CaAl₂. More recently, Ward et al. studied the reversible hydrogenation of the CaAl₂ system at 600 °C and 25 bar H₂ over 100 cycles.²⁵ In this case cycling was carried out following eqn (3), which has a theoretical H₂ capacity of 2.1 wt%. The cycling experiment only showed a slight capacity decrease of 3% from 1.9 to 1.85 wt% H₂. The thermal conductivity of CaAl₂ was also measured and increases from 6.8 W m⁻¹ K⁻¹ at 80 °C to 20.3 W m⁻¹ K⁻¹ at 600 °C.²⁵

CaH₂ + 4Al ⇌ CaAl₄ + H₂ (1)

CaH₂ + CaAl₄ ⇌ 2CaAl₂ + H₂ (2)

CaH₂ + 2Al ⇌ CaAl₂ + H₂ (3)

Previous studies have shown the CaH₂–Al system to be a promising TCES material due to its cyclability and reasonable cost of $14.9 USD per kW h_th.^20,24,25 Further work is required to determine the physical attributes of this material and determine whether cycling is achievable at higher temperatures (>650 °C). This study will determine the thermodynamics and activation energies for both steps of hydrogen desorption of the CaH₂–2Al system, while also ascertaining the exact decomposition pathway using X-ray diffraction techniques. Overall, this allows the determination of the operational conditions for cycling and a direct cost evaluation to be undertaken.

Experimental section

Sample preparation

All manipulations of chemicals were undertaken in an argon atmosphere using an Mbraun Unilab glovebox to prevent air exposure and to minimise oxygen (O₂ < 1 ppm) and water (H₂O < 1 ppm) contamination. CaH₂ + 2Al samples were prepared by ball milling (BM) CaH₂ (Sigma-Aldrich, >95%) and Al (Alfa Aesar, −100 + 325 mesh, >99.5% metals basis) in a 1 : 2 ratio at room temperature in a planetary ball mill (Fritsch Pulverisette 6, Germany). The powders were milled at 400 rpm for 6 h (15 min milling with 5 min break, bidirectional) with a ball-to-powder mass ratio of 30 : 1 using 316 stainless steel vial (80 mL) and balls (10 mm in diameter) under an Ar atmosphere.

Powder X-ray diffraction

In-house powder X-ray diffraction (XRD) was performed using a Bruker D8 Discovery diffractometer (Co K_α radiation) utilising XRD sample holders covered with a poly(methylmethacrylate) (PMMA) airtight dome to prevent oxygen/moisture contamination during data collection. The PMMA airtight dome results in a broad hump in XRD patterns centred at ∼23° 2θ. Data were acquired over a 2θ range of 10–90°, with a step size of 0.03° and count time of 1.8 s per step. Synchrotron powder X-ray diffraction (SR-XRD) was performed at the Australian Synchrotron in Melbourne, Australia.²⁶ Powder samples were loaded in quartz capillaries (outer diameter 0.7 mm, wall thickness 0.01 mm) while inside a glove box, and mounted using Swagelok tube fittings to a vacuum manifold and measured under dynamic vacuum. One-dimensional SR-XRD patterns (monochromatic X-rays with λ = 0.825040(5) Å) were continuously collected using a Mythen microstrip detector with an exposure time of 30 s at two different detector positions in order to cover the entire 2θ range (3–80°).²⁷ The capillary was continuously oscillated through 100° angle during data collection to improve the powder averaging and ensure even heating. The capillary caused a broad amorphous hump between 2θ = 10 and 15° that is visible throughout the in situ experiment. Diffraction patterns were quantitatively analysed with the Rietveld method using TOPAS (Bruker-AXS).²⁸

Thermal analysis

The hydrogen sorption properties of the CaH₂–2Al mixture were measured with a computer controlled Sieverts/volumetric apparatus previously described elsewhere.²⁹ Pressure Composition Isotherms (PCI) were collected between 520 and 591 °C on a sample weighing 1.7085 g in a system volume of 78.07 cm³. Each equilibrium data point was conducted with an initial wait time of 2 h followed by a check to ensure an increment of <0.008 wt% H₂ was not exceeded over the following 2 h, ensuring hydrogen desorption/absorption had ceased. Subsequent equilibrium data points were then collected after a 3 bar pressure decrement (reference volume pressure) was applied. Sample temperature and pressure were recorded every 30 s using a K-type thermocouple (uncertainty of ±1.5 °C) inserted directly into the sample and a digital pressure transducer (Rosemount 3051S) with a 0.035% span accuracy (200 bar span). Powder samples were loaded into a SiC sample cell (Saint-Gobain) to prevent hydrogen permeability at high temperature.³⁰ The sample cell was then placed under ∼25 bar H₂ back pressure to inhibit decomposition and heated at a ramp rate of 10 °C min⁻¹ to the desired temperature before each PCI was initiated. Hydrogen cycling experiments were carried out using the same experimental setup as for the PCI experiments. In this case, the ball milled powder (0.4376 g) was heated to 670 °C under vacuum for the purpose of commencing the experiment in a desorbed state. Absorption was then undertaken at 20 bar for 2.3 h, while desorption was carried out in vacuo for 1 h. A total of 66 cycles were measured.

Simultaneous Differential Scanning Calorimetry (DSC) was performed with a Netzsch STA 449 F3 Jupiter analyser equipped with a Pt furnace. Samples were measured under an argon flow of 40 mL min⁻¹ using Pt/Rh crucibles with Al₂O₃ liners containing approximately 15 mg of sample, loaded under argon and sealed with lids possessing a pin-hole to allow for gas release. The furnace containing the sample was evacuated prior to being placed under an argon flow to remove traces of air. The analysis was conducted by heating from 40–800 °C at 2, 5, 10 and 20 °C min⁻¹. The temperature and sensitivity of the DSC was calibrated using In, Bi, Al, Ag and Au reference materials, resulting in a temperature accuracy of ±0.2 °C, while the balance has an accuracy of ±20 μg. The DSC signal data was processed using a Gaussian deconvolution fitting model available in the OriginPro software. The Kissinger method was applied to determine the activation energy E_a for hydrogen desorption using the peak temperature T_p of the DSC signal at the different heating rates β.³¹ Equation (eqn (4)) was used to generate the Kissinger plot:³²

The activation energy was calculated by multiplying the slope of the resulting trendline by the universal gas constant R (8.314 J K⁻¹ mol⁻¹).³³

Results and discussion

SR-XRD analysis of the milled powder (Fig. 1a) indicated that only CaH₂ (45.7(1) wt%) and Al (54.3(1) wt%) were present in the sample with no oxide contamination. This ensures that no reaction occurred during milling and the mixture is in a molar ratio of 1 : 1.9 CaH₂ : Al. From these stoichiometries, a total practical H₂ capacity of 2.02 wt% for this prepared material is feasible compared to the theoretical capacity of 2.1 wt% H₂. During the in situ SR-XRD analysis, the sample was heated under dynamic vacuum from room temperature to 750 °C (Fig. 1b and c). No crystallographic changes occurred in the sample until ∼355 °C when the Bragg reflections of CaH₂ and Al began to diminish and CaAl₄ was observed. The quantity of CaAl₄ reached a maximum at 535 °C with 60 wt% being present in the sample, along with 22.5 wt% CaH₂ and 8 wt% Al remaining. The residual 9.5 wt% was CaAl₂, which was first observed at ∼480 °C. Elemental Al was no longer observed after 560 °C, while all CaH₂ was depleted by 610 °C. The Al was consumed at a greater rate than CaH₂ to facilitate the formation of the Al-rich CaAl₄ phase. The CaAl₄ was completely consumed by 700 °C leaving only CaAl₂. During the decomposition process, Ca or CaO were not detected. Therefore, the reaction pathway can be expressed as:

CaH₂ + 2Al ⇌ ½CaAl₄ + ½CaH₂ + ½H₂ (5)

½CaAl₄ + ½CaH₂ ⇌ CaAl₂ + ½H₂ (6)

Fig. 1 (a) Room temperature SR-XRD pattern and Rietveld refinement plot of CaH₂–2Al material after ball milling. Experimental data as red circles, calculated diffraction pattern as black line and the difference plot in blue. Tick marks show positions for: Al (54.3(1) wt%) and CaH₂ (45.7(1) wt%), top to bottom respectively. λ = 0.825040(5) Å. (b) In situ SR-XRD data of CaH₂–2Al during heating from room temperature to 750 °C (8 °C min⁻¹). The coloured circles indicate the peaks assigned to CaH₂ (white), Al (red), CaAl₄ (blue) and CaAl₂ (brown). (c) Quantitative Rietveld refinement analysis of the in situ SR-XRD data.

The kinetics of the hydrogen desorption reaction were studied by DSC at different heating rates in order to produce a Kissinger plot for each reaction step (Fig. 2a and b) and determine the activation energy (E_a) of the reactions. The DSC profile under flowing argon doesn't show two resolved peaks expected for this system under all ramp rates. Instead, the first decomposition step is denoted by a broad event, indicative of slow kinetics, which is convoluted with the second, well defined event. This convolution is corroborated by the in situ SR-XRD data (Fig. 1b) where the formation of CaAl₄ and CaAl₂ occur within overlapping temperature regions. Deconvolution of the data (Fig. 2c–e) shows peak maxima for the first step at 403, 427, 449 and 466 °C for ramp rates of 2, 5, 10 and 20 °C, respectively. This provides an E_a of 138 ± 12 kJ mol⁻¹ H₂. The peak maxima for the second step are 416, 464, 487 and 507 °C for ramp rates of 5, 10 and 20 °C, respectively. This provides E_a of 98 ± 8 kJ mol⁻¹ H₂. These values are of the same order of magnitude considering the uncertainties of the measurements, which suggests similar kinetics for hydrogen desorption for both steps. In addition, these E_a values are consistent compared to other hydrides that exhibit rapid kinetics. The second step of decomposition for SrH₂ + 2Al, which is analogous to this system, was determined to be 156 ± 10 kJ mol⁻¹ H₂,³⁴ and also comparable to other metal hydrides such as MgH₂ (161 ± 15 kJ mol⁻¹ H₂),³⁵ and NaMgH₃ (160 ± 9 kJ mol⁻¹ H₂).³⁶ Fast reaction kinetics are required for TES applications and hydrogen storage applications. In terms of TCES applications, fast absorption of hydrogen permits heat generation at rapid rates implying that electricity production is not hindered by the material physical properties. Conversely, a thermochemical battery can also charge quickly by rapid absorption of heat during hydrogen release.

Fig. 2 (a) Kissinger plot for CaH₂ + 2Al for the first decomposition step, and (b) the second decomposition step determined by DSC analysis. Peak deconvolution: (c) 2 °C min⁻¹. (d) 5 °C min⁻¹. (e) 10 °C min⁻¹. (f) 20 °C min⁻¹.

The thermodynamics for both steps of the decomposition reaction were evaluated by PCI analysis over five temperatures between 520 and 591 °C (Fig. 3a). The combined H₂ capacity for both steps is 2.1 wt% H₂ and corresponds to the theoretical amount of 2.1 wt% H₂, with step 1 and 2 having 1.1 wt% and 1 wt%, respectively. This also corroborates the 2.02 wt% H₂ determined by SR-XRD on the pristine sample. The next noticeable point is that each of the hydrogen release steps has a sloping equilibrium plateau. Ideally, a TCES material should have a flat plateau at its equilibrium pressure, both for absorption and desorption, with little hysteresis. This sloping plateau has been noted in various metal hydride systems such as NaH_xF_1−x,³⁷ Mg(H_xF_1−x)₂,³⁸ NaMgH₂F³⁹ and 2CaH₂ + Si,²¹ and is attributed to the formation of solid solutions of varying compositions within the material. Ca and Al can form alloy systems.⁴⁰ Particularly, at 7.7% Ca solubility in Al an Al–CaAl₄ eutectic forms at 616 °C (above the temperatures used in this study),⁴⁰ while CaAl₂ is known to form CaAl₂–xM_x solid solutions, where M can be Cu, Mg, Ni and Zn.⁴¹ Unfortunately, it is unclear which solid solutions formed in this study, due to there being no clear evidence from the SR-XRD experiments.

Fig. 3 (a) Pressure composition isotherms (desorption) of CaH₂ + 2Al (eqn (1)) between 520 and 591 °C, (b) van't Hoff plot for step 1 (eqn (2)) and (c) van't Hoff plot for step 2 (eqn (3)).

Van't Hoff plots were constructed for both steps of the reaction and are illustrated in Fig. 3b and c. Step 1 has a ΔH_des = 79 ± 3 kJ mol⁻¹ H₂ and ΔS_des = 113 ± 4 J K⁻¹ mol⁻¹ H₂ with a R² factor of 0.9998, which equates to a T_des(1bar) = 426 ± 22 °C. Step 2 has a ΔH_des = 99 ± 4 kJ mol⁻¹ H₂ and ΔS_des = 128 ± 5 J K⁻¹ mol⁻¹ H₂ with a R² factor of 0.9995, which equates to a T_des(1bar) = 500 ± 28 °C. These thermodynamics show that the addition of Al to CaH₂ has destabilised the hydrogen release from CaH₂, causing a decrease in the ΔH_des, ΔS_des, and operating temperature compared to pure CaH₂. Balakrishnan et al. recently determined the decomposition thermodynamics of molten CaH₂ to be ΔH_des = 216 ± 10 kJ mol⁻¹ H₂ and ΔS_des = 177 ± 9 J K⁻¹ mol⁻¹ H₂, giving T_des(1bar) of 947 ± 65 °C.¹⁸ This is 524 °C higher than the first step of decomposition of CaH₂ + 2Al and 447 °C higher than the second step.

Since the thermodynamics of the CaH₂ + 2Al system were determined by PCI analysis, the operating temperatures can be calculated for any operating temperature and pressure, and these are illustrated in Fig. 4a. The ideal scenario for this system to achieve technological application as a storage material for a thermochemical battery is to operate at temperatures above 600 °C at a pressure as low as possible to reduce safety hazards and minimise engineering costs. A temperature of 670 °C was chosen for the cycling experiment, which has a theoretical equilibrium pressure of 15.1 bar, but due to the sloping plateau observed by PCI a working pressure of 20 bar was chosen to ensure full H₂ absorption. Decomposition was undertaken in vacuo for 1 h. This allows complete cycling over the 2^nd step at a theoretical hydrogen capacity of 1 wt% without operating over the 1^st reaction step to maintain a single-step reaction pathway. This is complementary to the previous study by Ward et al. who cycled over both steps but at a temperature of 600 °C and 23 bar H₂ (see Fig. 4a).²⁵ Lower operating pressure and higher operating temperatures ensure better operating conditions for thermal battery applications utilising a Stirling engine and also integration in to CSP. As such, this study aimed to investigate the performance of this system at higher temperatures and at lower or similar pressures. Sixty-six sorption cycles were undertaken with fast kinetics observed and hydrogen absorption reaching 91% capacity in 2 h (Fig. 4b and c). The cycling capacity appears to decrease over cycles following a linear trend (Fig. 4b), which suggests that the capacity will be nil after 821 cycles. However, it is likely that the decreasing trend won't be linear over a large number of cycles and will converge towards an asymptote, as it is observed with most TCES materials.

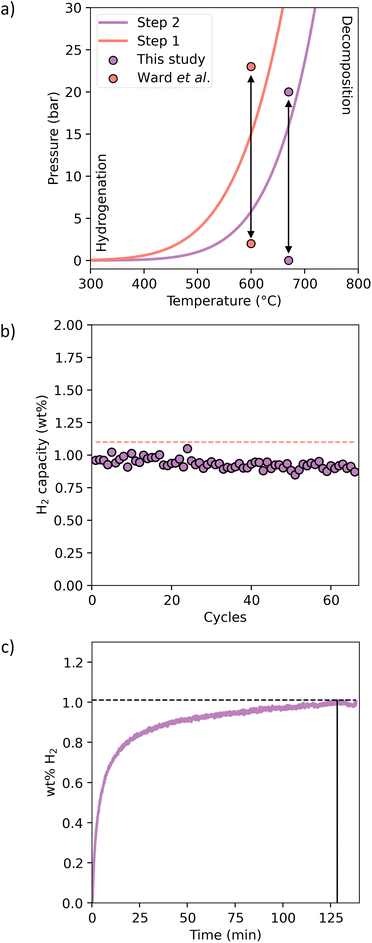Fig. 4 (a) Ca–Al–H₂ equilibrium diagram deduced from the determined enthalpy and entropy for both reaction steps showing the cycling parameters of this study compared to a previous study (ref. 25). (b) Hydrogen sorption capacity over 66 cycles relative to the theoretical maximum sorption capacity. (c) Hydrogen absorption kinetics on cycle 1.

Cycling was finalised after an absorption step and allowed to cool under 20 bar H₂. The material retrieved from the reactor had agglomerated into a solid ingot. This likely attributed to the reduction in cycling capacity as H₂ is restricted from reacting with the sample in a short timescale. This could be reduced by the addition of a particle refinement material such as TiB₂, which has been shown to aid cyclic reversibility.³⁶ Unfortunately, the ingot was impossible to powderise and so adequate XRD analysis and subsequent quantitative Rietveld refinement analysis was impossible. XRD analysis was performed after PCI analysis (Fig. S1†), where the same sample was employed throughout the experiments, and therefore cycled at least five times. The sample was left in a desorbed state and phase identification of the material by XRD determined only CaAl₂ to be present with no other impurities such as oxides.

A materials-based cost analysis of the system was undertaken to determine the ultimate feasibility of CaH₂–2Al as a TCES material (Table 1). This was calculated for systems using just the first reaction step (eqn (1)), just the second step (eqn (2)) and both reactions (eqn (3)). Assuming a cost of $2.57, $2.68 and $3 USD per kg for Ca, Al and H₂, respectively, a cost of $2.6 USD per kg was determined for the starting raw material without processing costs.⁴² If the first step was to be used, where ΔH_des = 79 kJ mol⁻¹ H₂, an energy density of 822 kJ kg⁻¹ is determined and therefore leads to a materials cost of $11.6 USD per kW h_th.

Table 1 Cost comparison study of the CaH₂ + 2Al system with other metal hydride TES materials and molten salt for thermal battery applications^a

TES material	ΔH (kJ mol^−1 H2)	ΔH (kJ kg^−1)	Material cost (US$ per tonne)	Material cost (US$ per kW hth)	T (°C)	H2P (bar)	Mass required^b (tonnes)	Ref.
a Note that values were calculated in an assumption of 100% conversion of reactants. b To generate 1 TJ of energy.
CaH2 + 2Al (1^st step)	79	822	2641	11.6	426	1	2810	This work
CaH2 + 2Al (2^nd step)	99	1031	2637	9.2	500	1	2360	This work
CaH2 + 2Al (both steps)	178	1853	2641	5.1	500	1	1310	This work
CaH2	216	5131	2591	1.8	947	1	254	18
SrH2	183	2041	5443	9.6	1070	1	322	34
SrH2 + 2Al	132	919	4375	17.1	846	1	742	34
CaH2 + 11Zn	131	172	2649	55.4	597	1	8920	43
3CaH2 + 2Al2O3	100	909	2460	9.7	636	1	1650	43
Molten salt (40NaNO3 : 60KNO3)	39	413	630	5.8	565	—	5250	44

---

Employing just the second step of the reaction leads to a higher ΔH_des = 99 kJ mol⁻¹ H₂, which leads to a higher energy density of 1031 kJ kg⁻¹ and therefore a lower material cost of $9.2 USD per kW h_th. Allowing the TES material to operate over both steps may be advantageous as the ΔH_des is 178 kJ mol⁻¹ H₂, which provides a material cost of $5.1 USD per kW h_th. As such, there are advantages in operating the CaH₂–2Al system over both the steps from an energy density and materials cost perspective. However, operating over both steps requires a larger gas pressure swing in the system, which may negatively impact the engineering cost of the system by requiring larger hydrogen gas storage tanks and/or reducing the efficiency of gas compression/expansion.

Compared to the other high temperature metal hydrides in Table 1, the CaH₂–2Al system operates at a lower temperature (500 °C at 1 bar H₂) than others, for example, CaH₂–11Zn which operates at 597 °C. This is also below the maximum operating temperature for molten nitrate salts (565 °C) but is similar in cost to molten salts ∼5–6 US$ per kW h_th. Conversely, due to the significantly higher energy density possessed by CaH₂–2Al, only 25% of the total mass is required, which may save on storage space, despite the requirement for H₂ gas storage facilities. Overall, CaH₂–2Al has the potential to operate at elevated temperatures, despite the requirement for higher pressures, and is cost competitive compared to other high temperature metal hydrides. In addition, there is little chance of materials to sublime and condense (and ultimately segregate) compared to materials such as NaH_xF_1−x or Mg(H_xF_1−x)₂.^37,38

Conclusions

A stoichiometric mixture of CaH₂ and 2Al was prepared by ball milling and its physical properties analysed to determine its feasibility as a high temperature TCES material for TCB applications. The initial decomposition reaction was determined by synchrotron radiation powder X-ray diffraction, DSC and PCI to be a two-step reaction with the onset of CaAl₄ formation occurring at ∼350 °C and CaAl₂ formation starting at ∼480 °C, with the two reactions being highly convoluted under vacuum or flowing argon conditions. The kinetics of both reactions were determined using the Kissinger method on the DSC data, deeming that both activation energies are of the same order of magnitude at 138 ± 12 kJ mol⁻¹ and 98 ± 8 kJ mol⁻¹, respectively. Determination of the thermodynamics by PCI analysis between 520 and 591 °C established that step one has a ΔH_des = 79 ± 3 kJ mol⁻¹ H₂ and ΔS_des = 113 ± 4 J K⁻¹ mol⁻¹ H₂ giving T_des(1bar) = 426 ± 22 °C. Step 2 has a ΔH_des = 99 ± 4 kJ mol⁻¹ H₂ and ΔS_des = 128 ± 5 J K⁻¹ mol⁻¹ H₂, T_des(1bar) = 500 ± 28 °C. These results allow for a determination of the operating conditions required for a sample to undergo hydrogen cycling for solely the second step. As such, a sample was cycled 66 times at 670 °C with a capacity loss of 9%. Overall, this study has shown that the CaH₂–2Al system is a potential thermal energy storage material that can be used to store energy produced by renewable energy sources or high temperature waste heat.

Author contributions

All authors have given approval to the final version of the manuscript.

Conflicts of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

The authors CEB, MP, and TDH acknowledge the financial support the Department of Industry Science and Resources for the 2019 Global Innovation Linkage (GIL73589) grant and the Australian Research Council (ARC) for Discovery Project (DP200102301). The synchrotron powder diffraction data was collected on the Powder Diffraction beamline at the Australian Synchrotron, part of ANSTO. LD acknowledges the support of the Future Energy Exports CRC (https://www.fenex.org.au) whose activities are funded by the Australian Government's Cooperative Research Centre Program. This is FEnEx CRC Document 2023/22.RP2.0121-PHD-FNX-011.

References

1. IPCC, Strengthening and Implementing the Global Response, in Global Warming of 1.5 °C: IPCC Special Report on Impacts of Global Warming of 1.5 °C above Pre-industrial Levels in Context of Strengthening Response to Climate Change, Sustainable Development, and Efforts to Eradicate Poverty, Cambridge University Press, Cambridge, 2022, ch. 4, pp. 313–444,  DOI:10.1017/9781009157940.006.
2. A. Palacios, C. Barreneche, M. E. Navarro and Y. Ding, Renewable Energy, 2020, 156, 1244–1265.
3. J. Sunku Prasad, P. Muthukumar, F. Desai, D. N. Basu and M. M. Rahman, Appl. Energy, 2019, 254, 113733.
4. C. Corgnale, B. Hardy, T. Motyka, R. Zidan, J. Teprovich and B. Peters, Renewable Sustainable Energy Rev., 2014, 38, 821–833.
5. M. S. Salman, Q. Lai, X. Luo, C. Pratthana, N. Rambhujun, M. Costalin, T. Wang, P. Sapkota, W. Liu, A. Grahame, J. Tupe and K.-F. Aguey-Zinsou, J. Alloys Compd., 2022, 920, 165936.
6. X. Qu, Y. Li, P. Li, Q. Wan and F. Zhai, Front. Mater. Sci., 2015, 9, 317–331.
7. M. Adams, C. E. Buckley, M. Busch, R. Bunzel, M. Felderhoff, T. W. Heo, T. D. Humphries, T. R. Jensen, J. Klug, K. H. Klug, K. T. Møller, M. Paskevicius, S. Peil, K. Peinecke, D. A. Sheppard, A. D. Stuart, R. Urbanczyk, F. Wang, G. S. Walker, B. C. Wood, D. Weiss and D. M. Grant, Prog. Energy, 2022, 4, 032008.
8. B. J. Hardy, C. Corgnale and S. N. Gamble, Sustainability, 2021, 13, 12117.
9. P. Feng, Y. Liu, I. Ayub, Z. Wu, F. Yang and Z. Zhang, Energy Convers. Manage., 2018, 174, 239–247.
10. D. Dong, T. D. Humphries, D. A. Sheppard, B. Stansby, M. Paskevicius, M. V. Sofianos, A. L. Chaudhary, M. Dornheim and C. E. Buckley, Sustainable Energy Fuels, 2017, 1, 1820–1829.
11. M. Paskevicius, D. A. Sheppard, K. Williamson and C. E. Buckley, Energy, 2015, 88, 469–477.
12. L. Poupin, T. D. Humphries, M. Paskevicius and C. E. Buckley, Int. J. Hydrogen Energy, 2021, 46, 38755–38767.
13. R. Urbanczyk, K. Peinecke, S. Peil and M. Felderhoff, Int. J. Hydrogen Energy, 2017, 42, 13818–13826.
14. Z. Z. Fang, C. Zhou, P. Fan, K. S. Udell, R. C. Bowman, J. J. Vajo, J. J. Purewal and B. Kekelia, J. Alloys Compd., 2015, 645, S184–S189.
15. P. K. Singh, A. Singh, V. Kain, Y. Kojima, H. Miyaoka and S. Kumar, Int. J. Hydrogen Energy, 2021, 46, 34362–34368.
16. G. Lundholm, The Experimental V4X Stirling Engine - A Pioneering Development Report number: NEI-SE-497OSTI ID: 20475297, Lund Inst. of Tech., Dept. of Heat and Power Engineering, Sweden, 2003.
17. K. Manickam, P. Mistry, G. Walker, D. Grant, C. E. Buckley, T. D. Humphries, M. Paskevicius, T. Jensen, R. Albert, K. Peinecke and M. Felderhoff, Int. J. Hydrogen Energy, 2019, 44, 7738–7745.
18. S. Balakrishnan, T. D. Humphries, M. Paskevicius and C. E. Buckley, Int. J. Hydrogen Energy, 2023, 48(78), 30479–30488.
19. J. J. Vajo and G. L. Olson, Scr. Mater., 2007, 56, 829–834.
20. S. Balakrishnan, M. V. Sofianos, T. D. Humphries, M. Paskevicius and C. E. Buckley, Phys. Chem. Chem. Phys., 2020, 22, 25780–25788.
21. A. C. M. Griffond, M. V. Sofianos, D. A. Sheppard, T. D. Humphries, A.-L. Sargent, M. Dornheim, K.-F. Aguey-Zinsou and C. E. Buckley, J. Alloys Compd., 2021, 858, 158229.
22. M. V. Sofianos, S. Randall, M. Paskevicius, K.-F. Aguey-Zinsou, M. R. Rowles, T. D. Humphries and C. E. Buckley, J. Alloys Compd., 2020, 819, 153340.
23. S. Balakrishnan, M. V. Sofianos, M. Paskevicius, M. R. Rowles and C. E. Buckley, J. Phys. Chem. C, 2020, 124, 17512–17519.
24. E. Veleckis, J. Less-Common Met., 1981, 80, 241–255.
25. P. A. Ward, J. A. Teprovich, Y. Liu, J. He and R. Zidan, J. Alloys Compd., 2018, 735, 2611–2615.
26. K. S. Wallwork, B. J. Kennedy and D. Wang, AIP Conf. Proc., 2007, 879, 879.
27. B. Schmitt, C. Bronnimann, E. F. Eikenberry, F. Gozzo, C. Hormann, R. Horisberger and B. Patterson, Nucl. Instrum. Methods Phys. Res., Sect. A, 2003, 501, 267–272.
28. R. A. Young and R. A. Young, The Rietveld Method, Oxford University Press, 1995.
29. M. Paskevicius, D. A. Sheppard and C. E. Buckley, J. Am. Chem. Soc., 2010, 132, 5077–5083.
30. R. A. Causey, J. D. Fowler, C. Ravanbakht, T. S. Elleman and K. Verghese, J. Am. Ceram. Soc., 1978, 61, 221–225.
31. S. Vyazovkin, Molecules, 2020, 25, 2813.
32. H. E. Kissinger, Anal. Chem., 1957, 29, 1702–1706.
33. T. Renner, Quantities, Units and Symbols in Physical Chemistry, The Royal Society of Chemistry, 2007.
34. T. D. Humphries, M. Paskevicius, A. Alamri and C. E. Buckley, J. Alloys Compd., 2022, 894, 162404.
35. J. F. Fernández and C. R. Sánchez, J. Alloys Compd., 2003, 356–357, 348–352.
36. L. Poupin, T. D. Humphries, M. Paskevicius and C. E. Buckley, Sustainable Energy Fuels, 2019, 3, 985–995.
37. T. D. Humphries, D. A. Sheppard, M. R. Rowles, M. V. Sofianos and C. E. Buckley, J. Mater. Chem. A, 2016, 4, 12170–12178.
38. M. S. Tortoza, T. D. Humphries, D. A. Sheppard, M. Paskevicius, M. R. Rowles, M. V. Sofianos, K. F. Aguey-Zinsou and C. E. Buckley, Phys. Chem. Chem. Phys., 2018, 20, 2274–2283.
39. D. A. Sheppard, C. Corgnale, B. Hardy, T. Motyka, R. Zidan, M. Paskevicius and C. E. Buckley, RSC Adv., 2014, 4, 26552–26562.
40. S. S. S. Kumari, R. M. Pillai and B. C. Pai, Int. Mater. Rev., 2005, 50, 216–238.
41. S. Amerioun, S. I. Simak and U. Häussermann, Inorg. Chem., 2003, 42, 1467–1474.
42. ISE Metal Quotes, https://ise-metal-quotes.com/, accessed Jan 22, 2023.
43. S. Balakrishnan, M. V. Sofianos, T. D. Humphries, M. Paskevicius and C. E. Buckley, Phys. Chem. Chem. Phys., 2020, 22, 25780–25788.
44. Q. A. Zhang, Y. Nakamura, K. Oikawa, T. Kamiyama and E. Akiba, Inorg. Chem., 2002, 41, 6547–6549.

---

Footnotes

† Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d3se01122d

‡ These authors contributed equally to this work.

---