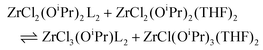View PDF VersionPrevious ArticleNext Article

Open Access Article
This Open Access Article is licensed under a
Creative Commons Attribution 3.0 Unported Licence
DOI: 10.1039/D4DT01299B (Paper) Dalton Trans., 2024, 53, 9862-9873

Complexation and disproportionation of group 4 metal (alkoxy) halides with phosphine oxides†

Carlotta Seno , Rohan Pokratath , Ajmal Roshan Unniram Parambil , Dietger Van den Eynden , Evert Dhaene , Alessandro Prescimone and Jonathan De Roo *
Department of Chemistry, University of Basel, Mattenstrasse 22, 4058 Basel, Switzerland. E-mail: Jonathan.DeRoo@unibas.ch

Received 2nd May 2024 , Accepted 3rd May 2024

First published on 28th May 2024

Abstract

Group 4 Lewis acids are well-known catalysts and precursors for (non-aqueous) sol–gel chemistry. Titanium, zirconium and hafnium halides, and alkoxy halides are precursors for the controlled synthesis of nanocrystals, often in the presence of Lewis base. Here, we investigate the interaction of Lewis bases with the tetrahalides (MX4, X = Cl, Br) and metal alkoxy halides (MXx(OR)4−x, x = 1–3, R = OiPr, OtBu). The tetrahalides yield the expected Lewis acid–base adducts MX4L2 (L = tetrahydrofuran or phosphine oxide). The mixed alkoxy halides react with Lewis bases in a more complex way. 31P NMR spectroscopy reveals that excess of phosphine oxide yields predominantly the complexation product, while a (sub)stoichiometric amount of phosphine oxide causes disproportionation of the MXx(OR)4−x species into MXx+1(OR)3−x and MXx−1(OR)5−x. The combination of complexation and disproportionation yields an atypical Job plot. In the case of zirconium isopropoxy chlorides, we fitted the concentration of all observed species and extracted thermodynamic descriptors from the Job plot. The complexation equilibrium constant decreases in the series: ZrCl3(OiPr) > ZrCl2(OiPr)2 ≫ ZrCl(OiPr)3, while the disproportionation equilibrium constant follows the opposite trend. Using calculations at the DFT level of theory, we show that disproportionation is driven by the more energetically favorable Lewis acid–base complex formed with the more acidic species. We also gain more insight into the isomerism of the complexes. The disproportionation reaction turns out to be a general phenomenon, for titanium, zirconium and hafnium, for chlorides and bromides, and for isopropoxides and tert-butoxides.

1. Introduction

Lewis acids play an essential role in many catalytic and biological processes and understanding their interaction with Lewis bases has driven chemistry forward. Group 4 metals (Ti, Zr, Hf) produce an interesting class of Lewis acids, almost exclusively featuring the +IV oxidation state.1 The metal halides are economical precursors for the synthesis of group 4 materials, ranging from amorphous gels,2,3 to crystalline nanoparticles.4–8 Given that the halides are often dissolved in alcohol or combined with metal alkoxides, the actual reactive species in solution are often mixed alkoxy halides.9–11 In the synthesis of zirconia nanocrystals from zirconium chloride and zirconium isopropoxide, zirconium di-isopropoxy di-chloride is a confirmed intermediate, which interacts with the Lewis base present: tri-n-octylphosphine oxide (TOPO). The formed complex decomposes at 340 °C to zirconia nanocrystals.11–13
 
image file: d4dt01299b-t1.tif(1)

The same chemistry is relevant for titania and hafnia nanocrystals, where the choice of metal halide, metal alkoxide, and of the Lewis base plays a crucial role in the determination of the final properties of the product.4,14–18 Although the nature and stability of the formed metal complexes are of direct consequence to the outcome of nanocrystal syntheses, detailed insights into the coordination of alkoxy halides are lacking.

The metal halides were more extensively studied. All twelve possible MX4 compounds are known, with M = Ti, Zr, Hf and X = F, Cl, Br, I.1 All of them are strong Lewis acids. Some are monomeric molecules (e.g., TiCl4, TiBr4 and TiI4), while others form polymeric solids (TiF4, ZrCl4 and HfCl4).19–21 The polymeric structure consists of bridging halides, which increases the coordination number. It is preferred for the larger metals (Zr/Hf) and/or smaller halides (F).7 The metal halides react with Lewis bases (L), forming the MX4L2 adducts, with a coordination number of 6.22 In case of titanium fluoride, the solution behavior is often complex with the ionic species TiF3L3+ and Ti4F182− present, next to TiF4L2.22 Under-supply of Lewis base (e.g., only one equivalent) further promotes ionization:

 
3TiF4L → TiF3L3+ + Ti2F9−(2)

The isolated species in the solid state is usually cis-TiF4L2. A mixture of trans-TiF4L2 and cis-TiF4L2 complexes were found in solution in case the Lewis base was sufficiently steric. While triphenylphosphine oxide produces cis-TiF4L2 in the solid state, 2,6-dimethylpyridine-N-oxide produces the trans configuration. The cis/trans equilibrium was rationalized as follows.23

• Steric repulsion between halides favors the trans configuration

• Steric repulsion between the ligands favors the trans configuration

• To maximize halide-metal pπ–dπ bonding, the cis configuration is favored.

The last argument is justified by the shorter bond length of the halide trans to the Lewis base, compared to the two fluorides trans with respect to each other. The halide competes more effectively for the dπ with a neutral Lewis base than with another halide. While the above arguments were originally made for titanium fluoride, they explain a more general pattern in the literature.

The halide effect is apparent from the THF complexes of TiF4 and TiCl4. TiF4(THF)2 has the cis configuration,24 but TiCl4(THF)2 is found both as cis and trans.25,26 For a larger metal, the steric repulsion between the chloride ligands is mitigated, evidenced by the sole isolation of cis-ZrCl4(THF)2 and cis-HfCl4(THF)2.27,28 Using tetramethylethylenediamine as bidentate Lewis base, cis-ZrCl4L2 was obtained and again, the Zr–Cl bond distances are longer for the two trans chloride ligands (2.43 Å) and shorter for the chloride ligands trans to the Lewis base (2.41 Å).29 Acetonitrile (ACN) is a less steric ligand than THF and only cis configurations were determined for TiCl4(ACN)2,30,31 TiBr4(ACN)2,32 ZrCl4(ACN)2,33,34 and ZrBr4(ACN)2.35,36 On the other hand, tri-n-octylphosphine oxide is more sterically bulky than THF and favors the trans configuration for ZrCl4(R3PO)2.11

Ligand exchange of Lewis bases bound to TiF4 was shown to occur via a dissociative mechanism featuring an intermediate with coordination number of 5.22,37,38 The kinetic stability of the complexes follows the order of Lewis base strength39

 
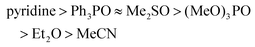image file: d4dt01299b-t2.tif(3)

Mixed alkoxy halide species are formed when a metal halide is mixed with a metal alkoxide.2,40,41 The stoichiometry of the final product can be varied:

 
3MCl4 + M(OR)4 → 4MCl3(OR)(4)
 
MCl4 + M(OR)4 → 2MCl2(OR)2(5)
 
MCl4 + 3M(OR)4 → 4MCl(OR)3(6)

The mixed alkoxy chloride also forms by reacting the metal alkoxide with acetyl chloride.42 The ester is formed as a by-product.

 
M(OR)4 + xRCOCl → MClx(OR)4−x + xRCOOR(7)

The distinction between the different MClx(OR)4−x complexes via1H NMR is highly challenging due to the complex structure of the resonances.2,40 After complexation with phosphine oxides, the different species can be distinguished via31P NMR.11 However, the Lewis acid behavior of these mixed halide alkoxides is largely unexplored.

Here, we gain insight into the interaction between group 4 MCl4−x(OR)x Lewis acids (M = Ti, Zr or Hf) and phosphine oxide Lewis bases, relevant for nanocrystal syntheses. Focusing first on pure metal halides, we synthesize and analyze the structure of previously unreported ZrBr4(THF)2 and various MX4(TPPO) (TPPO = triphenylphosphine oxide, X = Cl or Br) complexes with single-crystal XRD. All obtained complexes feature the trans configuration of the Lewis bases. Second, we study the interaction of mixed isopropoxy chloride with tri-n-octylphosphine oxide using 31P NMR spectroscopy. We observe both complexation and disproportionation reactions. The latter are especially prevalent at sub-stoichiometric amounts of Lewis base and this behavior highly complicates the Job plot. Focusing on the case of zirconium, we model the Job plot using a set of chemical equations (in COPASI43), thus extracting the equilibrium constants for both complexation and disproportionation. With decreasing Lewis acidity (MCl3(OR) > MCl2(OR)2 > MCl(OR)3), we find a lower complexation constant but a higher propensity for disproportionation. We showed the generality of the disproportionation behavior for all three metals, for different halides and different alkoxides.

2. Results and discussion

2.1. Lewis base adducts of MCl4

The Lewis base adducts of titanium tetrahalides are well-reported in the literature but zirconium and hafnium tetrahalides are less well-documented. For completion, we synthesized and crystallized trans-ZrBr4(THF)2, see Fig. 1. The bond distances are reported in Table S1,† together with selected structures from the literature. While ZrCl4(THF)2 is cis, ZrBr4(THF)2 has the trans configuration. The M–O bond distance is slightly shorter in ZrBr4(THF)2, which is likely due to the absence of steric repulsion between the two THF ligands since the same effect is observed for cis-TiCl4(THF)2 and trans-TiCl4(THF)2, see Table S1.†
image file: d4dt01299b-f1.tif
Fig. 1 Structures of zirconium halide complexes determined by single-crystal XRD. The hydrogen atoms are omitted for clarity. Table S2† reports the resolved crystallographic parameters.

We previously showed that phosphine oxides quantitatively displace THF from zirconium chloride.11 Therefore, we synthesized the triphenylphosphine oxide (TPPO) complexes of ZrCl4, ZrBr4 and HfCl4 by ligand exchange from the THF complex and single-crystals were grown.

 
MX4(THF)2 + 2TPPO → MX4(TPPO)2 + 2THF(8)

Structural analysis via single-crystal XRD indicates that the TPPO complexes have the trans configuration, see Fig. 1 for the structure of trans-ZrCl4(TPPO)2 (the other structures are shown in Fig. S1†). Including literature data, we conclude that all TPPO adducts of zirconium and hafnium tetrahalides adopt the trans configuration (Table 1). Compared to the THF complexes, the TPPO complexes generally feature a longer M–X distance and a shorter M–O distance (Table S1†). This is a reflection of the higher Lewis basicity of phosphine oxides. In general, Table 1 follows the general trend laid out in the introduction. Larger halides and ligands promote the trans configuration. In addition, a larger metal size promotes the cis configuration as it allows to alleviate steric repulsion between the halides. A notable exception to this trend is the contrast between cis-TiF4(TPPO)2 and trans-ZrF4(TPPO)2. Even though the zirconium atom is larger than titanium, the configuration switches from cis to trans. We hypothesize that the energy difference between the fluorine p-orbitals and the zirconium d-orbitals is too high to allow for sufficient interaction, thereby removing the driving force for the cis configuration. All synthesized complexes were characterized by 1H and 31P NMR spectroscopy (Fig. S2†), thermogravimetric analysis (Table S3†) and infrared spectroscopy (Fig. S3†). Powder XRD confirmed that the bulk material has the same structure as the single crystals (Fig. S4†). The triphenylphosphine oxide complexes are all poorly soluble in chloroform and effectively insoluble in benzene, but ligand exchange for tri-n-octylphosphine oxide (TOPO) generates soluble complexes, with 31P NMR shifts consistent with previous assignments (Fig. S5–S7†).11 TiCl4(TOPO)2 appears as a sharp singlet at 77.4 ppm, ZrCl4(TOPO)2 at 72.9 ppm and HfCl4(TOPO)2 at 73.0 ppm. The Gutmann-Beckett method ranks the effective Lewis acidity by the 31P NMR shift of triethylphosphine oxide (TEPO) bound to a Lewis acid.44,45 A high chemical shift is related to a high effective Lewis acidity. Given the similar structure of TEPO and TOPO, we conclude that TiCl4 has a higher effective Lewis acidity than ZrCl4 and HfCl4. The latter two have about the same effective Lewis acidity.

Table 1 Overview of cis/trans configuration in different group 4 metal halide complexes with either tetrahydrofuran (THF) or triphenylphosphine oxide (TPPO) as Lewis base
  THF TPPO
a This work.
TiF4 cis 24 cis 24
TiCl4 cis 25 and trans26 trans [thin space (1/6-em)]46
 
ZrF4 — trans 47
ZrCl4 cis 27 trans
ZrBr4 trans trans
 
HfCl4 cis 28 trans

2.2. Complexation and disproportionation of ZrClx(OiPr)4−x

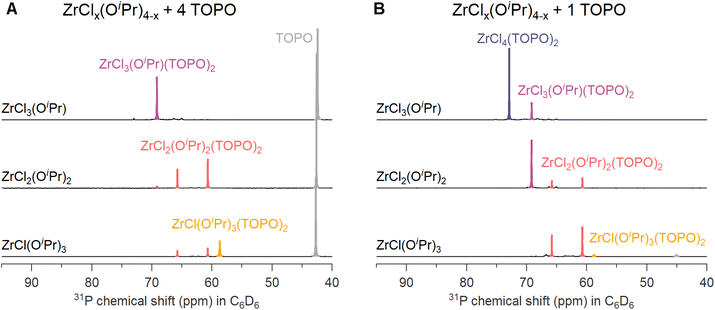
We synthesized three ZrClx(OiPr)4−x (x = 1–3) compounds by reacting Zr(OiPr)4·iPrOH with acetylchloride in the correct ratios, see eqn (7). We then added an excess (4 equivalents) of TOPO to each compound to assess the complexation behavior. In case of MCl3(OiPr), the 31P NMR spectrum (Fig. 2A) shows one main signal for MCl3(OiPr)(TOPO)2. In the case of MCl2(OiPr)2, we observe two resonances pertaining to 2 isomers, see further details in the computational section. In case of MCl(OiPr)3, we observe a less intense signal for the MCl(OiPr)3(TOPO)2 complex and also the resonances of the two MCl2(OiPr)2(TOPO)2 isomers are present. Based on the assignment and the positions in 31P NMR spectrum, the effective Lewis acidity is ranked: ZrCl4 > ZrCl3(OiPr) > ZrCl2(OiPr)2 > ZrCl(OiPr)3.
image file: d4dt01299b-f2.tif
Fig. 2 31P NMR spectra at room temperature of zirconium alkoxy chloride complexes MClx(OiPr)4−x in benzene-d6 with either (A) 4 equivalents or (B) 1 equivalent of TOPO. The concentration of zirconium is 0.05 M when mixed with 4 equivalents, and 0.125 M with 1 equivalent.

When only 1 equivalent of TOPO is added, the speciation changes, see Fig. 2B. Starting from MCl3(OiPr), we detect MCl4(TOPO)2 as the main species in the 31P NMR spectrum. For MCl2(OiPr)2, we retrieve mostly MCl3(OiPr)(TOPO)2 and for MCl(OiPr)3, we find almost exclusively the two isomers of MCl2(OiPr)2(TOPO)2. Note that species, which are not coordinated by TOPO, are not detected by 31P NMR. To explain the data, we hypothesize a disproportionation reaction and formation of the stronger Lewis acid–base adduct. Take the example of ZrCl3(OiPr). The reaction with 1 equivalent of TOPO can be split in a disproportionation (eqn (9)) and a complexation reaction (eqn (10)):

 
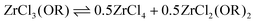image file: d4dt01299b-t3.tif(9)
 
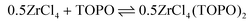image file: d4dt01299b-t4.tif(10)

Giving the total:

 
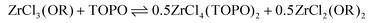image file: d4dt01299b-t5.tif(11)

After disproportionation, the Lewis base forms an adduct with the most Lewis acidic species, ZrCl4. Hence, ZrCl4(TOPO)2 is detected in NMR, while ZrCl2(OR)2 is undetectable by 31P NMR. In the absence of a disproportionation, complexation could only result in either 1 equivalent of ZrCl3(OR)(TOPO) or in 0.5 equiv. ZrCl3(OR)(TOPO)2 and 0.5 equiv. ZrCl3(OR). We thus assign the driving force of the disproportionation to the formation of stronger Lewis acid–base adduct; ZrCl4(TOPO)2. When supplying an excess of Lewis base, the disproportionation pathway is suppressed, the extent of which depends on the alkoxy chloride. For the case of MCl(OiPr)3, the MCl2(OiPr)2(TOPO)2 isomers (i.e., the disproportionation product) were still significantly present at 4 equivalents of TOPO, see Fig. 2A.

There is no literature precedent for ligand-induced disproportionation in group 4. However, TiCl3(OMe) disproportionates upon heating and cannot be purified by distillation:48

 
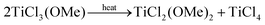image file: d4dt01299b-t6.tif(12)

Precedent for ligand-induced disproportionation is found in group 13. Wiberg and coworkers reported in 1935 that BClx(OR)3−x species disproportionate into BClx+1(OR)3−x−1 and BClx−1(OR)3−x+1 (with x = 1, 2) when trimethylamine or an ether is introduced.49,50 A quantitative analysis of the phenomenon was not reported.

2.3. Quantitative fit of the Job plot

Job plots are a popular technique to determine the stoichiometry of binding events.51–54 In such a plot, the relative mole fraction of reagents is varied while keeping the sum of the concentrations constant. In case of zirconium isopropoxy chlorides interacting with TOPO, the Job plot becomes highly complicated since the reaction is not limited to complexation but also features disproportionation, see Fig. 3.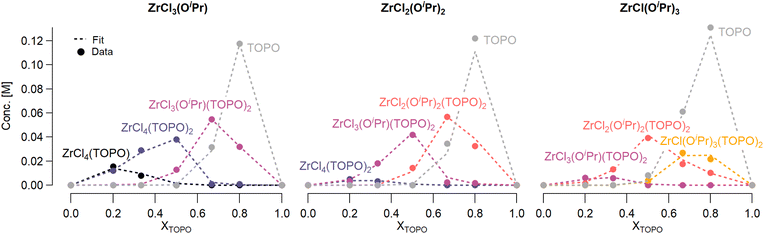
image file: d4dt01299b-f3.tif
Fig. 3 Job plots of ZrCl3(OiPr), ZrCl2(OiPr)2 and ZrCl(OiPr)3. The dots represent the data and the dashed lines the quantitative fit. Various species formed during the reaction are indicated and plotted against the mole fraction of TOPO (XTOPO). The concentration of each species was calculated by integrating the corresponding peak from the 31P NMR, see ESI.†

To quantify the disproportionation observed in the previous section, we modeled the Job plots with a series of equilibrium reactions. For the case of ZrCl3(OiPr), we take a set of three equilibria, composed of a complexation (eqn (13)), a disproportionation (eqn (14)) and a decomplexation equilibrium (eqn (15)), where L = TOPO.

 
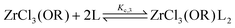image file: d4dt01299b-t7.tif(13)
 
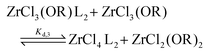image file: d4dt01299b-t8.tif(14)
 
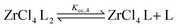image file: d4dt01299b-t9.tif(15)

For the Job plots of ZrCl2(OiPr)2 and of ZrCl(OiPr)3 we used a different set of reactions. Eqn (16) and (19) represent the complexation of the respective alkoxy chloride complex with TOPO. This is complemented by two disproportionations eqn (17) and (18) for ZrCl2(OiPr)2, and eqn (20) and (21) for ZrCl(OiPr)3. The second disproportionation equation has been added in each case since the NMR spectrum of ZrCl2(OiPr)2 features the presence of ZrCl3(OiPr)(TOPO)2 and ZrCl4(TOPO)2 when TOPO is added. Similar observations are made for ZrCl(OiPr)3.

 
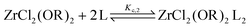image file: d4dt01299b-t10.tif(16)
 
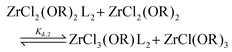image file: d4dt01299b-t11.tif(17)
 
image file: d4dt01299b-t12.tif(18)
 
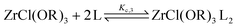image file: d4dt01299b-t13.tif(19)
 
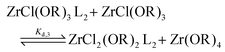image file: d4dt01299b-t14.tif(20)
 
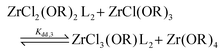image file: d4dt01299b-t15.tif(21)

For all three cases, we obtain excellent fits to the data (Fig. 3 and details in the ESI†). The equilibrium constants are reported in Table 2. The complexation constant (Kc,x) decreases with decreasing chloride content but the trend is not linear. Kc,x is slightly higher for ZrCl3(OiPr) than for ZrCl2(OiPr)2, but drops two orders of magnitude for ZrCl(OiPr)3, which might be due to steric effects of the isopropoxides in addition to a decreasing Lewis acidity. The opposite trend is found for the disproportionation constant (Kd,x), which is statistically the same for ZrCl3(OiPr) and ZrCl2(OiPr)2 but increases for ZrCl(OiPr)3. This is likely due to the larger difference in complexation strength between ZrCl2(OiPr)2 and ZrCl(OiPr)3 compared to the difference between ZrCl3(OiPr) and ZrCl2(OiPr)2.

Table 2 Equilibrium constants for the sets of equilibrium reactions that describe the complexation and disproportionation mechanisms of the three zirconium alkoxy chloride complexes
  ZrCl3(OiPr) ZrCl2(OiPr)2 ZrCl(OiPr)3
K c,x 9.1 × 106 ± 6.9 × 106 1.6 × 106 ± 1.3 × 106 4.6 × 104 ± 2.6 × 104
K cc,4 1.3 × 10−5 ± 0.6 × 10−5
K d,x 13.9 ± 3.2 14.5 ± 5.8 116.9 ± 63.5
K dd,x 0.07 ± 0.01 0.16 ± 0.02

2.4. Computational insights

We sought to compare our experimental data with computations and further strengthen our hypothesis for the disproportionation driving force. Following our earlier established method based on DFT (see Experimental section), we optimized the structures of ZrClx(OiPr)4−x (x = 1–4) and their complexes with THF or with triethylphosphine oxide (TEPO). TEPO is computationally less demanding than TOPO and forms identical complexes, as shown in Fig. S11.† All optimized structures are available as structure files in the ESI.† We considered all isomers for both THF and TEPO complexes, optimized their geometry, and calculated their energy, see Fig. 4 for TEPO (and Fig. S12† for THF). For all THF complexes, we find that the lowest energy isomer has the THF ligands in the cis configuration, consistent with the single-crystal data of ZrCl4(THF)2. The TEPO complexes show surprising trends. The ZrCl3(OiPr)(TEPO)2 complex is most stable in the TEPO-trans configuration (Fig. 4), again consistent with the single-crystal data of ZrCl4(TPPO)2. However, the ZrCl2(OiPr)2(TEPO)2 complex prefers the Cl-trans isomer, with the TEPO ligands cis to each other. This configuration is most effective in reducing the steric interactions between isopropoxides and TEPO ligands. It is interesting to examine the structure of the Cl-trans isomer. The octahedral coordination is distorted, with the Cl–Zr–Cl bond angle measuring 166 degrees and the chloride atoms pushed towards the TEPO side. The alpha carbon of the isopropoxide ligands (i.e., the branching point or the origin of sterical hindrance) is located at 3.19 and 3.34 Å from the central Zr atom. This makes isopropoxide a more steric ligand than TEPO since the Zr–P distance is longer: 3.50 and 3.68 Å (for TEPO, phosphorus is the branching point). The ZrCl(OiPr)3(TEPO)2 complex prefers the OR-fac isomer (Fig. 4), again with the TEPO ligands in the cis configuration, and the octahedral coordination distorted with chloride being pushing towards TEPO. The TEPO-trans isomer is only 7 kJ mol−1 less stable though.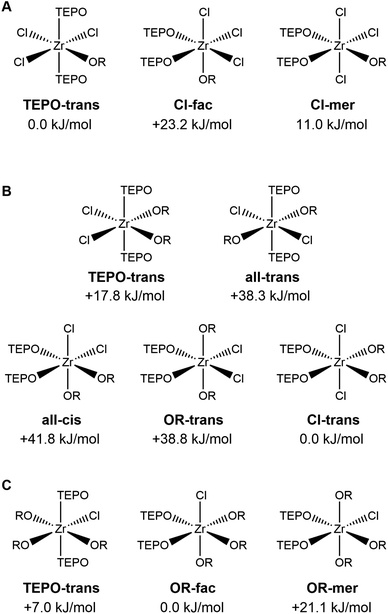
image file: d4dt01299b-f4.tif
Fig. 4 The different possible isomers for the ZrClx(OiPr)4−x(TEPO)2 complexes (x = 1–3). The relative energy compared to the most stable isomer is indicated.

Using the calculated energies, we computed the enthalpy changes for the (gas-phase) complexation reactions (Table 3), which represent the global Lewis acidity since the latter is defined as the thermodynamic tendency to form Lewis acid–base pairs.55 All reactions are exothermic and the complexation with TEPO is favored over complexation with THF, in agreement with experimental results. The complexation reaction becomes less exothermic in the series: ZrCl4 > ZrCl3(OiPr) > ZrCl2(OiPr)2 > ZrCl(OiPr)3. This trend in global Lewis acidity agrees with the trend in effective Lewis acidity, given by the 31P NMR shifts. The calculated values of ΔH cannot be directly compared to the experimental equilibrium constants since the latter depend on ΔG. For example, for ZrCl3(OiPr), the experimental equilibrium constant translates in ΔG = −39.7 kJ mol−1. The large theoretical value of ΔH is thus likely offset by an unfavorable change in entropy, as one would expect for an association equilibrium. Furthermore, the uncoordinated Lewis acid is a tetrahedral monomer in the calculations, and thus dimerization (or polymerization in case of ZrCl4) may account for discrepancies with experimental results.55 As a final disclaimer, the calculations were performed in the gas phase and no solvent effects were taken into account.

Table 3 Calculated changes in enthalpy for the complexation reaction of the different zirconium isopropoxy chlorides with either tetrahydrofuran (THF) and triethylphosphine oxide (TEPO). The lowest energy isomer was used for the calculation
  ΔH (kJ mol−1)
L = THF L = TEPO
ZrCl4 + 2L ⇌ ZrCl4L2 −117.1 −235.0
ZrCl3(OiPr) + 2L ⇌ ZrCl3(OiPr)L2 −105.3 −188.0
ZrCl2(OiPr)2 + 2L ⇌ ZrCl2(OiPr)2L2 −90.3 −177.4
ZrCl(OiPr)3 + 2L ⇌ ZrCl(OiPr)3L2 −65.3 −113.6

When comparing the experimental data of ZrCl2(OiPr)2(TEPO)2 and the theoretical calculations, we are faced with a discrepancy. Two peaks are observed in the 31P NMR spectrum and we previously assigned them to the TEPO-trans and the all-trans isomers, without considering the other isomers.11 From our current calculations, Cl-trans isomer is clearly preferred. This is not an effect of the chain length since the two peaks appear in the same ratio for both TEPO and TOPO. The two signals always behave as one and we did not observe one without the other. We discarded the hypothesis that the two resonances represent the two phosphine oxide ligands in the same complex since the integrals are not 1[thin space (1/6-em)]:[thin space (1/6-em)]1 but 0.35[thin space (1/6-em)]:[thin space (1/6-em)]0.65. Our current best hypothesis is that the TEPO-trans structure is more favorable than we currently predict by DFT. The existence of multiple isomers in solution could also explain the occurrence of many more resonances in deuteroform compared to benzene (for a given composition and stoichiometry).11 It also points to the importance of the solvent in stabilizing certain isomers.

While acknowledging the above limitations, we computed ΔH for the spontaneous disproportionation reaction and for the Lewis base-induced disproportionation, see Table 4. The disproportionation in the absence of Lewis bases is quite endothermic (entries 1 and 2). However, when we compute ΔH for the TEPO-assisted disproportionation, the reactions become more favorable (entries 3 and 4). These calculations correspond to eqn (17) and (20) and the experimental equilibrium constants were reported in Table 2. When calculating ΔG from Kd,3, we obtain −6.5 kJ mol−1, significantly smaller than the computed enthalpy change (−29 kJ mol−1). We attribute this to the presence of weak Lewis bases in the reaction mixture. Indeed, it is known that esters produced during the synthesis of the mixed alkoxy chlorides can coordinate to the metal center (see eqn (7)). The effect of the presence of a weaker Lewis base (e.g., THF), can be also theoretically computed, see entries 5 and 6 in Table 4. It is clear that the driving force for disproportionation decreases when THF is available to coordinate the Lewis acid sites which are not coordinated by phosphine oxide. This is reproduced experimentally for ZrCl3(OiPr), see Fig. S13.†

Table 4 Calculated changes in enthalpy for the disproportionation reaction of the different zirconium isopropoxy chlorides with L = TEPO. The lowest energy isomer was used for the calculation
Entry Equilibrium ΔH (kJ mol−1)
1 2ZrCl3(OiPr) ⇌ ZrCl4 + ZrCl2(OiPr)2 18.0
2 2ZrCl2(OiPr)2 ⇌ ZrCl3(OiPr) + ZrCl(OiPr)3 16.9
 
3 image file: d4dt01299b-t16.tif −29.0
4 image file: d4dt01299b-t17.tif 6.3
 
5 image file: d4dt01299b-t18.tif −13.9
6 image file: d4dt01299b-t19.tif 31.2

2.5. Generalization

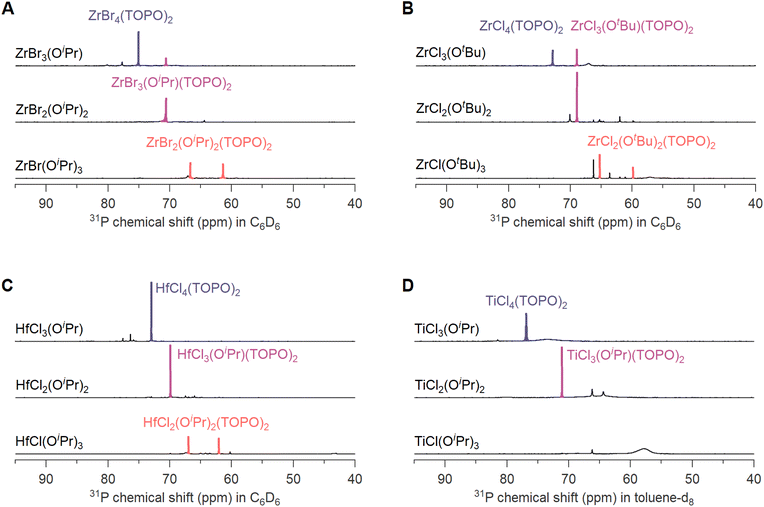
To test the generality of the disproportionation behavior, we also investigated other alkoxy halides, varying the metal, the alkoxide and the halide. Fig. S14–S17† show the NMR spectra with 2 or 4 equivalents TOPO, to identify the complexation products. Fig. 5 shows the spectra with one equivalent of TOPO and in all four cases, the disproportionation products are detected. We observe some interesting differences. In case of zirconium isopropoxy tribromide, we notice a stronger tendency for disproportionation compared to the above zirconium isopropoxy trichloride in the presence of THF (Fig. S13†). We synthesized the alkoxy bromide by mixing ZrBr4(THF)2 and Zr(OiPr)4(iPrOH) due to the low solubility of ZrBr4. In case of zirconium tert-butoxy trichloride (synthesized from ZrCl4(THF)2 and Zr(OtBu)4) we observe a stronger disproportionation compared to zirconium isopropoxy trichloride, but weaker than zirconium isopropoxy tribromide. The hafnium isopropoxy chlorides are synthesized from HfCl4 and Hf(OiPr)4(iPrOH) so there is no THF or ester present, only 0.25 equivalents of isopropanol. A complete disproportionation is the result. In case of titanium, we observe the expected disproportionation products for TiCl3(OiPr) and TiCl2(OiPr)2, but not for TiCl(OiPr)3. As demonstrated in Fig. S17,† TiCl4(TOPO)2 and TiCl3(OiPr)(TOPO)2 are detectable species with narrow resonances in the 31P NMR spectrum, but TiCl2(OiPr)2(TOPO)2 is not detectable, featuring very broad lines, indicative of chemical exchange between bound and free TOPO.
image file: d4dt01299b-f5.tif
Fig. 5 31P NMR spectra of (A) zirconium isopropoxy bromide (B) zirconium tert-butoxy chloride (C) hafnium isopropoxy chloride (D) titanium isopropoxy chloride complexes mixed with 1 equivalent of TOPO. In case of A and B, the alkoxy halides were synthesized from the respective zirconium halide THF complex.

3. Conclusions

We studied the interaction of group 4 metal halides and metal alkoxy halides with Lewis bases. We found that phosphine oxides are excellent hard bases for these types of Lewis acids and form Lewis acid–base adducts with a 1[thin space (1/6-em)]:[thin space (1/6-em)]2 stoichiometry. Sub-stoichiometric amounts of Lewis bases lead to disproportionation of the metal alkoxy halides. We were able to extract the experimental equilibrium constants for both complexation and disproportionation equilibria from the Job plot. The complexation equilibrium constant decreases with the Lewis acidity of the complex, i.e. with the chloride content. Calculations at the DFT level of theory confirmed the trend and allowed us to rationalize the driving force for disproportionation: the formation of more stable Lewis acid–base adducts. These results thus provide fundamental insight into metal alkoxy halide Lewis acids and are also relevant for group 13 Lewis acids. The results are of direct relevance to nanocrystal reactions with TOPO as a coordinating solvent.

4. Experimental

4.1. Materials

ZrCl4 (99.9%), HfCl4 (99.9%), Ti(OiPr)4 (98%) and Zr(OtBu)4 (99.99%) were purchased from Strem Chemicals. TiCl4 (99.99%) was bought from ACROS Organics. Acetyl chloride (≥99.0%) was provided by Sigma Aldrich. ZrCl4(THF)2, TiCl4(THF)2 and HfCl4(THF)2 were synthesized following the procedure reported by Manzer et al.56 ZrBr4(THF)2 was synthesized in an identical fashion to ZrCl4(THF)2 (yield = 20%). Zr(OiPr)4·iPrOH and Hf(OiPr)4·iPrOH were synthesized following the procedure reported firstly by Bradley et al.57 and slightly modified by Dhaene et al.58 Tri-n-octylphosphine oxide (Strem Chemicals, 99%) was recrystallized according to the procedure described by Owen et al.59 while triphenylphosphine oxide (Sigma Aldrich, 98%) was used without further purification. In benzene-d6 (Apollo scientific, 99.5 atom%), toluene-d8 (VWR, >99.5 atom%), chloroform-d (Eurisotop, 99.5 atom%) and anhydrous pentane (Sigma Aldrich, ≥99%) 10 vol% of activated 4 Å molecular sieves were added and left to stand for 3 days in the glovebox to remove residual water. Toluene and DCM were dried over a solvent system before being transferred into a glovebox. All operations were performed air-free in a nitrogen-filled glovebox.

4.2. Methods

Preparation of the NMR samples for the spectra in Fig. 2 and 3. ZrCl3(OiPr), ZrCl2(OiPr)2 and ZrCl(OiPr)3 were synthesized according to the procedure reported by Bradley et al.,42 by mixing Zr(OiPr)4 and acetyl chloride in different molar ratios. After stirring for 48 hours at room temperature, the solvent is removed and three 0.5 M solutions were prepared in C6D6 (1 mmol of ZrClx(OiPr)4−x in 2 mL C6D6), together with various volumes of a 0.5 M solution of TOPO (773.3 mg, 2 mmol) in C6D6 (3.12 mL). The different NMR tubes were prepared according to Table 5.
Table 5 Details of the preparation of the NMR tubes. For each ZrClx(OiPr)4−x the 5 NMR have been prepared
X TOPO n ZrClx(OiPr)4−x (mmol) n TOPO (mmol) V ZrClx(OiPr)4−x (μL) V TOPO (μL) V C6D6 (μL)
0.2 0.12 0.03 240 60 300
0.33 0.1 0.05 200 100 300
0.5 0.075 0.075 150 150 300
0.66 0.05 0.1 100 200 300
0.8 0.03 0.12 60 240 300

The 1H NMR and 31P NMR spectra are shown in Fig. S8–S10.† All the peaks in the 31P NMR spectra were integrated and their sum was normalized for the total amount of TOPO in the solution. For the complexes with two TOPO ligands, the concentration of the complex is half of the bound TOPO concentration. The detailed procedure to obtain the Job Plot is provided in the ESI.†

Preparation of the NMR samples for the spectra in Fig. 5. ZrBrx(OiPr)4−x complexes were synthesized by mixing ZrBr4(THF)2 with Zr(OiPr)4 in the right molar ratios and dissolved in C6D6 to obtain three 0.1 M stock solutions. The NMR tubes are prepared by mixing the 0.1 M C6D6 solution of ZrBrx(OiPr)4−x (200 μL) with a 0.5 M C6D6 solution of TOPO (40 μL for 1 equivalent, 80 μL for 2 equivalents) and C6D6 is added to make up the tubes to a total volume of 400 μL.

ZrClx(OtBu)4−x complexes were synthesized by mixing ZrCl4(THF)2 with Zr(OtBu)4 in the right molar ratios and dissolved in C6D6 to obtain three 0.5 M stock solutions (0.5 mmol ZrClx(OtBu)4−x in 1 mL C6D6). To improve the solubility of the complexes, 1 equivalent of TOPO is added in each of the three stock solutions (1 mL of 0.5 M C6D6 solution of TOPO). The NMR tubes for 1 equivalent are prepared by diluting the solution of ZrClx(OtBu)4−x with 1 equiv. TOPO (100 μL) with C6D6 (400 μL). To prepare the NMR tubes with 4 equiv., the solution of ZrClx(OtBu)4−x with 1 equiv. TOPO (100 μL) is mixed with 0.5 M C6D6 of TOPO (150 μL) and C6D6 (250 μL).

HfClx(OiPr)4−x complexes were synthesized by mixing HfCl4 with Hf(OiPr)4 in the right molar ratios and dissolved in C6D6 to obtain three 0.5 M stock solutions (0.5 mmol HfClx(OiPr)4−x in 1 mL C6D6). To improve the solubility of the complexes, 1 equivalent of TOPO is added in each of the three stock solutions (1 mL of 0.5 M C6D6 solution of TOPO). The NMR tubes for 1 equivalent are prepared by diluting the solution of HfClx(OiPr)4−x with 1 equiv. TOPO (100 μL) with C6D6 (400 μL). To prepare the NMR tubes with 4 equiv., the solution of HfClx(OiPr)4−x with 1 equiv. TOPO (100 μL) is mixed with 0.5 M C6D6 of TOPO (150 μL) and C6D6 (250 μL).

TiClx(OiPr)4−x complexes were synthesized by mixing 0.5 M C6D6 solution of TiCl4 with a 0.5 M C6D6 solution of Ti(OiPr)4 in the right volumes. The NMR tubes are prepared by mixing the 0.5 M C6D6 solution of TiClx(OiPr)4−x (150 μL for 1 equiv. TOPO, 60 μL for 4 equiv. TOPO) with a 0.5 M C6D6 solution of TOPO (150 μL for 1 equivalent, 240 μL for 4 equivalents) and C6D6 is added to make up the tubes to a total volume of 500 μL.

Crystal growth of ZrCl4(TPPO)2. 1.5 mL of a 0.1 M toluene solution of TPPO (69.6 mg, 0.25 mmol TPPO in 2.5 mL of toluene) was layered over a 0.1 M DCM solution of ZrCl4(THF)2 (37.7 mg, 0.1 mmol ZrCl4(THF)2 in 1 mL of DCM) in a 4 mL vial. White crystals grew after 4 days and a single crystal was chosen for X-ray diffraction, while the rest was dried under vacuum and analyzed by PXRD and 31P NMR. Yield of 44%. A detailed characterization consisting of 1H NMR and 31P NMR (Fig. S2), PXRD (Fig. S4), IR (Fig. S3) and TGA (Table S3) is reported in the ESI.†
Crystal growth of HfCl4(TPPO)2. 1.5 mL of a 0.1 M toluene solution of TPPO (69.6 mg, 0.25 mmol TPPO in 2.5 mL of toluene) was layered over a 0.1 M DCM solution of HfCl4(THF)2 (46.5 mg, 0.1 mmol HfCl4(THF)2 in 1 mL of DCM) in a 4 mL vial. White crystals grew after 4 days and a single crystal was chosen for X-ray diffraction, while the rest was dried under vacuum and analyzed by PXRD and 31P NMR. Yield of 38%. A detailed characterization consisting of 1H NMR and 31P NMR (Fig. S2), PXRD (Fig. S4), IR (Fig. S3) and TGA (Table S3) is reported in the ESI.†
Crystal growth of TiCl4(TPPO)2. Anhydrous toluene (0.5 mL) was layered over a 0.1 M DCM solution of TiCl4(THF)2 (33.3 mg, 0.1 mmol TiCl4(THF)2 in 1 mL DCM) in a 4 mL vial. On top of it, 1 mL of a 0.05 M toluene solution of TPPO (69.6 mg, 0.25 mmol TPPO in 5 mL of toluene) was layered. Yellow crystals grew after 20 hours and a single crystal was chosen for X-ray diffraction, while the rest was dried under vacuum and analyzed by PXRD and 31P NMR. Yield of 74%. A detailed characterization consisting of 1H NMR and 31P NMR (Fig. S2), PXRD (Fig. S4), IR (Fig. S3) and TGA (Table S3) is reported in the ESI.†
Crystal growth of ZrBr4(TPPO)2. ZrBr4 (4.1 mg, 0.01 mmol) is dissolved in anhydrous DCM (2 mL) and TPPO (7.0 mg, 0.025 mmol) is added to the solution. Anhydrous pentane (2 mL) was layered over it. A single crystal was selected for X-ray diffraction. Quantitative yield. A detailed characterization consisting of 1H NMR and 31P NMR (Fig. S2), PXRD (Fig. S4), IR (Fig. S3) and TGA (Table S3) is reported in the ESI.†
Crystal growth of ZrBr4(THF)2 by recrystallization. ZrBr4(THF)2 is dissolved in anhydrous DCM, and filtrated to remove ZrBr4 impurities. ZrBr4(THF)2 is then precipitated from the solution with anhydrous pentane, vacuum dried and again dissolved in anhydrous DCM. White crystals grew after 2 days through diffusing pentane vapor. A single crystal was selected for X-ray diffraction. A detailed characterization consisting of 1H NMR and 31P NMR (Fig. S2), PXRD (Fig. S4), IR (Fig. S3) and TGA (Table S3) is reported in the ESI.†

4.3. General instrumentation

Nuclear magnetic resonance (NMR) measurements were recorded at 298 K on Bruker UltraShield 500 spectrometer operating at a frequency of 500.13 MHz. Regular 1H, and 31P NMR spectra were acquired using the standard pulse sequences with a 30 degree pulse with a recycle delay of 1.5, and 1.0 second from the Bruker library; zg30, zgpg30 respectively. 31P NMR spectra were acquired using inverse gated decoupling and 64 scans and were processed with a line broadening of 5 Hz. All resonances are background-corrected. Chemical shifts (δ) are given in parts per million (ppm), and the residual solvent peak was used as an internal standard (C6D6: δH = 7.16 ppm, CDCl3: δH = 7.26 ppm, tol-d8: δH = 2.09 ppm).
Single crystal XRD. Single crystal data were collected on STOE STADIVARI diffractometer with a microfocused Cu source. The crystals were kept at a steady T = 150 K during data collection. The structures were solved with the ShelXT60 solution program using dual methods and by using Olex261 as the graphical interface. The model was refined with ShelXL 2018/362 using full matrix least squares minimization on F2.
Quantum chemical calculations. All calculations were performed with the B3LYP functional together with the aug-cc-pVDZ basis set for C, H, O, Cl, and P atoms using Gaussian09.63–66 The aug-cc-pVDZ pseudopotential and associated basis set of Peterson et al. was taken from the Basis Set Exchange and applied to the Zr atoms.67,68 This level of theory was previously shown to be accurate for these types of metal complexes.11 Only calculations in the gas phase were carried out. To calculate the 31P NMR chemical shifts from the optimized structures, we followed the protocol of Willoughby et al.69
Powder X-ray diffraction (PXRD). PXRD patterns were collected at room temperature in transmission mode using a Stoe Stadi P diffractometer with a micro-focused Cu-Kα-source (λ = 1.542 Å) equipped with a DECTRIS MYTHEN 1K detector.
Fourier transform infrared spectroscopy (FTIR). FTIR spectra were recorded air-free in a nitrogen-filled glovebox in transmission mode on a Bruker Alpha II FTIR-Spectrometer. The measured pellets were prepared air-free by mixing the sample with potassium bromide and compressing them.
Thermogravimetric analysis (TGA). TGA was performed on a TGA5500 (TA instruments) instrument. The samples were heated to 800 °C at a ramping rate of 5 °C min−1. At the end an isotherm of 15 min is given to ensure that all the organics are burned out.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The authors thank the SNSF Eccelenza funding scheme (project number: 194172) and the Swiss Nanoscience Institute for funding. The authors thank Prof. Daniel Häussinger for fruitful discussions and scientific assistance regarding NMR spectroscopy. The authors thank Dr Sven Sahle and Dr Frank Bergmann for their support in operating the modeling program COPASI. The authors thank Prof. Markus Meuwly and Dr Mike Devereux for setting up the computational calculations and fruitful discussions. The authors acknowledge Jikson Pulparayil Mathew for helping with TGA measurements, Prof. Murielle F. Delley for providing the air-free IR instrumentation setup, and Vanessa Wyss for helping with the IR measurements.

References

  1. N. N. Greenwood and A. Earnshaw, in Titanium, Zirconium and Hafnium, Elsevier, 1997, book section 21, pp. 954–975 Search PubMed.
  2. P. Arnal, R. J. P. Corriu, D. Leclercq, P. H. Mutin and A. Vioux, A Solution Chemistry Study of Nonhydrolytic Sol-Gel Routes to Titania, Chem. Mater., 1997, 9, 694–698 CrossRef CAS.
  3. A. Vioux, Nonhydrolytic Sol-Gel Routes to Oxides, Chem. Mater., 1997, 9, 2292–2299 CrossRef.
  4. T. J. Trentler, T. E. Denler, J. F. Bertone, A. Agrawal and V. L. Colvin, Synthesis of TiO2 Nanocrystals by Nonhydrolytic Solution-Based Reactions, J. Am. Chem. Soc., 1999, 121, 1613–1614 CrossRef CAS.
  5. M. Niederberger, M. H. Bartl and G. D. Stucky, Benzyl alcohol and titanium tetrachloride - A versatile reaction system for the nonaqueous and low-temperature preparation of crystalline and luminescent titania nanoparticles, Chem. Mater., 2002, 14, 4364–4370 CrossRef CAS.
  6. M. Niederberger and G. Garnweitner, Organic Reaction Pathways in the Nonaqueous Synthesis of Metal Oxide Nanoparticles, Chemistry, 2006, 12, 7282–7302 CrossRef CAS PubMed.
  7. D. Van den Eynden, R. Pokratath and J. De Roo, Nonaqueous Chemistry of Group 4 Oxo Clusters and Colloidal Metal Oxide Nanocrystals, Chem. Rev., 2022, 122, 10538–10572 CrossRef CAS PubMed.
  8. M. Parvizian and J. De Roo, Precursor chemistry of metal nitride nanocrystals, Nanoscale, 2021, 13, 18865–18882 RSC.
  9. R. Deshmukh and M. Niederberger, Mechanistic Aspects in the Formation, Growth and Surface Functionalization of Metal Oxide Nanoparticles in Organic Solvents, Chem. – Eur. J., 2017, 23, 8542–8570 CrossRef CAS PubMed.
  10. C. Suchomski, D. Weber, P. Dolcet, A. Hofmann, P. Voepel, J. Yue, M. Einert, M. Möller, S. Werner and S. Gross, et al., Sustainable and surfactant-free high-throughput synthesis of highly dispersible zirconia nanocrystals, J. Mater. Chem. A, 2017, 5, 16296–16306 RSC.
  11. R. Pokratath, D. Van den Eynden, S. R. Cooper, J. K. Mathiesen, V. Waser, M. Devereux, S. J. L. Billinge, M. Meuwly, K. M. O. Jensen and J. De Roo, Mechanistic Insight into the Precursor Chemistry of ZrO2 and HfO2 Nanocrystals; towards Size-Tunable Syntheses, JACS Au, 2022, 2, 827–838 CrossRef CAS PubMed.
  12. J. Joo, T. Yu, Y. W. Kim, H. M. Park, F. Wu, J. Z. Zhang and T. Hyeon, Multigram Scale Synthesis and Characterization of Monodisperse Tetragonal Zirconia Nanocrystals, J. Am. Chem. Soc., 2003, 125, 6553–6557 CrossRef CAS PubMed.
  13. R. Pokratath, L. Lermusiaux, S. Checchia, J. P. Mathew, S. R. Cooper, J. K. Mathiesen, G. Landaburu, S. Banerjee, S. Tao, N. Reichholf and J. De Roo, An Amorphous Phase Precedes Crystallization: Unraveling the Colloidal Synthesis of Zirconium Oxide Nanocrystals, ACS Nano, 2023, 17, 8796–8806 CrossRef CAS.
  14. J. Tang, J. Fabbri, R. D. Robinson, Y. Zhu, I. P. Herman, M. L. Steigerwald and L. E. Brus, Solid-Solution Nanoparticles: Use of a Nonhydrolytic Sol-Gel Synthesis To Prepare HfO2 and HfxZr1−xO2 Nanocrystals, Chem. Mater., 2004, 16, 1336–1342 CrossRef CAS.
  15. S. W. Depner, K. R. Kort and S. Banerjee, Precursor control of crystal structure and stoichiometry in twin metal oxide nanocrystals, CrystEngComm, 2009, 11, 841–846 RSC.
  16. S. W. Depner, N. D. Cultrara, K. E. Farley, Y. Qin and S. Banerjee, Ferroelastic Domain Organization and Precursor Control of Size in Solution-Grown Hafnium Dioxide Nanorods, ACS Nano, 2014, 8, 4678–4688 CrossRef CAS PubMed.
  17. G. R. Waetzig, S. W. Depner, H. Asayesh-Ardakani, N. D. Cultrara, R. Shahbazian-Yassar and S. Banerjee, Stabilizing metastable tetragonal HfO2 using a non-hydrolytic solution-phase route: ligand exchange as a means of controlling particle size, Chem. Sci., 2016, 7, 4930–4939 RSC.
  18. T. Ohlerth, H. Du, T. Hammoor, J. Mayer and U. Simon, Tailoring of Colloidal HfO2 Nanocrystals with Unique Morphologies and New Self-Assembly Features, Small Sci., 2024, 2300209 CrossRef CAS.
  19. R. L. Davidovich, D. V. Marinin, V. Stavila and K. H. Whitmire, Structural chemistry of fluoride and oxofluoride complexes of titanium(IV), Coord. Chem. Rev., 2015, 299, 61–82 CrossRef CAS.
  20. B. Krebs, Die Kristallstruktur von Zirkoniumtetrachlorid, Z. Anorg. Allg. Chem., 1970, 378, 263–272 CrossRef CAS.
  21. H. Bialowons, M. Müller and B. G. Müller, Titantetrafluorid – Eine überraschend einfache Kolumnarstruktur, Z. Anorg. Allg. Chem., 1995, 621, 1227–1231 CrossRef CAS.
  22. G. B. Nikiforov, H. W. Roesky and D. Koley, A survey of titanium fluoride complexes, their preparation, reactivity, and applications, Coord. Chem. Rev., 2014, 258–259, 16–57 CrossRef CAS.
  23. D. S. Dyer and R. O. Ragsdale, Fluorine-19 nuclear magnetic resonance study of some titanium tetrafluoride-substituted pyridine 1-oxide adducts, Inorg. Chem., 1969, 8, 1116–1120 CrossRef CAS.
  24. M. Jura, W. Levason, E. Petts, G. Reid, M. Webster and W. Zhang, Taking TiF4 complexes to extremes-the first examples with phosphine co-ligands, Dalton Trans., 2010, 39, 10264–10271 RSC.
  25. M. Nieger, L. Szarvas and D. Gudat, CSD Communication, 2022 Search PubMed.
  26. F. Calderazzo, U. Englert, G. Pampaloni and M. Volpe, Redox reactions with bis (η6-arene) derivatives of early transition metals, J. Organomet. Chem., 2005, 690, 3321–3332 CrossRef CAS.
  27. M. Eberle and C. Röhr, Tetrachlorobis (tetrahydrofuran-O) zirconium(IV), Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1996, 52, 566–568 CrossRef.
  28. S. Duraj, R. Towns, R. Baker and J. Schupp, Structure of cis-tetrachlorobis (tetrahydrofuran) hafnium(IV), Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1990, 46, 890–892 CrossRef.
  29. C. E. F. Rickard, M. W. Glenny and A. J. Nielson, Tetra-chloro(N,N,N′,N′-tetra-methyl-ethyl-enediamine)-zirconium(IV), Acta Crystallogr., Sect. E: Struct. Rep. Online, 2003, 59, m183–m184 CrossRef CAS.
  30. S. Troyanov, G. Mazo and M. Simonov, Koord. Khim., 1985, 11, 1147 CAS.
  31. S. Rabe and U. Müller, Die Kristallstrukturen von PPh4 [MCl5(NCMe)]·MeCN (M = Ti, Zr), zwei Modifikationen von PPh4 [TiCl5 (NCMe)] und von cis-TiCl4(NCMe)2·MeCN, Z. Anorg. Allg. Chem., 2001, 627, 201–205 CrossRef CAS.
  32. S. Troyanov, G. Mazo, M. Simonov and A. Il'inskii, Zh. Neorg. Khim., 1986, 31, 3022 CAS.
  33. S. Troyanov, G. Mazo and M. Simonov, Koord. Khim., 1986, 12, 1000 CAS.
  34. J. Guery, C. Leblanc and M. Jacoboni, Eur. J. Solid State Inorg. Chem., 1989, 26, 289 CAS.
  35. S. Troyanov, G. Mazo and M. Simonov, Vestn. Mosk. Univ., Ser. 2: Khim., 1986, 27, 575 CAS.
  36. S. Troyanov and M. Subbotin, Zh. Neorg. Khim., 1987, 32, 58 CAS.
  37. D. S. Dyer and R. O. Ragsdale, cis–trans-Isomerism in titanium tetrafluoride–substituted pyridine 1-oxide adducts, Chem. Commun., 1966, 601–602 RSC.
  38. D. S. Dyer and R. O. Ragsdale, The Mechanism of Fluorine-19 Exchange in the TiF4·2(Donor) Complexes, J. Am. Chem. Soc., 1967, 89, 1528–1529 CrossRef CAS.
  39. G. B. Nikiforov, C. Knapp, J. Passmore and A. Decken, Interaction of TiF4 with the donor solvents SO2, PhCN, and MeCN: Isolation and structural characterization of the first trimeric fluorine bridged donor–acceptor adduct {TiF4 (PhCN)}3, J. Fluorine Chem., 2006, 127, 1398–1404 CrossRef CAS.
  40. H. Weingarten and J. R. Van Wazer, Exchange of Parts between Molecules at Equilibrium. VI. Scrambling on Titanium of the Alkoxyl, Dimethylamino, and Halogen Substituents, J. Am. Chem. Soc., 1965, 87, 724–730 CrossRef CAS.
  41. D. C. Bradley, R. C. Mehrotra, I. P. Rothwell and A. Singh, Alkoxo and Aryloxo Derivatives of Metals, Academic Press, London, 2001 Search PubMed.
  42. D. Bradley, F. Abd-El Halim, R. Mehrotra and W. Wardlaw, 898. Reactions of acetyl chloride with zirconium alkoxides, J. Chem. Soc., 1952, 4609–4612 RSC.
  43. S. Hoops, S. Sahle, R. Gauges, C. Lee, J. Pahle, N. Simus, M. Singhal, L. Xu, P. Mendes and U. Kummer, COPASI: a COmplex PAthway SImulator, Bioinformatics, 2006, 22, 3067–3074 CrossRef CAS.
  44. U. Mayer, V. Gutmann and W. Gerger, The acceptor number – A quantitative empirical parameter for the electrophilic properties of solvents, Monatsh. Chem., 1975, 106, 1235–1257 CrossRef CAS.
  45. M. A. Beckett, G. C. Strickland, J. R. Holland and K. Sukumar Varma, A convenient n.m.r. method for the measurement of Lewis acidity at boron centres: correlation of reaction rates of Lewis acid initiated epoxide polymerizations with Lewis acidity, Polymer, 1996, 37, 4629–4631 CrossRef CAS.
  46. M.-F. Li and Y.-K. Shan, Crystal structure of trans-bis (triphenylphospine oxide) tetrachlorotitanum (IV), TiCl4[(C6H5)3PO]2, Z. Kristallogr. - New Cryst. Struct., 2006, 221, 41–42 CAS.
  47. S. L. Benjamin, W. Levason, D. Pugh, G. Reid and W. Zhang, Preparation and structures of coordination complexes of the very hard Lewis acids ZrF4 and HfF4, Dalton Trans., 2012, 41, 12548–12557 RSC.
  48. D. C. Bradley, D. C. Hancock and W. Wardlaw, 524. Titanium chloride alkoxides, J. Chem. Soc., 1952, 2773–2778 RSC.
  49. E. Wiberg and W. Sütterlin, Zur Kenntnis einiger Verbindungen vom Typus BCl3-n(OR)n 2. Mitteilung. Über Disproportionierungen in der Verbindungsreihe BCl3-n(OCH3)n, Z. Anorg. Allg. Chem., 1935, 222, 92–97 CrossRef CAS.
  50. E. Wiberg and H. Smedsrud, Über Verbindungen des Typus BCl3-n(OR)n. 3. Mitteilung. Darstellung von BCl2OCH3 und BCl(OCH3)2 aus Borchlorid und Borsäure-methylester, Z. Anorg. Allg. Chem., 1935, 225, 204–208 CrossRef CAS.
  51. I. Ostromisslensky, Über eine neue, auf dem Massenwirkungsgesetz fußende Analysenmethode einiger binären Verbindungen, Ber. Dtsch. Chem. Ges., 1911, 44, 268–273 CrossRef CAS.
  52. R. B. Denison, Contributions to the knowledge of liquid mixtures—I. Property-composition curves and the molecular changes which take place on forming binary liquid mixtures, Trans. Faraday Soc., 1912, 8, 20–34 RSC.
  53. P. Job, Formation and stability of inorganic complexes in solution, Ann. Chim., 1928, 9, 113–134 CAS.
  54. E. J. Olson and P. Bühlmann, Getting more out of a Job plot: determination of reactant to product stoichiometry in cases of displacement reactions and n:n complex formation, J. Org. Chem., 2011, 76, 8406–8412 CrossRef CAS PubMed.
  55. P. Erdmann and L. Greb, What Distinguishes the Strength and the Effect of a Lewis Acid: Analysis of the Gutmann–Beckett Method, Angew. Chem., 2022, 134, e202114550 CrossRef.
  56. L. E. Manzer, J. Deaton, P. Sharp and R. R. Schrock, in 31. Tetrahydrofuran Complexes of Selected Early Transition Metals, 1982, vol. 21, pp. 135–140 Search PubMed.
  57. D. Bradley, R. Mehrotra and W. Wardlaw, 330. Hafnium alkoxides, J. Chem. Soc., 1953, 1634–1636 RSC.
  58. E. Dhaene, C. Seno and J. De Roo, Synthesis of zirconium(IV) and hafnium(IV) isopropoxide, sec-butoxide and tert-butoxide, ChemRxiv, 2023,  DOI:10.26434/chemrxiv-2023-4s67j-v2.
  59. J. S. Owen, J. Park, P.-E. Trudeau and A. P. Alivisatos, Reaction chemistry and ligand exchange at cadmium- selenide nanocrystal surfaces, J. Am. Chem. Soc., 2008, 130, 12279–12281 CrossRef CAS PubMed.
  60. G. M. Sheldrick, SHELXT-Integrated space-group and crystal-structure determination, Acta Crystallogr., Sect. A: Found. Adv., 2015, 71, 3–8 CrossRef PubMed.
  61. O. V. Dolomanov, L. J. Bourhis, R. J. Gildea, J. A. Howard and H. Puschmann, OLEX2: a complete structure solution, refinement and analysis program, J. Appl. Crystallogr., 2009, 42, 339–341 CrossRef CAS.
  62. G. M. Sheldrick, Crystal structure refinement with SHELXL, Acta Crystallogr., Sect. C: Struct. Chem., 2015, 71, 3–8 Search PubMed.
  63. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery, J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, J. M. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, Ö. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 09 Revision B.01, Gaussian, Inc., Wallingford, 2009 Search PubMed.
  64. A. D. Becke, Density-functional exchange-energy approximation with correct asymptotic behavior, Phys. Rev. A, 1988, 38, 3098–3100 CrossRef CAS PubMed.
  65. R. A. Kendall, T. H. Dunning and R. J. Harrison, Electron affinities of the first-row atoms revisited. Systematic basis sets and wave functions, J. Chem. Phys., 1992, 96, 6796–6806 CrossRef CAS.
  66. C. Lee, W. Yang and R. G. Parr, Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785–789 CrossRef CAS PubMed.
  67. K. A. Peterson, D. Figgen, M. Dolg and H. Stoll, Energy-consistent relativistic pseudopotentials and correlation consistent basis sets for the 4d elements Y–Pd, J. Chem. Phys., 2007, 126, 124101 CrossRef PubMed.
  68. B. P. Pritchard, D. Altarawy, B. Didier, T. D. Gibson and T. L. Windus, New Basis Set Exchange: An Open, Up-to-Date Resource for the Molecular Sciences Community, J. Chem. Inf. Model., 2019, 59, 4814–4820 CrossRef CAS PubMed.
  69. P. H. Willoughby, M. J. Jansma and T. R. Hoye, A guide to small-molecule structure assignment through computation of (1H and 13C) NMR chemical shifts, Nat. Protoc., 2014, 9, 643–660 CrossRef CAS PubMed.

Footnote

† Electronic supplementary information (ESI) available: Crystallographic data of previously unreported single crystals and their characterization with 1H NMR and 31P NMR, PXRD, IR and TGA. 1H NMR and 31P NMR spectra of metal complexes and detailed description of job plot analysis. CCDC 2219601, 2219602 and 2219604–2219606. The raw data for all the figures are openly available on Zenodo: https://doi.org/10.5281/zenodo.11192035. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d4dt01299b
This journal is © The Royal Society of Chemistry 2024
Click here to see how this site uses Cookies. View our privacy policy here.