From benzene to naphthalene: direct measurement of reactions and intermediates of phenyl radicals and acetylene

Abstract

Hydrogen-abstraction–C₂H₂-addition (HACA) is one of the most important pathways leading to the formation of naphthalene, the simplest two-ring polycyclic aromatic hydrocarbon (PAH). The major reaction channels for naphthalene formation have previously been calculated by Mebel et al., but few experiments exist to validate the theoretical predictions. In this work, time-resolved molecular beam mass spectrometry (MBMS) was used to investigate the time-dependent product formation in the reaction of a phenyl radical with C₂H₂ for the first time, at temperatures of 600 and 700 K and pressures of 10 and 50 Torr. A pressure-dependent model was developed with rate parameters derived from Mebel et al.'s calculations and from newly calculated pathways on the C₈H₇ PES at the G3(MP2,CC)//B3LYP/6-311G** level of theory. The model prediction is consistent with the MBMS product profiles at a mass-to-charge ratio (m/z) of 102 (corresponding to the H-loss product from C₈H₇, phenylacetylene), 103 (the initial C₈H₇ adduct and its isomers plus the ¹³C isotopologue of phenylacetylene), 128 (naphthalene), and 129 (C₁₀H₉ isomers plus the ¹³C isotopologue of naphthalene). An additional C₈H₇ isomer, bicyclo[4.2.0]octa-1,3,5-trien-7-yl, not considered by Mebel et al.'s calculations, contributes significantly to the signal at m/z 103 due to its stable energy and low reactivity. At high C₂H₂ concentrations, bimolecular reactions dominated the observed chemistry, and the m/z 128 and m/z 102 MBMS signal ratio was measured to directly determine the product branching ratio. At 600 K and 10 Torr, branching to the H-loss product (phenylacetylene) on the C₈H₇ PES accounted for 7.9% of phenyl radical consumption, increasing to 15.9% at 700 K and 10 Torr. At 50 Torr, the branching was measured to be 2.8% at 600 K and 6.2% at 700 K. Adduct stabilization is favored at higher pressure and lower temperature, which hinders the formation of the H-loss product. The pressure-dependent model predicted the observed branching ratios within the experimental uncertainty, indicating that the rate parameters reported here can be used in combustion mechanisms to provide insights into phenyl HACA reactions and PAH formation.

---

1. Introduction

Polycyclic aromatic hydrocarbons (PAHs) have attracted wide attention in combustion as precursors of soot,^1–5 and their build-up process from small molecules has a significant impact on process efficiency and the desired product selectivity.^6–8 A number of kinetic models have been proposed to understand the formation of naphthalene, one of the simplest two-ring PAHs, most notably the hydrogen-abstraction–C₂H₂-addition (HACA) mechanism starting from benzene and a phenyl radical (C₆H₅) due to its accessible entrance barrier and significant exothermicity.^1,3,9 The potential energy surface (PES) of C₆H₅ + acetylene (C₂H₂) was reported by Richter et al. at the B3LYP/cc-PVDZ level of theory with pressure-dependent rate constant calculations.¹⁰ Tokmakov et al. further investigated the C₆H₅ + C₂H₂ reaction using the G2M method and applied Rice–Ramsperger–Kassel–Marcus (RRKM) theory to predict the kinetics and product branching ratios.¹¹ To investigate the chemistry beyond the C₈H₇ PES, Kislov et al. used the G3(MP2,CC) level of theory to explore the reactions involving a second acetylene addition, which include C₈H₅, C₁₀H₇, and C₁₀H₉ PESs, as well as other important reactions in HACA chemistry.¹² With the kinetics evaluated from those calculations, the Diels–Alder mechanism was found to be much slower than the HACA mechanism at high combustion temperatures. More recent calculations reported by Mebel et al. improved Kislov et al.'s calculations¹² by performing RRHO-based variational transition state theory (VTST) calculations for low-barrier reactions and variable reaction coordinate-transition state theory (VRC-TST)¹³ for barrierless H-elimination reactions on the C₁₀H₇ PES.¹⁴ Branching ratios in a wide range of temperatures and pressures were evaluated, and naphthalene was predicted to be the dominant product at temperatures of up to 2500 K.

Several experimental kinetics studies of C₆H₅ HACA reactions have also been performed. Fahr and Stein conducted pyrolysis experiments in a flow reactor to measure the kinetics of phenylacetylene + H formation at 1000–1330 K.¹⁵ Yu et al. used cavity ringdown spectroscopy to measure the total C₆H₅ + acetylene rate constant at 297–523 K, and by assuming a constant C₆H₅ recombination rate, they correlated their rates measured at relatively low temperatures with those measured in Fahr et al.'s experiments.^15–17 Using a reflectron time of flight mass spectrometer, Parker et al. directly observed the formation of phenylacetylene and naphthalene in the phenyl + acetylene reaction.¹⁸ Subsequent work by Yang et al. found further evidence of the styrenyl and ortho-vinylphenyl radicals, which supported previous theoretical work.¹⁹ However, the time-dependent products and the product branching ratio have not been directly measured previously.

In this work, we directly measured the time dependent formation of products in the C₆H₅ + C₂H₂ HACA reaction using molecular beam mass spectrometry (MBMS) under a range of T and P conditions (600–700 K and 10–50 Torr). A pressure-dependent model was developed using Mebel et al.'s ab initio calculations¹⁴ and additional pathways on the C₆H₅ + C₂H₂ PES calculated here, and compared with our experimental data to assess the validity of the proposed reaction channels for accurately predicting the formation of naphthalene via the HACA mechanism.

2. Experimental

The experimental apparatus used in this work has been described previously.^20–22 Time-resolved molecular beam mass spectrometry (MBMS) was used to measure product signals at pressures of 10 and 50 Torr and temperatures of 600 and 700 K. Experiments were performed in a custom quartz flow reactor (1.6 cm inner diameter in the reaction region) in a high vacuum chamber equipped with a reflectron time-of-flight mass spectrometer (Kore TOF-MS). Gases were well-mixed before flowing into the reactor and were pumped using a Roots blower (Leybold). The pressure in the reactor was held constant at 10 or 50 Torr by throttling a butterfly valve leading to the pump at the reactor outlet. The reactor was wrapped with nichrome ribbon wire for heating up the gas to the desired temperatures, and two thermocouples were located inside the reactor to control the temperature. Prior to the experiments, axial temperature profiles with 2.5 cm resolution along the length of the reactor were measured to obtain the real temperatures of the gas mixture used for kinetic modeling.

A collimated pulsed 266 nm laser beam (fourth harmonic frequency of an Nd:YAG laser) with 1 Hz repetition rate was focused coaxially into the reactor to photolyze the radical precursor. The total gas flow rate was adjusted to maintain one flash per refresh condition. Additional experiments with higher C₂H₂ concentration were performed under each set of temperature and pressure conditions. The gas mixture was sampled through a pinhole in the reactor center and formed a molecular beam intersecting with a focused 118 nm (10.5 eV) photoionization laser beam (ninth harmonic frequency of an Nd:YAG laser), which formed cations measured by MBMS. Time-of-flight mass spectra were measured at reaction times of up to 8 ms after the photolysis pulse in order to observe products from both the first and the second C₂H₂ addition. Peaks on the mass spectra at different mass-to-charge ratios (m/z) were integrated and compared with model predictions. Before the laser beam entered the reactor, an adjustable iris was placed in its path to control the size of the laser beam. The laser diameter was clipped from 1.8 cm to 1.5 cm, slightly smaller than the reactor inner diameter, which gives two main advantages: (1) clipping the edges of the beam can decrease inhomogeneities in the radical concentration produced by the laser beam. (2) No direct contact was made between the reactor inner wall and the laser beam, reducing undesired wall reactions which apparently promote the fast hydrogenation of the phenyl radical to benzene, observed in MBMS experiments when the laser beam size was not optimized.²¹ Nitrosobenzene (C₆H₅NO; Sigma-Aldrich, 98%) was used as the photolytic precursor of the phenyl radical (C₆H₅).^16,17 To remove oxygen and impurities, several freeze–pump–thaw cycles were performed on C₆H₅NO.²¹ Helium (He) was the carrier gas and bath gas for all the experiments with UHP grade purity (≥99.999%) obtained from Airgas; acetylene (C₂H₂) with ≥99.5% purity was used (CP grade) and was also purchased from Airgas; and acetone in the acetylene was removed using a cartridge activated charcoal gas purifier (SGD80003) obtained from Airgas.

3. Theoretical

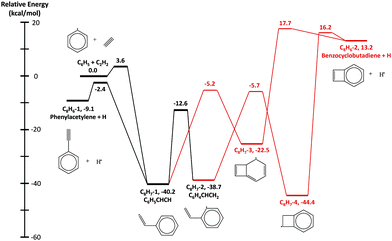
The HACA routes of C₆H₅ were previously explored by Mebel et al. at the G3(MP2,CC)//B3LYP/6-311G** level of theory¹² including C₈H₇, C₁₀H₇, and C₁₀H₉ potential energy surfaces (PESs) and other reactions such as H-abstraction, radical recombination, or radical disproportionation.^12,14 In this work, two additional C₈H₇ isomers (bicyclo[4.2.0]octa-2,4,7-trien-1-yl, C₈H₇-3, in Fig. 1 and bicyclo[4.2.0]octa-1,3,5-trien-7-yl, C₈H₇-4, in Fig. 1) and one C₈H₆ (benzocyclobutadiene, C₈H₆-2 in Fig. 1) + H product along with four transition states are included in the updated C₈H₇ PES, shown in Fig. 1. These species were reported by Richter et al.¹⁰ and Tokmakov and Lin¹¹ in previous theoretical literature; the geometries were optimized and frequencies were calculated at the B3LYP/6-311G** level of theory using Richter et al.'s¹⁰ geometry as the initial guess. The only exception is the transition state between C₈H₇-4 and C₈H₆-2 + H, which was optimized at the M062X/cc-pVTZ level of theory, because the B3LYP/6-311G** method could not find a correct converged geometry. Its frequencies were later calculated at the B3LYP/6-311G** level to keep it consistent with other species on the C₈H₇ PES. The energies of the stationary points and transition states were calculated using the same method as Mebel et al.,¹⁴ G3(MP2,CC)//B3LYP/6-311G**. All the calculations were performed in Gaussian 16,²³ except for the rCCSD(T) energy used in the G3(MP2,CC) method which was obtained from Molpro 2015.^24–34 Molecular parameters for the newly calculated stationary points are reported in the ESI.† For the rest of the species on the C₈H₇, C₁₀H₇ and C₁₀H₉ PESs, ab initio data were directly taken from the work of Mebel et al.¹⁴

Fig. 1 Potential energy surface of the G3(MP2,CC) stationary point energies for the C₆H₅ + C₂H₂ reaction. Black species and pathways were calculated by Mebel et al. Newly added species and pathways are marked in red.

Arkane,³⁵ a package included in the open-source software Reaction Mechanism Generator (RMG),³⁶ was used to calculate phenomenological pressure-dependent rate coefficients k(T,P). The collisional energy-transfer parameter, 〈ΔE_down〉 = α(T) = 424 × (T/300 K)^0.62 cm⁻¹, was taken from the work of Mebel et al.³⁷ for argon bath gas, and scaled down for helium bath gas using Jasper et al.'s method.³⁸ While the theoretical rate coefficients reported by Mebel et al.¹⁴ were calculated using the direct diagonalization method to solve the master equation,^39,40 the present work used the modified strong collision approximation to calculate k(T,P) from pressure-dependent networks incorporating the additional pathways discussed above. cis/trans isomers with interconversion barriers <4 kcal mol⁻¹ are treated as lumped single species in our rate calculations. Lennard-Jones parameters for the different PESs were estimated from the reported transport properties of PAHs by Wang et al.⁴¹ Thermochemical properties for important species were obtained from the Narayanaswamy library in the RMG database, which focuses on aromatics formation and considers accurate experimental data along with G3MP2//B3 calculations.⁸ For other species not in the Narayanaswamy library, RMG was used to estimate their thermochemical properties with the group additivity method, which has recently been improved and extended by similarity matching and bicyclic decomposition for predicting fused cyclics.⁴² The above-mentioned k(T,P) and thermochemical properties were used to construct a pressure-dependent kinetic mechanism for C₆H₅ + C₂H₂. In addition to the main HACA routes, the mechanism includes side reactions that are important under our experimental conditions, such as addition of H atoms formed in HACA reactions to C₂H₂ forming vinyl radicals. The mechanism is attached in the ESI† in Chemkin format with the rate parameters and the species thermochemistry.

The detailed chemical mechanism for various C₆H₅ HACA pathways was used to simulate the time-dependent species concentrations under the conditions of the MBMS experiments to compare the theoretical calculations with the measured product signals. A similar approach was used by Buras et al.²¹ for experiments performed in the same apparatus. To account for the transport effects in molecular beam sampling and the reactor, a constant 0.2 ms time delay with a simple model with a first-order rate constant (k_sampling) was utilized, which was adapted from Baeza-Romero et al.⁴³ A previous work²¹ used the rise time of the I atom signal to estimate the sampling rate, which is not available in the present work, since we did not use the iodide. Here nitric oxide (NO), cogenerated with C₆H₅ through photolysis, was chosen to fit k_sampling, because it is barely affected by the C₆H₅ + C₂H₂ reactions. An example of the NO time series measured by MBMS experiments is given in the ESI† to compare with the model prediction with k_sampling. The fitted k_sampling only affects the time dependence of the absolute predicted signals, but has no effect on the relative abundance of species at any given time point. For most experiments, the fitted value of k_sampling was 1600–2000 s⁻¹, whereas a smaller value (1200 s⁻¹) was found for one experiment, possibly due to a shifted laser alignment. To further convert the species concentration into signals compared to the observed signals in the experiments, the predicted species concentration (C_i) after sampling was weighted by the photoionization cross section (PICS) for each species at 10.5 eV (118.2 nm): σ_NO = 3.1 Mb,⁴⁴σ_{phenylacetylene} = 63.0 Mb⁴⁵ (10% uncertainty), σ_{C8H7 radical} = 8 Mb (a factor of two uncertainty), σ_naphthalene = 51.4 Mb^18,46,47 (50% uncertainty), and σ_{C10H9 radical} = 8 Mb (a factor of two uncertainty). Lower PICS values were estimated for the radical species relative to the close-shell species, because of the correspondingly lower occupancy of the HOMO from which the electron is ejected.⁴⁸ However, aromatic resonance stabilized radicals (RSRs) commonly have PICS similar to the corresponding closed-shell structure, i.e. the benzyl radical (25.5 Mb⁴⁹) compared to toluene (32.0 Mb⁴⁵). Since both C₈H₇-3 and C₈H₇-4 in the C₈H₇ PES are RSRs, their PICSs are estimated to be 40 Mb (a factor of two uncertainty), similar to their closed-shell analogues, benzocyclobutane, with >50 Mb PICS at 10.5 eV ionization energy estimated by Li et al.⁴⁶ Due to the difference of PICS between some isomers, the MBMS measured signals cannot be converted to the lumped concentration of isomers; thus, the signals are compared instead of the species concentration in this work. The impact of ¹³C isotopes was assumed to contribute 1.1% per carbon atom for each of the products, and the same PICS was assumed for each set of isotopomers. Finally, a scaling constant C_i obtained from the internal calibration mixture for each experimental condition was multiplied with the PICS to obtain the time-dependent predicted product signals. Steps of the described modeling approach are depicted in the ESI.† For the rate of production (ROP) analysis, Chemical Workbench⁵⁰ was used to evaluate the reactant consumption under MBMS experimental conditions.

4. Results & discussion

4.1 Hydrogen-abstraction–C₂H₂-addition (HACA) routes of the phenyl radical

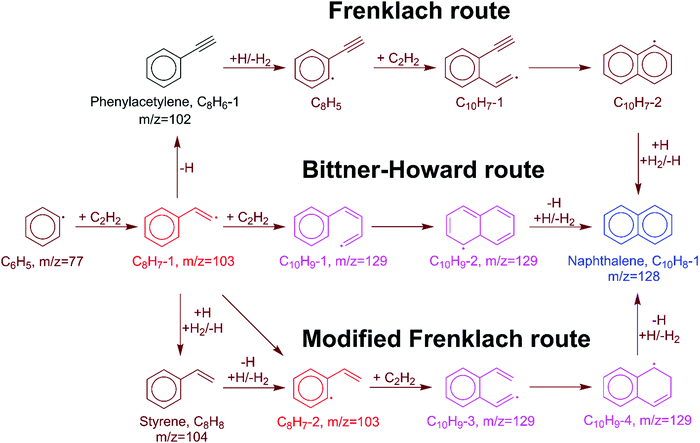
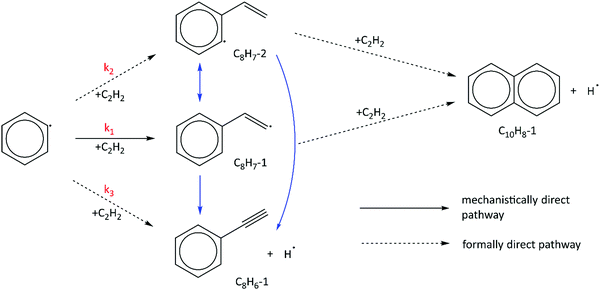
In the HACA mechanism for the reaction of the phenyl radical with C₂H₂, three pathways have been identified leading to naphthalene formation: the Frenklach,^3,9,51 Bittner–Howard,⁵² and modified Frenklach^53,54 routes. Reactions involved in each route are depicted in Fig. 2. In our previous study on methane-rich combustion at high temperature (>1400 K), the Frenklach route was the most important pathway of naphthalene formation,⁶ which involves external H abstraction from phenylacetylene (C₈H₆) forming a C₈H₅ radical before the second C₂H₂ addition. However, this route is not expected to be significant under the experimental conditions (600–700 K, 10–50 Torr) of this work where H atoms are generated from H-loss reactions. Due to high C₂H₂ concentration in our MBMS experiments, H atoms are mostly consumed by C₂H₂ instead of reacting with phenylacetylene; therefore, no clear time-dependence of the m/z = 127 signal corresponding to C₁₀H₇ isomers was observed, which supports this hypothesis.

Fig. 2 HACA reactions of the phenyl radical including the Frenklach, Bittner–Howard, and modified Frenklach routes. The main products observed in the MBMS experiments are indicated in black (phenylacetylene, m/z = 102), red (C₈H₇ isomers, m/z = 103), blue (naphthalene, m/z = 128), and pink (C₁₀H₉ isomers, m/z = 129).

In the Bittner–Howard route, the initial adduct of C₆H₅ + C₂H₂, C₈H₇-1, directly reacts with the second C₂H₂ to form the C₁₀H₉-1 radical, followed by isomerization and β-scission to the final product, naphthalene. In the modified Frenklach route, C₈H₇-2 can be generated via the direct well-skipping reaction of C₆H₅ + C₂H₂, isomerization of C₈H₇-1, or an H-assisted pathway involving the formation of styrene. The existence of styrene in this route suggests that we may expect observation of a signal at m/z = 104 in the MBMS experiments. Naphthalene is formed in a similar manner to the Bittner–Howard route by the reaction of C₈H₇-2 with C₂H₂, isomerization, and β-scission. Both the Bittner–Howard route and the modified Frenklach route involve reactions on the C₁₀H₉ PES. Under low pressure or high temperature conditions, well-skipping reactions become important even for fairly large molecules;⁵⁵ therefore, the C₈H₇-1 + C₂H₂ and C₈H₇-2 + C₂H₂ reactions can produce naphthalene + H without stabilization of C₁₀H₉ isomers. All of these reactions have been included in the pressure-dependent model developed in this work.

4.2 Products measured by MBMS experiments

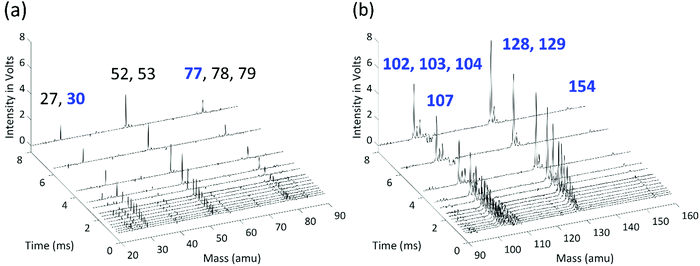
Time-resolved mass-spectra after background subtraction measured at 700 K and 10 Torr are shown in Fig. 3. A negative signal appears at m/z 107 (C₆H₅NO) after t = 0 due to the photolysis of the chemical precursor, and m/z = 30 (NO) and m/z = 77 (C₆H₅) peaks appear as the photolysis products are co-generated. The signal at m/z = 30 slowly increases to its maximum value due to the molecular beam sampling effect, and it remains nearly constant at longer reaction times, indicating that NO is not reactive. The signal of the phenyl radical, on the other hand, decreases rapidly due to its fast reaction with C₂H₂. The products of the reaction between C₆H₅ and the first C₂H₂ appear at m/z = 102 (H-loss product, phenylacetylene), 103 (the initial adduct C₈H₇-1 and its isomers plus the ¹³C isotopologue of phenylacetylene), and 104 (styrene, the side product in the modified Frenklach route). As the C₈H₇ isomers further react with a second C₂H₂, signals at m/z = 128 (naphthalene) and 129 (C₁₀H₉ isomers plus the ¹³C isotopologue of naphthalene) are observed in the mass spectra. The signal at m/z = 154 corresponds to biphenyl produced from phenyl radical recombination, which is less significant at higher temperature.

Fig. 3 Representative background-subtracted, time-resolved mass spectra, measured at 700 K and 10 Torr: (a) 20–90 amu; (b) 90–160 amu. Peaks related to phenyl + C₂H₂ reaction pathways and precursor photodissociation are labeled in blue; peaks labeled in black are attributed to the side products of the vinyl + C₂H₂ reaction.

When H atoms are eliminated in β-scission reactions, they rapidly react with the high concentration C₂H₂ in the reactor to generate C₂H₃ (vinyl radical, m/z = 27). Similar to the HACA reactions of the phenyl radical to form naphthalene, a vinyl radical can react with two C₂H₂ molecules to form benzene. The observed signals at m/z = 52 (vinylacetylene), m/z = 53 (C₄H₇ isomers plus the ¹³C isotopologue of m/z = 52), m/z = 78 (benzene), and m/z = 79 (C₆H₇ isomers plus the ¹³C isotopologue of m/z = 78) are attributed to the products of vinyl HACA reactions. The time-resolved signal intensities and the main reaction pathways for vinyl + C₂H₂ are available in the ESI.† This reaction network is under thorough study in our group, and will be discussed in detail in a separate publication. H atom consumption to form a vinyl radical is significant and is included in the model, but under our conditions further reactions of vinyl have negligible effects on the main peaks of interest here (m/z = 77, 102, 103, 104, 128, 129). Other peaks observed in the MBMS experiments are attributed to the calibration mixture and hydrocarbon impurities in C₂H₂ or C₆H₅NO, and show no discernible time dependence.

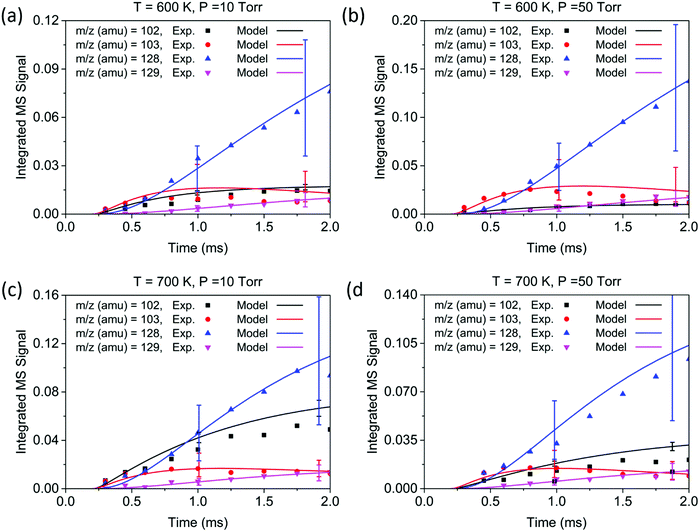
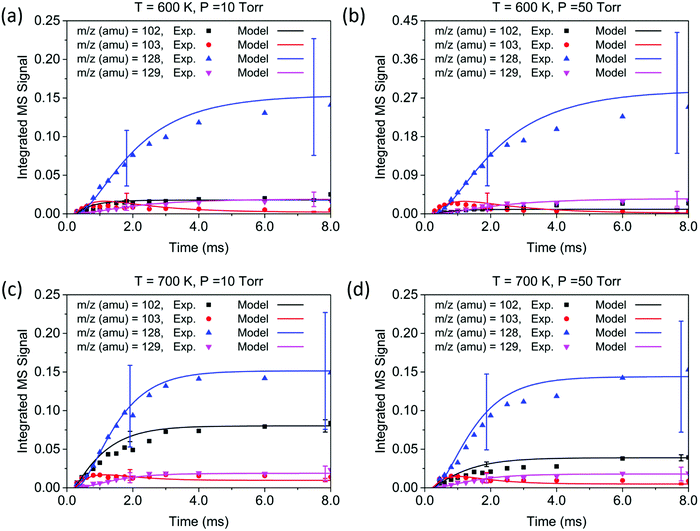
The conditions for each experiment are summarized in Table 1. In experiments 1–4, the concentration of C₂H₂ was controlled to achieve a rise time of ∼1.0 ms for the signal at m/z = 103. Under these conditions, the signals corresponding to the main products—at m/z = 102, 103, 128, and 129—appear within 2.0 ms, and reach a steady state at ∼8.0 ms. The reaction temperature T was determined by averaging over temperature measurements at different points in the reactor, in this case averaged over the 5 cm length on the upstream side of the center pinhole, which corresponds to the MBMS sampling region during the time after the flash. The initial concentration of C₆H₅ after photolysis is the same as that of NO; therefore, the NO signal (m/z = 30) together with its known ionization cross section (3.1 Mb⁴⁴) was used to determine the initial radical concentration. Time profiles of experimental measurements and the modeling results are shown in Fig. 4 for a short time scale (2.0 ms) and Fig. 5 for a long time scale (8.0 ms). The results for experiments 5–8 are given in the ESI,† and show that the product signals rise and reach a steady state earlier due to the faster reaction at higher C₂H₂ concentration.

Table 1 Conditions of MBMS experiments measuring the products of C₆H₅ + C₂H₂

Exp. #	Nominal T (K)	Real T (K)	P (Torr)	[Precursor]^a (10^13 cm^−3)	[C6H5]0^b^,^c (10^12 cm^−3)	[C2H2]^a (10^16 cm^−3)	k sampling (s^−1)
a 20% uncertainty due to mass flow controller calibrations. b Calculated from a stable NO signal at m/z = 30 after photolysis, σ118 nm,NO = 3.1 Mb.^44 c Photolysis energy is 15 mJ per pulse.
1	600	607.4 ± 0.6	10	2.2	1.16 ± 0.23	3.82	2000
2	600	595.0 ± 0.7	50	2.1	0.93 ± 0.19	3.77	2000
3	700	705.0 ± 0.7	10	2.2	0.92 ± 0.18	2.74	1600
4	700	693.1 ± 1.0	50	2.1	0.83 ± 0.17	2.69	2000
5	600	607.4 ± 0.6	10	2.2	1.05 ± 0.21	5.46	1800
6	600	595.0 ± 0.7	50	2.1	0.99 ± 0.20	5.38	2000
7	700	705.0 ± 0.7	10	2.2	0.80 ± 0.16	3.83	1200
8	700	693.1 ± 1.0	50	2.1	0.91 ± 0.18	3.77	1600

---

Fig. 4 Experimental time profiles (experiments 1–4, symbols) of primary product peaks measured by MBMS experiments at short time scales (2 ms). The solid curves are the model predictions. Uncertainty ranges of the model predictions (due to uncertainties in PICS) are shown as error bars. Conditions: (a) 600 K, 10 Torr, [C₂H₄] = 3.82 × 10¹⁶ molecule per cm³; (b) 600 K, 50 Torr, [C₂H₄] = 3.77 × 10¹⁶ molecule per cm³; (c) 700 K, 10 Torr, [C₂H₄] = 2.74 × 10¹⁶ molecule per cm³; (d) 700 K, 50 Torr, [C₂H₄] = 2.69 × 10¹⁶ molecule per cm³.

Fig. 5 Experimental time profiles (experiments 1–4, symbols) of primary product peaks measured by MBMS experiments at long time scales (8 ms). The solid curves are the model predictions. Uncertainty ranges of the model predictions are shown as error bars. Conditions: (a) 600 K, 10 Torr, [C₂H₄] = 3.82 × 10¹⁶ molecule per cm³; (b) 600 K, 50 Torr, [C₂H₄] = 3.77 × 10¹⁶ molecule per cm³; (c) 700 K, 10 Torr, [C₂H₄] = 2.74 × 10¹⁶ molecule per cm³; (d) 700 K, 50 Torr, [C₂H₄] = 2.69 × 10¹⁶ molecule per cm³.

Overall, the measurement and the model show quantitative agreement within the error bars for the experiments in Fig. 4 and 5. The model shows that phenylacetylene comprises almost 100% of the m/z = 102 signal under all experimental conditions, as does naphthalene to the m/z = 128 signal. At early time scales of up to 1.0 ms, the signal at m/z = 103 increases rapidly due to the generation of initial adduct C₈H₇-1 by the C₆H₅ + C₂H₂ (+M) → C₈H₇-1 (+M) reaction. The rise time for this species is faster than those for all other major products under our experimental conditions, except for the experiment at 700 K and 10 Torr. Under these relatively high temperature and low pressure conditions, the “formally direct” pathway C₆H₅ + C₂H₂ (+M) → phenylacetylene + H (+M) leads to a faster rate of phenylacetylene generation and thus a larger m/z = 102 signal was observed in both the experiment and the model. We define “formally direct” pathways as reactions traversing more than one transition state; conversely, “mechanistically direct” pathways refer to reactions that have one transition state connecting reactants and products.^56–59 At the beginning of the C₆H₅ + C₂H₂ reaction, a rate-of-production (ROP) analysis indicates that 8.0% of C₆H₅ consumption directly forms C₈H₆ at 600 K and 10 Torr, compared to 16.3% at 700 K and 10 Torr. Furthermore, more m/z = 102 signal was observed at higher temperature. At 700 K, in addition to the “formally direct” pathway leading to C₈H₆ formation, thermalized C₈H₇-1 and C₈H₇-2 have more energy to overcome the barriers to H elimination, e.g. C₈H₇-1 (+M) → phenylacetylene + H (+M). As more C₈H₇ isomers react to form the H-elimination product instead of reacting with a second C₂H₂ at higher temperatures, the relative yield of the phenylacetylene product at m/z = 102 is expected to increase over naphthalene at m/z = 128. The ROP analysis shows that, at 1.0 ms, the reaction channel C₈H₇-1 (+M) → C₈H₆ + H (+M) accounts for only 0.41% of C₈H₇-1 consumption at 600 K and 10 Torr, and it increases to 16.3% at 700 K and 10 Torr. The product distribution also exhibits a dependence on pressure in both the measurement and the model: at higher pressure, the formation of C₈H₇ adducts is favored over the bimolecular products C₈H₆ + H; therefore, the signal at m/z = 102 becomes less significant when the pressure is increased from 10 to 50 Torr.

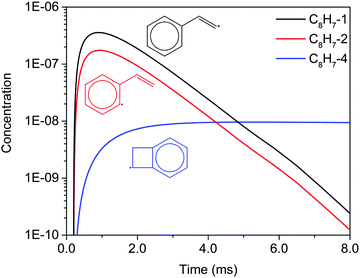
Unlike the m/z = 102 and m/z = 128 signals which are attributed to single species under our experimental conditions, the origin of the m/z = 103 and m/z = 129 signals is more complicated due to the combination of signals from multiple isomers as well as the contribution from ¹³C-substituted species. After reaching its maximum, the m/z = 103 signal is expected to decay to near zero because C₈H₇-1 and C₈H₇-2 are both highly reactive with C₂H₂. However, at 700 K after 3.0 ms in Fig. 5(c) and (d), the m/z = 103 signal remains constant and above zero. According to the model, part of this signal comes from ¹³C-substituted phenylacetylene, which contributes 8 × 1.1% of the m/z = 102 signal to the signal at m/z = 103. An interesting discovery is that the resonantly stabilized radical C₈H₇-4 has the highest concentration among all C₈H₇ isomers at later times, and accounts for 25% of the m/z = 103 signal at 700 K and 50 Torr at 8.0 ms in the model. Fig. 6 shows the modeled concentration of C₈H₇ isomers at 700 K and 50 Torr. The initial adduct C₈H₇-1 and its rapidly equilibrated isomer C₈H₇-2 both increase in concentration at early reaction times, but decrease significantly after 2.0 ms. The concentration of C₈H₇-4 is negligible at early time scales, but exceeds the concentration of other isomers after 6.0 ms. As seen in Fig. 1, C₈H₇-4 has the lowest energy of all species on the PES, and is surrounded by high barriers ∼40 kcal mol⁻¹. After thermalized C₈H₇-2 isomerizes into C₈H₇-4, the high energy barrier inhibits further reactions and thus leads to a long lifetime for the C₈H₇-4 radical. The signal at m/z = 129 is attributed mainly to ¹³C-substituted naphthalene, and none of the C₁₀H₉ isomers included in the model are predicted to produce observable signals at any time scale under our experimental conditions. The model prediction implies that naphthalene is mainly formed by “formally direct” pathways: C₈H₇-1 + C₂H₂ (+M) → naphthalene + H (+M) and C₈H₇-2 + C₂H₂ (+M) → naphthalene + H (+M). According to the ROP analysis, C₈H₇-1 + C₂H₂ (+M) → naphthalene + H (+M) accounts for 72.1% and C₈H₇-2 + C₂H₂ (+M) → naphthalene + H (+M) accounts for 27.5% of naphthalene formation for the experiment at 700 K and 10 Torr at 1.0 ms.

Fig. 6 Time dependent concentrations of C₈H₇ isomers predicted by the model at 700 K and 50 Torr.

4.3 Important reaction pathways and direct determination of branching ratios

We have shown that the kinetic model developed in this work, containing reactions on the C₈H₇, C₁₀H₇, and C₁₀H₉ PESs calculated by Mebel et al. in addition to other important HACA reactions, is well validated by the MBMS experiments. Using the validated model, the most important reaction pathways can be identified under our experimental conditions (600–700 K, 10–50 Torr). As shown in Fig. 7, C₆H₅ reacts with C₂H₂ to form the initial adduct C₈H₇-1 via a mechanistically direct pathway, and generates C₈H₇-2 and the bimolecular products C₈H₆-1 + H via formally direct pathways on the C₈H₇ PES. Isomerization between C₈H₇-1 and C₈H₇-2 appears to be significant in the model, as is the β-scission of both isomers into C₈H₆-1 + H. As discussed before, naphthalene (C₁₀H₈-1) is formed mainly through formally direct pathways for the reactions of C₈H₇-1 and C₈H₇-2 with a second C₂H₂ molecule, without thermalizing the C₁₀H₉ isomers. The validated model shows that naphthalene dominates by at least three orders of magnitude over other C₁₀H₈ isomers; therefore, the present m/z = 129 signal observed in the MBMS experiments is fully attributed to the ¹³C isotopologue of naphthalene. (Our model includes other C₁₀H₉ species (m/z = 129), but they have short lifetime, not persistent.)

Fig. 7 Important reaction pathways identified by the validated phenyl + C₂H₂ HACA model at 600–700 K and 10–50 Torr. Reaction pathways shown in blue can be ignored at high C₂H₂ concentration, where unimolecular reactions (reactions between C₈H₇-1, C₈H₇-2, and C₈H₆-1 + H) become negligible.

At high C₂H₂ concentration, all the bimolecular reactions with C₂H₂ dominate over unimolecular reactions. The isomerization reactions between C₈H₇-1 and C₈H₇-2 and their β-scission into C₈H₆-1 + H become negligible, such that only the bimolecular reactions including C₆H₅ + C₂H₂ (+M) → C₈H₇-1 (+M), C₆H₅ + C₂H₂ (+M) → C₈H₇-2 (+M), C₆H₅ + C₂H₂ (+M) → C₈H₆-1 + H (+M), C₈H₇-1 + C₂H₂ (+M) → C₁₀H₈-1 + H (+M), and C₈H₇-2 + C₂H₂ (+M) → C₁₀H₈-1 + H (+M) are significant. Since C₁₀H₈-1 + H are the sole final products for the bimolecular reactions of C₈H₇-1 and C₈H₇-2 with C₂H₂ and C₁₀H₈-1 fully dominates the m/z = 128 signal, as is the case for C₈H₆-1 in the m/z = 102 signal, we can use the signal ratio of m/z = 128 and m/z = 102 to determine the product branching ratios of C₆H₅ + C₂H₂ to C₁₀H₈-1 and C₈H₆-1:

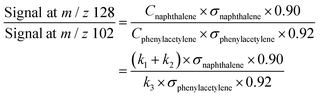The 0.90/0.92 factor in eqn (3) accounts for the ¹³C isotope abundance; k₁ is the rate coefficient for C₆H₅ + C₂H₂ (+M) → C₈H₇-1 (+M); k₂ is the rate coefficient for C₆H₅ + C₂H₂ (+M) → C₈H₇-2 (+M); and k₃ is the rate coefficient for C₆H₅ + C₂H₂ (+M) → C₈H₆-1 + H (+M).

Experiments to evaluate product branching ratios were conducted under similar conditions to those of the MBMS experiments discussed in the previous section, but using much higher C₂H₂ concentrations. To avoid the molecular sampling effect on MBMS signals at early time scales, all the signal ratios were obtained at least 1.0 ms after photolysis. At least six different delay times were measured for each set of experimental conditions, and the ratio of m/z = 128 to m/z = 102 was observed to remain stable within the measured time range, as seen in Fig. 8. As this ratio increases, the yield of H-loss products decreases in favor of radical adduct stabilization (followed by reaction with C₂H₂ to form naphthalene). Uncertainty propagation was performed to estimate the uncertainty of H-loss branching measured in the experiments. The main source of uncertainty is the uncertainty in PICS of phenylacetylene and naphthalene; further details for the uncertainty analysis are included in the ESI.†Table 2 summarizes the experimental conditions and the H-loss branching measured by the MBMS experiments and predicted by the model developed in this work.

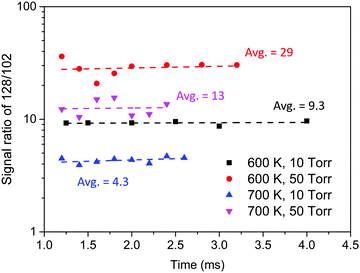Fig. 8 Signal ratio of m/z = 128 to m/z = 102 measured in the MBMS experiments with high C₂H₂ concentration.

Table 2 Experimental conditions and results of C₆H₅ + C₂H₂ product branching measurements

Nominal T (K)	Real T (K)	P (Torr)	k′^a (10^4 s^−1)	Time range (ms)	Number of time points	Predicted H-loss branching (%)	Measured H-loss branching (%)
a k′ = k × [C2H2], where k is the total rate constant of C6H5 + C2H2 calculated by Mebel et al.^14
600	607.4 ± 0.6	10	1.3	1.2–4.0	6	8.0	7.9 ± 4.9
600	595.0 ± 0.7	50	2.4	1.2–3.2	8	2.6	2.8 ± 1.6
700	705.0 ± 0.7	10	2.2	1.2–2.6	8	16.4	15.9 ± 8.0
700	693.1 ± 1.0	50	6.4	1.2–2.4	7	6.3	6.2 ± 3.4

---

The product branching predicted by the model falls within the uncertainty of the measurements under all the conditions studied in this work. At 600 K and 10 Torr, 7.9% of C₆H₅ reacts directly with C₂H₂ to form C₈H₆-1 + H, but this branching fraction decreases to 2.8% when the pressure is increased to 50 Torr. The reaction of C₆H₅ + C₂H₂ can also form chemically activated C₈H₇-1, the fate of which depends on the competition between collisional stabilization (k₁), isomerization followed by stabilization (k₂), and β-scission (k₃) under various temperature and pressure conditions. When the pressure increases, the greater efficiency of collisional stabilization of C₈H₇-1 by the bath gas reduces the probability of the formally direct H-loss pathway to form C₈H₆-1 + H, since C₈H₇-1 is more likely to become stabilized before other reactions can occur. Therefore, higher pressure leads to a lower branching fraction for the H-loss pathway, as was observed in the experiments at 700 K when the pressure was increased from 10 to 50 Torr (experiments 3 and 4). When the temperature is increased with pressure held constant, the H-loss branching fraction increases by a factor of two from 7.9% (600 K) to 15.9% (700 K) at 10 Torr, and from 2.8% (600 K) to 6.2% (700 K) at 50 Torr. Higher temperatures lead to a greater probability that chemically activated C₈H₇-1 formed from the reaction of C₆H₅ with C₂H₂ has sufficient internal energy to overcome the barrier to undergo β-scission. Given the excellent agreement between the model and experiment for the time-dependent signals of main products and the direct H-loss branching fractions, we conclude that our model has been thoroughly validated under the range of T and P conditions considered in this work. For future development of PAH formation mechanisms, the updated model derived from Mebel et al.¹⁴ presented here is therefore recommended to describe the HACA reactions of the phenyl radical with C₂H₂ as well as the formation of naphthalene.

Conclusions

C₆H₅ + C₂H₂ HACA reaction pathways were studied experimentally with MBMS under a range of T and P conditions (600–700 K, 10–50 Torr) and with a pressure-dependent kinetic model built using Mebel's ab initio calculations on the C₈H₇, C₁₀H₇, and C₁₀H₉ PESs in addition to newly calculated pathways on the C₈H₇ PES at the G3(MP2,CC)//B3LYP/6-311G** level of theory and other important side reactions. Time-dependent signals at various mass-to-charge ratios (m/z) were measured, and the corresponding species concentrations predicted by the model were converted into expected signals for direct comparison with the MBMS experiments.

Under the conditions in this work, major product peaks at m/z = 102 (H-loss product on the C₈H₇ PES, phenylacetylene), 103 (the initial adduct C₈H₇-1 and its isomers plus the ¹³C isotopologue of phenylacetylene), 128 (naphthalene), and 129 (C₁₀H₉ isomers plus the ¹³C isotopologue of naphthalene) were observed. The modeled signals show excellent agreement with the measurements. As the temperature increases from 600 to 700 K, the m/z = 102 signal increases significantly relative to the m/z = 128 signal, which indicates that product branching toward the H-loss pathway increases at higher temperature. When the pressure is increased from 10 to 50 Torr, more efficient collisional stabilization led to a higher rate of C₈H₇ adduct formation and a reduced yield of phenylacetylene. At long time scales (up to 8 ms after photolysis) at 700 K, C₈H₇ radicals were expected to be completely consumed by bimolecular reactions due to the excess C₂H₂ concentrations in the reactor. However, the signal at m/z = 103 corresponding to C₈H₇ remained constant and nonzero after 4.0 ms. The model revealed that part of this signal came from ¹³C-substituted phenylacetylene, but more importantly, part of the signal was also attributed to a resonantly stabilized aromatic radical C₈H₇-4 which was newly calculated in this work.

At high C₂H₂ concentrations, C₂H₂ addition reactions become dominant. By measuring the signal ratio of m/z = 128 to m/z = 102, the relative product branching of the formally direct H-loss reaction pathway forming phenylacetylene on the C₈H₇ PES was directly determined. The measured phenylacetylene branching fractions are 7.9% at 600 K and 10 Torr, 2.8% at 600 K and 50 Torr, 15.9% at 700 K and 10 Torr, and 6.2% at 700 K and 50 Torr; the corresponding branching fractions predicted by the model agreed with the measurements within the experimental uncertainty under all the T and P conditions studied. The time-dependent products and product branching measured with MBMS successfully validate the kinetic model constructed in this work, and the rate parameters are recommended for future modeling of the phenyl + C₂H₂ HACA reaction in mechanisms of PAH formation.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

We gratefully acknowledge SABIC for financial support for this research, and Prof. Alexander Mebel for valuable discussions.

References

1. H. Richter and J. B. Howard, Prog. Energy Combust. Sci., 2000, 26, 565–608.
2. M. R. Kholghy, A. Veshkini and M. J. Thomson, Carbon, 2016, 100, 508–536.
3. M. Frenklach and H. Wang, Symp. (Int.) Combust., 1991, 23, 1559–1566.
4. M. Frenklach, Combust. Flame, 2019, 201, 148–159.
5. A. M. Valencia-López, F. Bustamante, A. Loukou, B. Stelzner, D. Trimis, M. Frenklach and N. A. Slavinskaya, Combust. Flame, 2019, 207, 265–280.
6. T.-C. Chu, Z. J. Buras, P. Oßwald, M. Liu, M. J. Goldman and W. H. Green, Phys. Chem. Chem. Phys., 2019, 21, 813–832.
7. V. Chernov, M. J. Thomson, S. B. Dworkin, N. A. Slavinskaya and U. Riedel, Combust. Flame, 2014, 161, 592–601.
8. K. Narayanaswamy, G. Blanquart and H. Pitsch, Combust. Flame, 2010, 157, 1879–1898.
9. M. Frenklach, D. W. Clary, W. C. Gardiner and S. E. Stein, Symp. (Int.) Combust., 1985, 20, 887–901.
10. H. Richter, O. A. Mazyar, R. Sumathi, W. H. Green, J. B. Howard and J. W. Bozzelli, J. Phys. Chem. A, 2001, 105, 1561–1573.
11. I. V. Tokmakov and M. C. Lin, J. Am. Chem. Soc., 2003, 125, 11397–11408.
12. V. V. Kislov, N. I. Islamova, A. M. Kolker, S. H. Lin and A. M. Mebel, J. Chem. Theory Comput., 2005, 1, 908–924.
13. Y. Georgievskii and S. J. Klippenstein, J. Phys. Chem. A, 2003, 107, 9776–9781.
14. A. M. Mebel, Y. Georgievskii, A. W. Jasper and S. J. Klippenstein, Proc. Combust. Inst., 2017, 36, 919–926.
15. A. Fahr and S. E. Stein, Symp. (Int.) Combust., 1989, 22, 1023–1029.
16. T. Yu, M. C. Lin and C. F. Melius, Int. J. Chem. Kinet., 1994, 26, 1095–1104.
17. T. Yu and M. C. Lin, J. Am. Chem. Soc., 1993, 115, 4371–4372.
18. D. S. N. Parker, R. I. Kaiser, T. P. Troy and M. Ahmed, Angew. Chem., Int. Ed., 2014, 53, 7740–7744.
19. T. Yang, T. P. Troy, B. Xu, O. Kostko, M. Ahmed, A. M. Mebel and R. I. Kaiser, Angew. Chem., Int. Ed., 2016, 55, 14983–14987.
20. J. E. Middaugh, Z. J. Buras, M. Matrat, T.-C. Chu, Y.-S. Kim, I. M. Alecu, A. K. Vasiliou, C. F. Goldsmith and W. H. Green, Rev. Sci. Instrum., 2018, 89, 074102.
21. Z. J. Buras, T. C. Chu, A. Jamal, N. W. Yee, J. E. Middaugh and W. H. Green, Phys. Chem. Chem. Phys., 2018, 20, 13191–13214.
22. J. E. Middaugh, PhD thesis, Massachusetts Institute of Technology, 2014.
23. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, G. A. Petersson, H. Nakatsuji, X. Li, M. Caricato, A. V. Marenich, J. Bloino, B. G. Janesko, R. Gomperts, B. Mennucci, H. P. Hratchian, J. V. Ortiz, A. F. Izmaylov, J. L. Sonnenberg, D. Williams-Young, F. Ding, F. Lipparini, F. Egidi, J. Goings, B. Peng, A. Petrone, T. Henderson, D. Ranasinghe, V. G. Zakrzewski, J. Gao, N. Rega, G. Zheng, W. Liang, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, K. Throssell, J. A. Montgomery Jr., J. E. Peralta, F. Ogliaro, M. J. Bearpark, J. J. Heyd, E. N. Brothers, K. N. Kudin, V. N. Staroverov, T. A. Keith, R. Kobayashi, J. Normand, K. Raghavachari, A. P. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, J. M. Millam, M. Klene, C. Adamo, R. Cammi, J. W. Ochterski, R. L. Martin, K. Morokuma, O. Farkas, J. B. Foresman and D. J. Fox, Gaussian 16 Rev. B.01, Gaussian Inc., Wallingford, CT, 2016.
24. H. J. Werner, P. J. Knowles, G. Knizia, F. R. Manby and M. Schütz, WIREs Comput. Mol. Sci., 2012, 2, 242–253.
25. P. J. Knowles, C. Hampel and H. J. Werner, J. Chem. Phys., 1993, 99, 5219–5227.
26. P. J. Knowles and H. J. Werner, Theor. Chim. Acta, 1992, 84, 95–103.
27. P. J. Knowles, J. S. Andrews, R. D. Amos, N. C. Handy and J. A. Pople, Chem. Phys. Lett., 1991, 186, 130–136.
28. R. D. Amos, J. S. Andrews, N. C. Handy and P. J. Knowles, Chem. Phys. Lett., 1991, 185, 256–264.
29. P. J. Knowles and N. C. Handy, Comput. Phys. Commun., 1989, 54, 75–83.
30. H. J. Werner and P. J. Knowles, J. Chem. Phys., 1988, 89, 5803–5814.
31. P. J. Knowles and H. J. Werner, Chem. Phys. Lett., 1988, 145, 514–522.
32. H. J. Werner and P. J. Knowles, J. Chem. Phys., 1985, 82, 5053.
33. P. J. Knowles and H. J. Werner, Chem. Phys. Lett., 1985, 115, 259–267.
34. P. J. Knowles and N. C. Handy, Chem. Phys. Lett., 1984, 111, 315–321.
35. J. W. Allen, C. F. Goldsmith and W. H. Green, Phys. Chem. Chem. Phys., 2012, 14, 1131–1155.
36. C. W. Gao, J. W. Allen, W. H. Green and R. H. West, Comput. Phys. Commun., 2016, 203, 212–225.
37. A. M. Mebel, Y. Georgievskii, A. W. Jasper and S. J. Klippenstein, Faraday Discuss., 2016, 195, 637–670.
38. A. W. Jasper, C. M. Oana and J. A. Miller, Proc. Combust. Inst., 2015, 35, 197–204.
39. Y. Georgievskii and S. J. Klippenstein, MESS.2016.3.23.2016.
40. Y. Georgievskii, J. A. Miller, M. P. Burke and S. J. Klippenstein, J. Phys. Chem. A, 2013, 117, 12146–12154.
41. H. Wang and M. Frenklach, Combust. Flame, 1994, 96, 163–170.
42. K. Han, A. Jamal, C. A. Grambow, Z. J. Buras and W. H. Green, Int. J. Chem. Kinet., 2018, 50, 294–303.
43. M. T. Baeza-Romero, M. A. Blitz, A. Goddard and P. W. Seakins, Int. J. Chem. Kinet., 2012, 44, 532–545.
44. T. Adam and R. Zimmermann, Anal. Bioanal. Chem., 2007, 389, 1941–1951.
45. Z. Zhou, M. Xie, Z. Wang and F. Qi, Rapid Commun. Mass Spectrom., 2009, 23, 3994–4002.
46. Y. Y. Li, J. Z. Yang and Z. J. Cheng, Photonionization Cross Section Database (Version2.0), http://flame.nsrl.ustc.edu.cn/database/.
47. Y. Li, L. Zhang, Z. Tian, T. Yuan, J. Wang, B. Yang and F. Qi, Energy Fuels, 2009, 23, 1473–1485.
48. H. Xu and S. T. Pratt, J. Phys. Chem. A, 2013, 117, 9331–9342.
49. Y. A. Dyakov, J. Chem. Phys., 2012, 137, 064314.
50. Chemical Workbench 4.1.18493, Kintech Lab, Moscow, Russia, 2016.
51. H. Wang and M. Frenklach, J. Phys. Chem., 1994, 98, 11465–11489.
52. J. D. Bittner and J. B. Howard, Symp. (Int.) Combust., 1981, 18, 1105–1116.
53. N. W. Moriarty, N. J. Brown and M. Frenklach, J. Phys. Chem. A, 1999, 103, 7127–7135.
54. M. Frenklach, N. W. Moriarty and N. J. Brown, Symp. (Int.) Combust., 1998, 27, 1655–1661.
55. B. M. Wong, D. M. Matheu and W. H. Green, J. Phys. Chem. A, 2003, 107, 6206–6211.
56. J. Zádor, C. A. Taatjes and R. X. Fernandes, Prog. Energy Combust. Sci., 2011, 37, 371–421.
57. A. M. Knepp, G. Meloni, L. E. Jusinski, C. A. Taatjes, C. Cavallotti and S. J. Klippenstein, Phys. Chem. Chem. Phys., 2007, 9, 4315–4331.
58. E. G. Estupiñán, J. D. Smith, A. Tezaki, S. J. Klippenstein and C. A. Taatjes, J. Phys. Chem. A, 2007, 111, 4015–4030.
59. J. D. DeSain, S. J. Klippenstein, J. A. Miller and C. A. Taatjes, J. Phys. Chem. A, 2003, 107, 4415–4427.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c9cp04554f

---