DOI:
10.1039/C7RA08435H
(Paper)
RSC Adv., 2017,
7, 48120-48126
Viscoelastic properties of supramolecular gemini-like surfactant solutions in the absence of inorganic salts†
Received
31st July 2017
, Accepted 23rd September 2017
First published on 13th October 2017
Abstract
Exploration of novel wormlike micelle systems is important for both the theory and applications of surfactant self-assembly. A new anionic surfactant, sodium 2-phenoxyhexadecanoate (sodium Ph-C16), was synthesized in this work. This surfactant can self-assemble in solution to form wormlike micelles with the help of a bolaform salt, ethane-1,2-bis(trimethyl ammonium bromide) (N-2-N bromide), by removing inorganic salts. The resultant aqueous system was investigated using rheological measurements, freeze-fractured-TEM and 2D NOESY 1H NMR experiments. A supramolecular gemini-like structure constructed from electrostatic interactions was a prerequisite for the wormlike micelle formation. The zero shear viscosity (η0) of the system had a very strong dependence on the surfactant concentration with a scaling exponent of 16.8 in the semi-dilute region, which is attributed to the extremely strong electrostatic attraction between the surface-active anions and bolaform cations. These results are beneficial for understanding anionic wormlike micelles in the absence of inorganic salts.
1. Introduction
Wormlike micelles are a type of supramolecular aggregate formed by surfactants under suitable conditions.1 These aggregates can have dimensions that are hundreds or even thousands of nanometers in size, which imparts remarkable viscoelasticity to aqueous solutions. As living polymers, wormlike micelles have wide applications as fracturing fluids in oil fields, drag reducing agents and shampoos. The most commonly reported wormlike micelles are those that form in cationic surfactant aqueous solutions in the presence of salts2–8 and in cationic–anionic9–11 surfactant systems. In these systems, a suitable molecular geometry can be obtained by screening the head group charge of the surfactant counter ions, which is beneficial for wormlike micelle formation.12–16
In recent years, investigations on inorganic salt-free surfactant systems in aqueous solutions have generated useful results on novel aggregate morphology and phase behavior.17–21 Because of the absence of inorganic salts, the ionic strength in the systems is quite low, resulting in unscreened aggregates and high osmotic pressures.22 Zemb et al. discovered aggregates of nanodisks and hollow icosahedra in catanionic salt-free systems.23–25 Hao et al. reported an onion phase in such systems.26 It is obvious that salt-free systems represent systems where electrostatic interactions occur under extreme conditions. Investigating stable wormlike micelles in inorganic salt-free systems can help further understanding of the effect of charge on wormlike micelle growth and evolution. However, the most common morphologies in inorganic salt-free systems are vesicles and liquid crystal phases. Wormlike micelles are only occasionally reported in these systems and usually only exist in a very narrow mixture ratio or concentration range.17,19,27–29
Gemini surfactants, which are composed of two alkyl chains, two head groups and a spacer, have an advantageous molecular geometry and can form wormlike micelles without the addition of salts.15,16,30 To avoid a tedious synthesis of new, structured gemini surfactants, a strategy has been developed to construct wormlike micelles using pseudo-gemini surfactants19,21,31–37 with gemini-like structures that are formed via intermolecular electrostatic interactions. Inspired by this strategy, a new anionic surfactant, sodium 2-phenoxyhexadecanoate (sodium Ph-C16), was synthesized in this work (Fig. 1) and used to construct supramolecular gemini surfactant structures with a bolaform electrolyte, ethane-1,2-bis(trimethyl ammonium bromide), abbreviated N-2-N bromide. In contrast to previously reported systems,19,21,31–36 the inorganic salts were removed in the present system. Wormlike micelle formation was observed over a wide range of concentrations in this inorganic salt-free system (di-Ph-C16/N-2-N), which provided insight into the properties of wormlike micelles in the absence of inorganic salts.
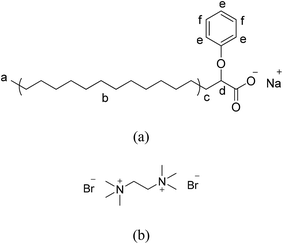 |
| | Fig. 1 The molecular structures of (a) sodium 2-phenoxyhexadecanoate, abbreviated sodium Ph-C16 and (b) ethane-1,2-bis(trimethyl ammonium bromide), abbreviated N-2-N bromide. | |
2. Materials and methods
2.1 Materials
All compounds used, including the palmitic acid, SOCl2, Br2, and phenol, were purchased from Aladdin Reagent Co., Ltd. The reagents and solvents used were analytical reagent grade. Sodium 2-phenoxyhexadecanoate and the bolaform salt, ethane-1,2-bis(trimethyl ammonium bromide), were synthesized in our lab. The detailed synthetic processes and NMR data can be found in the ESI.†
2.2 Sample preparation
The ethane-1,2-bis(trimethyl ammonium hydroxide) solution (N-2-N hydroxide) was prepared from an ethane-1,2-bis(trimethyl ammonium bromide) aqueous solution via ion exchange using a strong basic anion exchanger (Amberlite® IRA 402). To ensure the solution was acceptable for further use, it was tested with a nitric acid-acidified AgNO3 solution to ensure no precipitates appeared. The surfactant, sodium Ph-C16, was acidified with HCl to obtain its acid form. A certain amount of N-2-N hydroxide was neutralized with the Ph-C16 acid. After freeze-drying, the products were used to prepare a di-Ph-C16/N-2-N solution.
2.3 Rheology measurements
Rheological measurements were performed using a stress-controlled rheometer (Discovery DHR-2, TA instruments) with a cone-plate sensor. The cone was made of standard ETC steel with a diameter of 40 mm and a cone angle of 2°. The gap between the center of the cone and the plate was 48 μm. Each sample was allowed to equilibrate on the plate for 5 min before testing. A strain sweep was performed at a frequency of 2.82 rad s−1 before each test. A strain value was determined and used to ensure that the sample was in the linear viscoelastic region during the oscillatory measurements. The temperature was maintained at 25 °C throughout the experiments. All the solutions were prepared using Milli-Q water with a resistivity of 18.2 MΩ cm.
2.4 2D NOESY 1H NMR measurements
2D NOESY measurements was performed on a Bruker Avance III 400 spectrometer operating at 400 MHz. Deuterium oxide (99.9%) was purchased from Cambridge Isotope Laboratories and was used to prepare the di-Ph-C16/N-2-N solution in D2O. The center of the HDO signal (4.79 ppm) was used as the reference. The experiments were performed with a standard three-pulse sequence and a mixing time of 300 ms.
2.5 FF-TEM
The samples were prepared using freeze-fractured methods. A small amount of sample was placed on a 0.1 mm thick copper disk. The sample was quickly frozen by plunging it into liquid propane cooled by liquid nitrogen. The fracturing and replication were performed on a freeze-fracture apparatus (BalzersBAF400, Germany) at −160 °C. Carbon was deposited at an angle of 90°, and then, platinum was deposited at an angle of 45° to shadow the replicas. After removing the organic components, the resulting replicas were observed with a JEM-2100 electron microscope (200 kV).
3. Results and discussion
3.1 Steady state rheological measurements of the di-Ph-C16/N-2-N system
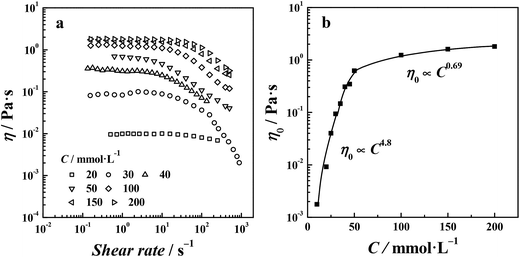
Steady shear viscosity measurements are widely used to probe the viscoelastic behavior of surfactant solutions. Fig. 2 shows the steady shear viscosity curves of the di-Ph-C16/N-2-N system at different Ph-C16 ion concentrations. As observed in Fig. 2, the viscosity was constant at low concentrations (C ≤ 40 mmol L−1) as the shear rate increased, and the solution exhibited a Newtonian behavior. At higher concentrations, the viscosity of the di-Ph-C16/N-2-N system decreased with the increasing shear rate. The shear-thinning behavior indicates the presence of wormlike micelles.38 The viscosity value that corresponds to the plateau in the steady shear viscosity curve at low shear rates is called the zero shear viscosity (η0). Over the range of the examined concentrations, the largest η0 was 16.2 Pa s at 200 mmol L−1. However, the sodium Ph-C16 solution remains liquid-like with an η0 value of 1.5 mPa s at the same concentration (ESI Fig. S2†). A similar system using the traditional hexadecanoate ion instead of Ph-C16 ion also gives an η0 value of 1.5 mPa s (ESI Fig. S3†). These comparisons reveal the peculiarity of di-Ph-C16/N-2-N system. Firstly, the di-cations with a short spacer cause the strong viscoelasticity in the di-Ph-C16/N-2-N system. Two anionic surfactant ions are drawn close by one N-2-N molecule via electrostatic attractions. A supramolecular gemini-like structure formed and led to the formation of wormlike micelles.39 Secondly, the phenyl group contained in the Ph-C16 ion enhances the molecular interactions and finely tuned the molecular geometry, also facilitating the wormlike micelle formation.
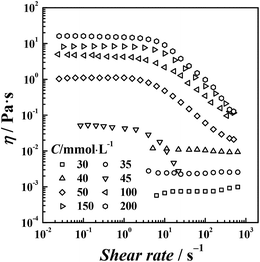 |
| | Fig. 2 Steady shear viscosity curves of di-Ph-C16/N-2-N at different Ph-C16 ion concentrations. | |
3.2 The scaling behavior of the di-Ph-C16/N-2-N system
In the wormlike micelle formation process, the spherical micelles that initially form gradually transform into rod-like micelles as the concentration increases. When the amount and length of the elongated aggregates reach a critical state, entanglements occur and impart the solution with viscoelasticity. The concentration at which the zero shear viscosity (η0) begins to show an obvious increase is called the overlap concentration.40 As shown in Fig. 3, that concentration is 35 mmol L−1 in this system. Then, the systems enter the semi-dilute regime, which is where η0 rapidly increases with the concentration. The dependence of η0 on the concentration, C, can be described by a power law: η0 ∝ Cα, where α is the exponent.41,42 The di-Ph-C16/N-2-N system has an exponent of 16.8, indicating the extremely strong concentration dependence of η0. This result is understandable because the di-Ph-C16/N-2-N system is an inorganic salt-free system43–46 with a low ionic strength. The surface charge of the wormlike micelles is large compared with that of the micelles in conventional surfactant systems. The electrostatic interactions contribute to the free energy of the end caps, which accelerates the growth of the wormlike micelles.47 When the Ph-C16 ion concentration reaches 50 mmol L−1 in the di-Ph-C16/N-2-N system, the variations in η0 as the concentration changes gradually level off, indicating the microstructure transition in the solution. This phenomenon is different from that in most wormlike micelle systems, where η0 goes through a maximum and then promptly decreases with the concentration because of micelle branching.48 The weak dependence of η0 on the concentration indicates that the average wormlike micelle length no longer obviously increases with the concentration. In addition, the scaling law gives an exponent of 1.96. This value is comparable with the value of 1.5 predicted using the polyelectrolyte solution theory49 and reflects the effect of the charge carried by the wormlike micelles on the rheological behavior of the solution. When wormlike micelles entangle, the repulsions between the charged segments in the wormlike micelles makes the fusion of the micelles with each other difficult. The production of joints, which are a key constituent of branched micelles, is more difficult than that in neutral wormlike micelles. The relaxation process of joints sliding along the contour of elongated micelles is also suppressed. Although micelle branching is still inevitable, a multi-connected network is a more suitable description of the microstructure in this region, and this has also been investigated in several systems.16,50 In the present system, this phenomenon is a direct result of the absence of inorganic salts.
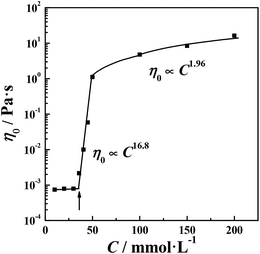 |
| | Fig. 3 The variation in the zero shear viscosity (η0) as a function of the concentration. | |
3.3 The dynamic rheological behavior of the di-Ph-C16/N-2-N system
Wormlike micelles participate in dynamic scission and recombination processes. The slowly increasing region of η0 in the di-Ph-C16/N-2-N system was further investigated via oscillatory shear measurements to obtain further information about the rheological properties. In most cases, the relaxation processes can be characterized by the Maxwell model with a single relaxation time,41,51 which is presented by the following equations:| | 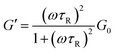 | (1) |
| | 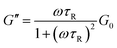 | (2) |
where G′ is the elastic modulus, G′′ is the viscous modulus, G0 is the plateau modulus, τR is the characteristic relaxation time, and ω is the oscillation angular frequency. As shown in Fig. 4(a), the elastic modulus (G′) is above the viscous modulus (G′′) at high frequencies and below G′′ at low frequencies. According to the Maxwell model, the frequency where two moduli cross each other is denoted ωc, and the reciprocal of this value can be used to estimate the relaxation time, τR. Fig. 4(b) shows the variation of G′′ with G′ (Cole–Cole plot) in the di-Ph-C16/N-2-N system, and the variation follows a perfect semicircle at low frequencies, indicating that the system behaves like a Maxwell fluid. The data deviation at higher frequencies implies the appearance of other relaxation mechanisms, such as Rouse-like motion.
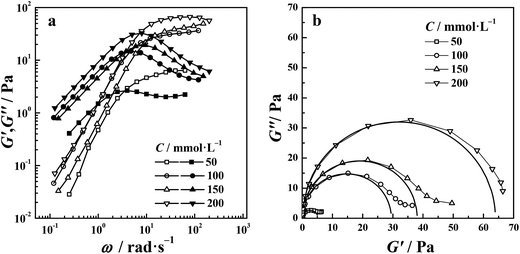 |
| | Fig. 4 (a) Dynamic rheological spectra and (b) the Cole–Cole plots of the di-Ph-C16/N-2-N system (filled and open symbols in (a) represent the elastic modulus (G′) and viscous modulus (G′′), respectively). | |
The relaxation time, τR, characterizes the timescale of a wormlike micelle experiencing relaxation processes and can be related to the average micelle length.52 As shown in Fig. 5, the τR slightly decreases with the increasing concentration, and this behavior is caused by the partial branching of the wormlike micelles, which was discussed above. The joints that are produced can slide along the micelle body and serve as a stress relaxation pathway. The diffusion of the wormlike micelles becomes faster, mimicking the behavior of micelles with shorter lengths. The elasticity of the aqueous solutions comes from the entanglement of the wormlike micelles. The plateau modulus, G0, is used to evaluate the number density of the entanglement points. The slow increase in G0 indicates the entanglements are enhanced with the increasing concentration. The above phenomenon can also been perceived as the switching in relaxation mode.53 The scaling law calculated an exponent of 1.37, which is close to the value of 1.5 predicted for polyelectrolyte solutions in the region of chain entanglement.49,54 This similarity in the scaling behavior reveals the charged nature of the investigated wormlike micelles.
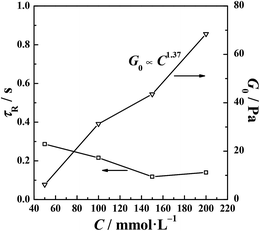 |
| | Fig. 5 The relaxation time, τR, and plateau modulus, G0, of the di-Ph-C16/N-2-N systems as a function of the Ph-C16 ion concentration. | |
3.4 FF-TEM images
The wormlike micelles formed in the di-Ph-C16/N-2-N system were further investigated using FF-TEM (Fig. 6). Massive threadlike micelles with a cross-sectional diameter of approximately 3 nm are densely entangled, preventing an estimation of the average micelle length of the wormlike micelles. Some connection joints can also be observed. The concentration of 50 mmol L−1 characterizes the transition from the quickly increasing region to the slowly increasing region of η0. The direct morphology image at this concentration showed that the wormlike micelles were entangled below this concentration.
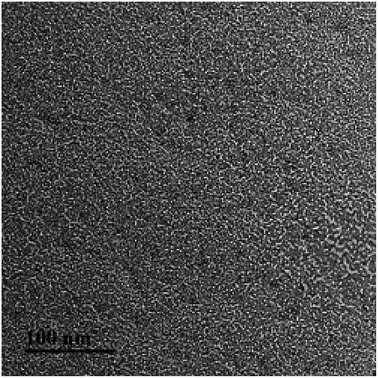 |
| | Fig. 6 The FF-TEM image of the di-Ph-C16/N-2-N system (concentration of the Ph-C16 ion: 50 mmol L−1). | |
3.5 2D NOESY experiments on the di-Ph-C16/N-2-N system
The formation of wormlike micelles by Ph-C16 ions requires the electrostatic attractions of counter di-cations. The interactions between groups are different in the presence or absence of N-2-N ions. The 2D NOESY experiments were performed to obtain a clear image of the supramolecular gemini-like surfactant constructed via electrostatic interactions. The cross-peak signals of protons in the 2D spectrum indicate the distance between protons within 5 Å,55,56 providing direct evidence of molecular interactions. The 2D NOESY spectra of the sodium Ph-C16 and di-Ph-C16/N-2-N system at the same Ph-C16 ion concentration (5 mmol L−1) are shown in Fig. 7. In the absence of N-2-N (Fig. 7(a)), the cross-peak signals are between the adjacent protons of the alkyl chains (b-H, c-H, and d-H, marked with a square). Because both the phenyl ring and the alkyl chain are hydrophobic in nature, the phenyl ring tends to bend toward the alkyl chain through the C–O–C bond. As a result, a weak signal from the interaction of d-H and e-H is observed (marked with a rhombus). While in the di-Ph-C16/N-2-N system (Fig. 7(b)), a new set of cross-peak signals arise, indicating the appearance of strong interactions between the phenyl ring and the alkyl chain (marked with an ellipse). This interaction obviously attributed to the effect of the N-2-N ions because these signals were not observed in the sodium Ph-C16 solution. A reasonable explanation for this observation is that one N-2-N ion bridges two Ph-C16 ions, drawing them close together and enhancing their hydrophobic interactions. The phenyl ring in one molecule has more opportunities to interact with the alkyl chain in the other molecule, resulting in the cross-peak signals of the protons in the 2D NOESY spectrum. The experiment also reveals that although the phenyl ring is located near the ionic head group, it resides in the hydrophobic region of the aggregate and serves as a hydrophobic driving force for wormlike micelle formation.
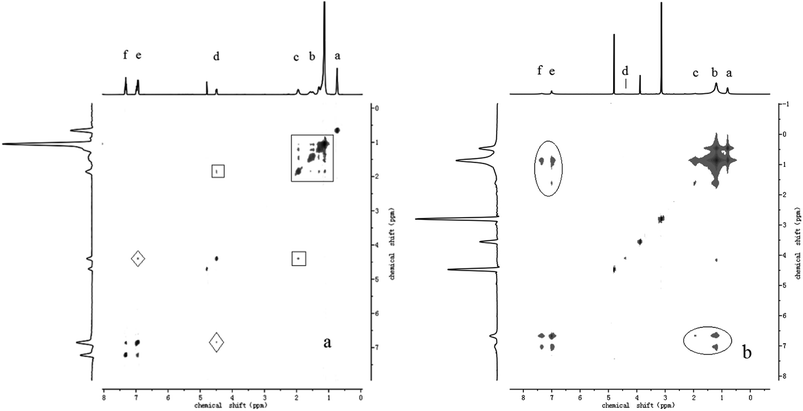 |
| | Fig. 7 The 2D NOESY spectra of the (a) sodium Ph-C16 and (b) di-Ph-C16/N-2-N systems (concentration of Ph-C16 ion: 5 mmol L−1). | |
3.6 The effect of inorganic salt on the behavior of the di-Ph-C16/N-2-N system
Salt additions usually enhance the viscoelasticity of wormlike micellar solutions. However, when equal molar NaBr (NaBr![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ph-C16 ion = 1
Ph-C16 ion = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1) was added into the di-Ph-C16/N-2-N system, the zero shear viscosity decreased at all investigated concentrations, as shown in Fig. 8(a). The scaling exponent was also far smaller than that of an inorganic salt-free system (Fig. 8(b)). This comparison shows the advantage of a system without an inorganic salt and reflects the importance of di-cations in promoting the formation of wormlike micelles. Although the addition of NaBr is beneficial for the growth of wormlike micelles via screening the ionic head group, the competitive binding of sodium ions with the Ph-C16 ions weakens the attractions between the N-2-N and Ph-C16 ions. The supramolecular, gemini-like Ph-C16 ion pairs, which are held together through the electrostatic attractions of N-2-N, are partially destroyed or weakened. As a result, the viscoelasticity of the system decreased.
1) was added into the di-Ph-C16/N-2-N system, the zero shear viscosity decreased at all investigated concentrations, as shown in Fig. 8(a). The scaling exponent was also far smaller than that of an inorganic salt-free system (Fig. 8(b)). This comparison shows the advantage of a system without an inorganic salt and reflects the importance of di-cations in promoting the formation of wormlike micelles. Although the addition of NaBr is beneficial for the growth of wormlike micelles via screening the ionic head group, the competitive binding of sodium ions with the Ph-C16 ions weakens the attractions between the N-2-N and Ph-C16 ions. The supramolecular, gemini-like Ph-C16 ion pairs, which are held together through the electrostatic attractions of N-2-N, are partially destroyed or weakened. As a result, the viscoelasticity of the system decreased.
 |
| | Fig. 8 (a) Steady shear viscosity curves of di-Ph-C16/N-2-N with equal molar NaBr; (b) the variation in η0 with the concentration of di-Ph-C16/N-2-N with equal molar NaBr. | |
4. Conclusions
The viscoelastic properties of an inorganic salt-free di-Ph-C16/N-2-N system were investigated. A bola-type cation and a newly synthesized anionic surfactant together generate a supramolecular gemini-like structure via electrostatic interactions, which facilitates the formation of wormlike micelles. Due to the absence of inorganic salts, an extremely high scaling exponent was calculated for the relationship of η0 and the concentration, which revealed the strong effect of charge on the growth and evolution of the aggregates. The investigated system provides an example of wormlike micelles formed by pseudo-gemini surfactants in the absence of inorganic salts over a wide concentration range, and these results expand the known conditions for wormlike micelle formation. In addition, the investigated system is an anionic surfactant-dominated wormlike micelle system, which is rarely reported.57–61 The exploration of new anionic surfactant systems is beneficial to understand anionic wormlike micelles and to determine and develop their advantages for applications.
Conflicts of interest
There are no conflicts to declare.
Acknowledgements
Support from the Foundation of Fujian Educational Committee (JK2015054, JA14325) and the Natural Science Foundation of Fujian Province (grant No. 2014J01052) are gratefully acknowledged.
References
- C. A. Dreiss, Soft Matter, 2007, 3, 956–970 RSC.
- W. J. Kim and S. M. Yang, J. Colloid Interface Sci., 2000, 232, 225–234 CrossRef CAS.
- T. Shikata, H. Hirata and T. Kotaka, Langmuir, 1987, 3, 1081–1086 CrossRef CAS.
- T. Shikata, H. Hirata and T. Kotaka, Langmuir, 1988, 4, 354–359 CrossRef CAS.
- T. Shikata, H. Hirata and T. Kotaka, Langmuir, 1989, 5, 398–405 CrossRef CAS.
- F. Kern, P. Lemarechal, S. J. Candau and M. E. Cates, Langmuir, 1992, 8, 437–440 CrossRef CAS.
- A. Khatory, F. Lequeux, F. Kern and S. J. Candau, Langmuir, 1993, 9, 1456–1464 CrossRef CAS.
- G. Porte, Y. Poggi, J. Appell and G. Maret, J. Phys. Chem., 1984, 88, 5713–5720 CrossRef CAS.
- H. Yin, Y. Lin and J. Huang, J. Colloid Interface Sci., 2009, 338, 177–183 CrossRef CAS.
- H. Fan, Y. Yan, Z. Li, Y. Xu, L. Jiang, L. Xu, B. Zhang and J. Huang, J. Colloid Interface Sci., 2010, 348, 491–497 CrossRef CAS.
- M. Bergstrom and J. S. Pedersen, Langmuir, 1999, 15, 2250–2253 CrossRef.
- V. M. Garamus, Langmuir, 2003, 19, 7214–7218 CrossRef CAS.
- V. Croce, T. Cosgrove, C. A. Dreiss, G. Maitland, T. Hughes and G. Karlsson, Langmuir, 2004, 20, 7984–7990 CrossRef CAS.
- D. P. Acharya, H. Kunieda, Y. Shiba and K. Aratani, J. Phys. Chem. B, 2004, 108, 1790–1797 CrossRef CAS.
- F. Kern, F. Lequeux, R. Zana and S. J. Candau, Langmuir, 1994, 10, 1714–1723 CrossRef CAS.
- B. Song, Y. Hu, Y. Song and J. Zhao, J. Colloid Interface Sci., 2010, 341, 94–100 CrossRef CAS.
- H. Li and J. Hao, J. Phys. Chem. B, 2008, 112, 10497–10508 CrossRef CAS.
- J. C. Hao and H. Hoffmann, Curr. Opin. Colloid Interface Sci., 2004, 9, 279–293 CrossRef CAS.
- Y. Li, H. Li, J. Chai, M. Chen, Q. Yang and J. Hao, J. Colloid Interface Sci., 2016, 472, 157–166 CrossRef CAS.
- X. Liu, J. Wang, Z. Cui, H. Yao, X. Ge, W. Chen and F. Sun, RSC Adv., 2017, 7, 26440–26445 RSC.
- H. Lu, Q. Shi, B. Wang and Z. Huang, Colloids Surf., A, 2016, 494, 74–80 CrossRef CAS.
- H. Li and J. Hao, J. Phys. Chem. B, 2009, 113, 2371–2377 CrossRef CAS.
- T. Zemb, M. Dubois, B. Deme and T. Gulik-Krzywicki, Science, 1999, 283, 816–819 CrossRef CAS.
- M. Dubois, B. Deme, T. Gulik-Krzywicki, J. C. Dedieu, C. Vautrin, S. Desert, E. Perez and T. Zemb, Nature, 2001, 411, 672–675 CrossRef CAS.
- M. Dubois, V. Lizunov, A. Meister, T. Gulik-Krzywicki, J. M. Verbavatz, E. Perez, J. Zimmerberg and T. Zemb, Proc. Natl. Acad. Sci. U. S. A., 2004, 101, 15082–15087 CrossRef CAS PubMed.
- A. X. Song, S. L. Dong, X. F. Jia, J. C. Hao, W. M. Liu and T. B. Liu, Angew. Chem., Int. Ed., 2005, 44, 4018–4021 CrossRef CAS PubMed.
- H. Li, S. A. Wieczorek, X. Xin, T. Kalwarczyk, N. Ziebacz, T. Szymborski, R. Holyst, J. Hao, E. Gorecka and D. Pociecha, Langmuir, 2010, 26, 34–40 CrossRef CAS.
- P. A. Hassan, B. S. Valaulikar, C. Manohar, F. Kern, L. Bourdieu and S. J. Candau, Langmuir, 1996, 12, 4350–4357 CrossRef CAS.
- K. Horbaschek, H. Hoffmann and C. Thunig, J. Colloid Interface Sci., 1998, 206, 439–456 CrossRef CAS.
- Q. Li, X. Wang, X. Yue and X. Chen, Soft Matter, 2013, 9, 9667–9674 RSC.
- Z. Chu and Y. Feng, Chem. Commun., 2010, 46, 9028–9030 RSC.
- J.-X. Zhao and D.-P. Yang, Acta Phys.-Chim. Sin., 2012, 28, 1218–1222 CAS.
- Y. Feng and Z. Chu, Soft Matter, 2015, 11, 4614–4620 RSC.
- Y. Zhang, P. An, A. Qin, J. Li, X. Lu and X. Liu, Colloid Polym. Sci., 2016, 294, 1743–1754 CrossRef CAS.
- X. Wang, Z. Zhang, Y. Cao and J. Hao, J. Colloid Interface Sci., 2017, 491, 64–71 CrossRef CAS.
- J. Wang, Z. Huang, C. Zheng and H. Lu, J. Dispersion Sci. Technol., 2017, 1–6, DOI:10.1080/01932691.2016.1277431.
- L. Zhu, Y. Tang and Y. Wang, J. Surfactants Deterg., 2016, 19, 237–247 CrossRef CAS.
- S. R. Raghavan and E. W. Kaler, Langmuir, 2001, 17, 300–306 CrossRef CAS.
- J. N. Israelachvili, D. J. Mitchell and B. W. Ninham, J. Chem. Soc., Faraday Trans. 2, 1976, 72, 1525–1568 RSC.
- R. Oda, J. Narayanan, P. A. Hassan, C. Manohar, R. A. Salkar, F. Kern and S. J. Candau, Langmuir, 1998, 14, 4364–4372 CrossRef CAS.
- M. E. Cates, Macromolecules, 1987, 20, 2289–2296 CrossRef CAS.
- M. S. Turner and M. E. Cates, J. Phys. II, 1992, 2, 503–519 CrossRef CAS.
- A. A. Ali and R. Makhloufi, Colloid Polym. Sci., 1999, 277, 270–275 CrossRef CAS.
- R. G. Shrestha, L. K. Shrestha and K. Aramaki, J. Colloid Interface Sci., 2007, 311, 276–284 CrossRef CAS PubMed.
- E. Cappelaere, R. Cressely and J. P. Decruppe, Colloids Surf., A, 1995, 104, 353–374 CrossRef CAS.
- S. J. Candau, A. Khatory, F. Lequeux and F. Kern, J. Phys. IV, 1993, 3, 197–209 CrossRef CAS.
- F. C. MacKintosh, S. A. Safran and P. A. Pincus, Europhys. Lett., 1990, 12, 697 CrossRef.
- S. A. Rogers, M. A. Calabrese and N. J. Wagner, Curr. Opin. Colloid Interface Sci., 2014, 19, 530–535 CrossRef CAS.
- M. Rubinstein, R. H. Colby and A. V. Dobrynin, Phys. Rev. Lett., 1994, 73, 2776–2779 CrossRef CAS.
- A. A. Ali and R. Makhloufi, Phys. Rev. E, 1997, 56, 4474–4478 CrossRef.
- R. Granek and M. E. Cates, J. Chem. Phys., 1992, 96, 4758–4767 CrossRef CAS.
- M. E. Cates, J. Phys., 1988, 49, 1593–1600 CrossRef.
- K. Morishima, S. Sugawara, T. Yoshimura and M. Shibayama, Langmuir, 2017, 33, 6084–6091 CrossRef CAS.
- A. V. Dobrynin, R. H. Colby and M. Rubinstein, Macromolecules, 1995, 28, 1859–1871 CrossRef CAS.
- Y. Fan, Y. Hou, J. Xiang, D. Yu, C. Wu, M. Tian, Y. Han and Y. Wang, Langmuir, 2011, 27, 10570–10579 CrossRef CAS.
- H. Kessler, M. Gehrke and C. Griesinger, Angew. Chem., Int. Ed., 1988, 27, 490–536 CrossRef.
- L. J. Magid, Z. Li and P. D. Butler, Langmuir, 2000, 16, 10028–10036 CrossRef CAS.
- P. A. Hassan, S. R. Raghavan and E. W. Kaler, Langmuir, 2002, 18, 2543–2548 CrossRef CAS.
- G. C. Kalur and S. R. Raghavan, J. Phys. Chem. B, 2005, 109, 8599–8604 CrossRef CAS.
- K. Nakamura and T. Shikata, Langmuir, 2006, 22, 9853–9859 CrossRef CAS.
- Y. Han, Y. Feng, H. Sun, Z. Li, Y. Han and H. Wang, J. Phys. Chem. B, 2011, 115, 6893–6902 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available: Synthesis details of sodium Ph-C16 and N-2-N bromide and showing the steady shear viscosity curve of sodium Ph-C16 at 200 mmol L−1. See DOI: 10.1039/c7ra08435h |
|
| This journal is © The Royal Society of Chemistry 2017 |
Click here to see how this site uses Cookies. View our privacy policy here.  Open Access Article
Open Access Article *a,
Donghong
You
a,
Shaoming
Ying
a,
Binglei
Song
b and
Jinnian
Tian
b
*a,
Donghong
You
a,
Shaoming
Ying
a,
Binglei
Song
b and
Jinnian
Tian
b

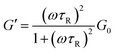
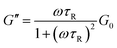



![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ph-C16 ion = 1
Ph-C16 ion = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1) was added into the di-Ph-C16/N-2-N system, the zero shear viscosity decreased at all investigated concentrations, as shown in Fig. 8(a). The scaling exponent was also far smaller than that of an inorganic salt-free system (Fig. 8(b)). This comparison shows the advantage of a system without an inorganic salt and reflects the importance of di-cations in promoting the formation of wormlike micelles. Although the addition of NaBr is beneficial for the growth of wormlike micelles via screening the ionic head group, the competitive binding of sodium ions with the Ph-C16 ions weakens the attractions between the N-2-N and Ph-C16 ions. The supramolecular, gemini-like Ph-C16 ion pairs, which are held together through the electrostatic attractions of N-2-N, are partially destroyed or weakened. As a result, the viscoelasticity of the system decreased.
1) was added into the di-Ph-C16/N-2-N system, the zero shear viscosity decreased at all investigated concentrations, as shown in Fig. 8(a). The scaling exponent was also far smaller than that of an inorganic salt-free system (Fig. 8(b)). This comparison shows the advantage of a system without an inorganic salt and reflects the importance of di-cations in promoting the formation of wormlike micelles. Although the addition of NaBr is beneficial for the growth of wormlike micelles via screening the ionic head group, the competitive binding of sodium ions with the Ph-C16 ions weakens the attractions between the N-2-N and Ph-C16 ions. The supramolecular, gemini-like Ph-C16 ion pairs, which are held together through the electrostatic attractions of N-2-N, are partially destroyed or weakened. As a result, the viscoelasticity of the system decreased.




