Magnetic evolution of spinel Mn1−xZnxCr2O4 single crystals
G. T. Linab,
X. Luo*a,
Q. L. Peia,
F. C. Chenab,
C. Yangab,
J. Y. Songab,
L. H. Yina,
W. H. Songa and
Y. P. Sun*acd
aKey Laboratory of Materials Physics, Institute of Solid State Physics, Chinese Academy of Sciences, Hefei, 230031, China. E-mail: xluo@issp.ac.cn
bUniversity of Science and Technology of China, Hefei, 230026, China
cHigh Magnetic Field Laboratory, Chinese Academy of Sciences, Hefei, 230031, China. E-mail: ypsun@issp.ac.cn
dCollaborative Innovation Center of Advanced Microstructures, Nanjing University, Nanjing, 210093, China
First published on 7th June 2016
Abstract
Mn1−xZnxCr2O4 (0 ≤ x ≤ 1) single crystals have been grown using the chemical vapor transport (CVT) method. The crystallographic, magnetic, and thermal transport properties of the single crystals were investigated by room-temperature X-ray diffraction, magnetization M(T) and specific heat CP(T) measurements. Mn1−xZnxCr2O4 crystals show a cubic structure, the lattice constant a decreases with the increasing content x of the doped Zn2+ ions and follows the Vegard law. Based on the magnetization and heat capacity measurements, the magnetic evolution of Mn1−xZnxCr2O4 crystals has been discussed. For 0 ≤ x ≤ 0.3, the magnetic ground state is the coexistence of the long-range ferrimagnetic order (LFIM) and the spiral ferrimagnetic one (SFIM), which is similar to that of the parent MnCr2O4. When x changes from 0.3 to 0.8, the SFIM is progressively suppressed and spin glass-like behavior is observed. When x is above 0.8, an antiferromagnetic (AFM) order presents. At the same time, the magnetic specific heat (Cmag.) was also investigated and the results are coincident with the magnetic measurements. The possible reasons based on the disorder effect and the reduced molecular field effect induced by the substitution of Mn2+ ions by nonmagnetic Zn2+ ones in Mn1−xZnxCr2O4 crystals have been discussed.
1 Introduction
The chalcogenide spinel compounds, named as ACr2X4 (A = 3d transition metals, Cd and Hg, X = O, S, Se), have attracted special interest in the past ten years because a variety of important physical effects have been found in these compounds, such as colossal magnetocapacitance (MC), multiferroicity, spin frustration and so on.1–9 Because of the strong coupling among charge, spin and lattice degrees of freedom, ACr2X4 presents not only an interesting phenomena but also complicated magnetic structures.Among ACr2X4 compounds, cubic spinel ACr2O4 oxides have special characters. The unfilled 3d3 shells of Cr3+ ions form isotropic S = 3/2 degree of freedom on a lattice of corner-sharing tetrahedron. When the tetrahedral A-site (A = Mg, Zn, Cd and Hg) is occupied by non-magnetic ions, the main magnetic interaction is the strong JCrCr antiferromagnetic (AFM) direct exchange between the nearest-neighbor ions.5–7 And these compounds show strongly geometrical frustration.4,8,10–14 On the other hand, when the tetrahedral A-site is occupied by a magnetic ion, such as A = Mn, Co, Fe, and Ni, it helps to overcome the frustration of the pyrochlore lattice by the JACr coupling between the A site and the Cr3+ S = 3/2 spins.5,15 For MnCr2O4, previous studies have calculated or evaluated from experimental data, JMnCr, JCrCr and JMnMn and obtained that JCrCr is larger than JMnCr or approximately equal to JMnCr.7,16,18,24 In this case, the system presents nearly degenerated ground states and it develops complex low temperature magnetic order. Among the spinel ACr2O4 compounds, MnCr2O4 and ZnCr2O4 are two typical ones. In MnCr2O4, the long-range ferrimagnetic (LFIM) temperature TC is observed around 41–51 K, which are dependent on the polycrystalline samples and single crystals. In addition, the sample exists a characteristic temperature TS. As T < TS, the long-range FIM and the short-range spiral FIM (SFIM) coexists. Between TC and TS, the long-range FIM with an easy axis parallel to the 〈110〉 direction occurs.7,9,17–19 Very recently, the multiferroicity has also been reported in MnCr2O4 below the TS.9 However, ZnCr2O4 shows strikingly different characters, such as strongly geometrical frustration (the frustration factor f ≈ 31) and high Curie–Weiss temperature θ = 390–400 K. The AFM with the spin-Jahn–Teller distortion, which favors a relief of the geometrical frustration, appears around TN = 12 K with the character of a first-order phase transition.8,20,21 From above reported works, it seems to mean that the molecular field of the A sites can be effectively tuned and has the important effect on the ground state of the spinel oxides. Because the emergent phenomena present in spinel MnCr2O4 and ZnCr2O4 compounds, the magnetic evaluation of Mn1−xZnxCr2O4 oxides are really deserved to be investigated. Although few work has been done on the Zn2+ ions doped MnCr2O4 compounds, the comprehensive study is still missing and the evolution of magnetic ground state is not very clear.22,23 In order to further understand magnetic evolution of the ground state, herein, we investigate the effect of non-magnetic Zn2+ ions doping at the magnetic A sites of Mn1−xZnxCr2O4 single crystals. The magnetic phase diagram of Mn1−xZnxCr2O4 single crystals is obtained. We also discussed the magnetic evolution based on the disorder effect and the reduced molecular field one induced by the substitution of Mn2+ ions by nonmagnetic Zn2+ ones in Mn1−xZnxCr2O4 crystals.
2 Experimental results
Mn1−xZnxCr2O4 single crystals were grown by the chemical vapor transport (CVT) method, with CrCl3 powders as the transport agent. Firstly, polycrystalline Mn1−xZnxCr2O4 were made by the solid state reaction. The stoichiometric amounts of Cr2O3 (99%, Alfa Aesar), ZnO (99.9%, Alfa Aesar) and MnO (99%, Alfa Aesar) powders were mixed in air and sintered at 1300 °C for 20 h for several times. Powder X-ray diffraction (XRD) at room temperature revealed a single-phase without any detected impurities. Secondly, polycrystalline Mn1−xZnxCr2O4 mixed with the CrCl3 powders were ground again and sealed into several quartz tubes. All were done in the Ar-filled glove-box. All sealed quartz tubes were put in a two-zone tube furnace. The hot side is about 1060 °C and the cold side is about 1015 °C, and dwelled for 10 days, then slowly cooled down to room temperature with a rate of 15 °C h−1. The crystals are octahedral with shining surfaces and the size is about 1.2 × 1.2 × 1.2 mm3. Heat capacity was measured using the Quantum Design physical properties measurement system (PPMS-9T) and magnetic properties were performed by the magnetic property measurement system (MPMS-XL5).3 Results and discussion
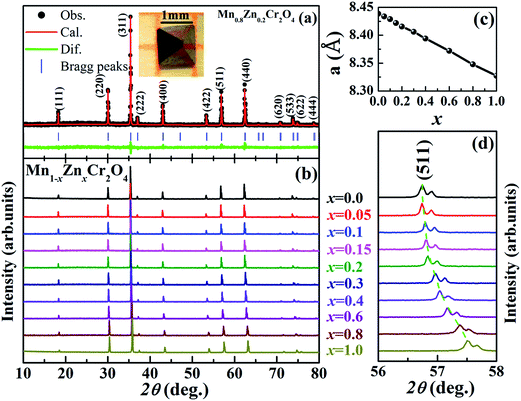
Fig. 1(a) shows the XRD pattern of Mn0.8Zn0.2Cr2O4 powder obtained by crushing the single crystals, which is selected as a typical sample. It also presents the structural Rietveld refinement profiles of the XRD data by the Highscore software. The refinements of the XRD data indicate that the crystals are single-phase since no extra peaks were observed. It is found that the crystals belong to the normal spinel structure and the space group is Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m (space group no. is 227). X-ray diffraction patterns of Mn1−xZnxCr2O4 powder are presented in Fig. 1(b). It also presents a single-phase for all samples. The crystal structure of these samples does not change with the Zn doping content x at room temperature, the lattice parameter a decreases from 0.844 nm for x = 0 to 0.833 nm for x = 1.0, which confirms that the partial Mn2+ sites are occupied by the Zn2+ ions. The reduced lattice parameter is related to the fact that the Zn2+ ion radius (0.06 nm) is smaller than that of Mn2+ one (0.066 nm). The lattice constant a for all samples follows the Vegard law. On the other hand, these Bragg peaks of XRD pattern move towards higher angles with increasing x, which is consistent with the refined lattice parameter decreases with increasing x. Fig. 1(d) shows the enlargements of XRD pattern near the peak (511), which is selected as a typical sample.
m (space group no. is 227). X-ray diffraction patterns of Mn1−xZnxCr2O4 powder are presented in Fig. 1(b). It also presents a single-phase for all samples. The crystal structure of these samples does not change with the Zn doping content x at room temperature, the lattice parameter a decreases from 0.844 nm for x = 0 to 0.833 nm for x = 1.0, which confirms that the partial Mn2+ sites are occupied by the Zn2+ ions. The reduced lattice parameter is related to the fact that the Zn2+ ion radius (0.06 nm) is smaller than that of Mn2+ one (0.066 nm). The lattice constant a for all samples follows the Vegard law. On the other hand, these Bragg peaks of XRD pattern move towards higher angles with increasing x, which is consistent with the refined lattice parameter decreases with increasing x. Fig. 1(d) shows the enlargements of XRD pattern near the peak (511), which is selected as a typical sample.
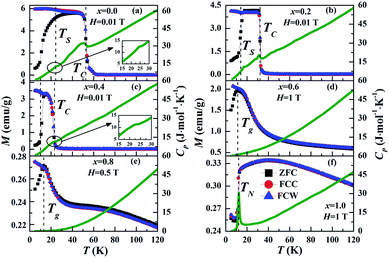
In order to investigate the macroscopic magnetic properties of Mn1−xZnxCr2O4 single crystals, we carried out the measurement of the magnetization M(T) as the function of temperature. Fig. 2(a)–(f) show the M(T) under the zero field-cooled (ZFC), field-cooled (FCC) and field-warming (FCW) modes with the applied magnetic field parallel to the 〈111〉 direction for Mn1−xZnxCr2O4 single crystals, respectively. Fig. 2(a) and (b) show a FIM behavior for x ≤ 0.2. A collapse of the cusp is observed for the compounds with higher x in Fig. 2(c), which indicates the magnetic ground state is changed by the Zn2+ ion doping, namely, the SFIM may be suppressed and the magnetic ground state changes into the spin glass state in the spinel oxides.9,24 For x ≥ 0.6, compared with the lower doped content x, the value of the magnetization M is much smaller and the ZFC and FCC curves are obviously irreversibility in x = 0.6 and 0.8 (as shown in Fig. 2(d) and (e)). It may mean that both AFM and FIM orders are perturbed, then destroyed and eventually the spin-glass-like ground state presents.24 In Fig. 2(f), accompanied by the sharp drop of magnetization M(T), the AFM order occurs at TN = 12 K, which is in agreement with the reported data. It is related to a structural phase transition from cubic Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m phase to the tetragonal I41/amd one at TN for ZnCr2O4.6,25 In order to further investigate the nature of the magnetic structure of Mn1−xZnxCr2O4 single crystals, the magnetic field dependence of the magnetization (M(H)) for all crystals at T = 5 K are shown in Fig. 3. Except for the parent MnCr2O4, it shows that the coercivity HC increases with the increasing x for x ≤ 0.6. The saturated magnetization MS is nearly decreasing with increasing content x.
m phase to the tetragonal I41/amd one at TN for ZnCr2O4.6,25 In order to further investigate the nature of the magnetic structure of Mn1−xZnxCr2O4 single crystals, the magnetic field dependence of the magnetization (M(H)) for all crystals at T = 5 K are shown in Fig. 3. Except for the parent MnCr2O4, it shows that the coercivity HC increases with the increasing x for x ≤ 0.6. The saturated magnetization MS is nearly decreasing with increasing content x.
Now we focus on the nature of Zn doped MnCr2O4 single crystals, we did the analysis on the temperature dependent inverse susceptibility χ−1(T). Firstly, we pay attention to the parent compound MnCr2O4, the FIM order TC and SFIM one TS are 52.7 K and 24.4 K obtained from the peak of the heat capacity Cp/T, respectively. From the mean-field theory, for a AFM system and FIM one, the temperature dependent inverse susceptibility above TC can be described by the Curie–Weiss law (eqn (1)) and the hyperbolic behavior characteristic of ferrimagnets (eqn (2)):7,26
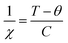 | (1) |
 | (2) |
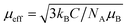 ) and magnetic fraction factor f (f = θ/TN) for increasing x. The tetragonal position occupied by the non-magnetic Zn2+ ions is responsible for the above results, which are consistent with the ones obtained from the above M(T) and M(H) measurements.
) and magnetic fraction factor f (f = θ/TN) for increasing x. The tetragonal position occupied by the non-magnetic Zn2+ ions is responsible for the above results, which are consistent with the ones obtained from the above M(T) and M(H) measurements.
 | ||
| Fig. 4 (a) The inverse susceptibility dependence of the temperature for Mn1−xZnxCr2O4 single crystals. The solid lines are the fitting results according to eqn (1) and (2); (b) the obtained effective moment μeff, Weiss temperature θ, the magnetic order temperature T (including TC and TN) and the frustration factor f dependence of the doping level x of the Zn2+ ions. | ||
To study the thermal property, we performed the detailed analysis on the temperature dependent specific heat CP/T of Mn1−xZnxCr2O4 single crystals, as shown in Fig. 5(a). For ZnCr2O4, which yields a sharp specific heat anomaly with the character of the first-phase transition at TN = 12.2 K. With the decrease of x, this heat capacity anomaly is clearly suppressed. For 0.6 ≤ x ≤ 0.8, the heat capacity peak disappears and the specific heat presents a monotonous smooth curve, which shows a spin glass behavior.25 It agrees well with the magnetic results. When x ≤ 0.4, just one specific heat peak is observed, as present in the inset of Fig. 5(a), which is related to the LFIM. When x continues to decrease, two specific heat peaks are observed for 0 ≤ x ≤ 0.2, and which are corresponding to the LFIM and SFIM orders. As x ≤ 0.2, it is obvious that the magnetic structure of Mn1−xZnxCr2O4 samples in the FIM regions is in good agreement with MnCr2O4. In addition, as shown in Fig. 5(b), the low-temperature magnetic specific heat presents linear variation in Mn1−xZnxCr2O4. The linear variation suggests a constant density of states of the low-temperature magnetic excitations, which is claimed to be a common feature of spin glasses.27,28 For 0.4 ≤ x ≤ 0.8, a broad magnetic specific heat anomaly is observed for spin glasses, indicating that short-range-order contributions extend up to very high temperatures. Fig. 5(b) shows the temperature dependence of the magnetic heat capacity Cmag. for Mn1−xZnxCr2O4. Since all the samples show insulating behavior, we can ignore the electronic contribution to the heat capacity, the Cmag. can be calculated by the following equations:26
 | (3) |
| Cmag.(T) = Cp(T) − nCVDebye(T) | (4) |
 | (5) |
The T dependence of Smag. is shown in Fig. 6. Smag. is monotonously decreasing with the increasing content x except for ZnCr2O4. In addition, the change of Debye temperature behaves as firstly decreasing and then increasing with the increasing content x except for ZnCr2O4, the x = 0.4 sample has a minimum value ΘD = 270 K. The abnormality of ZnCr2O4 may be attributed to the character of the first-phase transition at TN = 12.2 K.
 | ||
| Fig. 6 The temperature dependence of the magnetic entropy Smag. of Mn1−xZnxCr2O4 single crystals. The inset shows the doping content x dependence of the Debye temperature ΘD. | ||
Based on our obtained results, we summarize the magnetic phase diagram of Mn1−xZnxCr2O4 single crystals as plotted in Fig. 7. Now, let us try to understand the magnetic evolution in Mn1−xZnxCr2O4 single crystals. As we know, the substitution of Mn2+ (S = 5/2) by Zn2+ (S = 0) ions usually can induce following effects: the shrinkage of lattice, the disorder of A sites and the decreased content of magnetic Mn2+ ions. As shown in Fig. 2, for x ≤ 0.4, it can be seen that all the samples undergo a transition from PM to FIM. The ZFC and FCC curves are obviously irreversibility in 0.01 T, which can be attributed to magnetic frustration or a transition into a spin-glass phase.29–31 We note that the magnetization in 0.01 T decreases and the transition temperature TC is lower than that of MnCr2O4 with the increase of x. Meanwhile, the FIM order presents an easy axis along the [1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0] direction in MnCr2O4 below TC, which is identical with the axial component of Mn2+ ion.7,9,18,32 Although the shrinkage of lattice could enhance the exchange interaction between Cr–Mn ions via oxygen 2p orbits, the decreased content of Mn ions is the major factor, which is responsible for the above observed phenomenon.33 In MnCr2O4, the axial component of the tetrahedral A (Mn2+) site is antiparallel to that of the octahedral B (Cr3+) site.
0] direction in MnCr2O4 below TC, which is identical with the axial component of Mn2+ ion.7,9,18,32 Although the shrinkage of lattice could enhance the exchange interaction between Cr–Mn ions via oxygen 2p orbits, the decreased content of Mn ions is the major factor, which is responsible for the above observed phenomenon.33 In MnCr2O4, the axial component of the tetrahedral A (Mn2+) site is antiparallel to that of the octahedral B (Cr3+) site.
According to the previous experimental evaluations and theoretical calculations, JCrCr is larger than JMnCr or approximately equal to JMnCr.7,16,18,24 However, as it is shown in Fig. 3, the abnormality of MnCr2O4 may be attributed to the cation distribution of A and B sites.29 This is because Mn2+ site is slightly occupied by Cr3+ ions, which leads to the enhanced coercivity and the reduced magnetization.34 With the substitution of magnetic ions in A site by the Zn2+ (has preferentially A-site occupancy), the cation distribution of A and B sites will be consistent with that of normal spinel structure. For x ≥ 0.05, the replacement of Mn2+ with non-magnetic Zn2+ leads to the weakening of the A–O–B super exchange interaction. This would further disturb the magnetic couplings and lead to a reduction of the magnetization. The substitution of Zn2+ ion for Mn2+ or Co2+ ions has relatively similar physical properties. For example, Brent C Melot et al.24 has reported magnetic phase evolution in the spinel compounds Co1−xZnxCr2O4, which is similar to our results obtained in Mn1−xZnxCr2O4. At the same time, the structure of Co1−xZnxCr2O4 at low temperature (T = 5 K) is still modeled by the cubic space group Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m for x ≤ 0.9.25 For x ≥ 0.6, the magnetic coupling interactions become more complicated. The Mn–O–Cr superexchange interaction partially breaks the spin degeneracy of the ground state and the coupling between the spin and lattice degrees of freedom becomes weaker than that of ZnCr2O4 in Mn1−xZnxCr2O4. It is reasonable that the Mn–O–Cr superexchange interaction could disrupt the coherency of Cr–Cr exchange coupling paths, and then inhibit the spin-Jahn–Teller distortion in Mn0.2Zn0.8Cr2O4 and Mn0.6Zn0.4Cr2O4.25 However, more detail structural experiments at low temperature, including magnetic structure determined using neutron scattering method, are needed in future.
m for x ≤ 0.9.25 For x ≥ 0.6, the magnetic coupling interactions become more complicated. The Mn–O–Cr superexchange interaction partially breaks the spin degeneracy of the ground state and the coupling between the spin and lattice degrees of freedom becomes weaker than that of ZnCr2O4 in Mn1−xZnxCr2O4. It is reasonable that the Mn–O–Cr superexchange interaction could disrupt the coherency of Cr–Cr exchange coupling paths, and then inhibit the spin-Jahn–Teller distortion in Mn0.2Zn0.8Cr2O4 and Mn0.6Zn0.4Cr2O4.25 However, more detail structural experiments at low temperature, including magnetic structure determined using neutron scattering method, are needed in future.
4 Conclusion
From the room-temperature X-ray diffraction, magnetization M(T) and specific heat CP(T) measurements, the crystallographic, magnetic, and thermal transport properties of the Mn1−xZnxCr2O4 single crystals were obtained. The lattice constant a decreases with the increasing content x of the doped Zn2+ ions and follows the Vegard law. The magnetic evolution of Mn1−xZnxCr2O4 crystals based on the magnetization and specific heat measurements has been discussed. For 0 ≤ x ≤ 0.3, the magnetic ground state is the coexistence of LFIM and SFIM, which is similar to that of the parent MnCr2O4. x changes from 0.3 to 0.8, the SFIM is progressively suppressed and spin glass-like behavior is observed. While x is above 0.8, an AFM order presents. The magnetic specific heat (Cmag.) was also calculated and the results are coincident with the magnetic measurements. The possible reasons based on the disorder effect and the reduced molecular field effect induced by the substitution of Mn2+ ions by nonmagnetic Zn2+ ones in Mn1−xZnxCr2O4 crystals have been discussed.Acknowledgements
This work was supported by the Joint Funds of the National Natural Science Foundation of China and the Chinese Academy of Sciences' Large-Scale Scientific Facility under contracts U1432139, U1532152, the National Nature Science Foundation of China under contracts 51171177, 11404339, and the Nature Science Foundation of Anhui Province under contract 1508085ME103.References
- J. Hemberger, P. Lunkenheimer, R. Fichtl, H.-A. Krug von Nidda, V. Tsurkan and A. Loidl, Nature, 2005, 434, 364–367 CrossRef CAS PubMed.
- Y. J. Choi, J. Okamoto, D. J. Huang, K. S. Chao, H. J. Lin, C. T. Chen, M. van Veenendaal, T. A. Kaplan and S.-W. Cheong, Phys. Rev. Lett., 2009, 102, 067601 CrossRef CAS PubMed.
- K. E. Sickafus, J. M. Wills and N. W. Grimes, J. Am. Ceram. Soc., 1999, 82(12), 3279–3292 CrossRef CAS.
- C. Lacroix, P. Mendels and F. Mila, Introduction to Frustrated Magnetism, in Solid-State Sciences, Springer Series, 2011 Search PubMed.
- S. Bordács, D. Varjas, I. Kézsmárki, G. Mihály, L. Baldassarre, A. Abouelsayed, C. A. Kuntscher, K. Ohgushi and Y. Tokura, Phys. Rev. Lett., 2009, 103, 077205 CrossRef PubMed.
- M. C. Kemei, P. T. Barton, S. L. Moffitt, M. W. Gaultois, J. A. Kurzman, R. Seshadri, M. R. Suchomel and Y.-I. Kim, J. Phys.: Condens. Matter, 2013, 25, 326001 CrossRef PubMed.
- E. Winkler, S. Blanco Canosa, F. Rivadulla, M. A. López-Quintela, J. Rivas, A. Caneiro, M. T. Causa and M. Tovar, Phys. Rev. B: Condens. Matter Mater. Phys., 2009, 80, 104418 CrossRef.
- S.-H. Lee, C. Broholm, W. Ratcliff, G. Gasparovic, Q. Huang, T. H. Kim and S.-W. Cheong, Nature, 2002, 418, 856–858 CrossRef CAS PubMed.
- K. Dey, S. Majumdar and S. Giri, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 90, 184424 CrossRef.
- M. T. Rovers, P. P. Kyriakou, H. A. Dabkowska and G. M. Luke, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 66, 174434 CrossRef.
- I. Kagomiya, H. Sawa, K. Siratori, K. Kohn, M. Toki, Y. Hata and E. Kita, Ferroelectrics, 2002, 268, 327–332 CrossRef.
- J.-H. Chung, M. Matsuda, S.-H. Lee, K. Kakurai, H. Ueda, T. J. Sato, H. Takagi, K. P. Hong and S. Park, Phys. Rev. Lett., 2005, 95, 247204 CrossRef PubMed.
- R. Valdes Aguilar, A. B. Sushkov, Y. J. Choi, S.-W. Cheong and H. D. Drew, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 092412 CrossRef.
- H. Ueda, H. Mitamura, T. Goto and Y. Ueda, Phys. Rev. B: Condens. Matter Mater. Phys., 2006, 73, 094415 CrossRef.
- D. Tobia, J. Milano, M. Teresa Causa and E. L. Winkler, J. Phys.: Condens. Matter, 2015, 27, 016003 CrossRef PubMed.
- S. Blanco-Canosa, F. Rivadulla, V. Pardo, D. Baldomir, J.-S. Zhou, M. Garca-Hernndez, M. A. Lpez-Quintela, J. Rivas and J. B. Goodenough, Phys. Rev. Lett., 2007, 99, 187201 CrossRef CAS PubMed.
- J. M. Hastings and L. M. Corliss, Phys. Rev., 1962, 126, 556–565 CrossRef CAS.
- K. Tomiyasu, J. Fukunaga and H. Suzuki, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 70, 214434 CrossRef.
- D. Y. Yoon, S. Lee, Y. S. Oh and K. H. Kim, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 094448 CrossRef.
- A. Miyata, H. Ueda, Y. Ueda, H. Sawabe and S. Takeyama, Phys. Rev. Lett., 2011, 107, 207203 CrossRef PubMed.
- V. N. Glazkov, A. M. Farutin, V. Tsurkan, H.-A. Krug von Nidda and A. Loidl, Phys. Rev. B: Condens. Matter Mater. Phys., 2009, 79, 024431 CrossRef.
- K. Le Dang, M. C. Mery and P. Veillet, J. Magn. Magn. Mater., 1984, 43, 161–165 CrossRef CAS.
- F. Leccabue, B. E. Watts, D. Fiorani, A. M. Testa, J. Alvarez, V. Sagredo and G. Bocelli, J. Mater. Sci., 1993, 28, 3945–3950 CrossRef CAS.
- B. C. Melot, J. E. Drewes, R. Seshadri, E. M. Stoudenmire and A. P. Ramirez, J. Phys.: Condens. Matter, 2009, 21, 216007 CrossRef PubMed.
- M. C. Kemei, S. L. Moffitt and L. E. Darago, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 89, 174410 CrossRef.
- C. Kittle, Introduction to Solid State Physics, Wiley, New York, 4th edn, 1966 Search PubMed.
- N. P. Raju, E. Gmelin and R. K. Kremer, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 46, 5405–5411 CrossRef CAS.
- D. Meschede, F. Steglich, W. Felsch, H. Maletta and W. Zinn, Phys. Rev. Lett., 1980, 44, 102–105 CrossRef CAS.
- D. Y. Yoon, S. Lee, Y. S. Oh and K. H. Kim, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 094448 CrossRef.
- K. Binder and A. P. Young, Rev. Mod. Phys., 1986, 58, 801–976 CrossRef CAS.
- Z. Qu, Z. Yang, S. Tan and Y. Zhang, Phys. Rev. B: Condens. Matter Mater. Phys., 2005, 71, 184430 CrossRef.
- R. Plumier, J. Appl. Phys., 1968, 39, 635–636 CrossRef CAS.
- X. Luo, Y. P. Sun, W. J. Lu, X. B. Zhu, Z. R. Yang and W. H. Song, Appl. Phys. Lett., 2010, 96, 062506 CrossRef.
- A. Franco Jr and F. C. e. Silva, J. Appl. Phys., 2013, 113, 17B513 CrossRef.
| This journal is © The Royal Society of Chemistry 2016 |