Solar-to-hydrogen efficiency: shining light on photoelectrochemical device performance

Abstract

Illumination characteristics from artificial sources strongly influence the experimental performance of solar water-splitting devices, with the highest impact on tandem structures designed for optimum conversion efficiency. We highlight quantitative and qualitative flaws of common characterization techniques, discuss their impact on research results and strategy, and demonstrate approaches toward advanced measurement accuracy.

---

Broader context

Renewable energy research is of utmost importance for developing a long-term, sustainable energy economy. In addition to limited global reserves, conventional energy supply largely based on fossil fuels produces excessive domestic greenhouse gas emissions responsible for global warming, which is associated with unforeseeable risks and excessive societal costs. Solar and wind power have shown technological and economic feasibility, as well as generation potential of relevant scale, to supply the growing global energy demand. Hydrogen as a chemical energy carrier is essential for energy storage, ammonia production, and as transportation fuel. Direct photoelectrochemical hydrogen production aims to provide a clean, cost-effective, and locally produced solar fuel. Solar-to-hydrogen conversion efficiency is essential in evaluating and comparing research results, and it largely determines the prospect for successfully introducing commercial solar water-splitting systems. Current measurement techniques do not follow well-defined standards, and the common methods potentially impact research results and their implications. Our research demonstrates underestimated critical influence factors and experimental strategies for greatly improved accuracy.

Direct photoelectrochemical (PEC) conversion of sunlight into hydrogen and oxygen¹ represents a prominent concept for scalable and cost-effective solar energy storage and fuel supply. Despite decades of intense research, commercial solar water-splitting devices are not yet available. Critical technological and economical drawbacks include limited device lifetime in contact with PEC electrolytes² and fundamentally insufficient conversion efficiency³ of typical absorber materials. Research approaches on inherently stable absorber materials,⁴ protective coatings,⁵ and surface modifications⁶ promise improved PEC device durability. Theoretical device prediction^7–9 and experimental results^2,4,10 both clearly indicate the necessity of tandem devices for the practical realization of economically viable³ PEC performance.

The increased structural complexity (integration of two absorbers and their electronic interconnect) goes along with challenges in device design, preparation, and characterization. We have recently analysed the impact of device illumination through aqueous electrolytes⁹ and found PEC-specific system design guidelines based on an implied relationship between allowable electrolyte films and overvoltage losses. The conversion of established III–V tandem photovoltaic (PV) devices¹¹ for PEC applications² provides us with an ideal testbed for experimental verification, but the measured solar-to-hydrogen (STH) conversion rates put commonly used PEC characterization techniques increasingly in question.

In this analysis, we discuss potential systematic errors in typical, laboratory-scale PEC measurements, their impact on reported performance figures, and the implications on research strategy. Our focus is tandem devices that have the prospect for superior STH efficiency⁹ and greater complexity. The proposed approaches address PEC characterization accuracy by considering: (i) calibration and adjustment of the illumination light-source; (ii) confirmation of the consistency of results by incident photon-to-current efficiency (IPCE); and (iii) definition and confinement of the active area of the device.

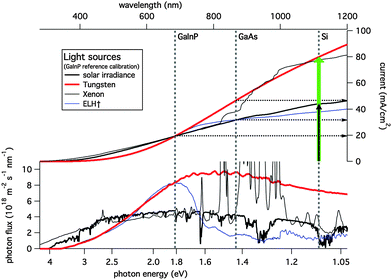
The definitions of both STH efficiency¹² and the solar spectrum¹³ are generally accepted standards; however, practical laboratory light sources (including solar simulators) deviate significantly in their spectral distribution from solar irradiance, and they require intensity calibration. Fig. 1 displays optical emission spectra of typical illumination sources used for PEC characterization (acquired with a StellarNet spectrometer) compared to solar irradiance (global, air mass 1.5, ASTM G173-3). In analogy to a classical pyranometer (or thermopile) calibration, intensities are set to equivalent power within the relevant wavelength regime (280–1800 nm), which comprises more than 95% of terrestrial solar power (1 kW m⁻²). Note the use of photon flux units (rather than power) for direct proportionality to current generation. Spectral integration then yields the theoretical current limit (for unity light absorption and charge-carrier extraction) associated with each absorber bandgap—an important measure for sub-cell current matching in tandem device design and for overall advances in STH conversion efficiency. Despite providing solar-equivalent power, laboratory white-light sources deviate from solar flux and introduce error in absorber current limits for most bandgaps.

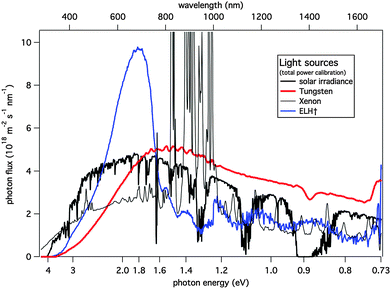Fig. 1 Spectral distribution of flux for AM 1.5 global irradiance compared to typical PEC laboratory white-light sources (adjusted to provide solar equivalent illumination power).

Advanced laboratory light-source calibration approaches often rely on calibrated reference PV devices instead of a spectral power measurement. Adjusting the light-source intensity to achieve the calibration current with the reference solar cell placed at measurement position removes systematic deviation of the current limit—when, and only when, testing absorbers with equal bandgap. Fig. 2 exemplifies the approach using a GaInP reference solar cell. At its bandgap energy of 1.81 eV, all light sources produce a solar-equivalent current-density limit of 19.4 mA cm⁻², but the spectral distribution of flux still strongly depends on the source type. The calibrated emission flux of the tungsten lamp actually only equals AM 1.5 global solar irradiance around 2.2 eV; the source systematically lacks emission in the ultraviolet (UV) range, but is compensated by an adequate excess of intensity in the visible range. In contrast, the xenon source produces a significant surplus in UV emission as well as excessive characteristic emission lines in the near infrared (NIR) region, particularly in the range of 800–1000 nm (1.2–1.6 eV).

Fig. 2 Current limitation vs. absorber bandgap for AM 1.5 global illumination as well as for various laboratory white-light sources (top) based on reference-cell calibration exemplified for an externally calibrated GaInP reference solar cell.

In general, the light-source calibration implies substitution of photon count between different emission wavelengths. Results only remain valid in the case where photon absorption and charge-carrier extraction probabilities are independent of the excitation energy. Both the reference cell and the tested material must comply with this idealized absorber condition (IAC), which is essentially equivalent with an experimental finding of constant IPCE above the respective bandgaps. Explicit IAC violations include advanced absorber concepts (multi-exciton generation, upconversion), molecular materials (dye sensitization, organic materials), and light management (photonic coupling, absorber thinning). In general, non-abrupt absorption edges affect virtually any semiconductor to some extent (in particular, Si and other indirect transition materials).

Mitigation strategies clearly benefit from detailed knowledge of the illumination spectrum and involve IPCE characterization. In principle, any white-light source can be adjusted to produce a solar-equivalent flux for any conventional single-junction absorber with any calibrated reference cell. For classical semiconductor absorbers (with sufficient electronic properties), the term “optically thick” refers to layer thicknesses exceeding the absorption length. It implies sufficient IAC compliance and enables straightforward light-source calibration with arbitrary sample and reference bandgaps, as demonstrated in Fig. 2, where the current-density limit for Si is 44.1 mA cm⁻² (thin black arrow), but the tungsten light source calibrated with a GaInP reference will provide 80.0 mA cm⁻² for its 1.12 eV bandgap (thick green arrow). Hence, the source could also be adjusted for GaInP characterization with a Si reference when adjusting the light level according to the ratio of currents (to 1.814 times the solar-equivalent calibration current). In contrast, neglect of the spectral mismatch may cause a tremendous under-illumination as well. In case of a tungsten source set to the calibration current of Si reference, GaInP samples would receive a light level of 10.7 mA cm⁻² – only 55% of the 1 Sun value for the AM 1.5 global spectrum. In principle, similar considerations also apply to power-derived calibration approaches (Fig. 1) practically carried out with pyranometers or thermopiles: Neglect of the spectral mismatch may induce substantial deviations of the light level (e.g. just 10.3 mA cm⁻² or 53% from tungsten source on GaInP); knowledge of the illumination spectrum enables dedicated compensation. The technique only provides indirect access to the solar current generation potential (thermal power vs. light level), therefore stable calibrated reference cells provide more accuracy. The complexity of laboratory light-source calibration increases significantly for tandem absorber measurements. The common series-connection device architecture benefits water-splitting operation by basically adding sub-cell voltages, but limits the total current to the minimum among the sub-cells.

We report on an improved upright epitaxial III–V tandem PEC structure grown by metalorganic vapor-phase epitaxy¹¹ (Fig. 3(a)) that closely matches the three main features of the classical GaInP/GaAs design:² (i) an optically thick, epitaxial, 4 μm p-type GaInP top absorber (Ga_0.51In_0.49P composition, 1.81 eV bandgap energy) forming the hydrogen-evolving PEC junction with the electrolyte at its surface; (ii) a buried GaAs bottom junction on a single-crystalline GaAs(100) substrate as joint growth template providing a PV bias to drive the water-splitting reaction without external voltage supply; and (iii) a tunnel junction for electrical series-connection of both sub-devices in a tandem configuration. Structural advances include aluminium incorporation in the tunnel junction, window layer, and back-surface field for better performance and lower parasitic absorption, as well as a heterojunction GaAs bottom cell and a sputtered PtRu co-catalyst.

Fig. 3 (a) Improved upright epitaxial GaInP/GaAs tandem PEC device structure and (b) current density vs. voltage characteristic of a 0.085 cm² sample under tungsten white-light illumination adjusted with GaInP reference.

Fig. 3(b) demonstrates traditional laboratory-based STH conversion-efficiency characterization. In a two-electrode configuration measured vs. IrO₂ counter electrode, we observe a direct water-splitting operation driven by a tungsten white-light source (with 3′′ water filter) set at a 1 Sun intensity employing a calibrated GaInP reference solar cell. At short-circuit condition (referring to the absence of an external bias potential), we observe a current density of 17.7 mA cm⁻² (on an 0.085 cm² sample), indicating a STH conversion efficiency of 21.8%.

In comparison, dotted horizontal arrows in Fig. 2 mark the expected GaInP (19.4 mA cm⁻²) and GaAs (31.7 mA cm⁻²) light levels for AM 1.5 global illumination. The optically thick top absorber (Fig. 3, inset) provides sufficient absorption length to filter all sunlight above its bandgap energy. In principle, the residual bottom-cell illumination light level of 12.3 mA cm⁻² should then constrain the series-connected device performance as the current-limiting junction. Although the historic result² still appears compatible (82%) with that fundamental limitation, our current data indicated a clear violation (144%).

Critical evaluation of the illumination light-source used (Fig. 2) quickly reveals a significant systematic error in our experimental configuration, causing a drastic overestimation of STH conversion efficiency: the spectral emission shape of the tungsten source causes a vast relative over-illumination of the GaAs bottom junction, associated with an effective bottom sub-cell light level of 27.3 mA cm⁻² (instead of 12.3 mA cm⁻² for AM 1.5 global; both values subsequent to GaInP filter) when using a GaInP reference cell for intensity adjustment at 1 Sun level. In effect, the much lower light level of the GaInP top absorber (19.4 mA cm⁻²) then imposes the current limitation of the tandem device for the laboratory measurement—in contrast to the situation in actual sunlight.‡ Note that our tandem result (Fig. 3) still requires 91% quantum conversion of the incident light in the GaInP top junction.

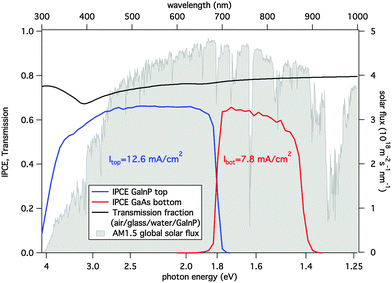
In contrast, the actual IPCE spectra of our PEC device in Fig. 4 show a very different situation. We applied appropriate bias illumination to saturate the other respective junction, enabling us to independently measure the quantum conversion of each absorber material at PEC short-circuit conditions. Note that no external bias potential was required as the tandem structure is capable of direct water-splitting operation. In Fig. 4, experimental GaInP (red) and GaAs (blue) external quantum efficiencies (EQE) are confined to maximum values of about 66%. In principle, the modelled transmission of the air/glass/electrolyte/semiconductor interface system (black line; by a simple Fresnel normal-incidence approach) restricts the maximum achievable EQE values to about 70–80% for the most relevant photon energy regime between 1.4 and 2.8 eV.

Fig. 4 Independent IPCE characterization of GaInP top (blue) and GaAs bottom (red) absorbers of our PEC tandem device acquired with appropriate bias illumination; modelled transmission through air/glass/electrolyte/semiconductor interface system (dark grey); and AM 1.5 global solar flux (light grey area).

The result confirms three important insights: (i) reflection losses of about 25% are a major constraint of the classical semiconductor/electrolyte PEC junction approach; (ii) high internal quantum efficiency (IQE) of up to 90% documents the excellent material quality of our device and epitaxial III–V absorbers in general; (iii) we measured PEC performance far beyond credible limits. The integration of experimental EQE over AM 1.5 global irradiance predicts realistic sub-device current limits of only about 12.6 and 7.8 mA cm⁻², respectively, for the GaInP top and GaAs bottom absorbers. The lower bottom-junction current constrains the expected tandem device to split water at no more than 9.6% STH conversion of sunlight.§ Incorrect illumination reversed the limiting junctions in our laboratory characterization (Fig. 3). Accordingly, a current density of up to 12.6 mA cm⁻² appears credible (Fig. 4), whereas we actually measured 17.7 mA cm⁻² (Fig. 3), already indicating further systematic error.

In general, these results demonstrate the critical importance of IPCE as an independent method to check the consistency of PEC results—in our case, indicating an excessive device efficiency overestimation by standard laboratory-based analysis, as shown in Fig. 3. A major share of the observed discrepancy apparently is caused by inadequate, not solar-equivalent, device illumination. The strong spectral mismatch of typical white-light sources with AM 1.5 global solar irradiance greatly limits opportunities for tandem PEC device characterization under artificial, but sunlight-equivalent, illumination. Single-source configurations may prove to be insufficient: even when IAC compliance of both absorbers is provided, two illumination intensity levels (E_hν ≥ E^top_bg as well as E^top_bg > E_hν ≥ E^bottom_bg) need to be matched to solar irradiance independently. A given light source (our tungsten lamp) fixes the ratio of light levels (1 : 2.2 for our GaInP/GaAs tandem). Any calibration will arbitrarily scale this ratio (e.g. to 0.55 : 1.22 by a Si reference) and device performance (Fig. 3(b); actual impact of light concentration is more complex). Besides illumination intensity, the operation point of the individual sub-devices also fundamentally differs from actual sunlight, with complex impact on the shape of the current density vs. voltage characteristic. Independent of the calibration, a single, spectrally mismatched source can never provide an accurate indication for the solar water-splitting performance of a tandem PEC device. Advanced design concepts, such as sub-cell current matching by top-absorber thinning,¹⁴ represent intentional IAC violations and further complicate the situation.

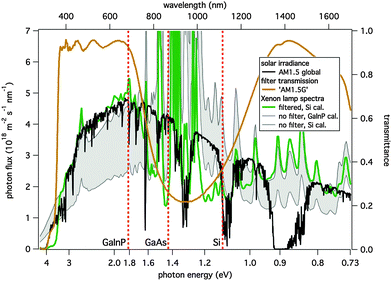
Even for simpler single-junction configurations, PEC characterization is particularly susceptible to spectral mismatch issues: The high voltage requirement for unassisted water-splitting particularly focuses high-bandgap materials and, accordingly, the high photon-energy onset of the solar spectrum—despite the minor contribution of the UV region to the total solar current-generation potential. The fundamental deviation of white-light-source emission shapes (Figs. 1 and 2) accentuates the mismatch, with only little chance for suppression through intensity calibration. For instance, xenon-emission-based illumination systems fundamentally over-supply the UV range, whereas tungsten sources fall short in that region. Popular solar simulators consist of xenon arc lamps with specific optical filters for spectral modification. Fig. 5 displays the transmission spectrum of the widespread Oriel AM 1.5G filter (yellow), featuring a steep UV cut-off around 3.6 eV, a broad attenuation feature in the near IR (between 1.0 and 1.6 eV), and more or less constant transmission (close to unity) anywhere else. Its application to the native xenon lamp spectrum (grey, various calibrations) creates a somewhat closer match (green) to the AM 1.5 global solar irradiance standard (black): still, flux distributions only agree well in the visible range, but both UV cut-off and near IR attenuation induce a certain balance of photon surpluses and deficits within either region. Among a pyranometer, Si and GaInP references, intensity calibrations deviate only marginally (by a few %). Also the light levels for individual absorbers (e.g. 102.6% for GaInP or 98.6% for GaAs, both based on Si reference) appear fairly reasonable. In contrast, the light-source configuration fixes incorrect light-level ratios for tandem devices, such as 1 : 0.895 for our exemplary GaInP/GaAs structure. Intensity calibration may only decide whether to over/undersupply the top/bottom absorber by this factor, but the overall performance of tandem absorbers critically depends on current matching between the sub-devices. Hence, both absolute STH efficiency figures and qualitative insights for advanced tandem device development may be flawed significantly.

Fig. 5 Transmission spectrum of an Oriel AM 1.5G optical filter (yellow) as well as filtered (green) and unfiltered (grey) xenon arc lamp spectra (various calibrations) in comparison to AM 1.5 global solar irradiance.

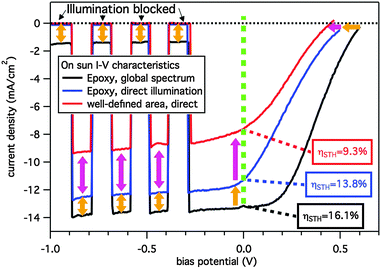
Attempting to minimize spectral-mismatch error, we performed outdoor PEC characterization under sunlight illumination. Of course, both the intensity and spectral composition of the terrestrial sunlight spectrum depend strongly on factors such as season, angle of incidence, air pollution, and altitude. All results shown in Fig. 6 were measured in Golden, CO, USA, in the direct vicinity of the NREL Solar Radiation Research Laboratory, where precise data on actual solar irradiance are continuously recorded and published.¹⁵ We normalized all data following the established PV convention of assuming 1 kW m⁻² intensity as a 1 Sun illumination level regardless of the applied spectrum.¹³ The spectral mismatch between the applied sunlight and the solar irradiance standards was mitigated by appropriate timing (to ensure nearly AM 1.5 global conditions). It still remains a potential source of error, but of negligible magnitude at the present accuracy level of PEC STH efficiency determination.

Fig. 6 Water-splitting characteristics measured with actual sunlight (outdoors) for standard epoxy PEC tandem cathodes vs. IrO anodes with normalized global (black) and direct (blue) illumination in comparison to proper area definition (red); current density at zero bias (green dashed line) is considered as indication of STH conversion efficiency η_STH.

In a first attempt, we found unbiased water-splitting operation driven only by sunlight to occur beyond 13 mA cm⁻² current density and 16% STH conversion (Fig. 6, black line) for the structure shown in Fig. 3(a). The values fell below the initial laboratory characterization result (Fig. 3(b)), but still greatly exceeded those expected based on IPCE (Fig. 4), and confirmed our suspicion of additional factors contributing to STH overestimation beyond an inadequate choice of illumination source. Significant current levels while blocking the direct light path (Fig. 6, black line) confirmed indirect device illumination that is part of the global solar spectrum, but the effect could be artificially enhanced by the glassware instrumentation surrounding the PEC sample. We avoided indirect light paths by using a dark compartment around the PEC cell, where the device is exclusively illuminated through a collimating tube¹⁶ designed to restrict the incident sunlight to the direct and circumsolar (5° field of view) portion. In that configuration, current density above 11 mA cm⁻² (Fig. 6, blue line) indicates almost 14% STH conversion. Note that normalization to the 1 kW m⁻² 1 Sun convention¹³ enables direct comparison between all data shown in Fig. 6, despite the change from global to direct irradiance.

Active-device-area definition and confinement remained the last area of possible experimental deficiency to explain the residual overestimation of PEC performance. Epoxy-encased electrode manufacturing is common throughout the scientific community for simplicity, flexibility, and corrosion resistance. Downsides may include: sample-to-sample variation of device area, unexpected under-etching or interaction with the electrolyte, and optical impact of light reflection and/or transmission. In an effort to exclude epoxy from impacting our results, we also used an alternative, compression-type PEC cell design, where an inert O-ring precisely defines the active area (of 0.185 cm²); we shielded the rest of the sample area with Al foil to avoid unintentional illumination. The result (Fig. 6, red line) might be perceived as a huge drop in PEC performance to about 7.6 mA cm⁻² in current density or 9.3% in STH conversion—values remarkably consistent with the current limitation estimated by IPCE (Fig. 4).

Pragmatically, only that final number truly represents STH energy-conversion efficiency,¶ whereas we significantly overrated the device performance in all earlier measurements due to uncontrolled systematic errors. Note that the IPCE-derived current only estimates an upper limit of device performance because it is measured at a much lower current density and without the appropriate load of maintaining the water-splitting reaction. Discrepancies only appear negligible for materials with excellent carrier extraction and operation in a light-limited current regime.

In total, typical—but inappropriate—PEC testing techniques led to a major inflation of the estimated STH performance—in our case, to more than double (17.7 vs. 7.6 mA cm⁻²) the correct value measured with advanced techniques inspired by multijunction PV characterization, but novel to the PEC community. Causes of the overrating lie in both spectral mismatch of the illumination source and insufficient active-area definition by epoxy-encased electrodes. To provide a better understanding of the latter effect, we measured the spectral transmission properties of our exemplary Loctite HySol 9462 epoxy (Fig. 7) commonly used for laboratory-scale PEC electrode assembly.

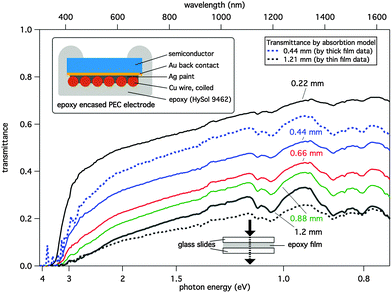Fig. 7 Spectral transmission through Loctite HySol 9462 epoxy films confined between glass slides, as well as schematic diagram (inset) of epoxy-encased PEC electrode manufacturing concept.

The inset in Fig. 7 illustrates the assembly concept: Rectangular semiconductor absorber chips usually receive an external electrical back-contact by Ag paint and Cu wire before the entire device, except the central area of the front surface, is coated by the chemically inert epoxy to prevent unintentional contact with the reactive electrolyte during operation. Epoxy layer thicknesses in the order of 1 mm shall ensure proper illumination-area definition because thinnest layers (<0.1 mm) appear optically semi-transparent. The optical transmission spectra of defined epoxy films confined between two glass slides (Fig. 7) demonstrate significant penetration even through thicker layers. In a simple absorbance model (Fig. 7, dashed lines), transmission of the air/glass/epoxy/glass/air layer stacks should only depend on reflection of interfaces (constant) and absorption in the epoxy (exponential decay). Calculations do not reproduce the data, but indicate sub-exponential behaviour consistent with significant translucence of the epoxy, probably based on scattering of incident light.

The consequence is an imprecise area definition for PEC devices, where the epoxy only defines the chemically active surface area, but also provides for partial illumination of the covered area (Fig. 7, inset). The contribution to device performance remains largely unpredictable because epoxy thickness and the fraction of covered area vary, and also, the lateral collection of charge carriers plays a significant role.|| Both effects supposedly contribute most for small, research-scale samples. We used an active area of about 0.085 cm² for epoxy electrodes represented in this study. A brief statistical survey over the range of 0.02–0.06 cm² samples (∼20 pieces) from the same growth run did not show an evident correlation between active area and observed light-limited photocurrent. Other epoxy types may provide more desirable light-transmission properties, but complete opacity to the full solar-irradiance spectrum remains to be confirmed.

Conclusions

Our analyses demonstrate the crucial importance of valid and reliable performance characterization and reporting for the scientific and economic progress of PEC solar fuel generation. Tandem device configurations enable superior STH conversion, but also show particular susceptibility to systematic spectral mismatch and device-area definition errors. We focused our consideration on an advanced version of the classical GaInP/GaAs design² and measured beyond 20% STH efficiency using a tungsten white-light source, a calibrated GaInP PV reference cell, and epoxy-encased photocathodes. In contrast, integrating the experimental IPCE over the AM 1.5 solar irradiance showed that less than 10% STH conversion appeared conceivable. We finally resolved the discrepancy by actual sunlight illumination (outdoors, direct, normalized) and epoxy-free area definition. The valid result of 9.3% STH efficiency most probably matches or exceeds the realistic achievement of the historic record.

We propose applying the following standards for future PEC performance reporting: (i) traceable disclosure of the illumination-source configuration (lamp, filters, optics, PEC configuration) and/or its measured spectral distribution; (ii) thorough device-area definition (including confinement of the illumination area and avoidance of indirect light paths); (iii) complementary IPCE confirmation of the solar-generation potential; and (iv) proper consideration of faradaic efficiency. In the long term, only standardized and validated PEC testing and STH efficiency determination techniques will provide a credible, objective base for scientific progress and technological deployment of solar water-splitting devices for solar fuel generation. The formation of acknowledged reference laboratories analogous to the PV community should be considered.

In terms of laboratory-based measurement technology, a smart optical assembly of multiple light sources and filters as well as sophisticated correction schemes¹⁷ may mitigate issues of spectral mismatch toward more realistic characterization of arbitrary PEC tandem devices. On a practical level, the efforts may shift from reproduction of the sunlight spectrum to device-specific illumination equivalents derived from IPCE analyses. For instance, a UV-deficient white-light source well matched in the visible range—such as a tungsten lamp—could be well suited for solar-equivalent bottom-cell illumination. In a second step, the spectral range of the top absorber could be independently supplemented by an adjustable line source, such as an LED, with appropriate photon energy.

Valid PEC characterization also provides crucial insights and guidance for developing tandem devices. The IPCE analysis shows a practical maximum of about 10% STH efficiency for the classical upright epitaxial GaInP/GaAs tandem PEC design. An internal quantum conversion of ∼90% leaves little room for advances in material quality. Additionally, the current design is incompatible with antireflective coatings that could reduce reflective loss during illumination. In contrast to the initial study,² our results show that the GaAs absorber strongly limits the device under AM 1.5 illumination. Appropriate current redistribution from top to bottom junction by light-management techniques such as top-absorber thinning¹⁴ or radiative coupling¹⁸ may improve the performance to about 12% actual STH conversion. Further advances require bandgap combinations⁹ inaccessible by classical III–V semiconductors at the lattice constant of GaAs substrates.

Acknowledgements

The authors thank Alan Kibbler for operating the epitaxy system. H. D. appreciates financial support by an EU Marie Curie fellowship (IOF no. 300971). J. Y. acknowledges support by a National Science Foundation Graduate Research Fellowship (Grant No. DGE1144083). This work was supported by the U.S. Department of Energy (DOE) under Contract No. DE-AC36-08GO28308 with the National Renewable Energy Laboratory.

Notes and references

1. A. Fujishima and K. Honda, Nature, 1972, 238, 37–38.
2. O. Khaselev and J. A. Turner, Science, 1998, 280, 425–427.
3. B. A. Pinaud, J. D. Benck, L. C. Seitz, A. J. Forman, Z. B. Chen, T. G. Deutsch, B. D. James, K. N. Baum, G. N. Baum, S. Ardo, H. L. Wang, E. Miller and T. F. Jaramillo, Energy Environ. Sci., 2013, 6, 1983–2002.
4. F. F. Abdi, L. H. Han, A. H. M. Smets, M. Zeman, B. Dam and R. van de Krol, Nat. Commun., 2013, 4, 2195.
5. S. Hu, M. R. Shaner, J. A. Beardslee, M. Lichterman, B. S. Brunschwig and N. S. Lewis, Science, 2014, 344, 1005–1009.
6. J. Mukherjee, S. Peczonczyk and S. Maldonado, Langmuir, 2010, 26, 10890–10896.
7. M. C. Hanna and A. J. Nozik, J. Appl. Phys., 2006, 100, 074510.
8. J. R. Bolton, S. J. Strickler and J. S. Connolly, Nature, 1985, 316, 495–500.
9. H. Döscher, J. F. Geisz, T. G. Deutsch and J. A. Turner, Energy Environ. Sci., 2014, 7, 2951–2956.
10. S. Y. Reece, J. A. Hamel, K. Sung, T. D. Jarvi, A. J. Esswein, J. J. H. Pijpers and D. G. Nocera, Science, 2011, 334, 645–648.
11. K. A. Bertness, S. R. Kurtz, D. J. Friedman, A. E. Kibbler, C. Kramer and J. M. Olson, Appl. Phys. Lett., 1994, 65, 989–991.
12. Z. B. Chen, T. F. Jaramillo, T. G. Deutsch, A. Kleiman-Shwarsctein, A. J. Forman, N. Gaillard, R. Garland, K. Takanabe, C. Heske, M. Sunkara, E. W. McFarland, K. Domen, E. L. Miller, J. A. Turner and H. N. Dinh, J. Mater. Res., 2010, 25, 3–16.
13. ASTM, in G 173–03, ASTM International, West Conshohocken, 2003.
14. S. R. Kurtz, P. Faine and J. M. Olson, J. Appl. Phys., 1990, 68, 1890–1895.
15. NREL-SRRL, http://www.nrel.gov/midc/srrl_bms/.
16. T. W. Cannon, Sol. Cells, 1986, 18, 233–241.
17. C. R. Osterwald, Sol. Cells, 1986, 18, 269–279.
18. M. A. Steiner and J. F. Geisz, Appl. Phys. Lett., 2012, 100, 251106.

---

Footnotes

† These authors contributed equally.

‡ In principle, the light-level ratio of an ELH source (tungsten halogen lamp with dichroic reflector) as used in ref. 2 appears much better (Fig. 2), but the spectral distribution strongly deviates from solar irradiance, and we cannot exclude significant calibration error affecting the historic measurements.

§ Conceivably, the GaAs bottom-junction IPCE in Fig. 3 can only be similar or superior to that of ref. 2. Today, we benefit from better epitaxial growth (defect density) and structural design (lower parasitic absorption), while the GaInP top filter is identical; without further error analysis, the actual historic STH efficiency must have been below 10%; current densities in the range of 6–8 mA cm⁻² (per Sun under concentrator operation) appear to be about the realistic achievement.

¶ Unity faradaic efficiency provided, i.e., absence of sacrificial reactions, to be confirmed by consistent gas chromatic product analysis.

|| Based on higher light transmission and better charge-carrier transport, the limiting GaAs bottom junction might benefit more than proportionally in epoxy-encased electrode configurations.

---