Investigation of plasma electrolytic oxidation on valve metals by means of molecular spectroscopy – a review
Stevan Stojadinović*a,
Rastko Vasilića and
Miljenko Perićb
aUniversity of Belgrade, Faculty of Physics, Studentski trg 12–16, 11000 Belgrade, Serbia. E-mail: sstevan@ff.bg.ac.rs; Fax: +381-11-3282619; Tel: +381-11-7158161
bUniversity of Belgrade, Faculty of Physical Chemistry, Studentski trg 12–16, 11000 Belgrade, Serbia
First published on 21st May 2014
Abstract
A review of results of molecular spectroscopic investigations during plasma electrolytic oxidation of valve metals is presented. Particular attention is paid to three spectral systems, B1Σ+ → X1Σ+ of MgO, and B2Σ+ → X2Σ+, and C2Π–X2Σ+ of AlO. It was shown that a reliable assignment of the observed spectral features can only be carried out by critical comparison with the data obtained from high-resolution spectroscopy, and by using the results of quantum mechanical structure calculations. Assuming the existence of partial local thermal equilibrium, we used our spectroscopic results to determine the plasma temperature. Although limited in quality, the obtained spectra are very rich, they cover large wavelength regions, and are used to obtain information about physical and chemical processes that take place in the course of plasma electrolytic oxidation of light metals and their alloys.
1. Introduction
In the present study, we review the results of investigations on molecular emission spectra recorded during plasma electrolytic oxidation of magnesium and aluminum. The goal of these investigations was to get information about the processes that take place in these systems, with the aim to become able to monitor them. Here we focus our attention on three spectral systems, B1Σ+ → X1Σ+ of MgO and B2Σ+ → X2Σ+ and C2Π–X2Σ+ of AlO. Random appearance of spectral sources (microdischarge plasmas), poor resolution, low intensities, and unfavorable signal/noise ratios, caused great difficulties in assignment of molecular bands. The assignment was carried out by comparison with the data obtained from high-resolution spectroscopy, but also by using the results of quantum mechanical structure calculations. Assuming the existence of partial local thermal equilibrium, we used our spectroscopic results to determine the plasma temperature.The paper is organized as follows: after the introductory section, in Section 2 we describe briefly the process of plasma electrolytic oxidation and in Section 3 we give the key information about our experimental setup. In the quite extensive Section 4 we present the theoretical background of spectral investigations we carried out. The formulae collected in this section are used to explain the features of recorded spectra. The results for MgO are presented in Section 5 and those for AlO in Section 6. We conclude the paper with Section 7, where we address briefly a model that describes the systems we have been dealing with.
2. Plasma electrolytic oxidation
The process, which is the topic of the present study, is based on the anodization of valve metals (aluminum, magnesium, titanium, zirconium, tantalum, etc.) in aqueous solutions at applied potential greater than breakdown voltage (typically from 400 V to 600 V) of the original oxide film. This process is known as plasma electrolytic oxidation (PEO), microarc oxidation (MAO) or anodic spark deposition (ASD). In this work, we will use the terminology plasma electrolytic oxidation, because it has become dominant in the literature.PEO is an economic, efficient, and environmentally benign processing technique capable of producing in situ oxide coatings on valve metals, as well as on their alloys. Oxide coatings have controllable morphology and composition, excellent bonding strength with the substrate, good electrical and thermal properties, high microhardness, high-quality wear and corrosion resistance, etc. The PEO process is coupled with the formation of plasma, as indicated by the presence of microdischarges on the metal surface, accompanied by gas evolution.1–3 The anodic gas consists predominantly of oxygen with minor fractions of other elements.4 Various processes including chemical, electrochemical, thermodynamical, and plasma-chemical reactions occur at the discharge sites, due to increased local temperature (103 K to 104 K) and pressure (∼102 MPa). These processes are responsible for modifying the structure, composition, and morphology of obtained oxide coatings.
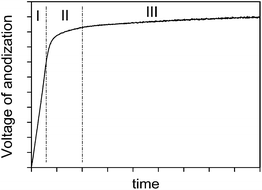
2.1. Voltage–time characteristic
Typical voltage versus time characteristic during the anodization of valve metals is shown in Fig. 1.5,6 Depending on increasing trend of the voltage, three regions can be clearly identified. From the beginning of anodization the voltage increases linearly with time, resulting in the constant formation rate of thin, compact barrier oxide layer on the substrate. This oxide layer is produced at both metal/oxide and oxide/electrolyte interfaces as a result of migration of O2−/OH− anions and metal cations Men+ (n = 2, 3, 4 or 5) across the oxide, assisted by a high electric field (∼107 V cm−1).7 Also, small amounts of anionic components of electrolyte are incorporated into the oxide at the oxide/electrolyte interface during the anodization. Barrier oxide layer is amorphous, it has ionic conductivity and high electrical resistance. Thickness of barrier oxide layer is limited to several hundred nanometers due to the dielectric breakdown under high electric field. This stage of anodization is followed by an apparent deflection from linearity in voltage–time curve, starting from so-called breakdown voltage (stage II). After the breakdown, voltage continually increases, but the voltage–time slope decreases and a large number of small-size microdischarges appear, evenly distributed over the whole sample surface. Further anodization results in relatively stable value of the voltage (stage III).In the anodization process the total current density is the sum of ionic current density and electron current density.8 In the stage I, the electric field strength, for a given current density, remains constant during the anodic growth and the ionic current is two to three orders of magnitude larger than the electronic component. In order to maintain the constant electric field strength, the voltage of anodization increases linearly with time. During anodization electrons are injected into the conduction band of the anodic oxide and accelerated by the electric field, producing avalanches by an impact ionization mechanism.8 When the avalanche electronic current reaches its critical value, the breakdown occurs.9 In the stage II a relatively low voltage increase (compared with the stage I) is required to maintain the same total current density, due to the independence of the electron current density of the anodic oxide film thickness. In the stage III, the fraction of electron current density in total current density becomes dominant. In this stage, the total current density is almost independent of the anodic oxide film thickness and the voltage–time slope is close to zero.
2.2. Mechanisms of formation of PEO coatings
PEO of valve metals is a complex process combining coexisting partial processes of oxide formation, dissolution, and dielectric breakdown. The main chemical reactions at the metal/oxide interface are:2,4,10,11| Me → Mesolidn+ + ne−, | (1) |
| Me2+ + Osolid2− → MeO, 2Me3+ + 3Osolid2− → Me2O3, Me4+ + 2Osolid2− → MeO2, 2Me5+ + 5Osolid2− → Me2O5. | (2) |
Also, direct ejection of metal into the electrolyte can occur through microdischarge channels during the breakdown:4
| Meejected + nOH− → Me(OH)n + ne−, | (3) |
The main reactions at oxide/electrolyte interface are the formation of gaseous oxygen:
| 4OH− → 2H2O + O2↑ + 4e−, | (4) |
| Men+ + (n + 1)OH− → Me(OH)(n+1)−, | (5) |
| MeO + 2OH− → MeO22− + H2O, Me2O3 + 2OH− → 2MeO22− + H2O. | (6) |
Three main steps lead to formation of oxide coatings during PEO.12 In the first step, a number of separated discharge channels are formed in the oxide layer as a result of loss in its dielectric stability in a region of low conductivity. This region is heated by generated electron avalanches up to temperatures of l04 K.13 Due to the strong electric field, the anionic components of electrolyte are drawn into the channels. Concurrently, the metal is melted out of the substrate, enters the channels, and becomes oxidized. As a result of these processes, plasma chemical reactions take place in the channels. These reactions lead to an increase in pressure inside the channels. At the same time, separation of oppositely charged ions occurs in the channel due to the presence of the electric field. The cations are ejected from the channels into the electrolyte by electrostatic forces. In the next step, oxidized metal is ejected from the channels into the coating surface in contact with the electrolyte and in that way the coating thickness around the channels increases. Finally, discharge channels get cooled and the reaction products are deposited on its walls. This process repeats itself at a number of discrete locations over the coating surface, leading to increase in the coating thickness. The coating material, formed at the sites of breakdown, contains crystalline and amorphous phases, with constituent species derived both from the metal and from the electrolyte.
2.3. Optical emission spectroscopy of microdischarges
The investigation and characterization of microdischarges are important steps in understanding the PEO process. Distribution and types of microdischarges have important effects on the formation mechanism, composition, morphology, and various properties of the resultant oxide coatings. Real-time imaging of the PEO process gives information of the microdischarge spatial density, surface fraction under microdischarges, and the dimensional distribution of microdischarges at various stages of PEO.11,14–17Given the liquid environment, optical emission spectroscopy (OES) is the best available technique for microdischarges characterization. The most popular application of OES for PEO diagnostics is spectral characterization and observation of temporal evolution of spectral lines in the visible and near UV spectral region. The main difficulty in an application of OES for PEO characterization comes from space and time inhomogeneity of microdischarges appearing randomly across the anode surface. Thus, the PEO spectra represent time integrated radiation recorded by spectrometer-detector system. The results of spectroscopic observation are even more complex to analyze if one takes into account that the radiation intensity is rather low and long exposure times are required. For this reason, high-light power spectrometers with low spectral resolution are usually employed for spectra recordings. Consequently, fine details of spectral line shape of hydrogen lines and narrow line-widths of nonhydrogenic lines are not widely used for PEO characterization.
It has been found that discharge optical emission spectra originate in general from both the species present in substrate and in electrolyte. When the substrate consists of elements with relatively low melting points (Al, Mg), the corresponding atomic and ionic lines appear independently of the type of electrolyte.18–23 On the other hand, the (non/) appearance of spectral lines of metals with high melting points (Ta, Ti, Zr) strongly depends on the electrolyte.16,24–27
Hydrogen Balmer lines Hα (656.28 nm) and Hβ (486.13 nm) can always be detected during the PEO process, and plasma broadened profiles of these lines were used for electron number density (Ne) measurement.16,17,21,22,24,25,28 Jovović et al. showed that the Balmer line Hα is very intense and strongly self-absorbed in the PEO process.17,21,22 For this reason Hα is not suitable for the spectral line shape analysis. Analysis of the Balmer line Hβ line profile during PEO of valve metals showed that the Hβ line shape can be properly fitted only if two Lorentzian profiles are used.16,17,21,22,24,25 These Lorentzian profiles correspond to electron number densities of Ne ≈ 1.0 × 1015 cm−3 and Ne ≈ 2.2 × 1016 cm−3. Spectral line shape analysis of single charge ionic lines of aluminum at 704.2 nm (ref. 21 and 22) and magnesium at 448.12 nm (ref. 21) were used for electron number density measurement in systems under consideration, and the larger electron number densities Ne ≈ (1.2–1.6) × 1017 cm−3 were obtained.
According to Hussein et al.28 three plasma discharge models have been proposed (see, Fig. 9 in ref. 28): discharging that occurs at metal/oxide interface (B) and discharging that occurs at oxide/electrolyte interface at either coating upper layer (A) or at the coating top layer (C). The highest Ne measured from single charge ion lines of aluminum and magnesium is emitted from metal plasma generated in the process of type B. Low and medium Ne are related to the processes of type A (discharge in relatively small holes near the surface of oxide layer) and of type C (discharge in the micropores at the surface of oxide layer). Microdischarges that result in evaporation of anodic material (type B) always occur during PEO of aluminum and magnesium (metals with lower melting point) regardless of the type of electrolyte. During PEO of titanium, zirconium, and tantalum (metals with high melting point) occurrence of this type of microdischarges strongly depends on the type of electrolyte.
For plasma electron temperature (Te) measurement during PEO, relative line intensities were used. This temperature is assumed equal to the electron excitation temperature, calculated from relative line intensities. The application of this approach is based on the assumption of Partial Local Thermal Equilibrium (PLTE) conditions. The discussion of the fulfillment of PLTE is given in ref. 21. For plasma electron temperature (Te) measurement during PEO of aluminum some research groups used the intensity ratio of two Al I lines at 396.2 nm and at 309.1 nm. Upon performed calculations, Te in the range of (4500–10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000) K was determined.28–30 Alongside, the intensity ratio of the Hβ and the Hα lines was used also for Te measurements and Te = 3480 K was determined.31 We suspect that these results are questionable. First, the Balmer line Hα is self-absorbed, while the Hβ interferes with an AlO band.24 Secondly, on the red side of the Al I line at 309.2 nm there is another weaker line from the same multiplet overlapping with the stronger one. In addition, these lines are Stark and Doppler broadened and positioned within the OH band, which obstructs any line shape analysis. Having this in mind, Jovović et al. used O II lines, which are always present during PEO, to determine Te.21 These lines are sometimes weak but this is an advantage from the point of view of self-absorption. Relative line intensities were used for Te measurements and upon application of Boltzmann plot technique Te ≈ 40
000) K was determined.28–30 Alongside, the intensity ratio of the Hβ and the Hα lines was used also for Te measurements and Te = 3480 K was determined.31 We suspect that these results are questionable. First, the Balmer line Hα is self-absorbed, while the Hβ interferes with an AlO band.24 Secondly, on the red side of the Al I line at 309.2 nm there is another weaker line from the same multiplet overlapping with the stronger one. In addition, these lines are Stark and Doppler broadened and positioned within the OH band, which obstructs any line shape analysis. Having this in mind, Jovović et al. used O II lines, which are always present during PEO, to determine Te.21 These lines are sometimes weak but this is an advantage from the point of view of self-absorption. Relative line intensities were used for Te measurements and upon application of Boltzmann plot technique Te ≈ 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 K was determined for aluminum.21,22 Using the same procedure, electron temperature obtained from Mg I lines is ∼4000 K,22 from Zr I lines is in the range (7500 ± 1000) K,27 and from Ti I lines in the range (3700 ± 500) K.25
000 K was determined for aluminum.21,22 Using the same procedure, electron temperature obtained from Mg I lines is ∼4000 K,22 from Zr I lines is in the range (7500 ± 1000) K,27 and from Ti I lines in the range (3700 ± 500) K.25
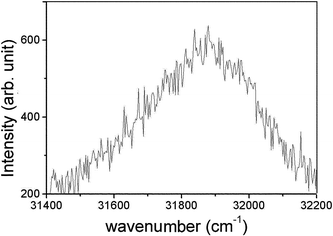
In contrast to the extensive use of atomic emission spectroscopy for investigation of PEO processes and determinations of plasma parameters, there has been very little information about the appearance of molecular bands in the considered systems. Early attempts on this field32–34 were restricted to identification of band heads and their more or less provisory assignment. Posuvailo and Klapkiv32 detected the v′ = 0; v′′ = 0, v′ = 0; v′′ = 1, and v′ = 1; v′′ = 0 bands of the B2Σ+ → X2Σ+ spectral system of AlO. In our previous study on Al,34 we assigned several bands of AlH, AlO, Al2, and possibly AlH2. Posuvailo33 claimed to identify several band heads of β and γ spectral systems of ZrO. However, as shown in our recent study on PEO of Zr,27 the corresponding emission spectrum, and particularly the structure of the ZrO bands are very complex and require much more careful investigation in order to make reliable assignment of the observed features.
3. Experimental
Rectangular samples of dimensions 25 mm × 10 nm of aluminum (99.999% purity, Goodfellow) and magnesium alloy AZ31 (96% Mg, 3% Al, 1% Zn, Alfa Aesar) were used as working electrodes in experiments. The working electrodes were sealed with insulation resin leaving only active surface area of 1.5 cm2. Before the anodization, the surface of the samples was degreased and dried in a warm air stream. The PEO process took place in an electrolytic cell with flat quartz glass windows.35 Two platinum wires (5 cm long and 1 mm in diameter) were used as cathodes. During the PEO, the electrolyte circulated through the chamber–reservoir system and the temperature of the electrolyte was maintained at (20 ± 1) °C. Aluminum was anodized in 0.1 M citric acid, while magnesium alloy was anodized in 4 g L−1 Na2SiO3·5H2O and 4 g L−1 KOH. The electrolytes were prepared by using double distilled and deionized water and PA (pro analysis) grade chemical compounds. Anodizing was carried out at current density of 100 mA cm−2Spectroscopic measurements were performed utilizing two different grating spectrometers. Spectral measurements during PEO of valve metals in a wavelength range from 380 nm to 850 nm were taken on a spectrometer system based on the intensified charge coupled device (ICCD). Optical detection system consisted of a large-aperture achromatic lens, a 0.3 m Hilger spectrometer (diffraction grating 1200 grooves per mm and inverse linear dispersion of 2.7 nm mm−1), and a very sensitive PI-MAX ICCD thermoelectrically cooled camera (−40 °C) with high quantum efficiency manufactured by Princeton Instruments.36 The CCD chip consisted of 430 × 256 active pixels, each approximately 26 μm × 26 μm. The system was used with several grating positions with overlapping wavelength range of 5 nm. Spectra were recorded in segments of 43 nm and the whole spectral range was obtained by adding one spectra interval to the previous one. The optical-detection system was calibrated using a LED based light source.37
Spectral measurements during PEO of valve metals in UV region were taken on a spectrometer system consisting of a quartz objective, Czerny–Turner spectrometer, and thermoelectrically cooled (−10 °C) CCD detector (2048 × 506 pixels, each approximately 12 μm × 12 μm) manufactured by Hamamatsu. The optical-detection system was calibrated using a hydrogen (D2) lamp. In both systems, the image of anode surface was projected with unity magnification to the entrance slit of the spectrometers.
4. Spectroscopy of diatomic molecules
4.1. Hamiltonian of a diatomic molecule
No matter how strange it might sound, the derivation of the complete Hamiltonian for a diatomic molecule is in a certain sense conceptually more demanding than that for a polyatomic molecule with non-linear equilibrium geometry. This is a consequence of the fact that one of the principal moments of inertia (that coinciding with the molecular axis) of a diatomic molecule is vanishing, what causes anomalous commutation relations between the components of angular momenta and leads to a peculiar form of the rotation part of the Hamiltonian.Let us consider a molecule with the nuclei A and B whose masses are mA and mB, and charge numbers ZA and ZB, respectively, and with N electrons (mass = me). The total mass of the nuclei (Mn) and of the molecule (M are thus Mn ≡ mA + mB, M = Mn + Nme), respectively. We start with the non-relativistic molecular Hamiltonian defined in a space-fixed coordinate system (SFS). To separate off the translational motion of the molecule, we use an intermediate frame with the axes parallel to those of the SFS and with the origin laying in the mass center of the molecule (involving also the electrons). However, we refer the electron coordinates to the mass center of the nuclei, and we call this frame nuclear center of mass system (NCMS). As the three linearly independent nuclear coordinates we chose the components X, Y, Z of the position vector, ![[r with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0072_20d1.gif) , of the nucleus B with respect to that of the nucleus A,
, of the nucleus B with respect to that of the nucleus A, ![[r with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0072_20d1.gif) =
= ![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) B −
B − ![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) A. We define the components of the angular momentum operator of the nuclei,
A. We define the components of the angular momentum operator of the nuclei, 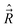 , spatial electron angular momentum,
, spatial electron angular momentum, 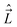 , electron spin operator,
, electron spin operator, 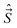 , total molecular angular momentum excluding spin,
, total molecular angular momentum excluding spin, 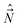 , and total (rovibronic) angular momentum,
, and total (rovibronic) angular momentum, 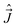 (we ignore possible presence of the nuclear spins). They satisfy the normal commutation relations,
(we ignore possible presence of the nuclear spins). They satisfy the normal commutation relations,
[![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) X, X, ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Y] = iℏ Y] = iℏ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Z, [ Z, [![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Y, Y, ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Z] = iℏ Z] = iℏ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) X, [ X, [![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Z, Z, ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) X] = iℏ X] = iℏ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) Y, Y,
| (7) |
 is
is  ,
,  ,
,  ,
,  , or
, or 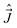 . Further, all the components of different angular momenta (
. Further, all the components of different angular momenta (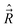 ,
, 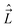 , and
, and 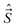 ) commute with one another because they act on different coordinates.
) commute with one another because they act on different coordinates.
We introduce finally a molecule-fixed coordinate system (MFS) with the goal to separate, as completely as possible, the vibrational from rotational degrees of freedoms. We need the real (original) SFS no more and we rename NCMS into SFS. We choose the MFS so that its z-axis lays along the molecular axis (the direction from the nucleus A towards B) – in this way the rotation of the nuclear skeleton is reduced to the rotation of the MFS. The orientation of this axis with respect to the NCMS is defined by the Euler angles ϕ and θ, which represent thus the rotational coordinates. We fix the value of the third Euler angle χ (that determines the orientation of the x and y axes) to χ = 0. The instantaneous distance between the nuclei, r, is the coordinate whose change corresponds to the vibrations of the nuclei. We denote the spatial coordinates of the electrons in the MFS by xμ, yμ, zμ. The Hamiltonian of the molecule transforms into38
Ĥ = ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) e + e + ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) n + n + ![[V with combining circumflex]](https://www.rsc.org/images/entities/i_char_0056_0302.gif) en + en + ![[V with combining circumflex]](https://www.rsc.org/images/entities/i_char_0056_0302.gif) ee + ee + ![[V with combining circumflex]](https://www.rsc.org/images/entities/i_char_0056_0302.gif) nn. nn.
| (8) |
![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) e is the electronic kinetic energy operator:
e is the electronic kinetic energy operator:
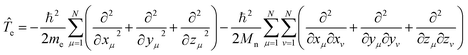 | (9) |
The nuclear kinetic energy operator has the form
![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) n = n = ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) vib + vib + ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) rot rot
| (10) |
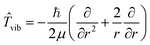 | (11) |
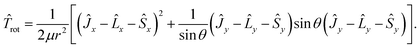 | (12) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin
sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θdrdθdϕ. The potential energy part of the Hamiltonian (8) is
θdrdθdϕ. The potential energy part of the Hamiltonian (8) is
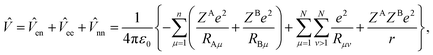 | (13) |
![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) μ −
μ − ![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) A| = |
A| = |![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) μ + mB
μ + mB![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) /Mn|, RBμ = |
/Mn|, RBμ = |![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) μ −
μ − ![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) B| = |
B| = |![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) μ − mA
μ − mA![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) /Mn|, Rμv = |
/Mn|, Rμv = |![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) v −
v − ![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) μ|.
μ|.
The components of the electronic spatial and spin angular momenta along the MFS-axes are defined in usual way. However, the components of the (rovibronic) angular momentum are38
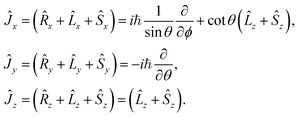 | (14) |
The components of the electronic angular momenta along the MFS-axes have normal commutation relations, analogous to (7). On the other hand, the components of the rovibronic angular momentum  along the MFS-axes fulfill the following anomalous commutation relations:38
along the MFS-axes fulfill the following anomalous commutation relations:38
[Ĵx, Ĵy] = −iℏ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cot cot![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θĴx − iℏ( θĴx − iℏ(![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z + Ŝz), [Ĵy, Ĵz] = 0, [Ĵz, Ĵx] = 0. z + Ŝz), [Ĵy, Ĵz] = 0, [Ĵz, Ĵx] = 0.
| (15) |
![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) e) being the electronic kinetic energy operator, the second (
e) being the electronic kinetic energy operator, the second (![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) vib) involving derivatives with respect to the coordinate r, and the third one (
vib) involving derivatives with respect to the coordinate r, and the third one (![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) rot) that contains the angular momenta. Note, however, that the separation of the electronic, vibrational, and rotational degrees of freedom is not complete: In the rotation part we also have the vibrational coordinate r as well as the electronic coordinates via the operators
rot) that contains the angular momenta. Note, however, that the separation of the electronic, vibrational, and rotational degrees of freedom is not complete: In the rotation part we also have the vibrational coordinate r as well as the electronic coordinates via the operators ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) α and Ŝα. The Schrödinger equation corresponding to the kinetic energy operator ((9)–(12)) and the potential energy (13) is solved successively. In the framework of the Born–Oppenheimer approximation,39 the electronic part of the Hamiltonian, which involves the electronic kinetic energy operator (9), and the potential energy operator (13), is replaced by the corresponding eigenvalue, V(r), being a function of the coordinate r. In the lowest-order (harmonic) approximation,
α and Ŝα. The Schrödinger equation corresponding to the kinetic energy operator ((9)–(12)) and the potential energy (13) is solved successively. In the framework of the Born–Oppenheimer approximation,39 the electronic part of the Hamiltonian, which involves the electronic kinetic energy operator (9), and the potential energy operator (13), is replaced by the corresponding eigenvalue, V(r), being a function of the coordinate r. In the lowest-order (harmonic) approximation,
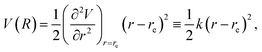 | (16) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θdrdθdϕ, eqn (11) transforms into
θdrdθdϕ, eqn (11) transforms into
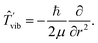 | (17) |
The vibration–rotation problem with the Hamiltonian involving the nuclear kinetic energy operator (10) and V(r), playing the role of the potential, can be handled simultaneously for both vibrations and rotations. An alternative way is first to solve the vibrational part of the Schrödinger equation [Ĥvib = ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) vib + V(r)] and after that the rotational part (Ĥrot =
vib + V(r)] and after that the rotational part (Ĥrot = ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) rot), where the quantity 1/r2 in eqn (12) is replaced by its electronically and vibrationally averaged counterpart 〈1/r2〉(in the lowest-order, “rigid-rotor” approximation, 〈1/r2〉 = 1/re2).
rot), where the quantity 1/r2 in eqn (12) is replaced by its electronically and vibrationally averaged counterpart 〈1/r2〉(in the lowest-order, “rigid-rotor” approximation, 〈1/r2〉 = 1/re2).
4.2. Isomorphic Hamiltonian
A linear molecule only has two rotational degrees of freedom described by the Euler angles ϕ and θ, being sufficient to define the orientation of the internuclear axis (z) in space. At the same time, the third Euler angle χ is arbitrary. The Hamiltonian ((8)–(13)) corresponds to the choice χ = 0, but in a more general treatment χ is considered as a certain function of ϕ and θ.40,41 The lack of the third Euler angles causes anomalies as unusual commutation relations, eqn (15), and a strange form of the rotational Hamiltonian, eqn (12). These problems can be solved introducing the “isomorphic Hamiltonian”.38,40–42 It has three rotational degrees of freedom (as that of a nonlinear molecule) so that the components of all angular momenta satisfy usual (or “almost” usual, i.e. up to the sign) commutation relations. On the other hand, the isomorphic Hamiltonian has a number of eigenvalues not being the eigenvalues of the real Hamiltonian. These artificial eigenvalues can, however, be easily eliminated when the Hamiltonian is represented in a suitable basis.In order to derive the isomorphic Hamiltonian, we introduce χ as an independent variable that defines a new coordinate frame (x′y′z′) tied to the molecule; it is obtained from the frame (xyz), corresponding to the choice χ = 0, by rotation through χ about the z-axis. The components of the total angular momentum along the (x′y′z′)-axes are38,41,43
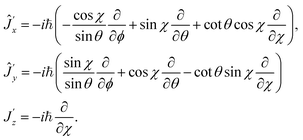 | (18) |
They commute with the components of the angular momentum along the SFS-axes, Ĵ′X, Ĵ′Y, and Ĵ′Z. The operators Ĵ′x, Ĵ′y, and Ĵ′z commute with the components of 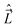 and
and 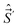 (along the axes of the same frame), because they act onto different coordinates. However, the commutation relations between Ĵ′x, Ĵ′y, and Ĵ′x are41,43–46
(along the axes of the same frame), because they act onto different coordinates. However, the commutation relations between Ĵ′x, Ĵ′y, and Ĵ′x are41,43–46
| [Ĵ′x, Ĵ′y] = −iℏĴ′z, [Ĵ′y, Ĵ′z] = −iℏĴ′x, [Ĵ′z, Ĵ′x] = −iℏĴ′y. | (19) |
They are anomalous [indeed not so extremely anomalous as (15)], because they differ from the normal commutation relations for angular momenta, like those in eqn (7), in the sign. On the other hand, the electronic angular spatial and spin momenta have normal commutation relations. The anomalous commutation relations of the type (19) have the components along the x′y′z′-axes of all angular momenta which involve nuclear coordinates, like  and
and 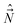 . Note that the components of the nuclear angular momentum along the MFS-axes in general do not commute with the components of the electronic angular momentum.
. Note that the components of the nuclear angular momentum along the MFS-axes in general do not commute with the components of the electronic angular momentum.
The components of the angular momenta along the (x′y′z′)-axes are related to the components of 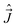 along the (xyz) axes, by
along the (xyz) axes, by
Ĵx − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) x − Ŝx = cos x − Ŝx = cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) χ(Ĵ′x − χ(Ĵ′x − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′x − Ŝ′x) − sin ′x − Ŝ′x) − sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) χ(Ĵ′y − χ(Ĵ′y − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′y − Ŝ′y), ′y − Ŝ′y), |
Ĵy − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) y − Ŝy = sin y − Ŝy = sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) χ(Ĵ′x − χ(Ĵ′x − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′x − Ŝ′x) + cos ′x − Ŝ′x) + cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) χ(Ĵ′y − χ(Ĵ′y − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′y − Ŝ′y), ′y − Ŝ′y), |
Ĵz − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z − Ŝz = Ĵ′z − z − Ŝz = Ĵ′z − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′z − Ŝ′z = 0. ′z − Ŝ′z = 0.
| (20) |
The rotational Hamiltonian (in cm−1) has in terms of new components of the angular momenta the form
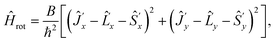 | (21) |
 | (22) |
Note that the Hamiltonians (12) and (22) are mutually isomorphic but not identical. The border between the identity and isomorphism is crossed by skipping from eqn (21) and (22), i.e. by using the condition Ĵ′z − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′z − Ŝ′z = 0. Since Ĥisorot commutes with the operator (Ĵ′z −
′z − Ŝ′z = 0. Since Ĥisorot commutes with the operator (Ĵ′z − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′z − Ŝ′z), these two operators have common eigenfunctions, and the mentioned condition will be fulfilled only when we use the basis functions which correspond to the zero eigenvalues of the operator (Ĵ′z −
′z − Ŝ′z), these two operators have common eigenfunctions, and the mentioned condition will be fulfilled only when we use the basis functions which correspond to the zero eigenvalues of the operator (Ĵ′z − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′z − Ŝ′z) – only these eigenfunctions of the isomorphic Hamiltonian will be the true wave functions of the operator (12). They are obtained as solutions of the eigenvalue problems
′z − Ŝ′z) – only these eigenfunctions of the isomorphic Hamiltonian will be the true wave functions of the operator (12). They are obtained as solutions of the eigenvalue problems
Ĵ′zψr = Ωℏψr, ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) ′zψe = Λℏψe, Ŝ′zψS = ΣℏψS. ′zψe = Λℏψe, Ŝ′zψS = ΣℏψS.
| (23) |
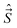 on the z′-axis, and Ω = Λ + Σ.
on the z′-axis, and Ω = Λ + Σ.
We shall use further the isomorphic rotation Hamiltonian. To simplify the notation we shall delete the primes and the superscript iso. The operator (22) can be written in several alternative forms, depending on whether the relation Ĵz − ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z − Ŝz = 0 is taken into account explicitly or implicitly e.g.
z − Ŝz = 0 is taken into account explicitly or implicitly e.g.
 | (24) |
The last term on the right-hand side of eqn (24), producing no dependence on J, shifts all rotational levels by the same amount and thus we shall consider it as a part of the electronic Hamiltonian (i.e. we neglect it when we only consider rotational structure). The terms involving the products of electronic ladder operators, ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) + ≡
+ ≡ ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) x + i
x + i![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) y,
y, ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) − ≡
− ≡ ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) x − i
x − i![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) y, and Ĵ±/Ŝ± only have off-diagonal electronic matrix elements (i.e. they couple different electronic state). When we calculate the rotational levels in a particular electronic state, the contribution of these terms is small and we shall in general neglect them. (However, they are responsible e.g. for the Λ-splitting in Π states.) Such a simplified rotation Hamiltonian can be written as
y, and Ĵ±/Ŝ± only have off-diagonal electronic matrix elements (i.e. they couple different electronic state). When we calculate the rotational levels in a particular electronic state, the contribution of these terms is small and we shall in general neglect them. (However, they are responsible e.g. for the Λ-splitting in Π states.) Such a simplified rotation Hamiltonian can be written as
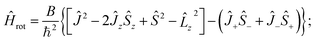 | (25) |
The rotational Hamiltonian (24) obviously commutes with Ĵ2, Ŝ2, and ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) 2, and its approximate form eqn (25) additionally with
2, and its approximate form eqn (25) additionally with ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z. Recall, however, that the rotational Hamiltonian only represents a part of the total molecular Hamiltonian. The complete molecular Hamiltonian involves besides Ĥrot the electronic and vibrational parts, Ĥe and Ĥvib, respectively. Since Ĥe does not commute with
z. Recall, however, that the rotational Hamiltonian only represents a part of the total molecular Hamiltonian. The complete molecular Hamiltonian involves besides Ĥrot the electronic and vibrational parts, Ĥe and Ĥvib, respectively. Since Ĥe does not commute with ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) 2, the quantum number L, which would correspond to
2, the quantum number L, which would correspond to ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) 2, is never good. Note also that in the rest of the Hamiltonian appear in general the terms that also involve the rotation coordinates, i.e. which take into account the coupling of rotations with the other degrees of freedom. Some of the couplings can be neglected, but there are several of them interfering qualitatively and quantitatively with the rotational structure of molecular spectra. The most important of these is usually the spin–orbit/rotation coupling, because the corresponding effects are on the energy scale comparable with those caused by molecular rotations. For this reason, we shall below complete the Hamiltonian by the terms describing the leading part of the spin couplings.
2, is never good. Note also that in the rest of the Hamiltonian appear in general the terms that also involve the rotation coordinates, i.e. which take into account the coupling of rotations with the other degrees of freedom. Some of the couplings can be neglected, but there are several of them interfering qualitatively and quantitatively with the rotational structure of molecular spectra. The most important of these is usually the spin–orbit/rotation coupling, because the corresponding effects are on the energy scale comparable with those caused by molecular rotations. For this reason, we shall below complete the Hamiltonian by the terms describing the leading part of the spin couplings.
In multiplet (i.e. non-singlet) Σ electronic states (Λ = 0, S ≠ 0) we have the spin–rotation coupling; we add then to the Hamiltonian (24) or (25) the corresponding operator. We shall represent it in the “phenomenological form”, ref. 43
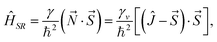 | (26) |
In multiplet Π, Δ,… (Λ ≠ 0, S ≠ 0) electronic states the main spin effect is the spin–orbit coupling. It is usually described by the phenomenological operator43
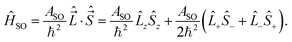 | (27) |
The matrix elements of the second term on the right-hand side of eqn (27) are vanishing within an electronic state and do not contribute to the energy in first order. Thus, we usually use the simplified form of the spin–orbit operator,
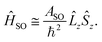 | (28) |
In the general case the only operators which commute with the Hamiltonian of a molecule are Ĵ2, Ŝ2, and the operator E* that inverts the coordinates of all particles (electrons and nuclei) in the SFS; the quantum numbers of these operators are J, S, and + or −, respectively. However, depending on the concrete situation, there may be various near (good) quantum numbers. Such quantum numbers correspond to the operators that do not commute with the complete Hamiltonian, Ĥ, but they do commute with the dominant part of it, say Ĥ1. The eigenfunctions of Ĥ1 build a suitable basis for representation of Ĥ, and the quantum numbers associated with the operators that commute with Ĥ1 label these basis functions. They represent “nearly good” quantum numbers, because the leading part of Ĥ, namely Ĥ1, is in such a basis represented by a diagonal matrix. The near quantum numbers are particularly useful for assignment of experimentally observed spectral features. Note that, strictly speaking, even J is not a good quantum number – it is such only in the case of vanishing nuclear spins. In the presence of nuclear spin, 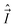 , the only good quantum number is that corresponding to the total angular momentum
, the only good quantum number is that corresponding to the total angular momentum 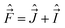 . However, in this case J is (usually) very nearly a good quantum number.
. However, in this case J is (usually) very nearly a good quantum number.
4.3. Basis functions
The fact that there are three operators Ĵ2, Ĵz, and ĴZ that commute with one another means that there exist sets of their common eigenstates |JΩM〉. Thus,| Ĵ2|JΩM〉 = J(J + 1)ℏ2|JΩM〉, ĴZ|JΩM〉 = Mℏ|JΩM〉, Ĵz|JΩM〉 = Ωℏ|JΩM〉. | (29) |
Non-vanishing matrix elements of the relevant operators in this basis are
 | (30) |
| Ĵs± ≡ ĴX ± iĴY, Ĵm± ≡ Ĵx ± iĴy. | (31) |
Note the sign differences in the expressions (30) for matrix elements of these operators. Since we do not deal with external fields, the choice of the quantum number M is arbitrary.
We shall normally use the basis functions corresponding to Hund's case (a) coupling scheme.41,43,46,47 According to it 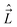 and
and 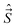 are both “tied” to the internuclear axis (the z axis of the MFS) making the signed projections Λ and Σ, respectively, while
are both “tied” to the internuclear axis (the z axis of the MFS) making the signed projections Λ and Σ, respectively, while  is perpendicular to this axis. Thus the total angular momentum
is perpendicular to this axis. Thus the total angular momentum 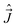 makes the projection M on the SFS axis Z and the projection Ω = Λ + Σ on the MFS axis z. Consequently, in this case the rotational basis functions are the symmetric top wave functions |JΩM〉. The total case (a) wave functions are labeled additionally by the electronic spatial quantum numbers n (“principal” quantum number) and Λ, the spin quantum numbers S, Σ, and the vibrational quantum number v. We write them in the product form41,43
makes the projection M on the SFS axis Z and the projection Ω = Λ + Σ on the MFS axis z. Consequently, in this case the rotational basis functions are the symmetric top wave functions |JΩM〉. The total case (a) wave functions are labeled additionally by the electronic spatial quantum numbers n (“principal” quantum number) and Λ, the spin quantum numbers S, Σ, and the vibrational quantum number v. We write them in the product form41,43
| |n, Λs; v; S, Σ, J, Ω, M〈 = |nΛs〉|v〉|SΣ〉|JΩ(M)〉, | (32) |
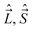 , and
, and  , appearing in the rotational Hamiltonian only acts on one set of the coordinates and their effect on the vector |nΛSΣ〉 is the same as the effect on the factorized (in the general case, fictitious) form of this vector |nΛ〉|SΣ〉. The basis (32) is convenient because the key operators
, appearing in the rotational Hamiltonian only acts on one set of the coordinates and their effect on the vector |nΛSΣ〉 is the same as the effect on the factorized (in the general case, fictitious) form of this vector |nΛ〉|SΣ〉. The basis (32) is convenient because the key operators ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z, Ĵ2, Ĵz, Ŝ2, and Ŝz are all diagonal in this coupling scheme.
z, Ĵ2, Ĵz, Ŝ2, and Ŝz are all diagonal in this coupling scheme.
Another frequently used basis consists of Hund's case (b) functions, where the intermediate nearly good quantum number N is used (its projection on the z-axis is Λ) and the spin quantum number Σ is omitted:
| |n, Λs; v; N, Λ, S, J, M〉 = |nΛs〉|v〉|SJNΛ(M)〉. | (33) |
The fact that the components along the MFS-axes of some of the momenta we use 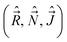 have anomalous commutation relations, whereas the commutation relations for the other class of operators
have anomalous commutation relations, whereas the commutation relations for the other class of operators 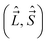 are normal, causes certain problems. The momenta
are normal, causes certain problems. The momenta  ,
, 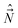 ,
,  , when expressed in terms of their MFS components are actually not proper angular momenta, because the angular momentum operator is defined as a vector operator whose components satisfy commutation relations of the type (7). Thus, when working with these operators we cannot directly use a number of relations derived for proper momenta (e.g. those based on the application of the Wigner–Eckart theorem, the Clebsh–Gordan coefficients etc.).48 Further, the composite momentum from the standpoint of the theory of addition of angular momenta,
, when expressed in terms of their MFS components are actually not proper angular momenta, because the angular momentum operator is defined as a vector operator whose components satisfy commutation relations of the type (7). Thus, when working with these operators we cannot directly use a number of relations derived for proper momenta (e.g. those based on the application of the Wigner–Eckart theorem, the Clebsh–Gordan coefficients etc.).48 Further, the composite momentum from the standpoint of the theory of addition of angular momenta, 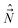 [see eqn (33)], is the difference and not the sum of “partial momenta”
[see eqn (33)], is the difference and not the sum of “partial momenta” 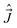 and
and 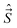 . These problems can generally be solved in three ways. (a) One can work with the components of all momenta along the SFS-axes – all of them satisfy normal commutation relations – and only after carrying out all algebraic manipulation, the results are expressed in terms of the MFS components.45,46 (b) The other possibility is to work consequently with the MFS components, bearing in mind anomalies and restrictions mentioned above. (c) The third way was proposed by Van Vleck:44 Instead of momenta
. These problems can generally be solved in three ways. (a) One can work with the components of all momenta along the SFS-axes – all of them satisfy normal commutation relations – and only after carrying out all algebraic manipulation, the results are expressed in terms of the MFS components.45,46 (b) The other possibility is to work consequently with the MFS components, bearing in mind anomalies and restrictions mentioned above. (c) The third way was proposed by Van Vleck:44 Instead of momenta ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) x,
x, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) y,
y, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) z, with anomalous commutation relations like those for Ĵx, Ĵy, Ĵz, eqn (19), we can use the “reverse” momenta
z, with anomalous commutation relations like those for Ĵx, Ĵy, Ĵz, eqn (19), we can use the “reverse” momenta
![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rx = − rx = −![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) x, x, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) ry = − ry = −![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) y, y, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rz = − rz = −![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) z, z,
| (34) |
[![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rx, rx, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) ry] = iℏ ry] = iℏ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rz, [ rz, [![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) ry, ry, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rz] = iℏ rz] = iℏ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rx, [ rx, [![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rz, rz, ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) rx] = iℏ rx] = iℏ![[P with combining circumflex]](https://www.rsc.org/images/entities/i_char_0050_0302.gif) ry. ry.
| (35) |
(Indeed, Van Vleck preferred to invert the momenta with normal commutation relations, ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) rx = −
rx = −![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) x, etc. so that all momenta along the MFS-axes consistently had anomalous commutation relation.) Consequently, all momenta along the MFS-axes (
x, etc. so that all momenta along the MFS-axes consistently had anomalous commutation relation.) Consequently, all momenta along the MFS-axes (![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) x, Ŝx,
x, Ŝx, ![[R with combining circumflex]](https://www.rsc.org/images/entities/i_char_0052_0302.gif) rx,
rx, ![[N with combining circumflex]](https://www.rsc.org/images/entities/i_char_004e_0302.gif) rx, Ĵrx,…) have normal commutation relations.
rx, Ĵrx,…) have normal commutation relations.
Each wavefunction associated with an energy level may be classified as even or odd according to whether it remains unchanged or changes sign on the operation E*, carrying out inversion of SFS spatial coordinates of all electrons and nuclei. Since the molecular Hamiltonian is invariant under this symmetry operation, it has non-vanishing matrix elements only between the state vectors of the same parity. This operation does not affect the vibrational coordinates. It does not act directly on the spin coordinates, but in the case when the spin angular momenta are quantized along the MFS-axes [Hunds's basis (a)], there is an indirect effect on the spin functions because the transformation from SFS to MFS involves the Euler angles, being functions of the SFS nuclear coordinates. It can be shown that the effect of E* on Hund's (a) basis functions is41,43
| E*|nΛs〉|v〉|SΣ〉|JΩ(M)〉 = (−1)p|n, −Λ〉|v〉|S, −Σ〉|J, −Ω, M〉, |
| p = J − S + s. | (36) |
For Hund's (b) basis,
| E*|nΛs〉|v〉|N, Λ, S, J, M〉 = (−1)N+s|n, −Λ〉|v〉|N, −Λ, S, J, M〉 | (37) |
It follows that the simple case (a) or case (b) basis functions are not eigenfunctions of E*. The appropriate combinations which do have a definite parity are in the case (a)41
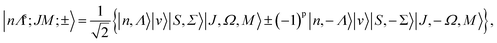 | (38) |
The ±(−1)J−S+s phase factor in eqn (38) causes the parity labels to alternate as J increases, so that the lower of the near-degenerate pair for a given J might be + and the upper −, the designation becomes opposite for the next J value and so on. To avoid this alternation, another parity labeling convention, e/f, has been introduced.49 For integral J, e-levels have parity (−1)J and f-levels (−1)J+1; for half-integral J, e-levels have parity (−1)J−1/2 and f-levels (−1)J+1/2. Following this convention, all lower components of parity doublets have the same label, e.g. e, and all the upper components have the opposite label, in this case f.
4.4. Transitions
| R′,′′ = 〈n′Λ′; v′; S′Σ′; J′Ω′M′|Â|n′′Λ′′; v′; S′′Σ′′; J′′Ω′′M′′〉 | (39) |
![[small mu, Greek, circumflex]](https://www.rsc.org/images/entities/i_char_e0b3.gif) , defined by
, defined by
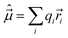 | (40) |
![[r with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0072_20d1.gif) i radius vector of the ith particle and the sum runs over all charged particles (electrons, nuclei). The proportionality factor between the intensity of the transition and |R′,′′|2 involves, beside natural constants and statistical weights, in the case of absorption the energy difference, ΔE, between the levels in question, and at emission ΔE4.
i radius vector of the ith particle and the sum runs over all charged particles (electrons, nuclei). The proportionality factor between the intensity of the transition and |R′,′′|2 involves, beside natural constants and statistical weights, in the case of absorption the energy difference, ΔE, between the levels in question, and at emission ΔE4.In the framework of usually applied non-relativistic, Born–Oppenheimer, and rigid rotator/infinitesimal vibrator approximations the state vectors are assumed in the form of products of partial state vectors for individual degrees of freedom, like on the right-hand side of eqn (32). If we are only interested in “allowed” transitions, the spin variables can be excluded from consideration since in this case transitions are only possible when both states in question have the same spin function (S′ = S′′, Σ′ = Σ′′). We start with the expression for the components of the transition moment, ![[small mu, Greek, circumflex]](https://www.rsc.org/images/entities/i_char_e0b3.gif) F, (F = X, Y, Z) along the axes of the SFS,
F, (F = X, Y, Z) along the axes of the SFS,
R′,′′F = 〈J′Ω′|〈v′|〈n′Λ′|![[small mu, Greek, circumflex]](https://www.rsc.org/images/entities/i_char_e0b3.gif) F|n′′Λ′′〉|v′′〉|J′′Ω′′〉, F|n′′Λ′′〉|v′′〉|J′′Ω′′〉,
| (41) |
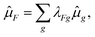 | (42) |
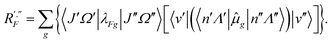 | (43) |
| 〈n′Λ′|μg|n′′Λ′′〉 ≡ Re′,e′′g, | (44) |
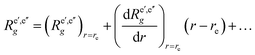 | (45) |
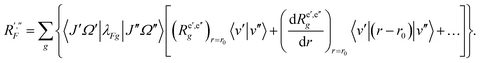 | (46) |
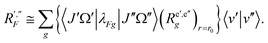 | (47) |
This expression will be nonvanishing if the electric transition moment does not equal zero. This is the case when the product of the irreducible representations of the electronic wave functions equals the irreducible representation of the x, y, or z-components of the dipole operator. In the case of heteronuclear diatomics, the z-component of this operator belongs to the Σ+ and x- and y-components building together the basis for a Π irreducible representation of the point group C∞v. This results in the selection rule ΔΛ = 0, ±1. Additional selection rule is that the transitions Σ+ ↔ Σ+ and Σ− ↔ Σ− are allowed, while the transitions Σ+ ↔ Σ− are forbidden. In the case of homonuclear diatomics, the z-component of this operator belongs to the Σu+ and x- and y-components to the Πu irreducible representation of the point group D∞v. This yields the additional selection rule that the electronic transition is allowed only if one of the combining states has g and the other u symmetry.
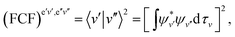 | (48) |
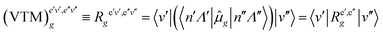 | (49) |
In the F–C approximation, 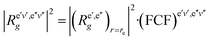 . The magnitude of |VTM|2 or (sometimes significantly less reliably) FCF determines the intensity distribution within a (rotationally unresolved) band system, which consists of bands, each one arising by rotational transitions between a pair of vibrational levels of two electronic states. The most prominent features in band systems are progressions (characterized in absorption by a fixed vibrational quantum number v′′ and variable quantum number v′, and fixed v′ and variable v′′ in emission) and sequences, v′–v′′ = const. If the equilibrium bond lengths and vibrational frequencies are similar in both electronic states, the most intense bands in an experimentally recorded spectrum correspond to the transitions v′–v′′ = 0. A long progression with the maximum at a quite large value of |v′–v′′| indicates that the equilibrium bond lengths in the combining electronic states are appreciably different. While the bands in a progression are separated from one another by several hundreds to few thousands cm−1 (corresponding to the wave numbers of vibrational fundamentals), the separation of the bands in a sequence is usually between several tens and several hundreds cm−1, reflecting the difference in the values of vibrational frequencies in the two electronic states in question.
. The magnitude of |VTM|2 or (sometimes significantly less reliably) FCF determines the intensity distribution within a (rotationally unresolved) band system, which consists of bands, each one arising by rotational transitions between a pair of vibrational levels of two electronic states. The most prominent features in band systems are progressions (characterized in absorption by a fixed vibrational quantum number v′′ and variable quantum number v′, and fixed v′ and variable v′′ in emission) and sequences, v′–v′′ = const. If the equilibrium bond lengths and vibrational frequencies are similar in both electronic states, the most intense bands in an experimentally recorded spectrum correspond to the transitions v′–v′′ = 0. A long progression with the maximum at a quite large value of |v′–v′′| indicates that the equilibrium bond lengths in the combining electronic states are appreciably different. While the bands in a progression are separated from one another by several hundreds to few thousands cm−1 (corresponding to the wave numbers of vibrational fundamentals), the separation of the bands in a sequence is usually between several tens and several hundreds cm−1, reflecting the difference in the values of vibrational frequencies in the two electronic states in question.
The F–C approximation is thus mathematically based on the assumption that the electric transition moment is a constant quantity. This corresponds to physical picture of the “vertical” electronic transitions, i.e. the transitions at unchanged distance between the nuclei. If we go beyond the F–C approximation, we have to take into account the dependence of the electric transition moment on the internuclear distance [the second term in of eqn (45)/(46)]. In a diatomic molecule (where the unique vibrational coordinate, r, is totally-symmetric) this only quantitatively influences the transition probabilities; in polyatomic linear molecules it may make the transitions, forbidden in the F–C approximation, “vibronically” allowed.
Measured electronic-vibration terms (in cm−1) are usually represented by the formula
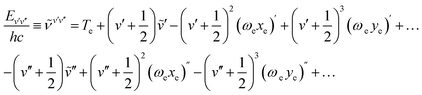 | (50) |
We have used the experimentally derived formula (50) for assignment of bands observed in our spectra. However, for determination of the plasma temperature we also need the FCFs and/or VTMs. In order to calculate these quantities we have to solve the vibrational Schrödinger equation for two electron states in question and to use the so obtained wave functions to compute the required quantities. If one wishes to avoid explicit ab initio calculations of the potential energy curves for the electronic states in question, he has first to find a way how to extract the potential energy function that, combined with the corresponding kinetic energy operator, gives the energy eigenvalues as close as possible to those presented by the formula (50). We have solved this problem combing the quantum-mechanical perturbative and variational approaches.
We assume the vibrational Hamiltonian in the form
| H = H0 + V′, | (51) |
 | (52) |
| V′ = k3x3 + k4x4 +…; | (53) |
| k = μω2 = 4μπ2c2ṽ2, | (54) |
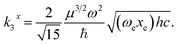 | (55) |
In variational calculations of the eigenvalues and eigenfunctions of an anharmonic oscillator, as well as of the FCFs and VTMs for particular combinations of vibrational levels of two electronic state, the following procedure is applied:53 one of the electronic states, a, (typically ground state) is chosen as the referent one. The basis functions (being the eigenfunctions of a suitably chosen harmonic oscillator) are centered with respect to its equilibrium bond length, re. If the potential energy curve is ab initio computed, it is fitted to a polynomial of the type (53). The potential energy curve of the other state, b, is fitted by polynomial series in the same variable as for the reference state,
| Vb = kb0 + kb1(r − re) + kb2(r − re)2 + kb3(r − re)3 +… | (56) |
 | (57) |
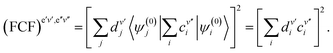 | (58) |
When the bond-length dependence of the electric transition moment is taken into account, it is fitted to the form
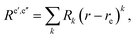 | (59) |
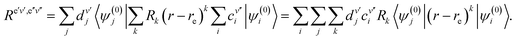 | (60) |
The intensity of the spectral band appearing as a consequence of the emission transition between the vibrational level v′ of the electronic state e′ and the vibrational level v′′ of the electronic e′′ is determined by the formula
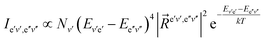 | (61) |
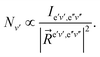 | (62) |
In the F–C approximation, it is assumed that the electric transition moment is constant, and in this case we replace in the formula (62) |![[R with combining right harpoon above (vector)]](https://www.rsc.org/images/entities/i_char_0052_20d1.gif) e′v′,e′′v′′|2 by the FCF.
e′v′,e′′v′′|2 by the FCF.
Assuming a partial local thermal equilibrium of the system, the ratio of the number of molecules Ne′v′ in the vibrational state v′ and in the lowest vibrational state v′ = 0 of the same electronic species, Ne′0 is determined by
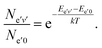 | (63) |
Combining the formulae (62) and (63), we obtain for the sequence v′–v′′ = −Δv
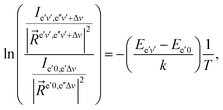 | (64) |
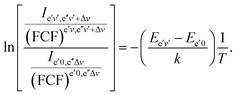 | (65) |
We use below the formulae (64) and/or (65) to determine the plasma temperature. This approach has the advantage that it does not require the knowledge of the Einstein transition probabilities and that the measured peaks appear in a narrow spectral range such that the problems caused by wavelength dependence of the measurement sensitivity are avoided.
| J′ − J′′ = 0, ±1, | (66) |
4.5. Sources of experimental data
The classical sources of information about molecular spectra represent the monographs by Herzberg47,54–56 and Pearse and Gaydon.57 In Herzberg's (H) volumes I47 and III53 are collected all relevant experimental data up to fifties/sixties of the preceding century. In the fourth volume, published in 1979 in collaboration with Huber (HH),56 one can find the data on diatomic molecules reported until the end of 1975. The fourth (to our knowledge the last one) edition of the book by Pearse and Gaydon (PG) is printed in 1976, i.e. almost at the same time as the HH's compilation of data on diatomics. This edition contains the data published until the end of 1974. Thus, at searching for information about molecular spectra, one has practically only to look at experimental papers published after appearance of the mentioned monographs, and to complete in this way the information collected by H/HH and PG. These books has become such a standard source of information on molecular spectra, that many authors of new studies do not cite explicitly the authors of old experimental works but they simply quote these studies as “H or PG and the references therein”. We will in general follow this praxis in the present review.While the three volumes by Herzberg47,54,55 involve also complete theory underlying molecular spectra, as well as numerical values for all molecular parameters like vibrational frequencies and anharmonicity constants, rotational constants, internuclear distances, etc., the book by PG only involves the results of direct spectral measurements, i.e. positions of band heads and their relative intensities. Thus, the books by H and PG are in a sense complementary. It should be noted that in the H's books the transition energies are given in wave numbers for vacuum, whereas they are collected by PG in wavelengths, as measured in air. Therefore, a PG wavelength is not exactly the inverse of the corresponding H's wave number.
4.6. On the interplay between molecular spectroscopy and quantum chemistry
One of the greatest spectroscopists, D. A. Ramsay, presented at a Conference (Breukelen, Holland) in 1976 an interesting table (Table 1, first and second column with error margins). It showed what experimentalists expected from the theoreticians at that time (i.e. forty years ago): Not necessarily data of spectroscopic accuracy but rather results with definite error margins that would help answer some important questions, which could not be answered on the basis of experiments alone. So, e.g., the theoretical information about the occurrence and mutual interactions of various electronic states in a certain energy range was very valuable for the experimentalists; the help of theoreticians was sought when it was not possible to assign unambiguously the spectral lines observed. Ramsay gave two sets of error margins, which he named “acceptable” and “good”. It is clear that the error limits quoted in Table 1 as “acceptable” hardly possessed any predictive value – they only could have been of some help in the interpretation of general features of spectra. On the other hand, the situation was different with the “good” theoretical results: In a number of cases it has been shown that results of such accuracy have lead to reliable predictions of spectra not observed before.| Quantity | Acceptable (1976) | Good (1976) | State of the art (1995) | State of the art (2014) |
|---|---|---|---|---|
| a r ≡ equilibrium bond length; θ ≡ equilibrium bond angle; ΔE ≡ vertical electronic transition energy; D ≡ dissociation energy; I ≡ ionization potential; ω ≡ vibrational frequency; μ ≡ dipole moment; f ≡ oscillator strength.b ≡ stretching frequency.c ≡ bending frequency. For further explanation see the text. | ||||
| r, Δr | 0.05 Å | 0.01 Å | 0.01 Å | 0.01 Å |
| θ | 5 degrees | 1 degrees | 1–2 degrees | |
| ΔE, D, I | 0.5 eV | 0.1 eV | 0.05 eV | 0.05 eV |
| ω | 250 cm−1 | 50 cm−1 | 100–200,b 50c cm−1 | 10b cm−1 |
| μ | 0.5 D | 0.1 D | ||
| f | 250% | 50% | ||
About twenty years ago (i.e. in 1995) one of the authors of the present study showed in a review paper51 another table with the accuracy of ab initio results for a number of triatomic molecules involving the atoms from the first three periods of the Periodic table. It is included in Table 1 in the column “state of the art (1995)”. The results were obtained in the eighties and nineties of the preceding century, and concerned the pairs of lowest-lying electronic species correlating with a degenerate (Π or Δ) electronic state at the linear molecular geometry. The calculations of the one-dimensional potential energy curves have been performed employing the atomic orbital basis sets involving s and p functions for hydrogen atoms, and s, p, and d functions for heavier atoms. Only in some cases f-type basis functions have been used. A typical number of contracted Gaussian basis functions employed in these calculations was, say, 50. The final results were obtained by means of quite modest multi-reference (single and) double excitation configuration interaction method (MRDCI) calculations. As seen from Table 1, the overall accuracy was comparable to that qualified by Ramsay as “good”.
Nowadays the ab initio calculations are carried out at a much higher level of sophistication, i.e. by employing tremendously more computer and human time (last column in Table 1). In the first of the two ab initio studies we will discuss below, published in 1999 (Table 3), only the oxygen atom was described by 80/109, and in the second one from 2010 (Table 7) even with 145 contracted Gaussians. At the first glance the accuracy of the results is, except for the vibrational frequencies, the same as before. However, it should be noted that the error margins for the electronic energies quoted in the last column concern great number of electronic states, many of them being highly excited.
The content of Table 1 justifies the strategy we apply at assignment of our spectral results and their use for determination of the plasma parameters, like the temperature and electron number density. In any case when we have at disposal the results of direct high-resolution spectroscopic measurements, like positions of spectral lines/bands, we use them to assign our spectra. The reason for that is not only that these results are more accurate than their ab initio counterparts: Even if the theoretical results for e.g. electronic energy differences and vibrational frequencies were exact, it would not be easy to assign with help of them unresolved structure of our molecular bands, where we only can identify the (sub-)band heads. We have similar situation with the rotational constants and equilibrium bond lengths. Although these quantities are not directly measured, their precise values can (in diatomic molecules) be easily determined based on the rotationally resolved spectra. On the other hand, the ab initio computed rotational constants are less accurate, because of their quadratic dependence on the equilibrium bond lengths, the latter quantities not yet being computed accurately enough by ab initio methods. On the contrary, there are some quantities that are easier to calculate than to measure. Such is, e.g. the electric transition moment, and particularly its dependence on the instantaneous bond length. If we have accurate potential energy curves and equilibrium bond lengths (and these quantities can be extracted from experimental findings) it is then easy, e.g. as described in Subsection 4.4.3, to compute accurate intensity distributions.
5. Spectrum of MgO
5.1. Spectral systems of MgO
Selected data for MgO, taken from HH,56 are presented in Table 2.| State | Te (cm−1) | ωe (cm−1) | ωexe (cm−1) | re (Å) | Observed transitions | v00 (cm−1) |
|---|---|---|---|---|---|---|
| G1Π | [40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 259.8] 259.8] |
[1.834] | G → A | 36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3654 3654 |
||
| G → X | 39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8686 8686 |
|||||
| F1Π | (37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 922) 922) |
[696] | [1.7728] | F → X | 37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8791 8791 |
|
| E1Σ+ | (37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 722) 722) |
[705] | [1.829] | E → A | 34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 180 180 |
|
| E → X | 37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6835 6835 |
|||||
| C1Σ− | 30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 080.6 080.6 |
632.4 | 5.2 | [1.8729] | C → A | 26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500.94 500.94 |
| e3Σ− | (e ← a) | |||||
| D1Δ | 29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 851.6 851.6 |
632.5 | 5.3 | 1.8718 | D → A | 26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 272.04 272.04 |
| d3Δi | (29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300) 300) |
(650) | (1.87) | (d ↔ a) | 26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 867 867 |
|
| c3Σ+ | (28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300) 300) |
(c ← a) | 25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 900 900 |
|||
| B1Σ+ | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 984.0 984.0 |
824.08 | 4.76 | 1.7371 | B → A | 16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500.29 500.29 |
| B ↔ X | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 003.57 003.57 |
|||||
| A1Π | 3563.3 | 664.44 | 3.91 | 1.8640 | ||
| a3Πi | (2400) | (650) | (1.87) | |||
| X1Σ+ | 0 | 785.06 | 5.18 | 1.7490 |
In the book by PG57 there are data about five spectral systems of MgO, namely of a strong green, a weaker red, and several ultra-violet systems. The red system, consisting of single-headed bands degraded to the violet, appears in the wavelength range 690–470 nm and is assigned to the B1Σ+–A1Π electronic transition. The data were taken from ref. 58–60. The information about the green system, assigned to B1Σ+–X1Σ+, was taken from Mahanti58 and Lagerqvist.61 This spectrum appears in standard sources like arc and flames, but also in the sun-spots. The bands are embedded in the wavelength region 521–476 nm. The system is dominated by a very marked (0, 0) sequence and the bands are degraded to the violet. The data about the violet systems of MgO, appearing between 396 and 364 nm originate from several studies.62–66 Two systems of red-degraded bands C1Σ−–A1Π and D1Σ−–A1Π, and a violet-degraded 3Δ–3Π system were detected. Besides, the identification of three other red-degraded ultra-violet systems appearing at 265.2 nm (E1Σ+–X1Σ+), 263.7 nm (F1Π–X1Σ+), and 255.6 nm (G1Π–X1Σ+) was reported.67–69 A violet-degraded band, assigned to G1Π–A1Π, was detected at 275.01 nm.
Newer experimental studies, including optical spectroscopy, laser-magnet resonance, laser-induced fluorescence, two-color resonance-enhanced two-photon ionization studies, and vibrationally resolved photoelectron spectroscopy70–82 have been cited in the comprehensive theoretical study by Maatouk et al.83
5.2. Quantum chemical studies on MgO
The MgO molecule has been subject of several ab initio studies.75,83–96 We shall rely our discussion on the most exhaustive one, carried out by Maatouk et al.83 These benchmark electronic structure calculations were performed using the MOLPRO program suite.97 The procedure involved complete active self-consistent field (CASSCF)98 approach with the cc-pV5Z atomic orbital basis sets,99,100 followed by the internally contracted multi-reference configuration interaction (MRCI) method.101,102 The potential energy curves, electric transition moments, and spin–orbit matrix elements were computed for a large number of singlet, doublet, and quintet electronic states. Selected results of these calculations are presented in Table 3. They are compared with their experimentally derived counterparts, in order to present to the reader the state of the art of modern ab initio computations.| State | Te (cm−1) | (Te)exp (cm−1) | ωe (cm−1) | (ωe)exp (cm−1) | ωexe (cm−1) | (ωexe)exp (cm−1) | re (Å) | (re)exp (Å) |
|---|---|---|---|---|---|---|---|---|
| a Ref. 56, and references therein.b Ref. 80.c Ref. 79.d Ref. 82.e Ref. 72.f Ref. 103.g Ref. 70.h Ref. 74.i Ref. 73.j Ref. 77.k Ref. 81.l Ref. 71. | ||||||||
| 33Δ | 52![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 321 321 |
340.8 | 1.85 | 2.367 | ||||
| 33Σ− | 51![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 748 748 |
337.5 | 2.03 | 2.356 | ||||
| 21Σ− | ∞ | |||||||
| 41Π | 44![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 987.9 987.9 |
891.8 | 1.31 | 2.172 | ||||
| 15Π | 41![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 390.0 390.0 |
141.2 | 5.42 | 2.577 | ||||
| G1Π | 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 364.1 364.1 |
40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 259.8a 259.8a |
621.4 | 2.59 | 1.869 | 1.834a | ||
| 21Δ | 39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 173.6 173.6 |
601.3 | 85.71 | 2.650 | ||||
| E1Σ+ | 39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 113.1 113.1 |
37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 722a 722a |
698.1 | 705a | 10.95 | 4.18b | 1.837 | 1.829a |
37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 719b 719b |
714.2b | 1.83b | ||||||
| 33Π | 38![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 050.9 050.9 |
39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 967c 967c |
880.5 | 59.25 | 1.921 | |||
| F1Π | 37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 322.6 322.6 |
37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 922a 922a |
699.2 | 696a | 5.12 | 1.786 | 1.772a | |
37![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 919c 919c |
705c | 4.5c | 1.766c | |||||
| 711d | 6.9d | 1.77d | ||||||
| 23Σ− | 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 520.3 520.3 |
798.4 | 28.95 | 1.991 | ||||
| e3Σ− | 30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 076 076 |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 250a 250a |
∞ | ∞a | ||||
| C1Σ− | 29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 516.1 516.1 |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 080.6a 080.6a |
626.9 | 632.4a | 4.19 | 5.2a | 1.886 | 1.873a |
| D1Δ | 29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 228.2 228.2 |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 851.6a 851.6a |
625.1 | 632.5a | 4.27 | 5.3a | 1.886 | 1.8718a |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 835.4e 835.4e |
631.6e | 5.2e | 1.8606e | |||||
| d3Δ | 28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 930.5 930.5 |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300a 300a |
653.5 | 650a | 4.34 | 1.875 | 1.87a | |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 466.2e 466.2e |
655.2e | 4.9e | 1.8710e | |||||
| 23Π | 28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 218.4 218.4 |
283.4 | 1.61 | 2.799 | ||||
| c3Σ+ | 27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 703.0 703.0 |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300a 300a |
642.4 | 4.60 | 1.880 | |||
| B1Σ+ | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 332.7 332.7 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 984.0a,f,j 984.0a,f,j |
808.2 | 824.08a | 3.79 | 4.76a | 1.753 | 1.737a |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 982.6g 982.6g |
||||||||
| b3Σ+ | 7726.6 | 673.7 | 4.37 | 1.807 | ||||
| A1Π | 3078.5 | 3563.3af | 654.3 | 664.4a | 4.03 | 3.91a | 1.879 | 1.864a |
| 3561.9g | ||||||||
| 3563.8377h | 664.3929h | 3.9293h | 1.864325h | |||||
| 3560.1i | 664.3i | 3.8i | 1.8636i | |||||
| 3563j | 664.4765j | 3.9264j | ||||||
| 3558.50124k | 664.4360k | 3.92853k | ||||||
| a3Π | 1645.4 | 2400a | 644.8 | 650a | 5.3 | 1.885 | 1.87a | |
| 2492.5c | 691.1c | 4.0c | ||||||
| 2623g | 648g | 3.9g | ||||||
| 648.3l | 3.9l | |||||||
| 2620.6i | 650.2i | 4.2i | 1.8687i | |||||
| 2618.9453k | 650.18028k | 4.2k | ||||||
| X1Σ+ | 0 | 0 | 769.0 | 785.06af | 4.45 | 5.18af | 1.766 | 1.749a |
| 0 | 785.2183j | 5.1327j | ||||||
| 0 | 785.14g | 5.07g | ||||||
| 0 | 785.262621k | 5.12379k | ||||||
We consider here the results for the X1Σ+ and B1Σ+ electronic states (and the neighboring species), being involved in the electronic transition we shall discuss below.
In the ground electronic state, X1Σ+, and not far from the equilibrium geometry [F–C region], the MgO molecule has two dominating electronic configurations, … 5σ26σ12π47σ1 and … 5σ26σ22π4. At large MgO distances, the latter one becomes predominating. In the F–C region, the lowest-lying excited electronic states (embedded only about 0.2–0.4 eV above the ground state) of MgO are a3Π and A1Π, both of them corresponding to the… 5σ26σ22π37σ1 electronic configuration. All three electronic species are strongly mixed by rotational or spin–orbit couplings. The next two excited states are b3Σ+ and B1Σ+. The b3Σ+ state has… 5σ26σ12π47σ1 as the dominant configuration, while the B1Σ+ state, with the vertical energy of about 2.5 eV (corresponding roughly to 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1) is in the F–C region dominated by the same electronic configurations as the ground state. Consequently, the equilibrium bond lengths and vibrational frequencies are similar in the B1Σ+ and X1Σ+ states, but these spectroscopic parameters are quite different from their counterparts in the other electronic species mentioned. Upon enlarging bond length, the B1Σ+ electronic state is continuously more dominated by the electronic configurations… 5σ26σ22π4 and … 5σ26σ22π26σ2, and thus at these geometries it differs considerably from the X1Σ+ state. Because of that, the electric transition moment between these two species shows a strong dependence on the bond length, as seen in Fig. 5 of ref. 86. The other electronic states of MgO lie in the F–C region at considerably higher energies (>3.5 eV).
000 cm−1) is in the F–C region dominated by the same electronic configurations as the ground state. Consequently, the equilibrium bond lengths and vibrational frequencies are similar in the B1Σ+ and X1Σ+ states, but these spectroscopic parameters are quite different from their counterparts in the other electronic species mentioned. Upon enlarging bond length, the B1Σ+ electronic state is continuously more dominated by the electronic configurations… 5σ26σ22π4 and … 5σ26σ22π26σ2, and thus at these geometries it differs considerably from the X1Σ+ state. Because of that, the electric transition moment between these two species shows a strong dependence on the bond length, as seen in Fig. 5 of ref. 86. The other electronic states of MgO lie in the F–C region at considerably higher energies (>3.5 eV).
5.3. B1Σ+ → X1Σ+ system of MgO
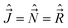 , and the isomorphic rotation Hamiltonian ((24)/(25)) reduces to
, and the isomorphic rotation Hamiltonian ((24)/(25)) reduces to
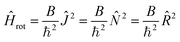 | (67) |
This Hamiltonian is analogous to the angular part of the Hamiltonian for the hydrogen atom. In this case the rotation wave functions, whose general form is |JΩM〉, reduce to |J0M〉 [the quantum number Ω(=K) = Λ + Σ equals in the present case zero], i.e. to spherical harmonics. The eigenvalues (in cm−1) of the Hamiltonian (67) are
| ṽ = BN(N + 1) = BJ(J + 1). | (68) |
The rotation levels are 2N + 1 degenerate because the wave functions depend on the quantum number M too, taking for a given N all integer values between −N and N. In Σ states the effect of the spatial inversion on the electronic wave functions is the same as that of the reflection in the planes involving the molecular axis, E∗Σ± = σvΣ± = ±Σ±. The parity of rotational levels is (−1)J, i.e. in 1Σ+ states the overall parity of the levels with even J is positive, whereas the levels with odd J have negative parity. Consequently, all the levels of a 1Σ+ state are e-levels.
The selection rule for rotational transitions when both electronic states are of 1Σ symmetry is J′ = J′′ + 1 (“R branch”) and J′ = J′′ − 1 (“P branch”). Let us take J′′ = J. When the effects of anharmonicity can be neglected, then we have for the R branch J′ = J + 1, and the term values are
| ṽ = ṽ0 + F′(J′) − F′′(J′′) = ṽ0 + B′(J + 1)(J + 2) − B′′J(J + 1) = ṽ0 + 2B′ + (3B′ − B′′)J + (B′ − B′′)J2, | (69) |
| ṽ = ṽ0 + F′(J′) − F′′(J′′) = ṽ0 + B′(J − 1)J − B′′J(J + 1) = ṽ0 − (B′ + B′′)J + (B′ − B′′)J2. | (70) |
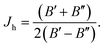 | (71) |
Replacing in eqn (70) J with Jh given by eqn (71), we obtain for the term difference between the bad head and the (real or extrapolated) band origin
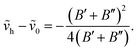 | (72) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 950–24
950–24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1). In this narrower wavelength range we recorded a series of spectra corresponding to different time delays with respect to the beginning of the PEO process. A typical spectrum is displayed in Fig. 2. It appears as a broad peak with clearly pronounced structure. The most intense sub-peak is at 19
000 cm−1). In this narrower wavelength range we recorded a series of spectra corresponding to different time delays with respect to the beginning of the PEO process. A typical spectrum is displayed in Fig. 2. It appears as a broad peak with clearly pronounced structure. The most intense sub-peak is at 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 976 cm−1, and the other sub-peaks are blue-shifted with respect to it. It turned out that the overall intensity of this broad peak significantly varied with time, but relative intensities of local peaks within it showed quite small variations. The results for relative intensity of the local peaks we used were obtained by averaging over about 30 recorded spectra. An inspection of the data collected by HH56 and PG57 indicated that these bands could belong to the B1Σ+ → X1Σ+ spectral system of MgO.
976 cm−1, and the other sub-peaks are blue-shifted with respect to it. It turned out that the overall intensity of this broad peak significantly varied with time, but relative intensities of local peaks within it showed quite small variations. The results for relative intensity of the local peaks we used were obtained by averaging over about 30 recorded spectra. An inspection of the data collected by HH56 and PG57 indicated that these bands could belong to the B1Σ+ → X1Σ+ spectral system of MgO.
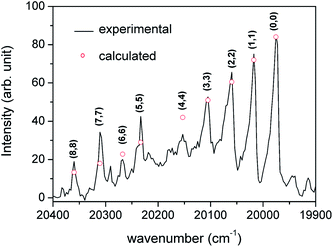 | ||
| Fig. 2 A part of the emission spectrum recorded during PEO of Mg. Anode luminescence contribution is subtracted. The peaks are assigned to (v′, v′′) bands of the B1Σ+ → X1Σ+ band system of MgO. Circles denote intensities of peaks obtained in the simulation procedure described in text.52,104 | ||
The most important experimental studies on the B1Σ+–X1Σ+ spectral system of MgO were discussed in two of our previous studies.52,104 We repeat here the key points.
Ghosh et al.105 recorded about thirty rotationally unresolved violet-degraded emission bands in the wave number region between 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1 and 21
000 cm−1 and 21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1 and assigned them to the sequences v′–v′′ = 0, ±1, with the vibrational quantum number v up to ten. Interestingly, neither HH nor PG cited this important reference. Mahanti58 and Lagerqvist and Uhler60,103 carried out a vibrational and rotational analysis of the bands of this system and assigned it to B1Σ+–X1Σ+. This analysis was confirmed by Pešić,64,106 who measured isotopic 24MgO18/26MgO16 shifts. In several studies the rotational constants for the X1Σ+ and B1Σ+ states were precisely determined (Table 3). It has been found that they are very similar in these two electronic states, that for the upper state being slightly larger. Similarity of the equilibrium bond lengths and vibrational frequencies, as well as the fact that the B–X system involves the 1Σ species, determines the general features of the spectrum. It is dominated by the v′ − v′′ = 0 band sequence, the bands have P and R branches, and the head of each P branch is quite far from the corresponding band origin. The position of the band heads (ṽh) with respect to the band origins (ṽ0) can be estimated using the rotational constants B′′ = 0.5743 cm−1 and B′ = 0.5822 cm−1.56 By means of the formula (72) we obtain ṽh − ṽ0 ≅ 40 cm−1.
000 cm−1 and assigned them to the sequences v′–v′′ = 0, ±1, with the vibrational quantum number v up to ten. Interestingly, neither HH nor PG cited this important reference. Mahanti58 and Lagerqvist and Uhler60,103 carried out a vibrational and rotational analysis of the bands of this system and assigned it to B1Σ+–X1Σ+. This analysis was confirmed by Pešić,64,106 who measured isotopic 24MgO18/26MgO16 shifts. In several studies the rotational constants for the X1Σ+ and B1Σ+ states were precisely determined (Table 3). It has been found that they are very similar in these two electronic states, that for the upper state being slightly larger. Similarity of the equilibrium bond lengths and vibrational frequencies, as well as the fact that the B–X system involves the 1Σ species, determines the general features of the spectrum. It is dominated by the v′ − v′′ = 0 band sequence, the bands have P and R branches, and the head of each P branch is quite far from the corresponding band origin. The position of the band heads (ṽh) with respect to the band origins (ṽ0) can be estimated using the rotational constants B′′ = 0.5743 cm−1 and B′ = 0.5822 cm−1.56 By means of the formula (72) we obtain ṽh − ṽ0 ≅ 40 cm−1.
In Table 4 (Exp. (1)) we present the positions of the band heads of the v′–v′′ = 0 sequence measured by Ghosh et al.105 The term values relative to the position of the (0–0) band are given in parentheses below the absolute term values. Like in all later studies, these bands were fitted to the formula of type (50), quadratic in the vibrational quantum number. The parameters derived by adapting the original formula by Ghosh et al. to the form (50), as well as the term values computed by means of it, are presented in column Fit 1 of Table 4. The experimental results by Lagerqvist,60 and Pešić106 (for 24Mg18O) are given in columns Exp. 2 and Exp. 3, respectively, and those taken by Pearse and Gaydon57 from the original references by Mahanti58 and Lagerqvist60 in column Exp. 4. Pešić106 fitted the position of both band origins and heads (columns Fit 2 and Fit 3, respectively) using the parameters given on the top of column Fit 3 for origins, and a set of parameters corrected to account for the difference ṽh − ṽ0 for calculating the positions of the band heads. In column Fit 4 are presented the results of calculations of band origins by means of the most reliable set of parameters, adopted by HH.56 Comparing the results of direct measurements with those obtained by calculating the band positions with the formulae of type (50) we conclude that they agree reasonably with one another only for small vibrational quantum numbers (v′ = v′′ ≤ 4). No formula quadratic in the vibrational quantum number v, applied thus far, has given a good reproduction of the measured band positions for higher v values. The explanation of this fact is simple: Looking at the differences in wave numbers of the successive bands observed (e.g. in column Exp. 4) we find that they are 42, 44, 46, 50, 51, 58, and 47 cm−1, i.e. they follow quadratic dependence only for first few terms. This fact will be kept in mind in the following discussion.
| Exp. 1a | Exp. 2b | Exp. 3c | Exp. 4d | Exp. 5e | Fit 1a | Fit 2c | Fit 3c | Fit 4e | Fit 5f | |
|---|---|---|---|---|---|---|---|---|---|---|
| a Ref. 105.b Ref. 60.c Ref. 106.d Ref. 57.e Ref. 56.f Our study, Ref. 52 and 104. | ||||||||||
| ṽ′′ | 721.96 | 758.38 | 785.06 | |||||||
| (ωexe)′′ | 5.96 | 4.83 | 5.18 | |||||||
| Te | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 950.23 950.23 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 983.96 983.96 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 984.0 984.0 |
|||||||
| ṽ′ | 754.06 | 796.08 | 824.08 | |||||||
| (ωexe)′ | 3.06 | 4.44 | 4.76 | |||||||
| v′–v′′ | ṽh | ṽh | ṽh | ṽh | ṽh | ṽh | ṽh | ṽ0 | ṽ0 | ṽ0 |
| 0–0 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 966 966 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 965 965 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 967 967 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 971 971 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 976 976 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 967 967 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 966 966 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 003 003 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 004 004 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 004 004 |
| (0) | (0) | (0) | (0) | (0) | (0) | (0) | (0) | (0) | (0) | |
| 1–1 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 007 007 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 007 007 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 008 008 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 013 013 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 018 018 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 005 005 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 008 008 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 241 241 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 044 044 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 044 044 |
| (41) | (42) | (41) | (42) | (42) | (38) | (41) | (38) | (40) | (40) | |
| 2–2 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 049 049 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 051 051 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 049 049 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 057 057 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 060 060 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 049 049 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 049 049 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 081 081 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 084 084 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 084 084 |
| (83) | (86) | (82) | (86) | (84) | (82) | (83) | (78) | (81) | (81) | |
| 3–3 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 093 093 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 097 097 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092 092 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 103 103 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 106 106 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 098 098 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092 092 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 121 121 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 126 126 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 127 127 |
| (127) | (132) | (125) | (132) | (130) | (131) | (125) | (118) | (122) | (123) | |
| 4–4 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 146 146 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 137 137 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 153 153 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 153 153 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 153 153 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 134 134 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 162 162 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 168 168 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 171 171 |
|
| (180) | (170) | (182) | (177) | (186) | (168) | (159) | (164) | (167) | ||
| 5–5 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 204 204 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 231 231 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 215 215 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 203 203 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 211 211 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 216 216 |
|||
| (234) | (233) | (255) | (248) | (200) | (208) | (213) | ||||
| 6–6 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 257 257 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 262 262 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 268 268 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 281 281 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 245 245 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 255 255 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 265 265 |
|||
| (291) | (291) | (292) | (314) | (243) | (252) | (261) | ||||
| 7–7 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 304 304 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 309 309 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 310 310 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 354 354 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 289 289 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 316 316 |
|||
| (338) | (338) | (334) | (387) | (286) | (297) | (312) | ||||
| 8–8 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 347 347 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 360 360 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 433 433 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 333 333 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 346 346 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 372 372 |
||||
| (381) | (384) | (466) | (330) | (342) | (368) | |||||
| 9–9 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 388 388 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 517 517 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 377 377 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 393 393 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 434 434 |
|||||
| (422) | (550) | (374) | (389) | (430) | ||||||
The results of our measurements104 are presented in column Exp. 5. The accuracy of the band head positions is estimated to ±5 cm−1. The agreement with the results of previous more precise gas-phase spectral measurements is within this error margin.
| V(X1Σ+) = 0.111991x2 − 0.07426x3, V(B1Σ+) = 0.0910532 + 0.12331(x + 0.0225)2 − 0.07844(x + 0.0225)3, | (73) |
| Re (au) = −1.2 + 0.7x. | (74) |
The vibrational Schrödinger equation corresponding to the potentials (73) was solved variationally, with the basis consisting of eigenfunctions of the harmonic oscillator approximating the X1Σ+ state.
The results for the band origins obtained in these calculations are presented in column Fit 5 of Table 4. For low vibrational quantum numbers (v′ = v′′ = 0–3) they coincide with the numbers in column Fit. 4, generated employing the formula of type (50) with the same set of molecular parameters. The agreement becomes continuously poorer with increasing v′ = v′′ ≥ 4, reflecting the restricted reliability of the perturbative approach used to determine the force constants that appear in the formula (73). However, as stated above, the levels with v′ ≥ 4 are in any case unsatisfactorily described by the formulae of type (50) and they were not used for estimation of the plasma temperature.
The computed FCFs and (squared) VTMs (in atomic units) for the levels up to v = 4 are given in Table 5. For comparison, we give the FCFs computed by Prasad and Prasad107 and those quoted by Ikeda et al.70 Our results either agree well with those from the previous studies or lie between the values published in ref. 70 and 107. The ratio |VTM|2/FCF decreases uniformly with increasing vibrational quantum number within the v′–v′′ = 0 sequence, reflecting the decrease of the absolute value of the electric transition moment with increasing bond length.
| v′′ | v′ | ||||
|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | 4 | |
| a Ref. 107.b Ref. 70. | |||||
| 0 | 0.9826 | 0.0170 | 0.0004 | ||
| 0.983a | 0.017a | 0.000a 0.0003b | |||
| 1.421 | 0.0089 | 0.0002 | |||
| 1 | 0.0173 | 0.9464 | 0.0351 | 0.0011 | |
| 0.017a | 0.948a | 0.033a 0.0375b | 0.001a | ||
| 0.0480 | 1.344 | 0.0180 | 0.0001 | ||
| 2 | 0.0364 | 0.9067 | 0.0544 | 0.0024 | |
| 0.035a | 0.911a 0.901b | 053a 0.0576b | 0.0018b | ||
| 0.0969 | 1.263 | 0.0274 | 0.0011 | ||
| 3 | 0.0002 | 0.0573 | 0.8632 | 0.0751 | |
| 0.000a | 0.053a 0.0606b | 0.881a 0.857b | 0.0804b | ||
| 0.0017 | 0.1471 | 1.179 | 0.0371 | ||
| 4 | 0.0005 | 0.0801 | 0.8154 | ||
| 0.0010b | 0.061a 0.0827b | 0.806b | |||
| 0.0039 | 0.1981 | 1.090 | |||
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 K. In Fig. 3b we also present the result obtained when the |VTM|2 are replaced by the corresponding FCFs. In this case we obtained T ≈ 9800 K. The difference between these two temperature values points at the importance of taking into account the variation of the electric transition moment with the bond length. Accounting for the limited accuracy of our experimental results, as well as of the electric transition moment function employed, we estimated the temperature of our plasma to be T = 11
500 K. In Fig. 3b we also present the result obtained when the |VTM|2 are replaced by the corresponding FCFs. In this case we obtained T ≈ 9800 K. The difference between these two temperature values points at the importance of taking into account the variation of the electric transition moment with the bond length. Accounting for the limited accuracy of our experimental results, as well as of the electric transition moment function employed, we estimated the temperature of our plasma to be T = 11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 ± 2000 K. Assuming T = 11
000 ± 2000 K. Assuming T = 11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 K and using the vibrational transition moments for v′ = v′′ = 0–8, we simulate in Fig. 2 the complete v′–v′′ = 0 band sequence of the B1Σ+–X1Σ+ spectral system of MgO. While some discrepancies between the experimental and simulated results are obvious, the general agreement can be considered as satisfactory.
000 K and using the vibrational transition moments for v′ = v′′ = 0–8, we simulate in Fig. 2 the complete v′–v′′ = 0 band sequence of the B1Σ+–X1Σ+ spectral system of MgO. While some discrepancies between the experimental and simulated results are obvious, the general agreement can be considered as satisfactory.
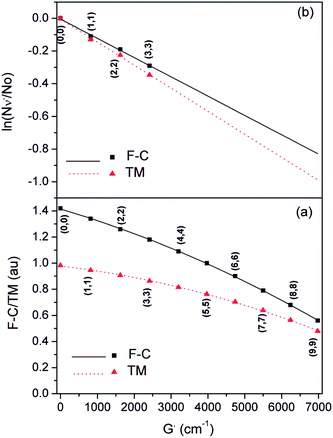 | ||
| Fig. 3 (a) Computed FCFs (F–C) and squared VTMs (TM) for the (v′ = v′′) bands of the B1Σ+ → X1Σ+ band system of MgO as functions of vibrational term values of the B1Σ+ electronic state; (b) logarithm of the relative population of v′ = 0, 1, 2 and 3 vibrational levels as function of the corresponding term values.52,104 | ||
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 and 33
000 and 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1 we recorded four groups of unresolved rotational lines with the maxima at 32
000 cm−1 we recorded four groups of unresolved rotational lines with the maxima at 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 364, 32
364, 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 484, 32
484, 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 597, and 32
597, and 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 622 cm−1. They were assigned to the heads of the Q2, Q1, R2, and R1 sub-branches corresponding to the A2Σ+ (v′ = 0)–X2Π (v′′ = 0) transition (Fig. 4). Employing the approach proposed by de Izarra,108 we estimated the temperature of 3500 ± 500 K. The significant difference between this temperature and that referred in Subsection 5.3.3 could be an indication for non-existence of thermal equilibrium between vibrational and rotational degrees of freedom in our plasma. However, as discussed in ref. 52, it can also be explained in terms of the two-plasma-zones model, that will be briefly discussed in Conclusions.
622 cm−1. They were assigned to the heads of the Q2, Q1, R2, and R1 sub-branches corresponding to the A2Σ+ (v′ = 0)–X2Π (v′′ = 0) transition (Fig. 4). Employing the approach proposed by de Izarra,108 we estimated the temperature of 3500 ± 500 K. The significant difference between this temperature and that referred in Subsection 5.3.3 could be an indication for non-existence of thermal equilibrium between vibrational and rotational degrees of freedom in our plasma. However, as discussed in ref. 52, it can also be explained in terms of the two-plasma-zones model, that will be briefly discussed in Conclusions.
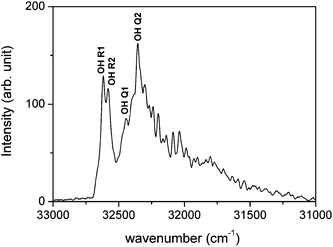 | ||
Fig. 4 A2Σ+ (v′ = 0)–X2Π (v′′ = 0) luminescence spectrum of OH between 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 and 33 000 and 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1.52,104 000 cm−1.52,104 | ||
6. Spectrum of AlO
6.1. Spectral systems of AlO
The part of the data for AlO we need below, as collected by HH,56 is presented in Table 6. HH constructed this table based on about 50 studies published from 1927 to 1975. All spectra observed until 1975 involved doublet electronic states.| State | Te (cm−1) | ωe (cm−1) | ωexe (cm−1) | re (Å) | Observed transitions | v00 (cm−1) |
|---|---|---|---|---|---|---|
| F2Σ+ | [47![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 677.3] 677.3] |
[1.8164] | F → A | 41![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 843.52 843.52 |
||
41![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 972.36 972.36 |
||||||
| E2Δi | 45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 562 562 |
(503) | [1.8444] | E ↔ A | 39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 979.81 979.81 |
|
45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 431 431 |
39![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 977.17 977.17 |
|||||
| D2Σ+ | 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 266.7 266.7 |
819.6 | 5.8 | 1.7234 | (D → B) | (19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 552) 552) |
| D ↔ A | 34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 841.23 841.23 |
|||||
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 970.09 970.09 |
||||||
| D↔X | 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 187.2 187.2 |
|||||
| C2Πr | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 153 153 |
856 | 6 | (C → B) | (12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 457) 457) |
|
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 079 079 |
(12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 383) 383) |
|||||
| C ↔ X | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092 092 |
|||||
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 018 018 |
||||||
| B2Σ+ | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 688.95 688.95 |
870.05 | 3.52 | 1.6670 | B ↔ X | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 635.22 635.22 |
| A2Πi | 5470.6 | 728.5 | 4.15 | [1.7708] | A ↔ X | 5346 |
| 5341.7 | 5217 | |||||
| X2Σ+ | 0 | 979.23 | 6.97 | 1.6179 |
PG57 gave information about six spectral systems of AlO. The green B2Σ+–X2Σ+ system, appearing in the wavelength range 541–433 nm, is described in most detail. It was stated that it occurred in a variety of sources including arcs and flames, and that it appeared in form of marked sequences of red-degraded single-headed bands. PG presented twenty-one band heads taken from Lagerqvist et al.109 and Tyte and Nicholls.110 Five ultra-violet spectral systems of AlO were briefly described in the book by PG. The information about them was based on ref. 111–116. These systems occur in emission from arcs, hollow-cathode discharges, and microwave excitation. The bands of the C2Π–X2Σ+ (extended from 332 to 287 nm), E2Σ+–A2Π (around 250 nm), D2Σ+–X2Σ+ (280–230 nm), and F2Π–A2Π (at 238.0 nm) systems are degraded to the red, and are double-headed, except of the F2Π–A2Π, which are double double-headed. The bands of the D2Σ+–A2Π system (300–280 nm) are double-headed and degraded to the violet.
Comparing the data collected by HH and PG, we state that HH included in their book the A2Σ+–X2Σ systems in the (infra-red) region at about 1900 nm, only indirectly mentioned by PG. The reason might be that the first ref. 117 used by HH appeared between third and fourth edition of PG's book, and the second one118 even later.
The third comprehensive source of literature data on the spectra of AlO represents the theoretical paper by Zenouda et al.119 The electronic ground state was well characterized by infra-red optical double resonance spectroscopy,120 by purely rotational transitions121–123 and fine structure splitting.124 The infra-red A2Σ+–X2Σ+ system was used to determine accurate spectroscopic constants for both electronic species in question.125–128 The blue-green B2Σ+–X2Σ+ system was investigated in ref. 128–135. Precise rotational constants were determined132 for many vibrational levels. A series of studies were devoted to the C2Σ+–X2Σ+ system.136–141 The systems D2Σ+–X2Σ+,136 C2Π–A2Π,137,138 D2Σ+–A2Π,139 and E2 Δ–A2Π136 were also investigated. Low-lying electronic states of AlO were studied by photoelectron spectroscopy, too.142,143 Potential energy functions for the X2Σ+, A2Π, X2Σ+, B2Σ+, and D2Σ+ states and FCFs for the corresponding transitions were computed by means of the RKR method.133,144,145
6.2. Quantum chemical studies on AlO
The early ab initio calculations on the AlO molecule146–151 were carried out employing similar approaches and presented the results of similar type (equilibrium geometries, vibrational frequencies, excitation energies, transition moments) as their MgO counterparts.75,82–96 We skip immediately to the benchmark study by Zenouda et al.119 Like the study by Maatouk et al.83 on MgO, it was carried out using the MOLPRO program package97 in its somewhat older performance. The computations were performed at the CASSCF and the MRCI level of sophistication, involving basis sets of quintuple zeta quality.99 The potential curves and electric dipole/transition moments for the ground state and all doublet and quartet excited states up to 50![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1 were calculated. We concentrate on the states we are interested in, namely on X2Σ+, B2Σ+, and C2Π.
000 cm−1 were calculated. We concentrate on the states we are interested in, namely on X2Σ+, B2Σ+, and C2Π.
The completely populated 1s, 2s, 2p AOs of Al and 1s, 2s of O build the molecular orbitals (MOs) 1σ–5σ, and 1π of AlO. These orbitals are found to be completely populated in all Slater determinants significantly contributing to the electronic states computed in the framework of the study. As expected, the most important role in building of lower-lying (i.e. up to 50![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1) states of AlO play the MOs involving the 3s, 3p AOs of Al and 2p of O. The seven AOs, 3sσ, 3pσ, 3pπ of Al and 2pσ, 2pπ of O built the next four σ and two π orbitals. It was found that the most important electron configurations are those in which seven valence electrons (three originating from the Al atom, a and four from oxygen) are distributed among the following MOs: the 6σ bonding orbital, built by the 2pσ AO of oxygen and admixtured by 3s and 3pσ of Al; the 2π orbital representing mainly the 2pπ orbital of oxygen; the non-bonding 7σ orbital being a combination of the 3s and 3p orbitals of Al; the 3π orbital built predominantly by 3pπ orbital of Al.
000 cm−1) states of AlO play the MOs involving the 3s, 3p AOs of Al and 2p of O. The seven AOs, 3sσ, 3pσ, 3pπ of Al and 2pσ, 2pπ of O built the next four σ and two π orbitals. It was found that the most important electron configurations are those in which seven valence electrons (three originating from the Al atom, a and four from oxygen) are distributed among the following MOs: the 6σ bonding orbital, built by the 2pσ AO of oxygen and admixtured by 3s and 3pσ of Al; the 2π orbital representing mainly the 2pπ orbital of oxygen; the non-bonding 7σ orbital being a combination of the 3s and 3p orbitals of Al; the 3π orbital built predominantly by 3pπ orbital of Al.
The dominant configurations for the X2Σ+ and B2Σ+ electronic states are 6σ22π47σ1 (Al2+O2−) and 6σ12π47σ2 and 6σ22π37σ13π1 (Al+O−). The two main configurations for the D2Σ+ state are 6σ22π37σ13π1 and 6σ22π48σ1. The main configuration for the A2Π state is 6σ22π37σ2. The C2Π state is strongly multiconfigurational with two dominant configurations 6σ22π43π1 and 6σ17σ12π43π1. The main configuration of the C′2Π state is 6σ22π27σ23π1. A consequence of an avoided crossing between the C and C′ states is an energy barrier of 0.68 eV above the dissociation asymptote for the C2Π state. Zenouda et al. claimed that this fact explained why excited vibrational levels up to v′ = 10 had been observed,138 even though the highest of them are located above the dissociation limit.
In the F–C region, the largest electric transition moment involving these species is that for the X2Σ+–B2Σ+ transition. It was found to be between approximately 0.7 and 0.4 a.u. in a rather broad F–C region; it decreases with increasing bond length, becoming negligible when the Al–O distance overestimates by roughly 1 Å its equilibrium value. The electric moment for the C2Π–X2Σ+ transition was found to be fairly large (0.5 a.u.), indeed like all those involving the ground and the low-lying excited doublet electronic states.
The results of the ab initio study by Zenouda et al. are compared in Table 7 with the corresponding experimental findings.
| State | Te (cm−1) | (Te)exp (cm−1) | ωe (cm−1) | (ωe)exp (cm−1) | ωexe (cm−1) | (ωexe)exp (cm−1) | re (Å) | (re)exp (Å) |
|---|---|---|---|---|---|---|---|---|
| a Ref. 114.b Ref. 136.c Ref. 115.d Ref. 140.e Ref. 111.f Ref. 132.g Ref. 127. | ||||||||
| F2Σ+ | 48![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 895 895 |
47![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 677a 677a |
850 | 1.78 | 1.816a | |||
| C′2Π | 47![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 380 380 |
770 | 2.19 | |||||
| e4Π | 47![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 320 320 |
590 | 1.79 | |||||
| E′2Σ− | 47![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 225 225 |
490 | 1.865 | |||||
| E2Δ | 46![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 250 250 |
45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 431b 431b |
496 | 503b | 1.858 | 1.844b | ||
| d4Π | 41![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 190 190 |
768 | 1.72 | |||||
| D2Σ+ | 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 685 685 |
40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 268c 268c |
833 | 817.5c | 1.726 | 1.727c | ||
| C2Π | 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 875 875 |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 108d 108d |
846 | 856e | 1.679 | 1.671d | ||
| G′2Σ− | 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 351 351 |
716 | 1.791 | |||||
| G2Δ | 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 852 852 |
719 | 1.786 | |||||
| c4Σ− | 30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 678 678 |
690 | 1.788 | |||||
| b4Δ | 29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 350 350 |
699 | 1.785 | |||||
| a4Σ+ | 27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 222 222 |
723 | 1.776 | |||||
| B2Σ+ | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 192 192 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 689f 689f |
869 | 870.44f | 4.18 | 3.668f | 1.677 | 1.667 |
| A2Π | 5050 | 5460g | 720 | 729.7g | 4.18 | 4.88g | 1.777 | 1.7678g |
| X2Σ+ | 0 | 0 | 977 | 979.5g | 6.8 | 7.08g | 1.623 | 1.6179g |
6.3. B2Σ+ → X2Σ+ spectral system of AlO
 | (75) |
Since this operator only involves the scalar products operators, the Hamiltonian is so symmetric that the SFS and MFS are equally appropriate for working. We choose the SFS to avoid any complications caused by anomalous commutation relations. We use here the Hund's case (b) basis functions (33). In view of eqn (37), each individual of them has a definite parity, being (−1)N for Σ+, and (−1)N+1 for Σ− electronic states. In this basis the first operator on the right-hand side of eqn (75) has only diagonal matrix elements equal BN(N + 1). To calculate the matrix elements of the second operator, we use the relation
 | (76) |
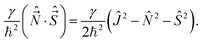 | (77) |
Also this operator is diagonal in the chosen basis. Its matrix elements are γ[J(J + 1) − N(N + 1) − S(S + 1)]/2. For each value of the quantum number N there are two close-lying levels of the same parity, corresponding to J = N ± 1/2. The J = N + 1/2 and J = N − 1/2 levels are called F1 and F2, respectively. Their term values are
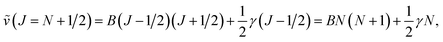 | (78) |
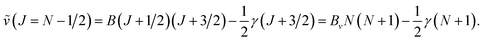 | (79) |
The magnitude of the splitting (except for the N = 0, J =± 1/2 level that is not split) is
| ṽ(J = N + 1/2) − ṽ(J = N − 1/2) = (N + 1/2)γ. | (80) |
This shows that the magnitude of the spin-rotation splitting linearly increases with N.
The selection rule for rotational transitions when both electronic states are of 2Σ symmetry is the same as for 1Σ–1Σ electronic transitions, namely J′ = J′′ + 1 (“R branch”) and J′ = J′′ − 1 (“P branch”). Additionally, we have here not strict, but very pronounced N′ = N′′ ± 1 selection rule. In the case B2Σ+ → X2Σ+ electronic transition of AlO, we have B′ < B′′ and thus red degraded bands with the head in the R-branch at (see eqn (69))
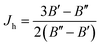 | (81) |
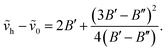 | (82) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 and 20
000 and 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1. It consisted of two broad peaks with clearly pronounced structure, extending from roughly 18
000 cm−1. It consisted of two broad peaks with clearly pronounced structure, extending from roughly 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 to 18
200 to 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 800 cm−1 and from 19
800 cm−1 and from 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 to 19
000 to 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 700 cm−1 with the maxima at approximately 18
700 cm−1 with the maxima at approximately 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 and 19
500 and 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 cm−1, respectively. Thus, they appeared in the spectral region where the appearance of the bands of the B2Σ+–X2Σ+ emission transition of AlO was expected. We estimated the uncertainty of measured spectral lines/peak maxima to be 5 cm−1, and of their relative intensities (after subtracting the anodic luminescence contribution) to roughly 10%.
500 cm−1, respectively. Thus, they appeared in the spectral region where the appearance of the bands of the B2Σ+–X2Σ+ emission transition of AlO was expected. We estimated the uncertainty of measured spectral lines/peak maxima to be 5 cm−1, and of their relative intensities (after subtracting the anodic luminescence contribution) to roughly 10%.In order to assign the observed spectral features, we constructed a Deslandres table for the B2Σ+–X2Σ+ system of AlO (Table 8). We used for that the band origins published by Saksena et al.135 Using the rotational constants from that reference, B′′ = 0.64165 and B′ = 0.60897, and eqn (81), with J replaced by N [see eqn (78)/(79) for Σ states], we estimated in the lowest-order approximation the quantum number N corresponding to the head of the R branch to be 18. The position of the band head with respect to the band origin is thus, according to eqn (82), about 12 cm−1 blue-shifted. At our resolution of the spectrum we did not observed the spin-rotation splitting, eqn (80).
| Tv′′ | Tv′ | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6350 6350 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4980 4980 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3540 3540 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2030 2030 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0440 0440 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8770 8770 |
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7040 7040 |
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5230 5230 |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3350 3350 |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1390 1390 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| v′ | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | |
| v′′ | |||||||||||
| 00 | 0 | 20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6350 6350 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4980 4980 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3540 3540 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2030 2030 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0440 0440 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8770 8770 |
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7040 7040 |
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5230 5230 |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3350 3350 |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1390 1390 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 646h 646h |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 508h 508h |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 362h 362h |
|||||||||
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 640 640 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
||||||||||
| 9650 | 1 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6700 6700 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5330 5330 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3890 3890 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2370 2370 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0780 0780 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9120 9120 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7380 7380 |
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5580 5580 |
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3690 3690 |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1740 1740 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 682h 682h |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 544h 544h |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 398h 398h |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 246h 246h |
||||||||
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 680 680 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 550 550 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 390 390 |
|||||||||
| 19170 | 2 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7180 7180 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5820 5820 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4370 4370 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2860 2860 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1270 1270 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9610 9610 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7870 7870 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6060 6060 |
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4180 4180 |
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2220 2220 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 733h 733h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 594h 594h |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 295h 295h |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 135h 135h |
||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 730 730 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 590 590 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 440 440 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 280 280 |
||||||||
| 28540 | 3 | 17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7810 7810 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6440 6440 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5000 5000 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3480 3480 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1890 1890 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0230 0230 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8500 8500 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6690 6690 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4810 4810 |
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2850 2850 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 660h 660h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 513h 513h |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 199h 199h |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 032h 032h |
||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 660 660 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 510 510 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 190 190 |
|||||||||
| 37780 | 4 | 16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8580 8580 |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7210 7210 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5770 5770 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4250 4250 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2660 2660 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1000 1000 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9260 9260 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7460 7460 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5570 5570 |
24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3620 3620 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 593h 593h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 439h 439h |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 935h 935h |
|||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 590 590 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 435 435 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 270 270 |
|||||||||
| 46870 | 5 | 15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9490 9490 |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8120 8120 |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6670 6670 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5160 5160 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3570 3570 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1910 1910 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0170 0170 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8360 8360 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6480 6480 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4530 4530 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 533h 533h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 371h 371h |
||||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 530 530 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 365 365 |
||||||||||
| 55820 | 6 | 15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0530 0530 |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9160 9160 |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7720 7720 |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6240 6240 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4620 4620 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2960 2960 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1220 1220 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9410 9410 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7530 7530 |
22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5570 5570 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 480h 480h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 310h 310h |
||||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 475 475 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 305 305 |
||||||||||
| 64630 | 7 | 14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1720 1720 |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0350 0350 |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8910 8910 |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7390 7390 |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5810 5810 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4140 4140 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2410 2410 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0600 0600 |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8720 8720 |
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6760 6760 |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 435h 435h |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 257h 257h |
||||||||||
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 430 430 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 250 250 |
||||||||||
| 73300* | 8 | 13![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3050* 3050* |
14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1680* 1680* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0240* 0240* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8730* 8730* |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7140* 7140* |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5470* 5470* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3740* 3740* |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1930* 1930* |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0050* 0050* |
20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8090* 8090* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 385 385 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 205 205 |
||||||||||
| 81830* | 9 | 12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4520* 4520* |
13![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3150* 3150* |
14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1710* 1710* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0200* 0200* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8610* 8610* |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6940* 6940* |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5210* 5210* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3400* 3400* |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1520* 1520* |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9560* 9560* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 350 350 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 160 160 |
||||||||||
| 90230* | 10 | 11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6120* 6120* |
12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4750* 4750* |
13![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3310* 3310* |
14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1800* 1800* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0210* 0210* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8540* 8540* |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6810* 6810* |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5000* 5000* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3120* 3120* |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1160* 1160* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 320 320 |
19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 115 115 |
||||||||||
| 98480* | 11 | 10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7870* 7870* |
11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6500* 6500* |
12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5060* 5060* |
13![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3550* 3550* |
14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1960* 1960* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0290* 0290* |
15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8560* 8560* |
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6750* 6750* |
17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4870* 4870* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2910* 2910* |
18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 295 295 |
|||||||||||
We completed the data from Table 3 of the original ref. 135 by computing the term values for the quantum numbers v′′ = 8–11 by means of the molecular parameters from Table 5 of that paper. The positions of the experimentally observed band heads (mean values for the R1/R2 branches) are also presented in Table 8. The results of our study match very reasonably the v′–v′′ = −1 and −2 sequences of the B2Σ+–X2Σ+ system. Moreover, we detected several bands [(v′, v′′) = (7, 8),…, (11, 12) and (6, 8),…, (10, 12); not all of them are presented in Table 8] that have not been analyzed experimentally thus far. They involve the ground state levels v′′ = 8–12 which were suspected to be heavily perturbed and/or predissociated.109,135,153
We calculated the FCFs and VTMs for the B2Σ+–X2Σ+ system of AlO in the same way as for the B1Σ+–X1Σ+ system of MgO, using a set of experimentally derived molecular parameters132 and the ab initio computed electronic transition function.119 The results of our calculations of the FCFs and squared VTMs (in atomic units) for the bands we are interested in are presented in Table 9 and Fig. 6(a). For comparison, we give in column FCFexp the FCFs computed by Londhe et al.145 The agreement between these results and ours is quite good (two slightly different sets of molecular parameters are used in these two studies).
| v′, v′′ | T | FCF | FCFexp | |VTM|2 | I | I/FCF | I/FCFexp | I/VTM |
|---|---|---|---|---|---|---|---|---|
| 0, 1 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 680 680 |
0.235 | 0.243 | 0.112 | 74 | 315 → 1.00 | 305 → 1.00 | 660 → 1.00 |
| 1, 2 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 590 590 |
0.3315 | 0.352 | 0.1545 | 81 | 244 → 0.77 | 230 → 0.75 | 524 → 0.79 |
| 2, 3 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 510 510 |
0.357 | 0.384 | 0.162 | 86 | 241 → 0.76 | 224 → 0.73 | 530 → 0.80 |
| 3, 4 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 435 435 |
0.352 | 0.373 | 0.155 | 63 | 179 → 0.57 | 169 → 0.55 | 406 → 0.62 |
| 4, 5 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 365 365 |
0.340 | 0.342 | 0.1445 | 56 | 165 → 0.52 | 164 → 0.54 | 388 → 0.59 |
| 5, 6 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 305 305 |
0.332 | 0.304 | 0.1355 | 47 | 141 → 0.45 | 155 → 0.51 | 347 → 0.53 |
| 6, 7 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 250 250 |
|||||||
| 7, 8 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 205 205 |
|||||||
| 8, 9 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 160 160 |
|||||||
| 9, 10 | 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 115 115 |
|||||||
| 0, 2 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 730 730 |
0.0354 | 0.032 | 0.0151 | 11 | 311 → 1.00 | 344 → 1.00 | 728 → 1.00 |
| 1, 3 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 660 660 |
0.0806 | 0.077 | 0.0334 | 20 | 248 → 0.80 | 260 → 0.76 | 599 → 0.82 |
| 2, 4 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 590 590 |
0.124 | 0.126 | 0.0496 | 30 | 242 → 0.78 | 238 → 0.69 | 605 → 0.83 |
| 3, 5 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 530 530 |
0.160 | 0.171 | 0.0618 | 30 | 187 → 0.60 | 175 → 0.51 | 485 → 0.67 |
| 4, 6 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 475 475 |
0.189 | 0.211 | 0.0695 | 30 | 159 → 0.51 | 142 → 0.41 | 432 → 0.59 |
| 5, 7 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 430 430 |
0.208 | 0.245 | 0.0725 | 31 | 149 → 0.48 | 127 → 0.37 | 427 → 0.59 |
| 6, 8 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 385 385 |
|||||||
| 7, 9 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 350 350 |
|||||||
| 8, 10 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 320 320 |
|||||||
| 9, 11 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 295 295 |
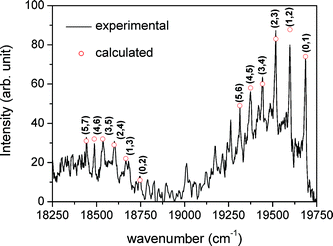 | ||
| Fig. 5 A part of the emission spectrum recorded during PEO of Al. Anode luminescence contribution is subtracted. The peaks are assigned to v′–v′′ = −1 and −2 band sequences of the B2Σ+ → X2Σ+ system of AlO. Circles denote intensities of peaks obtained in the simulation procedure described in text.152 | ||
 | ||
| Fig. 6 (a) Computed relative population of v′ = 0, 1, 2, 3, 4, and 5 vibrational levels (from left to right) of the B2Σ+ electronic state of AlO. Measured intensity distributions within recorded band progressions are combined with the FCFs (F–C) and VTMs (TM) calculated in our study 152, as well as with the FCFs (FCFexp) published in ref. 145. (b) Logarithm of the relative population of v′ = 1,…, 5 vibrational levels as function of the corresponding term values. For detailed explanation see text.152 | ||
Assuming again the existence of partial (vibrational) thermal equilibrium, we can extract from the above results the value for the mean temperature of our plasma. In Fig. 6b are displayed the values for ln(Ne′v′/Ne′0) as functions of the vibrational term values (G) of the B2Σ+ electronic state. In spite of some deviations of the points from the straight lines in Fig. 6b, one can extract quite unambiguously three temperature values: T ≈ 8000 K (FCF data), T ≈ 6800 K (FCFexp data), and T ≈ 9400 (VTM data). The differences in the F–C factors computed in the present study and those published by Londhe et al.145 cause a discrepancy between the corresponding temperatures of over 1000 K. On the other hand, when the variation of the electronic transition moment with the bond length is taken into account, the estimated temperature is almost 1500 K higher than that obtained by neglecting this dependence. If this dependence was combined with the F–C factors by Londhe et al., we would again obtain the temperature value of roughly 8000 K. Since we were not able to decide unambiguously which of these three results is the most correct one, we found it correct to conclude that the mean temperature of our plasma is T = 8000 ± 2000 K. In Fig. 5 we present the intensity distribution simulated by employing the temperature value of 8000 K and the FCFs computed in the present study (circles). Similar simulated spectrum is obtained when instead of the FCFs the VTMs combined with T = 9400 are employed.
Let us note that the above vibrational temperatures are very similar to the temperature we obtained very recently (the results are not yet published) employing several atomic lines of Al. This is an indication that the concept of partial “local thermal equilibrium” can be extended such that we can speak about “local thermal equilibrium” that involves at least vibrational and (electron) excitation modes.
6.4. C2Π → X2Σ+ system of AlO
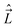 . On the other hand, the projections of
. On the other hand, the projections of 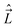 along the axes perpendicular to the molecular axis, are completely undetermined and their mean values equal zero.
along the axes perpendicular to the molecular axis, are completely undetermined and their mean values equal zero.In a 2Π state, Λ = ±1, S = 1/2, so that Σ = ±1/2. Hence Ω can take the values −3/2, −1/2, 1/2, 3/2. Except for the J = 1/2 level (which can be exclusively associated with |Ω| = 1/2) each J level has four associated rotational sublevels, two of each parity. We use the simplified rotational Hamiltonian (25) plus the spin–orbit operator (28). Thus, we neither consider the Λ-splitting, nor the spin-rotation coupling, being in the concrete case (C2Π of AlO) we handle a much weaker effect, than the spin–orbit coupling. We will express the Hamiltonian in terms of the components of angular momenta along the MFS-axes, because this coordinate system is obviously more natural when the presence of the internuclear axis plays any role. This Hamiltonian will be partitioned as Ĥ = Ĥ1 + Ĥ2, where Ĥ1 is the dominating part. Its form will depend on whether the spin–orbit coupling is so strong to force the spin to be tied to the molecular axis [Hund's case (a)], or the effect of the rotation is stronger, so that the spin is torn off the molecular axis and is freely oriented in space [Hund's case (b)]. We shall choose as the basis for the representation of the complete Hamiltonian the eigenstates of Ĥ1. Of course, the eigenvalues of the total Hamiltonian do not depend on the choice of the basis. However, if we succeed to carry out a sensible partitioning of the Hamiltonian, its matrix representation will be characterized by relatively small off-diagonal elements and the calculation of the matrix elements will be easier.
(a) Hund's case (a). When the spin–orbit coupling is strong, i.e. at |ASO|≫ B, it is natural to partition the Hamiltonian in the following way:
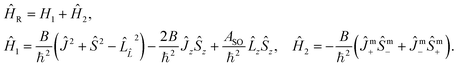 | (83) |
The complete Hamiltonian (83) commutes with the operators Ĵ2, Ŝ2, and ![[L with combining circumflex]](https://www.rsc.org/images/entities/i_char_004c_0302.gif) z, and its dominant part, Ĥ1 additionally with the z-components of the spin and total angular momentum operator, Ŝz and Ĵz, respectively. Thus we have three good quantum numbers, J, S, and Λ, and nearly good quantum numbers Σ (for Ŝz) and Ω = Λ + Σ (for Ĵz). We can use as basis functions:
z, and its dominant part, Ĥ1 additionally with the z-components of the spin and total angular momentum operator, Ŝz and Ĵz, respectively. Thus we have three good quantum numbers, J, S, and Λ, and nearly good quantum numbers Σ (for Ŝz) and Ω = Λ + Σ (for Ĵz). We can use as basis functions:
| |−3/2〉 ≡ |−1〉|S, −1/2〉|J, −3/2〉, |−1/2〉 ≡ |−1〉|S, 1/2〉|J, −1/2〉, |1/2〉 ≡ |1〉|S, −1/2〉|J, 1/2〉, |3/2〉 ≡ |1〉|S, 1/2〉|J, 3/2〉, | (84) |
The dominant part of the Hamiltonian, Ĥ1, has in the chosen basis only diagonal elements. On the other hand, the operator Ĥ2 has only the off-diagonal non-vanishing matrix elements 〈∓ 3/2|Ĥ|∓1/2〉. Thus the Hamiltonian matrix has a block structure with two identical 2 × 2 blocks, one involving the basis functions |−3/2〉 and |−1/2〉, and the other with |3/2〉 and |1/2〉. The same block-structure is obtained when instead of the basis functions (84) their parity adapted linear combinations,
 | (85) |
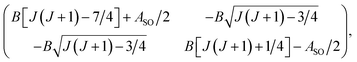 | (86) |
The eigenvalues (in cm−1) of the Hamiltonian are
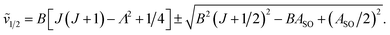 | (87) |
The + and − signs in eqn (87) correspond to the F2 and F1 levels, respectively. Their eigenfunctions are43
| |ψ(F2)〉 = aJ|2Π3/2, J, ±〉 − bJ|2Π1/2, J, ±〉, |ψ(F1)〉 = bJ|2Π3/2, J, ±〉+ aJ|2Π1/2, J, ±〉, | (88) |
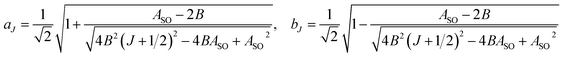 | (89) |
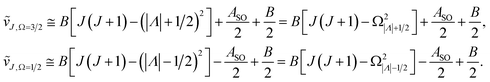 | (90) |
The term B/2, not depending on J can be neglected. Thus in the first approximation each rotation level is split into two levels separated from each other by ASO. For the “inverted” case, ASO < 0, the energy ordering of the Ω = 3/2 and Ω = 1/2 levels is reversed. When |ASO|≫B, we have aJ ≅ 1 and bJ ≅ 0 (at ASO > 0), or aJ ≅ 0 and bJ ≅ 0 (at ASO < 0). In this case we can assign the F2 and F1 levels as belonging to separate 2Π3/2 and 2Π1/2 states. It is for this situation that Ω is practically a good quantum number. The coupling scheme of the angular momenta can be understood in terms of two phases: the orbit and spin angular momenta couple first into the total electronic angular momentum, Ĵe, and then Ĵe couples with the angular momentum of the nuclear rotation  giving the total angular momentum
giving the total angular momentum 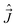 .
.
In the other extreme case, when the spin–orbit constant is much smaller that the rotational constant [as mentioned above, from the spectroscopic point of view, in this case Hund's scheme (a) is not convenient], i.e. at |ASO|/B≪1, we have in the first approximation
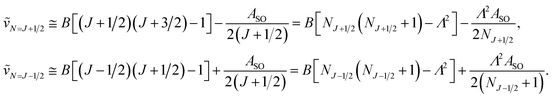 | (91) |
In this case the eigenfunction (88) involve appreciable contributions of both |2Π3/2, J, ±〉 and |2Π1/2, J, ±〉 basis functions, and at high rotation quantum numbers J, 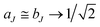 .
.
Since [see eqn (91)] the contribution of the spin–orbit operator (28) to the energy becomes continuously smaller at increasing J, the spin–rotation coupling term from the Hamiltonian (26), neglected up to now, grows in importance compared to the 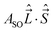 . The eigenvalues of the Hamiltonian with both ĤSO and ĤSR terms are
. The eigenvalues of the Hamiltonian with both ĤSO and ĤSR terms are
 | (92) |
In the limit B≫ASO, γ we obtain from eqn (92)
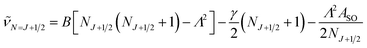 | (93) |
 | (94) |
Thus the contribution of the spin–rotation term (26) to the energy increases linearly with increasing J. These formulae are analogous to their counterparts ((78)/(79)) for Σ electronic states.
(b) Hund's case (b). When the effect of the spin–orbit coupling is small compared to the effect of the rotation, i.e. at B≫|ASO|, the Hamiltonian ((25)+(26)+(28)) is conveniently written in the form
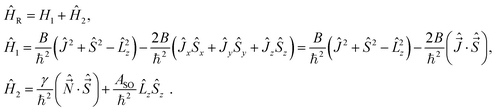 | (95) |
The dominant part of the Hamiltonian, Ĥ1, does not commute with Ŝz and Ĵz, and thus neither Σ nor Ω are near good quantum numbers. However, it commutes with the components of the operator 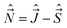 , and consequently with
, and consequently with ![[N with combining circumflex]](https://www.rsc.org/images/entities/i_char_004e_0302.gif) 2 and thus the quantum numbers N for
2 and thus the quantum numbers N for ![[N with combining circumflex]](https://www.rsc.org/images/entities/i_char_004e_0302.gif) 2, and Λ for are nearly good. This case can be conveniently handled e.g. using the formalism of reversed momenta
2, and Λ for are nearly good. This case can be conveniently handled e.g. using the formalism of reversed momenta 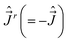 and
and 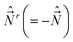 . The eigenvalues of the Hamiltonian matrix are the same as in the Hund's basis (a), i.e. they are given by eqn (92). The basis vectors of the case (b) are related to their case (a) counterparts by the Clebsch–Gordan coefficients.
. The eigenvalues of the Hamiltonian matrix are the same as in the Hund's basis (a), i.e. they are given by eqn (92). The basis vectors of the case (b) are related to their case (a) counterparts by the Clebsch–Gordan coefficients.
From the spectroscopic point of view, the coupling scheme in Hund's case (b) can be imagined as consisting of the following two phases: the spatial electronic angular momentum 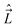 and the angular momentum of the nuclei,
and the angular momentum of the nuclei, 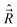 , couple first into the spinless total angular momentum
, couple first into the spinless total angular momentum 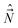 . In the second phase
. In the second phase 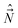 couples with the spin momentum
couples with the spin momentum 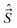 in the total angular momentum
in the total angular momentum 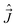 . Recall that here the projection of the spin on the molecular axis is not a constant of motion and consequently, Ω is not a nearly good quantum number.
. Recall that here the projection of the spin on the molecular axis is not a constant of motion and consequently, Ω is not a nearly good quantum number.
In electronic transitions that do not involve two Σ species, beside the P and R branches there is also a Q branch corresponding to the selection rule ΔJ = 0. The term values are
| ṽ = ṽ0 + F′(J′) − F′′(J′′) = ṽ0 + B′J(J + 1) − B′′J(J + 1) = ṽ0 + (B′ − B′′)J(J + 1). | (96) |
In electronic transitions which involve orbitally (Π, Δ,…) and spin (doublet, triplet,…) electronic species there are several P, Q, and R subbranches.
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 to 45
000 to 45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1. The spectrum we obtained was dominated by several broad peaks with hardly visible structure; the most intense of them extended between 31
000 cm−1. The spectrum we obtained was dominated by several broad peaks with hardly visible structure; the most intense of them extended between 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 and 32
400 and 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 cm−1, with the maximum at about 31
200 cm−1, with the maximum at about 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 900 cm−1 (Fig. 7). An inspection of the standard molecular data sources, like ref. 47, 56 and 57 showed that the only electronic band-system involving aluminum in combinations with the other species, like hydrogen and oxygen, which are present in our plasma is the C2Π → X2Σ+ transition of AlO appearing in the region 30
900 cm−1 (Fig. 7). An inspection of the standard molecular data sources, like ref. 47, 56 and 57 showed that the only electronic band-system involving aluminum in combinations with the other species, like hydrogen and oxygen, which are present in our plasma is the C2Π → X2Σ+ transition of AlO appearing in the region 30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100–34
100–34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 800 cm−1.57 However, the assignment of the observed feature to this transition was a very difficult task because a poor quality of the spectrum and the possibility that the bands of the A2Σ+ → X2Π system of OH, whose presence is usual under given experimental conditions, appear in the same wavelength region. The most prominent band (0–0) of this electronic transition lies around 32
800 cm−1.57 However, the assignment of the observed feature to this transition was a very difficult task because a poor quality of the spectrum and the possibility that the bands of the A2Σ+ → X2Π system of OH, whose presence is usual under given experimental conditions, appear in the same wavelength region. The most prominent band (0–0) of this electronic transition lies around 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 cm−1.57
500 cm−1.57
We found the peak shown in Fig. 7 too broad to be assigned to a single vibrational transition and it seemed to have a richer structure than that expected from a purely vibrational spectrum. In order to identify the structure of this peak, a smoothing procedure based on the Savitzky–Golay method,155 implemented in the software package ORIGIN, was applied. The results obtained in this way are depicted in Fig. 8.154
 | ||
| Fig. 8 The spectrum obtained after 5-point smoothing of the original data. Thin vertical lines: estimated positions of the AlO branches taken and positions of possible OH bands. | ||
The central question at this stage was whether the structure of the spectrum in Fig. 8 has physical origin or represents artifacts caused by the low signal–noise ratio, limited accuracy of measurements, and/or mathematical manipulation with the experimental results. As a preliminary test, we handled the spectrum of the D2 lamp in the same way. Though the D2 spectrum was not completely smooth, it was quite monotonous and the relative intensity of local peaks was by a factor of ten smaller than that in the spectrum of the alumina sample. That seemed to be a strong indication that the structure of the spectrum such as shown in Fig. 8 was not simply caused by statistical noise fluctuations.
In the second step, we carefully checked whether the features of the spectra extracted in this way depended on the choice of the smoothing criterion. We specified the number of points that control the degree of smoothing (“filter width”) from 3 to 9. It turned out that the main consequence of the enlargement of the number of points involved in the smoothing procedure was disappearance of some (smaller) peaks; the positions of those, which did not disappear were in general not significantly changed. It was decided to work further using the smoothing procedure that involves the groups of 5 points to calculate each averaged result.
Another test of reliability of our results was made by carrying out 15 experiments under same conditions. The result of these measurements was the systematic appearance of 16 peaks in the wave number region between 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 620 cm−1 and 32
620 cm−1 and 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 040 cm−1. The variation of their positions from one experiment to another remained within the error margin of about 10 cm−1.
040 cm−1. The variation of their positions from one experiment to another remained within the error margin of about 10 cm−1.
As mentioned in the first paragraph of this subsection, we expected in our spectrum the appearance of the AlO and, possibly, OH bands. We carried out an analysis of the reliability of three possibilities: (i) the observed spectrum corresponded to the A2Σ+ → X2Π transition of OH; (ii) it had to be assigned to the C2Π → X2Σ+ transition of AlO; (iii) both of these transitions were involved in the spectrum.
(i) The equilibrium bond lengths in the X2Π and A2Σ+ electronic states of OH are 0.96966 and 1.0121 Å, and the vibrational wave numbers are 3738 and 3179 cm−1, respectively.56 Using these data, we computed in the harmonic approximation the FCFs for transitions between the low-lying vibrational levels of both electronic states in questions. We concluded that the most intense transitions are those with v′ = v′′, being a consequence of the relatively small change of the internuclear distance (0.042 Å), Because of quite different values of the vibrational fundamentals, the 0–0, 1–1 and 2–2 members of this sequence of bands should be clearly separated from one another. Further, the position of none of the most intense bands of OH is close to the maximum of the recorded spectrum. Thus, although this tentative analysis did not exclude the possibility that the OH bands contribute to the observed spectrum, it seemed to be clear that the spectrum cannot be caused only by this transition.
(ii) The equilibrium bond lengths in the X2Σ+ and C2Π states of AlO are 1.618 and 1.685 Å, and the vibrational fundamentals are 979 cm−1 and 856.5 cm−1, respectively.56 Using these parameters we computed the FCFs for the transitions between the low-lying vibrational levels of the X2Σ+ and C2Π states. Since the electric moment for the transition between these two electronic species does not dramatically vary with the change of the internuclear distance (see Fig. 5 of ref. 119), the FCFs should reliably represent the intensity distributions in the spectrum. In this case, the change of the bond length is significantly larger (0.067 Å) than in the A2Σ+ → X2Π transition of OH. This causes that in general the Δv = ±1, and at larger v values even Δv = ±2 transitions are of comparable intensity as their Δv = 0 counterparts. It was even found that the transitions with Δv = ±1 should be stronger than those with Δv = ±0 (the exception is the 0–0 vibrational transition, being the strongest one when the population of the v = 0 level in the initial state is the largest one). For this reason the C2Π → X2Σ+ transition of AlO should produce a complex spectrum, capable to cover the whole wave number region recorded. Thus, in the following we focused our attention on this system.
The rotational energy levels, with incorporated spin–rotation coupling in the X2Σ+ electronic state of AlO are given by the formulae (78) and (79). Since the spin–rotation constant is positive (γ = 51.66 MHz),122 the F1 (J = K + 1/2) sublevels are above their F2 (J = K − 1/2) counterparts. The X2Σ+ state belongs to Hund's (b) case, and thus the F1 and F2 levels corresponding to the same K are of the same parity, (−1)N. The energy level schema is presented in Fig. 9, bottom (of course, with exaggerated spin–rotation splitting).
The C2Π electronic state of AlO belongs to Hund's (a) case, with |ASO|≫B. Since the 2Π state in question is of… π1-type, the spin–orbit constant if positive (ASO = 73.9230 cm−1),140 i.e. the state is “regular”, with the Ω = 3/2 (F2) sub-state above its Ω = 1/2 (F1) counterpart. Thus, when speaking about the bands of the C2Π–X2Σ+ system, we may use the terminology 2Π3/2–2Σ+ and 2Π1/2–2Σ+ sub-bands.47 If we neglect the spin rotation coupling, in accord with the results presented in ref. 140, the rotation plus spin–orbit structure of the spectrum is determined by eqn (90). According to it, the separation between the levels with the same J is in the present approximation (note that we also neglect the dependence of the rotational constant on J) in the 2Π3/2–2Σ+ and 2Π1/2–2Σ+ sub-bands is ASO − 2B′. On the other hand, the separation between lowest-lying rotational levels in two 2Π sub-states, J = 3/2 of the 2Π3/2 component, and J = 1/2 of 2Π1/2 is ASO + B′. The energy schema is shown in Fig. 9, top. Additionally, the Λ-type splitting (again exaggeratedly) is indicated. The two Λ-sublevels have opposite parity and ordering of + and − levels is an alternating function of J, i.e. the upper sublevel has the (−1)J−1/2 parity. Besides, in Fig. 9 we label the rotational levels of the C2Π state by (K′), although in Hund's scheme (b) K is not a good quantum number. Note that with increasing J Hund's case (a) tends toward the case (b). A consequence thereof is that the “satellite” branches (with ΔJ ≠ ΔK) become less intense.
The selection rules for the rotational transitions are J′ − J′′ = −1 (P branch), 0 (Q branch) and +1 (R branch), and + ↔ − [The near selection rule N′ − N′′ = 0, ±1, being appropriate for Hund's case (b), does not hold in Hund's case (a).]. Thus we have in total twelve branches, Pnm, Qnm, Rnm, depending on whether the subscript n of the upper level, Fn, and m of the lower level, Fm, is 1 or 2. For the 2Π3/2–2Σ+ sub-band we have thus P11 ≡ P1, P12, Q11 ≡ Q1, Q12, R11 ≡ R1, and P12, whereas for the 2Π1/2–2Σ+ sub-band there are P21, P22 ≡ P2, Q21, Q22 ≡ Q2, R21, and R22 ≡ R2 branches.
Using the rotational constants B′′ = 0.64136 (ref. 47) and B′ = 0.600792 (ref. 140) and the above quoted spin–orbit constant, we computed by means of the formulae (90) (with γ put equal 0) and ((69), (70) and (96)) the Fortrat diagrams presented in Fig. 10. In the approximation applied, the pairs of parabolas P1 and Q12, Q1 and R12, Q2 and P21, R2 and Q21 coincide when drawn as functions of the quantum number J′. For small J values (<10) these results agree very well with their experimentally derived counterparts presented in Table 1 of ref. 140; at higher J values slight systematic discrepancies become apparent. All branches are degraded to the red. The branches Q1/R12, R1, R2/Q21, and R21 build heads at J′ = 7.5, 23.5, 7.5, and 23.5, respectively at 2, 21, 75 and 94 cm−1 with respect to the origin of the 2Π1/2 sub-state.
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000–35
000–35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 cm−1 and photographed some of them at high resolution. However, the authors were not able to detect all of the expected branches but in most cases only the heads of the Q1, R1, and R2 ones. Average wave number differences between the positions of these branches were found to be roughly 30 cm−1 (R1 − Q1) and 50 cm−1 (R2 − R1). These numbers are similar to those we calculated above using the theoretical energy expressions and experimentally derived molecular parameters (about 20 cm−1 and 50 cm−1, respectively).
000 cm−1 and photographed some of them at high resolution. However, the authors were not able to detect all of the expected branches but in most cases only the heads of the Q1, R1, and R2 ones. Average wave number differences between the positions of these branches were found to be roughly 30 cm−1 (R1 − Q1) and 50 cm−1 (R2 − R1). These numbers are similar to those we calculated above using the theoretical energy expressions and experimentally derived molecular parameters (about 20 cm−1 and 50 cm−1, respectively).In the present case we refrained from trying to calculate the vibrational levels of the C2Π electronic state. We estimated instead the positions of the Q1, R1, and R2 branch heads for all relevant vibrational levels, using the experimental data published by Singh and Saksena.138 They are given on the top of Table 10. Combining these numbers with vibrational term values for the X2Σ+state, we constructed the complete Deslandres table for the C2Π–X2Σ+ spectral system of AlO. The experimental results, where available, are given in parentheses. It can be seen that the results of simulation of the spectrum agree in all cases within few wave numbers with the corresponding experimental findings. This is an indication for the internal consistency and reliable interpretation of the experimental results published in ref. 138.
| Tv′ | Q1 | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 012c 012c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 862c 862c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 693c 693c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 512c 512c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 322c 322c |
|---|---|---|---|---|---|---|
| R1 | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 045c 045c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 895c 895c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 726c 726c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 545c 545c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 355c 355c |
|
| R2 | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 096c 096c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 946c 946c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 777c 777c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 596c 596c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 406 406 |
|
| Tv′′ | v′–v′′ | 0 | 1 | 2 | 3 | 4 |
| 0c | 0 | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 012c 012c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 862c 862c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 693c 693c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 512c 512c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 322c 322c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 045c 045c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 895c 895c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 726c 726c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 545c 545c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 355c 355c |
||
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 096c 096c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 946c 946c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 777c 777c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 596c 596c |
36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 406c 406c |
||
(33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 096) 096) |
(33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 946) 946) |
(34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 778) 778) |
||||
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 047c 047c |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 897c 897c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 728c 728c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 547c 547c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 357c 357c |
||
| 965c | 1 | 33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 080c 080c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 930c 930c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 761c 761c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 580c 580c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 390c 390c |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 131c 131c |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 981c 981c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 812c 812c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 631c 631c |
35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 441c 441c |
||
(32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 131) 131) |
(32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 981) 981) |
(33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 812) 812) |
||||
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 095c 095c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 945c 31 945c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 945 945 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 776c 776c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 595c 595c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 405c 405c |
||
(31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 098) 098) |
||||||
| 1917c | 2 | 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 128c 128c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 978c 31 978c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 972 972 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 809c 809c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 628c 628c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 438c 438c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 179c 179c |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 029c 32 029c 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 034 034 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 860c 860c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 679c 679c |
34![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 489c 489c |
||
(31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 180) 180) |
(32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 030) 030) |
|||||
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 158c 158c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 008c 008c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 839c 31 839c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 836 836 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 658c 658c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 468c 468c |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 161) 161) |
||||||
| 2854c | 3 | 30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 191c 191c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 041c 041c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 872c 31 872c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 875 875 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 691c 691c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 501c 501c |
302![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 42c 42c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092c 092c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 923c 31 923c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 921 921 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 742c 742c |
33![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 552c 552c |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 234) 234) |
||||||
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 236c 236c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 086c 086c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 917c 917c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 736c 736c |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 546c 546c |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 087) 087) |
||||||
| 3776c | 4 | 29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 269c 269c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 119c 119c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 950c 950c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 769c 31 769c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 767 767 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 579c 579c |
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 117) 117) |
||||||
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 320c 320c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 170c 170c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 001c 001c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 820c 31 820c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 812 812 |
32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 630c 630c |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 163) 163) |
||||||
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 327c 327c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 177c 177c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 008c 008c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 827c 827c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 637c 31 637c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 632 632 |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 008) 008) |
||||||
| 4685c | 5 | 28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 360c 360c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 210c 210c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 041c 041c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 860c 860c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 670c 31 670c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 667 667 |
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 042) 042) |
||||||
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 411c 411c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 261c 261c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092c 092c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 911c 911c |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 721c 31 721c 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 722 722 |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 092) 092) |
||||||
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 432c 432c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 282c 282c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 133c 133c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 932c 932c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 742c 742c |
||
(29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 932) 932) |
||||||
| 5580c | 6 | 27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 465c 465c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 315c 315c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 146c 146c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 965c 965c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 775c 775c |
(29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 965) 965) |
||||||
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 516c 516c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 366c 366c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 197c 197c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 016c 016c |
30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 826c 826c |
||
(30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 016) 016) |
||||||
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 552c 552c |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 402c 402c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 233c 233c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 052c 052c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 862c 862c |
||
| 6460c | 7 | 26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 585c 585c |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 435c 435c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 266c 266c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 085c 085c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 895c 895c |
(29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 894) 894) |
||||||
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 636c 636c |
27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 486c 486c |
28![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 317c 317c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 136c 136c |
29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 946c 946c |
||
(29![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 946) 946) |
In ref. 154 we presented the results of 15 experiments carried out under the same conditions. In each case a smoothing involving five points is performed. We identified sub-branches Q1, R1, and R2 for the transitions (v′, v′′) = (1, 2), (2, 3), (3, 4), and (4, 5), as shown in Fig. 8. These results are included in Table 10. To our knowledge, only the (0, 1) and (1, 2) members of the sequence v′–v′′ = −1 have been analyzed in the experiments preceding our study 154. Our results agree within the error margin of 10 cm−1 with the corresponding positions of band heads, estimated on the basis of previous experimental data. Thus, we believe that a great part of the spectrum we observed in the region between 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 and 32
400 and 32![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 cm−1 belongs to the v′–v′′ = −1 sequence of the C2Π–X2Σ+ spectral system of AlO.
200 cm−1 belongs to the v′–v′′ = −1 sequence of the C2Π–X2Σ+ spectral system of AlO.
Beside the peaks we assign to the Q1, R1, and R2 sub-branches of the C2Π–X2Σ+ system of AlO, we identified several features that cannot be incorporated in this scheme. We suppose that they could correspond to the 1–1 transition of the A2Σ+–X2Π system of OH, as e.g. feature observed at 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 992 cm−1 (average values for 15 experiments), being close to the position of the R2 branch of this OH band at 31
992 cm−1 (average values for 15 experiments), being close to the position of the R2 branch of this OH band at 31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 986 cm−1.57 It is also possible that some of the unassigned spectral features correspond to the heads of the R21 sub-branch of the C2Π–X2Σ+ system of AlO (see Fig. 10).
986 cm−1.57 It is also possible that some of the unassigned spectral features correspond to the heads of the R21 sub-branch of the C2Π–X2Σ+ system of AlO (see Fig. 10).
7. Conclusions
In the present review, we describe a part of our investigations on plasma electrolytic oxidation of light metals and their alloys. We recorded emission spectra in order to get information about the processes that take place in our systems, with the aim to become able to monitor them. Due to inherently stochastic appearance of spectral sources (microdischarge plasmas), poor resolution, usually low intensities, and unfavorable signal/noise ratios, we were not able to carry out the assignment of spectral features, particularly of molecular bands, solely based on use of standard sources of spectral data. Instead, we had to compare our spectra with those obtained in previous spectroscopic measurements, carried out under completely different conditions and giving the results of substantially different kind. On the other hand, it turned out that the systems we are dealing with can serve as a source of new spectroscopic information in large wavelength regions. For estimation of important parameters, like temperature and electron number density, various plasma diagnostics methods had to be applied. The results of measurements of molecular spectra were combined with quantum-mechanical calculations when the standard approaches failed.Determination of plasma parameters based on spectral measurement supposes the existence of at least partial thermal equilibrium. It was hardly to expect any kind of thermal equilibrium in the systems like those being the subject of the present review. However, there are a number of indications speaking against this skepticism. First, we have shown that there seems to exist at least local thermal equilibrium for individual degrees of freedom. For example, in our recent study on Zr,27 we determined the plasma temperature of T = 7500 ± 1000 by using 45 Zr I (atomic) lines in the wavelength region between 420 and 516 nm. The fact that the majority of them lay very close to the Boltzmann straight line ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) I ∼ E/k, represents a strong indication for the existence of the local thermal equilibrium for electronic motions. The above temperature value agrees with those obtained by a number of other authors, who used similar, but also quite different methods for their determination.21,22,28,29,156,157 On the other hand, in the present study we demonstrate that the intensity distributions within band sequences of two spectral systems, B1Σ+ → X1Σ+ MgO104 and B2Σ+ → X2Σ+ of AlO152 also obeyed the Boltzmann law. The values of T = 11
I ∼ E/k, represents a strong indication for the existence of the local thermal equilibrium for electronic motions. The above temperature value agrees with those obtained by a number of other authors, who used similar, but also quite different methods for their determination.21,22,28,29,156,157 On the other hand, in the present study we demonstrate that the intensity distributions within band sequences of two spectral systems, B1Σ+ → X1Σ+ MgO104 and B2Σ+ → X2Σ+ of AlO152 also obeyed the Boltzmann law. The values of T = 11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 ± 2000 K in the former, and T = 8000 ± 2000 K in the later case, being quite similar to that obtained on the basis of relative intensities of Zr atomic lines, show that it is not excluded that the thermal equilibrium exist between different degree of freedom, too. However, this opens the question why the temperature obtained using the A2Σ+ (v′ = 0)–X2Π band system of OH was much lower, 3500 ± 500 K, as obtained in our study on Mg,152 and T = 2800 ± 500 in PEO of Zr.27 A possible and even quite probable explanation of this discrepancy can be found in the results of previous investigations on the systems similar to the present one. They have led to the conclusion that the discharge plasma consists of a central core, with the temperature at roughly 7000 K,156–158 surrounded by lower-temperature (about 3500 K) regions.31 In terms of this model, OH is predominantly present in the colder zone, whereas the atoms and ions of the metal investigated, as well as molecules built in plasma-chemical reactions, occupy the high-temperature zone.
000 ± 2000 K in the former, and T = 8000 ± 2000 K in the later case, being quite similar to that obtained on the basis of relative intensities of Zr atomic lines, show that it is not excluded that the thermal equilibrium exist between different degree of freedom, too. However, this opens the question why the temperature obtained using the A2Σ+ (v′ = 0)–X2Π band system of OH was much lower, 3500 ± 500 K, as obtained in our study on Mg,152 and T = 2800 ± 500 in PEO of Zr.27 A possible and even quite probable explanation of this discrepancy can be found in the results of previous investigations on the systems similar to the present one. They have led to the conclusion that the discharge plasma consists of a central core, with the temperature at roughly 7000 K,156–158 surrounded by lower-temperature (about 3500 K) regions.31 In terms of this model, OH is predominantly present in the colder zone, whereas the atoms and ions of the metal investigated, as well as molecules built in plasma-chemical reactions, occupy the high-temperature zone.
Acknowledgements
We thank the Ministry of Education, Science, and Technological Development of the Republic of Serbia for financial support (Projects no. 171035 and 172040).References
- S. Stojadinovic, R. Vasilic, I. Belca, M. Petkovic, B. Kasalica, Z. Nedic and Lj. Zekovic, Corros. Sci., 2010, 52, 3258 CrossRef CAS PubMed.
- M. Petković, S. Stojadinović, R. Vasilić and Lj. Zeković, Appl. Surf. Sci., 2011, 257, 10590 CrossRef PubMed.
- S. Stojadinović, N. Radić, R. Vasilić, M. Petković, P. Stefanov, Lj. Zeković and B. Grbić, Appl. Catal., B, 2012, 126, 334 CrossRef PubMed.
- L. O. Snizhko, A. L. Yerokhin, A. Pilkington, N. L. Gurevina, D. O. Misnyankin, A. Leyland and A. Matthews, Electrochim. Acta, 2004, 49, 2085–2095 CrossRef CAS PubMed.
- S. Stojadinović, R. Vasilić, M. Petković, I. Belča, B. Kasalica, M. Perić and Lj. Zeković, Electrochim. Acta, 2012, 59, 354 CrossRef PubMed.
- H. F. Guo, M. Z. An, H. B. Huo, S. Xu and L. J. Wu, Appl. Surf. Sci., 2006, 252, 7911 CrossRef CAS PubMed.
- F. C. Walsh, C. T. J. Low, R. J. K. Wood, K. T. Stevens, J. Archer, A. R. Poeton and A. Ryder, Trans. Inst. Met. Finish., 2009, 87, 122 CrossRef CAS PubMed.
- J. M. Albella, I. Montero and J. M. Martinez-Duart, Electrochim. Acta, 1987, 32, 255 CrossRef CAS.
- S. Ikonopisov, Electrochim. Acta, 1977, 22, 1077 CrossRef CAS.
- C.-J. Liang, In-situ Impedance Spectroscopy Studies of the Plasma Electrolytic Oxidation Coating Process, PhD thesis, University of Sheffield, 2013.
- M. Petković, S. Stojadinović, R. Vasilić, I. Belča, Z. Nedić, B. Kasalica and U. B. Mioć, Appl. Surf. Sci., 2011, 257, 9555 CrossRef PubMed.
- G. Sundararajan and L. Rama Krishna, Surf. Coat. Technol., 2003, 167, 269 CrossRef CAS.
- B. H. Long, H. H. Wu, B. Y. Long, J. B. Wang, N. D. Wang, X. Y. Lu, Z. S. Jin and Y. Z. Bai, J. Phys. D: Appl. Phys., 2005, 38, 3491 CrossRef CAS.
- A. L. Yerokhin, L. O. Snizhko, N. L. Gurevina, A. Leyland, A. Pilkington and A. Matthews, J. Phys. D: Appl. Phys., 2003, 36, 2110 CrossRef CAS.
- A. L. Yerokhin, L. O. Snizhko, N. L. Gurevina, A. Leyland, A. Pilkington and A. Matthews, Surf. Coat. Technol., 2004, 177–178, 779 CrossRef CAS PubMed.
- S. Stojadinović, R. Vasilić, M. Petković and Lj. Zeković, Surf. Coat. Technol., 2011, 206, 575 CrossRef PubMed.
- S. Stojadinović, J. Jovović, M. Petković, R. Vasilić and N. Konjević, Surf. Coat. Technol., 2011, 205, 5406 CrossRef PubMed.
- B. Kasalica, M. Petkovic, I. Belca, S. Stojadinovic and Lj. Zekovic, Surf. Coat. Technol., 2009, 203, 3000 CrossRef CAS PubMed.
- L. Wang, L. Chen, Z. Yan and W. Fu, Surf. Coat. Tehnol., 2010, 205, 1651 CrossRef CAS PubMed.
- L. Wang, W. Fu and L. Chen, J. Alloys Compd., 2011, 509, 7652 CrossRef CAS PubMed.
- J. Jovović, S. Stojadinović, N. M. Šišović and N. Konjević, Surf. Coat. Tehnol., 2011, 206, 24 CrossRef PubMed.
- J. Jovović, S. Stojadinović, N. M. Šišović and N. Konjević, J. Quant. Spectrosc. Radiat. Transfer, 2012, 113, 1928 CrossRef PubMed.
- S. Stojadinović, R. Vasilić, M. Petković, I. Belča, B. Kasalica, M. Perić and Lj. Zeković, Electrochim. Acta, 2012, 59, 354 CrossRef PubMed.
- S. Stojadinović, R. Vasilić, M. Petković, I. Belča, B. Kasalica and Lj. Zeković, Electrochem. Commun., 2013, 35, 22 CrossRef PubMed.
- S. Stojadinović, R. Vasilić, M. Petković, B. Kasalica, I. Belča, A. Žekić and Lj. Zeković, Appl. Surf. Sci., 2013, 265, 226 CrossRef PubMed.
- S. Stojadinović, R. Vasilić, M. Petković, I. Belča, B. Kasalica, M. Perić and Lj. Zeković, Electrochim. Acta, 2012, 79, 133 CrossRef PubMed.
- S. Stojadinović, J. Radić-Perić, R. Vasilić and M. Perić, Appl. Spectrosc., 2014, 68, 161 CrossRef PubMed.
- R. O. Hussein, X. Nie, D. O. Northwood, A. Yerokhin and A. Matthews, J. Phys. D: Appl. Phys., 2010, 43, 105203 CrossRef.
- M. D. Klapkiv, H. M. Nykyforchyn and V. M. Posuvailo, Mater. Sci., 1994, 30, 333 CrossRef.
- R. O. Hussein, X. Nie and D. O. Northwood, Surf. Coat. Technol., 2010, 205, 1659 CrossRef CAS PubMed.
- C. S. Dunleavy, I. O. Golosnoy, J. A. Curran and T. W. Clyne, Surf. Coat. Technol., 2009, 203, 3410 CrossRef CAS PubMed.
- V. M. Posuvailo and M. D. Klapkiv, Mater. Sci., 1997, 33, 383 CrossRef CAS.
- V. M. Posuvailo, Mater. Sci., 2001, 37, 677 CrossRef CAS.
- B. Kasalica, I. Belča, S. Stojadinović, M. Sarvan, M. Perić and Lj. Zeković, J. Phys. Chem. C, 2007, 111, 12315 CAS.
- S. Stojadinovic, I. Belca, M. Tadic, B. Kasalica, Z. Nedic and Lj. Zekovic, J. Electroanal. Chem., 2008, 619–620, 125 CrossRef CAS PubMed.
- B. Kasalica, S. Stojadinović, I. Belča, M. Sarvan, Lj. Zeković and J. Radić-Perić, J. Anal. At. Spectrom., 2013, 28, 92 RSC.
- B. V. Kasalica, I. D. Belca, S. Đ. Stojadinovic, Lj. Zekovic and D. Nikolic, Appl. Spectrosc., 2006, 60, 1090 CrossRef CAS PubMed.
- P. R. Bunker, Molecular Symmetry and Spectroscopy, Academic Press, New York, 1979 Search PubMed.
- M. Born and J. R. Oppenheimer, Ann. Phys., 1927, 389, 457 CrossRef.
- J. K. G. Watson, Mol. Phys., 1970, 19, 465 CrossRef CAS.
- J. Brown and A. Carrington, Rotational Spectroscopy of Diatomic Molecules, Cambridge University Press, 2003 Search PubMed.
- J. T. Hougen, J. Chem. Phys., 1962, 36, 519 CrossRef CAS PubMed.
- R. N. Zare, Angular Momentum, John Willey&Sons, Inc., 1988 Search PubMed; V. D. Kleiman, H. Park, R. J. Gordon and R. N. Zare, Companion to Angular Momentum, J. Wiley&Sons, Inc., 1998 Search PubMed.
- J. H. Van Vleck, Rev. Mod. Phys., 1951, 23, 213 CrossRef CAS.
- J. M. Brown and B. J. Howard, Mol. Phys., 1976, 31, 1517 CrossRef CAS; J. M. Brown and B. J. Howard, Mol. Phys., 1976, 32, 1197 CrossRef.
- H. Lefebvre-Brion and R. W. Field, Perturbations in the Spectra of Diatomic Molecules, Academic Press, 1986 Search PubMed.
- G. Herzberg, Molecular Spectra and Molecular Structure. I. Spectra of Diatomic Molecules, D. Van Nostrand Co. Inc., Princeton, New Jersey, 1950 Search PubMed.
- A. Messiah, Quantum Mechanics, John Willey&Sons, Inc., New York, 1964 Search PubMed.
- J. M. Brown, J. T. Hougen, K. P. Huber, J. W. C. Johns, I. Kopp, H. Lefebvre-Brion, A. J. Merer, D. A. Ramsay, J. Rostas and R. N. Zare, J. Mol. Spectrosc., 1975, 55, 500 CrossRef CAS.
- M. Perić, The electrons in the molecules, the electron - one hundred years of discovery, the first volume, The electron and the world around us, ed. M. Kurepa, Institute for Textbooks and Teaching Aids, Belgrade, 1997, p. 311 Search PubMed.
- M. Perić and B. Engels, Theoretical spectroscopy of small molecules: Ab initio investigations of vibronic structure, spin-orbit splittings and magnetic hyperfine effects in the electronic spectra of triatomic molecules, in Understanding Chemical Reactivity, Quantum Mechanical Electronic Structure Calculations with Chemical Accuracy, ed. S. R. Langhoff, Kluwer Academic, Dordrecht, The Netherlands, 1995, vol. 13, p. 261 Search PubMed.
- R. Ranković, S. Stojadinović, M. Sarvan, B. Kasalica, M. Krmar, J. Radić-Perić and M. Perić, J. Serb. Chem. Soc., 2012, 77, 1483 CrossRef , supplemetary material S202.
- M. Perić, R. Runau, J. Römelt, S. D. Peyerimhoff and R. J. Buenker, J. Mol. Spectrosc., 1979, 78, 309 CrossRef.
- G. Herzberg, Molecular Spectra and Molecular Structure II. Infrared and Raman Spectra of Polyatomic Molecules, Van Nostrand Reinhold Reinhold, New York, 1945 Search PubMed.
- G. Herzberg, Molecular Spectra and Molecular Structure III. Electronic Spectra of Polyatomic Molecules, Van Nostrand, New York, 1967 Search PubMed.
- K. P. Huber and G. Herzberg, Molecular Spectra and Molecular Structure IV. Constants of Diatomic Molecules, Van Nostrand Reinhold, New York, 1979 Search PubMed.
- R. W. B. Pearse and A. G. Gaydon, The Identification of Molecular Spectra, Chapman and Hall, London, 1976 Search PubMed.
- P. C. Mahanti, Phys. Rev., 1932, 42, 609 CrossRef CAS.
- P. C. Mahanti, Indian J. Phys., 1935, 9, 455 CAS.
- A. Lagerqvist and U. Uhler, Nature, 1949, 164, 665 CrossRef CAS.
- A. Lagerqvist, Ark. Mat., Astron. Fys., 1943, A29, 1 Search PubMed.
- L. Brewer and R. F. Porter, J. Chem. Phys., 1954, 22, 1867 CrossRef CAS PubMed.
- D. Pešić and A. G. Gaydon, Proc. Phys. Soc., 1959, 73, 244 CrossRef.
- D. Pešić, Proc. Phys. Soc., 1960, 76, 844 CrossRef.
- L. Brewer, S. Trajmar and R. A. Berg, Astrophys. J., 1962, 135, 955 CrossRef CAS.
- S. Trajmar and G. E. Ewing, Astrophys. J., 1965, 142, 77 CrossRef CAS.
- M. Singh, J. Phys. B: At., Mol. Opt. Phys., 1971, 4, 565 CrossRef CAS.
- M. Singh, J. Phys. B: At., Mol. Opt. Phys., 1973, 6, 1339 CrossRef CAS.
- M. Singh, J. Phys. B: At., Mol. Opt. Phys., 1973, 6, 1917 CrossRef CAS.
- T. Ikeda, N. B. Wong, D. O. Harris and R. W. Field, J. Mol. Spectrosc., 1977, 68, 452 CrossRef CAS.
- B. Bourguignon, J. C. McCombie and J. Rostas, Chem. Phys. Lett., 1985, 113, 323 CrossRef CAS.
- B. Bourguignon and J. Rostas, J. Mol. Spectrosc., 1991, 146, 437 CrossRef CAS.
- P. C. F. Ip, K. J. Cross, R. W. Field, J. Rostas, B. Bourguignon and J. McCombie, J. Mol. Spectrosc., 1991, 146, 409 CrossRef CAS.
- E. Kagi, T. Hirano, S. Takano and K. Kawaguchi, J. Mol. Spectrosc., 1994, 168, 109 CrossRef CAS.
- P. Mürtz, H. Thümmel, C. Pfelzer and W. Urban, Mol. Phys., 1995, 86, 513 Search PubMed.
- J. H. Kim, X. Li, L. S. Wang, H. L. de Clercq, C. A. Fancher, O. C. Thomas and K. H. Bowen, J. Phys. Chem. A, 2001, 105, 5709 CrossRef CAS.
- J. W. Daily, C. Dreyer, A. Abbud-Madrid and M. C. Branch, J. Mol. Spectrosc., 2002, 214, 111 CrossRef CAS.
- D. Bellert, K. L. Burns, N. T. Van-Oanh, J. Wang and W. H. Breckenridge, Chem. Phys. Lett., 2003, 381, 381 CrossRef CAS PubMed.
- D. Bellert, K. L. Burns, N. T. Van-Oanh, J. Wang and W. H. Breckenridge, Chem. Phys. Lett., 2003, 381, 725 CrossRef CAS PubMed.
- J. Wang, N. T. Van-Oanh, D. Bellert, W. H. Breckenridge, M. A. Gaveau, E. Gloaguen, B. Soep and J. M. Mestdagh, Chem. Phys. Lett., 2004, 392, 62 CrossRef CAS PubMed.
- E. Kagi and K. Kawaguchi, J. Mol. Struct., 2006, 795, 179 CrossRef CAS PubMed.
- J. Wang and W. H. Breckenridge, J. Chem. Phys., 2006, 124, 124308 CrossRef PubMed.
- A. Maatouk, A. Ben Houria, O. Yazidi, N. Jaidane and M. Hochlaf, J. Chem. Phys., 2010, 133, 144302 CrossRef CAS PubMed.
- J. Schamps and H. Lefebvre-Brion, J. Chem. Phys., 1972, 56, 573 CrossRef CAS PubMed.
- S. R. Langhoff, C. W. Bauschlicher, Jr and H. Partridge, J. Chem. Phys., 1986, 84, 4474 CrossRef CAS PubMed.
- H. Thümmel, R. Klotz and S. D. Peyerimhoff, Chem. Phys., 1989, 129, 417 CrossRef.
- A. F. Jalbout, J. Mol. Struct., 2002, 618, 85 CrossRef CAS.
- C. W. Bauschlicher, Jr, D. M. Silver and D. R. Yarkony, J. Chem. Phys., 1980, 73, 2867 CrossRef PubMed.
- C. W. Bauschlicher, Jr, B. H. Lengsfield III, M. Silver and D. R. Yarkony, J. Chem. Phys., 1981, 74, 2379 CrossRef PubMed.
- C. W. Bauschlicher, Jr, S. R. Langhoff and H. Partridge, J. Chem. Phys., 1994, 101, 2644 CrossRef PubMed.
- C. W. Bauschlicher and H. Partridge, Chem. Phys. Lett., 2001, 342, 441 CrossRef CAS.
- D. R. Yarkony, J. Chem. Phys., 1988, 89, 7324 CrossRef CAS PubMed.
- R. N. Diffenderfer and D. R. Yarkony, J. Phys. Chem., 1982, 86, 5098 CrossRef CAS.
- R. N. Diffenderfer, D. R. Yarkony and P. J. Dagdigian, J. Quant. Spectrosc. Radiat. Transf., 1983, 29, 329 CrossRef CAS.
- B. Huron, J. P. Malrieu and P. Rancurel, Chem. Phys., 1974, 3, 277 CrossRef CAS.
- H. Thümmel, R. Klotz and S. D. Peyerimhoff, Chem. Phys., 1989, 135, 229 CrossRef.
- MOLPRO, a package of ab initio programs written by H.-J. Werner and P. J. Knowles, Version 2008.1, R. D. Amos, A. Bernhardsson, A. Berning et al..
- P. J. Knowles and H. J. Werner, Chem. Phys. Lett., 1985, 115, 259 CrossRef CAS.
- T. H. Dunning, Jr, J. Chem. Phys., 1989, 90, 1007 CrossRef PubMed.
- Unofficial set from D. Feller for Mg, https://bse.pnl.gov/bse/portal.
- H. J. Werner and P. J. Knowles, J. Chem. Phys., 1988, 89, 5803 CrossRef CAS PubMed.
- J. Knowles and H. J. Werner, Chem. Phys. Lett., 1988, 145, 514 CrossRef.
- A. Lagerqvist and U. Uhler, Ark. Fys., 1949, 1, 459 CAS.
- S. Stojadinović, M. Perić, J. Radić-Perić, R. Vasilić, M. Petković and Lj. Zeković, Surf. Coat. Technol., 2012, 206, 2905 CrossRef PubMed.
- P. N. Ghosh, P. C. Mahanti and B. C. Mukkerjee, Phys. Rev., 1930, 35, 1491 CrossRef CAS.
- D. S. Pešić, Proc. Phys. Soc., 1964, 83, 885 CrossRef.
- S. S. Prasad and K. Prasad, Proc. Phys. Soc., 1962, 80, 311 CrossRef CAS.
- C. de Izarra, J. Phys. D: Appl. Phys., 2000, 33, 1697 CrossRef CAS.
- A. Lagerqvist, N. E. L. Nilsson and R. F. Barrow, Ark. Fys., 1957, 12, 543 CAS.
- D. C. Tyte and R. W. Nicholls, Identification Atlas of Molecular Spectra, 1, Univ. Western Ontario, 1964 Search PubMed.
- S. L. N. G. Krishnamachari, N. A. Narasimham and M. Singh, Can. J. Phys., 1966, 44, 2513 CrossRef CAS.
- J. K. McDonald and K. K. Innes, J. Mol. Spectrosc., 1969, 32, 501 CrossRef CAS.
- M. Singh, Proc. - Indian Acad. Sci., Sect. A, 1973, 71, 82 Search PubMed.
- M. Singh, J. Phys. B: At., Mol. Opt. Phys., 1973, 6, 521 CAS.
- M. Singh and M. K. Saksena, J. Phys. B: At., Mol. Opt. Phys., 1973, 77, 139 CAS.
- V. W. Goodlett and K. K. Innes, Nature, 1959, 183, 243 CrossRef CAS.
- L. B. Knight and W. Weltner, Jr, J. Chem. Phys., 1971, 55, 5066 CrossRef CAS PubMed.
- S. Rosenwaks, R. E. Steele and H. P. Broida, J. Chem. Phys., 1975, 63, 1963 CrossRef CAS PubMed.
- C. Zenouda, P. Blottiau, G. Chambaud and P. Rosmus, J. Mol. Struct., 1999, 458, 61 CrossRef CAS.
- S. J. Bares, M. Haak and J. W. Nibler, J. Chem. Phys., 1985, 82, 670 CrossRef CAS PubMed.
- T. Törring and R. Herrmann, Mol. Phys., 1989, 68, 1379 CrossRef.
- C. Yamada, E. A. Cohen, M. Fujitake and E. Hirota, J. Chem. Phys., 1990, 92, 2146 CrossRef CAS PubMed.
- M. Goto, S. Takano, S. Yamamoto, H. Ito and S. Saito, Chem. Phys. Lett., 1994, 227, 287 CrossRef CAS.
- H. Ito and M. Goto, Chem. Phys. Lett., 1994, 227, 293 CrossRef CAS.
- A. Bernard and R. Gravina, Z. Naturforsch., A: Phys. Sci., 1984, 39, 1049 Search PubMed.
- M. J. Sayers and J. L. Gole, J. Chem. Phys., 1977, 67, 5442 CrossRef CAS PubMed.
- O. Launila and J. Jonsson, J. Mol. Spectrosc., 1994, 168, 1 CrossRef CAS.
- O. Launila and L. E. Berg, J. Mol. Spectrosc., 2011, 265, 10 CrossRef CAS PubMed.
- D. M. Lindsay and J. L. Gole, J. Chem. Phys., 1977, 66, 3886 CrossRef CAS PubMed.
- M. Singh, G. V. Zope and S. L. N. G. Krishnamachari, J. Phys. B: At., Mol. Opt. Phys., 1985, 18, 1743 CrossRef CAS.
- J. A. Coxon and S. Naxakis, J. Mol. Spectrosc., 1985, 111, 102 CrossRef CAS.
- M. D. Saksena, G. S. Ghodgaonkar and M. Singh, J. Phys. B: At., Mol. Opt. Phys., 1989, 22, 1993 CrossRef CAS.
- H. Ito, Can. J. Phys., 1994, 72, 1082 CrossRef CAS.
- N. Sato, H. Ito and K. Kuchitsu, Chem. Phys. Lett., 1995, 240, 10 CrossRef CAS.
- M. D. Saksena, M. N. Deo, K. Sunanda, S. H. Behere and C. T. Londhe, J. Mol. Spectrosc., 2008, 247, 47 CrossRef CAS PubMed.
- M. Singh and M. D. Saksena, Can. J. Phys., 1981, 59, 955 CrossRef CAS.
- M. Singh and M. D. Saksena, Can. J. Phys., 1982, 60, 1730 CrossRef CAS.
- M. Singh and M. D. Saksena, Can. J. Phys., 1983, 61, 1347 CrossRef CAS.
- M. Singh and M. D. Saksena, Can. J. Phys., 1985, 63, 1162 CrossRef CAS PubMed.
- J. P. Towle, A. M. James, O. L. Boure and B. Simard, J. Mol. Spectrosc., 1994, 163, 300 CrossRef CAS.
- J. P. Towle, A. M. James, O. L. Boure, B. Simard, M. Singh and M. D. Saksensa, J. Mol. Spectrosc., 1994, 167, 472 CrossRef.
- S. R. Desai, H. Wu, C. M. Rohlfing and L. S. Wang, J. Chem. Phys., 1997, 106, 1309 CrossRef CAS PubMed.
- S. R. Desai, H. Wu and L. S. Wang, Int. J. Mass. Spectrom. Ion. Processes, 1996, 159, 75 CrossRef CAS.
- N. V. Joshy, T. K. Mandal and B. L. Jha, Acta Phys. Pol., A, 1988, 73, 613 Search PubMed.
- C. T. Londhe, K. Sunanda, M. D. Saksena and S. H. Behere, J. Mol. Spectrosc., 2010, 263, 178 CrossRef CAS PubMed.
- M. Yoshimine, A. D. McLean and B. Liu, J. Chem. Phys., 1973, 58, 4412 CrossRef CAS PubMed.
- G. Das, T. Janis and A. C. Wahl, J. Chem. Phys., 1974, 61, 1274 CrossRef CAS PubMed.
- B. H. Lengsfield III and B. Liu, J. Chem. Phys., 1982, 77, 6083 CrossRef PubMed.
- J. Schamps, Chem. Phys., 1973, 2, 352 CrossRef CAS.
- V. M. Kovba and I. A. Topol, J. Mol. Struct., 1986, 137, 65 CrossRef.
- A. Marquez, M. J. Capitan, J. A. Odriozola and J. F. Sanz, Int. J. Quant. Chem., 1994, 52, 1329 CrossRef CAS.
- S. Stojadinović, M. Perić, M. Petković, R. Vasilić, B. Kasalica, I. Belča and J. Radić-Perić, Electrochim. Acta, 2011, 56, 10122 CrossRef PubMed.
- B. Rosen, Phys. Rev., 1945, 68, 124 CrossRef CAS.
- M. Sarvan, M. Perić, Lj. Zeković, S. Stojadinović, I. Belča, M. Petković and B. Kasalica, Spectrochim. Acta A, 2011, 81, 672 CrossRef CAS PubMed.
- A. Savitzky and M. J. E. Golay, Anal. Chem., 1964, 36, 1627 CrossRef CAS.
- R. O. Hussein, P. Zhang, X. Nie, Y. Xia and D. O. Northwood, Surf. Coat. Technol., 2011, 206, 1990 CrossRef CAS PubMed.
- M. D. Klapkiv, Mater. Sci., 1995, 31, 494 CrossRef.
- M. D. Klapkiv, Mater. Sci., 1999, 35, 279 CrossRef CAS.
| This journal is © The Royal Society of Chemistry 2014 |