Mechanical properties and thermal stability of ultrathin tungsten nanowires
Abstract
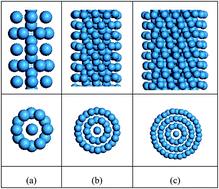
The most stable structures of three ultrathin tungsten nanowires were predicted by the simulated annealing basin-hopping method (SABH) with the penalty algorithm. The predicted structures of tungsten nanowires indicate the tungsten nanowires at this small scale do not possess the B.C.C. configuration in bulk tungsten material. By molecular dynamics (MD) simulation, the mechanical properties including the Young's modulus, yielding stress, and strength of these wires were determined by the tensile test after the analysis of the stress–strain profiles. Besides, in order to understand the feasibility of application of tungsten nanowire on nanodevices, the thermal stability of these ultrathin tungsten nanowires was also investigated at room temperature (300 K) by molecular dynamics (MD).
- This article is part of the themed collection: ChinaNANO

 Please wait while we load your content...
Please wait while we load your content...