Electrically evoking and electrochemically resolving quantal release on a microchip

Abstract

A microchip was applied to electrically depolarize rat pheochromocytoma (PC12) cells and to simultaneously detect exocytotic catecholamine release amperometrically. Results demonstrate exocytosis elicited by flowing cells through an electric field generated by a potentiostat circuit in a microchannel, as well as exocytosis triggered by application of an extracellular voltage pulse across. Electrical finite element model (FEM) analysis illustrated that larger cells experienced greater depolarizing excitation from the extracellular electric fields due to the smaller shunt path and higher resistance to current flow in the channel around the cell. Consistent with these simulations, data recorded from cell clusters and large cells exhibited increased release rates relative to data from the smaller cells. Overall, the system was capable of resolving single vesicle quantal release, in the zeptomole range, as well as the kinetics associated with the vesicle fusion process. Analysis of spike population statistics suggested detection of catecholamines from multiple release sites around the cells. The potential for such a device to be used in flow cytometry to evoke and detect exocytosis was demonstrated.

---

Introduction

Controlled exocytosis of biochemical transmitters is the principal means of communication utilized by neuronal cells and secretory endocrine cells. Certain congenital disorders, toxins/drugs, and diseases can alter exocytotic events and thereby lead to serious physiological problems.^1–3 Consequently, studies of the underlying mechanisms of exocytosis are crucial to our basic understanding of how this means of communication is effected as well as to the development of potential therapeutic interventions. One approach to studying exocytosis is to place an electrode adjacent to the cell membrane in close vicinity to the release site and to electrochemically detect single vesicle, or quantal release. The power of the technique lay in the high temporal resolution and sensitivity of detection with the ability to resolve microsecond scale events⁴ and zeptomole quantities⁵ of released neurotransmitter, respectively. Since its inception,⁶ this method has been extensively applied to studies of cellular exocytosis as has been previously reviewed.⁷

Conventional electrochemical detection of transmitter release immobilizes the cell and uses a micromanipulator to position a carbon fiber electrode (CFE) in direct contact with the plasma membrane. Release is evoked by application of chemical stimuli or by depolarization of the cell using whole-cell voltage/current clamp. In the present work, we were specifically interested in developing systems to facilitate rapid throughput by circumventing the need for micromanipulation and whole-cell voltage/current clamp.

Recently, a number of groups have advanced standard electrochemistry technology by leveraging micro-electromechanical systems (MEMS) fabrication techniques.^8–22 With MEMS, it is possible to achieve microfluidic and electrode-array architectures for facilitated selection and precise positioning of cells relative to the electrodes of interest. Currently, the majority of MEMS-implemented detection schemes involve the culture or seeding of cells on top of solitary electrodes or electrode arrays.^{12,13,17,18,21–23} This approach has the advantage of ensuring direct contact of cells with the electrode(s) of interest. Furthermore, as the cells are immobilized, often through adhesion-promoters such as poly-lysine,¹³ it is straightforward to deliver solutions to the cells for depolarization, differentiation and/or pharmacology, without displacing the cells. An alternative approach is to use microfluidic-based MEMS to position cells.^9,19 With both designs, cells were customarily stimulated using depolarizing solutions of elevated potassium, nicotine, or barium chloride. This requires a separate fluidic infrastructure to consistently and rapidly deliver and exchange solutions to the cell²⁴ and methods to account for the time delay between solution delivery and depolarization.²⁵ Photo-released caged Ca²⁺ has also been used to stimulate chromaffin cells and avoid some of the challenges associated with chemical delivery systems.¹² However, one drawback of this approach is that it required a permeabilizing and pre-loading of the cells with the caged Ca²⁺. We present here a microchip-based approach to electrically depolarize individual PC12 cells and cell clusters and to amperometrically detect neurotransmitter release. Specifically, results demonstrate that release can be triggered by flowing the cell through the electric field generated by the potentiostat circuit used for electrochemical detection. In separate experiments, release was initiated with the application of an extracellular voltage pulse. We demonstrate that this system resolves individual quantal biochemical release events, in the zeptomole range, and the kinetics associated with the vesicle fusion process. Furthermore, we demonstrate the potential for such a device to be used in low-rate flow cytometry for population studies of cellular exocytosis.

Methods

The present study used the PC12 cell line derived from a rat pheochromocytoma, a tumor in the rat adrenal gland.²⁶ These cells release catecholamines, principally dopamine,²⁶ an electroactive molecule that can be readily detected without electrode functionalization using conventional electrochemical methods. The advantages of PC12 cells for exocytosis studies have been well documented as has been previously reviewed.²⁷

Cells were cultured in suspension in 75 mm² tissue culture flasks with RPMI 1640 (Invitrogen, Carlsbad, CA) cell culture media supplemented with 15% horse serum (Invitrogen), 2.5% fetal bovine serum (Invitrogen) and 0.2 mg/ml gentamicin (Invitrogen). Flasks were kept in an incubator at 37 °C with 5% CO₂. Media was refreshed every 3-4 days, retaining 10% of the existing media each time. Cells were mechanically agitated to break up cell clusters through repeated washing through a pipettor tip. Additional separation was achieved through weekly, overnight treatment of the cells in 1 mM ethylene glycol tetraacetic acid (EGTA, Sigma-Aldrich, St. Louis, MO) with subsequent washing and trituration as previously described.²⁸ Cell media was supplemented with 5 µM dexamethasone (Sigma-Aldrich) to differentiate the cells into more chromaffin-like cells in an effort to increase quantal yield and release efficiency.²⁹

Prior to use, cells were transferred to Locke's buffer consisting of 154 mM NaCl, 5.6 mM KCl, 3.6 mM NaHCO₃, 2.3 mM CaCl₂, 5.6 mM glucose, and 5 mM Hepes (pH 7.4). An aliquot of the solution was immediately transferred to the MEMS device via manual pipette aspiration. Cells were moved back and forth in the channel through application of vacuum or pressure using 2 ml Pipette Pumps (Bel-Art, Pequannock, NJ) connected through the previously described device interface.³⁰ The interface provided the ability to dynamically view the cells in the chamber with an inverted microscope (IM35, Zeiss, Thornwood, NY). Manual control of 2 ml pipette pumps in conjunction with this direct visual feedback enabled exact positioning of the cell over the desired WE in a static or no-flow condition (as shown in Fig. 1). The pipette pumps were also used to generate the vacuum pressure necessary for channel flow in the flow-through cell experiments.

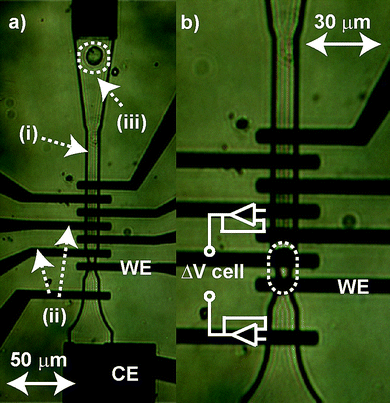Fig. 1 (a) Optical micrographs of the MEMS microchip recording chamber with labeled: (i) fluidic channel (10 µm deep), (ii) two of the electrodes in the interdigitated array and (iii) a PC12 cell being pulled into the chamber. Also labeled are the working electrode (5 µm × 10 µm, WE) and counter electrode (CE) used in the potentiostat circuit. A separate Ag/AgCl reference electrode (not shown) is located further downstream. (b) The cell is isolated over the WE via a 5 µm constriction in the channel ensuring direct contact of the cell with the WE. Electrodes on either side of the cell are connected to high-impedance amplifiers for passive measurement of voltage across the cell (as shown).

Three-dimensional (3-D) finite element models (FEM) of PC12 cells in the recording chamber were generated to estimate perturbations in cell membrane potential induced by the inhomogeneous extracellular electric field (Comsol Multiphysics, AC/DC Module, Burlington, MA). Simulations used a 10 µm × 10 µm cross-sectional area channels to match the microchips. Two cell sizes were simulated, a small 8 µm diameter sphere positioned in the center of the channel, and an 18 µm diameter cell squeezed into the microchannel with a 100 nm saline shunt path between the cell and the channel walls at its tightest point. Dielectric properties were estimated from analogous rat adrenal gland cells based on Brandt et al.³¹ The cell plasma membrane was modelled using a distributed dielectric layer comprised of an area specific conductivity of 2.85 S/m² and a relative permittivity of 26. The cell cytoplasm was modelled with a relative permittivity of 52 and a conductivity of 1.5 S/m. No electrode double-layer effects were modelled as the emphasis was on the voltage differential across the cell in the microchamber. The surrounding cell media was modelled with a relative permittivity of 78 and conductivity of 1.4 S/m (e.g. saline).

Microchips were fabricated at the Utah Nano-Science and Engineering Laboratories at the University of Utah using surface micromachining techniques as previously described.³⁰ A photomicrograph of the microchip is shown in Fig. 1. Fig. 1a shows a close-up view of the recording chamber as a PC12 cell (Fig. 1, (iii)) is pulled into it. The chamber is formed as the 10 µm (depth) fluidic channel (Fig. 1, (i)) channel narrows to a 10 µm width over an interdigitated electrode array of eight independently addressable gold (Au) electrodes (Fig. 1, (ii)). The cell is immobilized over the working electrode (Fig. 1, WE) by a ∼5 µm constriction in the channel as shown in Fig. 1b (dotted ellipse).

Neurotransmitter release from the PC12 cells was measured using a three electrode arrangement with a Au WE (Fig. 1, WE), a Au counter electrode (Fig. 1, CE) and silver/silver chloride (Ag/AgCl) reference electrode (RE) with all controlled by a NPI VA-10 potentiostat (National Precision Instruments (NPI), Tamm, Germany). In a subset of the experiments, individual electrodes on either side of the cell were wired to high-impedance voltage following op-amps (OPA356, Texas Instruments, USA) for passively measuring the voltages in the channel. These outputs were passed to a differential amplifier (DPA-2FX, NPI) to determine the voltage differential across the cell as schematized in Fig. 1. The specific electrode used as the WE was varied between experiments, but the relative positions of the three electrodes were maintained. Prior to each experiment the WE was electrochemically cleaned by cycling the electrodes from −300 mV to +1.5 V (vs Ag/AgCl) in a 0.1 M sulfuric acid (H₂SO₄, Sigma-Aldrich) solution until stable voltammograms were obtained. For chronoamperometry, the WE was held at a potential of +700 mV (vs Ag/AgCl) to ensure oxidation of any released catecholamines. The low-pass filtered (3 kHz, 2-pole Bessel) voltage (current-converted) output of the VA-10 was passed through two additional low-pass (3 kHz) Bessel filters (LPBF-01 G and BF-48DGX, NPI) creating an effective 10-pole Bessel filter and scaling amplifier gain of 10 in the third stage. For additional electrical stimulation, or triggering, of neurotransmitter release, two large, axially-positioned electrodes (not shown in Fig. 1) were used to apply a mirrored, 5 s voltage pulse across the cell with the electrode apical to the cell set to +800 mV and the electrode basal to the cell set to −800 mV. These relatively large voltages were applied to account for the high-impedance of the electrode double layers. Because of these impedances, fluid voltages in the channel would typically be more than an order of magnitude less than the applied voltage.

Dynamic control of the instrumentation was achieved through GPIB (IEEE-488; National Instruments) connections linked to a custom software (IGOR Pro; Wavemetrics, Lake Oswego, OR). All analog signals were sampled with a 16 bit A/D converter (ITC-1600, Heka, Bellmore, NY) at 5 kHz.

For off-line data analysis, the WE current was digitally band-pass filtered between 5 Hz and 250 Hz using a sliding Hanning window. Quantal release times were identified by thresholding at a level five times the standard deviation of the baseline noise. Once quantal event times were identified, the release parameters of half width (t_1/2), peak height, and quantal molar quantity were calculated directly from the raw (unfiltered) data. The number of molecules of transmitter released (N, used for determining molar quantity) was calculated by applying Faraday's law, Q = nFN, where n was the number of electrons transferred per molecule (assumed to be 2 for catecholamines), F was Faraday's constant (96,485 C/mol) and Q was the total charge per spike determined by integrating the area under each current spike. Goodness of fit comparisons of single versus double-Gaussian fits of the data were performed by comparing root mean squared deviations (RMSD) values of the fits to the actual data.

Results and discussion

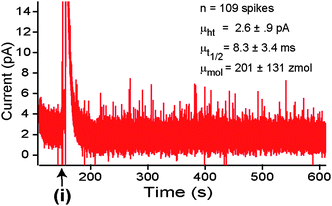
PC12 cells and PC12 cell clusters with dimensions on the order of the channel or larger (i.e.Fig. 1) spontaneously released catecholamines upon positioning over the WE. Fig. 2 shows a typical record for a cluster of six cells in the channel. Individual release events or “quanta” from the cells were clearly resolvable with the microchip. A representative quantal release event is shown in Fig. 2. (Inset (i)). Detection was sensitive enough to occasionally resolve “foot” events (Fig. 2. Inset (ii)) and “flicker” events (Fig. 2. Inset (iii)) that are presumed to be associated with fusion pore formation and transient release through a fusion pore without full vesicle fusion, respectively.⁷ Initial release typically resulted in a large burst of spikes and a concomitant rise in the baseline current level. We interpret these data as the transient increased vesicle release rate of the readily releasable intracellular vesicle pool and an overall increase in the chamber catecholamine concentration, respectively.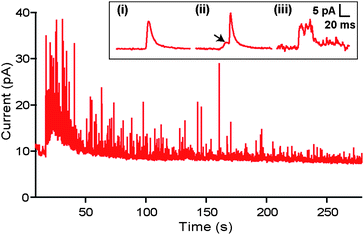

Fig. 2 Quantal events. Example current trace recorded during amperometric (+700 mV vs Ag/AgCl) measurement of release from a cluster of six cells in the recording chamber. (INSET) (i) A typical recorded current spike corresponding to a quantal release event. (ii) In a subset of spikes, “foot” events (indicated by arrow) were detected consistent with the fusion pore formation hypothesis. (iii) Example of a “flicker” event which has been previously interpreted as resulting from closure of the fusion pore prior to full fusion of the synaptic vesicle with the plasma membrane.

Spike analysis was performed for a population of cells (n = 209 cells, k = 25,463 spikes). Fig. 3 shows histograms of the key parameters of quantal release including: a) current, b) spike half-width (t_1/2), and c) cube root of quantal molar quantity. Examination of RMSD values of the fits reveal that all three parameters are significantly better fit with double Gaussian functions than with single Gaussian functions. Unlike their undifferentiated counterparts, dexamethasone-treated PC12 cells are considered to have a homogenous population of vesicle sizes. As a result, the cube root of the quantal molar quantity (which is presumed proportional to vesicle radius cubed) should provide a Gaussian distribution, assuming uniform intra-vesicle biochemical concentration.^27,32,33 Consequently, the emergence of two distinct populations of release events in our data indicated the system detected release events originating from multiple sites around the cell. One pool would represent the immediately oxidized catecholamine released at the site in wet contact with the WE and the other pool representing release from more remote regions of the cell. Detection of the latter pool was likely facilitated by the confined geometry of the channel which would serve to sequester the catecholamine release to the WE. This is supported by the larger t_1/2 and smaller peak amplitudes as diffusional effects would result in a broader, flatter spike as the distance from the release site to the electrode was increased. The cube root of quantal yield of 6.6 ± 08 zmol^1/3 corresponds well with previously reported data for dexamethasone-treated cells (6.7 ± 1.6 zmol^1/3).³² However, even the smaller of the two half-width means of the Gaussian fits (9.3 ± 17 ms) was much larger than that previously reported (2.23 ± 24 sec).²⁹ Rather, the value we report exactly matches that previously reported for undifferentiated cells.⁵ The difference can not be attributed solely to lack of cell contact with the WE as the longer half-widths were witnessed for very large cells and clusters in which direct contact with the WE was assured. One possibility is that the release sites of the cell were in distinct areas of the membrane from that touching the WE. The significantly lower peak height of both distributions (2.2 ± 1 pA and 5.2 ± 1.5 pA) as compared to this prior study (31.4 ± 5.6 pA)²⁹ supports this hypothesis as well, but it is possible that some other factor may have been at play.

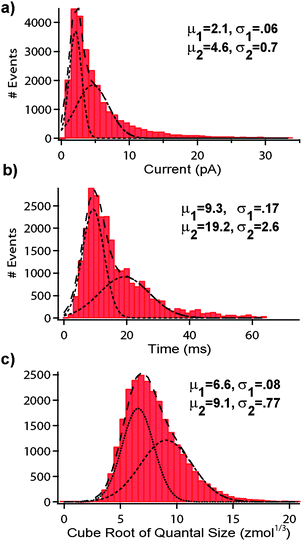Fig. 3 Quantal event analysis. Bin histograms were generated for a population of dexamethasone (5 µM)-treated PC12 cells (n = 209 cells, k = 25,463 spikes). (a) peak height (b) t_1/2 (c) cube root of quantal molar size. Fitting of the curves with double Gaussian functions suggested the detection of spikes from multiple release sites of the cells. Means (µ) and standard deviations (σ) for the Gaussian fits are shown.

Large cells and clusters of cells generally yielded more quantal recordings. To understand and quantify this, cell size was correlated with: (i) number of spikes released in the first sixty seconds of cell firing, (ii) moles per spike, (iii) t_1/2 and (iv) peak height. Cells were visually grouped into four size categories: (1) clusters (n = 61 samples)—consisting of 2 or more cells in the recording channel. (2) large cells (n = 76)—single cells that visually deformed/compressed upon placement over the WE (because they exceeded the channel dimensions). (3) medium cells (n = 34)—single cells that were roughly the size of the channel, with no deformation/compression upon entering. (4) small cells (n = 10)—single cells with visible gaps between the plasma membrane and the channel wall. As data for unresponsive cells was not incorporated into the analysis, the smaller sample sizes for medium and, particularly, small cells were reflective of the low percentage of these cells having detectable release events. Data is presented in Fig. 4 in the form of the mean parameter values ± one standard error. Statistically significant differences for all parameters were detected between large, medium and small cells (p < 0.05, ANOVA). However, for large cells versus cell clusters, the only statistically significant difference was in the number of spikes per cell(s) (p < 0.05, T-test). All other parameters showed no statistical differences between the large cells and cell clusters (p < 0.05, T-test). As both clusters and large cells should fully occlude the channel, the increase in number of spikes for clusters versus large cells was likely a function of the WE picking up spikes from other cells in the channel. However, the differences in spike numbers among the single cell sizes was most probably a function of the direct correlation between the degree of cell depolarization and the size of the cell, as discussed in detail below. Variation of spike frequency with stimulus strength, as we are reporting here, has also been observed in the exposure of bovine adrenal cells to varying-strength depolarizing solutions.⁴ The differences in peak height and t_1/2 for small and medium cells (p < 0.05, T-test) can be explained by diffusional effects. It has been shown that as the WE distance from the cell membrane increases, the spike t_1/2 values broaden and the peak heights decrease.⁴ Given the tightness of fit for larger cells, the seemingly contradictory increase in t_1/2 for large cells can be explained by the cell deformation in the channel. This deformation results in the cell elongating axially in the channel. As a result, release sites are further separated from the 5 µm electrode and there was an increased diffusion distance for detected spikes from remote regions of the cell. Similarly, for the clusters, we would expect that the contribution of neighboring cells to result in an increased t_1/2 and a decreased peak height.

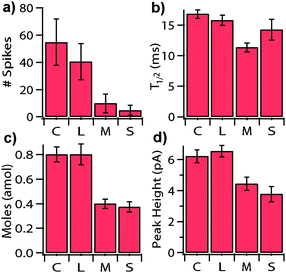Fig. 4 Correlates with cell size. Bars show the mean (±1 standard error) for various spike parameters as a function of cell size. Note: “# of spikes” (a) represents the number of quantal release events in the first 60 seconds of release. The four cell sizes (n = sample size) categories were: C = Clusters (two or more cells, n = 61), L = Large (n = 76), M = Medium (n = 34), S = Small (n = 10).

In a previous study it was suggested that mechanical stress can evoke exocytosis from bovine chromaffin cells,⁴ which, like PC12 cells, are also derived from the adrenal medula. Although mechanical stimuli may have contributed, it was likely a secondary effect in the present study for two reasons. First, small cells, which experienced no detectable mechanical deformation while in the channel, still exhibited detectable electrically evoked release. Additionally, exposure of a population of cells to cytochalasin-D, a known actin-disrupting agent, did not block the exocytosis activity (data not shown). This result rules out exocytosis evoked by cytoskeletal stretch-activated channels or other mechanical-stress related mechanisms. Instead, release in the present study was primarily evoked by the electric field generated by the potentiostat circuit. Fig. 5 shows a FEM result illustrating how the extracellular electric field leads to excitation of the cell. Current injected into the channel from the WE flows around the cell to the ground electrode. This causes an extracellular voltage drop across the cell, indicated in the figure using a color map and contour surfaces. Since the intracellular voltage is nearly isopotential, perturbation of the extracellular voltage depolarizes the side of the cell near the ground electrode and hyperpolarizes the region near the working electrode. We refer to this technique of using extracellular electrodes to pattern the membrane potential of a cell as micro-domain voltage clamp (µVC). Simulations predicted the voltage drop in the channel across the smaller cell (a) to be ∼.4 δv versus as compared to ∼.82 δv for the larger cell (b), where “δv” is the total voltage drop in the fluid media between the two electrodes. Large cells and clusters experience greater depolarizing excitation because of the smaller shunt path and higher resistance to current flow around the cell. Consistent with these simulations, cell clusters and large cells displayed greater release rates than the small cells (Fig. 4a).

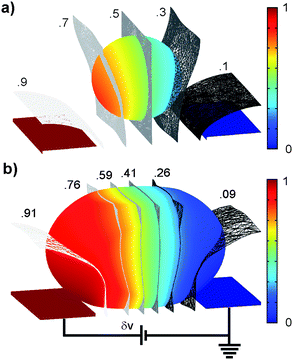Fig. 5 FEM simulations to study effect of cell size. Images show the distributions of the voltages in the channel for a small cell (a) and large cell (b) for an applied WE voltage (DC, “δv” denoted voltage level in the channel). The color scale, indicating the fractional voltage distribution, highlights the electric field patterning technique (µ-domain voltage clamp). Relative to the extracellular fluid, the membrane impedance is very high resulting in the majority of the applied voltage dropping across the cell. The tighter fit of the larger cell creates more depolarization simply due to the restriction of the extracellular shunt path.

In a subset of experiments we used two additional electrodes placed upstream and downstream of the cell to apply additional excitatory electrical stimuli. One example is shown in Fig. 6. Initially, there was no spontaneous exocytosis from this cell. Application of a ±800 mV, 5 s square pulse on the metal electrodes (at 150 s into the record) axially across the cell evoked a maintained catecholamine release. This triggered release was replicated in 11 cells. However, not all cells showed release even after application of an axial voltage pulse. One likely reason is that smaller cells would require a very large extracellular pulse to realize a sufficiently large depolarizing voltage to evoke release. Because the majority of the voltage would drop across the high-impedance gold electrode double-layer, the magnitude of voltage necessary to depolarize the very loose-fitting cell would exceed the “water window” or voltage at which the decomposition of water occurs. As a result, these voltages, and therefore stimulation, would not be realizable for the smallest cells. Additionally, the current densities required would often result in damage to the microelectrodes. Because the larger cells for the most part would fire “spontaneously” with the potentiostat electric field, the resulting subset of cells that were large enough to be depolarized by the triggered pulse and yet small enough to avoid spontaneous release would be expectedly small.

Fig. 6 Pulse evoked release. For small cells that were not sufficiently depolarized by potentiostat electric field, release could occasionally be triggered through the application of an extracellular voltage pulse. The graph above shows the working electrode current trace that shows onset of release following application of a voltage pulse (i).

One potential advantage of the present approach is the possibility for exocytosis flow cytometry and high-throughput. The ability to have the potentiostat in the chronamperometric circuitry serve as both the recording and stimulating mechanism lends itself towards prospective automation. Fig. 7 provides one example of chronoamperometric detection from three different cells as they successively flowed through the recording chamber. The red (lower) trace shows the chronoamperometric current response at the WE and the blue (upper) trace shows the recorded channel voltage differential across the cell using the wiring arrangement shown in Fig 1b. As each cell crossed the WE, quantal release events were observed. The magnitude of the voltage differential and the spike intensity were reflective of the relative cell sizes with the largest cell (iii) producing the greatest response and the smallest cell (ii) the least response.

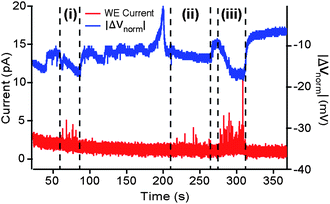Fig. 7 Exocytosis flow cytometry. Results for three separate cells flowing along the channel and crossing the WE in sequence are indicated at points marked i, ii, and iii respectively. Lower trace shows the working electrode current (red) and the upper trace shows the voltage drop (blue) recorded across the cell. Current spikes, corresponding to quantal release events, are detectable for all three cells, with a visible correlation between the spike intensity and the magnitude of (negative) change in ΔV across the cell.

The system reported here does have some drawbacks that are worthy of note. As chronoamperometry does not yield information regarding the type of neurotransmitter released, use of the system in such a mode would be susceptible to potential interference. Improved selectivity for dopamine over the most physiologically-relevant interferent, ascorbic acid, could be achieved by coating the WE with a cation-selective membrane such as Nafion as has been previously demonstrated.³⁴ Alternatively, more descriptive chemical identification of vesicle contents can be achieved with cyclic voltammetry. Additionally, it was observed that the mean spike amplitude for a particular WE on a given microchip would decrease with time (data not shown). This was most likely due to the reduction in electron transfer efficiency due to the build-up of poly-dopamine⁸ on the WE but could also have been due to non-specific adhesion of cellular constituents and extracellular proteins. For large populations of cells, a mechanism for periodic cleaning of the electrodes, for example with cycling in sulfuric acid, might be needed.

Conclusions

We have presented a microchip capable of electrically evoking and electrochemically resolving quantal release events from single cells and cell clusters. Overall detection of catecholamine release from PC12 cells was comparable to the conventional CFE electrochemical techniques of release detection with our ability to resolve quantal release events and even subcomponents of vesicle fusion in the exocytosis process. Potential advantages of the present approach are the simplicity in which cells are loaded using device microfluidics, automated positioning of the electrodes through photolithographic patterning, and exocytosis flow cytometry. Additionally, more rapid studies of populations of cells have the potential to facilitate discovery through statistical observations of rare events such as the formation of the fusion pore and/or flicker events.

Acknowledgements

The authors would like to thank Dr Patrick Tresco and the members of the Keck Lab of the University of Utah for use of their cell culture facilities and guidance in culture techniques, and Dr H.E. Ayliffe for sharing his expertise and valuable advice. This work was supported in part by the National Institutes of Health, NIDCD R01 DC04928, and by National Science Foundation, IGERT NSF DGE-9987616.

Notes and references

1. D. E. Knight, D. A. Tonge and P. F. Baker, Nature, 1985, 317, 719–721.
2. J. M. Edwardson, C.-T. Wang, B. Gong, A. Wyttenbach, J. Bai, M. B. Jackson, E. R. Chapman and A. J. Morton, J. Biol. Chem., 2003, 278, 30849–30853.
3. D. J. Keating, D. Dubach, M. P. Zanin, Y. Yu, K. Martin, Y.-F. Zhao, C. Chen, S. Porta, M. L. Arbone's, L. Mittaz and M. A. Pritchard, Hum. Mol. Genet., 2008, 17, 1020–1030.
4. R. M. Wightman, J. A. Jankowski, R. T. Kennedy, K. T. Kawagoe, T. J. Schroeder, D. J. Leszczyszyn, J. A. Near, J. E. J. Diliberto and H. Viveros, Proc. Natl. Acad. Sci. U. S. A., 1991, 88, 10754–10758.
5. T. K. Chen, G. Luo and A. G. Ewing, Anal. Chem., 1994, 66, 3031–3035.
6. D. J. Leszczyszyn, J. A. Jankowski, H. Viveros, J. Emanuel, J. Diliberto, J. A. Nears and R. M. Wightman, Journal of Biological Chemistry, 1990, 265, 14736–14737.
7. E. R. Travis and R. M. Wightman, Annu. Rev. Biophys. Biomol. Struct., 1998, 27, 77–103.
8. C. Spégel, A. Heiskanen, J. Acklid, A. Wolff, R. Taboryski, J. Emnéus and T. Ruzgas, Electroanalysis, 2007, 19, 263–271.
9. C. Spégel, A. Heiskanen, S. Pedersen, J. Emnéus, T. Ruzgas and R. Taboryski, Lab Chip, 2008, 8, 323–329.
10. P. Chen, B. Xu, N. Tokranova, X. Feng, J. Castracane and K. D. Gillis, Anal. Chem., 2003, 75, 518–524.
11. P. Chen, B. Xu, N. Tokranova, X. Feng, J. Castracane and K. D. Gillis, Anal. Chem., 2003, 75, 518–524.
12. X. Chen, Y. Gao, M. Hossain, S. Gangopadhyay and K. D. Gillis, Lab Chip, 2008, 8, 161–169.
13. X. Sun and K. D. Gillis, Anal. Chem., 2006, 78, 2521–2525.
14. A. F. Dias, G. Dernick, V. Valero, M. G. Yong, C. D. James, H. G. Craighead and M. Lindau, Nanotechnology, 2002, 13, 285–289.
15. I. Hafez, K. Kisler, K. Berberian, G. Dernick, V. Valero, M. G. Yong, H. G. Craighead and M. Lindau, Proc. Natl. Acad. Sci. U. S. A., 2005, 102, 13879–13884.
16. Y. Chen, H.-f. Cui, J.-s. Ye, S.-c. Chong, T.-m. Lim, F.-s. Sheu and W.-c. Hui, J. Phys. Conf. Ser., 2006, 34, 198–203.
17. H.-F. Cui, J.-S. Ye, Y. Chen, S.-C. Chong, X. Liu, T.-M. Lim and F.-S. Sheu, Sens. Actuators, B, 2006, 115, 634–641.
18. H.-F. Cui, J.-S. Ye, Y. Chen, S.-C. Chong and F.-S. Sheu, Anal. Chem., 2006, 78, 6347–6355.
19. W.-H. Huang, W. Cheng, Z. Zhang, D.-W. Pang, Z.-L. Wang, J.-K. Cheng and D.-F. Cui, Anal. Chem., 2004, 76, 483–488.
20. M. W. Li, D. M. Spence and R. S. Martin, Electroanalysis, 2005, 17, 1171–1180.
21. C. Amatore, S. Arbault, F. Lemaître and Y. Verchier, Biophys. Chem., 2007, 127, 165–171.
22. C. Amatore, S. Arbault, Y. Chen, C. Crozatier, F. Lemaíre and Y. Verchier, Angew. Chem., Int. Ed., 2006, 45, 4000–4003.
23. Y. Chen, C. Guo, L. Lim, S. Cheong, Q. Zhang, K. Tang and J. Reboud, Anal. Chem., 2008, 80, 1133–1140.
24. S. E. Hochstetler, M. Puopolo, S. Gustincich, E. Raviola and R. M. Wightman, Anal. Chem., 2000, 72, 489–496.
25. S. E. Zerby and A. G. Ewing, Journal of Neurochemistry, 1996, 66, 651–657.
26. L. A. Greene and A. S. Tischler, Proc. Natl. Acad. Sci. U. S. A., 1976, 73, 2424.
27. R. H. S. Westerink and A. G. Ewing, Acta Physiologica, 2008, 192, 273–285.
28. L. A. Greene, J. M. Aletta, A. Rukenstein and S. H. Green, in Methods in Enzymology, Academic Press, Inc, 1987, pp. 207–216.
29. A. Elhamdani, M. E. Brown, C. R. Artalejo and H. C. Palfrey, The Journal of Neuroscience, 2000, 20, 2495–2503.
30. G. M. Dittami, H. E. Ayliffe, C. S. King and R. D. Rabbitt, J. Microelectromech. Syst., 2008, 17, 850–862.
31. B. L. Brandt, S. Hagiwara, Y. Kidokoro and S. Miyazaki, Journal of Physiology, 1976, 263, 417–439.
32. R. H. S. Westerink and H. P. M. Vijverberg, J. Neurochem., 2002, 80, 861–873.
33. R. H. S. Westerink, A. d. Groot and H. P. M. Vijverberg, Biochem. Biophys. Res. Commun., 2000, 270, 625–630.
34. M. P. Brazell, R. J. Kasser, K. J. Rennter, J. Feng, B. Moghaddam and R. N. Adams, J. Neurosci. Methods, 1987, 22, 167–172.

---