Anion-dependent spin crossover in solution for an iron(II) complex of a 1H-pyrazolyl ligand

Abstract

The spin-crossover equilibrium midpoint temperature (T_1/2) in [Fe(3-bpp)₂]X₂ (3-bpp = 2,6-di{pyrazol-3-yl}pyridine) varies from 259 K when X⁻ = BPh₄⁻ to 277 K when X⁻ = Br⁻, at 10 mM concentrations in an acetone–water solvent mixture.

---

Metal-organic spin-crossover (SCO) materials continue to be heavily studied in the solid state,¹ with particular current interest in their applications in nanoscience.² However, while ultrafast spectroscopy in solution has elucidated the atomistic mechanism of the spin-transition event,³ interest in solution-phase SCO has otherwise developed more slowly.^4,5 Individual examples of cooperative SCO switching in micelles,⁶ a spin-state dependent MRI response from an iron complex,⁷ an SCO complex that binds barbiturate in solution⁸ and designs of anion-responsive SCO centre,^9,10 have all been demonstrated. These results imply the UV/vis and paramagnetic NMR changes induced by SCO could be of use for sensor applications.⁵

The anion-dependent complexes [Fe(H₂bip)₂L]²⁺ (H₂bip = 2,2′-bi{1,4,5,6-tetrahydropyrimidine}; L = H₂bip, bipy etc.) are the best characterised system where SCO is triggered by supramolecular host–guest binding.⁹ The low-spin state of these complexes is favoured in the presence of strongly associating halide anions, which interact with the chelating N–H groups at the periphery of the H₂bip ligands. Earlier investigations of anion-dependent SCO in other compounds had shown negative results,^11,12 possibly because those studies were performed in aqueous solution or water-containing solvent mixtures. Water tends to disrupt host–guest interactions to anions, all other things being equal, because of its polarity and strong hydrogen-bonding character.¹³ A contributing factor to the successful observation of anion binding by [Fe(H₂bip)₂L]²⁺ may be that those studies were performed in the less competitive solvent dichloromethane.⁹

The complex [Fe(3-bpp)₂]²⁺ (1²⁺; 3-bpp = 2,6-di{pyrazol-3-yl}pyridine) has been important to the development of several aspects of SCO research.¹⁴ Its chemistry was originally developed by Goodwin et al.,^12,15–17 but it has since been employed by others in a variety of supramolecular and multi-functional spin-crossover materials.^18,19 These studies have been facilitated by the unusual stability of 1²⁺ in water,²⁰ which has allowed a large number of salts of this complex to be precipitated and crystallised. Twenty years ago Goodwin et al. reported solution-phase SCO data for the I⁻, BF₄⁻ and PF₆⁻ salts of 1²⁺ in an unspecified acetone–water mixture, concluding that “…all three salts show essentially the same behaviour”.¹² However, reexamination of their data implies that the SCO midpoint temperature (T_1/2) for [Fe(3-bpp)₂]I₂ (1I₂) lies 10 K higher than for the other two salts (ESI†). That follows the trend expected from the [Fe(H₂bip)₂L]²⁺ system,⁹ and would be another rare observation of anion-dependent spin-crossover. This result required clarification, however, since SCO in 1²⁺ in acetone–water is sensitive to the composition of the solvent mixture.²⁰ We report here a re-examination of this system which confirms that guest-responsive SCO can be observed in 1²⁺, even in a competitive solvent.

The salts 1X₂ (X⁻ = BPh₄⁻, BF₄⁻, CF₃SO₃⁻, NO₃⁻ and Br⁻) were prepared by the literature procedures.‡^12,15,19 The BPh₄⁻, BF₄⁻ and CF₃SO₃⁻ salts were recrystallised from MeNO₂/Et₂O, while the other less soluble salts were recrystallised from MeOH/Et₂O. While 1[BF₄]₂ was isolated as a solvent-free powder after drying in vacuo, all the other salts contained water or methanol of crystallisation in their purified forms by microanalysis (ESI†; hydrate formation is a common feature of the chemistry of 1X₂ salts¹⁴). Preliminary screening by ¹H NMR in CD₃CN, (CD₃)₂CO and a 9 : 1 v/v (CD₃)₂CO–D₂O mixture at 293 K established a small, but consistent dependence of the paramagnetic isotropic shifts from 1X₂ on the anion X⁻ (ESI†). In both solvents, the contact shifts (and hence the magnetic moment²¹) of the sample followed the order in X⁻ : BPh₄⁻ ≈ BF₄⁻ > CF₃SO₃⁻ > NO₃⁻ ≈ Br⁻ (1[NO₃]₂ and 1Br₂ were only soluble in the mixed solvent system). This is the trend expected if the high–low-spin state population of the complex in solution is perturbed by more coordinating anions.²² Consistent with that suggestion, all five salts gave identical isotropic shifts within experimental error in the more polar solvent CD₃OD, where hydrogen bonding between 1²⁺ and X⁻ should be weaker.

These initial observations were quantified by variable temperature Evans method measurements (Fig. 1, Table 1). These were performed in the 9 : 1 v/v (CD₃)₂CO–D₂O solvent mixture, corresponding to 31.2 mol % D₂O. Addition of water to the solvent was necessary to afford a medium in which all five salts were sufficiently soluble. The data for 1[BF₄]₂ under these conditions are consistent with those we have reported for that salt in other (CD₃)₂CO–D₂O solvent compositions.²⁰

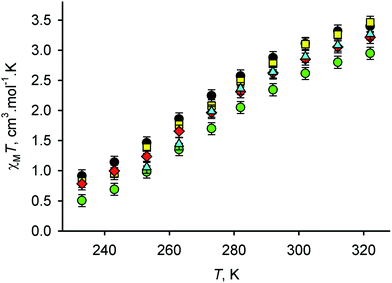Fig. 1 Variable temperature magnetic susceptibility data for 1X₂ in 9 : 1 v/v (CD₃)₂CO–D₂O, with X⁻ = BPh₄⁻ (black circles), BF₄⁻ (yellow squares), CF₃SO₃⁻ (red diamonds), NO₃⁻ (cyan triangles) and Br⁻ (green circles).§

Table 1 Spin-crossover parameters for the salts [Fe(3-bpp)₂]X₂ (1X₂) in 9 : 1 v/v (CD₃)₂CO–D₂O and pure (CD₃)₂CO, measured by Evans method (Fig. 1 and 2).§ See ref. 22 for the definition of β^N

X^−	β^N	T1/2^a, K	ΔH, kJ mol^−1	ΔS, J mol^−1 K^−1
a From ref. 12. The stoichiometry of the (CD3)2CO–D2O solvent mixture used in ref. 12 was not specified, but is probably similar to that in this work.^20b From ref. 20.
1X2, 9 : 1 v/v (CD3)2CO–D2O
BPh4^−	0	259(1)	31.5(4)	121(2)
PF6^−^a	0.64	258	—	—
BF4^−	0.69	261(1)	30.6(4)	117(2)
CF3SO3^−	0.74	264(1)	29.9(4)	113(2)
NO3^−	0.86	268(1)	33.2(4)	124(2)
I^−^a	0.88	268	—	—
Br^−	0.93	274(1)	25.9(4)	95(2)
1X2, (CD3)2CO
BPh4^−	0	243(1)	20.3(2)	83(1)
BF4^−^b	0.69	247(1)	24.8(2)	100(1)
CF3SO3^−	0.74	252(1)	22.0(2)	87(1)
1[BPh4]2 + y[NBu4]Br, 9 : 1 v/v (CD3)2CO–D2O
y = 0	—	259(1)	31.5(4)	121(2)
y = 0.78	—	264(1)	29.7(4)	111(2)
y = 1.71	—	269(1)	25.0(4)	93(2)

---

All five salts exhibit an SCO equilibrium under these conditions, centred just below room temperature (Fig. 1). The T_1/2 values obtained show the clear trend in X⁻:

BPh₄⁻ ≈ BF₄⁻ > CF₃SO₃⁻ > NO₃⁻ > Br⁻

This correlates perfectly with the hydrogen-bonding capability of those anions, as expressed by Lungwitz and Spange's β^N parameter (Fig. 2).²² Importantly, Goodwin's original data for 1[PF₆]₂ and 1I₂ in an unspecified (CD₃)₂CO–D₂O solvent composition also agree well with these new results (Table 1, Fig. 2).¹² The enthalpy and entropy of SCO for four of the salts in Table 1 (from van'T Hoff isochore plots) are similar, and are consistent with previously reported values for 1[BF₄]₂ in (CD₃)₂CO–D₂O mixtures.²⁰ The exception is 1Br₂, whose ΔH and ΔS values are unexpectedly lower, and closer to those shown by salts of 1²⁺ in pure organic solvents including (CD₃)₂CO (Table 1). A reduction in ΔH and ΔS was also observed when Br⁻ was titrated into 1[BPh₄]₂ (see below, Table 1). We suggest that it may reflect a weaker solvation shell about the 1²⁺ cations induced by the strongly associated Br⁻ anions, which would reduce the rearrangement of the solvent accompanying SCO. That remains to be confirmed, however. Notably nucleophilic displacement of 3-bpp from the iron centre by Br⁻, which is a potential side-reaction in the high-spin form of the complex, would have the opposite effect of raising ΔH and ΔS.⁴

Fig. 2 Plot of the spin-crossover mid-point temperature T_1/2 of the salts 1X₂ vs. the hydrogen-bonding power of the X⁻ anion (β^N (ref. 21)) in 9 : 1 v/v (CD₃)₂CO–D₂O (circles) and pure (CD₃)₂CO (squares).§ The black data points are from this work, while the white circles are the I⁻ and PF₆⁻ salts measured by Goodwin et al.¹² The solvent dependence of these data is discussed in ref. 20.

For comparison, the three 1X₂ salts that are soluble in pure (CD₃)₂CO were also measured in that solvent (Table 1, Fig. 2 and ESI†). The results are consistent with those above in showing a 9 K increase in T_1/2 for 1[CF₃SO₃]₂ compared to 1[BPh₄]₂, a slightly larger difference than in the more polar solvent mixture. Lastly, titration of [NBu₄]Br into 1[BPh₄]₂ in 9 : 1 (CD₃)₂CO–D₂O yielded an increase in T_1/2 with increasing bromide concentration, that is consistent with the behaviour of the pure 1[BPh₄]₂ and 1Br₂ salts (Table 1 and ESI†).

The salts 1[BPh₄]₂, 1[BF₄]₂, 1[CF₃SO₃]₂, 1[NO₃]₂ and 1Br₂ all show the same UV/vis metal-to-ligand charge-transfer (MLCT) maximum, at λ_max = 456 nm (ε_max = 3.6 ± 0.1 × 10³ dm³ mol⁻¹ cm⁻¹) in 9 : 1 v/v (CH₃)₂CO–H₂O at 293 K (ESI†). The invariance of these spectra with the anion present is inconsistent with the Evans method data, since ε_max of this MLCT band should increase with T_1/2 which raises the low-spin fraction of the complex at room temperature.²⁰ That might reflect the sample concentrations in the UV/vis measurements (0.2 mM), which were ca. 50× lower than for the Evans method experiments (10 mM). Low concentrations promote host–guest dissociation in solution, which would explain the discrepancy between the techniques.

In conclusion, we have demonstrated a dependence between spin-crossover in [Fe(3-bpp)₂]²⁺ (1²⁺) and the presence of hydrogen bonding anions, in a polar solvent mixture at NMR concentrations (ca. 10 mM). As with the [Fe(H₂bip)₂L]²⁺ system,⁹ more strongly associating anions favour the low-spin state of the complex and increase T_1/2. That is noteworthy, because evidence for the influence of hydrogen-bonding anions on T_1/2 in solid SCO materials has been contradictory up to now.²³ The sensitivity of 1²⁺ to hydrogen bonding to anions (and to solvent²⁰) arises because the hydrogen bond-donor N–H groups in 3-bpp are directly covalently bonded to the metal-donor N atoms. Hence small perturbations in the electronic character of the ligand, caused by changes in hydrogen bonding, are transmitted efficiently to the coordinated iron atom.

Although the response of T_1/2 to different anions in 1²⁺ is smaller than in [Fe(H₂bip)₂L]²⁺ derivatives, this work was performed in more competitive solvents (including an acetone–water mixture) where hydrogen bonding between 1²⁺ and X⁻ is expected to be weaker.¹³ The fact that any correlation between T_1/2 and X⁻ is observed under our conditions is noteworthy for a monodentate hydrogen bond-donor like 1²⁺, and confirms that SCO in 1²⁺ is sensitive to host–guest interactions. Therefore, the [Fe(3-bpp)₂]²⁺ motif is a promising platform for the development of SCO-based sensor applications. Our current work aims to modify the 3-bpp ligand design, to maximise its host–guest binding capabilities.

Acknowledgements

This work was funded by the University of Leeds.

Notes and references

1. Spin-crossover materials – properties and applications, ed. M. A. Halcrow, John Wiley & Sons, Chichester, UK, 2013, p. 568.
2. A. Bousseksou, G. Molnár, L. Salmon and W. Nicolazzi, Chem. Soc. Rev., 2011, 40, 3313.
3. A. Cannizzo, C. J. Milne, C. Consani, W. Gawelda, C. Bressler, F. van Mourik and M. Chergui, Coord. Chem. Rev., 2010, 254, 2677.
4. N. Hassan, A. B. Koudriavtsev and W. Linert, Pure Appl. Chem., 2008, 80, 1281.
5. M. P. Shores, C. M. Klug and S. R. Fiedler, in Spin-crossover materials – properties and applications, ed. M. A. Halcrow, John Wiley & Sons, Chichester, UK, 2013, ch. 10, pp. 281–301.
6. C. Gandolfi, C. Moitzi, P. Schurtenberger, G. G. Morgan and M. Albrecht, J. Am. Chem. Soc., 2008, 130, 14434; P. N. Martinho, Y. Ortin, B. Gildea, C. Gandolfi, G. McKerr, B. O'Hagan, M. Albrecht and G. G. Morgan, Dalton Trans., 2012, 41, 7461.
7. J. Hasserodt, New J. Chem., 2012, 36, 1707.
8. M. C. Young, E. Liew, J. Ashby, K. E. McCoy and R. J. Hooley, Chem. Commun., 2013, 49, 6331.
9. Z. Ni and M. P. Shores, J. Am. Chem. Soc., 2009, 131, 32; Z. Ni and M. P. Shores, Inorg. Chem., 2010, 49, 10727; Z. Ni, A. M. McDaniel and M. P. Shores, Chem. Sci., 2010, 1, 615; Z. Ni, S. R. Fiedler and M. P. Shores, Dalton Trans., 2011, 40, 944.
10. A. M. McDaniel, C. M. Klug and M. P. Shores, Eur. J. Inorg. Chem., 2013, 943.
11. H. L. Chum, J. A. Vanin and M. I. D. Holanda, Inorg. Chem., 1982, 21, 1146; J. W. Turner and F. A. Schultz, Inorg. Chem., 2001, 40, 5296.
12. K. H. Sugiyarto, D. C. Craig, A. D. Rae and H. A. Goodwin, Aust. J. Chem., 1994, 47, 869.
13. S. Kubik, Chem. Soc. Rev., 2010, 39, 3648.
14. M. A. Halcrow, Coord. Chem. Rev., 2005, 249, 2880.
15. K. H. Sugiyarto and H. A. Goodwin, Aust. J. Chem., 1988, 41, 1645; K. H. Sugiyarto, K. Weitzner, D. C. Craig and H. A. Goodwin, Aust. J. Chem., 1997, 50, 869.
16. K. H. Sugiyarto, M. L. Scudder, D. C. Craig and H. A. Goodwin, Aust. J. Chem., 2000, 53, 755.
17. T. Buchen, P. Gütlich and H. A. Goodwin, Inorg. Chem., 1994, 33, 4573; T. Buchen, P. Gütlich, K. H. Sugiyarto and H. A. Goodwin, Chem.–Eur. J., 1996, 2, 1134; S. Marcén, L. Lecren, L. Capes, H. A. Goodwin and J.-F. Létard, Chem. Phys. Lett., 2002, 358, 87; K. H. Sugiyarto, W.-A. McHale, D. C. Craig, A. D. Rae, M. L. Scudder and H. A. Goodwin, Dalton Trans., 2003, 2443.
18. E. Coronado, M. C. Giménez-López, C. Giménez-Saiz and F. M. Romero, CrystEngComm, 2009, 11, 2198; M. Clemente-León, E. Coronado, M. C. Giménez-López, F. M. Romero, S. Asthana, C. Desplanches and J.-F. Létard, Dalton Trans., 2009, 8087; I. A. Gass, S. R. Batten, C. M. Forsyth, B. Moubaraki, C. J. Schneider and K. S. Murray, Coord. Chem. Rev., 2011, 255, 2058.
19. P. King, J. J. Henkelis, C. A. Kilner and M. A. Halcrow, Polyhedron, 2013, 52, 1449.
20. S. A. Barrett, C. A. Kilner and M. A. Halcrow, Dalton Trans., 2011, 40, 12021.
21. B. Weber and F. A. Walker, Inorg. Chem., 2007, 46, 6794.
22. R. Lungwitz and S. Spange, New J. Chem., 2008, 32, 392.
23. M. A. Halcrow, Chem. Soc. Rev., 2011, 40, 4119 and references therein.
24. D. Fedaoui, Y. Bouhadja, A. Kaiba, P. Guionneau, J.-F. Létard and P. Rosa, Eur. J. Inorg. Chem., 2008, 1022; F. El Hallak, P. Rosa, P. Vidal, I. Sheikin, M. Dressel and J. van Slageren, EPL, 2011, 95, 57002.

---

Footnotes

† Electronic supplementary information (ESI) available: Experimental details, elemental microanalysis, NMR, Evans method and UV/vis data. See DOI: 10.1039/c4ra00230j

‡ The salt 1[NCS]₂ (ref. 16) was also investigated in this work. However, solutions of this compound substantial amounts of uncoordinated 3-bpp by ¹H NMR (ESI†), which probably reflects competitive displacement of 3-bpp from the metal centre by the nucleophilic NCS⁻ ion.²⁴ For this reason, 1[NCS]₂ was not investigated further during this study. Smaller amounts (<10%) of free 3-bpp are also present in solutions of 1[NO₃]₂ and 1Br₂ by NMR, and ligand displacement equilibria may make a small contribution to ΔH and ΔS of SCO in those salts.^4,20

§ The differing values of χ_MT at the high- and low-temperature ends of these plots reflect the temperature window of the measurements, which was limited by the liquid range of the solvent. Hence the data do not cover the full the spin-state equilibria, which will span a temperature range of ca. 150 K from start to finish.^4,5 The T_1/2 values in Table 1 and Fig. 2, and the van'T Hoff plots, were calculated assuming that the fully high-spin complex exhibits χ_MT = 3.5 ± 0.1 cm³ mol⁻¹ K under all the conditions used. That approximation is supported by our earlier work.²⁰

---