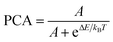DOI:
10.1039/C4RA02779E
(Paper)
RSC Adv., 2014,
4, 24170-24175
Optical temperature sensing of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor
Received
29th March 2014
, Accepted 22nd May 2014
First published on 23rd May 2014
Abstract
The hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor was prepared by the high temperature solid-state reaction. Novel six-photon ultraviolet up-conversion emissions in the range of 260–320 nm are observed under 1.54 μm excitation. Using the ratio of 523 nm and 542 nm fluorescence emissions, low-temperature optical temperature sensing in the resulting phosphor is studied. The temperature sensitivity of the resulting phosphor in the region of 5–300 K is obtained by measuring the temperature dependent fluorescence intensity ratio of the 523 nm and 542 nm emissions originated from two thermally coupled 2H11/2 and 4S3/2 emitting levels. The temperature sensitivity with a maximum sensitivity of 0.0022 K−1 at 338 K is obtained experimentally. The results reveal that the resulting phosphor may be a promising material for the sensing system at low temperature.
1. Introduction
Among the metal ions, rare earth ions have been reported as excellent luminescence centers in powders and glass-ceramics, due to their abundant 4f energy level structures.1–4 Notably, every rare earth ion has a couple of adjacent thermally coupled levels with a very small energy gap of about 1000 cm−1, such as Er3+: 2H11/2 and 4S3/2, Tm3+: 3F2,3 and 3H4, and so on. According to the Boltzmann distribution, the populations of these adjacent energy levels change the temperature environment around the rare earth ions.5 The luminescence intensity ratio originated from the two adjacent energy levels also changes with temperature. This temperature dependent fluorescence intensity ratio was reported as the precise evaluation scale of optical temperature sensing.6,7 Recently, based on up-conversion luminescence emissions, optical temperature sensing in the medium temperature range from 300 K to 800 K were explored in Er3+ doped fluorotellurite glass under 800 nm excitation, Er3+/Yb3+ co-doped Y2SiO5 powders under 975 nm excitation, Tm3+/Yb3+ codoped oxyfluoride glass ceramic under 980 nm excitation, and Er3+/Yb3+ co-doped chalcogenide glass under 1.06 μm excitation.8–10 The optical thermometry was achieved by measuring fluorescence intensity ratio of two thermally coupled levels 2H11/2 and 4S3/2 of Er3+ ion with slow increase of ambient temperature under a near-infrared diode laser excitation.
However, presently, the optical thermometry has been reported rarely in the low temperature range from 5 K to 300 K. Optical temperature sensors based on infrared up-conversion were mainly studied in the medium temperature range from 300 K to 800 K in oxides and glass ceramics with large phonon energy. In general, these materials have weak fluorescence emissions, due to thermal relaxation induced by large phonon energy.11 It is necessary to increase excitation power of optical pumping source to measure weak fluorescence emission signals with help of highly sensitive spectrophotometers. Instead of oxides and glass ceramics, fluoride crystals were reported as most efficient up-conversion materials, due to their low phonon energy and the high solubility between cations and doping ions.12–14 Thus, it remains a large space worthy of exploration in optical temperature sensors based on infrared up-conversion of fluoride crystals. Moreover, the thermal self-interference induced by the near-infrared diode laser excitation with the same power density will become smaller and smaller with increasing the wavelength of near-infrared diode laser from 800 nm to 1.06 μm.15 Thus, optical temperature sensors used long-wavelength near-infrared diode laser as excitation source will be a trend to obtain exact optical thermometry. In this work, we synthesize a novel hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor as the transducer element and study optical temperature sensing in low temperature range from 5 K to 300 K under 1.54 μm excitation. The transducer converts up-conversion excited green fluorescence emission of Er3+ ions into direct temperature information. The selection of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor as the doping host is motivated by its high chemical and thermal stability as well as low phonon energy, which favors suppression of nonradiative losses and thereby improves the luminescence of the optically active Er3+ ions.16
2. Experimental
The hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor was synthesized by a high temperature solid-state reaction. The starting materials are reagent grade 99.95% pure NaF and CaF2, 99.99% pure YF3 and 99.99% pure ErF3. The samples were synthesized according to the molar composition of Na0.82Ca0.08Er0.16Y0.853F4. Accurately weighed 5 g batches of raw materials were thoroughly mixed and moved into corundum crucibles. The batches with an addition of small amount of a fluoridating agent NH4HF2 were heated at 973 K for 6 h in an electric furnace.
Structures of the samples were investigated by X-ray diffraction (XRD) using a X'TRA (Switzerland ARL) equipment provided with Cu tube with Kα radiation at 1.54056 Å in the range of 10° ≤ 2θ ≤ 85°. Luminescence spectra were obtained by an Omni-λ3007 spectrophotometer with a photomultiplier tube equipped with a 1.54 μm laser as the excitation sources. Different temperatures were obtained using a cooling power closed cyclecryo cooler (DE-202).
3. Results and discussion
Fig. 1 shows representative X-ray diffraction pattern and crystal structure of the Na0.82Ca0.08Er0.16Y0.853F4 at the room temperature. X-ray diffraction in Fig. 1(a) shows that the sample has the pure hexagonal Na0.82Ca0.08Er0.16Y0.853F4 structure, and all diffraction peaks can be indexed by the standard powder diffraction file card no.77-0936. The crystal structure of Na0.82Ca0.08Er0.16Y0.853F4 is homeotypic to hexagonal β-NaYF4 (ICSD 51920).17 Y is substituted by Ca and Er, and its crystal structure is shown in Fig. 1(b). The space group is P63/m and, for the Na0.82Ca0.08Er0.16Y0.853F4, the unit cell dimensions are a = 5.986 Å and c = 3.547 Å. Each unit cell contains 1 formula unit. In the unit cell of Na0.82Ca0.08Er0.16Y0.853F4, there are 4e and 2d two Na1 and Na2 cation sites, 2d Y, Er and Ca same cation site, and 6 h F anion site, respectively.
 |
| | Fig. 1 (a) Power XRD pattern and (b) schematic 3D view of the crystal structure of hexagonal Na0.82Ca0.08Er0.16Y0.853F4. | |
Fig. 2(a) shows Raman scattering spectrum of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 at room temperature. The excitation light source of the Raman scattering spectrum is a 514 nm semiconductor laser. The result in the inset shows four strong peaks corresponding to phonon modes with energy 560 cm−1, 602 cm−1, 620 cm−1 and 640 cm−1, suggesting the hexagonal Na0.82Ca0.08Er0.16Y0.853F4 is an excellent up-conversion material with low phonon energy. The diffuse reflection spectrum of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor is shown in Fig. 2(b). According to the Kubelka–Munk equation in the ref. 18, the band gap of the semiconducting materials can be approximately calculated through measuring its diffuse reflection spectrum. The value of the band gap of the hexagonal Na0.82Ca0.08Er0.16Y0.853F4 crystal can be calculated approximately as 2.05 eV, shown in the inset of Fig. 2(b). The absorption peaks centered at 523 nm, 651 nm, 800 nm and 978 nm correspond to 4I15/2 → 2H11/2, 4I15/2 → 4F9/2, 4I15/2 → 4I9/2 and 4I15/2 → 4I11/2 transitions of Er3+ ions. A strong and broad absorption band centered at 1.54 μm in the near infrared region from 1.45 μm to 1.58 μm correspond to the 4I15/2 → 4I13/2 multiplet transitions of Er3+ ions.
 |
| | Fig. 2 (a) Raman scattering spectrum and (b) diffuse reflection spectrum of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 at room temperature. | |
Under 1.54 μm excitation, with a pump-power density of 60 mW mm−2, the hexagonal Na0.82Ca0.08Er0.16Y0.853F4 crystal emits ultraviolet and visible up-conversion fluorescence, as shown in Fig. 3. Five ultraviolet emission peaks centred at 270 nm, 298 nm, 328 nm, 358 nm, and 378 nm originate from the 4G9/2 → 4I15/2, 2K13/2 → 4I15/2, 2P3/2 → 4I15/2, 2G9/2 → 4I15/2, 2G11/2 → 4I15/2 transitions of Er3+ ion. Four visible and infrared emission peaks centred at 407 nm, 523 nm, 541 nm, 658 nm, and 805 nm originate from the 2H9/2 → 4I15/2, 2H11/2 → 4I15/2, 4S3/2 → 4I15/2, 4F9/2 → 4I15/2, and 4I9/2 → 4I15/2 transitions of Er3+ ion. As reported, ultraviolet emissions of Er3+ were obtained in Yb3+–Er3+ codoped β-NaYF4 by the energy transfer (ET) from Er3+ to Yb3+ and subsequently the back ET from Yb3+ to Er3+.19 However, ultraviolet emissions of Er3+ in the hexagonal Na0.82Ca0.08Er0.16Y0.853F4 crystal are observed without the assistance of Yb3+ ions. Thus, it is necessary to explore the ultraviolet and visible up-conversion processes. In order to understand the up-conversion mechanism, we investigate the pumping power dependence of up-conversion fluorescence intensity. For an unsaturated up-conversion process, the emission intensity IUC is proportional to the nth power of the infrared excitation intensity IIR:
where
n is the number of infrared photons absorbed per upconverted photon emitted.
20 Fig. 4 shows the double logarithmic plots of excitation power dependence of upconversion intensity under 1.54 μm excitation. If as a function of
IIR under 1.54 μm excitation, in which the
n values are obtained from the slope of the linear fit. The values of slope
n are 5.81, 5.71, 4.91, 5.01, 3.67, 2.86, 2.61, and 1.85 for the 270 nm, 298 nm, 328 nm, 378 nm, 406 nm, 542 nm, 658 nm, and 805 nm emissions, respectively, as shown in
Fig. 4. These
n values reveal that the above transitions may originate from six-, six-, five-, five-, four-, three-, three-, and two-photon up-conversion processes, respectively. The up-conversion emissions peaked at 270 nm and 298 nm come from the
4G
9/2 →
4I
15/2 and
2K
13/2 →
4I
15/2 transitions, which demonstrates that populating the
4G
9/2 and
2K
13/2 levels needs six 1.54 μm photons. The up-conversion emissions peaked at 328 nm and 378 nm originate from the transitions
2P
3/2 →
4I
15/2 and
2G
11/2 →
4I
15/2, which demonstrates that populating the
2P
3/2 and
2G
11/2 levels needs five1.54 μm photons. The up-conversion emissions peaked at 406 nm originates from the transition
2H
11/2 →
4I
15/2, indicating that populating the
2H
11/2 level needs four 1.54 μm photons. The up-conversion emissions peaked at 542 nm and 658 nm come from the transitions
4S
3/2 →
4I
15/2 and
4F
9/2 →
4I
15/2, indicating that populating the
4S
3/2 and
4F
9/2 levels needs three 1.54 μm photons. The up-conversion emissions peaked at 805 nm comes from the transition
4I
9/2 →
4I
15/2, indicating that populating the
4I
9/2 level needs two 1.54 μm photons. The number of photons decrease sharply when the excitation power exceeds some digital, owing to the competition between the linear decay and the upconversion processes for the depletion of the intermediate excited states.
20
 |
| | Fig. 3 Upconversion emission spectrum of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 at room temperature under 1.54 μm excitation. | |
 |
| | Fig. 4 Log intensity vs. log excitation power density of hexagonal Na0.82Ca0.08Er0.16Y0.853F4. | |
To further recognize the up-conversion mechanism in the sample, possible up-conversion processes are schematically given in the energy level diagram of Er3+, as shown in Fig. 5. The energy transfer between Er3+ ions is thought as the main upconversion mechanism, when the Er3+ concentration of optical active center is high.1 The Er3+–Er3+ energy transfer under 1.54 μm excitation is achieved by the following thirteen transfer processes:
| | |
(1) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (2) |
| | |
(2) 4I13/2 (Er3+) + 4I15/2 (Er3+) → 4I15/2 (Er3+) + 4I13/2 (Er3+),
| (3) |
| | |
(3) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (4) |
| | |
(4) 4I13/2 (Er3+) + 4I13/2 (Er3+) → 4I15/2 (Er3+) + 4I9/2 (Er3+),
| (5) |
| | |
(5) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (6) |
| | |
(6) 4I13/2 (Er3+) + 4I9/2 (Er3+) → 4I15/2 (Er3+) + 2H11/2 (Er3+),
| (7) |
| | |
(7) 2H11/2 (Er3+) → 4S3/2 (Er3+) + phonons
| (8) |
| | |
(8) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (9) |
| | |
(9) 4I13/2 (Er3+) + 4S3/2 (Er3+) → 4I15/2 (Er3+) + 2H9/2 (Er3+),
| (10) |
| | |
(10) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (11) |
| | |
(11) 4I13/2 (Er3+) + 2H9/2 (Er3+) → 4I15/2 (Er3+) + 2P3/2 (Er3+),
| (12) |
| | |
(12) 4I15/2 (Er3+) + 1.54 μm photon → 4I13/2 (Er3+),
| (13) |
| | |
(13) 4I13/2 (Er3+) + 2P3/2 (Er3+) → 4I15/2 (Er3+) + 4D7/2 (Er3+).
| (14) |
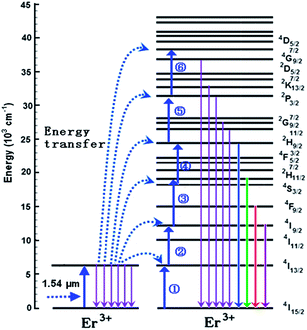 |
| | Fig. 5 Six-step upconversion process between two erbium Er3+ ions under 1.54 μm excitation. | |
As a result, the 4G9/2, 2K13/2, 2P3/2, 2G9/2, 2G11/2, 2H9/2, 2H11/2, 4S3/2, 4F9/2, and 4I9/2 levels are populated, and eight emission peaks at 270 nm, 298 nm, 328 nm, 378 nm, 406 nm, 542 nm, 658 nm, and 805 nm emissions are originated from the 4G9/2 → 4I15/2 (270 nm), 2K13/2 → 4I15/2 (298 nm), 2P3/2 → 4I15/2 (328 nm), 2G11/2 → 4I15/2 (378 nm), 2H11/2 → 4I15/2 (406 nm), 4S3/2 → 4I15/2 (542 nm), 4F9/2 → 4I15/2 (658 nm), and 4I9/2 → 4I15/2 (805 nm) transitions of Er3+ ions, respectively.
As reported in literature, 2H11/2 and 4S3/2 levels of Er3+ ion were proved as thermally coupled levels to achieve optical thermometry by measuring fluorescence intensity ratio of two thermally coupled with slow increase of ambient temperature under a near-infrared diode laser excitation.8,10 To verify that the 2H11/2 and 4S3/2 levels are thermally coupled in the hexagonal Na0.82Ca0.08Er0.16Y0.853F4, the 523 nm and 542 nm upconversion emissions were studied as a function of temperature at 150 K and 300 K. Fig. 6 shows the corresponding emission spectra for the sample at 150 K and 300 K under 1.54 μm excitation. To clearly observe the relative change in the spectra, the emission intensity has been normalized. It can be seen from Fig. 6 that the position of the emission bands hardly changes with the increase of temperature, and an evident enhancement has been achieved for the 523 nm emission at 300 K compared with that at 150 K. In fact, the intensity ratio between 523 nm and 542 nm luminescence obviously varies. The change in the ratio is attributed to the corresponding energy gap between the 2H11/2 and 4S3/2 levels, which allows the ions on 4S3/2 state to be thermally populated onto the 2H11/2 state with the increase of temperature.
 |
| | Fig. 6 The normalized upconversion emission spectra at 150 K and 300 K under 1.54 μm excitation of hexagonal Na0.82Ca0.08Er0.16Y0.853F4. | |
In order to verify that the 2H11/2 and 4S3/2 levels are suitable to be used as optical temperature sensing, the upconversion emission spectra of hexagonal Na0.82Ca0.08Er0.16Y0.853F4 are studied as a function of temperature ranging from 5 K to 300 K under 1.54 μm excitation. According to the theory in ref. 6 and 7, the FIR (fluorescence intensity ratio) from the thermally coupled levels of active ions is modified as
| |
FIR = IU/IL = A![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(−ΔE/kBT), exp(−ΔE/kBT),
| (15) |
where
IU and
IL are intensities of emissions from the upper and the lower thermally coupled levels,
A is a constant, Δ
E is the energy difference between thermally coupled levels,
kB is the Boltzmann constant, and
T is the absolute temperature.
Fig. 7(a) presents the FIR between 523 nm and 542 nm emissions as a function of temperature in the range of 5–300 K. The experimental data are fitted by
eqn (15). It can be observed that the fittings agree well with the experimental data. The
A is fitted to be 1.91, and Δ
E is fitted to be 675.3 cm
−1, close to the value calculated from the emission spectra (670.27 cm
−1). These results confirmed that the
2H
11/2 and
4S
3/2 states are thermally coupled levels. Considering practical applications, it is necessary to investigate the variation of sensitivity with temperature. For this purpose, the relative sensitivity
S has been defined as
 |
| | Fig. 7 (a) FIR between the 523 nm and 542 nm upconversion emissions as a function of temperature in the range of 5–300 K under 1.54 μm excitation. (b) Sensor sensitivity as a function of the temperature for excitation at 1.54 μm. | |
Fig. 7(b) presents the S as a function of temperature. It can be seen that the sensitivity keeps increasing in our experimental temperature range and reaches the maximum value 0.00221 K−1 at 338 K. It means that the hexagonal Na0.82Ca0.08Er0.16Y0.853F4 is sensitive in room temperature area.
As for above route to achieve temperature induced population re-distributions,21 the temperature dependent population re-distribution ability (PCA) can be defined as
| |
 | (17) |
where
IU and
IL are fluorescence intensity generated by the radiative transitions from the upper and lower thermally coupled levels to ground level. According to
eqn (15) and
(17), the PCA can be expressed as
| |
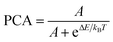 | (18) |
where
A is a fitting constant in
Fig. 7(a), Δ
E is the fitting energy difference between thermally coupled levels,
kB is the Boltzmann constant, and
T is the absolute temperature.
Fig. 8 shows the temperature dependent PCA of
2H
11/2 and
4S
3/2 thermally coupled levels. It can be seen that the value of the PCA is strong dependent on absolute temperature. When the temperature is lower than 100 K, the PCA is very low. On the contrary, the PCA increases sharply, when the temperature is higher than 100 K.
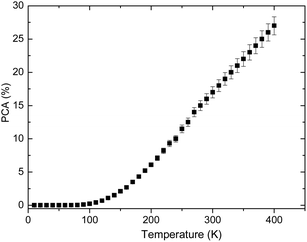 |
| | Fig. 8 Temperature dependent PCA of 2H11/2 and 4S3/2 thermally coupled levels. | |
4. Conclusion
Novel hexagonal Na0.82Ca0.08Er0.16Y0.853F4 phosphor was prepared by high temperature solid-state reaction. UV ultraviolet upconversion emissions centered at 270 nm, 298 nm, 328 nm, 358 nm, and 378 nm are observed under 1.54 μm excitation. Low-temperature optical temperature sensing is studied through measuring temperature dependent fluorescence intensity ratio of 523 nm and 542 nm emissions originated from two thermally coupled 2H11/2 and 4S3/2 levels. The temperature sensitivity with a maximum sensitivity of 0.0022 K−1 was experimentally obtained at 338 K. The resulting phosphor may be promising materials for the sensing system in low temperature.
Acknowledgements
This work was supported by National Natural Science Foundation of China (NSFC51032002, 11374162, 11274173), the key Project of the National High Technology Research and Development Program (“863” Program) of China (no. 2011AA050526), the Science and Technology Support Plan of Jiangsu Province (BE2011191), Natural Science Youth Foundation of Jiangsu Province (BK20130865), and the Scientific Research Foundation of Nanjing University of Posts and Telecommunications (Grant no. NY213022, and NY213113).
References
- F. Auzel, Chem. Rev., 2004, 104, 139–171 CrossRef CAS PubMed.
- H. Guo, H. Zhang, J. J. Li and F. Li, Opt. Express, 2010, 18, 27257–27262 CrossRef CAS PubMed.
- F. Wang, Y. Han, C. S. Lim, Y. H. Lu, J. Wang, J. Xu, H. Y. Chen, C. Zhang, M. H. Hong and X. G. Liu, Nature, 2010, 463, 1061–1065 CrossRef CAS PubMed.
- F. Lahoz, C. P. Rodríguez, S. E. Hernández, I. R. Martín, V. Lavín and U. R. Rodriguez-Mendoza, Sol. Energy Mater. Sol. Cells, 2011, 95, 1671–1677 CrossRef CAS PubMed.
- W. Xu, X. Y. Gao, L. J. Zheng, Z. G. Zhang and W. W. Cao, Sens. Actuators, B, 2012, 173, 250–253 CrossRef CAS PubMed.
- S. A. Wade, S. F. Collins and G. W. Baxter, J. Appl. Phys., 2003, 94, 4743–4756 CrossRef CAS PubMed.
- M. Quintanilla, E. Cantelar, F. CussÓ, M. Villegas and A. C. Caballero, Appl. Phys. Express, 2011, 4, 022601 CrossRef.
- S. F. Leon-Luis, U. R. Rodriguez-Mendoza, E. Lalla and V. Lavín, Sens. Actuators, B, 2011, 158, 208–213 CrossRef CAS PubMed.
- N. Rakov and G. S. Maciel, Sens. Actuators, B, 2012, 164, 96–100 CrossRef CAS PubMed.
- P. V. Santos, M. T. Araujo, A. S. Neto, J. A. M. Neto and A. S. B. Sombra, Appl. Phys. Lett., 1998, 73, 578–580 CrossRef PubMed.
- M. J. Weber, Phys. Rev., 1968, 171, 283–291 CrossRef CAS.
- Y. S. Liu, D. T. Tu, H. M. Zhu, R. F. Li, W. Q. Luo and X. Y. Chen, Adv. Mater., 2010, 22, 3266–3271 CrossRef CAS PubMed.
- J. F. Suyver, J. Grimm, M. K. Veen, D. Biner, K. W. Kramer and H. U. Gudel, J. Lumin., 2006, 117, 1–12 CrossRef CAS PubMed.
- W. P. Qin, C. Y. Cao, L. L. Wang, J. S. Zhang, D. S. Zhang, K. Z. Zheng, Y. Wang, G. D. Wei, G. F. Wang, P. F. Zhu and R. Kim, Opt. Lett., 2008, 33, 2167–2169 CrossRef CAS.
- S. Schietinger, T. Aichele, H. Q. Wang, T. Nann and O. Benson, Nano Lett., 2010, 10, 134–138 CrossRef CAS PubMed.
- F. Vetrone, R. Naccache, V. Mahalingam, C. G. Morgan and J. A. Capobianco, Adv. Funct. Mater., 2009, 19, 2924–2929 CrossRef CAS.
- A. Grzechnik, P. Bouvier, M. Mezouar, M. D. Mathews, A. K. Tyagi and J. Koehler, J. Solid State Chem., 2002, 165, 159–164 CrossRef CAS.
- A. J. Guthrie, R. Narayanaswamy and D. A. Russell, Analyst, 1988, 113, 457–461 RSC.
- K. Z. Zheng, D. Zhao, D. S. Zhang, N. Liu and W. P. Qin, Opt. Lett., 2010, 35, 2442–2444 CrossRef CAS PubMed.
- M. Pollnau, D. R. Gamelin, S. R. Lüthi, H. U. Güdel and M. P. Hehlen, Phys. Rev. B: Condens. Matter Mater. Phys., 2000, 61, 3337–3346 CrossRef CAS.
- X. Wang, J. Zheng, Y. Xuan and X. Yan, Opt. Express, 2013, 21, 21596–21606 CrossRef CAS PubMed.
|
| This journal is © The Royal Society of Chemistry 2014 |
Click here to see how this site uses Cookies. View our privacy policy here. 



![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(−ΔE/kBT),
exp(−ΔE/kBT),