DOI:
10.1039/D1RA07020G
(Paper)
RSC Adv., 2022,
12, 790-797
Study on the extraction of lanthanides by isomeric diglycolamide extractants: an experimental and theoretical study†
Received
19th September 2021
, Accepted 8th December 2021
First published on 24th December 2021
Abstract
Two isomeric diglycolamide (DGA) extractants, N,N′-dimethyl-N,N′-dioctyl diglycolamide (LI) and N,N-dimethyl-N′,N′-dioctyl diglycolamide (LII), were used to perform a comparative study on the extraction performances towards several lanthanides by extraction experiments and theoretical calculations. The experimental results show that both LI and LII show a positive sequence on the extraction of lanthanides, and LI exhibits the higher complex ability with these lanthanides than LII, except for La and Ce. Slope analysis shows that 1:2 or 1:3 complexes are formed between the two ligands and the metal ions. The geometrical structures of the complexes were optimized in the gas phase by density functional theory (DFT) on the basis of complex compositions. The results of bond lengths, MBOs and topological analysis indicated that the electrostatic interaction between metal ions and two amide O atoms in the LII ligand is not as homogeneous as in LI, and this inhomogeneity is likely to be related to the poor extraction performance of LII.
Introduction
Nuclear energy, as a clean energy, can effectively cope with the problems of shortage of fossil energy and carbon emission, and has been applied in more and more countries. Safe and efficient treatment and disposal of high level liquid waste (HLLW) generated from nuclear fuel is always a large challenge and trouble for the sustainable development of nuclear energy.1,2 A strategy of partitioning and transmutation (P&T) was proposed to separate trivalent minor actinides (MA) and other long-lived fission product elements from HLLW, and then transmute them to shorter-lived elements by neutron bombardment,3 so that the minimization of the volume and the radio- and chemo-toxicity of HLLW can be realized.
Diglycolamides (DGAs) are considered to be promising extractants in the field of separation of HLLW due to their exceptionally high affinity for trivalent actinides (An) and lanthanides (Ln) and their benignity to the environment. Asymmetric diglycolamides (ADGAs), which have two different alkyl substituents attached to the amide N atom, in contrast to symmetric DGAs with four same alkyl substituents such as N,N,N′,N′-tetraoctyl diglycolamide (TODGA) and N,N,N′,N′-tetra-2-ethyl-hexyl diglycolamide (TEHDGA), have gained more and more attention recently.4–8 The structure of DGAs, especially the alkyl substituents on the amide N atom, imposes significant influence on their physiochemical performances and extraction behaviors. For example, the DGAs with short chain alkyls such as methyl possess small space hindrance, which reduces the degree of crowding around the metal-ion bonding sites,9 and display admirable extraction capacity to Ln(III) and An(III).10,11 The DGAs with long chain alkyls such as octyl and dodecyl are lipophilic, and can increase the lipophilicity and the degree of solvation of the complexes in non-polar and weakly polar solvents, which is critical for the suppression of the third phase.12 The DGAs with branched chain alkyls such as 2-ethylhexyl and 3,7-dimethyloctyl can not only increase the lipophilicity by virtue of increased van der Waals dispersion forces,13,14 but also exclude some interfering metal ions.15 Due to the existence of two kinds of alkyl substituents attached on the amide N atoms, many developed ADGAs balance superior performances in terms of alleviation of the third phase formation, excellent extractability, enough solubility in aliphatic hydrocarbon diluent and high selectivity.7,16 For example, N,N′-dimethyl-N,N′-dihexyl-diglycolamide exhibits high extraction ability and good separation performance in the meantime.17 N,N-didodecyl-N′,N′-dioctyl-diglycolamide displays high loading concentration for nitric acid and trivalent metal ions and efficient extraction of trivalent metal ions.18
Currently, the asymmetric diglycolamides can be commonly produced in two types, and the structures are shown in Fig. 1: for one type, R1 = R3 ≠ R2 = R4; for the other type, R1 = R2 ≠ R3 = R4. The two types of diglycolamides are geometrical isomers for each other. Although extensive studies about the synthesis and extraction performances of each type of asymmetric diglycolamide have been reported, there are few studies on the differences of the two types of ADGAs. The isomers with different structures differ in electron donor ability, space steric hindrance and coordination ability, which is likely to affect their extraction performances and the structures of complexes with metal ions. Therefore, a comparative study concerning the extraction behavior and mechanism of two isomeric diglycolamides, N,N′-dimethyl-N,N′-dioctyl diglycolamide (LI) and N,N-dimethyl-N′,N′-dioctyl diglycolamide (LII), whose structures are shown in Fig. 1b and c, was performed in this work by extraction experiments combined with theoretical calculations. Density functional theory (DFT) was employed to explore the geometries and chemical bonding properties of M–L (M = La, Sm, Eu, Ho, and Lu) complexes.
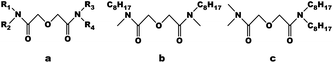 |
| | Fig. 1 (a) The structures of the asymmetric diglycolamides, (b) the structure of LI, and (c) the structure of LII. | |
Experimental
Materials
Diglycolic anhydride (98%), dioctylamine (95%), dimethylamine in THF (2 M), isobutyl chloroformate (98%), 4-methylmorpholine (98%), and lanthanide nitrate hexahydrate (99.9%) were purchased from Aladdin Industrial Corporation (Shanghai, China). The other chemicals were of analytical grade and purchased from Sino pharm Reagent Co., Ltd.
The synthetic protocol of the two ligands is briefly shown in Fig. 2. The ligand LI was synthesized by a two-step method.19 The ligand LII was prepared by the mixed acid anhydride method, and dioctyldiglycolamic acid (DODGAA) was selected as the raw material. The synthesis method has been described previously.4
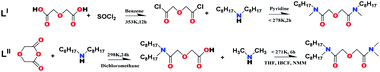 |
| | Fig. 2 The synthetic protocol of LII. | |
Solvent extraction
The solvent extraction experiments about ligand LI and various lanthanides were carried out in our previous studies;16 therefore, the experiments concerning the extraction performance of LII for lanthanide metal ions are reported here. The concentration of Ln(NO3)3·6H2O was set at 3 mM, and the aqueous solutions were generated with a stock solution of Ln(NO3)3·6H2O in different nitric acid concentrations. Organic phases were obtained by dissolving LII in 40/60 (v/v)% n-octanol/kerosene as the diluent. The two phases were equilibrated at a 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 volume ratio with a constant-temperature shaker for 30 min. It is demonstrated that 30 min is enough for the two phases to reach equilibrium. Then the two phases were separated by centrifugation at 2500 rpm for 10 min. An aliquot of the aqueous raffinate was taken into an individual tube containing 0.1 M HNO3 and the metal ion concentration was assayed by ICP-MS (X-II ICP-MS, Thermo Fisher Scientific, Waltham, MA, USA). The distribution ratio, DLn, was derived from
1 volume ratio with a constant-temperature shaker for 30 min. It is demonstrated that 30 min is enough for the two phases to reach equilibrium. Then the two phases were separated by centrifugation at 2500 rpm for 10 min. An aliquot of the aqueous raffinate was taken into an individual tube containing 0.1 M HNO3 and the metal ion concentration was assayed by ICP-MS (X-II ICP-MS, Thermo Fisher Scientific, Waltham, MA, USA). The distribution ratio, DLn, was derived from| |
 | (1) |
[Ln3+](o) and [Ln3+](aq) are the concentrations of Ln(III) in the aqueous phase and organic phase, respectively. The error of the data obtained in the experiment is within 5%.
Infrared spectroscopy
Infrared spectra of the LII and complexes were recorded with a Spectrum 100 FT-IR (PerkinElmer, Waltham, MA, USA), and the detailed information has been described in the previous study.9
Theory calculations
In order to elucidate the coordination structures of metal ions with LI and LII, a series of calculations using DFT with the Gaussian 16 program package were performed.20–22 The B3LYP was used for optimization.23,24 The relativistic effects were considered by small-core scalar-relativistic effective core potentials (RECP), and the 28 electron core pseudopotential basis set associated with a segmented contraction scheme (ECP28MWB_SEG) was chosen for Ln(III).25–27 For light atoms (C, H, O, and N), the polarized 6-311G(d) basis set was employed. To analyze the interaction between Ln(III) and O atoms in ligands, the topology analysis of quantum theory of atoms in molecules (QTAIM) and Mayer bond orders (MBOs) was carried out using Multiwfn software suite version 3.7.28,29
Results and discussion
Solvent extraction
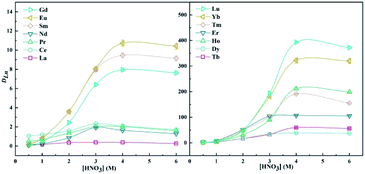
Effect of ligand concentration. As shown in Fig. 4, the distribution ratios of Ln(III) exhibit an increasing trend as the concentration of ligand LII increases from 0.024 M to 0.12 M. The general extraction reaction equation of the DGA class extractant can be expressed as6,32| | |
Ln(aq)3+ + 3NO−3(aq) + nLII(o) ↔ Ln(NO3)3·nLII(o)
| (2) |
where the subscripts (aq) and (o) denote the aqueous and organic phases, respectively.
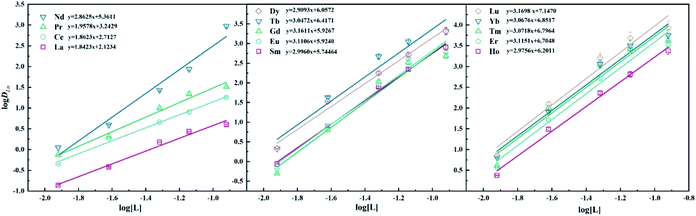 |
| | Fig. 4 Dependency of logDLn on extractant concentration in 40/60 (v/v)% n-octanol/kerosene (O![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1 A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M. 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M. | |
The extraction equilibrium constant K can be expressed as
| |
 | (3) |
where [
LII]
(o) and [Ln(NO
3)
3 ×
nLII]
(o) are the concentrations of the free extractant and the complexes in the organic phase, respectively. [Ln
3+]
(aq) and [NO
3−]
(aq) are the concentrations of Ln(
III) and NO
3− in the aqueous phase, respectively.
γLn3+ and
γNO3− are the ionic activity coefficients of Ln(
III) and NO
3−, respectively, and
γ±Ln(NO3)3 is the average ionic activity coefficient of Ln(NO
3)
3.
γ±Ln(NO3)3 can be addressed using a simplified Pitzer model in mixed electrolyte solutions,
33–35 and the related parameters and the average activity coefficients have been solved in previous studies.
16
Rearranging eqn (3) in the logarithmic form will give
| |
lg![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) DLn = n × lg[LII](o) + lg DLn = n × lg[LII](o) + lg![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Kγ±Ln(NO3)34[NO−3]3(aq) Kγ±Ln(NO3)34[NO−3]3(aq)
| (4) |
According to eqn (4), the number of extractant molecules in the complexes, ‘n’, could be obtained by the slope analysis method, as shown in Fig. 4. It can be seen that the slopes of La, Ce, and Pr are all around 2, indicating that the stoichiometric ratios of the extracted species are 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2. As for other lanthanides, the slopes are around 3; it is demonstrated that 1
2. As for other lanthanides, the slopes are around 3; it is demonstrated that 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3 of M:LII complexes exist in the organic phase. In the study of ligand LI, 2–3 stoichiometry for different lanthanides has also been obtained.16
3 of M:LII complexes exist in the organic phase. In the study of ligand LI, 2–3 stoichiometry for different lanthanides has also been obtained.16
Thermodynamics of extraction. The thermodynamic parameters for the extraction of Ln(III) by LII can be obtained by eqn (5) and Van't Hoff equation eqn (6):| |
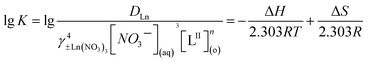 | (5) |
The values of ΔH and ΔS could be obtained by the values of the slope and intercept of the linear fitting equation of 1/T vs. log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) K. As shown in Fig. 5, the lower temperature corresponds to a larger equilibrium constant of the extraction reaction, indicating that the coordination of LII with lanthanides is an exothermic process, which is consistent with the previous studies.6
K. As shown in Fig. 5, the lower temperature corresponds to a larger equilibrium constant of the extraction reaction, indicating that the coordination of LII with lanthanides is an exothermic process, which is consistent with the previous studies.6
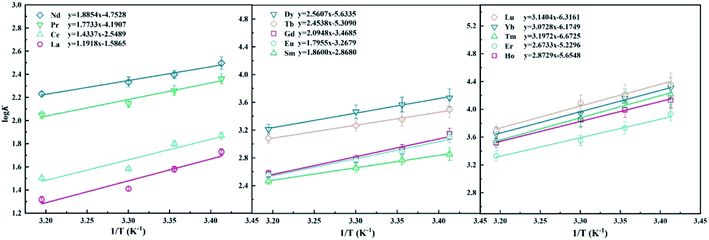 |
| | Fig. 5 Dependency of log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) K on extractant concentration in 40/60 (v/v)% n-octanol/kerosene (O K on extractant concentration in 40/60 (v/v)% n-octanol/kerosene (O![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1 A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M. 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M. | |
The thermodynamic data of the coordination reaction of different lanthanides with LI and LII are shown in Table 2. The negative values of ΔG indicate that the extraction reactions were spontaneous. As the atomic number increases, the ΔG values show a decreasing trend, suggesting that heavy lanthanides are more preferably extracted. Additionally, for the two metal ions of La and Ce, the ΔG value of LI is larger than that of LII, and for the ΔG values of Pr–Lu, LI is less than LII. Therefore, LI exhibits better extraction performance for heavy lanthanides.
Table 2 The calculated thermodynamic data of extraction reactions of Ln(III) ([L] = 0.024 M, [HNO3] = 3 M, O![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1
A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1)
1)
| Ln(III) |
LII |
LI |
| ΔH (kJ mol−1) |
ΔS (J mol−1 K−1−1) |
ΔG (kJ mol−1) |
ΔG (kJ mol−1) |
| La |
−22.82 ± 0.31 |
−30.38 ± 2.21 |
−13.77 ± 0.50 |
−10.28 ± 0.22 |
| Ce |
−27.45 ± 0.34 |
−48.80 ± 3.29 |
−12.91 ± 0.41 |
−10.58 ± 0.34 |
| Pr |
−33.95 ± 0.67 |
−80.24 ± 4.78 |
−10.04 ± 0.38 |
−12.90 ± 0.41 |
| Nd |
−36.10 ± 0.87 |
−64.55 ± 4.92 |
−15.46 ± 0.34 |
−17.31 ± 0.55 |
| Sm |
−35.61 ± 1.27 |
−54.91 ± 3.27 |
−19.25 ± 1.01 |
−28.45 ± 0.67 |
| Eu |
−34.38 ± 1.01 |
−62.57 ± 4.66 |
−15.73 ± 0.44 |
−30.09 ± 0.62 |
| Gd |
−40.11 ± 2.11 |
−66.41 ± 4.56 |
−20.32 ± 1.54 |
−29.13 ± 1.05 |
| Tb |
−46.98 ± 1.88 |
−101.65 ± 5.20 |
−16.69 ± 1.45 |
−33.21 ± 1.87 |
| Dy |
−49.03 ± 2.13 |
−107.87 ± 6.00 |
−16.89 ± 2.00 |
−34.00 ± 1.82 |
| Ho |
−55.01 ± 2.71 |
−108.27 ± 6.87 |
−22.74 ± 1.99 |
−35.34 ± 2.00 |
| Er |
−51.19 ± 2.81 |
−100.13 ± 5.94 |
−21.35 ± 1.84 |
−36.26 ± 1.88 |
| Tm |
−61.22 ± 2.09 |
−127.76 ± 4.88 |
−23.14 ± 1.52 |
−37.04 ± 2.03 |
| Yb |
−58.84 ± 2.01 |
−118.23 ± 5.98 |
−23.60 ± 1.59 |
−36.62 ± 2.05 |
| Lu |
−60.13 ± 3.11 |
−120.94 ± 5.78 |
−24.09 ± 2.01 |
−37.18 ± 2.11 |
| Ref. |
Present work |
16 |
Striping behavior of Ln(III) by nitric acid. The stripping efficiencies of La(III), Nd(III), Eu(III), Dy(III), and Tm(III) by deionized water and various concentrations of HNO3 are shown in Fig. S2.† The results show that, similar to the extraction system with LI as the extractant, 0.001 M HNO3 also exhibits the best stripping efficiency for the LII extraction system loaded with metal ions. The stripping efficiencies of different lanthanides with 0.001 M HNO3 are shown in Fig. S3,† and generally heavy lanthanides are more difficult to strip, which is consistent with the extraction results.Besides, the stripping efficiencies of all lanthanides can reach more than 70% after a single stripping. This case is different from the LI ligand, and the single stripping efficiency of LI for heavy lanthanides can only reach 35%.16 It implies that the interaction between LI ligands and heavy lanthanides is stronger. The effect of the times of stripping on the stripping efficiencies of La(III), Eu(III), Dy(III), and Tm(III) is shown in Fig. 6. All stripping efficiencies can reach 90% after three times of stripping.
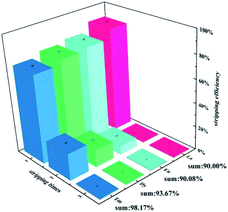 |
| | Fig. 6 Effect of the times of stripping on the stripping efficiency of La(III), Eu(III), Dy(III), and Tm(III) by nitric acid, O![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1 A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, [HNO3] = 0.001 M. 1, [HNO3] = 0.001 M. | |
Infrared spectroscopy studies. The FT-IR spectra of the ligand LII and the corresponding complexes are shown in Fig. 7. For the pure LII ligand, the absorption peaks at 2800 cm−1 to 3000 cm−1 correspond to the –CH3 asymmetric stretching vibration and symmetric stretching vibration. The peak at 1651 cm−1 is the symmetric stretching vibration of the amide carbonyl C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O, which also exists in symmetric DGAs, such as TODGA or TEHDGA, and LI type asymmetric DGAs. Different from LI, there is a new absorption band around the frequency of 1739 cm−1 (1700–1780 cm−1) in the IR spectra of LII, which seems to be also the symmetric stretching vibration of C
O, which also exists in symmetric DGAs, such as TODGA or TEHDGA, and LI type asymmetric DGAs. Different from LI, there is a new absorption band around the frequency of 1739 cm−1 (1700–1780 cm−1) in the IR spectra of LII, which seems to be also the symmetric stretching vibration of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O. In a DGA molecule, the conjugation effect and induction effect exist in the amide group at the same time, and the strong conjugation effect of N weakens the amide C
O. In a DGA molecule, the conjugation effect and induction effect exist in the amide group at the same time, and the strong conjugation effect of N weakens the amide C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O double bond. For the LII ligand, the kind of the alkyl substituents connected to the two amide groups is different, and the weakening degree of the two C
O double bond. For the LII ligand, the kind of the alkyl substituents connected to the two amide groups is different, and the weakening degree of the two C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O bonds is different due to the difference in the induction effect of the two alkyl groups. Therefore, there exists a clear split in the absorption band of C
O bonds is different due to the difference in the induction effect of the two alkyl groups. Therefore, there exists a clear split in the absorption band of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O stretching vibration. The bands with higher frequency and lower frequency correspond to the asymmetric and symmetric coupling vibrations of C
O stretching vibration. The bands with higher frequency and lower frequency correspond to the asymmetric and symmetric coupling vibrations of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O, respectively. The low frequency band is wider and stronger than the high frequency. After coordination with lanthanides, the peaks at 1739 cm−1 and 1651 cm−1 are both blue shifted, which indicates that both bands correspond to C
O, respectively. The low frequency band is wider and stronger than the high frequency. After coordination with lanthanides, the peaks at 1739 cm−1 and 1651 cm−1 are both blue shifted, which indicates that both bands correspond to C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O stretching vibration.
O stretching vibration.
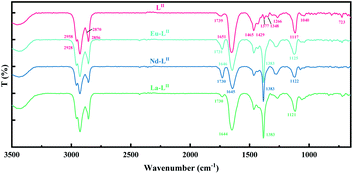 |
| | Fig. 7 The FT-IR of the ligand and the complexes. | |
In addition, 1465 cm−1 and 1429 cm−1 are C–N stretching vibration, which belongs to amide III bands; 1377 cm−1 and 1348 cm−1 may belong to variable-angle vibrations of H–C–H; 1266 cm−1 belongs to amide II bands or amide III bands. The asymmetric and symmetric stretching vibrations of the ether bond coincide, so the obvious peak can be only observed at 1117 cm−1; the amide V bands or amide VII bands at 723 cm−1 and 1040 cm−1 correspond to the O![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) C–N in-plane bending vibration or out-of-plane bending vibration.
C–N in-plane bending vibration or out-of-plane bending vibration.
In the infrared spectrum of the organic phase loaded with metal ions, the absorption peaks of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O have a different degree of blue shift compared to the pure extractant, from 1739 cm−1/1649 cm−1 of pure LII to 1730 cm−1/1644 cm−1 (La), 1730 cm−1/1645 cm−1 (Nd), and 1731 cm−1/1647 cm−1 (Eu), respectively. The symmetric stretching absorption peaks of the ether O bond shift from 1117 cm−1 of pure LII to 1121 cm−1 (La), 1122 cm−1 (Nd) and 1125 cm−1 (Eu), respectively.36 It indicates that the LII molecule can form tridentate complexes with La(III), Nd(III), and Eu(III) through two carbonyl groups and one ether group. The studies by Kou et al. illustrated the structure of the complex between LI and Eu(III),16,37,38 and the results showed that Eu(III), in the center of the complex, is surrounded by three LI molecules with tridentate coordination, and the LII complex also has a similar structure.
O have a different degree of blue shift compared to the pure extractant, from 1739 cm−1/1649 cm−1 of pure LII to 1730 cm−1/1644 cm−1 (La), 1730 cm−1/1645 cm−1 (Nd), and 1731 cm−1/1647 cm−1 (Eu), respectively. The symmetric stretching absorption peaks of the ether O bond shift from 1117 cm−1 of pure LII to 1121 cm−1 (La), 1122 cm−1 (Nd) and 1125 cm−1 (Eu), respectively.36 It indicates that the LII molecule can form tridentate complexes with La(III), Nd(III), and Eu(III) through two carbonyl groups and one ether group. The studies by Kou et al. illustrated the structure of the complex between LI and Eu(III),16,37,38 and the results showed that Eu(III), in the center of the complex, is surrounded by three LI molecules with tridentate coordination, and the LII complex also has a similar structure.
Theoretical calculation
A series of scalar-relativistic density functional theory calculations were carried out to explain the difference of complexing abilities between LI and LII. Firstly, the properties of the ligands were analyzed according to HSAB (Hard Soft Acid Base) theory. The hardness (η) is one of the parameters to judge the complexing ability between ligands and metal ions. η can be determined by eqn (7):where IP and EA represent the vertical ionization potential and vertical electron affinity of the neutral ligands, respectively. The η value of LI and LII is 3.805 and 3.784, respectively, indicating that the alkalinity of LI is harder. As the atomic number of the lanthanides increases, the positive charge density increases, and the acid hardness of the lanthanide metal ions gradually increases. Therefore, the ligand LI has better extraction performance for heavy lanthanides according to HSAB theory.
Optimized structures of the M–L complexes. The complex structures were optimized at the B3LYP/6-311G(d)/RECP level of theory using Gaussian 16. Since nitrates are in the second coordination layer, they are ignored in the optimization of the complex structures, and the dominant species are [M × nL]3+ (M = La–Pr, n = 2; M = Nd–Lu, n = 3). Fig. 8 shows the optimized structures of the complexes of La and Eu with LI and LII, and the structures of the other complexes such as Sm, Ho, and Lu are shown in Fig. S4.† Both LI and LII are coordinated with lanthanide ions as a tridentate ligand, which is in agreement with the previous studies.36,39
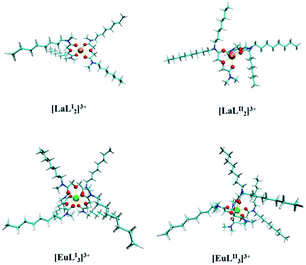 |
| | Fig. 8 The optimized complex structure of M–LI/LII (M = La, Eu); orange-La, green-Eu, cyan-carbon, white-hydrogen, red-oxygen, and blue-nitrogen. | |
The bond lengths of M–Oether, M–Oamide1, and M–Oamide2 and the average bond lengths of all bonds are listed in Table 3. The results show that generally the M–Oether bond lengths are longer than the M–Oamide1 and M–Oamide2 bond lengths for the same metal ion, and the bond lengths of Ln–Oether, Ln–Oamide1 and M–Oamide2 decrease with the increase of the atomic number of lanthanides. Except that the average bond length of La–LII is slightly less than that of La–LI, the average bond lengths of other M–LII (M = Sm, Eu, and Ho) complexes are slightly less than those of M–LI complexes. There is an ‘imbalance’ in the bond length between metal ions and the amide O atoms in the LII ligand, and the difference of the bond lengths between M–Oamide1 and M–Oamide2 in M–LII is larger than in M–LI. Moreover, the difference of the electrostatic potential of Oamide1 and Oamide2 in LII is also larger than that in LI.36 So there exists a larger difference in the ability of electron-donation of the two amide O atoms in LII than in LI. The calculation results of Mayer bond orders (MBOs) of M–Oether, M–Oamide1 and M–Oamide2 (M = La, Sm, Eu, Ho, and Lu) are listed in Table S1,† in which the ‘imbalance’ phenomenon of LII also exists. MBOs of M–Oamide1 and M–Oamide2 in LI are almost the same, but MBOs of M–Oamide1 and M–Oamide2 in LII are different. All MBO values are within the range of 0.10–0.34, indicating that the interactions between the ligands and the metal ions display weak covalency.40,41
Table 3 Average M–Oether, M–Oamide1 and M–Oamide2 bond lengths of M–L complexes (M = La, Sm, Eu, Ho, and Lu) at the B3LYP/6-311G(d)/RECP level of theory
| Elements |
Bond |
LI |
LII |
| La |
M–Oether |
2.724 |
2.5794 |
2.656 |
2.5159 |
| M–Oamide1 |
2.507 |
2.457 |
| M–Oamide2 |
2.507 |
2.435 |
| Sm |
M–Oether |
2.620 |
2.4854 |
2.622 |
2.4859 |
| M–Oamide1 |
2.418 |
2.430 |
| M–Oamide2 |
2.418 |
2.406 |
| Eu |
M–Oether |
2.600 |
2.4699 |
2.601 |
2.4701 |
| M–Oamide1 |
2.405 |
2.395 |
| M–Oamide2 |
2.405 |
2.414 |
| Ho |
M–Oether |
2.558 |
2.4230 |
2.561 |
2.4235 |
| M–Oamide1 |
2.356 |
2.343 |
| M–Oamide2 |
2.355 |
2.366 |
| Lu |
M–Oether |
2.509 |
2.3816 |
2.513 |
|
| M–Oamide1 |
2.318 |
2.309 |
2.3823 |
| M–Oamide2 |
2.318 |
2.325 |
Topology analysis. The average electron density ρ(r) and Laplacian ∇2ρ(r) at the M–Oether, M–Oamide1 and M–Oamide2 bond critical point (BCP) of five complexes are listed in Table 4. According to the QTAIM theory, ρ(r) > 0.2 a.u. and ∇2ρ(r) < 0 at the BCPs represent a covalent interaction, and an ionic interaction corresponds to ρ(r) < 0.1 a.u. and ∇2ρ(r) > 0 at the BCPs. The ρ(r) values at M–Oamide1, M–Oamide2 and M–Oether BCPs are <0.1 a.u. and all ∇2ρ(r) values are positive. Therefore, all of these bonds are ionic bonds. Combining electrostatic potential analysis, bond length, bond order and electron density, it is found that coordination ability of the ether oxygen bond in the two ligands is weaker than that of the two amide O atoms, and the coordination ability of the amide O atoms in the LII ligand is weaker than that of LI, which is reflected in the difference of their extraction performances.
Table 4 The electron density ρ(r) and Laplacian ∇2ρ(r) at the M–Oether and M–Oamide (M = La, Sm, Eu, Ho, and Lu) bond critical point (BCP) at the B3LYB/6-311G(d)/RECP level of theory
| Bond |
LI |
LII |
| Average ρ(r) |
Average ∇2ρ(r) |
Average ρ(r) |
Average ∇2ρ(r) |
| La–Oamide1 |
0.0452 |
0.1701 |
0.0465 |
0.1769 |
| La–Oamide2 |
0.0452 |
0.172 |
0.0438 |
0.1677 |
| La–Oether |
0.0266 |
0.1083 |
0.0269 |
0.124 |
| Sm–Oamide1 |
0.0488 |
0.2024 |
0.0474 |
0.1973 |
| Sm–Oamide2 |
0.0488 |
0.2021 |
0.0503 |
0.2078 |
| Sm–Oether |
0.0292 |
0.1309 |
0.0291 |
0.1303 |
| Eu–Oamide1 |
0.0491 |
0.2066 |
0.0511 |
0.2142 |
| Eu–Oamide2 |
0.0489 |
0.2064 |
0.047 |
0.1992 |
| Eu–Oether |
0.0292 |
0.1327 |
0.0291 |
0.1324 |
| Ho–Oamide1 |
0.0509 |
0.2256 |
0.0529 |
0.2403 |
| Ho–Oamide2 |
0.0509 |
0.2256 |
0.0494 |
0.2231 |
| Ho–Oether |
0.0312 |
0.1458 |
0.0304 |
0.1468 |
| Lu–Oamide1 |
0.0515 |
0.2359 |
0.0528 |
0.2417 |
| Lu–Oamide2 |
0.0515 |
0.2359 |
0.0504 |
0.2316 |
| Lu–Oether |
0.0312 |
0.1492 |
0.0301 |
0.1477 |
Molecular orbital (MO) analysis. In order to further learn the bonding nature of the complexes of two ligands and Ln(III), MO analysis was carried out. The α-spin valence MO diagrams of (MLI)3+ and (MLII)3+ (M = La, Sm, Eu, Ho, and Lu) were obtained to evaluate the involvement extent of various molecular orbitals for coordination. Fig. 9, 10, S5, S6, and S7† show the α-spin valence MO diagrams of the [LaL2]3+, [EuL3]3+, [SmL3]3+, [HoL3]3+, and [LuL3]3+, respectively, and the contribution of specific atoms to several representative molecular orbitals. d/f/O represent the 5d orbitals and 4f orbitals of lanthanides, and the 2p orbitals of Oamide1, Oamide2 and Oether, respectively. It can be seen that the lanthanide ions have orbital interactions with amide O atoms compared to ether O atoms, which demonstrates that the amide O atoms have the stronger complex ability. The composition of MO # 230, MO # 238 in the (LaL2I)3+ complex and MO # 230, MO # 235 in the (LaL2II)3+ complex, MO # 341 in the (EuL3I)3+, and 341 in the (EuL3II)3+ complex is mainly composed of the 4f orbital. In Fig. 10, the 4f orbital component in the molecular orbital of the complex formed by LII and La has the largest component 4f orbitals (91.56%, MO # 235, (LaL2II)3+), which is larger than the complex formed by LI and La (83.05%, MO # 233, (LaL2I)3+). However, for the Sm, Eu, Ho, and Lu the corresponding LI complexes possess MOs with the largest component 4f orbital. Similar results can be observed in the analysis of the component of 2p orbital in O atoms. Therefore, it seems that there exist less electron sharing and weaker interactions between heavy lanthanides and LII,42 which is probably caused by different electron donating capabilities of the two amide O atoms in the LII ligand.
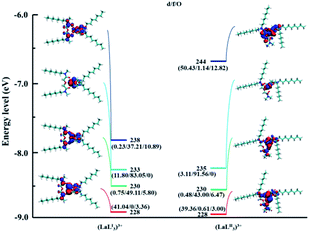 |
| | Fig. 9 The energy levels (eV) of the α-spin valence MOs and the corresponding diagrams in the [LaL2]3+ complexes (isosurface value: 0.02 a.u.). | |
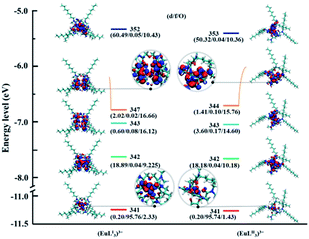 |
| | Fig. 10 The energy levels (eV) of the α-spin valence MOs and the corresponding diagrams in the [EuL3]3+ complexes (isosurface value: 0.02 a.u.). | |
Conclusions
A comparative study of the extraction behavior towards several lanthanides by N,N′-dimethyl-N,N′-dioctyl diglycolamide (LI) and N,N-dimethyl-N′,N′-dioctyl diglycolamide (LII) in 40/60 (v/v)% n-octanol/kerosene was carried out. Extraction thermodynamic results suggest that all extraction reactions are exothermic and both ligands exhibit positive sequential extraction of lanthanides, and LII has poorer extraction performance for these lanthanides except for La and Ce than LI. Analysis by the slope method and FT-IR analysis show that LII forms 1:2 complexes (La, Ce, and Pr) and 1:3 complexes (Nd–Lu) by two amide O atoms and one ether O atom, which is similar to LI.
To further elucidate the reason for the differences in the extraction performances of Ln(III), the DFT method was used to explore the structure of the complexes and the interaction between Ln(III) with amide and ether O atoms in LI and LII. The bond length, MBO and MO analysis demonstrate that except for La, the interaction between these lanthanides and LII is weaker than that for LI, which is in agreement with the experiment results. In particular, there exists “imbalance” in the bonding lengths and Mayer bond orders of metal ions and the two amide O atoms in LII, which is responsible partially for the poor extraction performances of LII. The study is expected to be helpful for evaluating the coordination ability of two types of isomeric asymmetric diglycolamides and further exploring the structure–performance relationship of diglycolamide extractants.
Author contributions
Yaoyang Liu: investigation, data curation, and writing – original draft. Chuang Zhao: investigation. Zhibin Liu: investigation. Sheng Liu: methodology. Yu Zhou: resource. Caishan Jiao: supervision and funding acquisition. Meng Zhang: supervision. Yang Gao: investigation, writing – review & editing, resources, and funding acquisition. Hui He: supervision, funding acquisition, and conceptualization. Shaowen Zhang: software.
Conflicts of interest
There are no conflicts to declare.
Acknowledgements
The work was supported by Special Fund of Central University Basic Scientific Research Fee (3072019CF1503).
Notes and references
- W. Yao, Y. Wu, H. Pang, X. Wang, S. Yu and X. Wang, Sci. China: Chem., 2018, 61, 812–823 CrossRef CAS.
- V. Manchanda, Sep. Purif. Technol., 2004, 35, 85–103 CrossRef CAS.
- M. Salvatores, Nucl. Eng. Des., 2005, 235, 805–816 CrossRef CAS.
- J. Ravi, T. Prathibha, K. A. Venkatesan, M. P. Antony, T. G. Srinivasan and P. R. Vasudeva Rao, Sep. Purif. Technol., 2012, 85, 96–100 CrossRef CAS.
- Y. Shen, X. Tan, L. Wang and W. Wu, Sep. Purif. Technol., 2011, 78, 298–302 CrossRef CAS.
- Y. Liu, Z. Liu, C. Zhao, Y. Zhou, Y. Gao and H. He, Prog. Chem., 2020, 21, 219–229 Search PubMed.
- J. Ravi, K. A. Venkatesan, M. P. Antony, T. G. Srinivasan and P. R. Vasudeva Rao, Radiochim. Acta, 2014, 102, 609–617 CrossRef CAS.
- P. K. Mohapatra, A. Sengupta, M. Iqbal, J. Huskens and W. Verboom, Chem.–Eur. J., 2013, 19, 3230–3238 CrossRef CAS PubMed.
- Y. Liu, Y. Gao, Z. Wei, Y. Zhou, M. Zhang, H. Hou, G. Tian and H. He, J. Radioanal. Nucl. Chem., 2018, 318, 2087–2096 CrossRef CAS.
- H. Cui, X. Feng, J. Shi, W. Liu, N. Yan, G. Rao and W. Wang, Sep. Purif. Technol., 2020, 234, 116096 CrossRef CAS.
- X. Peng, Y. Li, J. Ma, Y. Cui and G. Sun, J. Radioanal. Nucl. Chem., 2017, 313, 327–332 CrossRef CAS.
- K. R. Swami, K. A. Venkatesan and M. P. Antony, Solvent Extr. Ion Exch., 2019, 37, 500–517 CrossRef CAS.
- K. R. Swami and K. A. Venkatesan, J. Mol. Liq., 2019, 296, 111741 CrossRef CAS.
- P. R. Schreiner, L. V. Chernish, P. A. Gunchenko, E. Y. Tikhonchuk, H. Hausmann, M. Serafin, S. Schlecht, J. E. Dahl, R. M. Carlson and A. A. Fokin, Nature, 2011, 477, 308–311 CrossRef CAS PubMed.
- D. Stamberga, M. R. Healy, V. S. Bryantsev, C. Albisser, Y. Karslyan, B. Reinhart, A. Paulenova, M. Foster, I. Popovs, K. Lyon, B. A. Moyer and S. Jansone-Popova, Inorg. Chem., 2020, 59, 17620–17630 CrossRef CAS PubMed.
- Y. Liu, C. Zhao, Z. Liu, Y. Zhou, C. Jiao, M. Zhang, H. Hou, Y. Gao, H. He and G. Tian, J. Radioanal. Nucl. Chem., 2020, 325, 409–416 CrossRef CAS.
- Y. Sasaki and G. R. Choppin, J. Radioanal. Nucl. Chem., 1996, 12, 225–230 CAS.
- J. Ravi, K. A. Venkatesan, M. P. Antony, T. G. Srinivasan and P. R. Vasudeva Rao, J. Radioanal. Nucl. Chem., 2012, 295, 1283–1292 CrossRef.
- S. Guoxin, L. Min, C. Yu, Y. Meilong and Y. Shaohong, Solvent Extr. Ion Exch., 2010, 28, 482–494 CrossRef.
- M. Frisch, G. Trucks, H. Schlegel, G. Scuseria, M. Robb, J. Cheeseman, G. Scalmani, V. Barone, G. Petersson and H. Nakatsuji, Gaussian 16 (Revision B.01), Gaussian Inc.,Pittsburgh, PA, 2016 Search PubMed.
- R. Nityananda, P. Hohenberg and W. J. R. Kohn, Resonance, 2017, 22, 809–811 CrossRef.
- J. Perdew., Phys. Rev. B: Condens. Matter Mater. Phys., 1986, 33, 8822–8824 CrossRef PubMed.
- B. D. Axel, Phys. Rev. B: Condens. Matter Mater. Phys., 1998, 98, 5648–5652 Search PubMed.
- C. Lee, W. Yang and R. G. Parr, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785 CrossRef CAS PubMed.
- M. Dolg, H. Stoll and H. J. Preuss, J. Chem. Phys., 1989, 90, 1730–1734 CrossRef CAS.
- W. Küchle, M. Dolg, H. Stoll and H. Preuss, J. Chem. Phys., 1994, 100, 7535–7542 CrossRef.
- X. Cao and M. Dolg, J. Mol. Struct., 2002, 581, 139–147 CrossRef CAS.
- T. Lu and F. J. Chen, J. Comput. Chem., 2012, 33, 580–592 CrossRef CAS PubMed.
- T. Lu and S. Manzetti, J. Struct. Chem., 2014, 25, 1521–1533 CrossRef CAS.
- F. McLachlan, K. Greenough, A. Geist, B. McLuckie, G. Modolo, A. Wilden and R. Taylor, Solvent Extr. Ion Exch., 2016, 34, 334–346 CrossRef CAS.
- E. A. Mowafy and H. F. Aly, Solvent Extr. Ion Exch., 2007, 25, 205–224 CrossRef CAS.
- A. N. Turanov and V. K. Karandashev, Solvent Extr. Ion Exch., 2018, 36, 257–271 CrossRef CAS.
- K. Pitzer, J. Phys. Chem., 1973, 77, 268–277 CrossRef CAS.
- K. S. Pitzer and G. Mayorga, in Molecular Structure And Statistical Thermodynamics: Selected Papers of Kenneth S Pitzer, World Scientific, 1993, pp. 396–404 Search PubMed.
- K. S. Pitzer, Activity Coefficients in Electrolyte Solutions, CRC press, 2018 Search PubMed.
- Y. Liu, S. Liu, Z. Liu, C. Zhao, C. Li, Y. Zhou, C. Jiao, Y. Gao, H. He and S. Zhang, RSC Adv., 2021, 11, 27969–27977 RSC.
- F. Kou, S. Yang, H. Qidan, L. Zhang, C. M. Beavers, S. J. Teat and G. Tian, Dalton Trans., 2016, 45, 18484–18493 RSC.
- F. Kou, S. Yang, L. Zhang, S. J. Teat and G. Tian, Inorg. Chem. Commun., 2016, 71, 41–44 CrossRef CAS.
- E. A. Mowafy and D. Mohamed, Sep. Purif. Technol., 2014, 128, 18–24 CrossRef CAS.
- I. Mayer, J. Quantum Chem., 1986, 29, 477 CrossRef CAS.
- X.-W. Chi, Q.-Y. Wu, Q. Hao, J.-H. Lan, C.-Z. Wang, Q. Zhang, Z.-F. Chai and W.-Q. Shi, Organometallics, 2018, 37, 3678–3686 CrossRef CAS.
- P. W. Huang, C. Z. Wang, Q. Y. Wu, J. H. Lan, G. Song, Z. F. Chai and W. Q. Shi, Phys. Chem. Chem. Phys., 2018, 20, 1030–1038 RSC.
Footnote |
| † Electronic supplementary information (ESI) available: Partial extraction and stripping experiment results; molecular orbital of [SmL3II]3+, [HoL3II]3+, [LuL3II]3+ analysis and Mayer bond orders analysis results. See DOI: 10.1039/d1ra07020g |
|
| This journal is © The Royal Society of Chemistry 2022 |
Click here to see how this site uses Cookies. View our privacy policy here.  Open Access Article
Open Access Article *a,
Hui He*ab and
Shaowen Zhang
*a,
Hui He*ab and
Shaowen Zhang c
c

![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 volume ratio with a constant-temperature shaker for 30 min. It is demonstrated that 30 min is enough for the two phases to reach equilibrium. Then the two phases were separated by centrifugation at 2500 rpm for 10 min. An aliquot of the aqueous raffinate was taken into an individual tube containing 0.1 M HNO3 and the metal ion concentration was assayed by ICP-MS (X-II ICP-MS, Thermo Fisher Scientific, Waltham, MA, USA). The distribution ratio, DLn, was derived from
1 volume ratio with a constant-temperature shaker for 30 min. It is demonstrated that 30 min is enough for the two phases to reach equilibrium. Then the two phases were separated by centrifugation at 2500 rpm for 10 min. An aliquot of the aqueous raffinate was taken into an individual tube containing 0.1 M HNO3 and the metal ion concentration was assayed by ICP-MS (X-II ICP-MS, Thermo Fisher Scientific, Waltham, MA, USA). The distribution ratio, DLn, was derived from
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1
A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, [Ln3+]init = 3 mM).
1, [Ln3+]init = 3 mM).
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1
A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M.
1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M.
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) DLn = n × lg[LII](o) + lg
DLn = n × lg[LII](o) + lg![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Kγ±Ln(NO3)34[NO−3]3(aq)
Kγ±Ln(NO3)34[NO−3]3(aq)
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2. As for other lanthanides, the slopes are around 3; it is demonstrated that 1
2. As for other lanthanides, the slopes are around 3; it is demonstrated that 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3 of M:LII complexes exist in the organic phase. In the study of ligand LI, 2–3 stoichiometry for different lanthanides has also been obtained.16
3 of M:LII complexes exist in the organic phase. In the study of ligand LI, 2–3 stoichiometry for different lanthanides has also been obtained.16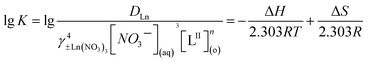
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) K. As shown in Fig. 5, the lower temperature corresponds to a larger equilibrium constant of the extraction reaction, indicating that the coordination of LII with lanthanides is an exothermic process, which is consistent with the previous studies.6
K. As shown in Fig. 5, the lower temperature corresponds to a larger equilibrium constant of the extraction reaction, indicating that the coordination of LII with lanthanides is an exothermic process, which is consistent with the previous studies.6
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) K on extractant concentration in 40/60 (v/v)% n-octanol/kerosene (O
K on extractant concentration in 40/60 (v/v)% n-octanol/kerosene (O![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1
A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M.
1), [Ln3+]init = 3 mM, [LII] = 0.012–0.12 M.![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) A = 1
A = 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1)
1)
![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O, which also exists in symmetric DGAs, such as TODGA or TEHDGA, and LI type asymmetric DGAs. Different from LI, there is a new absorption band around the frequency of 1739 cm−1 (1700–1780 cm−1) in the IR spectra of LII, which seems to be also the symmetric stretching vibration of C
O, which also exists in symmetric DGAs, such as TODGA or TEHDGA, and LI type asymmetric DGAs. Different from LI, there is a new absorption band around the frequency of 1739 cm−1 (1700–1780 cm−1) in the IR spectra of LII, which seems to be also the symmetric stretching vibration of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O. In a DGA molecule, the conjugation effect and induction effect exist in the amide group at the same time, and the strong conjugation effect of N weakens the amide C
O. In a DGA molecule, the conjugation effect and induction effect exist in the amide group at the same time, and the strong conjugation effect of N weakens the amide C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O double bond. For the LII ligand, the kind of the alkyl substituents connected to the two amide groups is different, and the weakening degree of the two C
O double bond. For the LII ligand, the kind of the alkyl substituents connected to the two amide groups is different, and the weakening degree of the two C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O bonds is different due to the difference in the induction effect of the two alkyl groups. Therefore, there exists a clear split in the absorption band of C
O bonds is different due to the difference in the induction effect of the two alkyl groups. Therefore, there exists a clear split in the absorption band of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O stretching vibration. The bands with higher frequency and lower frequency correspond to the asymmetric and symmetric coupling vibrations of C
O stretching vibration. The bands with higher frequency and lower frequency correspond to the asymmetric and symmetric coupling vibrations of C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O, respectively. The low frequency band is wider and stronger than the high frequency. After coordination with lanthanides, the peaks at 1739 cm−1 and 1651 cm−1 are both blue shifted, which indicates that both bands correspond to C
O, respectively. The low frequency band is wider and stronger than the high frequency. After coordination with lanthanides, the peaks at 1739 cm−1 and 1651 cm−1 are both blue shifted, which indicates that both bands correspond to C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O stretching vibration.
O stretching vibration.
![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) C–N in-plane bending vibration or out-of-plane bending vibration.
C–N in-plane bending vibration or out-of-plane bending vibration.![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O have a different degree of blue shift compared to the pure extractant, from 1739 cm−1/1649 cm−1 of pure LII to 1730 cm−1/1644 cm−1 (La), 1730 cm−1/1645 cm−1 (Nd), and 1731 cm−1/1647 cm−1 (Eu), respectively. The symmetric stretching absorption peaks of the ether O bond shift from 1117 cm−1 of pure LII to 1121 cm−1 (La), 1122 cm−1 (Nd) and 1125 cm−1 (Eu), respectively.36 It indicates that the LII molecule can form tridentate complexes with La(III), Nd(III), and Eu(III) through two carbonyl groups and one ether group. The studies by Kou et al. illustrated the structure of the complex between LI and Eu(III),16,37,38 and the results showed that Eu(III), in the center of the complex, is surrounded by three LI molecules with tridentate coordination, and the LII complex also has a similar structure.
O have a different degree of blue shift compared to the pure extractant, from 1739 cm−1/1649 cm−1 of pure LII to 1730 cm−1/1644 cm−1 (La), 1730 cm−1/1645 cm−1 (Nd), and 1731 cm−1/1647 cm−1 (Eu), respectively. The symmetric stretching absorption peaks of the ether O bond shift from 1117 cm−1 of pure LII to 1121 cm−1 (La), 1122 cm−1 (Nd) and 1125 cm−1 (Eu), respectively.36 It indicates that the LII molecule can form tridentate complexes with La(III), Nd(III), and Eu(III) through two carbonyl groups and one ether group. The studies by Kou et al. illustrated the structure of the complex between LI and Eu(III),16,37,38 and the results showed that Eu(III), in the center of the complex, is surrounded by three LI molecules with tridentate coordination, and the LII complex also has a similar structure.





