Network topologies dictate electromechanical coupling in liquid metal–elastomer composites
Abstract
Elastomers embedded with micro- and nanoscale droplets of liquid metal (LM) alloys like eutectic gallium–indium (EGaIn) can exhibit unique combinations of elastic, thermal, and electrical properties that are difficult to achieve using rigid filler. For composites with sufficient concentrations of liquid metal, the LM droplets can form percolating networks that conduct electricity and deform with the surrounding elastomer as the composite is stretched. Surprisingly, experimental measurements performed on LM-embedded elastomers (LMEEs) show that the total electrical resistance of the composite increases only slightly even as the elastomer is stretched to several times its natural length. In contrast, Pouillet's law would predict an exponential increase in resistance (Ω) with stretch (λ) due to the incompressibility of liquid metal and elastomer. In this manuscript, we perform a computational analysis to examine the unique electromechanical properties of conductive LMEE composites. Our analysis suggests that the gauge factor that quantifies electromechanical coupling (i.e. 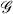 = {ΔΩ/Ω0}/λ) decreases with increasing tortuosity of the conductive pathways formed by the connected LM droplets. A dimensionless parameter for path tortuosity can be used to estimate
= {ΔΩ/Ω0}/λ) decreases with increasing tortuosity of the conductive pathways formed by the connected LM droplets. A dimensionless parameter for path tortuosity can be used to estimate 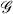 for statistically homogeneous LMEE composites. These results rationalize experimental observations and provide insight into the influence of liquid metal droplet assembly on the functionality of the composite.
for statistically homogeneous LMEE composites. These results rationalize experimental observations and provide insight into the influence of liquid metal droplet assembly on the functionality of the composite.

- This article is part of the themed collection: Soft Matter Most Popular 2020


 Please wait while we load your content...
Please wait while we load your content...
