Structural evolution and electronic properties of boron sulfides (B₂S₃)_n (n = 1–6): insights from DFT calculations

Abstract

The low-energy isomers of boron sulfides (B₂S₃)_n (n = 1–6) were constructed by using B₂S₃ as the building block, and their structure, stability, and reactivity toward small molecules have been explored by density functional theory (DFT) calculations. It is found that the low-energy isomers of (B₂S₃)_n clusters are of rich bonding characteristics for their structural constituents, such as [S−B−S], [B₂S₃], [B₃S₃], [S], etc. The planar or non-planar B₂S₂ rings, as the basic structural units, are bridged together by the S atoms to form the most stable structures of (B₂S₃)_n clusters with n ≥ 2. These low-energy (B₂S₃)_n clusters are predicted to be stable both structurally and electronically, and their boron centers show relatively high activity to the binding of small molecules NH₃ and CO, until all boron atoms are ligated by NH₃ or CO. The present results provide new insights into the structure and properties of (B₂S₃)_n clusters, which are beneficial to the development of boron chemistry and the application of boron-based materials.

---

1. Introduction

Boron clusters and boron-containing compounds have attracted considerable attention due to their intriguing physical and chemical properties,^1–3 and numerous studies of theory and experiments have been conducted to explore the structural and bonding properties, spectroscopic characteristics, and chemical reactivity of boron clusters,^4–16 borophenes,^17–23 boron oxide clusters,^24–36 and so on. In particular, boron sulfide clusters and materials are of great interest because of their diverse structural features and bonding modes.^37–43

In the 1960s and 1970s, White and Gilles et al. confirmed the existence of boron sulfides, including BS₂⁺,⁴⁴ B₂S₂⁺,^45,46 B₂S₃⁺,^47,48 and so on, making a significant advancement in boosting the development of boron sulfide chemistry. The existing boron sulfide clusters can be categorized into three main types: proportional, sulfur-rich, and boron-rich boron sulfide clusters. In 2015, Li and co-workers presented a set of perfectly planar dicyclic B₆S₆^0/−/2− clusters featuring a twin B₃S₂ five-membered ring as the core. It was found that the closed-shell B₆S₆²⁻ exhibits a delocalized characteristic of 10π-electrons, closely analogous to the bonding pattern of the aromatic naphthalene.⁴⁹ Subsequently, Zheng and co-workers investigated the structural, electronic, and bonding properties of BS^0/− and BS₃^0/− by combining experimental and theoretical methods.⁵⁰ The results reveal that the ground state of BS₃⁻ has a planar structure with C_2v symmetry, whereas BS₃ presents an S−B−S−S bending structure. Recently, Chen and co-workers explored the structural evolution of B_nS₂^0/− (n = 2–13) clusters through the DFT calculations combined with particle swarm optimization algorithm software, and the predicted global minima for these clusters suggest a conformational evolution from a linear-chain structure to planar or quasi-planar ones.⁵¹ Obviously, while the above theoretical and experimental studies show that boron sulfide clusters exhibit very rich chemical bonding modes, depending on the B/S atomic ratio and the charged state, our understanding of B₂S₃-type clusters remains insufficient.

Notably, B₂O₃-type clusters were proved to play a crucial role in boron chemistry and oxidative dehydrogenation of propane.^52–57 In 2013, Li and Cheng theoretically studied the structure evolution and electronic properties of (B₂O₃)_n (n = 1–6) clusters, and found that the global minima structures of these clusters are planar up to n = 3, and cages at n = 4–6.⁵⁸ Given the similarity in the valence electron configurations of sulfur and oxygen atoms, (B₂S₃)_n and (B₂O₃)_n may exhibit similar structural and electronic features. This also implies that B₂S₃-type clusters could display analogous chemical properties and stability, making them ideal candidates for organic catalysis and materials science.

Recently, B₂S₃-type two-dimensional (2D) materials were predicted to be promising catalysts for splitting water^59,60 and suppressing the shuttle effect in lithium–sulfur batteries.⁶¹ However, our knowledge of B₂S₃-type clusters in terms of their structural evolution and chemical properties is still very limited. Further investigation into the growth of B₂S₃-type clusters could lead to the development of more stable and efficient materials for these applications.

Under this background, B₂S₃ has been chosen as a fundamental structural unit to search for the stable structures of a series of (B₂S₃)_n (n = 1–6) clusters by using the artificial bee colony algorithm.^62,63 Subsequent DFT calculations were used to determine the structural and electronic properties of the corresponding low-energy structures of (B₂S₃)_n. Additionally, the interaction of selected clusters with small molecules was discussed to explore their potential applications in small molecule activation and capture. The present work aims to further understand the structures, properties, and potential applications of boron sulfide clusters, and to provide a theoretical basis for the development of 2D boron-based materials.

2. Computational details

The low-energy structures of the studied (B₂S₃)_n clusters are predicted by using the artificial bee colony algorithm, implemented in the ABCluster software for the global optimization of chemical clusters.^62,63 The initial configurations, ranging from 1000 to 80 000 depending on the number of atoms in the studied clusters, are auto-generated and pre-optimized by the semiempirical PM6 and GFN2-xTB method with ABCluster.^64–67 Then 80 searched structures with relatively low energy for the studied clusters are selected and fully optimized with the more accurate DFT method at the M06-2X-D3^68,69/6-31G(d,p)^70,71 level of theory, implemented in the Gaussian 16 package.⁷² The final energy ranking of low-energy structures is obtained through a combination of PM6 and GFN2-xTB methods, effectively reducing the risk of missing important structures. All the optimized cluster structures underwent harmonic vibrational analysis at the same level, confirming that all the related species are stable without imaginary frequency. In addition, the composite quantum chemical method G4(MP2)-6X⁷³ is adopted to evaluate the reliability of the M06-2X-D3/6-31G(d,p) level. Mayer bond order,⁷⁴ adaptive natural density partitioning (AdNDP),⁷⁵ and orbital composition analyses are performed by the Multiwfn program.⁷⁶ Geometric structures and molecular orbitals were visualized by using the CYLview,⁷⁷ IboView,⁷⁸ and VMD programs.⁷⁹

3. Results and discussion

Low-energy structures

Based on the results searched by using ABCluster, some low-energy structures of (B₂S₃)_n (n = 1–6) clusters have been identified. Prior to investigating the structural and electronic properties of these clusters in detail, a comparative analysis was conducted on the equilibrium structures and relative energy order of selected low-energy clusters evaluated by both the M06-2X-D3/6-31G(d,p) and G4(MP2)-6X methods, and there are no qualitative differences between the results obtained by the two methods, thereby confirming that the M06-2X-D3/6-31G(d,p) approach is capable of accurately evaluating the structures and properties of the studied boron-based clusters (see more details in Fig. S1–S6, ESI†). Herein, the following discussion mainly focuses on clusters with relative Gibbs free energies below 30.0 kcal mol⁻¹ at the M06-2X-D3/6-31G(d,p) level, as well as the isomers similar to the most stable (B₂O₃)_n cluster structures in previous studies.^25,58

As shown in Fig. 1, the most stable isomer I of the B₂S₃ cluster exhibits a V-shaped structure with alternating bonding between B and S atoms. In contrast, isomers II (T-shaped) and III (Y-shaped) with a B−B motif are less stable than I by 22.5 and 29.0 kcal mol⁻¹, respectively. For the B₄S₆ cluster, the most stable isomer I features a B₂S₂ rhombic core with two linear [S−B−S] arms in a trans-configuration, and its corresponding cis-configuration II is almost isoenergetic with I. The isomer III of B₄S₆ with two non-planar B₂S₂ units linked by S atoms is lying at 12.8 kcal mol⁻¹ above I. Notably, both isomers IV and V without the B₂S₂ moiety, with the boron-centered structure connecting three linear [S−B−S] motifs, are higher in energy than I by 19.4 and 22.3 kcal mol⁻¹, respectively. For relatively large clusters of B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈, their most stable isomers generally consist of several planar or non-planar B₂S₂ rings as the main structural units, connected by S atoms to form a closed structure. Obviously, their higher-energy structures tend to feature six-membered [B₃S₃] rings or linear [S−B−S] structural units. Overall, the low-energy isomers of (B₂S₃)_n clusters with n = 1 and 2 mainly exhibit a planar configuration, whereas a non-planar or cage configuration is adopted for the large-sized clusters with n = 3–6.

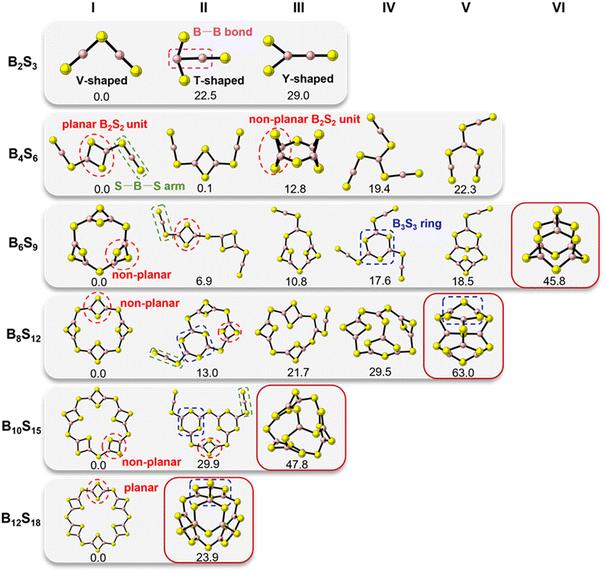Fig. 1 The optimized low-energy structures and relative free energies (in kcal mol⁻¹) of the studied boron sulfide clusters, where B and S atoms are in pink and yellow, respectively.

Interestingly, it was found that the most stable isomers of B₂S₃ and B₄S₆ have the same configuration as that of the corresponding B₂O₃ and B₄O₆ clusters.^25,58 Conversely, for relatively large clusters such as B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈, the isomers with structures similar to the corresponding boron oxide clusters are less stable than the most stable species by more than 20.0 kcal mol⁻¹. For instance, VI in B₆S₉, V in B₈S₁₂, III in B₁₀S₁₅, and II in B₁₂S₁₈ are higher in energy than their respective most stable isomers by 45.8, 63.0, 47.8, and 23.9 kcal mol⁻¹, respectively. Presumably, there may be significant differences in structural and electronic properties between the boron sulfide (B₂S₃)_n and boron oxide (B₂O₃)_n clusters.

Fig. 2a presents key bond lengths, Mayer bond orders, and bond angles for the most stable isomers of (B₂S₃)_n (n = 1–6) clusters. For planar B₂S₃ and B₄S₆ featuring linear [S−B−S] arms, the bonding between the terminal S and B atoms is intermediate between a double and a triple bond, with a Mayer bond order of 2.453 and bond lengths around 1.605 Å. For relatively large clusters, their most stable isomers are composed of planar or non-planar B₂S₂ cyclic structures bonded together by the sulfur atom, such as B₄S₆, B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈, where the B−S single bonds vary from 1.809 to 1.832 Å. Furthermore, the natural adaptive orbital (NAdO) analyses are performed to describe the distribution, character, and intensity of the chemical bonding.^80,81 The NAdO analysis reveals that the terminal B−S bond in B₄S₆ is characterized by three major NAdOs, one σ-type (0.914) and two π-type (0.621 and 0.601) bonding categories, and the occupancy sum of σ and π NAdOs is 2.116, indicating that the terminal B−S bond is between double and triple bonds, as shown by the Mayer bond order (2.453) analysis.

Fig. 2 (a) The optimized global minimum configurations of the studied (B₂S₃)_n clusters, where the key bond lengths (Å), Mayer bond orders, and bond angles (°) are given in black, blue, and red, respectively. (b) The NAdO analysis on the bonding modes of B₄S₆ is given, where B and S atoms are in pink and yellow, respectively.

Bonding and stability

In order to gain a deeper understanding of the bonding properties of the most stable isomer of the studied clusters, the adaptive natural density partitioning (AdNDP) method is employed to describe the key delocalized orbitals and bonding characteristics, as depicted in Fig. 3 and Fig. S7 (ESI†). For B₂S₃ in Fig. 3a, there are two lone pairs (LPs) of electrons on the S atoms with the occupied number (ON) of 1.96 |e|, four two-center two-electron (2c–2e) σ-bonds (ON = 1.99–2.00 |e|) from the inner B−S bond, four 2c–2e π-bonds (ON = 1.98–2.00 |e|) from the terminal B−S bond, and one 3c–2e π-bond (ON = 1.96–1.97 |e|). For B₄S₆ in Fig. 3b, there are four lone pairs of electrons mainly originating from the S atoms, ten 2c–2e σ bonds, four 2c–2e π bonds, two 3c–2e π bonds, one 4c–2e π bond, and one 2c–2e π* bond. It was found that the cyclic B₂S₂ structure is characterized by σ, π, and π* bonds. Note that the large-sized B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈ clusters have similar bonding characteristics, mainly including LPs on the S atoms, 2c–2e σ-bonds for the B−S single bonds, delocalized 3c–2e π-bonds contributed by the B−S−B units, and 4c–2e π-bonds and 2c–2e π*-bonds from the planar or non-planar B₂S₂ rings. Overall, the AdNDP analysis further deepens our understanding of the structures and bonding properties of the selected clusters.

Fig. 3 AdNDP delocalized bonding patterns and the corresponding occupied numbers for (a) B₂S₃, (b) B₄S₆, (c) B₆S₉, and (d) B₈S₁₂. The color of atoms: B-pink; S-yellow.

Additionally, the bonding strength between (B₂S₃)_n clusters and the B₂S₃ monolayer⁵² are compared under the same theoretical level (see more details in Table S3, ESI†). The results show that the bonding energy of the B−S bonds in (B₂S₃)_n clusters is generally stronger than that of the B₂S₃ monolayer (except B₆S₉ cluster). The B₆S₉ cluster has a lower bonding energy than the B₂S₃ monolayer, indicating its lower stability.

The key frontier molecular orbitals and the corresponding energy levels of the most stable clusters are shown in Fig. 4. It was found that the HOMO of B₂S₃ originates from the terminal B−S π bonding and the non-bonding 3p orbital of the meso S atoms, whereas the LUMO is characterized by the B−S antibonding (π*). Similarly, the HOMO of B₄S₆ is derived from the terminal B−S π bonding and the 3p orbitals of the connected S atoms, and its LUMO is mainly contributed by the S atoms of the B₂S₂ moiety. For the large-sized clusters, such as B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈, their HOMOs and LUMOs are primarily composed of the 3p orbitals of S atoms and the 2p orbitals of B atoms, respectively. As shown in Fig. 4b, these boron sulfide clusters generally have relatively large HOMO–LUMO gaps (E_GAP = 4.36–5.14 eV), indicating that all the studied (B₂S₃)_n (n = 1–6) clusters may possess highly stable electronic structures. As the number of B₂S₃ basic units increases, the E_GAP value decreases first and then maintains at about 4.4 eV.

Fig. 4 (a) The key molecular orbitals (isosurface = 0.05) and (b) the corresponding energy levels (in eV) of the studied (B₂S₃)_n clusters.

In order to understand the electronic gain and loss characteristics as well as the relative stability of these studied boron sulfide clusters, their adiabatic/vertical electron affinities (EA_a and EA_v) and adiabatic/vertical ionization potentials (IP_a and IP_v), average binding energies (E_b), and second-order energy difference (Δ²E) were calculated as follows:

EA_a = E⁰_therm − E⁻¹_therm

EA_v = E⁰ − E⁻¹

IP_a = E¹_therm − E⁰_therm

IP_v = E¹ − E⁰

E_therm = E_elec + E_corr

Δ²E([B₂S₃]_n) = E([B₂S₃]_n−1) + E([B₂S₃]_n+1) − 2E([B₂S₃]_n)

where E⁰, E¹, and E⁻¹ denote the electronic energies of the neutral (B₂S₃)_n clusters, and their negative and positive ions with ±1 charge, respectively, and E⁰_therm, E¹_therm, and E⁻¹_therm denote the sum of the corresponding electronic energies (E_elec) and thermally corrected electronic energies (E_corr) for the ground states of the neutral (B₂S₃)_n clusters and their charged states. To obtain the adiabatic quantities of EA and IP, we have conducted independent unbiased searches for the charged clusters, and the corresponding low-energy structures are presented in Fig. S7–S18 (ESI†). It should be note that global minima are partly different for neutral and charged clusters.

As shown in Table 1, as the cluster size increases, the EA_v values gradually increase overall, while the IP_a and IP_v values correspondingly decrease first and then remain less varied. Additionally, the EA_a value of B₁₂S₁₈ is exceptionally low at 1.25 eV, primarily attributed to significant structural reorganization upon the formation of the anion cluster B₁₂S₁₈⁻ (see more details in Fig. S16, ESI†). Notably, the relatively low EA_a and EA_v values suggest a diminished capability of the molecule to accommodate additional electrons, whereas the relatively large IP_a and IP_v values indicate that the corresponding clusters are highly stable in their neutral state. These observations collectively underscore the high chemical stability of the studied boron sulfide clusters. Note that the evaluated EAs and IPs are closely correlated with the frontier molecular orbital properties of the studied molecules. There is a sharp drop in IPs from B₄S₆ to B₆S₉, which can be ascribed to the transition of the HOMO composition from predominant π orbitals associated with B−S bonds to the 3p orbitals of S atoms.

Table 1 Calculated EA_a, EA_v, IP_a, IP_v, E_b, and Δ²E of the studied clusters at the B3LYP/def2-TZVP level^a

Cluster	EAa (eV)	EAv (eV)	IPa (eV)	IPv (eV)	E b (eV)	Δ^2E
a Adiabatic electron affinities EAa, vertical electron affinities EAv, adiabatic ionization potentials IPa, vertical ionization potentials IPv, average binding energies Eb, and second-order energy difference Δ^2E.
B2S3	1.79	0.69	9.60	9.73	0.1860	—
B4S6	1.63	1.17	9.13	9.23	0.1922	0.2720
B6S9	1.77	1.18	8.08	8.51	0.1936	−0.9400
B8S12	1.87	1.30	8.26	8.42	0.1961	0.2335
B10S15	2.00	1.77	8.50	8.45	0.1972	0.2345
B12S18	1.25	2.03	8.51	8.42	0.1976	—

---

The slightly increasing E_b suggests that the stability of (B₂S₃)_n (n = 1–6) clusters gradually enhances with the increase in cluster size. Furthermore, the second-order differences (Δ²E) reveal a different perspective on stability. Although most isomers have positive Δ²E values, B₆S₉ stands out with a significantly negative Δ²E value of −0.9400, suggesting a higher reactivity structurally, compared to the other clusters. Overall, these studied clusters show relatively high stability both structurally and electronically.

Infrared spectroscopy

To facilitate future identification of B₂S₃-type clusters, the predicted infrared spectra of the selected clusters are collected in Fig. 5. Note that the infrared spectrum of B₂S₃ shows two notable absorption bands, where the stronger peak at 1299 cm⁻¹ and the weaker one at 1337 cm⁻¹ arise from the asymmetric and symmetric stretching vibrations of both [S−B−S] units, respectively. The infrared spectrum of B₄S₆ displays three key vibrational absorption bands, where the adsorptions at 841 and 1320 cm⁻¹ are attributed to the symmetric and asymmetric stretching vibrations of the [S−B−S] unit, respectively, whereas the peak at 893 cm⁻¹ arises from the symmetric deformation of the B₂S₂ ring along the direction of B atoms. Additionally, the infrared spectra of B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈ share a common pattern due to their primary cyclic B₂S₂ structural units, and their main vibrational peaks all located at 842 cm⁻¹, which are attributed to the breathing vibrations of the B₂S₂ unit. Overall, the infrared spectral characteristics are closely tied to the core structural units of each cluster, which provide a theoretical basis to identify and distinguish the structure of B₂S₃-type clusters.

Fig. 5 Predicted infrared spectra of the studied (B₂S₃)_n clusters at the B3LYP/def2-TZVP level of theory.

Interactions of (B₂S₃)_n with small molecules

Due to the electron-deficient nature of boron, boron clusters and their derivatives often exhibit chemical reactivity toward small molecules.^82–86 Herein, it was found that the selected boron sulfide clusters can interact with small molecules, particularly NH₃ and CO, and the main binding modes were elucidated, based on DFT calculations. The binding energies (ΔE) were determined as follows:

ΔE = E_B2S3Xm − (E_B2S3 + m × E_X)

in which X and m represent the involved small molecules and their corresponding numbers, respectively.

As shown in Fig. 6, main interaction modes between (B₂S₃)_n (n = 1–6) clusters and NH₃ are explored. Note that the complexation between NH₃ and the B atom of (B₂S₃)_n is actually a Lewis acid–base interaction. As shown in Fig. 6b, the complex of NH₃ and B₂S₃ exhibits two configurations, arising from the nucleophilic attack of NH₃ on the B site from both inside and outside the V-shaped structure, while the overall structure remains planar. Energetically, [B₂S₃][NH₃]-I is a more stable configuration. According to the relative energies of various binding modes between B₄S₆ and NH₃ in Fig. 6c, it was found that NH₃ more readily binds to the cyclic B₂S₂ moiety rather than the linear [S−B−S] unit, with a difference in ΔE exceeding 10.0 kcal mol⁻¹. The binding interaction of NH₃ with the global minimum structure of clusters B₆S₉, B₈S₁₂, B₁₀S₁₅, and B₁₂S₁₈, forms a similar stable configuration, with the B atom in the B₂S₂ moiety as the main active site. Notably, the N−H bond (1.015 Å) and the H−N−H bond angle (106°) of NH₃ increase to some extent, upon binding to the selected clusters, as shown in Fig. 6. Overall, the studied clusters can stably adsorb NH₃ molecules through the Lewis acidity of their B atoms, which may be used for capture and separation of small molecules.

Fig. 6 The optimized structures and the relative energies (ΔE, in kcal mol⁻¹) of the NH₃ molecule (a) and studied complexes [B₂S₃]_n[NH₃] (b–g), taking the energy sum of individual components as the reference. Key N−H bond lengths (Å) and H−N−H angles (°) are given in black and red, respectively. The color of atoms: S-yellow; B-green; N-blue; H-white.

Subsequently, the interactions of (B₂S₃)_n (n = 1–6) clusters with carbon monoxide (CO) are further explored, as depicted in Fig. 7. We note that the bond length of the CO adsorbed at the B atom of the [S−B−S] unit is almost unchanged (1.132∼1.134 Å), compared to the free molecule, but the CO molecule bound to the B atom of the cyclic B₂S₂ moiety has a shortened C≡O bond with the bond length of about 1.27 Å, suggesting that the formation of a B−CO dative bond is beneficial to the dispersion of excess electrons on the carbon atom and thus further enhances the CO bonding interaction. The predicted binding energies (ΔE) range from −13.3 to −4.7 kcal mol⁻¹, where the B₆S₉ cluster has the strongest interaction toward CO among these clusters considered here.

Fig. 7 The optimized structures of the CO molecule (a) and studied complexes [B₂S₃]_n[CO] (b–g), and their corresponding CO binding energies (ΔE, in kcal mol⁻¹), where key C−O bond lengths (Å) are given in black. The color of atoms: S-yellow; B-green; C-gray; O-red.

As Fig. 6 and 7 show, B₆S₉ has the strongest binding interaction with both NH₃ and CO molecules among the boron sulfide clusters here. In addition, we investigated the interactions of (B₂S₃)_n clusters with multiple NH₃ or CO molecules, and the optimized structures and corresponding bind energies of these complexes [B₂S₃]_n[NH₃]_m and [B₂S₃]_n[CO]_m, are presented in Fig. S8 and S9 (ESI†). Overall, all B atoms of these boron sulfide clusters can bind these small molecules until the boron sites are saturated.

Overall, the present results suggest that B₂S₃-type clusters exhibit potential applications in organic catalysis and materials engineering, specifically reflected in the following aspects: (a) the strong Lewis acidity of electron-deficient boron centers may facilitate the catalytic activation of small molecules, analogous to established B–N catalytic systems;^87,88 (b) the structural stability and delocalized electron characteristics observed in these clusters provide design principles for developing novel 2D boron–sulfide materials with graphene-like architectures; (c) the bonding energy of the B−S bonds in (B₂S₃)_n clusters is generally stronger than that in the reported B₂S₃ monolayer,⁵² suggesting that the predicted clusters may offer broader application prospects compared to the B₂S₃ monolayer materials due to their enhanced stability.

4. Conclusions

Herein, DFT calculations combined with the artificial bee colony algorithm were used to investigate the structural evolution, bonding and reactivity of (B₂S₃)_n clusters. The computational results indicate that the low-energy isomers of these boron sulfides have different structural features as the number of B₂S₃ basic units increases. In particular, the global minimum structures of B₂S₃ and B₄S₆ present a planar configuration, while for the larger-sized clusters of (B₂S₃)_n with n = 3–6, their global minimum configurations are mainly non-planar or cage-like structures. Overall, the linear [S−B−S] and cyclic B₂S₂ structures are the primary constituent units of these clusters. These structural features differ from those observed in (B₂O₃)_n-type clusters to some extent. The most stable structures of relatively large (B₂S₃)_n clusters are generally assembled by the cyclic B₂S₂ motifs through S atoms as the linker, and the multiple bonding interactions, including 2c-2e σ and π bonds, 3c-2e and 4c-2e π bonds, lone pairs, etc., are responsible for their relatively high stability both structurally and electronically. These boron sulfides show high activity toward the capture of small molecules NH₃ and CO. The computational findings suggest that these (B₂S₃)_n clusters are promising for the development of boron-based functional materials.

Data availability

The data supporting this article have been included as part of the ESI.†

Conflicts of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgements

This work is supported by the National Natural Science Foundation of China (22373078 and 21933009).

References

1. R. Núñez, M. Tarrés, A. Ferrer-Ugalde, F. F. de Biani and F. Teixidor, Chem. Rev., 2016, 116, 14307–14378.
2. V. Avdeeva, E. Malinina and N. Kuznetsov, Coordin. Chem. Rev., 2022, 469, 214636.
3. R. J. Grams, W. L. Santos, I. R. Scorei, A. Abad-García, C. A. Rosenblum, A. Bita, H. Cerecetto, C. Viñas and M. A. Soriano-Ursúa, Chem. Rev., 2024, 124, 2441–2511.
4. S. Pan, J. Barroso, S. Jalife, T. Heine, K. R. Asmis and G. Merino, Acc. Chem. Res., 2019, 52, 2732–2744.
5. N. G. Szwacki, A. Sadrzadeh and B. I. Yakobson, Phys. Rev. Lett., 2007, 98, 166804.
6. A. Ray, I. Howard and K. Kanal, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 45, 14247–14255.
7. A. P. Sergeeva, Z. A. Piazza, C. Romanescu, W. L. Li, A. I. Boldyrev and L. S. Wang, J. Am. Chem. Soc., 2012, 134, 18065–18073.
8. S. Li, Z. Zhang, Z. Long, G. Sun and S. Qin, Sci. Rep., 2016, 6, 25020.
9. A. P. Sergeeva, D. Y. Zubarev, H. J. Zhai, A. I. Boldyrev and L. S. Wang, J. Am. Chem. Soc., 2008, 130, 7244–7246.
10. J. I. Aihara, H. Kanno and T. Ishida, J. Am. Chem. Soc., 2005, 127, 13324–13330.
11. A. P. Sergeeva, I. A. Popov, Z. A. Piazza, W. L. Li, C. Romanescu, L. S. Wang and A. I. Boldyrev, Acc. Chem. Res., 2014, 47, 1349–1358.
12. I. Boustani, Chem. Phys. Lett., 1995, 240, 135–140.
13. I. Boustani, Phys. Rev. B: Condens. Matter Mater. Phys., 1997, 55, 16426–16438.
14. T. Jian, X. Chen, S. D. Li, A. I. Boldyrev, J. Li and L. S. Wang, Chem. Soc. Rev., 2019, 48, 3550–3591.
15. H. Kato, K. Yamashita and K. Morokuma, Chem. Phys. Lett., 1992, 190, 361–366.
16. W. L. Li, Y. F. Zhao, H. S. Hu, J. Li and L. S. Wang, Angew. Chem., Int. Ed., 2014, 53, 5540–5545.
17. W. L. Li, X. Chen, T. Jian, T. T. Chen, J. Li and L. S. Wang, Nat. Rev. Chem., 2017, 1, 0071.
18. T. Jian, W. L. Li, X. Chen, T. T. Chen, G. V. Lopez, J. Li and L. S. Wang, Chem. Sci., 2016, 7, 7020–7027.
19. J. Wang, T. Yu, Y. Gao and Z. Wang, Sci. China. Mater., 2017, 60, 1264–1268.
20. L. S. Wang, Int. Rev. Phys. Chem., 2016, 35, 69–142.
21. H. J. Zhai, Y. F. Zhao, W. L. Li, Q. Chen, H. Bai, H. S. Hu, Z. A. Piazza, W. J. Tian, H. G. Lu and Y. B. Wu, Nat. Chem., 2014, 6, 727–731.
22. Q. Chen, W. L. Li, Y. F. Zhao, S. Y. Zhang, H. S. Hu, H. Bai, H. R. Li, W. J. Tian, H. G. Lu and H. J. Zhai, ACS Nano., 2015, 9, 754–760.
23. Y. J. Wang, Y. F. Zhao, W. L. Li, T. Jian, Q. Chen, X. R. You, T. Ou, X. Y. Zhao, H. J. Zhai and S. D. Li, J. Chem. Phys, 2016, 144, 064307.
24. H. J. Zhai, S. D. Li and L. S. Wang, J. Am. Chem. Soc., 2007, 129, 9254–9255.
25. B. I. Loukhovitski, A. V. Pelevkin and A. S. Sharipov, Phys. Chem. Chem. Phys., 2022, 24, 13130–13148.
26. S. D. Li, H. J. Zhai and L. S. Wang, J. Am. Chem. Soc., 2008, 130, 2573–2579.
27. D. Z. Li, L. Y. Feng, L. J. Zhang, L. Pei, W. J. Tian, P. F. Li and H. J. Zhai, J. Phys. Chem. A, 2018, 122, 2297–2306.
28. T. B. Tai, M. T. Nguyen and D. A. Dixon, J. Phys. Chem. A, 2010, 114, 2893–2912.
29. S. J. Gao, P. F. Han, J. C. Guo and H. J. Zhai, J. Mol. Struct., 2024, 1295, 136769.
30. T. B. Tai and M. T. Nguyen, Chem. Phys. Lett., 2009, 483, 35–42.
31. W. J. Tian, X. R. You, D. Z. Li, T. Ou, Q. Chen, H. J. Zhai and S. D. Li, J. Chem. Phys, 2015, 143, 064303.
32. W. J. Tian, Z. Y. Guo and M. Yan, J. Chem. Phys, 2024, 161, 084301.
33. S. J. Gao, J. C. Guo and H. J. Zhai, Front. Chem., 2022, 10, 868782.
34. M. L. Drummond, V. Meunier and B. G. Sumpter, J. Phys. Chem. A, 2007, 111, 6539–6551.
35. M. T. Nguyen, M. H. Matus, V. T. Ngan, D. J. Grant and D. A. Dixon, J. Phys. Chem. A, 2009, 113, 4895–4909.
36. H. J. Zhai, Q. Chen, H. Bai, S. D. Li and L. S. Wang, Acc. Chem. Res., 2014, 47, 2435–2445.
37. B. M. Gimarc and N. Trinajstic, Inorg. Chem., 1982, 21, 21–25.
38. W. Z. Yao, J. C. Guo, H. G. Lu and S. D. Li, Int. J. Quantum Chem., 2010, 110, 2689–2696.
39. X. Y. Tang, Z. H. Cui, C. B. Shao and Y. H. Ding, Int. J. Quantum Chem., 2012, 112, 1299–1306.
40. D. Z. Li, R. Li, L. J. Zhang, T. Ou and H. J. Zhai, Phys. Chem. Chem. Phys., 2016, 18, 21412–21420.
41. D. Z. Li, S. G. Zhang and C. C. Dong, Chem. – Eur. J., 2016, 2016, 1103–1107.
42. L. J. Zhang, L. Y. Feng, H. Bian, L. Pei, D. Z. Li and H. J. Zhai, Int. J. Quantum Chem., 2020, 120, e26229.
43. S. P. Kuntar, A. Ghosh and T. K. Ghanty, J. Phys. Chem. A, 2022, 126, 7888–7900.
44. A. Sommer, P. N. Walsh and D. White, J. Chem. Phys, 1960, 33, 296.
45. F. T. Greene and P. W. Gilles, J. Am. Chem. Soc., 1962, 84, 3598–3599.
46. H. Y. Chen and P. W. Gilles, J. Am. Chem. Soc., 1970, 92, 2309–2312.
47. F. T. Greene and P. W. Gilles, J. Am. Chem. Soc., 1964, 86, 3964–3969.
48. H. Y. Chen and P. W. Gilles, J. Phys. Chem., 1972, 76, 2035–2038.
49. D. Z. Li, H. Bai, T. Ou, Q. Chen, H. J. Zhai and S. D. Li, J. Chem. Phys, 2015, 142, 014302.
50. L. J. Zhao, X. L. Xu, H. G. Xu, G. Feng and W. J. Zheng, New J. Chem., 2018, 42, 16021–16026.
51. S. X. Li, Y. J. Yang and D. L. Chen, ACS Omega, 2023, 8, 30757–30767.
52. W. D. Lu, D. Wang, Z. Zhao, W. Song, W. C. Li and A. H. Lu, ACS Catal., 2019, 9, 8263–8270.
53. R. W. Dorn, L. O. Mark, I. Hung, M. C. Cendejas, Y. Xu, P. L. Gor’kov, W. Mao, F. Ibrahim, Z. Gan and I. Hermans, J. Am. Chem. Soc., 2022, 144, 18766–18771.
54. P. Han, R. Yan, Y. Wei, L. Li, J. Luo, Y. Pan, B. Wang, J. Lin, S. Wan and H. Xiong, J. Am. Chem. Soc., 2023, 145, 10564–10575.
55. J. Tian, G. Collinge, S. F. Yuk, J. Lin, V. A. Glezakou, M. S. Lee, Y. Wang and R. Rousseau, ACS Catal., 2023, 13, 8219–8236.
56. J. Tian, J. Tan, Z. Zhang, P. Han, M. Yin, S. Wan, J. Lin, S. Wang and Y. Wang, Nat. Commun., 2020, 11, 5693.
57. H. Yan, S. Alayoglu, W. Wu, Y. Zhang, E. Weitz, P. C. Stair and J. M. Notestein, ACS Catal., 2021, 11, 9370–9376.
58. L. Li and L. Cheng, J. Chem. Phys, 2013, 138, 094312.
59. J. Guan, X. Zhang, Q. Li, K. Deng, P. Jena and E. Kan, Nanoscale, 2021, 13, 3627–3632.
60. X. Li, B. Cui, W. Zhao, Y. Xu, D. Zou and C. Yang, Nanotechnology, 2021, 32, 225401.
61. L. Li, X. Chen, Y. Huang, P. Zhang, D. Zhou, G. Zhang and B. Xiao, Appl. Surf. Sci., 2022, 602, 154295.
62. J. Zhang and M. Dolg, Phys. Chem. Chem. Phys., 2015, 17, 24173–24181.
63. J. Zhang and M. Dolg, Phys. Chem. Chem. Phys., 2016, 18, 3003–3010.
64. J. P. Doye and D. J. Wales, Phys. Rev. Lett., 1998, 80, 1357.
65. S. Kirkpatrick, C. D. Gelatt Jr and M. P. Vecchi, Science, 1983, 220, 671–680.
66. J. J. Stewart, J. Mol. Model., 2007, 13, 1173–1213.
67. C. Bannwarth, S. Ehlert and S. Grimme, J. Chem. Theory Comput., 2019, 15, 1652–1671.
68. S. Grimme, J. Antony, S. Ehrlich and H. Krieg, J. Chem. Phys, 2010, 132, 154104.
69. Y. Zhao and D. G. Truhlar, Theor. Chem. Acc., 2008, 120, 215–241.
70. P. C. Hariharan and J. A. Pople, Theor. Chim. Acta., 1973, 28, 213–222.
71. W. J. Hehre, R. Ditchfield and J. A. Pople, J. Chem. Phys, 1972, 56, 2257–2261.
72. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. J. Montgomery, J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, N. J. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, O. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 16, Revision A.03, Wallingford CT, 2016, vol. 2016.
73. B. Chan, J. Deng and L. Radom, J. Chem. Theory Comput., 2011, 7, 112–120.
74. K. B. Wiberg, Tetrahedron, 1968, 24, 1083–1096.
75. D. Y. Zubarev and A. I. Boldyrev, Phys. Chem. Chem. Phys., 2008, 10, 5207–5217.
76. T. Lu and F. Chen, J. Comput. Chem., 2012, 33, 580–592.
77. C. Legault, CYLview20, 2020, https://www.cylview.org.
78. G. Knizia, J. Chem. Theory Comput., 2013, 9, 4834–4843.
79. W. Humphrey, A. Dalke and K. Schulten, J. Mol. Graphics, 1996, 14, 33–38.
80. E. Francisco, A. Martín Pendás, M. García-Revilla and R. Álvarez Boto, Comput. Theor. Chem, 2013, 1003, 71–78.
81. M. Menéndez, R. Álvarez Boto, E. Francisco and A. Martín Pendás, J. Comput. Chem., 2015, 36, 833–843.
82. E. V. Bukovsky, A. M. Pluntze and S. H. Strauss, J. Fluorine. Chem., 2017, 203, 90–98.
83. J. C. Axtell, L. M. Saleh, E. A. Qian, A. I. Wixtrom and A. M. Spokoyny, Inorg. Chem., 2018, 57, 2333–2350.
84. K. R. Asmis, B. B. Beele, C. Jenne, S. Kawa, H. Knorke, M. C. Nierstenhöfer, X. B. Wang, J. Warneke, Z. Warneke and Q. Yuan, Chem. – Eur. J., 2020, 26, 14594–14601.
85. M. E. Kilic and P. J. Jena, Phys. Chem. Lett., 2023, 14, 8697–8701.
86. M. E. Kilic and P. J. Jena, Phys. Chem. A, 2024, 128, 1993–2002.
87. B. Miao, Z. Qiu, Z. Zhen, Y. Yang, Z. Yang, T. Xiao, J. Lv, S. Huang, Y. Wang and X. Ma, Phys. Chem. Chem. Phys., 2024, 26, 10494–10505.
88. M. M. Shmakov, S. A. Prikhod’ko, V. N. Panchenko, M. N. Timofeeva, V. V. Bardin, V. N. Parmon and N. Y. Adonin, Catal. Rev., 2024, 1–47.

---

Footnote

† Electronic supplementary information (ESI) available: Functional testing; low-energy structures of charged clusters; AdNDP analysis; optimized structures of studied molecular compounds; key interaction analysis between [B₂S₃] with NH₃ and CO molecules; absolute free energies and electronic energies; and Cartesian coordinates. See DOI: https://doi.org/10.1039/d4cp04699d

---