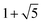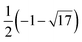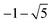Exactly solvable 1D model explains the low-energy vibrational level structure of protonated methane

Abstract

A new one-dimensional model is proposed for the low-energy vibrational quantum dynamics of CH₅⁺ based on the motion of an effective particle confined to a 60-vertex graph Γ₆₀ with a single edge length parameter. Within this model, the quantum states of CH₅⁺ are obtained in analytic form and are related to combinatorial properties of Γ₆₀. The bipartite structure of Γ₆₀ gives a simple explanation for curious symmetries observed in numerically exact variational calculations on CH₅⁺.

---

Protonated methane, CH₅⁺, also called methonium, is considered to be the prototype of pentacoordinated nonclassical carbonium ions.^1–3 The curious carbonium cations yielded an extremely rich chemistry and a Nobel prize to their discoverer, George Olah.⁴ Nevertheless, these are not the only sources of fame for carbonium ions and in particular for CH₅⁺. Over the last two decades,⁵ the internal motion of CH₅⁺ has been posing a formidable challenge to high-resolution spectroscopists.^5–15 The most outstanding issue is that the observed spectra of CH₅⁺ remain exceptionally complex even when they are observed at temperatures of a few K,^9,13 due to the quasistructural nature¹⁶ of this molecular ion.

As to the utilization of quantum chemistry to solve the experimental puzzle, through huge numerical efforts accurate rovibrational energy levels and eigenstates have been made available for CH₅⁺ in recent years.^7,12,14 These studies have revealed close-lying clusters in the rovibrational energy levels, with fascinating symmetry characteristics. These features have defied explanation by conventional means, motivating the development of novel models for CH₅⁺. The most important models put forward so far are as follows: (a) particle-on-a-sphere (POS),^17–23 (b) five-dimensional (5D) rotor (superrotor),^24–26 and (c) quantum graph.^15,27 So far, the quantum-graph model seems to have resulted in the most satisfactory explanation of the low-energy quantum dynamics of CH₅⁺, including both vibrations¹⁵ and rotations.²⁷

Quantum graphs have a long history in chemistry and physics, dating back to Linus Pauling's description of electrons in organic molecules in the 1930s.²⁸ They have only recently been introduced to the study of nuclear dynamics, where they have proved useful in high-resolution spectroscopy^15,27 and also in explaining α-cluster dynamics in nuclear physics.^29,30 Quantum graphs³¹ are metric graphs, that is each of their edges possesses a length. In the context of rovibrational dynamics of molecules, each vertex of the graph represents a version³² of an equilibrium structure. Depending on the nuclear permutation-inversion symmetry³² of the molecule of a given composition, even if the molecule has a single minimum on a given potential energy surface it may possess a large number of versions. The vertices defined by the versions are connected by edges which represent collective internal motions converting different versions into each other. Once a quantum graph is set up, one constructs the one-dimensional (1D) Schrödinger equation for an effective particle confined to the graph and solves it to determine the energy levels and eigenstates (ESI†). In this way, the complex multidimensional rovibrational quantum dynamics of a polyatomic molecule is mapped onto the effective motion of a 1D particle confined to a much simpler space.

In the case of CH₅⁺, the equilibrium structure, the only one found on its ground electronic state, is composed of a H₂ unit sitting on top of a CH₃⁺ tripod, an arrangement with C_s point-group symmetry. The five protons can be rearranged in 5! = 120 ways, generating 120 symmetry-equivalent versions. These versions become the 120 vertices of a quantum graph Γ₁₂₀.¹⁵ There are two types of motion interconverting the 120 versions, equivalent to scrambling the H atoms of CH₅⁺: the internal rotation of the H₂ unit by 60° (both clockwise and counterclockwise), and the flip motion that exchanges a pair of protons between the H₂ and CH₃⁺ units. The barriers to these motions on the potential energy hypersurface of CH₅^+ 33 are known to be relatively low. It is plausible that the low-energy dynamics is dominated by motion along these particular paths, so that motions other than the internal rotation and flip motions can be disregarded. Thus, one can take these motions to correspond to the edges of Γ₁₂₀. As one flip edge and two internal rotation edges are connected to each vertex of Γ₁₂₀, each vertex has a degree of three (Γ₁₂₀ is a 3-regular graph). Due to the nature of the underlying internal motions, the 120 internal rotation and 60 flip edges are assigned effective lengths L_rot and L_flip, respectively.

As shown before,^15,27 the quantum graph Γ₁₂₀ reproduces the low-energy rovibrational energy levels of CH₅⁺, as well as of CD₅⁺, remarkably well when optimized values are used for L_fip and L_rot (ESI†). For instance, the Γ₁₂₀ model perfectly reproduces the curious block structure (states occuring in groups of 15 and 30, see Table 1) of the vibrational eigenstates of CH₅⁺, first noted in a variational study of Wang and Carrington¹² and later confirmed in ref. 14. As seen in Table 1, rovibrational eigenstates of CH₅⁺ are labelled by irreducible representations (irreps) of the molecular symmetry (MS) group³²S₅* = S₅ × {E,E*}, generated by S₅ permutations of the five protons together with spatial inversion E* (E denotes the identity operation).

Table 1 The block structure characterizing the first 60 vibrational states of CH₅⁺, revealed in variational nuclear-motion computations.^12,14 The numbers in parentheses give the total number of positive and negative parity states within a block

Block 1	Block 2
0–60 cm^−1 (15,15)	110–200 cm^−1 (15,15)
A 1 ^+ ⊕ G1^+ ⊕ H1^+ ⊕ H2^+⊕	G 1 ^+ ⊕ H1^+ ⊕ I^+ ⊕
G 2 ^− ⊕ H2^− ⊕ I^−	A 2 ^− ⊕ G2^− ⊕ H1^− ⊕ H2^−

---

Beyond the existence of blocks, in Table 1 one can notice other clear symmetry relations for the first 60 quantum states. A comparison of the group-theoretic relation

(A₁⁺ ⊕ G₁⁺ ⊕ H₁⁺ ⊕ H₂⁺) ⊗ A₂⁻ ≃ A₂⁻ ⊕ G₂⁻ ⊕ H₂⁻ ⊕ H₁⁻ (1)

with the data in Table 1 suggests a direct correspondence between the 15 positive-parity states in Block 1 [appearing on the left-hand side (LHS) of eqn (1)] and the 15 negative-parity states in Block 2 [right-hand side (RHS) of eqn (1)]. Likewise,

(G₂⁻ ⊕ H₂⁻ ⊕ I⁻) ⊗ A₂⁻ ≃ G₁⁺ ⊕ H₁⁺ ⊕ I⁺, (2)

suggesting a link between the 15 negative-parity states in Block 1 and the positive-parity states in Block 2. These remarkable symmetry relations have been lacking any simple explanation, even in terms of the Γ₁₂₀ model. As this paper proves, introduction of the simplest quantum-graph model, Γ₆₀, of the quantum dynamics of CH₅⁺, derived from Γ₁₂₀, is sufficient to explain the curious energy-level and symmetry structure of the lowest vibrational states of CH₅⁺, and, as a bonus feature, it allows the analytic determination of the quantum states of the model problem.

Let us start our journey toward the simplest model with the quantum graph Γ₁₂₀. We recall two important characteristics of our original study.¹⁵ First, we neglect the potential energy along the edges of Γ₁₂₀, since the barriers to the internal rotation and flip motions are small (about 30 cm⁻¹ and 300 cm⁻¹, respectively³³). Second, we fix the effective edge lengths L_flip and L_rot. In ref. 15 this was done by an optimization procedure to give the best fit to either 7D or 12D reference data. In both cases the optimized L_flip was much smaller than the optimized L_rot, with the ratio L_flip/L_rot = 1.0/61.2 in the 7D case.

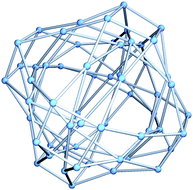
Our new model is based on the following idea: the ratio L_flip/L_rot is so small that it is tempting to imagine shrinking the flip edges to zero length, identifying the two vertices at the endpoints of each flip edge to give a single vertex. Setting L_flip = 0 has a negligible effect on the accuracy of the fit, at least at low energies. At the same time, this approximation gives a huge simplification: the number of vertices is halved and we get a new quantum graph, Γ₆₀, with only the internal rotation edges remaining. It is reasonable to identify each new vertex with the midpoint of the (now contracted) flip edge, which is a C_2v-symmetric transition state, as illustrated in Fig. 1. Γ₆₀ represents 60 symmetry-equivalent versions of this configuration. We propose that the most important characteristics of the low-energy vibrational quantum states of CH₅⁺ can be understood in terms of a 1D, potential-free motion between these versions corresponding to the vertices of the quantum graph Γ₆₀. Note that each vertex is connected to precisely four other vertices, as shown also in Fig. 1, giving rise to the 4-regular (quartic) quantum graph Γ₆₀, illustrated in Fig. 2.

Fig. 1 Local structure of the quantum graphs Γ₁₂₀ (blue and red edges) and Γ₆₀ (black edges). The red edges correspond to the flip motion and the labels indicate which proton is exchanged from a H₂ unit to a CH₃⁺ unit. The blue edges correspond to an internal rotation and the labels indicate the H₂ unit which rotates relative to the CH₃⁺ unit in a clockwise (+) or anticlockwise (−) fashion. The midpoint of each red flip edge is a C_2v-symmetric transition state (ts). In going from Γ₁₂₀ to Γ₆₀, the red edges shrink so that we are left with just the transition states connected by black edges.

Fig. 2 Illustration of the 4-regular quantum graph Γ₆₀. In this model of the quantum dynamics of CH₅⁺ there is a single edge length, connecting versions of C_2v-symmetric transition states, corresponding to midpoints of the flip edge of Γ₁₂₀.

There is an alternative way of rationalizing the above contraction procedure. At the energies we are interested in, one can show that the Γ₁₂₀ wave functions for the energy eigenstates are approximately constant along the flip edges. In this limit, the boundary conditions of Γ₁₂₀ become equivalent to those of Γ₆₀ (ESI†). Either way, Γ₆₀ only retains edges corresponding to the internal rotation. Our simplified model therefore has the feature of explaining the low-energy dynamics solely in terms of the internal rotation motion without the flip motion, with the constant wave function argument allowing for backstage full exchange of the protons. This model is thus set up in clear violation of the claim of the authors of ref. 34, namely that “the combination of the two [internal motions] enables large-amplitude motion and thus full scrambling … whereas partial scrambling leads to the well-known small-amplitude motion only”.

We now seek the quantum states corresponding to motion on the Γ₆₀ graph. The eigenenergies are found by solving the time-independent Schrödinger equation for a freeeffective particle moving along the edges, with the so-called Neumann boundary conditions³¹ imposed on the eigenstates. These conditions are that the wave function should be continuous everywhere, with zero total momentum flux out of each vertex. As we have already pointed out, Γ₆₀ is a 4-regular graph with all edges having a common length l = L_rot. Perhaps surprisingly, these properties imply that the structure of the quantum energy levels can be determined entirely from combinatorial properties of the graph.

More precisely, given a wave function ψ defined on the graph Γ₆₀ and obeying the time-independent Schrödinger equation along each edge,

where x is a mass-scaled coordinate, consider the vector of its values at each vertex v = (ψ(v₁),ψ(v₂),…). It is straightforward to prove (ESI†) that ψ is an eigenfunction with energy E satisfying the Neumann boundary conditions if and only ifi.e., if and only if is an eigenvalue of the adjacency matrix A for the graph Γ₆₀, with v in the corresponding eigenspace. A is simply a matrix whose elements indicate whether given pairs of vertices are connected by an edge or not:and is a familiar concept in elementary graph theory.³⁵

Eqn (4) therefore relates the quantum spectrum (the eigenvalues of the Hamiltonian) to the so-called combinatorial spectrum (the eigenvalues of the adjacency matrix). The combinatorial spectrum is a concept already utilized in molecular spectroscopy,³⁶ and only depends on the connectivity of the graph as encoded in A.

To find the combinatorial spectrum of Γ₆₀, we look for roots of the characteristic polynomial χ_A(λ) = det(λI − A) associated with the adjacency matrix A. An explicit expression for A is easily derived by considering paths of the form illustrated in Fig. 1. In the end, we obtain

χ_A(λ) = (λ⁴ − 9λ² + 16)⁵(λ⁴ − 12λ² + 16)⁴(λ² − 1)¹¹(λ² − 16), (6)

and the full combinatorial spectrum is given in Table 2. Table 2 also shows the dimensions of the corresponding eigenspaces and the irreps of the MS group S₅*.

Table 2 The combinatorial spectrum of the quantum graph Γ₆₀, where dim (λ) gives the degeneracy of a given eigenvector corresponding to the eigenvalue λ [see eqn (6)]

λ	dim(λ)	S 5* irrep
4	1	A 1 ^+
	4	G 2 ^−
	5	H 1 ^+
	5	H 2 ^−
	4	G 1 ^+
1	11	H 2 ^+ ⊕ I^−
−1	11	H 1 ^− ⊕ I^+
	4	G 2 ^−
	5	H 1 ^+
	5	H 2 ^−
	4	G 1 ^+
−4	1	A 2 ^−

---

We pause here to note the striking similarity between Tables 1 and 2. First, note that the combinatorial spectrum splits into positive λ and negative λ, with each corresponding to a total eigenspace dimension of 30. Moreover, the eigenspaces associated with positive λ transform in precisely the same irreps as Block 1 of Table 1, while those associated with negative λ transform precisely like Block 2. Thus, purely combinatorial properties of the quantum graph Γ₆₀ have captured the block structure of the lowest vibrational states of CH₅⁺. Even more interestingly, we have an explanation for the curious relationship between Block 1 states and Block 2 states: this corresponds to a λ → −λ symmetry of the combinatorial spectrum (see Table 2), under which the S₅* irreps are related by multiplication with A₂⁻. The symmetry of the combinatorial spectrum under λ → −λ is a simple consequence³⁵ of the fact that the quantum graph Γ₆₀ is bipartite: the set of vertices V can be divided into two disjoint and independent sets A and B such that every edge connects a vertex in A to one in B. The sets A and B are related by odd permutations of the protons (ESI†).

Eqn (4) relates the combinatorial spectrum to the quantum spectrum, as illustrated in Fig. 3. We can see the consequences of the λ → −λ symmetry for the quantum energy levels: each state in Block 1 comes with a partner in Block 2, with their corresponding values of being related by reflection in the line . In particular, the dimensionless ratios

are all equal to 1 in the Γ₆₀ model. These dimensionless ratios agree with the variational seven-dimensional model^7,12,14 results to within 20 percent (see the ESI†).

Fig. 3 Illustration of the block structure and the symmetry properties of the spectrum of the quantum graph Γ₆₀. Black dots indicate energies of the quantum states.

In this paper we have drastically simplified the quantum graph model of the low-energy rovibrational quantum dynamics of CH₅⁺ by reducing the original 120-vertex quantum graph to a 60-vertex graph, Γ₆₀. Γ₆₀ was constructed by shrinking the edges corresponding to the flip internal motion that exchanges a pair of protons between the H₂ and CH₃⁺ units of the equilibrium structure of CH₅⁺. Thus, at first sight we neglect one of the two important large-amplitude internal motions characterizing the exchange dynamics (scrambling) of the H atoms of CH₅⁺. This allows us to obtain the quantum states of Γ₆₀ in analytic form, with the structure of the energy levels depending only on combinatorial properties. The eigenvalues of this simple 1D, potential-free model are in excellent agreement with the energies of the first 60 vibrational states determined by sophisticated variational nuclear-motion computations utilizing a potential energy hypersurface. Furthermore, the bipartite structure of Γ₆₀ gives a natural explanation for symmetries in the vibrational energy-level structure of CH₅⁺, again in perfect agreement with the results of variational nuclear-dynamics computations. Note that neither the variational computations^7,12,14 nor the quantum-graph models^15,27 yield only the Pauli-allowed states of CH₅⁺ (states with A₂^±, G₂^±, and H₂^± symmetry have non-zero spin-statistical weights), so our discussion focused on all possible states; the non-existing states can be filtered out a posteriori.

The work of JIR was supported by the EPSRC grant CHAMPS EP/P021123/1. The work performed in Budapest received support from NKFIH (grant no. K119658) and from the ELTE Institutional Excellence Program (TKP2020-IKA-05) financed by the Hungarian Ministry of Human Capacities.

Conflicts of interest

There are no conflicts to declare.

Notes and references

1. G. A. Olah and R. H. Schlosberg, J. Am. Chem. Soc., 1968, 90, 2726–2727.
2. G. A. Olah, G. Klopman and R. H. Schlosberg, J. Am. Chem. Soc., 1969, 91, 3261–3268.
3. G. A. Olah, Carbocations and Electrophilic Reactions, VCH-Wiley Publishers, Weinheim, 1974.
4. G. A. Olah, My Search for Carbocations and Their Role in Chemistry, 1994, pp. 149–176.
5. E. T. White, J. Tang and T. Oka, Science, 1999, 284, 135–137.
6. O. Asvany, P. Padma Kumar, B. Redlich, I. Hegemann, S. Schlemmer and D. Marx, Science, 2005, 309, 1219–1222.
7. X.-G. Wang and T. Carrington Jr., J. Chem. Phys., 2008, 129, 234102.
8. S. D. Ivanov, O. Asvany, A. Witt, E. Hugo, G. Mathias, B. Redlich, D. Marx and S. Schlemmer, Nat. Chem., 2010, 2, 298–302.
9. O. Asvany, K. M. T. Yamada, S. Brünken, A. Potapov and S. Schlemmer, Science, 2015, 347, 1346–1349.
10. R. Wodraszka and U. Manthe, J. Phys. Chem. Lett., 2015, 6, 4229–4232.
11. H. Schmiedt, S. Schlemmer and P. Jensen, J. Chem. Phys., 2015, 143, 154302.
12. X.-G. Wang and T. Carrington, J. Chem. Phys., 2016, 144, 204304.
13. S. Brackertz, S. Schlemmer and O. Asvany, J. Mol. Spectrosc., 2017, 342, 73–82.
14. C. Fábri, M. Quack and A. G. Császár, J. Chem. Phys., 2017, 147, 134101.
15. C. Fábri and A. G. Császár, Phys. Chem. Chem. Phys., 2018, 20, 16913–16917.
16. A. G. Császár, C. Fábri and J. Sarka, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2020, 10, e1432.
17. G. A. Natanson, G. S. Ezra, G. Delgado-Barrio and R. S. Berry, J. Chem. Phys., 1984, 81, 3400–3406.
18. G. A. Natanson, G. S. Ezra, G. Delgado-Barrio and R. S. Berry, J. Chem. Phys., 1986, 84, 2035–2044.
19. D. M. Leitner, G. A. Natanson, R. Berry, P. Villarreal and G. Delgado-Barrio, Comput. Phys. Commun., 1988, 51, 207–216.
20. J. E. Hunter, D. M. Leitner, G. A. Natanson and R. Berry, Chem. Phys. Lett., 1988, 144, 145–148.
21. M. P. Deskevich and D. J. Nesbitt, J. Chem. Phys., 2005, 123, 084304.
22. M. P. Deskevich, A. B. McCoy, J. M. Hutson and D. J. Nesbitt, J. Chem. Phys., 2008, 128, 094306.
23. F. Uhl, L. Walewski, H. Forbert and D. Marx, J. Chem. Phys., 2014, 141, 104110.
24. H. Schmiedt, P. Jensen and S. Schlemmer, Phys. Rev. Lett., 2016, 117, 223002.
25. H. Schmiedt, P. Jensen and S. Schlemmer, Chem. Phys. Lett., 2017, 672, 34–46.
26. H. Schmiedt, P. Jensen and S. Schlemmer, J. Mol. Spectrosc., 2017, 342, 132–137.
27. J. I. Rawlinson, J. Chem. Phys., 2019, 151, 164303.
28. L. Pauling, J. Chem. Phys., 1936, 4, 673–677.
29. J. I. Rawlinson, Nucl. Phys. A, 2018, 975, 122–135.
30. J. I. Rawlinson, PhD thesis, University of Cambridge, 2020.
31. G. Berkolaiko and P. Kuchment, Introduction to Quantum Graphs, American Mathematical Society, 2013, vol. 186.
32. P. R. Bunker and P. Jensen, Molecular symmetry and spectroscopy, NRC Research Press, Ottawa, 2006.
33. P. R. Schreiner, S.-J. Kim, H. F. Schaefer III and P. von Ragué Schleyer, J. Chem. Phys., 1993, 99, 3716–3720.
34. A. Witt, S. D. Ivanov and D. Marx, Phys. Rev. Lett., 2013, 110, 083003.
35. N. Biggs, Algebraic Graph Theory, Cambridge University Press, 1993.
36. P. Árendás, T. Furtenbacher and A. G. Császár, J. Math. Chem., 2016, 54, 806–822.

---

Footnote

† Electronic supplementary information (ESI) available: Mathematical derivations and proofs relevant for the paper, technical details and vibrational energy levels of CH₅⁺. See DOI: 10.1039/d1cc01214b

---