 Open Access Article
Open Access Articlem-s-m cationic gemini and zwitterionic surfactants – a thermodynamic analysis of their mixed micelle formation
Aleisha McLachlana,
Kulbir Singh ab,
Michael McAlduffab,
D. Gerrard Marangoni
ab,
Michael McAlduffab,
D. Gerrard Marangoni *a,
Samantha Shortall
*a,
Samantha Shortall cd and
Shawn D. Wettig
cd and
Shawn D. Wettig cd
cd
aDept of Chemistry, St. F. X. University, Antigonish, NS B2G 2W5, Canada. E-mail: gmarango@stfx.ca; Fax: +1 902 867 2414; Tel: +1 902 867 2324
bSona Nanotech, 101 Research Drive, Dartmouth, NS B2Y 4M9, Canada
cSchool of Pharmacy, University of Waterloo, 200 University Ave. W., Waterloo, ON N2L 3G1, Canada. E-mail: wettig@uwaterloo.ca
dWaterloo Institute for Nanotechnology, University of Waterloo, 200 University Ave. W., Waterloo, ON N2L 3G1, Canada
First published on 20th January 2020
Abstract
Micelle formation enthalpies (ΔmicH values) have been calorimetrically determined at 298 K for three sets of mixed zwitterionic/cationic gemini systems consisting of N-dodecyl-N,N-dimethyl-3-ammonio-1-propanesulfonate (ZW3-12) and a series of structurally related gemini surfactants, the N,N'-bis(dimethyldodecyl)-α,ω-alkanediammonium dibromide (12-s-12) systems. From the experimental and the estimated ideal micelle formation enthalpies, the excess enthalpies were obtained. The degrees of nonideality of the interaction in the mixed micelle (βm) from our previous work was used along with the excess enthalpy values to determine excess thermodynamic quantities of the surfactants in the mixed system according to Regular Solution Theory (RST) and Motomura's theory. The excess enthalpies for the ZW3-12/12-4-12 were positive in magnitude and rose sharply when small amounts of the zwittergent were distributed into the gemini micelles. The excess enthalpies for the ZW3-12/12-5-12 and the ZW3-12/12-6-12 systems were also >0 kJ mol−1, and as a function of zwittergent composition, were quite different to those of the ZW3-12/12-4-12 mixed micelles. These results indicate that the heat of mixed micelle formation is strongly dependent on electrostatic interactions and the structure of the surfactants involved, specifically, the length of the tether group for the 12-s-12 gemini surfactants. From the calorimetric data and the application of RST and Motomura's theory, we have obtained estimates of the excess Gibbs energy and entropy of mixing. An analysis of the three thermodynamic properties suggests that the relative contributions of enthalpic and entropic effects to nonideal behavior for mixed micelles involving gemini surfactants are strongly dependent on the gemini structure.
Introduction
Surfactants possessing unusual architectures have been gaining increasing attention in the literature over the last decade. One such example of these architectures are so-called gemini or dimeric surfactants;1–8 they have a structure that can be represented as two monomeric surfactant units connected at or near the head groups by a spacer. This kind of architecture provides solution properties that are dependent upon the nature and size of the head groups as well as the spacer groups. Gemini surfactants are found to be superior to corresponding conventional monomeric surfactants in a number of ways, including lower critical micelle concentration (cmc) values, better lime-soap dispersion, and better wetting properties.8–12Gemini surfactants, like most conventional surfactants, are likely to be used in mixtures in applications. Zwitterionic surfactants are similar to gemini surfactants in that they have a dipolar head bearing both positive and negative charges, but generally only a single tail.13–22 In general, zwitterionic surfactants are mild to skin and eyes, have low toxicity, and display excellent water solubility, high foam stability, and excellent surface tension reducing properties.19,23,24 In combination with other surfactants, zwitterionic surfactants find applications in laundry detergents, shampoos, and other cosmetic products.
Although it is well known that surfactant mixtures often have improvements in performance of their mixtures versus the individual surfactant (i.e., synergism),25–34 there are few studies in the literature that examine the intricate molecular details that govern the thermodynamics of the formation of mixed micelles. In fact, most of the studies of synergism in mixed surfactant systems in the literature have dealt with how the critical micelle concentration (cmc value) compares in the mixture versus an ideal cmc calculated using an equation like that of Clint.35 While the mixture cmc is important, developing a more detailed fundamental understanding of the behavior of the surfactants in the formation of the mixed micellar solutions is required for enhancing their performance in applications where improved wettability and detergency is required.
Synergism is often modeled in the literature by employing the regular solution theory (RST)36 with an interaction parameter βm. Although RST has proven quite successful in accounting for the nonideal behavior of a number of binary surfactant systems, it does not adequately address the excess Gibbs energy in mixed surfactant systems.37 In the context of RST, the interaction parameter, βm, quantifies the nonidealities between the surfactants in the real mixed micelles relative to the interactions in the ideal mixed micelles of the pure surfactant micelles as being wholly enthalpic. Other thermodynamic treatments have been developed to interpret the deviations from the ideal micellar composition in terms of both enthalpic and entropic contributions.38 Motomura's theory is one such theory,39–43 and it is often used in the literature for the calculation of the Gibbs energy of micelle formation as a measure of molecular interactions.
Although there have been numerous reports on synergism in mixtures involving gemini surfactants with ionic and non-ionic conventional surfactants,31,44–57 there are only a few reports in the literature where a detailed thermodynamic analysis of synergistically interacting surfactant systems have been carried out calorimetrically.46,49,58–60 In this paper, the thermodynamics of the mixed micellization process for three sets of mixed zwitterionic/cationic gemini systems have been obtained using titration calorimetry (ITC). Specifically, the mixed surfactant systems investigated here consist of three members of the N,N′-bis (dimethyldodecyl)-α,ω-alkanediammonium dibromide (12-s-12) series (m-s-m gemini surfactants 12-4-12, 12-5-12, and 12-6-12) and the zwitterionic surfactant N-dodecyl-N,N-dimethyl-3-ammonio-1-propanesulfonate (ZW3-12). The interaction parameters from our previous paper have been used with the calorimetrically determined enthalpies to obtain the excess enthalpies and Gibbs energies on the basis of regular solution theory. Motomura's theory was also used to obtain excess thermodynamic functions, namely the excess Gibbs energy and entropy. The activity coefficients of each surfactant in the mixed micelles are obtained, and the tendency of surfactants to form mixed micelles and the interactions between the constituent surfactants are discussed.
Experimental
Materials
N,N-Dimethyldodecylamine, 1,4-dibromobutane, 1,5-dibromopentane, and 1,6-dibromohexane having purities of 97%, 99%, 97%, and 97% respectively were received from Sigma-Aldrich. N-dodecyl-N,N-dimethyl-3-ammonio-1-propanesulfonate (ZW3-12) with purity of 99% was received from Sigma-Aldrich and used without further purification.Methods
Results and discussion
Cmc values and mixed micelle thermodynamics
For the zwittergent/gemini surfactant mixed micelle, the ΔmicH values refer to the enthalpy change that occurs when NS moles of surfactant S (charge a), NC moles of counterion C (charge b), and NZ moles of zwittergent Z aggregate in aqueous solution to form the mixed micelle, M, of charge ±v| NsSa + NcCb + NzZ ⇌ M±v | (1) |
A typical plot of the heat evolution from the surfactant demicellization process being monitored is presented in Fig. 1a for the dilution of a 50.12 mM solution of ZW3-12, and Fig. 1b for the dilution of an approximately 50 mM solution containing both the zwittergent and the 12-4-12 gemini surfactant at a total fraction of zwittergent (αZW3-12) equal to 0.30. The regions in Fig. 1a correspond to (i) the dilution of the concentrated micellar containing solution to a more dilute micellar containing solution; (ii) the dilution of the concentrated micellar containing solution to a dilute solution containing surfactant monomers; and (iii) the transition between a solution containing predominantly monomers and a micellar containing solution. In order to evaluate the calorimetric cmc values, we have used the method of Yan63,64, and van Os.65 The ΔmicH values are obtained directly from the difference between the observed enthalpies in the two linear regions of the enthalpic titration curve; the cmc values are taken as the concentration where the deviation in linearity first appears in the transition region labelled (iii) in Fig. 1. The enthalpy change in that region is equal to the enthalpy change that occurs when the monomers transition to stable micelles. The enthalpy data for the three 12-s-12 surfactants and the mixed ZW3-12/12-s-12 systems are presented in Fig. 2 and given in Table 1 as a function of the composition of the surfactant solution (αZW3-12). In all cases, break points in the enthalpic titration curves correspond to the calorimetric cmc values and are in good agreement with the conductometric cmc values from our previous work.31 Fig. 2 clearly demonstrates that the enthalpy of micelle formation changes quite substantially as the composition of the mixed surfactant system is varied. As well, the cmc values from the previous conductometric investigations exhibit identical trends as the cmc values obtained calorimetrically. It could be argued that the deviations from ideal behavior seem to be more pronounced using the calorimetric cmc values versus the conductometric cmc values of the previous paper. This may indicate that the calorimetric technique is more sensitive to deviations from ideality versus the conductometric cmc values; this is currently being investigated in the lab for some well-known classical surfactant mixtures.
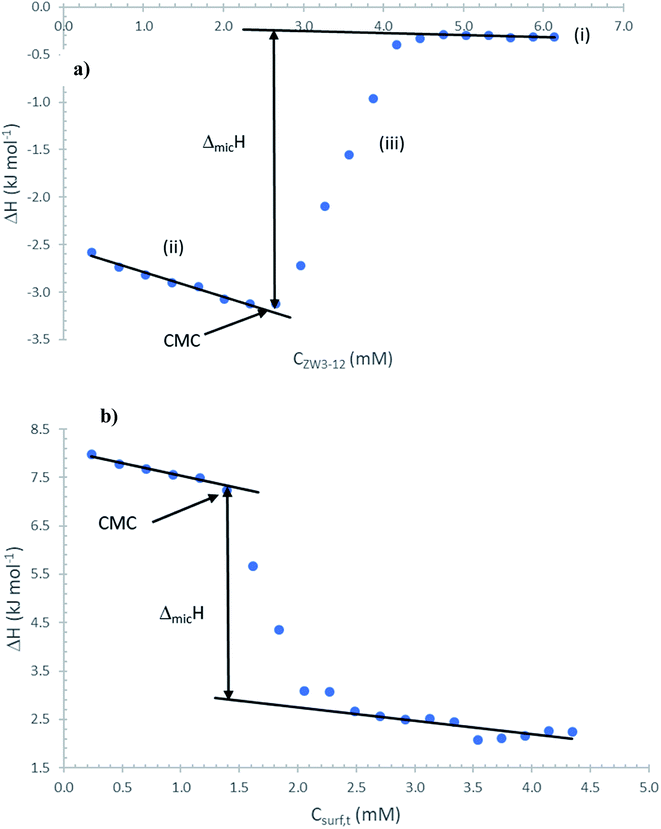 | ||
| Fig. 1 Enthalpograms for the titration of (a) 50.12 mM ZW3-12; (b) titration of a 47.58 mM ZW3-12/12-4-12 at αZW3-12 = 0.30 into water at 298.2 K. | ||
| αZW3-12 | ΔmicH (kJ mol−1) | Cmc (mM) | Cmcid (mM) | Xidzw3-12 | ΔmicHid (kJ mol−1) |
|---|---|---|---|---|---|
| 12-4-12 | |||||
| 0.000 | −9.36 | 1.10 | 1.10 | 0.000 | −9.36 |
| 0.100 | −5.80 | 1.25 | 1.17 | 0.044 | −8.81 |
| 0.300 | −4.38 | 1.37 | 1.33 | 0.152 | −7.48 |
| 0.500 | −1.84 | 1.52 | 1.55 | 0.295 | −5.71 |
| 0.700 | 1.50 | 1.58 | 1.86 | 0.494 | −3.25 |
| 0.900 | 3.44 | 2.22 | 2.31 | 0.790 | 0.42 |
| 1.000 | 3.02 | 2.63 | 2.63 | 1.000 | 3.02 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
|||||
| 12-5-12 | |||||
| 0.000 | −7.73 | 0.998 | 0.998 | 0.0000 | −7.73 |
| 0.100 | −6.14 | 1.07 | 1.06 | 0.040 | −7.30 |
| 0.300 | −3.93 | 1.22 | 1.23 | 0.140 | −6.23 |
| 0.500 | −2.31 | 1.47 | 1.45 | 0.275 | −4.77 |
| 0.700 | 2.40 | 1.63 | 1.76 | 0.470 | −2.68 |
| 0.900 | 2.80 | 1.98 | 2.26 | 0.774 | 0.59 |
| 1.000 | 3.02 | 2.63 | 2.63 | 1.000 | 3.02 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
|||||
| 12-6-12 | |||||
| 0.000 | −7.39 | 0.986 | 0.986 | 0.0000 | −7.39 |
| 0.100 | −5.58 | 0.993 | 1.05 | 0.040 | −6.97 |
| 0.300 | −3.84 | 1.06 | 1.21 | 0.138 | −5.95 |
| 0.500 | −1.75 | 1.15 | 1.43 | 0.273 | −4.55 |
| 0.700 | 2.50 | 1.31 | 1.75 | 0.467 | −2.53 |
| 0.900 | 2.84 | 1.67 | 2.25 | 0.771 | 0.64 |
| 1.000 | 3.02 | 2.63 | 2.63 | 1.000 | 3.02 |
In order to assess the ideal/nonideal nature of the micelle formation enthalpies, it is necessary to estimate the ideal micelle formation enthalpies by first calculating the ideal micellar mole fractions. Several theories have been developed to predict and analyze ideal cmc values, and hence, the ideal mole fractions of surfactant in the micellar phases in binary surfactant mixtures. Clint's eqn (2) is one such treatment.
 | (2) |
In this equation CMCmix is the cmc of the mixture; αi is the mole fraction of each component i in the solution; and CMCi is the cmc of each component i.35,66 Therefore, for a zwittergent/gemini surfactant mixture:
| 1/CMCmix = αZW3-12/CMCZW3-12 + αgem/CMCgem | (3) |
From the ideal mole fractions, ideal estimates of the micelle formation enthalpies were calculated as follows
| ΔmicHid = XidZW3-12ΔmicH(ZW3-12) + XidgemΔmicH(gem) | (4) |
The standard Gibbs energy of micellization (ΔmicG) represents the decrease in the molar Gibbs energy when one mole of surfactant transfer from the aqueous phase to the micellar phase. Using the phase separation model, for a zwitterionic micellar system, it is calculated as follows
ΔmicG = RT![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Xcmc Xcmc
| (5) |
Zana has derived the standard Gibbs energy changes for several different surfactant architectures, including bolaform surfactants and gemini surfactants.68 For gemini surfactants, the standard Gibbs energy of micellization is given by
ΔmicG = (0.5 + β)RT![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Xcmc Xcmc
| (6) |
| ΔmicS = (ΔmicH − ΔmicG)/T | (7) |
The values of the ΔmicH, ΔmicG, and ΔmicS are given in Table 2 for all the systems studied here. The cmc of the gemini surfactants is slightly less than that of the ZW3-12, meaning that the standard Gibbs energy change for the formation of the gemini pseudophases is less than that of the zwitterionic pseudophase. The gemini surfactants are also characterized by negative enthalpies of micelle formation, versus the positive ΔmicH values we observe for ZW3-12 which is likely due to the large attractive interactions between the hydrocarbon tails when the double tailed surfactants are brought in close proximity in the micellar phase. Although this decrease in the enthalpy is partially offset by hydrophobic effects and electrostatic interactions between the doubly charged surfactant headgroups, the dispersive van der Waal's interactions dominate the total observed enthalpy. In all cases, the ΔmicS values are positive, reflecting the disruption in the ordering of water molecules surrounding the hydrocarbon chains of the monomeric surfactants in the bulk solution. These positive entropy values are consistent with the decrease in partial molar entropy of the surfactants as they undergo co-micellization being offset by the increase in entropy of the water molecules that are “released” due to aggregation of the surfactants in the micelle interior.
| αZW3-12 | 12-4-12 | 12-5-12 | 12-6-12 | |||
|---|---|---|---|---|---|---|
| ΔmicG (kJ mol−1) | ΔmicS (J K−1 mol−1) | ΔmicG (kJ mol−1) | ΔmicS (J K−1 mol−1) | ΔmicG (kJ mol−1) | ΔmicS (J K−1 mol−1) | |
| 0.00 | −33.9 | 82.2 | −33.4 | 86.3 | −33.6 | 88.1 |
| 0.10 | −33.0 | 91.1 | −32.9 | 89.6 | −32.7 | 91.0 |
| 0.30 | −31.5 | 90.8 | −31.7 | 93.0 | −31.8 | 93.8 |
| 0.50 | −29.3 | 92.2 | −30.1 | 93.1 | −28.5 | 89.8 |
| 0.70 | −27.3 | 96.7 | −26.5 | 96.8 | −26.5 | 97.2 |
| 0.90 | −20.6 | 80.5 | −20.4 | 77.7 | −19.7 | 75.4 |
| 1.00 | −24.6 | 92.6 | −24.6 | 92.6 | −24.6 | 92.6 |
These results indicate that the micellization process for the gemini surfactants investigated here is driven both by entropic (hydrophobic effects) and enthalpic effects (alkyl chain interactions). For ZW3-12, the positive values of both ΔmicS and ΔmicH are consistent with the micellization process being entropy driven; this is clearly the case as more zwittergent is present in the mixed micelles, although the increases in enthalpy are greater than that expected of an ideal mixing of the surfactants. We note that, as expected, the enthalpies of mixed micellization trend towards more positive values as the fraction of zwittergent in the mixed system is increased. However, for low fractions of ZW3-12 in the mixed systems, the increase in the enthalpy is greater than what would be predicted from an ideal mixing of the two micellar pseudophases.
Regular solution theory, as applied to the interaction between two surfactants, uses the micellar interaction parameter, βm,69 to discuss the deviations from ideality in the context of micelle formation that can lead to the presence of synergism. While a number of papers in the literature use the existence of a negative βm parameter to suggest synergistic interactions are taking place between the surfactants comprising the mixed micelles, the following two conditions must be met in order for synergism to exist in mixed micelle formation: (1) βm must be negative, and (2) |βm| > |ln(CMC1/CMC2)|.69
In Fig. 4, we compare the ideal cmcs (obtained from Clint's equation, eqn (1)) and calorimetric cmcs for the ZW3-12/12-s-12 gemini systems. It can be seen from Table 1 that, in agreement with previous conductometric data, the three systems exhibit identical trends in the CMC values as a function of the surfactant mixture composition. As was stated in our previous paper, the negative βm values indicate attractive interactions in mixed systems; however, the zwittergent/12-4-12 and 12-5-12 systems only satisfy this condition at αZW3-12 = 0.9, whereas the ZW3-12/12-6-12 systems satisfy this above αZW3-12 = 0.6. Given the similarities in the trends in the calorimetric cmc values for the ZW3-12/12-s-12 mixed surfactant systems to the conductometric values, we expect a similar conclusion here.
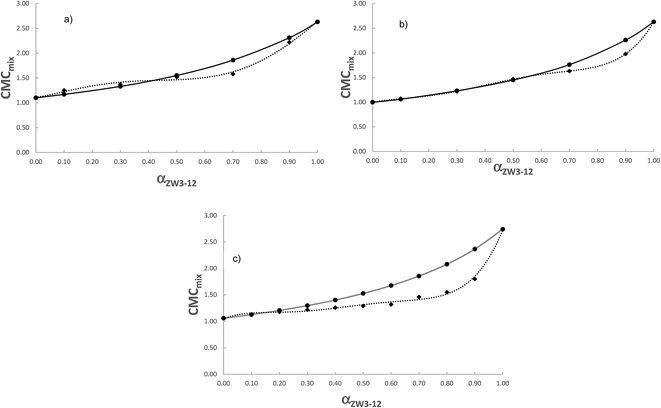 | ||
| Fig. 4 Comparison between ideal (●) and experimental cmc's (◆) for the ZW3-12/12-s-12 system. (a) 12-4-12; (b) 12-5-12; and (c) 12-6-12. | ||
Calculation of excess thermodynamic properties
The excess enthalpies are obtained by subtracting the ideal enthalpies (calculated above) from the calorimetric enthalpies as follows| HE = ΔmicH − ΔmicHid | (8) |
The excess enthalpies are given in Table 3 and plotted in Fig. 5 as a function of the fraction of ZW3-12 in the total surfactant amount. From Fig. 5, the excess enthalpies are observed to be more endothermic as the zwittergent molecules replace the gemini molecules in the mixed micelle; we also see a very different trend in the HE values for the 12-4-12/ZW3-12 system versus the values for either the 12-5-12/ZW3-12 and 12-6-12/ZW3-12 systems. The HE values for the 12-5-12 and the 12-6-12/ZW3-12 mixed micelles are virtually identical with one another, reflecting a similarity in the way these surfactants interact with each other in the formation of the mixed micelles. This is in excellent agreement with the NMR 2D-NOESY results in our previous paper that indicated differences in the manner in which 12-4-12 self-assembled with the ZW3-12 surfactant versus both the 12-5-12 and the 12-6-12.31 This can be explained in terms of the change in the radius of curvature of the micelles introduced when the zwittergent surfactants replace the gemini surfactants in the micelle,70 resulting in a highly asymmetric shape for the excess enthalpy curves for all the ZW3-12/12-s-12 systems with respect to αZW3-12. If we interpret the excess functions in terms of RST, we would expect the plot of the excess enthalpies to be symmetric as it would only consider pairwise interactions that lead to deviations from ideal behaviour. Clearly, in the systems studied here, the excess enthalpy curves are characterized by a rapid change in enthalpy versus composition. For the ZW3-12/12-4-12 system, this rapid change in the enthalpy occurs near αZW3-12 = 0.20, whereas for the 12-5-12 and the 12-6-12 systems, the rapid enthalpy change occurs near αZW3-12 = 0.60. This must be due to the slight extension that occurs in the tether group of the gemini surfactants with the addition of the methylene groups in the spacer, meaning that for the ZW3-12/12-4-12 systems, the enthalpically favourable interactions are weaker and less cooperative. Note that most of the values for HE are about a few kilojoules per mol, consistent with a random arrangement of molecules in the mixed micelle. By combining the NMR-NOESY data from our previous work31 with the enthalpic data in this paper, we conclude that steric and electrostatic interactions between headgroups and hydrophobic interactions between the surfactant tether groups and the hydrophobic chains play a significant role in the mixed aggregation process.
| αZW3-12 | 12-4-12 | 12-5-12 | 12-6-12 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| GE (kJ mol−1) | fZ | fgem | GE (kJ mol−1) | fZ | fgem | GE (kJ mol−1) | fZ | fgem | |
| 0.00 | 0.0 | — | 1.00 | 0.0 | — | 1.00 | 0.0 | — | 1.00 |
| 0.10 | 0.0 | 2.70 | 0.99 | 0.0 | 0.96 | 0.99 | 0.0 | 0.82 | 1.01 |
| 0.30 | 0.0 | 1.14 | 1.00 | 0.0 | 0.92 | 1.00 | −0.2 | 0.73 | 0.99 |
| 0.50 | −0.1 | 0.93 | 0.99 | 0.0 | 0.98 | 1.00 | −0.4 | 0.70 | 0.92 |
| 0.70 | −0.5 | 0.81 | 0.83 | −0.2 | 0.90 | 0.95 | −0.6 | 0.77 | 0.80 |
| 0.90 | −0.2 | 0.94 | 0.83 | −0.3 | 0.91 | 0.78 | −0.7 | 0.87 | 0.53 |
| 1.00 | 0.0 | 1.00 | — | 0.0 | 1.00 | — | 0.0 | 1.00 | — |
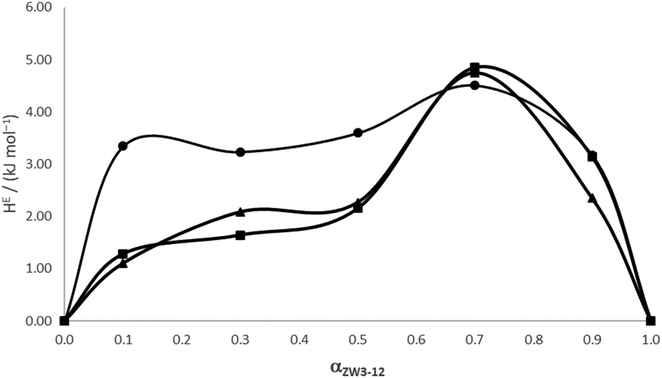 | ||
| Fig. 5 Excess enthalpies of mixing data for ZW3-12/12-s-12 system as a function of the mole fraction of zwittergent (αZW3-12). ● 12-4-12; ▲ 12-5-12; ■ 12-6-12. | ||
Regular solution theory calculates the activity coefficients from the mole fractions of the surfactants in the mixed micelles using the following equations
| fZ = exp(βm(1 − χZ)2) | (9) |
| fgem = exp(βm(1 − χgem)2) | (10) |
| GE = RTβm(χZ − χZ2) | (11) |
The excess Gibbs energy is a measure of the stability of the real mixed micelle relative to the stability of the ideal mixed micelles. The more negative the value of GE, the more stable the real mixed micelles are compared to aggregates created by mixing the pure micelles in the appropriate proportions. According to RST, this means that the formation of real mixed micelles are intrinsically more favoured than the formation of single surfactant micelles because the ideal micelles are aggregates in which no additional interactions exist!
The activity coefficients, and the GE values calculated according to RST are given in Table 3. From Table 3, we observe that the values of fZ and fgem both decrease at a constant αZW3-12 value. According to Rubingh and Holland, the activity coefficients reflect the strength of the intermolecular interactions that lead to the formation of mixed micelles. The values of the excess Gibbs energies are all quite small, consistent with the values of the interaction parameters from our previous paper. The positive values of GE for the 12-4-12/ZW3-12 mixed system at low values of αZW3-12 indicate that the Gibbs energy of micelle formation of mixed micelles is more positive than that of ideally mixing micelles, consistent with the interaction between the zwittergent and the gemini leading to micellar destabilization at low values of αZW3-12, in agreement with the HE values above.
The deviations from ideal mixing in the micelles can also be categorized by applying the following equations, resulting from Motomura's theory39–43
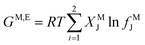 | (12) |
| CmcmixXZ = C0ZfMZXMZ | (13) |
| CmcmixXgem = C02fM2XM2 | (14) |
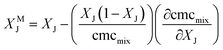 | (15) |
The values of XMZ and XMg, the activity coefficients, and the GM,E values calculated for all the zwittergent/gemini systems are given in Table 4; the GM,E values are plotted in Fig. 6. The excess entropy is calculated as follows
| SM,E = (HE − GM,E)/T | (16) |
| αZW3-12 | XMZ | XMg | fMZ | fMg | GM,E (kJ mol−1) | SM,E (J K−1 mol−1) |
|---|---|---|---|---|---|---|
| 12-4-12 | ||||||
| 0.00 | 0.000 | 1.000 | — | 1.00 | 0.00 | 0.0 |
| 0.10 | 0.045 | 0.955 | 0.49 | 1.08 | 0.11 | 10.9 |
| 0.30 | 0.155 | 0.845 | 0.55 | 1.22 | 0.19 | 10.2 |
| 0.50 | 0.301 | 0.699 | 0.60 | 1.35 | 0.13 | 11.6 |
| 0.70 | 0.502 | 0.498 | 0.68 | 1.54 | 0.06 | 14.9 |
| 0.90 | 0.795 | 0.205 | 0.81 | 1.83 | −0.10 | 11.0 |
| 1.00 | 1.000 | 0.000 | 1.00 | — | 0.00 | 0.0 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||
| 12-5-12 | ||||||
| 0.00 | 0.000 | 1.000 | — | 1.00 | 0.00 | 0.00 |
| 0.10 | 0.042 | 0.955 | 0.44 | 0.98 | −0.13 | 4.1 |
| 0.30 | 0.146 | 0.854 | 0.50 | 1.11 | −0.03 | 7.1 |
| 0.50 | 0.288 | 0.712 | 0.58 | 1.31 | 0.09 | 7.3 |
| 0.70 | 0.490 | 0.510 | 0.64 | 1.48 | −0.04 | 16.1 |
| 0.90 | 0.790 | 0.210 | 0.76 | 1.79 | −0.23 | 8.7 |
| 1.00 | 1.000 | 0.000 | 1.00 | — | 0.00 | 0.0 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||
| 12-6-12 | ||||||
| 0.00 | 0.000 | 1.000 | — | 1.00 | 0.00 | 0.0 |
| 0.10 | 0.040 | 0.960 | 0.42 | 0.94 | −0.23 | 4.3 |
| 0.30 | 0.141 | 0.859 | 0.45 | 1.02 | −0.25 | 5.5 |
| 0.50 | 0.280 | 0.720 | 0.47 | 1.08 | −0.39 | 7.2 |
| 0.70 | 0.480 | 0.520 | 0.53 | 1.23 | −0.50 | 16.3 |
| 0.90 | 0.784 | 0.216 | 0.65 | 1.55 | −0.60 | 10.5 |
| 1.00 | 1.000 | 0.000 | 1.00 | — | 0.00 | 0.0 |
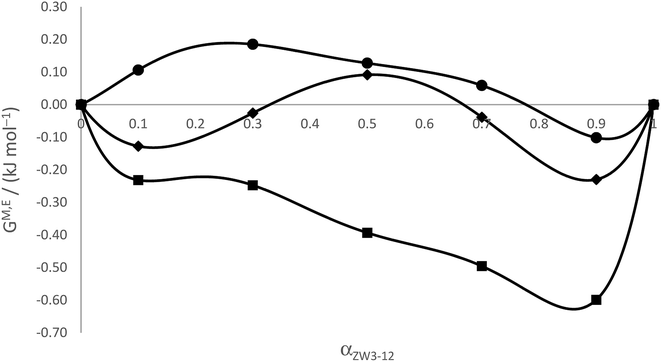 | ||
| Fig. 6 Excess Gibbs energies of mixing from Motomura's theory for ZW3-12/12-s-12 system as a function of the mole fraction of zwittergent (αZW3-12). ● 12-4-12; ♦ 12-5-12; ■ 12-6-12. | ||
The values for the excess entropy are also presented in Table 4 and plotted in Fig. 7. For the ZW3-12/12-4-12 system, the calculated values of the excess Gibbs energy are small and positive, particularly in the region of low values of αZW3-12, indicating that mixed micelle formation by these two surfactants is not energetically favoured in this region, in agreement with RST and the excess enthalpies above. This is likely due to the combination of an increased steric effect due to the size of the zwitterionic headgroup in the ZW3-12, coupled with the presence of the permanent positive charge in the zwitterionic headgroups that leads to significant repulsions as the two dissimilar headgroups attempt to pack in the micellar pseudophase, particularly at low values of αZW3-12. The trends in the GE values somewhat follow the variation in the βm values, and if both conditions for synergism are considered, the agreement between Motomura's theory and RST is quite satisfying!
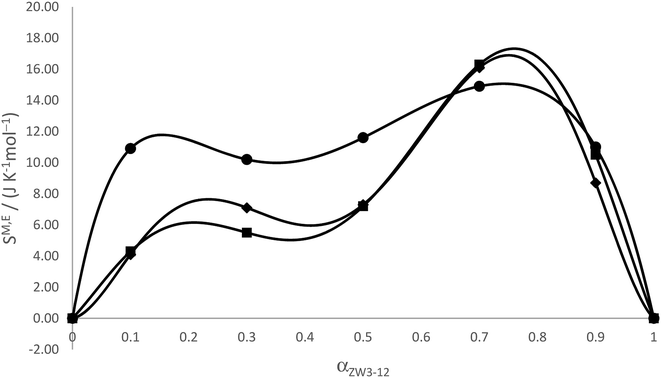 | ||
| Fig. 7 Excess entropies of mixing from Motomura's theory for ZW3-12/12-s-12 system as a function of the mole fraction of zwittergent (αZW3-12). ● 12-4-12; ♦ 12-5-12; ■ 12-6-12. | ||
An analysis of the results from both RST and Motomura's theory indicates nonideality for all the binary mixed systems and that synergistic interactions are observed, but only in certain compositions of the mixed surfactant system. From our previous paper and the work of Bakshi and Singh, we know that combinations of this zwitterionic surfactant could exhibit strong synergism.31,74 Surprisingly, although the zwitterionic surfactant possesses a permanent positive charge, when it is transported into the gemini surfactant pseudophases the micellization will still proceed spontaneously (ΔmicG < 0), even with the significant repulsions that would be experienced in the headgroup regions due to the presence of the permanent positive quaternary ammonium group in the zwittergent. In the case of the 12-4-12 mixed with the zwittergent, the GE values from both theories indicate some destabilization in the mixed micelle versus the mixture of the pure surfactant micelles at the same mole fraction. From Motomura's theory, the GE values and the calorimetrically obtained HE values also show the effect of the lengthening spacer. At small amounts of added zwittergent in the case of the 12-4-12 system, the positive charge localized on the quaternary ammonium interacts significantly with the two cationic quaternary ammonium groups on the gemini surfactants. Due to the short average length of the spacer chain (termed dS) versus the length where the electrostatic repulsions between the gemini head groups would be minimized (termed dT), the zwittergent will have difficulty mixing with gemini micelles as it is undergoing electrostatic interactions with the gemini headgroups. The increase in the excess entropy at these compositions may indicate that only some of the added zwittergent will form mixed micelles, i.e., the system will contain a large fraction of pure gemini micelles and possibly some pure zwittergent micelles. As the amount of the zwittergent is increased, the excess Gibbs energy, excess enthalpy, and excess entropy values plateau, consistent with a lessening of the electrostatic interactions as the zwittergent molecules replace more gemini molecules in the mixed aggregates. This has been observed in previous zwitterionic/cationic surfactant systems.74 In all three systems when the mixed micelles are rich with zwitterionic component, the excess enthalpies, Gibbs energies and entropies are close in magnitude, although in the case of the ZW3-12/12-4-12 system, the GE values only become negative at high amounts of added zwittergent, consistent with their synergistic interactions in this composition range.
The incorporation of zwitterionic surfactant monomers into the micelles of gemini surfactants, and their subsequent synergistic mixing, is clearly affected by the spacer length. This is most likely due to the increase in hydrophobicity at the level of the headgroups with the increase in the number of spacer methylene groups, and the change in the distance, dS. Mixed micelle formation is thermodynamically favorable (ΔmicG < 0), and the variation of the excess thermodynamic parameters of mixing indicate that the surfactants have a greater tendency to synergistically mix at high amounts of zwittergent and longer spacer lengths. At low zwittergent amounts, the steric interactions between the intercharge arms of the two surfactants are likely influencing the ability of the alkyl chains of the surfactants to fold back into the mixed aggregates. This corresponds well with the observations made by Bakshi and Singh,74 i.e., increased hydrophobic interactions occur when the dual chains of a gemini amphiphile are packed into a mixed micelle with the single tail of a conventional surfactant. These interactions play a predominant role in the appearance of synergistic interactions.46,58,74 At αZW3-12 = 0.2, when the excess thermodynamic values plateau for the ZW3-12/12-4-12 system, the tails of the gemini are likely not as prohibited from folding back. The excess thermodynamic properties in all the studied systems are relatively similar at high αZW3-12 values: the GE values are all negative, and the HE values and SE values are almost identical in value. For the ZW3-12/12-6-12 systems, the excess Gibbs energy is negative over all values of αZW3-12, indicating the interactions between the headgroups of the two surfactants and the tails are more favourable with the 6 carbon spacer length gemini surfactant. This is again consistent with the increase in dS, i.e., the dual cationic groups of the gemini are able to achieve greater separation which in turn provides room to accommodate the zwittergent head groups with its own cationic group. This increased distance between the quaternary nitrogens in the gemini allows for better packing in the headgroup regions, permitting the folding back of the alkyl chains of both surfactants and increased interactions in the mixed aggregates of the ZW3-12/12-6-12 system.
Conclusions
The mixed micelle formation enthalpies (ΔmicH values) were used along with regular solution theory and Motomura's theory to determine excess thermodynamic quantities of the surfactants in the mixed system. The non-zero excess enthalpies of mixed micelle formation increased rapidly as the zwittergent surfactant replaced the gemini surfactant in the ZW3-12/12-4-12 system; the HE values were observed to follow a different trend for both the ZW3-12/12-5-12 and the ZW3-12/12-6-12 systems. The excess thermodynamic properties indicate the mixed micelle formation mixing is strongly dependent on electrostatic interactions and the structure of the surfactants involved, specifically, the length of the tether group for the 12-s-12 gemini surfactants. An analysis of the excess thermodynamic properties suggests that the relative contribution of enthalpic and entropic effects to the nonideal behavior for mixed micelles involving gemini surfactants is strongly dependent on the gemini structure. The excess Gibbs energies thus obtained are in excellent agreement with the conclusion of our previous paper concerning the synergistic interactions in these systems, i.e., these systems are not truly synergistic over all compositions. The favourable alkyl tail interactions in these systems, coupled with the repulsive interactions observed between the headgroups in the ZW3-12 and the gemini surfactants, leads to increased hydrophobic interactions in the mixed micelles and greater attractive interactions as the spacer length of the gemini surfactant is increased.Conflicts of interest
The authors declare no conflict of interest.Acknowledgements
Funding support from the Natural Science and Engineering Research Council of Canada (NSERC Discovery Grant #335626-2011, SW and #6055-2018, DGM), the Canadian Foundation for Innovations (CFI), the Ontario Graduate Scholarship Program (OGS), St. F. X. University, and the University of Waterloo are gratefully acknowledged. We are grateful to Derek Leaist for stimulating discussions.References
- F. M. Menger and C. A. Littau, J. Am. Chem. Soc., 1991, 113, 1451–1452 CrossRef CAS.
- M. J. Rosen, Chemtech, 1993, 23, 30–33 CAS.
- R. Zana, M. Benrraou and R. Rueff, Langmuir, 1991, 7, 1072–1075 CrossRef CAS.
- E. Alami, H. Levy, R. Zana and A. Skoulios, Langmuir, 1993, 9, 940–944 CrossRef CAS.
- D. Danino, Y. Talmon and R. Zana, Langmuir, 1995, 11, 1456 CrossRef.
- L. Liu and M. J. Rosen, J. Colloid Interface Sci., 1996, 179, 454–459 CrossRef CAS.
- L. D. Song and M. J. Rosen, Langmuir, 1996, 12, 1153 Search PubMed.
- R. Zana, Curr. Opin. Colloid Interface Sci., 1996, 1, 566–571 CrossRef CAS.
- S. K. Hait and S. P. Moulik, Curr. Sci., 2002, 82, 1101–1111 CAS.
- R. Zana and E. Alami, in Novel Surfactants. Preparation, Application and Biodegradability, ed. K. Holmberg, CRC Press, New York, 1998, p. 398 Search PubMed.
- M. J. Rosen and D. J. Tracy, J. Surfactants Deterg., 1998, 1, 554 Search PubMed.
- F. M. Menger and J. S. Keiper, Angew. Chem., Int. Ed., 2000, 39, 1906–1920 CrossRef PubMed.
- M. Takai, H. Hidaka and M. Moriya, J. Am. Oil Chem. Soc., 1979, 56, 537–541 CrossRef CAS.
- B. Faucompre and B. Lindman, J. Phys. Chem., 1987, 91, 383–389 CrossRef CAS.
- A. Amin-Alami, N. Kamenka and S. Partyka, Thermochim. Acta, 1987, 122, 171–179 CrossRef CAS.
- P. M. MacDonald, J. R. Rydall and S. C. Kuebler, Langmuir, 1991, 7, 2602–2606 CrossRef CAS.
- E. W. Kaler, K. L. Herrington, A. K. Murthy and J. A. N. Zasadzinski, J. Phys. Chem., 1992, 96, 6698–6707 CrossRef CAS.
- Y. Chevalier, N. Kamenka, M. Chorro and R. Zana, Langmuir, 1996, 12, 3225–3232 CrossRef CAS.
- E. G. Lomax, Amphoteric surfactants, M. Dekker, New York, 1996 Search PubMed.
- M. K. Mullally and D. G. Marangoni, Can. J. Chem., 2004, 82, 1223–1229 CrossRef CAS.
- A. A. McLachlan, K. Singh and D. G. Marangoni, Colloid Polym. Sci., 2010, 288, 653–663 CrossRef CAS.
- K. Singh, Z. O'Toole, A. McLachlan and D. G. Marangoni, Langmuir, 2014, 30, 3673–3680 CrossRef CAS PubMed.
- M. Y. Pletnev, in Studies in Interface Science, Elsevier, 2001, vol. 13, pp. 1–97 Search PubMed.
- F. E. Friedli, Detergency of Specialty Surfactants, Marcel Dekker, Goldschmidt Chemical Corporation, Dublin, OH, USA, 2001, vol. 98, p. 98 Search PubMed.
- M. J. Rosen, Z. H. Zhu and T. Gao, J. Colloid Interface Sci., 1993, 157, 254–259 CrossRef CAS.
- M. J. Rosen, T. Gao, Y. Nakatsuji and A. Masuyama, Colloids Surf., A, 1994, 88, 1–11 CrossRef CAS.
- F. Li, M. J. Rosen and S. Sulthana, Langmuir, 2001, 17, 1037–1042 CrossRef CAS.
- A. F. Olea and C. Gamboa, J. Colloid Interface Sci., 2003, 257, 321–326 CrossRef CAS PubMed.
- Z.-X. Chen, S.-P. Deng and X.-K. Li, J. Colloid Interface Sci., 2008, 318, 389–396 CrossRef CAS PubMed.
- Kabir-ud-Din, U. Siddiqui, S. Kumar and A. A. Dar, Colloid Polym. Sci., 2006, 284, 807–812 CrossRef CAS.
- M. Aleisha, K. Singh, E. Piggott, M. McAlduff, S. MacLennan, S. Victoria, T. Reid, D. G. Marangoni, A. McLachlan, K. Singh, E. Piggott, M. McAlduff, S. MacLennan, V. Sandre, T. Reid and D. G. Marangoni, J. Phys. Chem. B, 2019, 123, 1855–1868 CrossRef PubMed.
- K. Singh and D. G. Marangoni, J. Colloid Interface Sci., 2007, 315, 620–626 CrossRef CAS PubMed.
- A. A. McLachlan and D. G. Marangoni, J. Colloid Interface Sci., 2006, 295, 243–248 CrossRef CAS PubMed.
- L. M. Zhou, X. H. Jiang, Y. T. Li, Z. Chen and X. Q. Hu, Langmuir, 2007, 23, 11404–11408 CrossRef CAS PubMed.
- J. H. Clint, J. Chem. Soc., Faraday Trans. 1, 1975, 71, 1327–1334 RSC.
- P. M. Holland and D. N. Rubingh, J. Phys. Chem., 1983, 87, 1984–1990 CrossRef CAS.
- M. J. Hey, J. W. MacTaggart and C. H. Rochester, J. Chem. Soc., Faraday Trans. 1, 1985, 81, 207–213 RSC.
- A. Shiloach and D. Blankschtein, Langmuir, 1997, 13, 3968–3981 CrossRef CAS.
- K. Motomura, J. Colloid Interface Sci., 1978, 64, 348–355 CrossRef CAS.
- K. Motomura, M. Yamanaka and M. Aratono, Colloid Polym. Sci., 1984, 262, 948–955 CrossRef CAS.
- K. Motomura, S.-I. Iwanaga, S. Uryu, H. Matsukiyo, M. Yamanaka and R. Matuura, Colloids Surf., 1984, 9, 19–31 CrossRef CAS.
- M. Aratono, T. Kanda and K. Motomura, Langmuir, 1990, 6, 843–846 CrossRef CAS.
- M. Villeneuve, H. Sakamoto, H. Minamizawa, N. Ikeda, K. Motomura and M. Aratono, J. Colloid Interface Sci., 1997, 194, 301–310 CrossRef CAS PubMed.
- Q.-Q. Yang, Q. Zhou and P. Somasundaran, J. Mol. Liq., 2009, 146, 105–111 CrossRef CAS.
- A.-M. Misselyn-Bauduin, A. Thibaut, J. Grandjean, G. Broze and R. Jérôme, Langmuir, 2000, 16, 4430–4435 CrossRef CAS.
- M. S. Bakshi, J. Singh, K. Singh and G. Kaur, Colloids Surf., A, 2004, 234, 77–84 CrossRef CAS.
- X. Fang, S. Zhao, S. Mao, J. Yu and Y. Du, Colloid Polym. Sci., 2003, 281, 455–460 CrossRef CAS.
- Q. Yang, Q. Zhou and P. Somasundaran, Colloids Surf., A, 2007, 305, 22–28 CrossRef CAS.
- M. S. Bakshi and G. Kaur, J. Colloid Interface Sci., 2005, 289, 551–559 CrossRef CAS PubMed.
- G. Sugihara, S. Nagadome, S.-W. Oh and J.-S. Ko, J. Oleo Sci., 2008, 57, 61–92 CrossRef CAS PubMed.
- A. Kumar, E. Alami, K. Holmberg, V. Seredyuk and F. M. Menger, Colloids Surf., A, 2003, 228, 197–207 CrossRef CAS.
- Kabir-ud-Din, M. S. Sheikh, M. A. Mir and A. A. Dar, J. Colloid Interface Sci., 2010, 344, 75–80 CrossRef CAS PubMed.
- M. S. Bakshi, J. Singh and G. Kaur, J. Photochem. Photobiol., A, 2005, 173, 202–210 CrossRef CAS.
- M. S. Bakshi and S. Sachar, J. Colloid Interface Sci., 2006, 296, 309–315 CrossRef CAS PubMed.
- T. Yoshimura, A. Ohno and K. Esumi, J. Colloid Interface Sci., 2004, 272, 191–196 CrossRef CAS PubMed.
- M. L. Sierra and M. Svensson, Langmuir, 1999, 15, 2301–2306 CrossRef CAS.
- A. Lainez, P. d. Burgo, E. Junquera and E. Aicart, Langmuir, 2004, 20, 5745–5752 CrossRef CAS PubMed.
- M. S. Bakshi, J. Singh, K. Singh and G. Kaur, Colloids Surf., A, 2004, 237, 61–71 CrossRef CAS.
- M. S. Bakshi, A. Kaura and R. K. Mahajan, Colloids Surf., A, 2005, 262, 168–174 CrossRef CAS.
- M. S. Bakshi, J. Singh and J. Kaur, J. Colloid Interface Sci., 2005, 287, 704–711 CrossRef CAS PubMed.
- S. Wettig and R. E. Verrall, J. Colloid Interface Sci., 2001, 244, 377–385 CrossRef CAS.
- X. Li, S. D. Wettig and R. E. Verrall, J. Colloid Interface Sci., 2005, 282, 466–477 CrossRef CAS PubMed.
- G. Bai, Y. Wang, J. Wang, B. Han and H. Yan, Langmuir, 2001, 17, 3522–3525 CrossRef CAS.
- G. Bai, J. Wang, Y. Wang, H. Yan and R. K. Thomas, J. Phys. Chem. B, 2002, 106, 6614–6616 CrossRef CAS.
- N. M. van Os, G. J. Daane and G. Haandrikman, J. Colloid Interface Sci., 1991, 141, 199–217 CrossRef CAS.
- P. P. Singh, K. Anand and O. P. Yadav, Indian J. Chem., 1989, 28, 1034–1037 Search PubMed.
- M. Cox, N. Borys and T. Matson, J. Am. Oil Chem. Soc., 1985, 62, 1139–1143 CrossRef CAS.
- R. Zana, Langmuir, 1996, 12, 1208–1211 CrossRef CAS.
- F. Li, G.-Z. Li and J.-B. Chen, Colloids Surf., A, 1998, 145, 167–174 CrossRef CAS.
- J. Hu, L. Zhou, J. Feng, H. Liu and Y. Hu, J. Colloid Interface Sci., 2007, 315, 761–767 CrossRef CAS PubMed.
- D. N. Rubingh, in Solution Chemistry of Surfactants, ed. K. L. Mittal, Springer, New York, Boston, MA, 1979, vol. 1, pp. 337–354 Search PubMed.
- B. M. Razavizadeh, M. Mousavi-Khoshdel, H. Gharibi, R. Behjatmanesh-Ardakani, S. Javadian and B. Sohrabi, J. Colloid Interface Sci., 2004, 276, 197–207 CrossRef CAS PubMed.
- H. Gharibi, S. Javadian, B. Sohrabi and R. Behjatmanesh, J. Colloid Interface Sci., 2005, 285, 351–359 CrossRef CAS PubMed.
- M. S. Bakshi and K. Singh, J. Colloid Interface Sci., 2005, 287, 288–297 CrossRef CAS PubMed.
| This journal is © The Royal Society of Chemistry 2020 |


