Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Size-dependent melting point depression of nickel nanoparticles†
Alexander
van Teijlingen
 a,
Sean A.
Davis
a,
Sean A.
Davis
 *b and
Simon R.
Hall
*b and
Simon R.
Hall
 *b
*b
aDepartment of Pure and Applied Chemistry, University of Strathclyde, 295 Cathedral Street, Glasgow, G1 1XL, UK
bSchool of Chemistry, University of Bristol, Bristol, BS8 1TS, UK. E-mail: s.a.davis@bristol.ac.uk; Simon.Hall@bristol.ac.uk
First published on 21st April 2020
Abstract
We investigate the phase-transition behaviour of nickel nanoparticles (3–6 nm) via dynamic TEM. The nanoparticles were synthesized within a reverse microemulsion and then monitored via dynamic TEM simultaneously while undergoing controlled heating. The size-dependent melting point depression experimentally observed is compared with, and is in good agreement with existing thermodynamic and molecular dynamic predictions.
Introduction
Metal and metal oxide nanoparticles exhibit uniquely size-dependent properties which normally follow an inverse surface area to volume proportionality.1 This has a depressive effect on the melting point, and has been shown experimentally for many metals.2–9 This effect was first predicted by P. Pawlow in 190910 and demonstrated experimentally in 1954 by M. Takagi.11 Buffat et al. investigated the melting point depression of spherical gold nanoparticles using the same ED method as Takagi et al., and noted that this method could only determine the range of melting points for an ensemble of particles.4 This has long been a standard technology in determining nanoparticle melting points.12 It has been purported, however, that measurements of melting points via ED becomes increasingly inaccurate as nanoparticle size decreases due to line-broadening.8 Determination of melting point depression has also been undertaken through the use of nano-DSC, Lai et al. investigated this property of tin nanoparticles, determining the melting point to be when there was an abrupt increase (“jump”) in heat flow required to sustain the increasing temperature.8 We apply a similar method as Zhang et al. in order to measure individual nanoparticles by direct observation of nanoparticles via TEM recognising record melting as an abrupt increase in observed diameter.13Synthesis
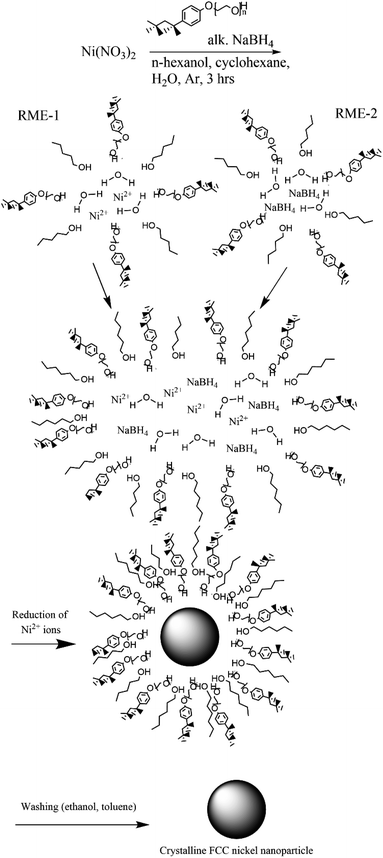
Nickel nitrate hexahydrate (Extra Pure SLR), cyclohexane (≥99.5%) and sodium hydroxide (≥97% NaOH) were purchased from Fisher Scientific. TX-100 (laboratory grade), 1-hexanol (98%) and NaBH4 (≥96%) were purchased from Sigma-Aldrich. The reaction procedure was scaled down from the work of Kumar et al.14 Four syntheses were conducted with different reactant concentrations (ESI Table 1†). The size of the nickel nanoparticles was controlled by varying the molar ratio of water to surfactant (W0). Each reaction does however produce a range of sizes (ESI Table 1 & ESI Fig. 1–4†), which we find itself useful in this study.In a typical synthesis (synthesis 1) a 5% (w/v) nickel nitrate solution was prepared by dissolving nickel nitrate hexahydrate (11.25 mg, 38.67 μmol) in water (0.225 mL). A 5% (w/v) alkaline NaBH4 solution was prepared in a similar fashion. Two reverse microemulsions (RME-1 and RME-2) were made up of cyclohexane (22.744 mL), 1-hexanol (0.3 mL) and TX-100 (1.731 mL, 2.50 mmol). To RME-1 under stirring 5% (w/v) nickel nitrate solution (0.225 mL) was added so that W0 was equal to 5 or 1 respectively. To RME-2 under stirring 5% (w/v) alkaline NaBH4 (0.225 mL) solution was added. Both reverse microemulsions were left to stir for 30 min to achieve homogeneity after which RME-2 was added dropwise to RME-1 with continuous stirring under an inert (argon) atmosphere. The resulting reverse micro-emulsion was left to stir for 3 hours to allow for nanoparticle growth via Ostwald ripening. The nickel nanoparticles were separated via centrifugation and washed with ethanol and separately with toluene then dispersed in water (Fig. 2). These reverse microemulsions continually collide and coalesce, mixing their internal constituents – this allows the sodium borohydride to reduce the nickel ions which then grow into a nanoparticle within the microemulsions. The synthesis has been visualized in Fig. 1.
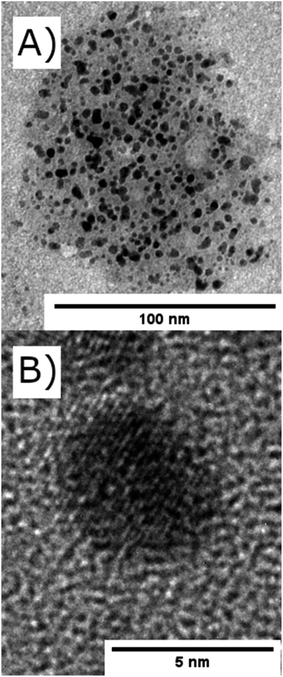 | ||
| Fig. 2 TEM images of (A) nickel nanoparticles encapsulated within a reverse microemulsions of TX-100/1-hexanol (d = 100 nm) and (B) a single nickel nanoparticle (d = 4.5 nm). | ||
Melting point characterization
Phase-pure and oxide free nickel nanoparticles on carbon coated copper TEM grids were prepared by drop-casting of a colloidal suspension of nickel nanoparticles in toluene. Using a Gatan heating stage (model number 628) with applied water cooling coupled to a JEOL-2100F TEM, we recorded the melting point of eight different nickel nanoparticles over a temperature range of 700–1100 °C at a rate of 5 °C min−1. Nanoparticle diameters were measured only when the video capture showed clearly de-fined edges. The heating stage independently measured the temperature of the sample and therefore heating effects from the electron beam are accounted for in our measurements. Recording the expansion of nanoparticles around the melting point allows the determination of this physical property unambiguously. Nanoparticles were determined to have melted when three requirements had been met:1. The average diameter of the spherical nanoparticle increased by at least 4.3% (this percentage was chosen as it is the difference in diameter for two equimolar spherical nickel nanoparticles, one a solid and one a liquid with perfect wetting on a substrate, densities 8.908 g cm−3 and 7.81 g cm−3 respectively).
2. The melting point is clearly identified by a rapid and sustained increase in size as opposed to gradual expansion due to heating of a solid.
3. The diameter of the liquid phase nanoparticle used to deter-mine the melting point does not have overlapping error bars with the diameter measurements of the solid nanoparticle.
Images at different temperatures were analysed using the soft-ware package ImageJ2, Fiji distribution15,16 by recording the projected TEM image surface area of individual nanoparticles (Fig. 3) and the diameter calculated according to (1).
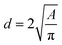 | (1) |
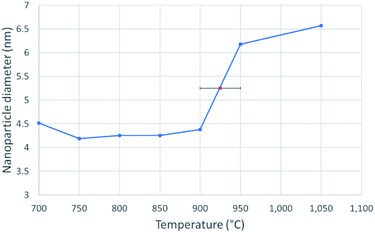
Fig. 3 shows the change in mean diameter as a function of projected nanoparticle surface area, for a single nickel nanoparticle over increasing temperatures. There is an abrupt increase at the melting point (925 °C ± 25 °C). The melting point was taken as the temperature halfway between the upper and lower points of the increase. This method is similar to the nano-DSC method described by Lai et al.8 This process was repeated for seven different nickel nanoparticles and the determined melting points plotted in Fig. 4. This experimental data is in good agreement with the MD studies and thermodynamic models also shown in Fig. 4.
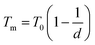 | (2) |
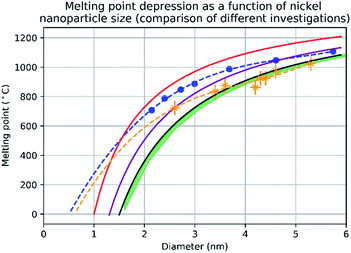 | ||
| Fig. 4 A graph showing the results of evaluating the melting point depression of nickel nanoparticles by four different methods. The red line denotes the melting point of nickel nanoparticles according to the empirical model (eqn (2)) from d = 1 nm to d = 6 nm with 1 nm increments. The blue trendline and markers plot the melting point results of the molecular dynamics study using the quantum-Sutton-Chen (QSC) force field reported by Qi et al.3 The purple line denotes values predict by the liquid-drop model24 with a β value given by Hanszen et al.25 The black line and markers represent values for the melting point as a function of size predicted by the Gibbs–Thompson (eqn (3)). The green area shows the temperatures between the upper and lower bounds of the liquid nucleation and growth model (eqn (4) and (5)) – this is in very close agreement with the experimental data between 3.5 nm and 5.3 nm, where most of the experimental data points where collected. The orange trendline and markers display the experimental data collected in this study, the vertical error bars show a ±50 °C uncertainty in temperature and the horizontal error bars show a ±0.1 nm uncertainty in diameter. | ||
The empirical relationship between diameter and melting point can be described by eqn (2) where T0 denotes the bulk melting point, d the diameter, Tm the melting point of the nanoparticle and the melting point is inversely related to the diameter.
 | (3) |
There exist several proposed mechanisms in which melting initialization and propagation in nanomaterials is described as a function of interface energies and the enthalpy of fusion (ΔmH). One of these models is the Gibbs–Thompson eqn (3), which assumes an isolated spherical nanoparticle situated within it's a liquid of its own type.7 While this model has some success in predicting melting point depression in our nanoparticles,2 it is generally believed that it is an incomplete model of solid–liquid phase transition in nanoparticles. More sophisticated models, such as the liquid nucleation and growth model (eqn (4) and (5)), which supposes that the liquid phase is initiated at a surface (a discontinuity) and propagates inwards towards the centre. At a critical radius (r*) an unstable equilibrium is formed, where r = r*. The temperature at which this is formed marks the upper boundary of the melting range (green area, Fig. 4), while the lower boundary is given by eqn (5).17
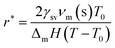 | (4) |
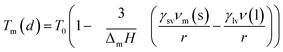 | (5) |
Thermodynamically, for the solid-to-liquid phase transformation to be energetically favourable, the sum of the energies of the newly formed solid–liquid and liquid–vapor interfaces must be less than or equal to the energy of the solid–vapor interface (eqn (6)).
| γsv ≥ γsl + γlv | (6) |
A key limitation of thermodynamic models is that melting is equivalent to stability such that changes in individual atoms in a system are not considered and therefore cannot reveal the mechanism of the melting process alone. In 2001 Qi et al. published a molecular dynamics study calculating the degree of melting point depression in nickel nanoparticles.3 By superheating and super-cooling nickel nanoparticles under the QSC potential (eqn (7)), they found the equilibrium melting temperature according to eqn (10). The data presented in Fig. 4 sheds light onto the accuracy of some thermodynamic and molecular dynamic models that have been used to predict the melting point depression as a function of size in nickel, showing that the liquid nucleation and growth model (green area) to be the model that fit best with the experimental data (orange line).
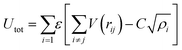 | (7) |
 | (8) |
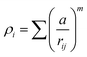 | (9) |
 | (10) |
Experimental data presented herein (Fig. 4) have shown that MD studies using the QSC force field, while generally in agreement with the experimental data,3,18,19 consistently over-predict the melting points of all sizes of nickel nanoparticles studied – a predictable outcome as their system was shown to determine the melting point of bulk nickel to be 1487 °C (32 °C higher than the real bulk melting point). This QSC force field has previously been used to accurately predict melting point depression in gold,20 copper,6 platinum21 and iron.22 It is of note that in each system the bulk melting point of each element was overpredicted. Finally we compared the normalised (ΔT = T/T0) and undercooled (ΔT = T0 − T) QSC MD study and find that, while closer to the experimental data they over- and under- predict the individual melting points respectively (ESI Fig. 5 and 6†).
Conclusions
In this study we have synthesized nickel nanoparticles of varying diameter via a reverse microemulsion synthesis and for the first time we report experimental studies which complement and validate previous modelling of nickel nanoparticle melting point depression. We have used this method to report a detailed investigation of the melting point depression in nickel nanoparticles. The method could be improved and expanded using alternative TEM grids, such as silicon nitride grids, as they are more stable than copper at elevated temperatures, allowing experiment and modelling to be tested over a larger size range. Future experiments may also include both heating/cooling cycles where the properties of different nanoparticles can be analysed at the 2nd, 3rd, 4th etc. cycle of heating as well as their behaviour upon cooling.23 With sufficient distribution on the grid, we speculate that this method would be appropriate to measure the properties of completely different nanoparticles simultaneously. Just as we have used it to study different sizes of nickel nanoparticles simultaneously, it could be used to determine melting point depression in magnesium oxide and aluminium oxide simultaneously or the sintering of any two or more different nanoparticles.Conflicts of interest
There are no conflicts to declare.Acknowledgements
The authors would like to acknowledge the Bristol Centre for Functional NanoMaterials funded by the Engineering and Physical Sciences Research Council (EPRSC) UK (grantsEP/G036780/1 and EP/L015544). TEM studies were carried out in the Chemistry Imaging Facility with equipment funded by UoB and EPSRC (EP/K035746/1 and EP/M028216/1).Notes and references
- D. K. Pattadar and F. P. Zamborini, J. Am. Chem. Soc., 2018, 140, 14126–14133 CrossRef CAS PubMed.
- W. Luo, W. Hu and S. Xiao, J. Phys. Chem. C, 2008, 112, 2359–2369 CrossRef CAS.
- Y. Qi, T. Çain, W. L. Johnson and W. A. Goddard, J. Chem. Phys., 2001, 115, 385–394 CrossRef CAS.
- P. Buffat and J.-P. Borel, Phys. Rev. A, 1976, 13, 2287–2298 CrossRef CAS.
- K. Dick, T. Dhanasekaran, Z. Zhang and D. Meisel, J. Am. Chem. Soc., 2002, 124, 2312–2317 CrossRef CAS PubMed.
- H. H. Kart, H. Yildirim, S. Ozdemir Kart and T. Çaǧin, Mater. Chem. Phys., 2014, 147, 204–212 CrossRef CAS.
- J. Sun and S. L. Simon, Thermochim. Acta, 2007, 463, 32–40 CrossRef CAS.
- S. L. Lai, J. Y. Guo, V. Petrova, G. Ramanath and L. H. Allen, Phys. Rev. Lett., 1996, 77, 99–102 CrossRef CAS PubMed.
- H. J. Kim, I. K. Beak, K. H. Kim and S. P. Jang, Trans. Korean Soc. Mech. Eng. B, 2014, 38, 695–700 CrossRef.
- P. Pawlow, Z. phys. Chem., 1909, 65, 545–548 CAS.
- M. Takagi, J. Phys. Soc. Jpn., 1954, 9, 359–363 CrossRef.
- J. Zhang, Y. Zheng, D. Zhao, S. Yang, L. Yang, Z. Liu, R. Zhang, S. Wang, D. Zhang and L. Chen, J. Phys. Chem. C, 2016, 120, 10686–10690 CrossRef CAS.
- M. Zhang, E. A. Olson, R. D. Twesten, J. G. Wen, L. H. Allen, I. M. Robertson and I. Petrov, J. Mater. Res., 2005, 20, 1802–1807 CrossRef CAS.
- A. Kumar, A. Saxena, A. De, R. Shankar and S. Mozumdar, Adv. Nat. Sci.: Nanosci. Nanotechnol., 2013, 4, 25009 Search PubMed.
- J. Schindelin, I. Arganda-Carreras, E. Frise, V. Kaynig, M. Longair, T. Pietzsch, S. Preibisch, C. Rueden, S. Saalfeld, B. Schmid, J. Y. Tinevez, D. J. White, V. Hartenstein, K. Eliceiri, P. Tomancak and A. Cardona, Nat. Methods, 2012, 9, 676–682 CrossRef CAS PubMed.
- C. T. Rueden, J. Schindelin, M. C. Hiner, B. E. DeZonia, A. E. Walter, E. T. Arena and K. W. Eliceiri, BMC Bioinf., 2017, 18, 1–26 CrossRef PubMed.
- G. Guenther and O. Guillon, J. Mater. Sci., 2014, 49, 7915–7932 CrossRef CAS.
- T. D. Nguyen, C. C. Nguyen and V. H. Tran, RSC Adv., 2017, 7, 25406–25413 RSC.
- T. Çagin, Y. Qi, H. Li, Y. Kimura, H. Ikeda, W. L. Johnson and W. A. Goddard III, MRS Symposium Ser., 1999, vol. 554, p. 43 Search PubMed.
- Z. Qiao, H. Feng and J. Zhou, Phase Transitions, 2014, 87, 59–70 CrossRef CAS.
- H. Akbarzadeh and G. A. Parsafar, Fluid Phase Equilib., 2009, 280, 16–21 CrossRef CAS.
- T. Shen, W. Meng, Y. Wu and X. Lu, Appl. Surf. Sci., 2013, 277, 7–14 CrossRef CAS.
- H. Chamati and K. Gaminchev, in J. Phys.: Conf. Ser., IOP Publishing, 2012, vol. 398, p. 012042 Search PubMed.
- K. K. Nanda, S. N. Sahu and S. N. Behera, Phys. Rev. A: At., Mol., Opt. Phys., 2002, 66, 132081–132088 CrossRef.
- K.-J. Hanszen, Z. Phys., 1960, 157, 523–553 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/d0na00153h |
| This journal is © The Royal Society of Chemistry 2020 |