Cyanine dyes as ratiometric fluorescence standards for the far-red spectral region†
Joshua K. G.
Karlsson
 a,
Owen J.
Woodford
a,
Heinz
Mustroph
b and
Anthony
Harriman
a,
Owen J.
Woodford
a,
Heinz
Mustroph
b and
Anthony
Harriman
 *a
*a
aMolecular Photonics Laboratory, School of Chemistry, Bedson Building, Newcastle University, Newcastle upon Tyne, NE1 7RU, UK. E-mail: anthony.harriman@ncl.ac.uk
bFEW Chemicals GmbH, Ortsteil Wolfen, Technikumstr. 1, 06766 Bitterfeld-Wolfen, Germany
First published on 4th December 2017
Abstract
Most quantitative fluorescence measurements report emission quantum yields by referring the integrated fluorescence profile to that of a well-known standard compound measured under carefully controlled conditions. This simple protocol works well provided an appropriate standard fluorophore is available and that the experimental conditions used for reference and unknown are closely comparable. Commercial fluorescence spectrophotometers tend to perform very well at wavelengths between 250 and 650 nm but are less responsive at longer wavelengths. There are no recognized emission standards for the far-red region. We now report fluorescence quantum yields for a series of commercially available cyanine dyes in methanol solution at room temperature. The compounds are selected to span the wavelength region from 600 to 850 nm, with absolute emission quantum yields being determined by thermal blooming spectrometry. Calibration of the instrument is made by reference to aluminium(III) phthalocyanine tetrasulfonate and aza-BODIPY in methanol.
Introduction
There appears to be an almost insatiable appetite for new fluorescent dyes. Indeed, a bewildering variety of applications has been found for such materials, sometimes by serendipity but more often by design. In characterizing new fluorescent dyes, the minimum information that must be provided is the fluorescence quantum yield under carefully specified conditions. This value, together with absorption and emission spectral profiles, can be used by other researchers seeking components for yet more applications. Of course, the excited-state lifetime and the extent of any environmental effects on this parameter and on the fluorescence yield add to the value of the basic information.1 As applications become more diverse, so does the awareness that compounds absorbing and emitting in the far-red region are of prime importance. This is especially so for bio-labels2 and fluorescent probes3 intended for biochemical or medicinal purposes, as soft tissues are best permeated by light of wavelength greater than 650 nm. In turn, this realization means that there is a growing need to measure emission quantum yields at relatively long wavelength where many commercial fluorescence spectrophotometers display limited sensitivity. There are few standard compounds4 with well documented emission properties, perhaps Indocyanine Green is the best known example,5 which is a further limitation for the determination of fluorescence yields via the comparative method. Other approaches, notably the use of an integrating sphere,6 are possible but the necessary equipment is not always at hand.In searching for likely emitters that could function as fluorescence standards, it has to be recognized that no single compound will suffice for the far-red region: we take the latter to cover the range from 650 to 900 nm. This translates to the need for a family of dyes of comparable chemical structures and physiological properties but differing conjugation lengths. Any standard compound must be commercially available in a pure form and soluble in common organic solvents. Ideally, the fluorescence quantum yield should be around 0.25 so that comparison can be made with strong and weak fluorophores.4 Secondary requisites include good stability and resistance to aggregation. With these limitations in mind, we have identified the family of cyanine dyes as strong contenders. This particular family includes fluorophores currently used in super-resolution microscopy.7
We now report the absolute fluorescence quantum yields, measured under highly comparable conditions, for a small set of cyanine dyes. These dyes cover the appropriate spectral range and show moderate emission yields in methanol solution at ambient temperature. To measure the quantum yields, we have used the photo-initiated thermal blooming protocol introduced many years ago by Magde and co-workers,8 but largely overlooked in the meantime. For the series of methanol-soluble cyanine dyes, it has been possible to use a single excitation wavelength of 635 nm. This greatly simplifies the experiments. The choice of a non-emissive standard is more problematic than one might think, but eventually was solved using Brilliant Green. This dye, which is a member of the triarylmethine family, has a well characterized viscosity-dependent, radiationless channel that serves to depopulate the excited-singlet state on a rapid timescale. In a non-viscous solvent such as methanol, Brilliant Green shows no significant fluorescence at ambient temperature.9
Experimental section
Background
The hardware requirements for a thermal blooming (also referred to as thermal lensing) apparatus, as well as the underlying theory for determining the absolute fluorescence quantum yield, have been outlined by Magde and co-workers.8,10,11 No commercial thermal blooming spectrometers exist, but the technique requires relatively few components, all of which are commercially available. A thermal blooming instrument must, as a minimum, have a cw laser with Gaussian mode TEM00 output at a wavelength appropriate for excitation of the chromophore, a suitable lens to expand the laser beam onto the detector, a digital optical shutter, a silicon photodiode detector and a digital oscilloscope for data collection. The set-up needs to be vibration-free and the laser excitation source needs to be properly screened.For a laser beam incident on a liquid sample, the output power, PL, will be the sum of three components according to eqn (1). Here, PT is the transmitted laser power, PF the emitted fluorescence, and PTH describes the amount of heat released into the system by way of nonradiative decay of the excited state. The thermal blooming experiment does not record fluorescence directly, but detects the radiationless processes described by PTH. The heat dissipated into the liquid solvent can be determined by accurately measuring the absorbance of the sample at the excitation wavelength and knowing the power of the excitation laser.
| PL = PT + PF + PTH | (1) |
In the thermal blooming experiment, fluorescence quantum yields are determined indirectly by recording the change in refractive index of the solvent as a result of brief exposure of the sample to laser excitation. It is essential that the solvent is transparent at the excitation wavelength. It is also important that, under illumination, the fluorophore does not react with the solvent, a valid assumption in the majority of cases. During excited-state decay, the combined nonradiative channels will equilibrate with the surrounding solvent by transferring excess heat. According to the nature of the solvent, heating will be manifest by a change in refractive index, which is seen as a “blooming” of the laser beam on a photodiode detector. The signal is recorded with a digital oscilloscope, and the output is analyzed in terms of eqn (2). The key variable is θ, which is a parameter directly proportional to the radiant thermal power. In eqn (2), I0 is the initial laser beam intensity and t is time. The value tc is a property of the solvent which characterizes thermal diffusion (related to thermal diffusivity) from the initial injection of heat to a steady-state level. Our experimental results, where methanol was the solvent of choice, tended to yield tc values of ca. 55 ms, largely consistent with reports in the literature.12
 | (2) |
By comparing the thermal blooming data collected for a non-fluorescent standard and for the compound of interest under identical conditions, it is reasonable to associate any change in optical properties to the solvent reservoir. Experimental traces can be analyzed in terms of eqn (2) to give a value for θ. Signal averaging is applied and numerous traces are used to construct a suitable database for averaging. With values recorded for both sample S and reference R, the fluorescence quantum yield, ΦF, can be obtained on the basis of eqn (3). Here, A is the relative number of photons absorbed at the excitation wavelength, νF is the mean wavenumber corresponding to the fluorescence maximum, and νex is the excitation energy in wavenumbers.
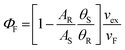 | (3) |
Methodology
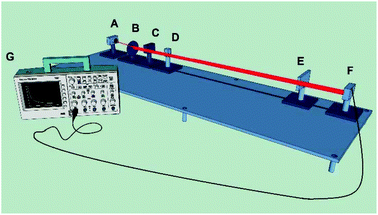
The experimental set-up for the thermal blooming measurements reported here (Fig. 1) comprises an aluminum optical table, 130 cm long, with slots and mounting points for all the main components. A laser diode (Coherent 3.0 mW; 635 nm; VHK Circular Beam Visible Laser Diode) provides the excitation source. A digital beam shutter (Thorlabs SC10) controls exposure of the sample to the incident laser beam. The signal is monitored with a silicon photodiode detector (Thorlabs PDA100A-EC). The detector was positioned sufficiently remote from the focussing lens to ensure that the expanding beam spot was larger than the surface area of the detector. Output was recorded with a Tektronix DPO71254 digital oscilloscope. The entire instrument is housed in a light-tight chamber.A plano-convex glass lens with a focal length of 10 cm was used to expand the laser beam. The shutter is placed between the lens and the sample. Liquid samples were measured in quartz cuvettes. The sample must be in a region where the waist of the beam is uniform through the path length. This is referred to as the Rayleigh distance and can be optimized by adjusting the distance between lens and sample until the maximum thermal blooming signal is observed.
Too much heat deposited in the solvent perturbs the thermal blooming signal due to aberrations and convection currents. In methanol, the upper limit for incident laser power before these detrimental effects become noticeable is 0.6 mW.10 For a given excitation source, the absorbed power must be kept below this threshold, either by adjusting the sample absorbance or attenuating the laser beam. As a benchmark, our set-up used liquid samples possessing an absorbance <0.1 (i.e. less than 20% of the incident laser power is absorbed by the sample) in a 1 cm pathlength cuvette.
Fig. 2 shows a typical output from a thermal blooming measurement and the general agreement of eqn (2) with the raw data. The protocol for fluorescence quantum yield measurements is as follows: a non-fluorescent standard, consisting of Brilliant Green in methanol, was measured against the fluorophore of interest after carefully matching the absorbance values at 635 nm. This absorbance was maintained in the range 0.07 to 0.10. The sample was contained in the same quartz cuvette (path length 1 cm) and kept in the same orientation to avoid any error arising from manufacturing differences in the quartz surfaces. The entire optical rail was isolated from stray light. Measurements were performed by opening the optical shutter for 0.5 s periods at regular intervals once a stable baseline had been attained. The oscilloscope collected the average signal across a pre-determined number of recordings. Data sampling was at a rate of 10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 counts per second. The photocurrent decay traces for both the standard and sample were analyzed according to eqn (2) to obtain the critical value θ. Finally, eqn (3) was used to determine the fluorescence yield. Each experiment was repeated at least 7 times. Absorption spectra were recorded with a Hitachi U-3310 spectrophotometer and fluorescence spectral measurements were made with a Horiba Fluorolog Tau-3 system.
000 counts per second. The photocurrent decay traces for both the standard and sample were analyzed according to eqn (2) to obtain the critical value θ. Finally, eqn (3) was used to determine the fluorescence yield. Each experiment was repeated at least 7 times. Absorption spectra were recorded with a Hitachi U-3310 spectrophotometer and fluorescence spectral measurements were made with a Horiba Fluorolog Tau-3 system.
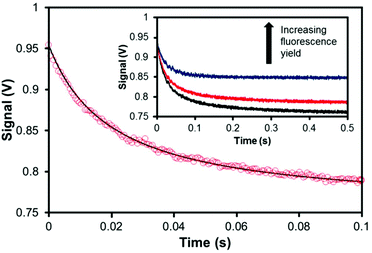 | ||
| Fig. 2 Typical oscilloscope output at early times for a thermal blooming measurement with a chromophore in liquid solution. Red circles: raw data. Black line: experimental fit according to eqn (2). Inset, thermal blooming measurement followed until a steady-state equilibration has been achieved. | ||
Errors and reproducibility
It must be emphasized that determining an absolute fluorescence quantum yield is a nontrivial task, despite advances in the underlying technology. The sources of errors in conventional fluorescence yield measurements have been well documented.13–15 With the thermal blooming technique, there are a number of potential sources of systematic error.8 Chief amongst these is the exact amount of laser power being transmitted through the sample. This requires an accurate measurement of the absorbance. As the fluorescence yield approaches zero, thermal blooming traces become increasingly similar to that of the non-fluorescent standard. In this situation the ratio θS/θR (eqn (3)) approaches unity and the main experimental error shifts to the measured absorbance. As such this technique is not well suited to measuring fluorescence quantum yields less than ca. 0.05. For very low quantum yields, minor variations in absorbance translate to major uncertainties in the recovered parameters, leading to overall errors in excess of ±5%.Conversely, thermal blooming can largely eliminate one of the key sources of error in a typical fluorescence measurement, namely minor contamination by highly emissive impurities. This is because thermal blooming does not measure emission and insignificant contributions to the overall absorbance made by trace impurities make no difference to the overall signal. Likewise, this technique is not affected by the inner-filter effect.16 Other pitfalls inherent to conventional fluorescence measurements, such as Raman and Rayleigh scattering, and the non-linear response of detectors and diffraction gratings can also be marginalized.
A key factor in the usefulness of the thermal blooming technique comes down to reproducibility of results. As a representative example, the fluorescence yield was measured for five samples of cy643 at different absorbance values within the range 0.05 to 0.1. Under carefully controlled conditions, the standard deviation of the recorded fluorescence yields was ±0.01 suggesting results are highly reproducible. Taking into account that the noise on a typical oscilloscope trace was found to be ±2% of the mean value, one can conclude that the accuracy of the measurement is well within ±5% for a reasonably emissive sample.
Materials
Methanol (Sigma-Aldrich) was spectroscopic grade and was used as received after confirming the absence of fluorescent impurities. Samples of the aza-BODIPY dyes were available from a previous study.17 Aluminium(III) phthalocyanine tetrasulfonate was a gift from Dr F. Hardy of The Procter & Gamble Co. According to elemental analyses, the sample is the tetrasulfonate derivative, although the positions of the sulfonate groups could not be determined. Samples of the cyanine dyes were obtained from FEW Chemicals GmbH and were used as received. Note NMR spectra are provided as part of the ESI.† A sample of Brilliant Green was obtained from Acros Organics and used without further purification after analysis by NMR spectroscopy. The sample displayed no significant fluorescence in methanol solution at room temperature. All other materials were obtained from Sigma-Aldrich. The molecular formulas for all compounds are provided in the ESI (Fig. S1 to S8†).Results
Calibration
To ensure the reliability of our thermal blooming apparatus, the fluorescence quantum yield (ΦF) of aluminium(III) phthalocyanine tetrasulfonate (AlPcS) in methanol was measured repeatedly over a four-month period. Excitation was made at 635 nm and a series of concentrations was used. The mean ΦF value was found to be 0.55 ± 0.02 (Table 1). This can be compared with 0.51 as reported by Beeby et al.18 A survey of the literature shows a general consensus for the AlPcS fluorescence yield falling between 0.51 and 0.55 in organic solvents,19,20 but dimerization in aqueous solution or at high concentration should be considered.21 The absorption spectrum of AlPcS (Fig. S1†) covers the wavelength range from 550 to 680 nm, with excellent absorption at the excitation wavelength. Fluorescence is readily observed in the red region (Fig. S1†). Using a conventional spectrophotometer (λEX = 610 nm), ΦF was found to be 0.54 ± 0.05 in methanol by comparison to free-base meso-tetraphenylporphyrin (TPP) in N,N-dimethylformamide (ΦF = 0.12).19 This set of results appears to be self-consistent and thereby confirm the validity of the thermal blooming set-up. Our sample of AlPcS gave a single-exponential decay profile, with an excited-state lifetime (τS) of 6.6 ± 0.2 ns, when excited at 635 nm under time-correlated, single photon counting conditions (Table 1). Overall, AlPcS is a promising fluorescence standard for excitation in the 580–640 nm spectral window.| Dye | Φ F | Method | Error |
τ
S![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) b/ns b/ns |
|---|---|---|---|---|
| a All measurements made in methanol at 20 °C. b Excited-singlet state lifetime measured by time-correlated, single photon counting. c Φ F taken from the literature. d Φ F measured in this work using conventional steady-state emission spectroscopy. e Φ F measured in this work by thermal blooming spectrometry. f Literature value in ethanol. | ||||
| AlPcS | 0.51 |
Ref. 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) c c |
±0.026 | 6.0 |
| 0.54 | SSFd | ±0.050 | 6.6 | |
| 0.55 | TBSe | ±0.016 | 6.6 | |
| aza-BOD-1 | 0.60f | Ref. 17 | ±0.030 | — |
| 0.56 | SSF | ±0.028 | 3.5 | |
| 0.58 | TBS | ±0.017 | 3.5 | |
| aza-BOD-2 | 0.33 | SSF | ±0.017 | 2.3 |
| 0.33 | TBS | ±0.017 | 2.3 | |
A further test of the experimental set-up was made using aza-BODIPY (aza-BOD-1) in methanol. The photophysical properties of this compound in methanol were established recently,17 where ΦF has a value of 0.56 and τS is 3.5 ns (Table 1). Thermal blooming studies in methanol gave rise to a ΦF value of 0.58 ± 0.02 (Table 1). This level of agreement is considered to be excellent. The same ΦF was recovered for different concentrations of aza-BODIPY and under various experimental configurations. The value, at least in methanol where the aza-nitrogen atom enters into hydrogen bonding with the solvent, is highly reproducible. Thus, aza-BODIPY seems to be a good secondary fluorescence standard for emission in the 650–730 nm window but it is not available commercially.
A second aza-BODIPY derivative was available, this differing only in terms of the peripheral substituents, but has not been reported in the literature. For this compound (aza-BOD-2) in methanol, the absorption and emission spectra closely resemble those recorded for aza-BOD-1. Conventional determination of the fluorescence yield using aza-BOD-1 as a reference gave a value of 0.33 (Table 1). The excited-state lifetime was found to be 2.3 ns, somewhat lower than that found for aza-BOD-1 under the same conditions. Thermal blooming studies allowed determination of the absolute fluorescence quantum yield for aza-BOD-2 as being 0.33 ± 0.02. This measurement is in excellent agreement with that made by ratiometric methods. It serves to emphasize the reliability of the thermal blooming technique and to extend the database of known emitters for the red region. The modest differences in fluorescence yield and lifetime found for these two aza-BODIPY emitters is explained in terms of enhanced nonradiative deactivation promoted by the O–H bond present in aza-BOD-2. This finding is precisely in line with expectations based on the Englman–Jortner energy-gap law.22
Cyanine dyes
Cyanine dyes, which were first synthesized over a century ago and used to sensitize photographic plates, comprise an inordinately diverse family of fluorophores. With their narrow absorption and fluorescence spectral profiles, together with high oscillator strengths for the lowest-energy absorption transition, these dyes are ideal candidates for fluorescence standards for the far-red region. To this effect, a series of five cyanine dyes was selected on the basis of covering the 600–800 nm absorption range by varying the conjugation length (Fig. 3). The target dyes are soluble in methanol and free from aggregation at the concentrations appropriate for this work.Their molecular formulas are provided in the ESI,† together with some basic spectroscopic information. For the two shorter conjugation lengths, cy643 and cy681, it was possible to determine ΦF values by ratiometric measurements using AlPcS and free-base meso-tetraphenylporphyrin as a reference. The values are collected in Table 2 and serve to indicate that these compounds are relatively strong emitters.
| Compound | λ ABS/nm | λ FLU/nm | ε MAX/M−1 cm−1 | τ S/ns | Φ F |
Φ
F![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) b b |
τ
SB![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) c/ns c/ns |
k
NR![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) d/107 s−1 d/107 s−1 |
|---|---|---|---|---|---|---|---|---|
| a Fluorescence quantum yield measured by conventional steady-state fluorescence spectroscopy. b Measured by thermal blooming. c Radiative lifetime measured as the inverse of the radiative rate constant determined from the Strickler–Berg expression. d Rate constant for nonradiative deactivation of the emitting state. | ||||||||
| cy643 | 643 | 668 | 218![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
0.95 | 0.41 | 0.43 | 1.44 | 60.0 ± 9.0 |
| cy681 | 681 | 706 | 166![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
0.87 | 0.24 | 0.24 | 1.10 | 87.6 ± 13.1 |
| cy746 | 746 | 774 | 258![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.20 | N/A | 0.20 | 0.68 | 66.8 ± 10.0 |
| cy761 | 760 | 782 | 312![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.00 | N/A | 0.18 | 0.72 | 81.5 ± 12.2 |
| cy794 | 794 | 817 | 246![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
0.98 | N/A | 0.056 | 0.24 | 96.0 ± 14.4 |
For the remaining cyanine dyes, it was not possible to generate meaningful ΦF values by conventional steady-state emission spectroscopy due to the lack of appropriate control compounds. Instead, the thermal blooming technique was successfully applied to this problem. Firstly, it was noted that ΦF values measured by the two techniques for cy643 and cy681 in methanol were within 5% of each other (Table 2). Fluorescence is not so pronounced for the other cyanine dyes but reliable quantum yields could be established by the thermal blooming technique under standardised conditions.
The measured ΦF values decrease steadily with increasing conjugation length (or decreasing emission energy) of the dye (Fig. 4), and fall from 0.43 for cy643 to 0.056 for cy794 (Table 2). Reproducibility remained high over multiple repeats and absolute errors were kept to within ±0.02, even for cy794 where ΦF is approaching the lower limit for this technique. It will be noted from Fig. 3 that the more red-shifted cyanine dyes are at the edge for excitation at 635 nm but nonetheless highly reproducible and reliable estimates of ΦF were forthcoming. A major benefit of the thermal blooming set-up relates to the absence of inner-filter effects, which would prevent the use of 635 nm excitation for conventional fluorescence measurements. The net result is that we now possess a useful set of fluorescence standards covering the far-red spectral range. Table 3 summarizes the wavelength ranges covered by each of the cyanine dyes and it should be noted that many more such dyes are available if needed to improve coverage of the spectral window.
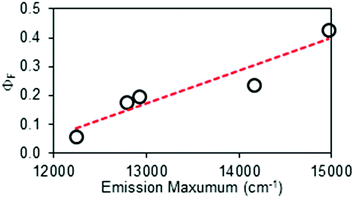 | ||
| Fig. 4 Correlation between the mean fluorescence spectral maximum and the fluorescence quantum yield measured for the cyanine dyes by thermal blooming. | ||
| Dye | Excitation range/nm | Emission range/nm |
|---|---|---|
| a These wavelength ranges should not be considered as being absolute but are intended to be representative of the spectral regions where particular dyes are most useful. | ||
| cy643 | 600–645 | 650–775 |
| cy681 | 640–680 | 680–820 |
| cy746 | 685–750 | 750–900 |
| cy761 | 700–760 | 750–900 |
| cy794 | 730–795 | 775–950 |
Common alternatives
Cyanine dyes are not the only compounds available as standards for the far-red spectral range but they have the advantage of forming a closely knit family where incremental changes in the optical properties can be achieved. Cresyl Violet23 is a popular fluorophore for the 630–700 nm window with excitation at ca. 560 nm but concerns have been raised about self-association,24 inner-filter effects25 and ΦF values that range from 0.54 to 0.70 in ethanol or methanol.10,11,26–29 Methylene Blue (ΦF = 0.02) is a well-known reference compound30 but the quantum yield is very low and the compound should be used only in water. A more suitable option might be Nile Blue.31 This dye, for which the purity of our commercial sample was <90% with notable contamination by coloured material, has λABS at 627 nm and λFLU at 665 nm. It is pH sensitive and has limited solubility in methanol but is workable over 550–650 nm for excitation and 650–750 nm for emission. After purification, we recorded ΦF values of 0.26 by steady-state fluorescence spectroscopy using TPP as reference and ΦF = 0.23 by thermal blooming. Literature data include ΦF values in ethanol of 0.23,26 by thermal phase grating, and 0.47,27 by thermal lensing. In our hands, fully reliable results were obtained only after addition of a trace amount of acid.Moving to lower energies, there is little real choice other than cyanine-based dyes. For example, Indocyanine Green has been used32 for many years in ophthalmology for imaging retinal blood vessels. This compound, which is a cyanine dye that aggregates extensively in solution,33 can be excited in the region around 750 nm to give fluorescence at wavelengths longer than 800 nm. It is widely used as a fluorescent label for tissue,34 during for example colorectal surgery where knowledge of quantum yields are not necessary. In methanol, the fluorescence quantum yield (ΦF = 0.043)35 is too low for many purposes.
Discussion
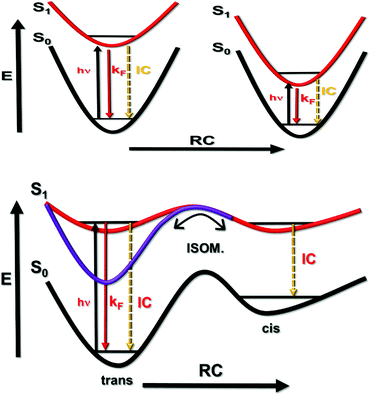
The concept of proposing cyanine dyes as fluorescence standards is not new5,28,35,36 and many such compounds are used in contemporary fluorescence microscopy as advanced probes.37 In this report, we have extended the library of useful far-red emitters by providing absolute fluorescence quantum yields for soluble dyes that emit within a spectral window covering the wavelength range 650–950 nm. It will be noted that the reported ΦF values decrease progressively as the emission maximum moves to lower energy (Fig. 4). Indeed, ΦF for the most red-shifted dye, cy794, is at the lower limit for accurate determination by thermal blooming. This finding suggests that cyanine dyes might not be appropriate standards for compounds that absorb at wavelengths longer than 800 nm. In our opinion, cyanine dyes are highly attractive reference compounds for excitation wavelengths stretching from 500 to 800 nm. A particular feature of these dyes concerns the sharp absorption profiles with pronounced shoulders on the high-energy side of the optical transition. This shoulder provides an ideal excitation point. We have not encountered any undue problems of poor stability or dye fatigue during prolonged spectroscopic studies.For the cyanine dyes described in this report, the Stokes’ shift falls within the range 350 to 580 cm−1, indicating only minor structural changes between absorbing and emitting species. The molar absorption coefficients at the peak maximum (εMAX) are high and possibly sensitive to the nature of the compound (Table 2). Nonetheless, the radiative rate constants calculated from the Strickler–Berg expression38 (kSB) evolve smoothly across the series, giving a clear correlation with the mean fluorescence maximum (νF) measured in terms of wavenumber. Somewhat surprisingly, the singlet-excited state lifetimes (τS) tend to remain at around 1 ns and do not follow the observed trend in ΦF (Table 2). There appears to be a crude relationship between the mean emission maximum and ΦF, as is illustrated by Fig. 4. This apparent trend is a result of the special circumstance where the rate constant (kNR) for nonradiative deactivation of the excited state is essentially balanced by opposing changes in the radiative rate constant (kRAD) across a series of emitters (Fig. 5). This situation is quite rare but arises here because of conflicting dependences on the relevant energy gap.22,39 Thus, the rate of internal conversion to the ground state is expected22 to increase with decreasing νF but the rate of isomerization from the excited-singlet state will decrease with decreasing νF due to a loss of potential energy.40 These two rate constants combine to give kNR and the indications are that, across this series of cyanine dyes, the latter is accidently fixed.
Conclusions
Combining thermal blooming with a series of structurally-related cyanine dyes, it has been possible to evaluate a set of commercially-available fluorophores as potential ratiometric standards for the 650–900 nm region. Cyanine dyes possess certain advantages as reference compounds, including ease of synthesis, ready availability, well-defined absorption and emission profiles that retain their spectral patterns in different environments, and strong fluorescence. Radiative rate constants tend to be high and poor spin–orbit coupling properties minimize intersystem crossing to the triplet manifold.40 Usually, cyanine dyes dissolve easily in alcohol solvents but much less so in water. Limited light fastness and competing light-induced isomerization are obvious drawbacks for the proposed application. The cyanine dyes studied here are not amenable for facile attachment to biological materials but strategies exist for appending appropriate anchoring groups to the backbone. In fact, cyanine dyes such as Alexa Fluor 647 are used extensively in super-resolution microscopy.41To establish absolute fluorescence quantum yields, we have re-introduced the technique known as photo-initiated thermal blooming.8 The ready availability of suitable laser diode modules means that it is feasible to construct a working prototype from relatively inexpensive components. Advantages of this approach include ease of operation, rapid screening of samples, and minimisation of inner-filter effects. To become popular, however, the technique needs diversifying to include solid samples, such as thin films, and operating devices, such as OLEDs. Preliminary work suggests such applications are possible with minor modifications to the basic set-up.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
We thank Newcastle University and EPSRC (Industrial CASE Award) for financial support of this work. We also thank Dr N. R. Walker (Newcastle University) for the loan of the Tektronix DPO71254 oscilloscope, Dr M. J. Hall (Newcastle University) for donating samples of aza-BODIPY dyes and Dr Corinne Wills and Prof. William McFarlane for recording the NMR spectra. Finally, we thank John Corner and Gary Day of the School of Chemistry Mechanical Workshop for help constructing the thermal blooming instrument.Notes and references
- J. R. Lakowicz, Radiative decay engineering: Biophysical and biomedical applications, Anal. Biochem., 2001, 298, 1–24 CrossRef CAS PubMed.
- K. Kolmakov, V. N. Belov, J. Bierwagen, C. Ringemann, V. Müller, C. Eggeling and S. W. Hell, Red emitting rhodamine dyes for fluorescence microscopy and nanoscopy, Chem. – Eur. J., 2010, 16, 158–166 CrossRef CAS PubMed.
- L. Yuan, W. Lin, K. Zheng, L. He and W. Huang, Far-red to near infrared analyte-responsive fluorescent probes based on organic fluorophore platforms for fluorescence imaging, Chem. Soc. Rev., 2013, 42, 622–661 RSC.
- A. M. Brouwer, Standards for photoluminescence quantum yield measurements in solution, Pure Appl. Chem., 2011, 83, 2213–2228 CrossRef CAS.
- R. C. Benson and H. A. Kues, Absorption and fluorescence properties of cyanine dyes, J. Chem. Eng. Data, 1977, 22, 379–383 CrossRef CAS.
- L. Porres, A. Holland, L. O. Pålsson, A. P. Monkman, C. Kemp and A. Beeby, Absolute measurements of photoluminescence quantum yields of solutions using an integrating sphere, J. Fluoresc., 2006, 16, 267–273 CrossRef CAS PubMed.
- A. Lampe, V. Haucke, S. J. Sigrist, M. Heilemann and J. Schmoranzer, Multicolour direct STORM with red emitting carbocyanines, Biol. Cell, 2012, 104, 229–237 CrossRef CAS PubMed.
- J. H. Brannon and D. Magde, Absolute quantum yield determination by thermal blooming, J. Phys. Chem., 1978, 82, 705–709 CrossRef CAS.
- Y. Nagasawa, Y. Ando, D. Kataoka, H. Matsuda, H. Miyasaka and T. Okada, Ultrafast excited state deactivation of triphenylmethane dyes, J. Phys. Chem. A, 2002, 106, 2024–2035 CrossRef CAS.
- D. Magde, R. Wong and P. G. Seybold, Fluorescent quantum yields and their relation to lifetimes of rhodamine 6G and fluorescein in nine solvents: Improved absolute standards for quantum yields, Photochem. Photobiol., 2002, 75, 327–334 CrossRef CAS PubMed.
- D. Magde, J. H. Brannon, T. L. Cremers and J. Olmsted, Absolute luminescence yield of cresyl violet, a standard for the red, J. Phys. Chem., 1979, 83, 696–699 CrossRef CAS.
- C. V. Bindhu, S. S. Harilal, V. P. N. Nampoori and C. P. G. Vallabhan, Thermal diffusivity measurments in organic liquids using transient lens calorimetry, Opt. Eng., 1998, 37, 2791–2794 CrossRef CAS.
- J. N. Demas and G. A. Crosby, Measurement of photoluminescent quantum yields. A review, J. Phys. Chem., 1971, 75, 991–1024 CrossRef CAS; T. Karstens and K. Koba, Rhodamine B and rhodamine 101 as reference substances for fluorescent quantum yield measurements, J. Phys. Chem., 1980, 84, 1871–1872 CrossRef.
- D. F. Eaton, Reference materials for fluorescence measurement, Pure Appl. Chem., 1988, 60, 1107–1114 CrossRef CAS; C. Würth, M. Grabolle, J. Pauli, M. Spieles and U. Resch-Genger, Relative and absolute determination of fluorescence quantum yields of transparent samples, Nat. Protoc., 2013, 8, 1535–1550 CrossRef PubMed.
- S. Fery-Forgues and D. Lavabre, Are fluorescence quantum yields so tricky to measure? A demonstration using familiar stationery products, J. Chem. Educ., 1999, 76, 1260–1264 CrossRef CAS.
- M. Fischer and J. Georges, Fluorescence quantum yield of rhodamine 6G in ethanol as a function of concentration using thermal lens spectroscopy, Chem. Phys. Lett., 1996, 260, 115–118 CrossRef CAS.
- J. K. Karlsson and A. Harriman, Origin of the red shifted optical spectra recorded for aza-BODIPY Dyes, J. Phys. Chem. A, 2016, 120, 2537–2546 CrossRef CAS PubMed.
- M. Ambroz, A. Beeby, A. J. MacRobert, R. K. Svensen and D. Phillips, Preparative, analytical and fluorescence spectroscopic studies of sulphonated aluminium phthalocyanine photosensitisers, J. Photochem. Photobiol., B, 1991, 9, 87–95 CrossRef CAS.
- J. W. Owens, R. Smith, R. Robinson and M. Robins, Photophysical properties of porphyrins, phthalocyanines and benzochlorins, Inorg. Chim. Acta, 1998, 279, 226–231 CrossRef CAS.
- J. W. Owens and M. Robins, Phthalocyanine photophysics and photosensitizer efficiency on human embryonic lung fibroplasts, J. Porphyrins Phthalocyanines, 2001, 5, 460–464 CrossRef CAS.
- S. Dhami, A. J. De Mello, G. Rumbles, S. M. Bishop, D. Phillips and A. Beeby, Phthalocyanine fluorescence at high concentration: Dimers or reabsorption effect?, Photochem. Photobiol., 1995, 61, 341–346 CrossRef CAS.
- R. Englman and J. Jortner, The energy gap law for radiationless transitions in large molecules, Mol. Phys., 1970, 18, 145–172 CrossRef CAS.
- A. Alvarez-Buylla, C.-Y. Ling and J. R. Kirn, Cresyl violet: A red fluorescent nissl stain, J. Neurosci. Methods, 1990, 33, 129–133 CrossRef CAS PubMed.
- Y. Sakai, M. Kawahigashi, T. Minami, T. Inoue and S. Hirayama, Deconvolution of non-exponential decays arising from reabsorption of emitted Light, J. Lumin., 1989, 42, 317–324 CrossRef CAS; G. Zhang and W. Chen, Fluorescence spectroscopic studies on intermolecular energy transfer of RH6G-cresyl violet mixture solution, Acta Phys.-Chim. Sin., 1990, 6, 163–168 Search PubMed.
- S. J. Isak and E. M. Eyring, Fluorescence quantum yield of cresyl violet in methanol and water as a function of concentration, J. Phys. Chem., 1992, 96, 1738–1742 CrossRef CAS.
- V. A. Petukhov, M. B. Popov and A. I. Krymova, Determination of the quantum efficiency of the fluorescence of the solutions of organic dyes by diffraction on a thermal phase grating induced by laser radiation, Kvantovaya Elektron., 1986, 13, 777–786 CAS.
- T. Wang, B. Q. Zhang, J. L. Pan, P. Gu, Q. Xu and Q. Sun, Determination of absolute fluorescence quantum yield of laser dyes by thermal lens calorimetry, Chin. Sci. Bull., 1989, 34, 1756–1757 CAS.
- J. Olmsted, Calorimetric Determinations of absolute fluorescence quantum yields, J. Phys. Chem., 1979, 83, 2581–2584 CrossRef CAS.
- K. H. Drexhage, What's ahead in laser dyes, Laser Focus, 1973, 35–39 Search PubMed; K. H. Drexhage, Fluorescence efficiency of laser dyes, J. Res. NBS A Phys. Chem., 1976, 80, 421–428 CrossRef.
- S. J. Atherton and A. Harriman, Photochemistry of intercalated methylene blue: Photoinduced hydrogen atom abstraction from guanine and adenine, J. Am. Chem. Soc., 1993, 115, 1816–1822 CrossRef CAS.
- A. Douhal, Photophysics of nile blue in proton-accepting and electron-donating solvents, J. Phys. Chem., 1994, 98, 13131–13137 CrossRef CAS.
- J. T. Alander, I. Kaartinen, A. Laakso, T. Pätilä, T. Spillmann, V. V. Turchin, M. Venermo and P. Välisuo, A review of indocyanine green fluorescence imaging in surgery, Int. J. Biomed. Imaging, 2012, 940585 Search PubMed.
- M. L. Landsman, G. Kwant, G. A. Mook and W. G. Zijlstra, Light-absorbing properties, stability and spectral stabilization of indocyanine green, J. Appl. Physiol., 1976, 40, 575–583 Search PubMed; B. Yuan, N.-G. Chen and Q. Zhu, Emission and absorption properties of indocyanine green in intralipid solution, J. Biomed. Opt., 2004, 9, 497–503 CrossRef CAS PubMed.
- T. Desmettre, J. M. Devoisselle and S. Mordon, Fluorescent properties and metabolic features of indocyanine green (ICG) as related to angiography, Surv. Ophthalmol., 2000, 45, 15–27 CrossRef CAS PubMed.
- R. Philip, A. Penzkofer, W. Bäumler, R. M. Szeimies and C. Abels, Absorption and fluorescent spectroscopic investigation of indocyanine green, J. Photochem. Photobiol., A, 1996, 96, 137–148 CrossRef CAS.
- K. Rurack and M. Spieles, Fluorescence quantum yields of a series of red and near infrared dyes emitting at 600–1000 nm, Anal. Chem., 2011, 83, 1232–1242 CrossRef CAS PubMed.
- J. C. Vaughan, G. T. Dempsey, E. Sun and X. Zhuang, Phosphine quenching of cyanine dyes as a versatile tool for fluorescence microscopy, J. Am. Chem. Soc., 2013, 125, 1197–1200 CrossRef PubMed.
- N. Panchuk-Voloshina, R. P. Haugland, J. Bishop-Stewart, M. K. Bhalgat, P. J. Millard, F. Mao, W. Y. Leung and R. P. Haugland, Alexa dyes, a series of new fluorescent dyes that yield exceptionally bright, photostable conjugates, J. Histochem. Cytochem., 1999, 47, 1179–1188 CrossRef CAS PubMed.
- S. J. Strickler and R. A. Berg, Relationship between absorption intensity and fluorescence lifetime of molecules, J. Chem. Phys., 1962, 37, 814–822 CrossRef CAS.
- A. Harriman, (Photo)isomerization dynamics of merocyanine dyes in solution, J. Photochem. Photobiol., A, 1992, 65, 79–93 CrossRef CAS.
- M. Bates, B. Huang, G. T. Dempsey and X. Zhuang, Multicolour super resolution imaging with photoswitchable fluorescent probes, Science, 2007, 317, 1749–1753 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available: Individual molecular formulae, absorption/fluorescence spectra and Beer–Lambert plots, analytical data and additional details. See DOI: 10.1039/c7pp00333a |
| This journal is © The Royal Society of Chemistry and Owner Societies 2018 |