DOI:
10.1039/C6RA16481A
(Paper)
RSC Adv., 2016,
6, 95644-95655
Strychnos nuxvomica, Piper longum and Mucuna pruriens seed extracts as eco-friendly corrosion inhibitors for copper in nitric acid
Received
26th June 2016
, Accepted 25th September 2016
First published on 26th September 2016
Abstract
The inhibitive effect of the Strychnos nuxvomica (SN), Piper longum (PL) and Mucuna pruriens (MP) seeds extract on the corrosion of copper in 3 M HNO3 solution was studied using gravimetric, potentiodynamic polarization and electrochemical impedance spectroscopic (EIS) studies. Inhibition efficiency increased with increasing concentration of the extract and maximum inhibition efficiency was 91.60%, 80.00% and 71.6% obtained from gravimetric studies at 0.2 g L−1 of SN, PL and MP respectively. Above this concentration no noticeable change was observed. The adsorption of the inhibitor on copper surface was in accordance with the Langmuir adsorption isotherm. Potentiodynamic polarization study showed predominantly cathodic type inhibition. SEM and AFM study was carried out to support the inhibition data.
1. Introduction
The protection of metallic objects against corrosion is a major industrial problem. The use of inhibitors is one of the best options for protecting metals against corrosion in acidic media. Acid solutions are used in many industries for chemical cleaning, pickling, etc. and thus the chances of copper corrosion are particularly high in such industrial processes.1–3 Organic compounds, especially those with N, O and S atoms4,5 have frequently been used to prevent corrosion in acid solution. Although most of these compounds are not expensive, they are toxic for living beings and very hazardous to the environment.
Therefore, the present trend in research on eco-friendly corrosion inhibitors has been concentrating on products of natural origin owing to their low cast and biodegradability. Plant extracts have become important as inexpensive, non toxic and renewable sources of wide range of corrosion inhibitors. The yield of these natural products as well as the corrosion inhibition abilities of plant extracts highly depends on the part of the plant and the location in which it is found. The different parts of plant like seeds,6–9 leaves,10–12 flowers,13–16 and fruits17 had been extracted and used as corrosion inhibitors.
An inhibition efficiency of 90.7% has earlier been reported with 0.1 g L−1 of Myrtus comminis (seed oil) for copper in sulfuric acid at 298 K by Mansoor et al.18 The peel extract of Gnetum fricana and Musa acuminate exhibit 80.5 and 76.1% inhibition efficiencies at 3 g L−1 on the corrosion of copper in HNO3 solution at 303 K.19 The effect of fruit extract of Gingko biloba on corrosion inhibition of J55 steel in 3.5% NaCl solution saturated with CO2 was studied and the maximum efficiency was reported to be 95% at 1000 ppm by polarization measurements.20 The inhibition efficiencies as high as 91.0 and 65.3% at optimum concentration (500 ppm) of Zygophllum coccineum L. extract on the corrosion of copper in 1 M HNO3 was reported at 298 and 318 K, respectively.21 Earlier Vitex nigundo, Adhatoda vasica and Saraca, asoka leave extracts was successfully used as inhibitor of copper corrosion in nitric acid.22
In the present investigation, seed extract of Strychnos nuxvomica (SN), Piper longum (PL) and Mucuna pruriens (MP) have been used for the study on copper corrosion inhibition in nitric acid. The inhibition study was conducted by gravimetric, potentiodynamic polarization and electrochemical impedance spectroscopy measurements. The experimental inhibition results were complemented with UV-Visible spectroscopy, SEM and AFM investigation.
2. Experimental
2.1. Coupon preparation
The coupons of size 2 × 2 × 0.1 cm and 3 × 1 × 0.1 cm cut from copper were used for gravimetric and electrochemical measurements, respectively. The specimens were mechanically abraded with 600, 1000, 1500 and 2000 grade of emery papers, washed successively with double distilled water and acetone. The samples were dried at room temperature in a desiccator then used for the experiments.
2.2. Electrolytic solution
The corrosive solution 3 M HNO3 was prepared by dilution of analytical grade HNO3 of predetermined normality with double distilled water. The concentration range of the extract used was 0.050–0.20 g L−1 and the volume of electrolyte used was 150 mL for each experiment.
2.3. Inhibitor
Strychnos nuxvomica (SN), Piper longum (PL) and Mucuna pruriens (MP) seeds were dried and ground to powder form. 10 g of the powder was soaked for 24 h in 500 mL double distilled water and then refluxed in a round bottom flask for 4 h. After that, it was filtered and the resulting filtrate was concentrated to 100 mL by evaporation using rotavapor at temperature 328 K. The obtained extract was used for the measurements of inhibitive properties. The residue was air dried and weighed. The amount of extract in the filtrate was determined by subtracting the weight of the residue obtained from the total weight of the seeds taken.
A plant extract is a complex mixture of various phytochemical compounds and the major component present in the extracts of SN, PL and MP are brucine,23 piperine24 and L-DOPA,25 respectively. There are a number of minor constituents present in each of the three extracts. Some of them may have synergistic effects while others may have antagonistic effects. However, in the present case only major constituents are being considered as the species mainly responsible for corrosion inhibition and their structures are given in Table 1.
Table 1 Name and molecular structure of active constituents present in seed extracts
| Leaf extract |
Major component |
Structure |
| SN |
Brucine |
 |
| PL |
Piperine |
 |
| MP |
L-DOPA |
 |
2.4. Gravimetric measurement
The abraded Cu specimens of size 2 × 2 × 0.1 cm were weighed on a balance with 1 mg sensitivity and immersed for 4 h in 3 M HNO3 in absence and the presence of 0.05, 0.08, 0.1 and 0.2 g L−1 at 308 K. A hole was made in the upper corner of the sample and was hanged in the electrolyte by tying it with the help of a thread. Study of temperature effect on inhibition efficiency was performed in the temperature range from 308 to 323 K for the highest concentration of inhibitor (0.20 g L−1). To compensate the volume change due to addition of inhibitor, volume correction was done while preparing 3 M HNO3 acid. The corrosion rate (CR) in mg cm−2 h−1 was calculated from the following equation| |
 | (1) |
where, ΔW is the average weight loss, S the total area of the specimen and t is the immersion time. From the corrosion rate thus obtained, the inhibition efficiency (η%) was calculated as below:| |
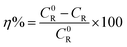 | (2) |
where, C0 and CR are the corrosion rates of copper in the absence and presence of inhibitors, respectively.
2.5. Electrochemical measurements
Electrochemical tests were carried out in a conventional three electrode cell consisting of a flat bottom Pyrex glass flask with three inlets for working, reference and counter electrodes. A rectangular size working electrode of copper metal with an exposed surface area of 1 cm2 was attached to a self-designed holder and the rest of the surface was covered with lacquer. Silver–silver chloride electrode (3 M KCl) and platinum wire were used as a reference and counter electrodes, respectively. The working electrode was first immersed in test solution for establishing a steady state open circuit potential (OCP) and then the cathodic and anodic polarization studies with repeated to OCP were conducted using electrochemical analyzer (CHI604C).
Linear polarization resistance (LPR) measurements were potentiodynamically performed in the potential range +10 to −10 mV on both sides of OCP at a scan rate of 0.01 mV s−1. Polarization resistance (RP) values were obtained from slope of the resulting linear plot between current density and potential plot. From the measured RP values, the η% has been calculated using the relation.
| |
 | (3) |
where,
R0P and
RP are the polarization resistance values in absence and the presence of inhibitor.
In potentiodynamic polarization study the potential was scanned from −250 to +250 mV with respect to OCP at a rate of 0.5 mV s−1. The inhibition efficiency has been calculated by substituting respective corrosion current densities (i0corr and icorr) in place of corrosion rates (C0R and CR) in eqn (2).
AC impedance measurements were carried out using signals of 5 mV amplitude in the frequency range from 100 kHz to 0.01 Hz after 4 h of immersion. The obtained impedance data were analyzed with Zsimpwin software. The charge transfer resistance (Rct), was obtained from the diameter of the semicircle of the Nyquist plot. The inhibition efficiencies of these inhibitors have been determined by replacing RP and R0P with Rct and R0ct respectively, in eqn (3).
2.6. Spectral analysis
The composition of electrolyte after corrosion inhibition study at different time intervals from 4 to 24 h was determined by the UV spectroscopy. UV-Visible spectra were recorded in the range 200–1100 nm on a Shimadzu spectrophotometer, Pharmaspec. UV-1700 model.
2.7. Density functional theory (DFT) study
Quantum chemical calculations were performed using DFT method and structural parameters were geometrically optimized using functional hybrid RB3LYP with electron basis set DGDZVP for all atoms. All the calculations were performed with Gaussian 03, E.01 as reported in literature.26 The quantum chemical parameters obtained were EHOMO, ELUMO, ΔE (ELUMO − EHOMO), I (ionization energy), A (electron affinity), μ (dipole moment) and ΔN (fraction of electron transferred).
2.8. Surface analysis
The surface morphology of the inhibited and uninhibited copper surfaces in 3 M HNO3 was analyzed by scanning electron microscope. An electron microscope, FEI Qunanta 200f, was used for Scanning Electron Microscopy (SEM). Further characterization of the corroded and inhibited copper surface in term of roughness was done by Atomic Force Microscopy. The experiments were performed by contact mode AFM (Model no. BT 02218, nanosurfeasyscan 2 basic AFM, (Switzerland)) supported by Si3N4 cantilever (Nanosensor, CONTR Type) having spring constant of 0.1 Nm−1 and tip radius more than 10 nm. The measurements were done over a square area with an image size of 50.0 μm. The resolution was 256 points per line; the image were obtained at scan forward mode and scanning was carried out from down to up in static regime.
3. Results and discussion
3.1. Gravimetric measurements
3.1.1. Effect of inhibitor concentration. The corrosion inhibition studies of copper in 3 M HNO3 solution at 308 K containing 0.05, 0.08, 0.1 and 0.2 g L−1 of the SN, PL and MP seed extracts have been conducted using weight loss measurements and the data obtained after 4 h of immersion time are recorded in Table 2. It is apparent from the data that weight loss is substantially decreased on the addition of inhibitors and on increasing its concentration there is an exponential decrease in the corrosion rate. The inhibition efficiency with different concentration of three seeds is illustrated in Fig. 1a. It can be seen from the figure that gradually increased inhibitor concentration increases the percentage inhibition efficiency (η%). The inhibitive action of the inhibitor is due to the presence of hetero atoms such as oxygen, nitrogen and aromatic rings with pi bonds in their constituents, which act as active centers for adsorption and create a protective film on metal surface. The maximum inhibition efficiency (91.6%) was obtained for SN at 0.2 g L−1. The orders of inhibition efficiency are as follows:
| SN (91.6%) > PL (80.0%) > MP (71.6%) |
Table 2 Corrosion parameters for copper in 3 M HNO3 in absence and presence of different concentrations (0.05–0.2 g L−1) of SN, PL and MP gravimetric measurement at 308 K for 4 h
| Inhibitor |
Concentration (g L−1) |
Weight loss (mg cm−2 h−1) |
CR (mg cm−2 h−1) |
η (%) |
| Blank SN |
— |
60 |
1.666 |
— |
| 0.05 |
21.0 |
0.583 |
65.0 |
| 0.08 |
18.0 |
0.500 |
70.0 |
| 0.1 |
15.0 |
0.416 |
75.0 |
| 0.2 |
5.0 |
0.138 |
91.6 |
| 0.3 |
5.3 |
0.147 |
91.2 |
| 0.4 |
5.1 |
0.141 |
91.5 |
| PL |
0.05 |
23.0 |
0.638 |
61.6 |
| 0.08 |
19.0 |
0.527 |
68.3 |
| 0.1 |
16.0 |
0.444 |
73.3 |
| 0.2 |
12.0 |
0.333 |
80.0 |
| 0.3 |
12.0 |
0.333 |
80.0 |
| 0.4 |
12.3 |
0.341 |
79.5 |
| MP |
0.05 |
26.0 |
0.722 |
56.6 |
| 0.08 |
23.0 |
0.638 |
61.6 |
| 0.1 |
19.0 |
0.527 |
68.3 |
| 0.2 |
17.0 |
0.472 |
71.6 |
| 0.3 |
17.6 |
0.488 |
70.6 |
| 0.4 |
17.0 |
0.472 |
71.6 |
 |
| | Fig. 1 Variation of inhibition efficiency (η%) of seed extract for copper in 3 M HNO3 (a) with concentration and (b) with immersion time. | |
The variation of inhibition efficiency with immersion time at optimum concentration (0.2 g L−1) of SN, PL and MP at 308 K is shown in Fig. 1b. It is found that the inhibition efficiency decreases with increase in immersion time from 4 to 24 h in all the cases of three seed extracts.
3.1.2. Effect of temperature. To evaluate the stability of adsorbed layer/film of inhibitor on copper surface as well as activation parameters of the corrosion process of copper in acidic medium, weight loss measurements were carried out in the range of temperature 308–323 K in the absence and presence of extracts at their optimum concentration (0.20 g L−1). The results obtained are listed in Table 3. It is evident from this table that corrosion rate increases in the absence as well as the presence of inhibitor with the increases in temperature. Thus, because of the increase in corrosion rate at higher temperatures the inhibition efficiency decreases with temperature.27 The inhibition efficiency decreases from 92–75%, 80–65% and 78–48% for three inhibitors. The decreases in inhibition efficiency is due to physisorption of inhibitor where in the desorption process of inhibitor molecules is enhanced at higher temperatures.28 It is further noted that the extent to which the corrosion rate increases with temperature is much larger than the extent to which η% decreases, thus, it can be argued that a large part of the film still remains intact even at higher temperatures.
Table 3 Various corrosion parameters for copper in 3 M HNO3 in absence and presence of highest concentration (0.2 g L−1) of SN, PL and MP at different temperature
| Inhibitor |
Temperature (K) |
CR (mg cm−2 h−1) |
θ |
η (%) |
| Blank |
308 |
1.66 |
— |
— |
| 313 |
2.38 |
— |
— |
| 318 |
3.36 |
— |
— |
| 323 |
4.58 |
— |
— |
| SN |
308 |
0.138 |
0.92 |
92 |
| 313 |
0.222 |
0.89 |
89 |
| 318 |
0.555 |
0.83 |
83 |
| 323 |
1.138 |
0.75 |
75 |
| PL |
308 |
0.333 |
0.80 |
80 |
| 313 |
0.583 |
0.75 |
75 |
| 318 |
0.972 |
0.71 |
71 |
| 323 |
1.583 |
0.65 |
65 |
| MP |
308 |
0.500 |
0.78 |
78 |
| 313 |
0.861 |
0.71 |
71 |
| 318 |
1.361 |
0.68 |
68 |
| 323 |
2.194 |
0.48 |
48 |
The apparent activation energy for corrosion reaction of copper in 3 M HNO3 is calculated using Arrhenius equation:
| |
 | (4) |
where,
Ea is the apparent activation energy,
A is the Arrhenius pre-exponential factor and
R is the universal gas constant. The
Ea values were determined by linear regression between log
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR
CR and 1/
T Fig. 2. The
Ea value in absence of inhibitor is 56.13 kJ mol
−1 and in the presence of SN, PL and MP, these values are 120.05 kJ mol
−1, 86.16 kJ mol
−1 and 81.18 kJ mol
−1 respectively. Higher
Ea values for inhibitor molecules indicate retardation in mass/charge transfer from the surface to the electrolyte due to surface barrier.
29
 |
| | Fig. 2 Arrhenius plots of log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR versus 1/T at optimum concentration (0.2 g L−1) of SN, PL and MP. CR versus 1/T at optimum concentration (0.2 g L−1) of SN, PL and MP. | |
The values of the enthalpy of activation (ΔH0a) and entropy of activation (ΔS0a) were calculated using the following equation.30
| |
 | (5) |
A plot of log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR/T verses 1/T shown in Fig. 3 gave a straight line with a slope of ΔHa/2.303R and an intercept of log(R/Nh) + ΔSa/2.303. The values of ΔH0a and ΔS0a were calculated from three parameters and are listed in Table 4. The standard enthalpy of activation energy ΔH0a showed similar trend like the activation energy values. The entropy of activation ΔS0a values is negative in the absence and positive in presence of inhibitors. The negative ΔS0a value indicates the activated complex is more ordered in the blank solution. The positive values of ΔS0a suggest that an increase in disordering takes place in going from reactants to the activated complex on metal/solution interface22 which is driving force for the adsorption.
CR/T verses 1/T shown in Fig. 3 gave a straight line with a slope of ΔHa/2.303R and an intercept of log(R/Nh) + ΔSa/2.303. The values of ΔH0a and ΔS0a were calculated from three parameters and are listed in Table 4. The standard enthalpy of activation energy ΔH0a showed similar trend like the activation energy values. The entropy of activation ΔS0a values is negative in the absence and positive in presence of inhibitors. The negative ΔS0a value indicates the activated complex is more ordered in the blank solution. The positive values of ΔS0a suggest that an increase in disordering takes place in going from reactants to the activated complex on metal/solution interface22 which is driving force for the adsorption.
 |
| | Fig. 3 Arrhenius plots of log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR/T versus 1/T at optimum concentration (0.2 g L−1) of SN, PL and MP. CR/T versus 1/T at optimum concentration (0.2 g L−1) of SN, PL and MP. | |
Table 4 Activation parameters Ea, ΔH0a and ΔS0a for the copper dissolution in 3 M HNO3 in the absence and presence of SN, PL and MP at highest concentration
| Inhibitor (g L−1) |
Linear regression coefficient (R2) |
Ea (kJ mol−1) |
ΔH0a (kJ mol−1) |
ΔS0a (J mol−1 K−1) |
| Blank |
0.999 |
56.13 |
53.45 |
−67.69 |
| SN |
0.999 |
120.05 |
117.56 |
118.48 |
| PL |
0.998 |
86.16 |
83.48 |
16.75 |
| MP |
0.999 |
81.18 |
78.80 |
4.51 |
3.2. Electrochemical measurements
3.2.1. Effect of inhibitor on polarization behavior. The effects of seed extracts at highest concentration on the anodic and cathodic polarization behavior of copper in 3 M HNO3 solution are shown in Fig. 4. The visual examination of polarization curves depicted in the figure reveals that the cathodic curves are shifted towards lower current density side with increase in inhibitor concentration while the shape remains unchanged. However, in case of anodic polarization there is little deviation in the curves. This indicates that the inhibitor affects the rate of cathodic process more prominently than anodic process.
 |
| | Fig. 4 Potentiodynamic polarization behavior of copper in 3 M HNO3 with the addition of optimum concentration of SN, PL and MP at 308 K. | |
HNO3 is a strong oxidizer capable of attacking copper. The potentiodynamic polarization curves exhibit no steep slope in the anodic range which proves that no passive films are formed on the copper surface. The Pourbaix diagram for copper–water system31,32 is drawn in Fig. 5. It shows that copper is corroded to Cu2+ in HNO3 solutions and no oxide film is formed to protect the surface from the attack of the corrosive medium. Copper dissolution is thus expected to be the dominant reaction in HNO3 solution. The electrochemical reactions33 for copper in HNO3 solution can be described as following equation:
 |
| | Fig. 5 Potential–pH equilibrium diagram for the system, copper–water, at 25 ± 1 °C. | |
Anodic reaction:
Cathodic reaction:
| | |
NO3− + 3H+ + 2e− → HNO2 + H2O
| (7) |
| | |
NO3− + 4H+ + 3e− → NO + 2H2O
| (8) |
| | |
O2 + 4H+ + 4e− → 2H2O
| (9) |
The corrosion parameters, viz., corrosion potential (Ecorr), current density (icorr), anodic Tafel slope (βa), cathodic Tafel slope (βc), polarization resistance (RP) and inhibition efficiency (η%) calculated from polarization curves are presented in Table 5. It is observed from the table that icorr is lowered on the addition of inhibitors indicating their retarding effect. The minimum value of icorr is obtained for SN followed by PL and MP. It is further noted from the table that the values of Ecorr shifts slightly towards more negative direction for all the inhibitors. The shift in Ecorr values was lower than 85 mV in each case suggesting that these extracts are mixed type inhibitors.30 In present study the displacement in the range (51–70 mV) of Ecorr due to the presence of these inhibitors suggests that all the inhibitors are predominantly cathodic mixed inhibitor. The values of βa in the presence of inhibitor did not show any significant change with respect to those observed in the blank solution. However, βc values increase drastically in the presence of inhibitor further indicating retardation in cathodic process. Thus, these extracts behave as mixed inhibitors and are predominantly cathodic. The values of RP obtained from LPR studies are listed in Table 5. It can be noted that RP increased on the addition inhibitors which increased further on increasing their concentrations. Apparently, all the extracts retard copper corrosion and their inhibition efficiency regularly increases with increase in concentration.
Table 5 Electrochemical parameters obtained by Tafel polarization for copper in 3 M HNO3 solution in without and with of SN, PL and MP at 308 K
| Inhibitor (g L−1) |
Ecorr (mV) |
icorr (μA cm−2) |
βa (mV dec−1) |
−βc (mV dec−1) |
η% |
RP (Ω cm2) |
η% |
| Blank |
−31 |
1305 |
96.1 |
307.3 |
— |
31.1 |
— |
| SN |
−70 |
121.9 |
66.2 |
269.1 |
90.6 |
298.3 |
89.2 |
| PL |
−58 |
276.5 |
78.6 |
289.7 |
78.7 |
158.7 |
80.4 |
| MP |
−51 |
395.1 |
95.3 |
302.6 |
69.7 |
101.3 |
69.2 |
3.2.2. Effect of inhibitor on electrochemical impedance. Corrosion behaviour of copper in nitric acid solution in the absence and presence of optimum concentrations of SN, PL, and MP was investigated by EIS at 308 K, and corresponding Nyquist plots are shown in Fig. 6. It is observed that the Nyquist plots consist of capacitive loop in high frequency region and a straight line in low frequency region. The slightly depressed capacitive loop which has the centre below the real axis depends on the roughness and other inhomogeneity of copper electrode surface. The straight line, representing the Warburg impedance, is attributed to diffusion from the surface. The addition of all three extracts leads to change of the Nyquist plot both in shape and size. The size of capacitive loop at a fixed optimized inhibitor concentration, in the sequence: SN > PL > MP, is in the conformity with the highest inhibitive influence of SN.
 |
| | Fig. 6 Impedance diagram of copper with optimum concentration of SN, PL, MP. | |
The impedance data have been analyzed using an electrochemical equivalent circuit represented in Fig. 7 where Rs, Rct, W, and CPE stand for solution resistance, charge transfer resistance, Warburg impedance and constant phase element respectively. The impedance of the CPE is defined as follows:34
where
Y0−1 is a proportionality factor and ‘
n’ has the meaning of phase shift. The value of ‘
n’ represents the deviation from the ideal behavior
35 and it lies between 0 and 1. The calculated values of
Rct, CPE and chi square (goodness of fit) were obtained from the above mentioned equivalent circuit and are listed in
Table 6. The value of
Rct increases while CPE decreases with the addition of inhibitor in nitric acid solution. The major effect was observed at 0.2 g L
−1 of seed extract which provided
Rct value to 272.82 Ω cm
2 and CPE value is 12.44 μΩ s
n cm
−2. The increase in
Rct can be attributed to the formation of an insulating protective film at the metal–solution interface. The values of
n are 0.85 in blank and 0.89, 0.87 and 0.86 in presence of SN, PL and MP, respectively. The increase in
n values in presence of seed extracts indicating reduction of surface inhomogeneity due to the adsorption of inhibitor molecules on the active adsorption sites at copper surface.
36 The diffusion process is controlled by the transport of dissolved oxygen from the bulk to the electrode surface and this accounts for
W observed in low frequency region.
33,37 The value of Warburg impedance increases after addition of inhibitor with respect to blank and maximum value is observed for SN.
 |
| | Fig. 7 The best fitted equivalent circuit for Cu metal in the electrolyte. | |
Table 6 Impedance parameter obtained fitting the Nyquist plots with the equivalent circuit in absence and presence of highest concentration of SN, MP and PL at 308 K
| Inhibitor |
Rs (Ω cm2) |
Rct (Ω cm2) |
CPE |
Chi square |
W (Ω−1 cm2 s1/2) |
η% |
| Y0 (μΩ−1 sn cm−2) |
n |
| Blank |
4.26 |
23.05 |
160.2 |
0.85 |
0.0016 |
0.0045 |
— |
| SN |
7.29 |
272.82 |
124.4 |
0.89 |
0.0082 |
0.0146 |
91.6 |
| PL |
5.91 |
115.64 |
97.1 |
0.87 |
0.0264 |
0.0384 |
80.0 |
| MP |
3.83 |
77.17 |
96.6 |
0.86 |
0.0287 |
0.0927 |
70.1 |
3.3. Adsorption isotherms
The nature of corrosion inhibition can be deduced in terms of adsorption isotherms by fitting the data in various isotherms like Freundlich, Temkin, Langmuir and Frumkin. Langmuir isotherm expressed by the following equation is found to be the best fitted.38,39| |
 | (11) |
where Kads is adsorption equilibrium constant, Cinh denotes the concentration of inhibitor and θ represents the surface coverage.
The plots of Cinh/θ and Cinh at different concentrations of SN, PL and MP gave straight lines (Fig. 8) suggesting the adsorption of inhibitors on the metal surface follows the Langmuir adsorption isotherm. It was found that R2 and slope value obtained from Langmuir plots are close to 1, a necessary condition for the validity of Langmuir isotherm. The adsorption equilibrium constant (Kads) is associated with standard free energy of adsorption ΔG0ads by the following equation:
| |
ΔG0ads = RT![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) (CH2OKads) (CH2OKads)
| (12) |
where
R is universal gas constant,
T is the absolute temperature and
C is the concentration of water (1000 g L
−1). Since the values of
Kads is represented here is in g
−1 L, so, the concentration of water is also taken in g L
−1.
 |
| | Fig. 8 Langmuir adsorption isotherm plot for copper in 3 M HNO3 in presence of optimum concentration of (0.2 g L−1) SN, PL and MP. | |
The values of Kads and ΔG0ads were calculated and recorded in Table 7. It is seen that the negative value of ΔG0ads is found in all cases indicating the spontaneity of adsorption processes.
Table 7 Thermodynamics parameters for adsorption of SN, PL, and MP inhibitors on copper in 3 M HNO3 solution at 308 K
| Inhibitor |
Kads (L g−1) |
R2 |
−ΔGads (kJ mol−1) |
| SN |
10.54 |
0.998 |
27.21 |
| PL |
10.35 |
0.999 |
27.00 |
| MP |
10.20 |
0.998 |
26.14 |
3.4. Effect of time on the stability of adsorbed extract
The stability of adsorbed layer of inhibitors (SN, PL, MP) with respect to time was analyzed by UV-Visible spectral analysis of the resulting electrolyte. Absorbance of electrolyte were measured after corrosion studies at 4, 8, 12 and 24 h immersion time shown in Fig. 9a–e. The maximum absorbance found at 795, 346, 337 and 336 nm for blank, SN, PL and MP, respectively. These peaks presented copper1 in blank and also brucine,40 piperine41,42 and L-DOPA43,44 which are major component present in SN, PL and MP, respectively. The peak observed in blank because of copper ions introduced in the electrolyte as result of corrosion. Whereas, no peak was observed with SN, PL and MP indicate inhibition of copper corrosion due to these extracts. From Fig. 9b–d it was observed that peak intensity of each leaf extract has decreased and correspondingly copper peak intensity has increased with immersion time. This shows that the adsorbed layer of these extracts destabilizes with time indicating physical adsorption. The peak intensity of characteristic peak of each seed extract is greater than corresponding copper which indicates that these inhibitors were still effective after 24 h of immersion time (Fig. 9e) but the inhibition efficiency decreased.
 |
| | Fig. 9 UV-Visible spectra of seed extracts in 3 M HNO3 after corrosion study (a) SN, PL, MP after 4 h, (b) SN after 4–24 h, (c) PL after 4–24 h, (d) MP after 4–24 h and (e) SN, PL, MP after 24 h. | |
3.5. Quantum chemical calculation
The inhibitive property of an inhibitor depends upon the electronic structure of the molecules.45 The quantum chemical calculation gives an idea about the mechanism of inhibitor action and recently a large number of articles have been published which are focusing on the corrosion inhibition.46 Major component present in the extracts of SN, PL and MP (Table 1) are considered for DFT study. The HOMO and LUMO population of the studied major components in both molecular and protonated forms are shown in Fig. 10 and 11, respectively. The quantum chemical parameters i.e. EHOMO, ELUMO, ΔE, ionization potential (I), electron affinity (A) and the fraction of electron transferred (ΔN) of each major constituent are listed in Table 8.
 |
| | Fig. 10 Energy level diagrams for the frontier MOs of molecular form of major components brucine (SN), piperine (PL) and L-DOPA (MP). | |
 |
| | Fig. 11 Energy level diagrams for the frontier MOs of protonated form of major components brucine (SN), piperine (PL) and L-DOPA (MP). | |
Table 8 Quantum chemical parameters calculated for SN (brucine), PL (piperine), MP (L-DOPA) in both molecular and protonated forms
| Inhibitor |
Total energy (a.u.) |
HOMO (kJ mol−1) |
LUMO (kJ mol−1) |
ΔE (kJ mol−1) |
μ (Debye) |
I |
A |
χ |
γ |
ΔN |
| SN |
−1302.3 |
−484.1 |
−273.0 |
211.0 |
3.54 |
484.1 |
273.0 |
378.5 |
105.5 |
0.824 |
| Protonated SN |
−940.3 |
−1297.8 |
−1246.0 |
51.8 |
5.12 |
1297.8 |
1246.0 |
1271.9 |
25.9 |
−15.61 |
| PL |
−941.0 |
−566.5 |
−82.1 |
484.4 |
4.50 |
566.5 |
82.1 |
324.3 |
242.2 |
0.572 |
| Protonated PL |
−703.1 |
−2449.8 |
−2380.7 |
69.1 |
6.06 |
2449.8 |
2380.7 |
2415.2 |
34.5 |
−28.29 |
| MP |
−705.4 |
−606.7 |
−66.1 |
540.5 |
2.79 |
606.7 |
66.1 |
336.4 |
270.2 |
0.468 |
| Protonated MP |
−1112.1 |
−1316.9 |
−1230.7 |
86.2 |
5.00 |
1316.9 |
1230.7 |
1273.8 |
43.1 |
−9.40 |
According to the frontier molecular orbital (FMO) theory, the ability of an inhibitor to donate their electrons to metal surface is associated with the EHOMO values i.e. higher the EHOMO values easier will be the donation of electrons from inhibitor to empty metal d-orbital. On the other hand, the ability of inhibitor molecules to accept the electrons is associated with the ELUMO values i.e. lower its value easier will be the acceptance of additional negative charges by the inhibitor molecules. Also the stability index of any inhibitor is related to the energy difference (ΔE) between EHOMO and ELUMO. Thus, the lower ΔE values, will correspond to higher stability of inhibitor on the metal surface.47 From Table 8, it is evident that EHOMO values are as follows SN (−484.1 kJ mol−1) > PL (−566.5 kJ mol−1) > MP (−606.7 kJ mol−1), which suggests that electron donation ability of SN is the highest and it will adsorb to a greater extent over copper surface. In the same way ELUMO values are in the order: SN (−273.0) > PL (−82.1) > MP (−66.1), lower value of SN indicates its ability to accept electrons and thereby reducing corrosion of copper to a greater extent.48
In brucine, the presence of two methoxy groups directly attached to the benzene nucleus enhances the electron density of benzene ring which favors its greater adsorption on the copper surface. On other hand, piperidine has better inhibition efficiency as compared to L-DOPA because heteroatoms, O, and N are fused in ring structure. The presence of COOH (electron withdrawing) group which decreases the electron density on nitrogen atom of NH2 and OH moiety attached to benzene ring also decreases electron density of the ring in case of PL.
The adsorption/interaction between major component of an extract and copper–electrolyte interface can be described as follows: (i) charge-transfer-type interactions between unshared electron pairs (present on oxygen and nitrogen) or delocalized π electrons on aromatic ring(s) and the vacant low-energy d orbital's of surface copper atoms (chemisorptions); (ii) electrostatic interactions between protonated inhibitor and negatively charged Cu surface (physisorption) and (iii) a combination of the two (mixed type). The energy gap ΔE (ELUMO − EHOMO) is a function which quantifies the chemical reactivity of an inhibitor molecule for the adsorption on the metallic surface.49 Lower value of ΔE gives good inhibition efficiency because the energy to remove an electron from the last occupied orbital will be low.50 The order of energy gap in protonated inhibitor is similar to that obtained for molecular inhibitor. The observed trend of ΔE is the same for both molecular as well as protonated species. The inspection of the energy gap data in Table 8 reveals that protonated molecules show better inhibition than the molecular one. This may be due to increase in electrostatic interaction between protonated inhibitor and negatively charged Cu metal surface.
According to the Hartree–Fock theorem, the frontier orbital energies given by −EHOMO = I, −ELUMO = A, electronegativity (χ) and global hardness (γ) have proved to be very useful quantities. During a reaction between copper and inhibitor molecules, electrons move from the molecules with lower χ (inhibitor compound) to higher χ (copper) until their chemical potentials become equal. The fraction of electron transferred, ΔN is given by:
| |
 | (13) |
where,
γCu and
γinh are global hardness of copper and inhibitor, respectively.
51 In order to calculate the fraction of electron transferred, a theoretical value for the absolute electronegativity of copper (
χCu) was taken as 463.1 kJ mol
−1 as proposed by Pearson.
52 The global hardness (
γCu = 0) for copper was assigned a value equal to zero based on the assumption
53 that for metallic bulk
I =
A because they are softer than the neutral metallic atoms. The number of electrons transferred (Δ
N) has been tabulated in
Table 8. The value of Δ
N < 3.6 indicates the tendency of a molecule to donate electrons to the metal surface. Hence, the inhibition efficiency increases with increasing electron donating ability of these inhibitors to the metal surface.
30 The electron donating ability of inhibitor molecules follows the order brucine (0.824) > piperine (0.572) >
L-DOPA (0.468). The highest fraction of electrons transferred is associated with the best inhibitor, while the lower fraction is associated with lesser inhibition efficiency. After protonation electron may transfer from filled orbital of metal to vacant orbitals of heteroatoms of inhibitor molecules through back donation of electron. The theoretically calculated results are in good agreement with the experimentally determined data.
3.6. Scanning Electron Microscopy (SEM)
Surface morphology of the copper in 3 M HNO3 in absence and presence of SN, PL, and MP was investigated by SEM studies and illustrated in Fig. 12a–e. It can be seen that the surface is highly corroded in the case of copper surface exposed to 3 M HNO3 (Fig. 12b). Fig. 12c–e indicate that on the addition of best inhibitor (SN) to the solution, the damage has been reduced and the copper surface has been relatively smooth owing to the formation of a protective film on the surface, which prevents the dissolution of copper. These confirm that the presence of adsorbed layer of SN can protect copper from corrosion effectively.
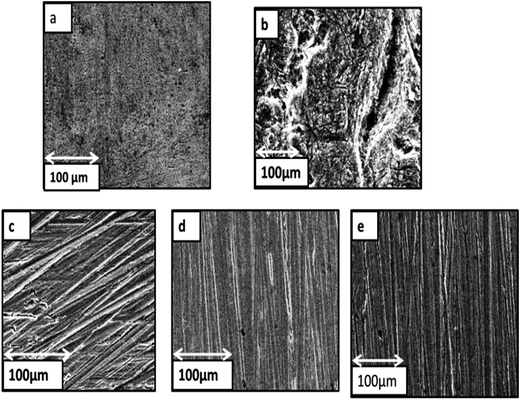 |
| | Fig. 12 SEM micrograph of copper surface (a) abraded, (b) in 3 M HNO3, (c–e) in presence of 0.2 g L−1 SN, PL and MP. | |
3.7. Atomic Force Microscopy (AFM)
The surface morphology of the copper specimens was further studied by Atomic Force Microscopy, which predicted the same trend as in SEM micrographs. Fig. 13a–e shows the 3D AFM micrographs of the corroded and inhibited copper samples in 3 M HNO3 and in the same electrolyte containing 0.2 g L−1 of SN. The average roughness factors calculated values are 162.3 and 108.5 nm, 130.2 nm and 115.4 nm for both blank and inhibitor SN, PL and MP are listed in Table 9. The smoothening of the surface was caused by the deposition of the inhibitor molecules on the surface which protected the surface from the attack of corrosive medium. Hence, both SEM and AFM studies of the metal surface coverage support the results obtained from other techniques.
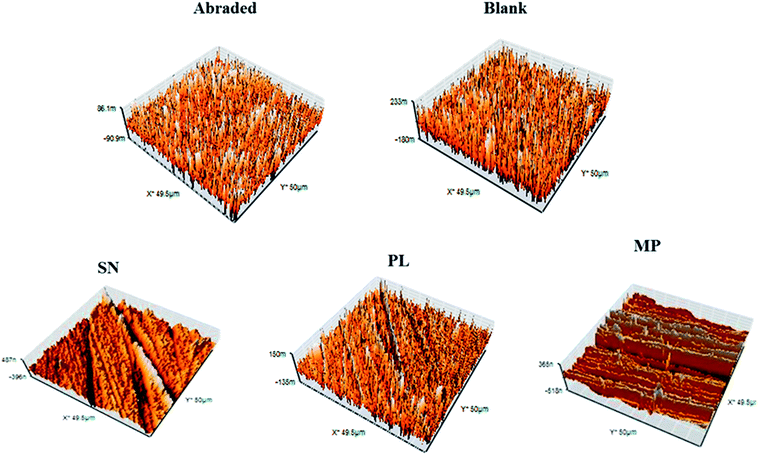 |
| | Fig. 13 AFM micrograph of copper surface (a) abraded, (b) in 3 M HNO3, (c–e) in presence of 0.2 g L−1 SN, PL and MP. | |
Table 9 The area and line roughness obtained from AFM of Cu surface in 3 M HNO3 solution absence and presence of SN, PL and MP at 308 K
| AFM data |
Abraded Cu |
Blank |
3 M HNO3 |
| SN |
PL |
MP |
| Area roughness (nm) |
131.5 |
162.3 |
108.0 |
130.2 |
115.4 |
| Line roughness (nm) |
107.2 |
152.7 |
90.0 |
144.7 |
116.5 |
4. Conclusions
SN, PL and MP extracts were found to act as good inhibitors for copper corrosion in 3 M HNO3 solution. Potentiodynamic polarization measurements have shown that the inhibitors affect both anodic and cathodic reactions and act as mixed inhibitors which are predominantly cathodic. The inhibition efficiency increases with increasing concentrations of extracts. The value of charge transfer resistance was found to be much larger in presence of these extracts than that in their absence. The adsorption process obeys the Langmuir adsorption isotherm. The theoretical study demonstrated that the inhibition efficiency increases with increase in EHOMO and decrease in ΔE. The results calculated from DFT study are in good agreement with the data obtained experimentally.
Acknowledgements
Authors are thankful to Prof. R. K. Mandal, Head, Department of metallurgical Engineering, I.I.T. (B.H.U.) Varanasi for providing SEM facility. Authors are also thankful to Prof. R. B. Rastogi, Department of Chemistry, I.I.T. (B.H.U.) Varanasi for providing AFM facility. They are also thankful to the Head, Chemistry Department, Faculty of Science, Banaras Hindu University, Varanasi, India, for carrying out theoretical calculations.
References
- S. Banerjee, A. Mishra, M. M. Singh, B. Maiti, B. Ray and P. Miti, RSC Adv., 2011, 1, 199–210 RSC.
- Savita, N. Chaubey, P. Mourya, V. K. Singh and M. M. Singh, Int. J. Innov. Res. Sci. Eng. Technol., 2015, 4, 4545–4553 Search PubMed.
- I. R. Glasgow, A. J. Rostron and G. Thomson, Corros. Sci., 1966, 6, 469–482 CrossRef CAS.
- K. C. Emregul, A. A. Akay and O. Atakol, Mater. Chem. Phys., 2005, 93, 325–329 CrossRef.
- M. Behpour, S. M. Ghoreishi, N. Mohammadi, N. Soltani and N. Salavati-Niasari, Corros. Sci., 2010, 52, 4046–4057 CrossRef CAS.
- M. Behpour and N. Mohammadi, Corros. Sci., 2012, 65, 331–339 CrossRef CAS.
- Y. Q. Feng, W. Q. Teo, K. S. Siow, Z. Q. Gao, K. L. Tan and A. K. Hsieh, J. Electrochem. Soc., 1997, 144, 55–64 CrossRef CAS.
- R. M. Saleh, A. A. Ismail and A. A. El Hosary, Corros. Sci., 1983, 23, 1239–1241 CrossRef CAS.
- F. Zucchi and I. H. Omar, Surf. Technol., 1985, 24, 391–399 CrossRef CAS.
- I. Ithaya Kumar, G. Udayabanu and N. S. Rawat, Ann. Univ. Ferrara, 1990, 735 Search PubMed.
- A. A. El-Hosary, R. M. Salah and H. A. El-Dahan, Ann. Univ. Ferrara, 1990, 725 Search PubMed.
- M. Kliškic, J. Radoševic, S. Gudic and V. Katalinic, J. Appl. Electrochem., 2000, 30, 823–830 CrossRef.
- A. Minhaj, P. A. Saini, M. A. Quraishi and I. H. Farooqi, Corros. Prev. Control, 1999, 46, 32–38 CAS.
- K. Srivastava and P. Srivastava, Corros. Prev. Control, 1980, 27, 5 Search PubMed.
- R. M. Saleh, A. A. Ismail and A. A. El Hosary, Corros. Prev. Control, 1984, 31, 21–23 CAS.
- A. A. El Hosary, R. M. Saleh and A. M. Shams El Din, Corros. Sci., 1972, 12, 897–904 CrossRef CAS.
- I. H. Farooqi, M. A. Quraishi and P. A. Saini, Corros. Prev. Control, 1999, 46, 93–96 CAS.
- M. Bozorg, T. S. Farahani, J. Neshati, Z. Chaghazard and G. M. Ziarani, Ind. Eng. Chem. Res., 2014, 53, 4295–4303 CrossRef CAS.
- N. Ogbonna, M. C. Matthew and O. D. Okechukwu, Int. J. Multidiscip. Res. Adv. Eng., 2011, 2, 5–9 Search PubMed.
- A. Singh, Y. Lin, W. Liu, D. Kuanhai Jie Pan, B. Huang, C. Ren and D. Zeng, J. Taiwan Inst. Chem. Eng., 2014, 45, 1918–1926 CrossRef CAS.
- A. S. Fouda, Y. M. Abdallah, G. Y. Elawady and R. M. Ahmed, Ind. Eng. Chem. Res., 2014, 2, 517–531 Search PubMed.
- Savita, P. Mourya, N. Chaubey, V. K. Singh and M. M. Singh, Metall. Mater. Trans. B, 2016, 47, 47–57 CrossRef CAS.
- A. Singh, V. K. Singh and M. A. Quraishi, Rasayan J. Chem., 2010, 3, 811–824 CAS.
- A. Singh, I. Ahamad, A. Mumtaz and M. A. Quraishi, Arabian J. Chem., 2012 DOI:10.1016/j.arabjc.2012.04.029.
- P. A. Raina and R. Khatri, Indian J. Pharm. Sci., 2011, 73, 459–462 Search PubMed.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman Jr J. A. Montgomery, T. Vreven, K. N. Kudin, J. C. Burant, J. M. Millam, S. S. Iyengar, J. Tomasi, V. Barone, B. Mennucci, M. Cossi, G. Scalmani, N. Rega, G. A. Petersson, H. Nakatsugi, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honada, O. Kitao, H. Nakai, M. Klene, X. Li, J. E. Knox, H. p. Hratchian, J. B. cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratman, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, P. Y. Ayala, K. Morokuma, G. A. Voth, P. Salvador, J. J. Dannenberg, V. G. Zakrzewski, S. Dapprich, A. D. Daniels, M. C. Strain, O. Farkas, D. K. Malick, A. D. Rabuck, K. Raghavachari, J. B. Foresman, J. V. Ortiz, Q. Cui, A. G. Baboul, S. Clifford, J. Cioslowski, B. B. Stefanov, G. Liu, A. Liashenko, P. Piskorz, I. Komaromi, R. L. Martin, D. J. Fox, T. Keith, M. A. Al-Laham, C. Y. Peng, A. Nanayakkara, M. Challacombe, P. M. W. Gill, B. Johnson, W. Chen, M. W. Wong, C. Gonzalez and J. A. Pople, Gaussian 03, Revision E.01, Gaussian Inc., Wallingford CT, 2004 Search PubMed.
- S. Martinez and I. Stem, Appl. Surf. Sci., 2002, 199, 83–89 CrossRef CAS.
- E. A. Noor, Int. J. Electrochem. Sci., 2007, 2, 996–1017 CAS.
- N. Chaubey, D. K. Yadav, V. K. Singh and M. A. Quraishi, Ain Shams Engineering Journal, 2015 DOI:10.1016/j.asej.2015.08.020.
- P. Mourya, P. Singh, A. K. Tewari, R. B. Rastogi and M. M. Singh, Corros. Sci., 2015, 95, 71–87 CrossRef CAS.
- M. Pourbaix, Atlas of Electrochemical Equilibria in Aqueous Solutions, NACE, Houston, TX, 1975 Search PubMed.
- H. E. Johnson and J. Leja, J. Electrochem. Soc., 1965, 112, 638 CrossRef CAS.
- K. F. Khaled, Corros. Sci., 2010, 52, 3225–3234 CrossRef CAS.
- Z. Tao, W. He, S. Wang and G. Zhou, Ind. Eng. Chem. Res., 2013, 52, 17891–17899 CrossRef CAS.
- S. K. Singh, S. P. Tambe, G. Gunasekaran, V. S. Raja and D. Kumar, Corros. Sci., 2009, 51, 595–601 CrossRef CAS.
- P. Mourya, S. Banerjee and M. M. Singh, Corros. Sci., 2014, 85, 352–363 CrossRef CAS.
- H. Ma, S. Chen, L. Niu, S. Zhao, S. Li and D. Li, J. Appl. Electrochem., 2002, 32, 65–72 CrossRef CAS.
- K. R. Ansari, M. A. Quraishi and A. Singh, Measurement, 2015, 76, 136–147 CrossRef.
- K. R. Ansari and M. A. Quraishi, Phys. E, 2015, 69, 322–331 CrossRef CAS.
- M. Z. Yates, D. L. Apodaca, M. L. Campbell, E. R. Birnbaum and T. M. McCleskey, Chem. Commun., 2001, 25–26, 10.1039/b007331h.
- A. Kamal, Y. T. Kamal, S. Ahmad, F. J. Ahmad and K. Saleem, J. Pharm. BioAllied Sci., 2012, 4, 134–139 CrossRef PubMed.
- S. C. B. Kotte, P. K. Dubey and P. M. Murali, Anal. Methods, 2014, 6, 8022–8029 RSC.
- J. R. Nakkala, R. Mata and S. R. Sadras, Process Saf. Environ. Prot., 2016, 100, 288–294 CrossRef CAS.
- E. Nalewajko, A. Wiszowata and A. Kojlo, J. Pharm. Biomed. Anal., 2007, 43, 1673–1681 CrossRef CAS PubMed.
- D. P. Yeggoni and R. Subramanyam, Mol. BioSyst., 2014, 10, 3101–3110 RSC.
- G. Gece, Corros. Sci., 2008, 50, 2981–2992 CrossRef CAS.
- G. Gao and C. Liang, Electrochim. Acta, 2007, 52, 4554–4559 CrossRef CAS.
- T. Ghailane, R. A. Balkhmima, R. Ghailane, A. Souizi, R. Touir, M. Ebn Touhami, K. Marakchi and N. Komiha, Corros. Sci., 2013, 76, 317–324 CrossRef CAS.
- S. Nofrizal, A. A. Rahim, B. Saad, P. Bothiraja, A. M. Shah and S. Yahya, Metall. Mater. Trans. A, 2012, 48, 1382–1393 CrossRef.
- V. Jaiswal, R. B. Rastogi, J. L. Maurya, P. Singh and A. K. Tewari, RSC Adv., 2014, 4, 13438–13445 RSC.
- N. Karakus and K. Sayin, J. Taiwan Inst. Chem. Eng., 2015, 48, 95–102 CrossRef CAS.
- R. G. Pearson, Inorg. Chem., 1988, 27, 734–740 CrossRef CAS.
- N. A. Wazzan, J. Ind. Eng. Chem., 2015, 26, 291–308 CrossRef CAS.
|
| This journal is © The Royal Society of Chemistry 2016 |
Click here to see how this site uses Cookies. View our privacy policy here. 
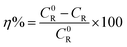



![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR and 1/T Fig. 2. The Ea value in absence of inhibitor is 56.13 kJ mol−1 and in the presence of SN, PL and MP, these values are 120.05 kJ mol−1, 86.16 kJ mol−1 and 81.18 kJ mol−1 respectively. Higher Ea values for inhibitor molecules indicate retardation in mass/charge transfer from the surface to the electrolyte due to surface barrier.29
CR and 1/T Fig. 2. The Ea value in absence of inhibitor is 56.13 kJ mol−1 and in the presence of SN, PL and MP, these values are 120.05 kJ mol−1, 86.16 kJ mol−1 and 81.18 kJ mol−1 respectively. Higher Ea values for inhibitor molecules indicate retardation in mass/charge transfer from the surface to the electrolyte due to surface barrier.29

![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) CR/T verses 1/T shown in Fig. 3 gave a straight line with a slope of ΔHa/2.303R and an intercept of log(R/Nh) + ΔSa/2.303. The values of ΔH0a and ΔS0a were calculated from three parameters and are listed in Table 4. The standard enthalpy of activation energy ΔH0a showed similar trend like the activation energy values. The entropy of activation ΔS0a values is negative in the absence and positive in presence of inhibitors. The negative ΔS0a value indicates the activated complex is more ordered in the blank solution. The positive values of ΔS0a suggest that an increase in disordering takes place in going from reactants to the activated complex on metal/solution interface22 which is driving force for the adsorption.
CR/T verses 1/T shown in Fig. 3 gave a straight line with a slope of ΔHa/2.303R and an intercept of log(R/Nh) + ΔSa/2.303. The values of ΔH0a and ΔS0a were calculated from three parameters and are listed in Table 4. The standard enthalpy of activation energy ΔH0a showed similar trend like the activation energy values. The entropy of activation ΔS0a values is negative in the absence and positive in presence of inhibitors. The negative ΔS0a value indicates the activated complex is more ordered in the blank solution. The positive values of ΔS0a suggest that an increase in disordering takes place in going from reactants to the activated complex on metal/solution interface22 which is driving force for the adsorption.

![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln
ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) (CH2OKads)
(CH2OKads)















