The effect of amino substituents on the interactions of quinazolone derivatives with c-KIT G-quadruplex: insight from molecular dynamics simulation study for rational design of ligands

Abstract

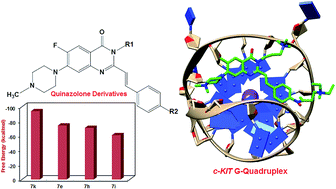
Stabilization of G-quadruplex structures in the oncogenic promoter regions with small molecules has attracted considerable attention as a promising target for cancer therapy. To discover such small molecules, understanding the nature of interactions between the ligand and G-quadruplex is of paramount importance. To precisely investigate how these interactions can be influenced by varying different substituents, binding interactions of some quinazolone derivatives (QDs) with c-KIT G-quadruplex were studied by molecular dynamics (MD) simulation. The results revealed that the QD–NH–CO– arrangement in quinazolone derivatives improve binding affinity toward c-KIT G-quadruplex and the amino substituents play a crucial role in hydrogen bond formation and electrostatic interactions with the phosphate backbone of the G-quadruplex. We also proposed a new derivative of quinazolone (7k) with a terminal amino substituent instead of a 3-phenyl group. The binding free energy analysis suggested that this derivative stabilizes the c-KIT G-quadruplex much better than other derivatives. Furthermore, the calculated changes in solvent-accessible surface area (ΔSASA) were consistent with the binding free energy calculations. Our studies provide insight into the effect of different substituents on binding interactions between the ligand and G-quadruplex which can pave the way to rational ligand design.

---

1. Introduction

Guanine-rich sequences of DNA can be self-assembled into four-stranded structures defined as G-quadruplexes^1–3 which are composed of stacked planar G-quartets, each of which is held together by a network of Hoogsteen hydrogen bonds between four guanine bases.^4,5 G-quadruplex structures are mainly found in telomeres⁶ and particularly widespread in gene promoters such as k-ras,⁷ bcl2,⁸ c-myc,⁹ c-KIT,^10,11 etc. It has been hypothesized that stabilization of G-quadruplex structures formed within the oncogene promoter regions with the aid of small molecules can attenuate gene overexpression and can be considered as a cancer therapeutic target.^12–15

The human c-KIT is a significant proto-oncogene that its expression is necessary to control different normal cells growth, whereas overexpression or mutation of this proto-oncogene has been noticed in various cancers including gastrointestinal stromal tumors (GIST), leukemias, melanoma and pancreatic cancers.^16–20 Therefore, there is substantial interest in the design of small molecules that selectively stabilize c-KIT G-quadruplex which can result in inhibition of gene expression in cancer cells.

In recent years, many different ligands such as bisquinoline–pyrrole oligoamide,²¹ porphyrine,²² substituted indenoisoquinolines,²³ benzo[a]phenoxazines,²⁴ trisubstituted isoalloxazines,²⁰ bis-indole carboxamides,²⁵ biaryl polyamides,²⁶ phenanthroline-2,9-bistriazoles,²⁷ etc., have been synthesized and employed to stabilize G-quadruplex of the c-KIT promoter. Most of G-quadruplex stabilizing ligands follow structural features that contain a planar aromatic surface which stack on a G-quartet, cationic side chains which interact with the phosphate backbone, grooves and loops and also a cationic core in the center of the ligand which interact with the negatively charged center of G-quadruplex.²⁸ In order to discover drug-like ligands which selectively bind to c-KIT G-quadruplex, Jia-Heng Tan's group synthesized a new class of quinazolone derivatives because these derivatives display broad-spectrum properties such as antibacterial, antidiabetic, anti-inflammatory, antitumor, and several others. These unfused aromatic ligands contain two cationic amino substituents and an expanded aromatic structure. The experimental studies showed that the variation of the substituents in quinazolone derivatives play a significant role on stabilizing c-KIT G-quadruplex.²⁹ To understand how the substituents can influence the binding affinity, it is essential to investigate the nature and contribution of the interactions between these ligands and c-KIT G-quadruplex that has not yet been reported.

In this work, molecular docking and molecular dynamics (MD) simulation were performed to precisely study the nature of interactions between three quinazolone derivatives (7e, 7h and 7i with the same numbering as the experimental study) (Fig. 1) and c-KIT G-quadruplex. These three ligands, selected based on their structural features, are used to study the impact of their structural variations on the stability of c-KIT G-quadruplex. In ligand 7i, the role played by inflexible pyrrolidino group at the side chain of this ligand on the interactions between the ligand and c-KIT G-quadruplex was studied. The pyrrolidino group of ligand 7i was substituted by diethylamino group in ligand 7h to investigate the effect of flexibility of the side chain on G-quadruplex stabilization. Finally, amido bond (QD–CO–NH–) arrangement in ligand 7h was interconverted in ligand 7e to explore how the arrangement of QD–NH–CO can influence on binding affinity. The MD simulations provide a valuable insight into how the minor change from QD–CO–NH– in ligand 7h and 7i to QD–NH–CO– arrangement in ligand 7e can play a role in increasing electrostatic interactions and stabilizing c-KIT G-quadruplex.

Fig. 1 Quinazolone derivatives.

Furthermore, molecular electrostatic potential maps (MEP) were plotted to support information about electrostatic interactions. In addition, Molecular Mechanics, Poisson–Boltzmann, Surface Area (MMPBSA) analysis was used to calculate binding free energies between these ligands and c-KIT G-quadruplex. The obtained results of free energy analysis provided valuable information regarding driving forces for G-quadruplex–ligand interaction and complex stability which are helpful in the design of potential ligands with high binding affinity. On this basis, we proposed a new derivative of quinazolone (7k) with a terminal amino side chain instead of 3-phenyl group. The aforementioned studies were carried out to explain in detail the effect of substituent modification on the binding of this ligand to c-KIT G-quadruplex.

2. Computational methods

For the molecular modeling studies, c-KIT-1 G-quadruplex (5′-AGGGAGGGCGCTGGGAGGAGGG-3′, PDB ID: 2O3M) was selected as a starting structure from the Protein Data Bank (PDB). The molecular structures of all ligands were optimized with DFT method at the hybrid functional B3LYP and the basis set 6-31+G(d) level using Gaussian 03 program.³⁰ The partial charges were assigned using AM1-BCC method via the ACPYPE tool.³¹ Other parameters were generated based on the Generalized Amber Force Field (GAFF)³² using ANTECHAMBER module of AmberTools14.³³

2.1. Molecular docking

The binding modes of the ligands with G-quadruplex were investigated with AUTODOCK 4.0 program.³⁴ Nonpolar hydrogens were merged to their corresponding carbons. Gasteiger and Kollman partial charges were assigned for the ligands and G-quadruplex respectively by AutoDockTools (ADT). The generated ADT format files for the ligands and target G-quadruplex were utilized as an input for the AUTOGRID program. The dimensions of the grid box was set to 70 Å × 70 Å × 70 Å with 0.375 Å spacing at the center of the G-quadruplex structure. One hundred docking runs were performed for each complex. Lamarckian genetic algorithm (LGA) was used for each docking calculation. Maximum number of energy evaluations and population size were set to 2 500 000 and 150 respectively. Other parameters were set as default. The results of docking calculations were analyzed using RMSD clustering based on 0.5 Å root mean square criterion.

2.2. Molecular dynamics (MD) simulation

MD simulations were carried out for the best conformers obtained from molecular docking studies using Gromacs 4.6.5 package³⁵ with Parmbsc0 force field.³⁶ For the stability of the G-quadruplex structure, two central K⁺ ions were located manually in the center of G-quartets. All complexes were solvated in a triclinic box of TIP3P³⁷ water molecules with a 10.0 Å buffer between the molecule(s) periphery and box edge. Periodic boundary conditions were applied in all dimensions. 17K⁺ counter ions were added to G-quadruplex–ligand complexes to neutralize the systems. The particle mesh Ewald (PME) method was applied to calculate long-range electrostatic interactions³⁸ and the LINCS algorithm³⁹ was used to constrain all bonds. A cut-off of 10.0 Å was used for non-bonded van der Waals interactions. At first, the solvated structures were minimized about 2000 steps using the steepest descent algorithm. The systems were then equilibrated under NVT ensemble at 300 K during 200 ps. This was followed by a 300 ps NPT equilibration run at 1 bar pressure and 300 K temperature. The velocity rescaling algorithm was used for the temperature coupling at 300 K with a time constant 0.1 ps.⁴⁰ The pressure was controlled via the Parrinello–Rahman barostat algorithm at 1 bar with time constant 1 ps.^41,42 Finally, MD production run was performed for 50 ns under equivalent conditions at 1 bar and 300 K. The time step of 2.0 fs was applied in all MD simulations and the output files were saved every 2 ps for the further analysis. Finally, the results were analyzed and visualized by means of the Discovery Studio Visualizer 4.1 (http://accelrys.com/products/discovery-studio) and Chimera 1.10 (ref.43) programs.

2.3. Molecular electrostatic potential (MEP)

Molecular electrostatic potentials were calculated at the B3LYP/6-31G+(d) optimized geometry for all ligands. For the G-quadruplex structures, MEP maps were generated using the Adaptive Poisson–Boltzmann Solver (APBS) program⁴⁴ in AutoDockTools. The dielectric constant values for G-quadruplex and the surrounding solvent were set to 2 and 80 respectively. The dielectric boundary was defined around the molecular surface by setting the solvent radius to 1.4 Å.

2.4. Free energy calculation

The binding free energies were calculated by MMPBSA (Molecular Mechanics, Poisson–Boltzmann, Surface Area) analysis⁴⁵ using GMXAPBS tool.^46,47 Note that explicit water molecules and counterions were removed from the MD trajectories whereas two bound K⁺ in the channel of G-quadruplex were considered in MMPBSA analysis as reported by Jayaram⁴⁸ and Štefl et al.⁴⁹

For each snapshot, the MMPBSA calculated the binding free energy using the following equation:

ΔG_bind = G_complex − (G_ligand + G_quadruplex) (1)

The free energy terms of the complex, ligand and G-quadruplex in above equation were calculated according to eqn (2):

〈G〉 = 〈E_MM〉 + 〈G_solv〉 − T〈S_MM〉 (2)

T〈S_MM〉 refers to the entropy term where T and S denote the temperature and entropy, respectively.

The molecular mechanics energy was obtained as the following sum:

E_MM = E_int + E_coul + E_vdw (3)

where E_int is the sum of the bond, angle, and dihedral energies, E_coul and E_vdw indicate the electrostatic and the van der Waals interactions, respectively.

The solvation energy term in eqn (2) was estimated with the eqn (4):

G_solv = G_ps + G_nps (4)

G_ps and G_nps were calculated with Adaptive Poisson–Boltzmann Solver (APBS) program.

For the calculation of polar solvation term, dielectric constants of 1.0 and 80.0 were considered for the solute and solvent, respectively.

Nonpolar solvation term was calculated using the following equation:

G_nps = γSASA + β (5)

where γ = 0.0227 kJ mol⁻¹ Å⁻² and β = 0 kJ mol. SASA is the solvent accessible surface area (Ų).

2.5. Solvent accessible surface area (SASA) calculation

The solvent accessible surface area (SASA) was computed for each complex using g_sas tool of Gromacs. The accessible surface is generated by tracing the center of the probe sphere rolling along the van der Waals surface. A probe radius of 1.4 Å was used for the solvent. Total SASA for each molecule is sum of the hydrophobic and hydrophilic SASA. The calculations were performed by eqn (6):

ΔSASA = SASA_complex − (SASA_quadruplex + SASA_ligand) (6)

3. Result and discussion

3.1. Molecular docking

Molecular docking is a computer-based technique that is used to predict the possible binding sites between small molecule drug candidates and a receptor.⁵⁰ In this study, molecular docking studies were carried out to predict the favorable binding mode of quinazolone derivatives with G-quadruplex. The results of clustering analysis revealed that all ligands stack at the 3′ end of G-quadruplex (Fig. S1 ESI†) and two protonated amino groups at the side chains of ligands interact with negatively charged backbone through hydrogen bonding and electrostatic interactions. The results of binding energies are listed in Table 1. The binding energies rank order is 7e > 7h > 7i that is consistent with experimental results.²⁹ The result also emphasized that 7k shows slightly higher binding energy than other derivatives.

Table 1 Binding energies of all ligands to c-KIT G-quadruplex (kcal mol⁻¹)

Complex	Binding energy (kcal mol^−1)
7e	−12.62
7h	−11.51
7i	−10.74
7k	−12.75

---

Finally, the lowest energy conformation of each complex was selected for molecular dynamics studies.

3.2. MD simulation

On the basis of docking results, 50 ns MD simulations were performed on all complexes. The final structure of each complex is shown in Fig. S2 (ESI†). To understand the nature of the interactions between the ligands and G-quadruplex, the MD simulation results were analyzed as follows.

3.2.1. Structural stability. Root mean square deviation (RMSD) values were calculated to assess conformational stability of each complex over the entire simulation. RMSD values of heavy atoms in G-quartet and backbone were measured for all MD trajectories relative to initial reference structures (Table 2) and plotted versus 50 ns time simulation (Fig. 2). The RMSD plots illustrate that the all trajectories have small fluctuations throughout 50 ns simulations indicating that all complexes become stable to further analyze the trajectories.

Table 2 Average RMSD values (Å) of the heavy atoms in the G-quartet and backbone from the MD simulations

Complex	Heavy atoms of G-quartet	Heavy atoms of backbone
7e	1.15	1.45
7h	1.16	1.49
7i	1.16	1.61
7k	1.13	1.45

---

Fig. 2 Time dependence of the RMSDs (Å) of the heavy atoms in G-quartet (black) and backbone (red).

As evident from Table 2, the RMSD values of G-quartet heavy atoms range from 1.13 to 1.16 Å, while the backbone of G-quadruplex has slightly higher RMSD fluctuations. This behavior is because of high rigidity of G-quartets which are held strongly by eight hydrogen bonds whereas the backbone residues are free to move over the course of the simulation.

3.2.2. Hydrogen bonding analysis. The Hoogsteen hydrogen bonds (N2–H⋯N7 and N1–H⋯O6) between the G-quartets are very important factors in G-quartets stabilization (Fig. 3). For all complexes, average hydrogen bond occupancies were calculated for each G-quartet during 50 ns MD simulation (Table 3). It is observed that the average hydrogen bond occupancy in the middle G-quartet (G-quartet 2) is lower than outer G-quartets. This is because the O6 atoms of guanine bases in G-quartet 2 can form strong attractive interactions with 2K⁺ ions. The results also indicate that G-quartet 3 is slightly less stable than G-quartet 1 for 7h and 7i. The average hydrogen bond occupancies of G-quartet 3 for 7h and 7i are 99.3% and 99.1%, respectively. This is due to the impact of ligands on stability of G-quartet 3 coplanar structure. In these two ligands with the QD–CO–NH– arrangement, O22 atom (Fig. 1) is close to the G15 of G-quartet 3 (G22–G15–G4–G8) which leads to the formation of the weak hydrogen bond (with occupancy of lower than 40%) between O22 atom of ligands and H21 of G15. As a result of these interactions, G15 move closer to carbonyl group of ligand 7h and 7i. This displacement can slightly disturb the Hoogsteen hydrogen bonds of G22–G15–G4–G8 quartet and destabilize the coplanar structure of this G-quartet. Consequently, a slight decrease occurs in hydrogen bond occupancy of G-quartet 3 as compared to that of G-quartet 1. Whereas, in the case of 7e and 7k with the QD–NH–CO– arrangement, O22 atoms are not close to guanine bases of G-quartet 3 and, consequently, they are not able to form hydrogen bond. In absence of any hydrogen bonding interactions between these ligands and guanine bases of G-quartet 3, the ligands do not disturb Hoogsteen hydrogen bonds of G22–G15–G4–G8 quartet. Therefore, G-quartet 3 has not lower occupancy in comparison with that of G-quartet 1. In the case of 7k, very slightly increased occupancy of G-quartets is a clear indication of this ligand stabilizing effect on G-quartets.

Fig. 3 Hoogsteen hydrogen bonds in one G-quartet.

Table 3 Average occupancy (%) of Hoogsteen hydrogen bond between the guanine bases present in each G-quartet during 50 ns MD simulation

G-quartet	7e	7h	7i	7k
G-quartet 1 (G10–G13–G2–G6)	99.5	99.5	99.5	99.6
G-quartet 2 (G21–G14–G3–G7)	97.0	96.4	96.4	97.0
G-quartet 3 (G22–G15–G4–G8)	99.5	99.3	99.1	99.7

---

According to Table 3, the average hydrogen bond occupancy of G-quartets is in the order of 7k > 7e > 7h > 7i. This means that the average hydrogen bond occupancy of G-quartets is slightly higher for 7e complex (98.7%) compared to 7h (98.4%) and 7i (98.3%) complexes, indicating that ligand 7e with the QD–NH–CO– arrangement has a stabilizing effect on G-quartets which is consistent with results reported from the experimental studies.²⁹ Moreover, the average hydrogen bond occupancy of ligand 7k (98.8%) implies that this new derivative of quinazolone provides slightly more stability to G-quartets.

In addition, hydrogen bonding data between G-quadruplex and ligands were analyzed and summarized in Table 4. Note that in hydrogen bonding calculations, the cutoff distance and angle deviation were set to 3.5 Å and 35° respectively. As shown in detail in Table 3, ligand 7e and 7k (Fig. 4) with the QD–NH–CO– arrangement form three hydrogen bonds with G-quadruplex structure contributing to its stabilization. In this case, one hydrogen bond was formed between O4′ atom of G20 and protonated terminal tertiary amine (N42) with a mean distance of 3.5 Å and 3.0 Å for 7e and 7k, respectively. On the other hand, ligand 7e and 7k were hydrogen bonded via hydrogen atom (H22) connected with the nitrogen atom no. 21 (N21) to O1 atom of G17 phosphate backbone and O3′ atom of A16.

Table 4 Hydrogen bonding data during 50 ns MD simulation between G-quadruplex and ligands over 40.0% of the time during the simulations

Complex	Donor	Acceptor	Occupancy (%)	Distance (Å)	Angle (°)
7e	25LIG(H45)N42	20 DG (O4′)	66.6	3.5(0.9)	26(14)
	25LIG(H22)N21	17 DG (O1P)	54.9	3.3(0.7)	35(27)
	25LIG(H22)N21	16 DA (O3′)	63.9	3.2(0.6)	32(26)
7h	25LIG(H45)N42	20 DG (O4′)	72.3	3.2(0.4)	26(12)
	25LIG(H28)N27	17 DG (O1P)	76.3	3.2(0.7)	21(20)
7i	25LIG(H45)N42	20 DG (O4′)	91.3	3.0(0.2)	22(8)
	25LIG(H28)N27	17 DG (O1P)	87.6	3.0(0.5)	19(21)
7k	25LIG(H45)N42	20 DG (O4′)	93.3	3.0(0.2)	21(8)
	25LIG(H22)N21	17 DG (O1P)	48.5	3.3(0.6)	41(32)
	25LIG(H22)N21	16 DA (O3′)	66.9	3.1(0.4)	32(27)

---

Fig. 4 Hydrogen bonds formed from MD simulation for 7e, 7h, 7i and 7k complexes.

As shown in Fig. 4, for ligand 7h and 7i with the QD–CO–NH– arrangement, two protonated terminal amines at the side chains (N42 and N27) were close to the O4′ atom of G20 and O1 atom of G17 to form the hydrogen bonding interactions. The mean hydrogen bond distances between these two amines and the backbone of G-quadruplex (O4′ atom of G20 and O1 atom of G17) are 3.2 Å (7h) and 3.0 Å (7i).

Therefore, it can be concluded that the formed hydrogen bonds between G-quadruplex and the ligands play an active role to enhance binding affinity of the ligands toward G-quadruplex.

3.2.3. Molecular electrostatic potential. Molecular Electrostatic Potential (MEP) describes charge distribution of a molecule in its surrounding space and is an important descriptor to study electrophilic and nucleophilic interactions of small molecules. In order to determine the electrostatic interactions, MEP maps were plotted for all ligands in Fig. 5. The range of color code is between −0.24 (red) and 0.24 (blue) and blue region represents the positive electrostatic potential associated with electron deficient. The electrostatic potential map of ligand 7e, 7h and 7i are represented that the electrostatic potential of N21 atom in ligand 7e is slightly more positive than their counterpart in ligand 7h and 7i. The implication is that ligand 7e has stronger electrostatic interactions with c-KIT G-quadruplex than 7h and 7i. Therefore, the nature of the QD–NH–CO– side chain in ligand 7e plays a key role in the electrostatic interactions providing the driving force for binding.

Fig. 5 Electrostatic potential map of ligands.

As can be seen from Fig. 5, the positive electrostatic potential areas for ligand 7k are mainly focused on the three terminal tertiary amines. Therefore, these amino groups are considered as active sites for the electrostatic interactions. It is worth noting that the introduction of a third amino group instead of 3-phenyl group provides more opportunities for electrostatic interactions with c-KIT G-quadruplex compared to other ligands, and as such increases complex stabilization.

Furthermore, MEPs for all complexes were mapped on the molecular surface after MD simulations. As shown in Fig. 6, the negative charge of G-quadruplex provides an overall negative electrostatic potential (colored red) which can increase its electrostatic interactions with the positively charged regions of the ligands.

Fig. 6 Electrostatic potential maps of G-quadruplex–ligand complexes.

3.2.4. Free energy calculations. For further understanding of substituent effects upon G-quadruplex stabilization, the energy contributions and total binding free energies of all complexes were calculated based on 100 snapshots extracted during MD simulations. The obtained results are presented in Table 5.

Table 5 Binding free energy calculation of G-quadruplex–ligand complexes^a (kcal mol⁻¹)

Complex	ΔEcoul	ΔEvdw	ΔGps	ΔGnps	ΔGpolar	ΔGnonpolar	ΔGbind
a ΔEcoul, and ΔEvdw are the electrostatic and van der Waals energies. ΔGps and ΔGnps are the polar and nonpolar contributions to the solvation energy. ΔGpolar is sum of the electrostatic energy (ΔEcoul) and polar solvation energy (ΔGps). ΔGnonpolar is sum of the van der Waals energy (ΔEvdw) and nonpolar solvation energy (ΔGnps). ΔGbind is the binding free energy (ΔEcoul + ΔEvdw + ΔGps + ΔGnps).
7e	−4289.352	−238.151	4474.126	−20.932	184.774	−259.083	−74.309
7h	−4157.282	−237.727	4343.679	−19.669	186.397	−257.396	−70.999
7i	−3591.523	−192.934	3739.484	−16.014	147.961	−208.948	−60.987
7k	−5930.451	−238.484	6094.573	−19.610	164.122	−258.094	−93.973

---

In order to obtain favorable contribution of each energy term, electrostatic energy (ΔE_coul), van der Waals energy (ΔE_vdw), polar solvation energy (ΔG_ps) and nonpolar solvation energy (ΔG_nps) were investigated for each complex. As can be seen from Table 5, the contributions of nonpolar solvation energy (ΔG_nps) are slightly favorable for the interactions between ligands and G-quadruplex. On the contrary, the values of polar solvation energies (ΔG_ps) are very high positive and enormously unfavorable for binding. On the other hand, the comparison of total gas phase energies (ΔE_coul + ΔE_vdw) and solvation energies revealed that electrostatic, van der Waals and nonpolar solvation energies are the predominant factors for the binding of ligands to G-quadruplex. The very high negative values of electrostatic energies (ΔE_coul) for all complexes implied that the protonated terminal amino groups at the side chains of ligands interact strongly with the anionic charges on the G-quadruplex backbone. In addition, the van der Waals energy contribution (ΔE_vdw) is favorable for all ligands because the quinazolone and benzylidene pharmacophores make van der Waals contacts with G-quartet 3 surface as shown in Fig. S3 (ESI†).

The energy calculations exhibited the negative binding free energies for all complexes, indicating the G-quadruplex stability and binding affinity of quinazolone derivatives. As it can be realized from Table 5 and 7e complex is significantly more stable than 7h and 7i complexes which are in agreement with the reported experimental findings.²⁹ Detailed energy analysis illustrated that ligand 7i with pyrrolidino group at the side chain shows higher binding free energy (ΔG_bind = −60.987 kcal mol⁻¹) compared to other ligands. This behavior is due to the rigidity of the pyrrolidino group and inaccessibility of the loop. To increase the flexibility of the side chain, the pyrrolidino group was substituted by diethylamino group in ligand 7h. The binding free energy calculations showed that this substitution enhances binding affinity and G-quadruplex stabilization (ΔG_bind = −70.999 kcal mol⁻¹). In order to investigate the effect of amido bond (QD–CO–NH–) arrangement on c-KIT G-quadruplex stabilization, we studied ligand 7e with interconverted amido bond. The results indicated that ligand 7e with QD–NH–CO– arrangement provides strong affinity with c-KIT G-quadruplex (ΔG_bind = −74.309 kcal mol⁻¹) when compared to those of 7h and 7i. The current findings suggest that the minor change from QD–CO–NH– (7h and 7i) to QD–NH–CO– (7e) arrangement plays a significant role in increasing electrostatic interactions and stabilizing c-KIT G-quadruplex.

The results of free energy calculations allowed us identifying electrostatic interactions as the main driving force for the binding between quinazolone derivatives and c-KIT G-quadruplex. In addition, MD simulation studies indicated that the phenyl group at the position 3 is not in plane with respect to the quinazolone and benzylidene pharmacophores of these ligands and as a result this group cannot stack on G-quartet surface. Therefore, the phenyl group did not play an active role in interaction with c-KIT G-quadruplex and binding affinity. Moreover, it has been reported that the amino side chains are positively charged at physiological pH which contribute to the strong electrostatic interaction with the phosphate backbone of G-quadruplex.⁵¹ This evidence prompted us to design a new quinazolone derivative (7k) which contain a terminal amino side chain instead of 3-phenyl group. We hypothesized that the amino group can enhance electrostatic interactions and G-quadruplex stabilization.

To investigate this hypothesize, the binding free energy of 7k complex was assessed in order to ascertain whether the ligand 7k has a stronger binding affinity to G-quadruplex compared with the other ligands. Surprisingly, the binding free energy results emphasized that 7k complex is considerably more stable than the other complexes due to its lowest binding free energy of −93.973 kcal mol⁻¹. In fact, introduction of a terminal amino substituent instead of 3-phenyl group in ligand 7k dramatically improved electrostatic interactions which subsequently enhanced its binding affinity to c-KIT G-quadruplex.

3.2.5. Solvent accessible surface area analysis. The solvent accessible surface area (SASA) results are listed in Table 6. It is found that the ΔSASA values are reduced from ligand 7i to 7e. This means that the ligand 7e has high interaction with G-quadruplex in comparison to 7h and 7i. This reduction is also maximized in the case of ligand 7k binding to the G-quadruplex. These results accord with the free energy calculations.

Table 6 Calculations of change in solvent-accessible surface area (Ų)

Complex	SASAcomplex	SASAG-quadruplex	SASAligand	ΔSASA
7e	4839.70	4530.49	824.28	−515.07
7h	4851.14	4524.53	818.60	−491.99
7i	4864.03	4506.13	813.23	−455.33
7k	4881.76	4528.89	872.45	−519.58

---

4. Conclusions

Interactions of c-KIT G-quadruplex with four derivatives of quinazolone were studied by MD simulations to comparatively investigate the impact of substituent variations on the nature of binding. The energy calculations revealed that the van der Waals and electrostatic energies between c-KIT G-quadruplex and ligand 7h with diethylamino group was more favorable than ligand 7i with pyrrolidino group. As a result, the affinity of ligand 7h to G-quadruplex was higher than 7i. Nevertheless, the QD–CO–NH– arrangement in ligand 7h played a key role on stabilization ability of G-quadruplex. Our results demonstrated that the electrostatic interactions between c-KIT G-quadruplex and ligand 7h was enhanced by changing substituent arrangement from QD–CO–NH (7h) to QD–NH–CO (7e). These findings suggested that the contributions of the electrostatic interactions between c-KIT G-quadruplex and quinazolone derivatives are significantly more than other interactions. Based on the results, we introduced a new derivative of quinazolone (7k) with a terminal amino substituent instead of 3-phenyl group to increase the electrostatic interactions. The binding free energy analysis confirmed that the newly designed ligand (7k) interacts more strongly with c-KIT G-quadruplex compared to the other derivatives. Our computational studies help explain how the nature and contribution of interactions in G-quadruplex–ligand binding play crucial roles on G-quadruplex stabilization that directly influence designing of new potential ligands with high binding affinity.

Acknowledgements

We would like to thank Dr. Sabzyan for providing us with hardware and software facilities.

References

1. S. Burge, G. N. Parkinson, P. Hazel, A. K. Todd and S. Neidle, Nucleic Acids Res., 2006, 34, 5402.
2. J. L. Huppert and S. Balasubramanian, Nucleic Acids Res., 2005, 33, 2908.
3. A. K. Todd, M. Johnston and S. Neidle, Nucleic Acids Res., 2005, 33, 2901.
4. S. Neidle, Curr. Opin. Struct. Biol., 2009, 19, 239.
5. S. Neidle and S. Balasubramanian, Quadruplex nucleic acids, RSC, Cambridge, U.K., 2006.
6. H. J. Lipps and D. Rhodes, Trends Cell Biol., 2009, 19, 414.
7. S. Cogoi and L. E. Xodo, Nucleic Acids Res., 2006, 34, 2536.
8. J. Dai, T. S. Dexheimer, D. Chen, M. Carver, A. Ambrus, R. A. Jones and D. Yang, J. Am. Chem. Soc., 2006, 128, 1096.
9. A. Siddiqui-Jain, C. L. Grand, D. J. Bearss and L. H. Hurley, Proc. Natl. Acad. Sci. U. S. A., 2002, 99, 11593.
10. H. Fernando, A. P. Reszka, J. Huppert, S. Ladame, S. Rankin, A. R. Venkitaraman, S. Neidle and S. Balasubramanian, Biochemistry, 2006, 45, 7854.
11. S. Rankin, A. P. Reszka, J. Huppert, M. Zloh, G. N. Parkinson, A. K. Todd, S. Ladame, S. Balasubramanian and S. Neidle, J. Am. Chem. Soc., 2005, 127, 10584.
12. Z. A. Waller, S. A. Sewitz, S. T. D. Hsu and S. Balasubramanian, J. Am. Chem. Soc., 2009, 131, 12628.
13. J. Dash, P. S. Shirude, S. T. D. Hsu and S. Balasubramanian, J. Am. Chem. Soc., 2008, 130, 15950.
14. K. Jantos, R. Rodriguez, S. Ladame, P. S. Shirude and S. Balasubramanian, J. Am. Chem. Soc., 2006, 128, 13662.
15. M. Bejugam, S. Sewitz, P. S. Shirude, R. Rodriguez, R. Shahid and S. Balasubramanian, J. Am. Chem. Soc., 2007, 129, 12926.
16. S. Sakurai, T. Fukasawa, J. M. Chong, A. Tanaka and M. Fukayama, Cancer Sci., 1999, 90, 1321.
17. A. Yasuda, H. Sawai, H. Takahashi, N. Ochi, Y. Matsuo, H. Funahashi, M. Sato, Y. Okada, H. Takeyama and T. Manabe, Mol. Cancer, 2006, 5, 46.
18. A. McIntyre, B. Summersgill, B. Grygalewicz, A. J. Gillis, J. Stoop, R. J. van Gurp, N. Dennis, C. Fisher, R. Huddart and C. Cooper, et al., Cancer Res., 2005, 65, 8085.
19. M. Miettinen and J. Lasota, Appl. Immunohistochem. Mol. Morphol., 2005, 13, 205.
20. K. S. Smalley, K. L. Nathanson and K. T. Flaherty, Cancer Res., 2009, 69, 3241.
21. C. W. Ong, M. C. Liu, K. D. Lee, K. W. Chang, Y. T. Yang, H. W. Tung and K. R. Fox, Tetrahedron, 2012, 68, 5453.
22. S. Manaye, R. Eritja, A. Aviñó, J. Jaumot and R. Gargallo, Biochim. Biophys. Acta, Gen. Subj., 2012, 1820, 1987.
23. M. Bejugam, M. Gunaratnam, S. Müller, D. A. Sanders, S. Sewitz, J. A. Fletcher, S. Neidle and S. Balasubramanian, ACS Med. Chem. Lett., 2010, 1, 306.
24. K. I. McLuckie, Z. A. Waller, D. A. Sanders, D. Alves, R. Rodriguez, J. Dash, G. J. McKenzie, A. R. Venkitaraman and S. Balasubramanian, J. Am. Chem. Soc., 2011, 133, 2658.
25. J. Dash, R. Nath Das, N. Hegde, G. D. Pantoş, P. S. Shirude and S. Balasubramanian, Chem.–Eur. J., 2012, 18, 55.
26. K. M. Rahman, A. P. Reszka, M. Gunaratnam, S. M. Haider, P. W. Howard, K. R. Fox, S. Neidle and D. E. Thurston, Chem. Commun., 2009, 4097.
27. M. C. Nielsen, A. F. Larsen, F. H. Abdikadir and T. Ulven, Eur. J. Med. Chem., 2014, 72, 119.
28. J. E. Reed, A. A. Arnal, S. Neidle and R. Vilar, J. Am. Chem. Soc., 2006, 128, 5992.
29. X. Wang, C. X. Zhou, J. W. Yan, J. Q. Hou, S. B. Chen, T. M. Ou, L. Q. Gu, Z. S. Huang and J. H. Tan, ACS Med. Chem. Lett., 2013, 4, 909.
30. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, J. A. Montgomery, T. Vreven Jr, K. N. Kudin and J. C. Burant, et al., Gaussian 03, Gaussian, Inc, Wallingford, CT, 2004.
31. A. W. Sousa da Silva and W. F. Vranken, BMC Res. Notes, 2012, 23, 367.
32. J. Wang, R. M. Wolf, J. W. Caldwell, P. A. Kollman and D. A. Case, J. Comput. Chem., 2004, 25, 1157.
33. D. A. Case, T. E. Cheatham, T. Darden, H. Gohlke, R. Luo, K. M. Merz, A. Onufriev, C. Simmerling, B. Wang and R. J. Woods, J. Comput. Chem., 2005, 26, 1668.
34. G. M. Morris, D. S. Goodsell, R. S. Halliday, R. Huey, W. E. Hart, R. K. Belew and A. J. Olson, J. Comput. Chem., 1998, 19, 1639.
35. D. van der Spoel, E. Lindahl, B. Hess, G. Groenhof, A. E. Mark and H. J. C. Berendsen, J. Comput. Chem., 2005, 26, 1701.
36. A. Pérez, I. Marchán, D. Svozil, J. Sponer, T. E. Cheatham, C. A. Laughton and M. Orozco, Biophys. J., 2007, 92, 3817.
37. D. J. Price and C. L. Brooks III, J. Chem. Phys., 2004, 121, 10096.
38. T. Darden, D. York and L. Pedersen, J. Chem. Phys., 1993, 98, 10089.
39. B. Hess, H. Bekker, H. J. C. Berendsen and J. G. Fraaije, J. Comput. Chem., 1997, 18, 1463.
40. G. Bussi, D. Donadio and M. Parrinello, J. Chem. Phys., 2007, 126, 014101.
41. M. Parrinello and A. Rahman, J. Appl. Phys., 1981, 52, 7182.
42. S. Nosé and M. L. Klein, Mol. Phys., 1983, 50, 1055.
43. E. F. Pettersen, T. D. Goddard, C. C. Huang, G. S. Couch, D. M. Greenblatt, E. C. Meng and T. E. Ferrin, J. Comput. Chem., 2004, 25, 1605.
44. N. A. Baker, D. Sept, S. Joseph, M. J. Holst and J. A. McCammon, Proc. Natl. Acad. Sci. U. S. A., 2001, 98, 10037.
45. P. A. Kollman, I. Massova, C. Reyes, B. Kuhn, S. Huo, L. Chong, M. Lee, T. Lee, Y. Duan, W. Wang, O. Donini, P. Cieplak, J. Srinivasan, D. A. Case and T. E. Cheatham, Acc. Chem. Res., 2000, 33, 889.
46. D. Spiliotopoulos, A. Spitaleri and G. Musco, PLoS One, 2012, 7, e46902.
47. C. Paissoni, D. Spiliotopoulos, G. Musco and A. Spitaleri, Comput. Phys. Commun., 2014, 186, 105.
48. B. Jayaram, D. Sprous, M. A. Young and D. L. Beveridge, J. Am. Chem. Soc., 1998, 120, 10629.
49. R. Štefl, T. E. Cheatham, N. A. Špačková, E. Fadrná, I. Berger, J. Koča and J. Šponer, Biophys. J., 2003, 85, 1787.
50. S. Alcaro, C. Musetti, S. Distinto, M. Casatti, G. Zagotto, A. Artese, L. Parrotta, F. Moraca, G. Costa, F. Ortuso, E. Maccioni and C. Sissi, J. Med. Chem., 2013, 56, 843.
51. T. M. Ou, Y. J. Lu, C. Zhang, Z. S. Huang, X. D. Wang, J. H. Tan, Y. Chen, D. L. Ma, K. Y. Wong, J. C. O. Tang, A. S. C. Chan and L. Q. Gu, J. Med. Chem., 2007, 50, 1465.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c5ra13615f

---