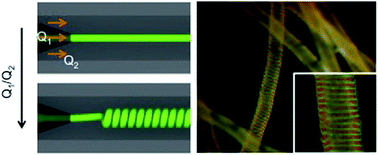
We have studied the instability of viscous liquid threads in less viscous liquids flowing in glass coaxial microchannels. Similar to the behavior of relatively non-viscous liquid threads falling in air, liquid threads in a fluid were found to form not only coils but also two-dimensional folds, depending on the flow rates and viscosities of the fluids. We examined the effect of the flow rate ratio, physical properties of the two fluids, and channel geometries on the liquid thread thickness, pitch, and amplitude of the folding and coiling in the microfluidic channel. Additionally, the viscous liquid threads were used as templates to form hydrogel microfibers by sufficiently delaying the gelation speed of the inner fluids until folds or coils were formed and stabilized.