High pressure effect on the electronic structure and thermoelectric properties of BiCuSeO: first-principles calculations

Abstract

The effects of high pressure on the electronic structure and thermoelectric properties of BiCuSeO have been investigated by using first-principles calculations and the semi-classical Boltzmann transport theory. The electronic band gap increases with increasing pressure, and the band structure near the Fermi level of BiCuSeO is modified by applying hydrostatic pressure. It is found that the electrical conductivity of BiCuSeO can be enhanced under pressure, and such dependence can be explained by the pressure-induced change of the electronic structure of BiCuSeO. Furthermore, the doping dependence of power factors of n- and p-type BiCuSeO at three different pressures are estimated, suggesting that n-type doping of this compound would be more favorable for improving the thermoelectric properties under external pressure.

---

1. Introduction

Thermoelectric materials are receiving increasing attention because they can directly and reversibly convert waste heat into electricity.^1,2 The efficiency of thermoelectric materials is quantified by the dimensionless figure of merit, ZT = S²σT/(κ_e + κ_l), where S, σ, T, κ_e and κ_l are the Seebeck coefficient, electrical conductivity, temperature, electric thermal conductivity and lattice thermal conductivity, respectively. In order to obtain high ZT, one needs to maximize the power factor S²σ, while keeping the thermal conductivity suppressed.

Recently, the layered oxychalcogenide BiCuSeO has been found to exhibit excellent thermoelectric performance in the medium temperature range, which mainly attributes to a very low lattice thermal conductivity of the compound.^3–18 Meanwhile, the figure of merit of this compound is limited by the moderate power factor due to its intrinsically low electronic conductivity,^5–18 suggesting that a strategy to enhance thermoelectric performance ZT of BiCuSeO is to increase its electrical transport properties. In order to increase the electronic conductivity of BiCuSeO, efforts to date have mainly focused on doping at the bismuth site or introducing Cu deficiencies into the crystal.^5–18 Recently, high pressure is suggested to be an effective tool to improve the thermoelectric properties in some thermoelectric semiconductors.¹⁹ The transport properties of some thermoelectric materials under pressure were examined, such as Bi₂Te₃,²⁰ PbTe,²¹ AgSbTe₂,²² Bi₂Sr₂Co₂O₉,²³ and SnSe,²⁴ among others, and remarkable improvements of their thermoelectric properties under pressure were observed.^20–24 On the theoretical side, pressure-induced phase transitions and enhancement of thermoelectric properties have been reported in PbTe.²⁵ Xu et al. investigated the transport properties of PbTe, SnTe, and GeTe under high pressure, and analyzed the possibility of pressure-induced thermoelectric performance enhancement.²⁶ Guo et al. have carried out a comprehensive investigation of the effect of high pressure on the crystal structure, electronic structure, and transport properties of 2H–MoS₂.²⁷ The thermoelectric properties of CoSb₃ and Fe₂VAl were investigated recently using first-principles calculations as well.^28,29 Since high hydrostatic pressure can be an effective way to improve the thermoelectric performance, it is necessary to understand how the high pressure affects the electronic structure and transport properties of BiCuSeO, and to explore the origins of the improvement of the thermoelectric parameters under pressure.

In this paper, the electronic structure of BiCuSeO under high hydrostatic pressure has been investigated using first-principles calculations. The transport properties of BiCuSeO under pressure have been estimated based on semi-classical Boltzmann transport theory, and the influence of pressure on the electronic conductivity is analyzed. The effect of high pressure on the electronic conductivity of BiCuSeO is interpreted by the change of the electronic structure near the Fermi surface. It is expected that applying high hydrostatic pressure can be an effective way to improve the thermoelectric performance of BiCuSeO.

2. Computational method

The electronic structure calculations of BiCuSeO were performed using the projector augment wave method as implemented in Vienna ab initio Simulation Package (VASP).^30–32 The exchange–correlation potential was treated by using the Perdew–Burke–Ernzerhof (PBE)³³ generalized gradient approximation (GGA). The cutoff energy of the plane-wave was set at 400 eV. The energy convergence criterion was chosen to be 10⁻⁶ eV per unit cell, and the forces on all relaxed atoms were less than 0.03 eV Å⁻². It is well known that density functional theory (DFT) cannot accurately describe the electronic structure when strongly correlated systems are considered. To correct this problem, we chose on-site Coulomb interactions (DFT + U) method for calculating electronic structure. As previously reported for some semiconductors,³⁴ the band edge features, which determines the thermoelectric properties, is correct. So, it is reasonably to choose DFT + U method for electrical transport calculations. The band gap of BiCuSeO can be modified by the effective Coulomb repulsion parameter U, while band structure characteristics and thermoelectric properties are not influenced by U parameter much (more discussion in the ESI†). The effective Coulomb repulsion parameter U here was set to be 4 eV based on the published literature of Cu-based ternary semiconductors.³⁴

The eigen-energies for transport properties calculations of BiCuSeO were obtained from ab initio results. In order to get reasonable transport properties, the Brillouin zones of the unit cells were represented by the Monkhorst–Pack special k-point scheme with 31 × 31 × 13 meshes. This provides well-converged transport quantities. Spin–orbit coupling was included in the calculation. The Seebeck coefficient S and electronic conductivity over relaxation time σ/τ were obtained using the semi-classical Boltzmann theory in conjunction with rigid band and constant relaxation time approximations. All the calculations of transport properties were implemented in the BoltzTraP package,³⁵ which are widely used in the theoretical calculations of thermoelectric materials.^25–29,36

3. Results and discussion

BiCuSeO is a member of a family of oxychalcogenides possessing the ZrCuSiAs-type structure with P4/nmm space group, which consists of alternating (Cu₂Se₂)²⁻ chalcogenide layers and (Bi₂O₂)²⁺ oxide layers stacked perpendicularly along the c axis of the tetragonal unit cell.^7,8 The crystal structure of BiCuSeO is shown in Fig. 1. The calculated lattice parameters of BiCuSeO at different pressures are listed in Table 1. It can be seen from Table 1, the calculated lattice constants of BiCuSeO at zero pressure are slightly larger than reported experimental ones,³⁷ and this is due to the problems associated with DFT + U used in our calculations,³⁸ which still cannot entirely describe the exchange–correlation effects of the localized Cu 3d electrons, though the errors here are all within 1%. Meanwhile, it can be found that the lattice parameter decreases with the increase of hydrostatic pressure. Since (Cu₂Se₂)²⁻ chalcogenide layer belongs to the conductive layer in BiCuSeO crystal,¹⁸ and the Cu–Se bond length can be substantially modified under pressure, it will cause substantial change of the transport properties of BiCuSeO.

Fig. 1 Crystal structure of BiCuSeO.

Table 1 The optimized and experimental lattice constants of BiCuSeO at different pressures

	0 GPa	5 GPa	10 GPa
a Ref. 37.
a (Å)	3.939	3.909	3.869
	3.928^a
c (Å)	9.022	8.840	8.633
	8.929^a
dBi–O (Å)	2.346	2.331	2.312
	2.33^a
dBi–Se (Å)	3.246	3.191	3.127
	3.23^a
dCu–Se (Å)	2.518	2.497	2.469
	2.51^a

---

Fig. 2 shows the band structures of BiCuSeO along the symmetry lines at different pressures. As for the electronic structure of BiCuSeO at zero pressure, the band shows an indirect band gap feature as reported,^37–40 that is, the conduction band minimum (CBM) is the Z point and the valence band maximum (VBM) is on the Γ–M line. The calculated energy band gap of BiCuSeO at 0 GPa is 0.38 eV, which is lower than the experimentally estimated value 0.8 eV,³⁷ and this is due to the problems associated with DFT + U used in our calculations, which still cannot entirely describe the exchange–correlation effects of the localized Cu 3d electrons.³⁴ From the upper part of the valence band near the Fermi surface, we can conclude that this material belongs to a multiband structure with several hole pockets located at X–M–Γ line. According to previous reports,^37–40 these hole pockets located at degenerated directions of the Fermi surface determine the electrical conductivity of p-type BiCuSeO. As the pressure increases from 0 to 10 GPa, the band gap of BiCuSeO increases, and these hole pockets around the M point become deeper. Such pressure-induced increase of band gap agrees with previous reports for semiconductors under hydrostatic pressure.^28,41 As these hole pockets near Fermi level become deeper under pressure, the effective hole mass becomes smaller, and it can cause the electrical conductivity of p-type BiCuSeO increase. With the pressure increasing, the conduction-band shifts toward the high energy region, and the conduction-band minimum along the Z–Γ direction becomes steeper. It means that the electron effective mass becomes smaller under pressure. The decrease of the effective mass of conduction-band results in the decrease of the Seebeck coefficient and the increase of the electrical conductivity of n-type semiconductors.⁴² The change of conduction band under pressure will have a great influence on the transport properties of n-type BiCuSeO.

Fig. 2 Calculated band structures of BiCuSeO at different pressures. (a) 0 GPa, (b) 5 GPa (c) 10 GPa. The Fermi levels are set to zero.

In order to further understand the effect of high pressure on electronic structure of BiCuSeO, we plot the total and projected density of states (DOS) at different pressures in the energy interval between −7 eV and 5 eV in Fig. 3. From the DOS at zero pressure, the conduction band near Fermi surface primarily comes from Bi 6p orbitals, and the upper part of the valence band near the Fermi surface is mainly due to the hybridization between Cu 3d and Se 4p orbitals in the Cu–Se antibonding states. As can be seen from Fig. 3, the obvious change of projected DOS of BiCuSeO under pressure lies in that the energy positions of these peaks of Se 4p orbitals near VBM shift to lower energy, suggesting that Se 4p orbitals become localized and the hybridization between Cu 3d and Se 4p orbitals increases. As the Cu–Se antibonding states become more hybridized under pressure, the band gap of BiCuSeO broadens as the pressure increases, and the transport properties of BiCuSeO will be changed under pressure as well. In addition, some changes of Bi 6p orbitals also emerge under pressure: the peaks of Bi 6p orbitals around the CBM reduce from 0 to 10 GPa. Since the conduction band near Fermi level comes from Bi 6p orbitals, the transport properties of n-type BiCuSeO can be tuned by external pressure.

Fig. 3 Calculated total and projected density of states of BiCuSeO at different pressures. (a) 0 GPa, (b) 5 GPa (c) 10 GPa. The Fermi levels are set to zero.

The total DOS of BiCuSeO near Fermi surface at different pressures are shown in Fig. 4. It is well known that the slope of the DOS near the band gap can clearly reflect the transport properties of thermoelectric materials.^42,43 The more flat of the DOS near the Fermi level, the larger of the electronic conductivity. The total DOS at the VBM and CBM both decrease under pressure as shown in Fig. 4, indicating that thermoelectric performance of BiCuSeO can be tuned by applying high pressure.

Fig. 4 Total density of states of BiCuSeO at different pressures with the zero energy set to the valence-band maximum.

To analyze the changes of the electrical conductivity of BiCuSeO under pressure, the partial charge density near Fermi energy at different pressures are shown in Fig. 5 and S3 (ESI†). Fig. 5 shows the partial charge density on the Bi–O–Bi plane in the lower portion of the conduction bands (0 to 2 eV) at different pressures. According to the calculated DOSs, the lower portion of the conduction bands mainly consist of Bi 6p orbitals. From the contour plots of the partial charge density at zero pressure, obvious antibonding characteristics between Bi and Bi atoms can be concluded. It indicates that the conductive network of n-type BiCuSeO is primarily determined by the Bi atoms. As can be seen from Fig. 5, the Bi 6p orbitals become localized with enhanced external pressure, suggesting that the Bi–Bi antibonding states become more strengthen under pressure, and it will lead to electrical conductivity of n-type BiCuSeO increase with increasing pressure. As for p-type BiCuSeO, the Cu–Se antibonding states become more hybridized under external pressure, which makes the electrical conductivity increase with increasing pressure (more discussion in the ESI†).

Fig. 5 Contour plots of the partial charge density in the lower portion of the conduction bands (0–2 eV) of BiCuSeO on the Bi–O–Bi plane at different pressures. (a) 0 GPa, (b) 5 GPa (c) 10 GPa. The Fermi levels are set to zero. The unit of charge density is e/ų.

To assess the thermoelectric performance of BiCuSeO under pressure, transport properties of BiCuSeO as a function of doping at different pressures were calculated by solving Boltzmann transport equation. According to Boltzmann theory, Seebeck coefficients are τ-independent, while the electrical conductivities and power factors have to be presented with respect to relaxation time τ. The electronic transport coefficients of p-type BiCuSeO at different pressures as a function of number of holes per unit cell at 700 K are shown in Fig. 6. As seen in Fig. 6(a), the Seebeck coefficients at three pressures decrease with increased doping levels. At the same doping level, the Seebeck coefficients of p-type BiCuSeO decrease with increasing pressure. As shown in Fig. 6(b), electrical conductivity with respect to relaxation time σ/τ increases under pressure, and significantly enhanced in these heavily doping regions. For example, compared with zeropressure, the value σ/τ of the 15% doped p-type BiCuSeO increased by 61% at 10 GPa. The electrical conductivity of p-type BiCuSeO increases under pressure, which is consistent with the previous analysis of the electronic structure of BiCuSeO under pressure. The power factors with respect to relaxation time S²σ/τ of p-type BiCuSeO under pressure as a function of holes per unit cell are shown in Fig. 6(c). It can be seen that the value S²σ/τ slightly increases under pressure at low doping concentration, while the value S²σ/τ decreases with increasing pressure in heavily doping regions. The power factors decreases with increasing pressure at high carrier concentration, since the enhancement of electrical conductivity cannot compensate the reduction of Seebeck coefficient under pressure.

Fig. 6 Calculated electronic transport coefficients of p-type BiCuSeO at different pressures as a function of number of holes per unit cell at 700 K. (a) Seebeck coefficient S, (b) electrical conductivity with respect to relaxation time σ/τ and (c) power factors with respect to relaxation time S²σ/τ.

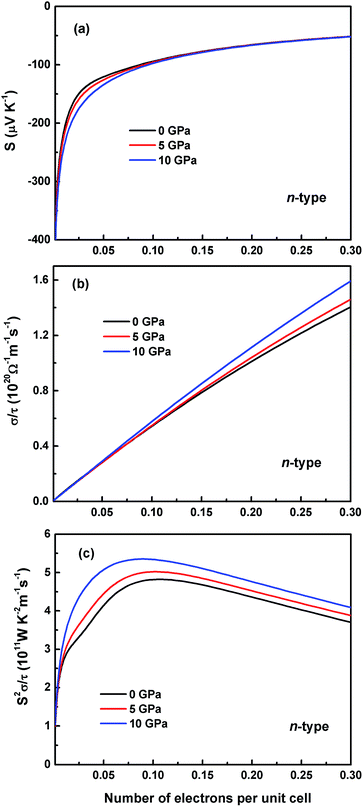
On the other sides, the thermoelectric performance of n-type BiCuSeO under pressure is also assessed. The results of n-type BiCuSeO for the Seebeck coefficient, electrical conductivity and power factors at different pressures as a function of number of electrons per unit cell at 700 K are shown in Fig. 7. As can be seen in Fig. 7(a), pressure reduces the Seebeck coefficient but very modestly, and there is no obvious change at high carrier concentration when applying external pressure. The electrical conductivity of n-type BiCuSeO increases when pressure increases from 0 to 10 GPa, especially at high carrier concentrations, as shown in Fig. 7(b). The electrical conductivity of n-type BiCuSeO increases under pressure, which also agrees well with the conclusion from the analysis of total DOS at the CBM under pressure. Combining the Seebeck coefficient with electrical conductivity, the power factor of n-type BiCuSeO under pressure is shown in Fig. 7(b). As can be seen, the power factor with respect to relaxation time S²σ/τ of n-type BiCuSeO can be enhanced by pressure within the considered doping level range. The power factor S²σ/τ of n-type BiCuSeO can be improved under pressure, while the S²σ/τ of p-doped decreases when BiCuSeO subjected external pressure, suggesting that n-type BiCuSeO should be more suitable for improvement of thermoelectric properties under pressure than that for p-type doping. Though the BiCuSeO compound has been found to exhibit inherent p-type semiconducting behavior at room temperature, it is significant to explore the n-type doping of BiCuSeO in experiment which can be produced by introducing electrons into the system at the Bi site.

Fig. 7 Calculated electronic transport coefficients of n-type BiCuSeO at different pressures as a function of number of holes per unit cell at 700 K. (a) Seebeck coefficient S, (b) electrical conductivity with respect to relaxation time σ/τ and (c) power factors with respect to relaxation time S²σ/τ.

4. Conclusions

In conclusion, the influence of high pressure on the electronic structure and thermoelectric performance of BiCuSeO have been investigated based on first-principles calculations. It is observed from the band structure that these hole pockets located at X–M–Γ line become deeper with increasing pressure. The analysis of projected DOS and partial charge density indicates that the antibonding states near Fermi surface become more hybridized under pressure, which brings about the improvement of the electrical conductivity. The transport properties of BiCuSeO have been estimated based on semi-classic Boltzmann transport theory, and the results show that the electrical conductivity of BiCuSeO can be enhanced by applying external pressure, and n-type BiCuSeO should be more suitable for improvement of thermoelectric properties under pressure than that for p-type doping. These insights can be helpful to understand the origins of the transport properties for BiCuSeO under pressure and offer an alternative route for improving the thermoelectric performance of BiCuSeO.

Acknowledgements

This work was partially supported by Natural Science Foundation of China (Approval nos 11172255, 51172189 and 11325420), National Basic Research Program of China (973 Program, Grant No. 2015CB755500), Scientific Research Fund of Hunan Provincial Education Department (14C0461), SIAT Innovation Program for Excellent Young Researchers (201405), and US National Science Foundation (CMMI-1235535).

References

1. G. J. Snyder and E. S. Toberer, Nat. Mater., 2008, 7, 105.
2. Y. Pei, H. Wang and G. J. Snyder, Adv. Mater., 2012, 24, 6125.
3. F. Li, J.-F. Li, L.-D. Zhao, K. Xiang, Y. Liu, B.-P. Zhang, Y.-H. Lin, C.-W. Nan and H.-M. Zhu, Energy Environ. Sci., 2012, 5, 7188.
4. J.-L. Lan, B. Zhan, Y.-C. Liu, B. Zheng, Y. Liu, Y.-H. Lin and C.-W. Nan, Appl. Phys. Lett., 2013, 102, 123905.
5. J. Li, J. Sui, C. Barreteau, D. Berardan, N. Dragoe, W. Cai, Y. Pei and L.-D. Zhao, J. Alloys Compd., 2013, 551, 649.
6. D. Sun Lee, T.-H. An, M. Jeong, H.-S. Choi, Y. Soo Lim, W.-S. Seo, C.-H. Park, C. Park and H.-H. Park, Appl. Phys. Lett., 2013, 103, 232110.
7. J. Li, J. Sui, Y. Pei, X. Meng, D. Berardan, N. Dragoe, W. Cai and L.-D. Zhao, J. Mater. Chem. A, 2014, 2, 4903.
8. F. Li, T.-R. Wei, F. Kang and J.-F. Li, J. Mater. Chem. A, 2013, 1, 11942.
9. Y.-L. Pei, J. He, J.-F. Li, F. Li, Q. Liu, W. Pan, C. Barreteau, D. Berardan, N. Dragoe and L.-D. Zhao, NPG Asia Mater., 2013, 5, e47.
10. L. D. Zhao, D. Berardan, Y. L. Pei, C. Byl, L. Pinsard-Gaudart and N. Dragoe, Appl. Phys. Lett., 2010, 97, 092118.
11. C. Barreteau, D. Bérardan, E. Amzallag, L. Zhao and N. Dragoe, Chem. Mater., 2012, 24, 3168.
12. J. Li, J. Sui, Y. Pei, C. Barreteau, D. Berardan, N. Dragoe, W. Cai, J. He and L.-D. Zhao, Energy Environ. Sci., 2012, 5, 8543.
13. L. Pan, D. Bérardan, L. Zhao, C. l. Barreteau and N. Dragoe, Appl. Phys. Lett., 2013, 102, 023902.
14. S. D. N. Luu and P. Vaqueiro, J. Mater. Chem. A, 2013, 1, 12270.
15. Y. Liu, J.-L. Lan, B. Zhan, J. Ding, Y. Liu, Y.-H. Lin, B. Zhang, C. Nan and P. F. Poudeau, J. Am. Ceram. Soc., 2013, 96, 2710.
16. Y. Liu, J. Lan, W. Xu, Y. L. Pei, B. Cheng, D. B. Liu, Y. H. Lin and L. D. Zhao, Chem. Commun., 2013, 49, 8075.
17. C. Barreteau, D. Bérardan, L. Zhao and N. Dragoe, J. Mater. Chem. A, 2013, 1, 2921.
18. Y. Liu, L. D. Zhao, J. Lan, W. Xu, F. Li, B. P. Zhang, D. Berardan, N. Dragoe, Y. H. Lin, C. W. Nan, J. F. Li and H. Zhu, J. Am. Chem. Soc., 2011, 133, 20112.
19. S. V. Ovsyannikov and V. V. Shchennikov, Chem. Mater., 2010, 22, 635.
20. D. A. Polvani, J. F. Meng, N. V. Chandra Shekar, J. Sharp and J. V. Badding, Chem. Mater., 2001, 13, 2068.
21. S. V. Ovsyannikov and V. V. Shchennikov, Appl. Phys. Lett., 2007, 90, 122103.
22. T. Su, X. Jia, H. Ma, F. Yu, Y. Tian, G. Zuo, Y. Zheng, Y. Jiang, D. Dong, L. Deng, B. Qin and S. Zheng, J. Appl. Phys., 2009, 105, 073713.
23. F. Chen, K. L. Stokes and R. Funahashi, Appl. Phys. Lett., 2002, 81, 2379.
24. A. Agarwal, P. H. Trivedi and D. Lakshminarayana, Cryst. Res. Technol., 2005, 40, 789.
25. Y. Wang, X. Chen, T. Cui, Y. Niu, Y. Wang, M. Wang, Y. Ma and G. Zou, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 76, 155127.
26. L. Xu, H.-Q. Wang and J.-C. Zheng, J. Electron. Mater., 2011, 40, 641.
27. H. Guo, T. Yang, P. Tao, Y. Wang and Z. Zhang, J. Appl. Phys., 2013, 113, 013709.
28. S. Li, X. Jia and H. Ma, Chem. Phys. Lett., 2012, 549, 22.
29. B. Xu, X. Li, G. Yu, J. Zhang, S. Ma, Y. Wang and L. Yi, J. Alloys Compd., 2013, 565, 22.
30. G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15.
31. G. Kresse and J. Furthmüller, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169.
32. G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 47, 558.
33. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
34. Y. Zhang, X. Yuan, X. Sun, B.-C. Shih, P. Zhang and W. Zhang, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 84, 075127.
35. G. K. H. Madsen and D. J. Singh, Comput. Phys. Commun., 2006, 175, 67.
36. J. Yang, Y. Yan, Y. X. Wang and G. Yang, RSC Adv., 2014, 4, 28714.
37. H. Hiramatsu, H. Yanagi, T. Kamiya, K. Ueda, M. Hirano and H. Hosono, Chem. Mater., 2007, 20, 326.
38. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
39. E. S. Stampler, W. C. Sheets, M. I. Bertoni, W. Prellier, T. O. Mason and K. R. Poeppelmeier, Inorg. Chem., 2008, 47, 10009.
40. D. Zou, S. Xie, Y. Liu, J. Lin and J. Li, J. Mater. Chem. A, 2013, 1, 8888.
41. H.-T. Xue, F.-L. Tang, W.-J. Lu, Y.-D. Feng, Z.-M. Wang and Y. Wang, Comput. Mater. Sci., 2013, 67, 21.
42. D. J. Singh and I. I. Mazin, Phys. Rev. B: Condens. Matter Mater. Phys., 1997, 56, R1650.
43. M.-S. Lee, F. P. Poudeu and S. D. Mahanti, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 83, 085204.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c4ra10073e

---