Carbon dioxide entrapment in an organic molecular host†
Tia
Jacobs
a,
Vincent J.
Smith
a,
Lynne H.
Thomas
b and
Leonard J.
Barbour
*a
aDepartment of Chemistry and Polymer Science, University of Stellenbosch, Matieland, 7602, South Africa. E-mail: ljb@sun.ac.za; Fax: +27 (0)21 808 3849; Tel: +27 (0)21 808 3335
bDepartment of Chemistry, University of Bath, Bath, BA2 7AY, UK
First published on 23rd October 2013
Abstract
4-Phenoxyphenol crystallises to yield discrete ∼60 Å3 cavities that are capable of enclathrating small solvent molecules; the cavities are capped by constricted 6-membered hydrogen-bonded rings and these potential apertures do not appear to facilitate gated porosity when the material is subjected to static CO2 pressure.
One of the main objectives in studies of networked porous materials such as zeolites,1 metal–organic frameworks (MOFs)2 and covalent organic frameworks (COFs)3 is to synthesise new materials with large permanent pores that give rise to correspondingly high surface areas. Indeed, this is also an important goal for porous molecular materials, with the highest reported surface areas currently in the order of 3000 m2 g−1.4 However, it has been shown that molecular crystals without any permanent pores can also exhibit porous behaviour if the host molecules are able to behave in a concerted fashion in order to facilitate gated diffusion of guests.5 We are interested in probing the lower limits of non-gated porosity in organic molecular crystals. That is, when the host system is non-cooperative as a result of possessing structural features (e.g. stable packing arrangements or strong intermolecular interactions) that prohibit the dynamic behaviour required for a gate-opening mechanism, what is the smallest aperture through which gas molecules may pass? This question has been partially addressed by the formulation of kinetic diameters1 for various guests, but a tantalising study of Dianin's compound has challenged this concept.
Dianin's compound (1, 4-p-hydroxyphenyl-2,2,4-trimethyl-chroman; Scheme 1) was first synthesised nearly a century ago6 and is an iconic example of an organic host system.7 The racemic mixture generally crystallises in the high symmetry space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) , where six molecules are arranged about a site of
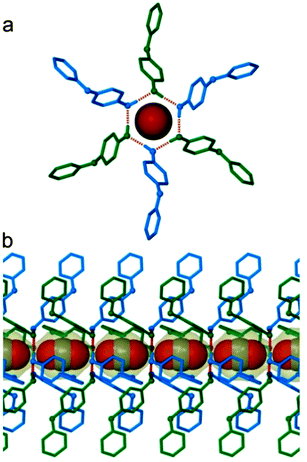
, where six molecules are arranged about a site of ![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) symmetry to form a hydrogen-bonded ring. This ⋯O–H⋯O–H hydrogen bonded ring is formed by the phenolic groups of three pairs of enantiomers, where alternating molecules extend towards opposites sides of the plane of the slightly distorted hexagonal ring (Fig. 1a). This hydrogen-bonded motif is well-established as the dominant structure-directing feature of 1 and it persists in a large number of its known clathrates, as well as in its apohost form.7 These hydrogen-bonded units stack in columns to produce hourglass shaped cavities capped at two ends by the hydrogen-bonded rings (Fig. 1c). These rings appear to serve as apertures that link successive cavities along [001] to form one dimensional channels.
symmetry to form a hydrogen-bonded ring. This ⋯O–H⋯O–H hydrogen bonded ring is formed by the phenolic groups of three pairs of enantiomers, where alternating molecules extend towards opposites sides of the plane of the slightly distorted hexagonal ring (Fig. 1a). This hydrogen-bonded motif is well-established as the dominant structure-directing feature of 1 and it persists in a large number of its known clathrates, as well as in its apohost form.7 These hydrogen-bonded units stack in columns to produce hourglass shaped cavities capped at two ends by the hydrogen-bonded rings (Fig. 1c). These rings appear to serve as apertures that link successive cavities along [001] to form one dimensional channels.
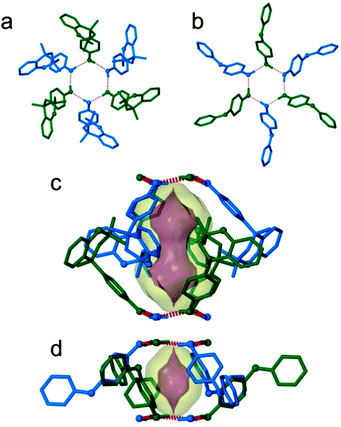 | ||
| Fig. 1 Projections along [001] of the hydrogen-bonded hexamers of (a) 1·vac·293K and (b) 2·vac·293K. Alternating molecules about the hydrogen-bonded ring are coloured blue (down) and green (up). The cavities formed by (c) 1 and (d) 2 viewed perpendicular to [001]. The transparent yellow surfaces define contact volumes of 226 and 68 Å3, respectively (using a probe of radius 1.6 Å) while the purple surfaces represent the accessible surfaces for the smallest spheres that cannot pass through the hydrogen bonded rings (1.249 Å for 1 and 1.174 Å for 2).15 The molecules are shown in capped-stick representation, with oxygen atoms shown as spheres of arbitrary radius. Hydrogen-bonds are shown as fragmented red cylinders and all hydrogen atoms not engaged in hydrogen-bonding have been omitted for clarity. | ||
In 1976 Barrer and Shanson8 described the porosity of Dianin's compound towards various gases such as argon, krypton, xenon, carbon dioxide and methane, stating that the “behaviour of Dianin's compound was in these respects the same as that of a zeolite … the framework of the host structure is however less rigid than that of a zeolite and guest molecules can penetrate into the cavities, even though wide windows giving access to these cavities do not exist”. Although this study predated the sudden surge of reports of synthetic non-zeolitic porous systems that started two decades later, it has largely been ignored, having accumulated only around 37 citations to date. Indeed, to the best of our knowledge, no follow-up study of this important observation has been undertaken as yet. There exist in the literature several analogues of Dianin's compound – compounds that form cyclic (O–H)6 hydrogen-bonded motifs and that have been shown to enclathrate solvent guest molecules in their interstitial cavities. Examples include endo-4,endo-8-dimethylbicyclo[3.3.1]-nonane-endo-2,endo-6-diol,9 chiral propargylic alcohols,10 β-hydroquinone,11 phenol12 and 4-phenoxyphenol.13
As part of our ongoing studies of the solid-state supramolecular chemistry of Dianin's compound,14 we have extended our investigations to include 4-phenoxyphenol (2, see Scheme 1), which bears a strikingly close structural resemblance to 1 despite being achiral. Compound 2 also crystallises in the space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) , with six molecules arranging themselves about a site of
, with six molecules arranging themselves about a site of ![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) symmetry to form a hydrogen-bonded ring (Fig. 1b). These units also stack to form cavities, but as a result of the decreased size and somewhat different shape of the molecules, the cavities are significantly smaller (Fig. 1d) relative to those of 1. A recent report13 on the enclathration of small solvents of crystallisation (i.e. acetonitrile and methanol) by 2 showed that larger solvents such as diethyl-ether are not included but rather yield the “empty” apohost form. The study also showed that methanol could only be removed from the cavity upon melting or sublimation of the host.
symmetry to form a hydrogen-bonded ring (Fig. 1b). These units also stack to form cavities, but as a result of the decreased size and somewhat different shape of the molecules, the cavities are significantly smaller (Fig. 1d) relative to those of 1. A recent report13 on the enclathration of small solvents of crystallisation (i.e. acetonitrile and methanol) by 2 showed that larger solvents such as diethyl-ether are not included but rather yield the “empty” apohost form. The study also showed that methanol could only be removed from the cavity upon melting or sublimation of the host.
| Crystallisation conditions | Sublimed (vacuum) | Sublimed (vacuum) | Sublimed (200 mbar CO2) | Sublimed (2 bar CO2) | Supercritical CO2 |
|---|---|---|---|---|---|
| a/Å | 29.2571(16) | 29.0785(18) | 29.000(4) | 28.906(10) | 28.908(6) |
| c/Å | 5.9378(3) | 5.8540(4) | 5.8505(8) | 5.862(2) | 5.8899(12) |
| Unit cell volume/Å3 | 4401.7(4) | 4286.7(5) | 4260.9(10) | 4242(3) | 4262.6(15) |
| Temperature/K | 293(2) | 100(2) | 100(2) | 100(2) | 100(2) |
| Electron count/e− | 4 | 2 | 7 | 16 | 23 |
| %CO2 | 0 | 0 | 32 | 73 | 100 |
As an extension of that study we have investigated the permeability of 2 to various gases (carbon dioxide in particular) (Table 1). To this end, diffraction-quality single crystals of 2 were grown by subliming pure host material at 78 °C under vacuum. A suitable crystal was placed under static vacuum in an environmental gas cell16 and single-crystal diffraction (SCD) data were collected at 293 K. After elucidation of the structure (2·vac·293K), summing electron densities using the SQUEEZE routine of PLATON17 indicated the presence of only four electrons within the guest-accessible cavity. The electron count decreased to two electrons when intensity data were recollected at 100 K (2·vac·100K). Using widely accepted van der Waals radii18 and the equilibrium atomic coordinates of the structure determined at 293 K, we calculate15 that the largest sphere that can pass through the hydrogen bonded ring has a radius of 1.174 Å. Similar analysis of the cavity (using a probe of radius 1.60 Å) reveals a free-space volume of 68 Å3 for 2·vac·293K or 64 Å3 for 2·vac·100K. Considering the many examples of gate-opening mechanisms in molecular crystals, as well as the reported porosity of 1, we continued to investigate the possibility that 2 may exhibit gas permeability despite its very narrow “pore”. Gas sorption experiments were carried out gravimetrically at room temperature and pressures up to 20 bar with nitrogen, carbon dioxide, hydrogen and helium as potential sorbates. In all cases, uptake of these gases under the specified conditions (see ESI†) was negligible.
Materials with narrow pores that are seemingly inaccessible to gases such as hydrogen and nitrogen have often been shown to absorb CO2 as a result of its high polarizability.19 To further test the permeability of 2 towards CO2, the crystal in the environmental gas cell was exposed to ca. 10 bar of CO2 for 20 hours, after which intensity data were recollected; no appreciable increase in electron density was detected within the cavity. In order to offset possible kinetic effects, the crystal was then kept under a constant atmosphere of 10 bar of CO2 for a further 6 days after which redetermination of the structure still yielded no evidence of gas uptake. It therefore appears that, under the conditions investigated, the “empty” apohost crystals obtained by sublimation do not absorb CO2 molecule into their cavities. Our study was then expanded in order to explore the converse – that if CO2 is trapped during the crystal assembly process, as is the case for the growth of a solvate, it is not possible for the guest to escape spontaneously. 2 was sublimed under a CO2 pressure of 200 mbar to produce needle-shaped crystals that were subjected to SCD analysis. The resulting electron count of 7 e− is consistent with partially occupied cavities that each contain, on average, 0.32 molecules of carbon dioxide. Repetition of the experiment using a CO2 pressure of 2 bar yielded 16 electrons per cavity, corresponding to an occupancy of 0.73 guest molecules per cavity. In both structures the CO2 atoms were located in difference electron density maps and refined with their site occupancies constrained according to their respective electron counts. In order to obtain crystals with fully occupied cavities, we ultimately resorted to using supercritical carbon dioxide as the crystallisation solvent. Crystals suitable for SCD analysis (2·CO2, Fig. 2) were grown by slow cooling of a solution of 2 in supercritical CO2, initially at 220 bar and 40 °C; summation of the electron density within the confines of the cavity yielded 23 e− (22 e− expected). The asymmetric unit contains half of the CO2 molecule, with the carbon atom situated on the ![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) site at the centre of the cavity and the oxygen atom located along the 3-fold axis. In our model the unconstrained C
site at the centre of the cavity and the oxygen atom located along the 3-fold axis. In our model the unconstrained C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O bond lengths of the 0.73 and fully occupied structures are 1.148(5) and 1.149(2) Å, respectively; these values are in good agreement with the reported length of 1.155(1) Å in a structure of pure CO2 determined at 150 K.20 Using a probe of radius 1.6 Å, the guest-accessible volume in 2·CO2 is calculated to be 77 Å3, representing a ca. 13% increase in cavity volume relative to that in 2·vac·100K. There appear to be no strong intermolecular interactions between host and guest in 2·CO2 and the closest contact present is that between the guest oxygen atom and the hydrogen-bonded ring of 2: O⋯O = 3.251(2) Å. Thermogravimetric analysis shows that desorption of CO2 is concomitant with melting of the host (m.p. 84 °C).21 An initial weight loss of 3.24% is observed below 100 °C while a weight loss of 3.79% is expected for a 6
O bond lengths of the 0.73 and fully occupied structures are 1.148(5) and 1.149(2) Å, respectively; these values are in good agreement with the reported length of 1.155(1) Å in a structure of pure CO2 determined at 150 K.20 Using a probe of radius 1.6 Å, the guest-accessible volume in 2·CO2 is calculated to be 77 Å3, representing a ca. 13% increase in cavity volume relative to that in 2·vac·100K. There appear to be no strong intermolecular interactions between host and guest in 2·CO2 and the closest contact present is that between the guest oxygen atom and the hydrogen-bonded ring of 2: O⋯O = 3.251(2) Å. Thermogravimetric analysis shows that desorption of CO2 is concomitant with melting of the host (m.p. 84 °C).21 An initial weight loss of 3.24% is observed below 100 °C while a weight loss of 3.79% is expected for a 6![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 host
1 host![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) guest ratio.
guest ratio.
We have shown that 4-phenoxyphenol, which possesses pore windows that are narrower than the kinetic diameter of the smallest gas, does not appear to be permeable to any of the gases under the conditions investigated. Neither does it display any dynamic behaviour, as is occasionally observed in other organic molecular crystals, even to the highly polarizable CO2 (which has previously been shown to diffuse through surprisingly small pores). We attribute this non-porosity and non-cooperativity within the crystals to the rigidity of the hexameric hydrogen-bonded pore that guards the entrance to the cavity. Owing to its lack of porosity, 2 has the ability to non-reversibly trap CO2 guest molecules within its interstices when crystals are grown in the presence of a CO2 rich medium. The problems of substantiating claims of porosity in molecular crystals have recently been discussed,22 but this work highlights the fact that the converse is also true: how do we unequivocally establish non-porosity in a molecular crystal with lattice voids? Is it possible to prove that a material is non-porous or is it merely a question of “guilty until proven innocent”?
Notes and references
- D. W. Breck, Zeolite Molecular Sieves, John Wiley & Sons Inc., New York, 1974 Search PubMed.
- O. M. Yaghi, M. O'Keeffe, N. W. Ockwig, H. K. Chae, M. Eddaoudi and J. Kim, Nature, 2003, 423, 705 CrossRef CAS PubMed.
- A. P. Cote, A. I. Benin, N. W. Ockwig, M. O'Keeffe, A. J. Matzger and O. M. Yaghi, Science, 2005, 310, 1166 CrossRef CAS PubMed.
- M. Mastalerz and I. M. Oppel, Angew. Chem., Int. Ed., 2012, 51, 5252 CrossRef CAS PubMed.
- (a) J. L. Atwood, L. J. Barbour and A. Jerga, Science, 2002, 296, 2367 CrossRef CAS PubMed; (b) J. L. Atwood, L. J. Barbour, A. Jerga and B. L. Schottel, Science, 2002, 298, 1000 CrossRef CAS PubMed; (c) S. J. Dalgarno, P. K. Thallapally, L. J. Barbour and J. L. Atwood, Chem. Soc. Rev., 2007, 36, 236 RSC.
- A. P. Dianin, J. Russ. Phys.-Chem. Soc., 1914, 31, 1310 Search PubMed.
- (a) W. Baker, A. J. Floyd, J. F. W. McOmie, G. Pope, A. S. Weaving and J. H. Wild, J. Am. Chem. Soc., 1956, 2010 CAS; (b) J. L. Flippen, J. Karle and I. L. Karle, J. Am. Chem. Soc., 1970, 92, 3749 CrossRef CAS; (c) F. Imashiro, M. Yoshimura and T. Fujiwara, Acta Crystallogr., Sect. C, 1998, 54, 1357 Search PubMed.
- R. M. Barrer and V. H. Shanson, J. Chem. Soc., Chem. Commun., 1976, 333 RSC.
- V. T. Nguyen, R. Bishop, I. Y. H. Chan, D. C. Craig and M. L. Scudder, CrystEngComm, 2008, 10, 1810 RSC.
- M. Hyacinth, M. Chruszcz, K. S. Lee, M. Sabat, G. Gao and L. Pu, Angew. Chem., Int. Ed., 2006, 45, 5358 CrossRef CAS PubMed.
- T. C. W. Mak and B. R. F. Bracke, in Comprehensive Supramolecular Chemistry, ed. J. L. Atwood, J. E. D. Davies, D. D. MacNicol and F. Vögtle, Pergamon, Oxford, 1996, vol. 6, p. 23 Search PubMed.
- L. Mandelcorn, Chem. Rev., 1959, 59, 827 CrossRef CAS.
- L. H. Thomas, E. Cheung, A. O. F. Jones, A. A. Kallay, M.-H. Lemee-Cailleau, G. J. McIntyre and C. C. Wilson, Cryst. Growth Des., 2012, 12, 1746 CAS.
- (a) E. J. C. de Vries, M. W. Bredenkamp, T. Jacobs and G. O. Lloyd, Acta Crystallogr., Sect. E, 2005, 61, O2871 CAS; (b) C. Esterhuysen, M. W. Bredenkamp and G. O. Lloyd, Acta Crystallogr., Sect. C, 2005, 61, O32 Search PubMed; (c) G. O. Lloyd and M. W. Bredenkamp, Acta Crystallogr., Sect. E, 2005, 61, O1512 CAS; (d) G. O. Lloyd, M. W. Bredenkamp and L. J. Barbour, Chem. Commun., 2005, 4053 RSC; (e) T. Jacobs, G. O. Lloyd and M. W. Bredenkamp, Acta Crystallogr., Sect. E, 2006, 62, O4400 CAS; (f) G. O. Lloyd, J. Alen, T. Jacobs, M. Bredenkamp and E. J. C. de Vries, Acta Crystallogr., Sect. E, 2006, 62, O691 CAS; (g) T. Jacobs and M. W. Bredenkamp, Acta Crystallogr., Sect. E, 2007, 63, O4444 CAS; (h) T. Jacobs, M. W. Bredenkamp and E. J. C. de Vries, Acta Crystallogr., Sect. E, 2007, 63, 03736 Search PubMed; (i) T. Jacobs, G. O. Lloyd, M. W. Bredenkamp and L. J. Barbour, Cryst. Growth Des., 2009, 9, 1284 CrossRef CAS; (j) T. Jacobs, G. O. Lloyd, M. W. Bredenkamp and L. J. Barbour, CrystEngComm, 2009, 11, 1545 RSC; (k) T. Jacobs, M. W. Bredenkamp, P. H. Neethling, E. G. Rohwer and L. J. Barbour, Chem. Commun., 2010, 46, 8341 RSC.
- M. L. Connolly, Science, 1983, 221, 709 CAS.
- D. S. Yufit and J. A. K. Howard, J. Appl. Crystallogr., 2005, 38, 583 CrossRef CAS.
- A. L. Spek, J. Appl. Crystallogr., 2003, 36, 7 CrossRef CAS.
- A. Bondi, J. Phys. Chem., 1964, 68, 441 CrossRef CAS.
- (a) M. P. Suh and D. H. Hong, Chem. Commun., 2012, 48, 9168 RSC; (b) J. Rabone, Y. F. Yue, S. Y. Chong, K. C. Stylianou, J. Bacsa, D. Bradshaw, G. R. Darling, N. G. Berry, Y. Z. Khimyak, A. Y. Ganin, P. Wiper, J. B. Claridge and M. J. Rosseinsky, Science, 2010, 329, 1053 CrossRef CAS PubMed.
- A. Simon and K. Peters, Acta Crystallogr., Sect. B, 1980, 36, 2750 CrossRef.
- CRC Handbook of Chemistry and Physics, ed. D. R. Lide, 88th edn, CRC Press, 2007–2008 Search PubMed.
- L. J. Barbour, Chem. Commun., 2006, 1163 RSC.
Footnote |
| † Electronic supplementary information (ESI) available: Crystallographic data, thermogravimetric analysis, powder X-ray diffraction and gas sorption isotherms. CCDC 959280–959284. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c3cc46784h |
| This journal is © The Royal Society of Chemistry 2014 |