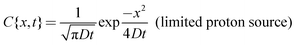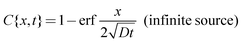Microchannel-induced change of chemical wave propagation dynamics: importance of ratio between the inlet and the channel sizes

Abstract

The ability to control chemical wave propagation dynamics could stimulate the science and technology of artificial and biological spatiotemporal oscillating phenomena. In contrast to the conventional chemical approaches to control the wave front dynamics, here we report a physical approach to tune the propagation dynamics under the same chemical conditions. By using well-designed microchannels with different channel widths and depths, the propagation velocity was successfully controlled based on two independent effects: (i) a transition in the proton diffusion mode and (ii) the formation of a slanted wave front. Numerical analysis yielded a simple relationship between the propagation velocity and the microchannel configuration, which offers a simple and general way of controlling chemical wave propagation.

---

The science of chemical waves offers basic understanding of biological spatiotemporal oscillating phenomena such as signal propagation in neural networks and formation of Turing patterns.¹ In biological systems, one important factor that defines each peculiarity and functionality is the propagation velocity or the oscillation rate. In other words, diversity in the propagation velocity brings biodiversity. Exploring the origin of the diversity in the propagation dynamics is a promising way of understanding complicated biological oscillating phenomena. Additionally, chemical wave technology has allowed the development of new functional systems such as information devices,^2,3 micropatterning,⁴ microfreight,⁵ and image processing.⁶ In these technological applications, the propagation velocity is a critical parameter that governs performance, and enhancements in controllability can improve their functionality. Thus, from both scientific and technological viewpoints, it is a critical task to understand and control the chemical wave propagation dynamics for further development.

A chemical approach to control chemical wave propagation dynamics has long been explored by many researchers. The simplest approach has been to investigate various chemical species to induce the chemical wave, e.g., systematic research has been reported that compared the proton wave velocity induced by reactions between S₂O₃²⁻, S₄O₆²⁻, S₂O₄²⁻, SO₃²⁻, S₂O₆²⁻ and N₂H₅⁺ with BrO₃⁻, IO₃⁻, ClO₃⁻, ClO₂⁻, and S₂O₈²⁻.⁷ After fixing the species to be used, tuning the concentration of each component is a next step to design the propagation velocity.^8–11 To accelerate the propagation velocity, propagation orientation is also an effective parameter, in which either upward^9–11 or downward^8,10,12–14 propagation can be promoted by convection that can accelerate the chemical reaction at the extended wave front. A recent study reported successful velocity control using water-in-oil microemulsions; the origin of control was explained as an effect of emulsion on the rates and equilibria of chemical reactions.¹⁵ Thus, these chemical parameters were capable of controlling the propagation velocity via tuning the chemical reaction kinetics.

However, it is well-known that chemical wave propagation is governed by the reaction–diffusion equation:

where R, D, and c account for the chemical reaction rate, diffusion coefficient, and concentration of the chemical species of interest, respectively.¹⁶ The above-mentioned chemical approaches enable control of the wave propagation via tuning the chemical reaction rate term R. If it were possible to tune diffusion behavior under the same chemical conditions, we could regulate propagation dynamics through independent chemical and physical parameters, which would strengthen the flexible control of chemical wave propagation. To address this situation, we have focused on the shape of the propagation channel by using well-designed micro-channels to control the diffusivity by changing the configuration. We used straight-type microchannels with 2 mm-diameter pores at each end for the solution inlet/outlet (Fluidware Technologies Inc., Japan) (Fig. 1). Aqueous solutions containing 70 mM Na₂S₂O₃, 100 mM KBrO₃, 2 mM NaOH, and a small amount of methyl orange (pH indicator) were introduced into the microchannel. Then, 1 μl of aqueous 100 mM H₂SO₄ solution was added to the inlet to initiate proton wave propagation, according to previously reported protocol for well-controlled pH wave propagation.⁷ All the experiments were done in a temperature-controlled room at 25 °C. After the H₂SO₄ addition, the inlet and the outlet were sealed. However, no change was observed in the propagation characteristics between the sealed and unsealed systems. Propagation of the proton wave was visualized by the pH indicator color change (Fig. 2). Since it requires several durations (typically within a few minutes) for the proton wave to propagate after the H₂SO₄ addition, we defined time = 0 as the time at which the low pH region that can be defined by the color change as described below propagates into the channel. Macroscopic fluid flow caused by the droplet addition settles down within one minute (see the ESI,† Fig. S1) and reaches equilibrium with zero flow, we can ignore the macroscopic flow effect on the pH wave propagation dynamics.

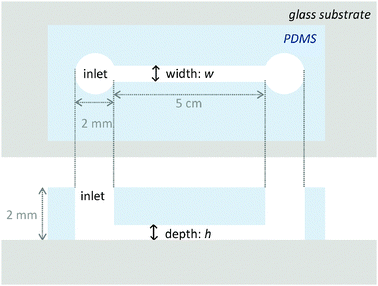Fig. 1 Schematic illustration of a straight-type microchannel with 2 mm-diameter pores at both ends for solution inlet/outlet.

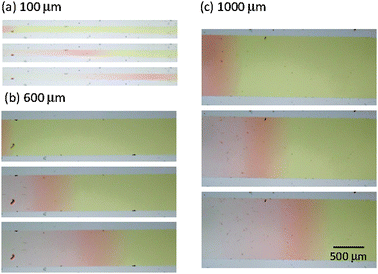Fig. 2 Snapshots of propagating proton waves in microchannels with (a) 100 μm, (b) 600 μm, and (c) 1000 μm widths. Photos are taken every 2 min. The inlet is positioned at the left side of each microchannel.

Since pH of the original solution before adding H₂SO₄ is ca. 11, the initial solution in the microchannels exhibited pale yellow colour. On the analogy of iodate–sulfite–thiosulfate system,¹⁹ transient formation of bisulfite takes place under initial high pH conditions.

2BrO₃⁻ + 3S₂O₃²⁻ + 3H₂O → 2Br⁻ + 6HSO₃⁻ (1)

The addition of proton (H₂SO₄) initiates the oxidation of bisulfate ions.

BrO₃⁻ + 3HSO₃⁻ + H⁺ → Br⁻ + 3SO₄²⁻ + 4H⁺ (2)

The process (2) is autocatalytic and the presence of a small amount of proton increases proton concentration rapidly, which induces a rapid decrease in pH. In the present system, the addition of H₂SO₄ initiates the autocatalytic reaction (2) and causes a rapid pH decrease at the inlet region. A pH decrease changes the color of solution near the inlet region (left side in Fig. 2) to red, indicating pH of this region becomes less than ca. 4. Autocatalytically produced proton diffuses to the nearby region with low proton concentration by a proton concentration gradient, which leads to the propagation of autocatalytic reaction. As such, the region with high proton concentration, i.e. the low pH region propagates like waves in the microchannel as shown in Fig. 2. After H₂SO₄ addition, the red region indicating low pH (less than 4) propagates from left to right in any channel with different width. Brightness of red color kept almost constant in the course of propagation. If simple diffusion of proton from the inlet to the microchannel were occurred, the red color should be highest at the inlet and gradually weakens toward the microchannel. Thus, the observed propagation of red color, i.e., propagation of high concentration proton, is a clear indication of the pH wave appearance under the present conditions at any channel width. Snapshot near the inlet clearly demonstrated that the color change corresponding to the pH change actually started in the inlet and propagated to the microchannel (see the ESI,† Fig. S2). The propagation length as a function of time is shown in Fig. 3 for various microchannel widths. As can be clearly seen, propagation velocity (v) increases as the channel width (w) decreases from 2000 μm to 100 μm. In contrast to the w-dependence of v, the width of propagating front that would anti-correlate with the characteristic time τ for chemical reactions in v ≈ (D/τ)^0.5 did not show clear w dependence (see the ESI,† Fig. S3). Thus, we can assume that only the diffusion term D would cause w-dependent propagation dynamics.

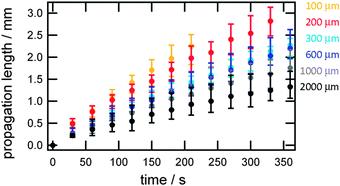Fig. 3 Proton wave propagation length in microchannels with different widths ranging from 100 μm (yellow) to 2000 μm (black).

The classical equation, v ≈ (D/τ)^0.5, indicates a dependence of v on D of the reaction species.¹⁷ In general, D is expressed as a function of viscosity of medium η

where k_B, T, and r are the Boltzmann constant, temperature, and size of diffusing species, respectively. Thus, one possibility to explain the observed w dependence is a change in the water viscosity η in the micro-scale confined space. However, this possibility can be ruled out because η increases only when the channel size is decreased below the submicron scale, and even the smallest of channels investigated in the present systems are much larger than this cutoff.¹⁸

While η and D can be assumed to be invariant over the size scale of our systems, influx of proton from the inlet to the microchannel can induce spatiotemporal dependence of the proton concentration gradient near the inlet, which may alter D∇²c in eqn (1) even if D is constant. We consider two extreme conditions: (i) diffusion from the inlet as a limited proton source and (ii) diffusion from the inlet as an infinite proton source. The former situation can be applied to wider channels, and the latter to narrower channels. The widest microchannel in the present system (w = 2000 μm) has the same width as the inlet (Fig. 4a). In this case, protons at the inlet are continuously depleted by diffusion into the microchannel. Thus, [H⁺] at the inlet is decreasing continuously with time, which allows us to assume the inlet as a limited proton source. On the other hand, the width of the narrowest microchannel (w = 100 μm) is one-twentieth of the inlet (Fig. 4b). Therefore, proton depletion at the entrance of the microchannel due to diffusion into the channel is rapidly supplemented by the vast proton reservoir in the inlet. Thus, the [H⁺] change at the inlet is relatively slow and the inlet can be assumed to be an infinite proton source. We calculated changes in the [H⁺] profile in the microchannels with time using the following equations.

It is clear that the [H⁺] rapidly decreases and the [H⁺] gradient vanishes at early stages of diffusion in the wider channel, whereas [H⁺] near the inlet remains high for long periods in the narrower channel (Fig. 4). This enables the narrower channels to promote and sustain a large proton influx from the inlet to the channel. Since the proton wave is initiated by an influx of protons to the reactive medium,¹⁹ the swift proton influx can propagate the proton wave rapidly. This quick onset provides an explanation for faster observed proton wave propagation in the narrower microchannels as shown in Fig. 3. Since the assumption that the proton diffuses from the limited and infinite source at wide and narrow channels, respectively, is an extreme approximation, we need further numerical treatment such as a weighted average of these two extreme equations. These numerical treatments should give physico-chemical insight into the w-dependent D∇²c term, and thus the w-dependent proton wave propagation. We will further investigate from both experimental and numerical approaches, and will report elsewhere.

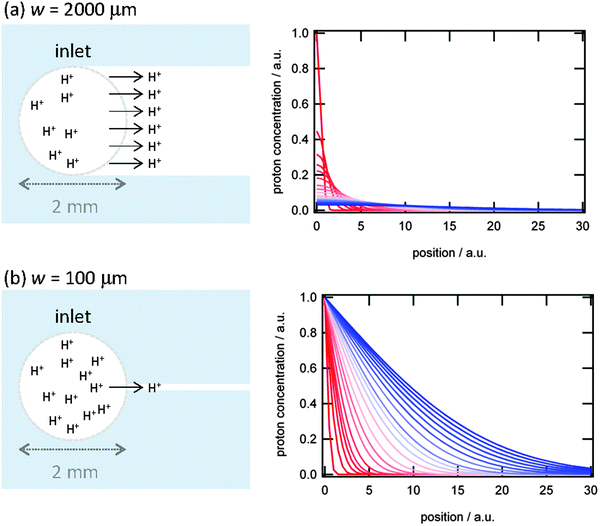Fig. 4 (left) Diffusion of protons from the inlet into the microchannels with different channel width. (right) Change in proton concentration profiles with time from red to blue assuming diffusion from (a) a limited proton source and (b) an infinite proton source.

To quantitatively formularize the channel size dependence, the propagation velocities were evaluated with various channel widths and depths (h) (Fig. 5). As was shown in Fig. 3, the propagation velocity increases with decreasing w. This characteristic feature was observed for both deep (h = 100 μm) and shallow (h = 50 μm) channels. With the same w, the propagation velocity increased in the deeper channel. This behavior is consistent with previous reports revealing that deeper glass capillaries exhibited faster chemical wave propagation because of the formation of slanted wave fronts that can increase the reaction interface area.⁹ In the present system, we also observed slanted wave fronts (see Fig. S4 in the ESI†). From data for each channel depth, we derived the following relationships between the propagation velocity and the channel width:

v(μm s⁻¹) = 135 × w^−0.5 (h = 100 μm)

v(μm s⁻¹) = 59 × w^−0.5 (h = 50 μm)

The inverse square root dependence on w describes the gradual transition from the limited source diffusion mode at large w to the infinite source diffusion mode at small w. The h-dependent pre-factor (135 for h = 100 μm and 59 for h = 50 μm) corresponds to the ability to form a slanted wave front. The deeper channel has a larger effect on the formation of a slanted wave front that can accelerate the wave propagation,⁹ which appears as a larger pre-factor. This simple expression v = C(h) × w^−0.5 enables easy design toward tailor-made microchannels that will elicit chemical wave propagation at a desired velocity. The simple relationship v = C(h) × w^−0.5 may have important physical implication on the effect of microchannels on the reaction–diffusion system. Our on-going numerical treatments could clarify the significance and the origin of this relation. Our model experiments offer the possibility for fine control of propagation dynamics by taking advantage of microfluidics technology, and will enable new functionality with the use of well-designed microfluidic chemical wave propagation. Of course, chemical conditions, such as chemical species and concentration, can be used as another independent control parameter (see Fig. S5 in the ESI†). The combination between physical and chemical conditions will allow versatile and flexible system design. This is an intrinsic feature of the chemical wave propagation that is described with eqn (1). Additionally, our model suggests a dependence of the propagating velocity on the steric confinement of diffusing species, which could have important implications for biological signal propagation such as a nerve pulse that has long been treated as one of the typical reaction–diffusion mechanism, where transport of nerve pulse propagates through axon with different sizes and shapes in living organism.²⁰

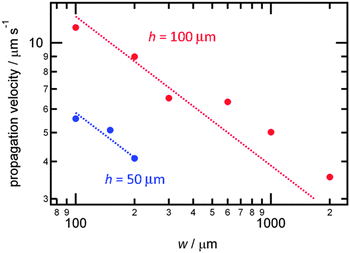Fig. 5 Proton wave propagation velocity as a function of the microchannel width with 100 μm (red) and 50 μm (blue) channel depth. Dotted lines are the least-square fitting results.

In conclusion, we have demonstrated that the width and depth of microchannels can be used as controlling parameters for the propagation of chemical wave velocity. The width dependence comes from a ratio of sizes between the inlet as a proton reservoir and the channel as a proton propagator. Propagation velocity was found to be expressed with a simple equation as a function of the channel width and depth, which offers a simple and general way of controlling chemical wave propagation. The present findings unlock a new frontier of chemical wave science and technology that could also help gain insight into oscillating biological phenomena.

Acknowledgements

This work was partially supported by the Hatakeyama Foundation, The Kao Foundation for Arts and Sciences, and Grants-in-Aid for Scientific Research 22655001 from the Ministry of Education, Culture, Sports, Science, and Technology, Japan.

References

1. (a) I. R. Epstein and J. A. Pojman, An Introduction to Nonlinear Chemical Dynamics, Oxford University Press, 1998; (b) A. GoldBeter, Biochemical Oscillations and Cellular Rhythms, Cambridge University Press, 2004.
2. O. Steinbock, P. Kettunen and K. Showalter, J. Phys. Chem., 1996, 100, 18970–18975.
3. K. Agladze, R. R. Aliev, T. Yamaguchi and K. Yoshikawa, J. Phys. Chem., 1996, 100, 13895–13897.
4. R. Klajn, M. Fialkowski, I. G. Bensemann, A. Bitner, C. J. Campbell, K. Bishop, S. Smoukov and B. A. Grzybowski, Nat. Mater., 2004, 3, 729–735.
5. T. Ichino, T. Asahi, H. Kitahata, N. Magome, K. Agladze and K. Yoshikawa, J. Phys. Chem. C, 2008, 112, 3032–3035.
6. L. Kuhnert, K. I. Agladze and V. I. Krinsky, Nature, 1989, 337, 244–247.
7. L. Szirovicza, I. Nagypál and E. Boga, J. Am. Chem. Soc., 1989, 111, 2842–2845.
8. J. A. Pojman, I. P. Nagy and I. R. Epstein, J. Phys. Chem., 1991, 95, 1306–1311.
9. J. A. Pojman, I. R. Epstein, T. J. McManus and K. Showalter, J. Phys. Chem., 1991, 95, 1299–1306.
10. I. Nagypál, G. Bazsa and I. R. Epstein, J. Am. Chem. Soc., 1986, 108, 3635–3640.
11. J. Masere, D. A. Vasquez, B. F. Edwards, J. W. Wilder and K. Showalter, J. Phys. Chem., 1994, 98, 6505–6508.
12. C. R. Chinake and R. H. Simoyi, J. Phys. Chem., 1994, 98, 4012–4019.
13. A. Komlósi, I. P. Nagy, G. Bazsa and J. A. Pojman, J. Phys. Chem. A, 1998, 102, 9136–9141.
14. G. Bazsa and I. R. Epstein, J. Phys. Chem., 1985, 89, 3050–3053.
15. R. E. McIlwaine, H. Fenton, S. K. Scott and A. F. Taylor, J. Phys. Chem. C, 2008, 112, 2499.
16. Y. Kuramoto, Chemical Oscillations, Waves, and Turbulence, Dover Publications Inc., 1984.
17. R. Z. Luther, Electrochemistry, 1906, 12, 596–598.
18. N. R. Tas, J. Haneveld, H. V. Jansen, M. Elwenspoek and A. Berg, Appl. Phys. Lett., 2004, 85, 3274–3276.
19. G. Rábai, M. Orbán and I. R. Epstein, Acc. Chem. Res., 1990, 23, 258.
20. I. B. Levitan and L. K. Kaczmarek, The Neuron: Cell and Molecular Biology, Oxford University Press, New York, 2nd edn, 1996.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c2cp43153j

---