Effect of YOYO-1 on the mechanical properties of DNA

Abstract

YOYO-1 is a green fluorescent dye which is widely used to image single DNA molecules in solution for biophysical studies. However, the question of whether the intercalation of YOYO-1 affects the mechanical properties of DNA is still not clearly answered. Investigators have put forth contradicting data on the changes in persistence length of DNA. Here, we use atomic force microscopy to systematically study the changes in the mechanical properties of DNA due to the intercalation of YOYO-1. We first measured the persistence length, contour length and the bending angle distribution of the DNA–YOYO-1 complex. We find that the persistence length of DNA remains unaffected with the intercalation of YOYO-1. However the contour length increases linearly with about 38% increase at full saturation of 1 YOYO-1 per 4 base pairs of DNA. Next we measured the change in topology of relaxed closed circular DNA after the intercalation of YOYO-1. We find that YOYO-1 introduces supercoiling in closed circular DNA. Our observations indicate that the intercalation of YOYO-1 results in the underwinding of DNA duplex, but does not significantly change the persistence length.

---

1 Introduction

YOYO-1 (YOYO) is a green fluorescent dye which belongs to the family of cyanine dye and is a tetracationic homodimer of oxazole. Though YOYO is fairly non-fluorescent in solution, it forms a highly fluorescent complex with double stranded DNA, with more than 1000-fold increase in fluorescent intensity.¹ Since the development of YOYO by Rye et al.,¹ it has been widely used to visualize DNA molecules to measure self diffusion of DNA,^2,3 study the hydrodynamic stretching of DNA^4,5 and understand DNA dynamics and conformation in confinement.^6,7 YOYO also enables visualizing the contour of elongated DNA for use in genomic mapping.^8–10 In these and other studies, the DNA is usually modeled as a wormlike chain polymer – an elastic filament with a given bending rigidity that is embodied in the persistence length.¹¹

These studies use fluorescently labeled DNA to study the mechanical and structural properties of DNA under varying conditions like ionic strength, binding of organic compounds and biological agents. As YOYO-1 displays a strong fluorescent enhancement upon binding to DNA with increased signal to noise ratio, it enables an easy single molecule observation. YOYO binds to DNA by bis-intercalation of its two chromophore units into the DNA.¹ The binding of YOYO to DNA changes the effective charge of DNA. Hence it is important to characterise whether YOYO binding changes the mechanical and structural properties of DNA.

Since the development of YOYO by Rye et al. in 1992, a variety of studies have been reported on the changes in persistence length and contour length of DNA due to the intercalation of YOYO. While there is consensus regarding the change in contour length upon YOYO binding to DNA, the results for changes in persistence length are conflicting. We summarize the results below – starting with the older studies and ending with the most recent findings.

In 1995 and 1997, Perkins et al. reported that the contour length of DNA increased by 35% when stained with TOTO and YOYO respectively at the saturation concentration.^4,12 In 1997, Quake et al. cited unpublished work, according to which the intercalation of YOYO increased the contour length and persistence length of DNA by 22% and 32% respectively.¹³ In 1998, Bakajin et al. measured a 30% increase in contour length of DNA incubated with TOTO-1, an analogue of YOYO-1. They assumed that the persistence length also increased by the same factor as that of the contour length.⁵ These works were commonly referred to by many authors, until recently when various single molecule studies reported a decrease in persistence length up to 12 nm.^14,15 A linear increase in contour length has also been reported by many authors.^16,17

In more recent studies, the most common tools used for measuring the persistence length and contour length of DNA are magnetic/optical tweezers and atomic force microscopy (AFM). In 2002, atomic force microscopy studies by Berge et al. reported that the intercalation of a bis-intercalator, ditercalinium, increased the persistence length of DNA by two fold and the contour length by 28% that of the bare DNA.²²

Tweezing experiments were reported in the subsequent years. In 2005, Sischka et al. showed that the persistence length of DNA decreased by 70% from 40 to 12 nm and the contour length increased by 36% when intercalated with YOYO.¹⁴ In 2010, Murade et al. reported a decrease in persistence length of DNA by about 71% from 52 to 15 nm when incubated with 500 nM YOYO.¹⁵ In tweezing experiments, a single DNA molecule in the desired buffer is stretched with a certain amount of force and the extension of the DNA molecule is measured. However, it has been observed that when a DNA molecule in a buffer containing YOYO is stretched, it exhibits a large hysteresis.²³ Moreover this behaviour was strongly dependent on the speed at which the measurements are performed.¹⁶ This is due to the fact that YOYO exhibits different binding modes depending on the concentration of YOYO and time of incubation.^24,25 The presence of free YOYO in the buffer has been shown to introduce time dependent changes in the mechanical properties of DNA.¹⁶ The studies mentioned above^14,15,23 were done in non-equilibrium conditions as the DNA was stretched in a solution containing excess YOYO-1 molecules.

Taking into account the above mentioned limitations when using tweezers, Gunther et al., in 2010, measured the persistence length of DNA–YOYO complex using magnetic tweezers under equilibrium conditions.¹⁸ In their work, background DNA molecules were introduced so as to maintain a fixed staining ratio throughout the experiments. It was observed that under any staining ratio the persistence length remained unaffected and the contour length increased by 47% at a staining ratio of 1 YOYO per 3.2 base pairs.¹⁸ In 2010, Reuter et al. investigated the kinetics of binding, for single, hydrodynamically-stretched DNA molecules and found that the contour length increased by 36% at 1 YOYO per 3 base pairs.¹⁹

Recently, in 2013, Maaloum et al. found that the persistence length of DNA–YOYO complex decreased by 44% from 50 to 28 nm and the contour length increased by 46% at a staining ratio of 1 YOYO per 1 base pair.²⁰ They used atomic force microscopy to measure the persistence length of DNA–YOYO complex from the mean square end to end distance and the contour length. A more recent work also reported a decrease in persistence length from 50 to 46 nm and an increase in contour length to 18%.²¹ A historical comparison of prior work on the effect of YOYO-1 on DNA properties is given in Table 1.

Table 1 Historical comparison of prior work on the effect of YOYO-1 on DNA properties

Year	Reference	Technique	Dye	No. of dye/base pair	Persistence lengths^a	Contour length
a This column shows the persistence length of bare DNA and the YOYO stained DNA at the maximum concentration of YOYO used in each case. The value in the parentheses show the percentage increase or decrease. b The data is not reported and was never published.
1997	Perkins et al.^12	Hydrodynamic stretching	YOYO-1	1YOYO/4 bp	Not measured	35% increase
1997	Quake et al.^13	Optical tweezers	YOYO-1	Not controlled	50–66 nm (32% increase)^b	22% increase
2005	Sischka et al.^14	Optical tweezers	YOYO-1	Not controlled	40–11.8 nm (70% decrease)	36% increase
2010	Murade et al.^15	Optical tweezers	YOYO-1	Not controlled	52–14.9 nm (71% decrease)	50% increase
2010	Gunther et al.^18	Magnetic tweezers	YOYO-1	1 YOYO/3.2 bp	Constant around 52 nm	47% Increase
2010	Reuter et al.^19	Hydrodynamic stretching	YOYO-1	1 YOYO/3 bp	Not measured	36% increase
2013	Maaloum et al.^20	Atomic force microscopy	YOYO-1	1 YOYO/1 bp	50–28 nm (44% decrease)	46% increase
2014	Shi et al.^21	Entropic force microscopy	YOYO-1	1 YOYO/0.8 bp	51–46 nm (9% decrease)	18% increase
2014	Present work	Atomic force microscopy	YOYO-1	1YOYO/4 bp	Constant around 57 nm	38% increase

---

Here we use atomic force microscope to systematically study the mechanical and the structural properties of DNA. Atomic force microscope allows easy visualization of the DNA–YOYO complex and is free from any artifacts related to aggregation, folding or bridging. Our study consist of two parts. In the first part we measure the persistence length, contour length and the bending angle distribution of the DNA–YOYO complex at different ratios of YOYO per base pair. In the second part, we measure the change in topology of relaxed closed circular DNA after incubating with YOYO. Eventually we rationalize our results in terms of unwinding of DNA by the interaction of YOYO.

2 Materials and methods

2.1 Sample preparation

Monodisperse DNA fragments of 1000 base pairs and PUC 19 (2686 base pairs) was purchased from Thermoscientific (Vilnius, Lithuania). DNA was prepared with different concentrations of YOYO in a buffer containing 1× TE (10 mM Tris base, 1 mM EDTA, pH 8 titrated with HCl). The concentration of the dye was adjusted so that there is one YOYO dimer per 4, 8, 16 and 100 base pairs of DNA. The DNA–YOYO was incubated at 55 °C for 2 hours for homogenous distribution of the dye along the DNA. 20 μl of the sample was then deposited on a freshly cleaved SPI Grade V-4 mica and incubated for 30 min. This is then washed with DI water and blown dry using nitrogen gas. For imaging of bare DNA, 5 mM MgCl₂ was used. This method allows the DNA molecules to equilibrate on the surface by diffusion. The interaction between DNA and mica mediated by Mg²⁺ is weak, so that the chain statistics is not affected. It has been shown previously that DNA deposited in this way equilibrates in a 2D conformation.^26–29 For imaging DNA intercalated with YOYO, the sample was deposited on mica without MgCl₂. YOYO being cationic facilitated the binding of the DNA–YOYO complex to mica. Here we assume that the binding of DNA–YOYO complex to the mica is 2D equilibrated due to the following reasons. (i) The size of the YOYO stained DNA molecules are similar to the 2D equilibrated DNA in the presence of Mg²⁺ as shown in panel A of Fig. 1. On the other hand, the kinetically trapped DNA molecules appear much smaller in size as shown in panel B of Fig. 1. The difference in size comes from considering a polymer equilibrated in 2D versus the projected image of a 3D polymer into a 2D surface. Rivetti et al. has shown that for a long DNA chain, the projection of the 3D image onto the 2D plane gives a mean-squared end-to-end size, 〈R²〉_proj, which is 1/3 the value of the size of DNA equilibrated 2D, 〈R²〉_2D.²⁶ This difference in size can be used to distinguish between the 2D equilibrated and kinetically trapped DNA. (ii) We do not see any overlapping of the molecules as would be observed in the case of kinetically trapped molecules.

Fig. 1 (A) PUC 19 DNA (2686 base pairs) equilibrated on the surface of mica in the presence of Mg²⁺ (B) PUC 19 DNA kinetically trapped on the surface of polylysine treated mica.

2.2 Atomic force microscopy

The imaging was done at room temperature in air with a Nanowizard II atomic force microscope (JPK Instruments, Berlin, Germany). Images were acquired in the tapping mode with Nanosensor silicon (Si) cantilevers (spring constant of 10–130 N m⁻¹) and operated below their resonance frequency (typically 200–500 kHz).

2.3 Data analysis

There are two ways to determine the persistence length of a polymer: (i) from the bending angle or (ii) from the end to end distance of the DNA molecule. For both cases, the first step is to obtain the centerline of the DNA molecules. This was done by moving along the DNA molecules in a small step size of half the cross sectional diameter and locating the point with maximum height. For a DNA molecule equilibrated in 2 dimensions, the energy needed to bend a segment of length, L through angle θ is given bywhere L_p is the persistence length. The normalised probability distribution of the bending angle, θ is given by,where q is a normalization constant. From the probability distribution of bending angles, one can derive the tangent-tangent correlation, 〈cos θ_s,s+L〉 which can be written as:

〈cos θ_s,s+L〉_2D = exp(−L/2L_p) (3)

where θ is the angle between tangent vectors at points s and s + L along the contour. The inverse of the exponential decay constant gives the persistence length, L_p of the polymer.

We can also find the mean-squared end-to-end distance by taking the integral of the tangent–tangent correlation function and it is written as follows:

where L_c is the contour length of the polymer. The above equations assume that excluded volume interactions (segment–segment interactions of the DNA) are negligible. We also note that in the case of kinetically trapped DNA molecules, the above equations need to be modified taking into consideration the 3D conformation and its projection on the surface.^26,30

3 Results

3.1 Persistence length

We first determined the persistence length of bare DNA. For this purpose, a DNA concentration of 1.37 mg l⁻¹ in 5 mM MgCl₂ and an incubation time of 5 min was used for acquiring the images. Here we used short DNA fragments of 1000 base pairs. DNA molecules of this size allows easy visualisation of structural changes in DNA with minimal effect due to aggregation and folding. Moreover, DNA molecules of size less than 1000 nm are shown to be unaffected by excluded volume interactions²⁶ and hence consistent with the use of eqn (1)–(4). For DNA–YOYO complex, a concentration of 2.1 mg l⁻¹ and an incubated time of 30 min was used. Fig. 2 show the representative images of bare DNA and the DNA–YOYO complex and also their corresponding contours.

Fig. 2 Representative AFM images (left panel) of 1000 base pairs DNA molecules and their contours (right panel) when incubated with different ratios of YOYO to base pairs. (A) Bare DNA (B) 1YOYO per 100 base pairs (C) 1 YOYO per 16 base pairs (D) 1 YOYO per 8 base pairs (E) 1 YOYO per 4 base pairs.

From the DNA contours, the tangent autocorrelation function, 〈cos θ_s,s+L〉 versus the separation, L was plotted. The curve thus obtained was fitted with eqn (3), from which the persistence length was obtained (Fig. 3). We obtained a persistence length of 56 nm for bare DNA which is in good agreement with previously reported values.^27,29

Fig. 3 The correlation function 〈cos θ_s,s+L〉 versus separation, L for DNA with (A) no YOYO (B) 1 YOYO per 100 base pairs (C) 1 YOYO per 16 base pairs (D) 1 YOYO per 8 base pairs (E) 1 YOYO per 4 base pairs. The filled circles are the experimental data obtained by averaging 30 molecules. The line is the fit to the experimental data using eqn (3).

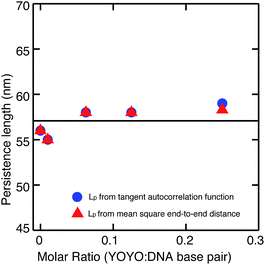
We then measured the persistence length of DNA–YOYO complex at various YOYO per base pair concentrations. We find that the persistence length of DNA–YOYO complex does not deviate much from that of bare DNA and stays within the error limits with an average value of 57 nm. We also measured the mean-squared separation, 〈(R_s,s+L)²〉 between pairs of points located at s and s + L and averaging for all values of s along the contour. Fig. 4 shows the plot of the mean-square end-to-end distance versus the separation, L for the same conditions as in Fig. 3. The closed circles are the experimental data. This curve is fitted with eqn (4), from which the persistence length is obtained. The persistence length obtained from the two methods are in good agreement (Fig. 5).

Fig. 4 The mean square end to end distance versus the separation, L for DNA with (A) no YOYO (B) 1 YOYO per 100 base pairs (C) 1 YOYO per 16 base pairs (D) 1 YOYO per 8 base pairs (E) 1 YOYO per 4 base pairs. The filled circles are the experimental data obtained by averaging 30 molecules. The line is the fit to the experimental data using eqn (4).

Fig. 5 Persistence length of DNA–YOYO complex remains constant with an average value around 57 nm (solid line) irrespective of the YOYO concentration. The circles are the persistence length obtained from the average tangent autocorrelation function of 30 molecules and the triangles are the data obtained from the average mean squared end-to-end distance versus the separation of 30 molecules.

3.2 Bending angle distribution

One might expect the persistence length of the DNA–YOYO complex to be reduced due to the charge neutralization of DNA by YOYO. However, persistence length is the bending rigidity of DNA which is derived from the bending angle distribution of DNA. The persistence length from Fig. 3 and 4 is obtained by averaging the bending rigidity along the contour of the DNA molecules. YOYO binding could cause structural changes in DNA which could lead to the local changes in the bending rigidity. The observed persistence length could be the result of larger bending angles due to kinks counterbalancing the smaller bending angle due to stiffening. This could lead to the overall persistence length being unaffected. To investigate this, we plotted the probability distribution of the bending angles obtained from all the molecules in case of bare DNA and DNA–YOYO complex. Fig. 6 shows the probability distribution of the bending angles for angle, θ between the tangents separated by a contour length of 20 nm. This length scale was used as it has been shown earlier that the DNA is well described by the wormlike chain model for length scales more than three helical turns of DNA (10.5 nm).³¹ Here we have used 20 nm to accommodate for any extension of the helical turns resulting from the YOYO intercalation. We find that the probability distribution of the bending angle is unaffected by the intercalation of YOYO and fits the curve which describes the wormlike chain model. Thus we conclude that the persistence length of DNA–YOYO complex is unaffected by the intercalation of YOYO.

Fig. 6 Probability distribution of the DNA bending angle for angle θ between tangents separated by the contour length of 20 nm for bare DNA (star), 1 YOYO per 100 base pairs (triangle), 1 YOYO per 16 base pairs (square), 1 YOYO per 8 base pairs (diamond), 1 YOYO per 4 base pairs (circle). The line is the wormlike chain prediction with the predetermined persistence length of 56 nm in the case of bare DNA.

3.3 Contour length

Next, we measured the contour length of the DNA–YOYO complex. From the AFM images, we find that the contour length of bare DNA is 328 ± 8 nm. With the staining ratio of 1 YOYO per 100 base pairs, there is almost no change in contour length. However, the contour length of the DNA–YOYO complex increases linearly with increase in staining ratio as shown in Fig. 7. At a staining ratio of 1 YOYO per 4 base pairs, the contour length of DNA increases by 38% from 328 ± 8 nm to 453 ± 17 nm. From these values, we find that each bound YOYO extends the DNA molecule by 0.5 nm.

Fig. 7 Contour length of DNA–YOYO complex increases linearly with increase in staining ratio. The circles represent the average contour length of DNA–YOYO complex averaged over 30 molecules. The data points correspond to the DNA–YOYO ratios mentioned in Fig. 2. The error bars are the standard deviations and the line is a linear fit to the data points.

3.4 YOYO changes the helical twist of DNA

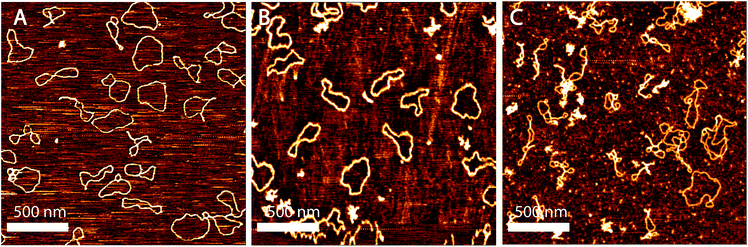
We find that YOYO increases the contour length of DNA–YOYO complex without affecting the persistence length. We propose that this elongation of DNA–YOYO complex is due to the under twisting of DNA. YOYO induced under twisting of DNA has been reported earlier, however there has not been a direct evidence for this process. Earlier NMR studies have shown that YOYO under twists the DNA by 106°.³² Magnetic tweezers experiments have shown that, a torsionally constrained DNA–YOYO complex becomes negatively supercoiled when destained. The authors measured an untwisting of 24° per YOYO molecule.¹⁸

In order to investigate the supercoiling of DNA by the intercalation of YOYO, we used relaxed closed circular DNA. For this purpose, PUC 19 DNA was treated with Topo 1 which relaxes both the left and the right handed supercoiling. Topo 1 is a class of DNA topoisomerase that cuts a single strand of the DNA helix and allows the complimentary strand to pass through the nick and reseals the nick, thereby reducing the linking number and relaxing the DNA.³³ The closed circular DNA thus obtained was then incubated with YOYO in the ratio 1 YOYO per 100 bp and 1 YOYO per 4 base pair at 55 °C for 2 hours. Panel A of Fig. 8 shows the relaxed closed circular PUC 19 DNA. When incubated with 1 YOYO per 100 bp, we find that there is no change in the topology of DNA (panel B of Fig. 8). However the DNA topology is fundamentally different when incubated with 1 YOYO per 4 base pairs as shown in panel C of Fig. 8. We find that the majority of the DNA population is plectonemically supercoiled. Less that 5% of the DNA is not supercoiled as the presence of nicks in one of the strands in DNA helix prevents the DNA from supercoiling. Some of the molecules appear compacted due to the increased helical twist in the DNA. We measure the length of the superhelical axis of the discernible plectonemes and found that the ratio of the average length of the superhelical axis of the plectonemes and the contour length of the circular DNA is 0.42 which is in agreement with the value reported in the literature.³⁴ Our observations show that the YOYO induced supercoiling in the relaxed closed circular DNA is due to the under twisting of DNA by the intercalated YOYO.

Fig. 8 (A) PUC 19 plasmid DNA relaxed using Topo 1 is mostly circular. (B) PUC 19 DNA when incubated with YOYO at a concentration of 1 YOYO per 100 base pairs does not affect the topology of DNA. (C) PUC 19 DNA when incubated with YOYO at a concentration of 1 YOYO per 4 base pairs becomes supercoiled.

4 Discussion

Here we used atomic force microscopy to study the mechanical and structural properties of the DNA–YOYO complex. We find that the persistence length of the DNA–YOYO complex does not deviate from that of the bare DNA for YOYO concentrations ranging from very low staining ratio of 1 YOYO per 100 base pairs to the saturation level of 1 YOYO per 4 base pairs. It should be noted that our methodology is able to measure an increase or decrease in persistence length precisely as reported in our previous work.²⁸ Our results are at odds with most of the previously reported results^{14,15,19–21} which showed that the persistence length of DNA decreases with increasing concentration of YOYO. Our results do however agree with the recent study by Gunther et al.¹⁸ These discrepancies in the literature can be due to the following reasons. (i) Most of the single molecule experiments were not done at equilibrium conditions. Single molecules experiments are usually done by stretching a single DNA molecule in a buffer containing excess of YOYO. This would mean that the stretching of DNA molecules would result in the association and dissociation of the YOYO-1 and hence affect the persistence length. This issue was taken into consideration by Gunther et al. who designed their experiments such that the number of YOYO per base pair remains fixed.¹⁸ (ii) YOYO binding has been shown to depend on the amount of force applied and the stretching speed.¹⁶

Using atomic force microcopy, we are able to visualize the molecules under equilibrium conditions and unperturbed by external force. However our results on persistence length do not agree with the previous atomic force microscopy results²⁰ due to the following reasons. The prior authors determined the persistence length from the end-to-end distance and the contour length of DNA.²⁰ They used polydispersed DNA molecules with sizes ranging from about 200 to 1500 nm. However it has been shown that for DNA molecules equilibrated in 2D, the excluded volume effects significantly alters the chain dimensions of DNA molecules with contour length more than 20 times the persistence length. Taking these factors into consideration, we have plotted the tangent auto correlation function and the mean square end-to-end distance for short length scales of 4–100 nm. We have used monodisperse DNA molecules of 1000 bp (340 nm) to avoid any effects due to the excluded volume interactions and other artifacts. It should be noted that our results in air well represent the results in solution due to our sample preparation methods. Zuccheri et al.³⁵ has shown earlier that while imaging DNA in solution, the DNA is slight mobile on the mica surface in the presence of buffer. However when deionized water is injected to replace the buffer, the DNA molecules freezes in the same conformation as they had been immediately before the injection of water.³⁵ In our sample preparation we freeze the conformation with deionized water before drying the sample. This enables us to capture the conformation of DNA in the desired buffer conditions.

Another important factor that affects the binding of YOYO to DNA is the time and temperature of incubation. We find that for the equilibrium distribution of YOYO along the DNA molecules, the sample has to be incubated at 55 °C for at least 2 hours. Previous electrophoresis studies have also shown this as a necessary condition for equilibration of the DNA–YOYO complex.²⁵ Most of the previously reported experiments were performed with samples incubated at room temperature.

Another factor which may be the cause of the inconsistency in the reported values of persistence length and contour length is the ionic strength. Firstly, the persistence length of DNA has been shown to be dependent on the ionic strength. At ionic strength more than 7.44 mM, the persistence length of DNA remains constant between 50 to 60 nm.³⁶ The ionic strength of 1× TE (10 mM Tris base, 1 mM EDTA, pH 8 titrated with HCl) is about 8.4 mM. At this ionic strength we measured the persistence length of DNA to be 56 nm. This agrees well with the previously reported values.^27,36 The binding of YOYO has also been shown to be dependent on the ionic strength. It has been shown by Gunther et al. that the binding of YOYO is stable at ionic strengths less than 100 mM.¹⁸ The choice of our buffer conditions was made to ensure that it is high enough to provide a stable condition for bare DNA and at the same time low enough for the stable binding of YOYO.

The contour length measurements show that the length of DNA increases by 38% at the maximum staining ratio of 1 YOYO per 4 base pairs. Thus each bound YOYO extends the DNA molecule by 0.5 nm which is in agreement with previously reported results.¹⁸ The measurement of the persistence length and the contour length of DNA shows that the intercalation of YOYO changes the contour length of DNA without affecting the persistence length. However one would expect the persistence length of DNA–YOYO complex to be reduced due to the charge neutralisation of DNA by YOYO. The bending angle distribution shows that the intercalation of YOYO does not introduce local changes in the bending angles along the DNA helix. The linear increase in contour length of DNA with increasing concentration of YOYO should be due to the underwinding of the DNA helix. In the case of closed circular DNA, such a change in helical twist should result in a change in the linking number deficit and hence the superhelical density. Hence the relaxed closed circular DNA should become supercoiled with the change in the helical twist.³³ Our experiments on closed circular DNA intercalated with YOYO have confirmed the fact YOYO changes the helical twist of DNA and hence leading to the supercoiling of DNA.

5 Conclusion

AFM is an effective tool which can be used to determine the mechanical and structural properties of DNA. Given the fact that AFM allows the measurement of persistence length and contour length of 2D equilibrated DNA under zero force, it is complementary to other methods such as magnetic and optical tweezers. Here we have used atomic force microscopy to measure the persistence length and the contour length of DNA and YOYO intercalated DNA by carefully considering the experimental factors that affect these measurements. Taking into consideration the experimental factors such as incubation time and temperature for equilibrium distribution of the YOYO on DNA, the ionic strength of the buffer and using short DNA fragments of DNA, we find that the our results agree with those reported by Gunther et al. Moreover, we have confirmed a possible mechanism due to which the mechanical and structural properties of DNA is affected when stained with YOYO. We have shown that YOYO binding leads to under winding of DNA which leads to the change in topology in the case of closed circular DNA.

Acknowledgements

This research was supported by the National Research Foundation Singapore through the Singapore MIT Alliance for Research and Technology's BioSystems and Micromechanics (BioSym) IRG research program and the National Science Foundation (CBET-1335938). Piewen Cong is thanked for the contribution of matlab program to determine the centreline of DNA.

References

1. H. S. Rye, S. Yue, D. E. Wemmer, M. A. Quesada, R. P. Haugland, R. A. Mathies and A. N. Glazer, Nucleic Acids Res., 1992, 20, 2803–2812.
2. D. Wirtz, Phys. Rev. Lett., 1995, 75, 2436–2439.
3. B. Maier and J. O. Rädler, Phys. Rev. Lett., 1999, 82, 1911–1914.
4. T. T. Perkins, D. E. Smith, R. G. Larson and S. Chu, Science, 1995, 268, 83–87.
5. O. Bakajin, T. Duke, C. Chou, S. Chan, R. Austin and E. Cox, Phys. Rev. Lett., 1998, 80, 2737–2740.
6. J. O. Tegenfeldt, C. Prinz, H. Cao, S. Chou, W. W. Reisner, R. Riehn, Y. M. Wang, E. C. Cox, J. C. Sturm and P. Silberzan, et al. , Proc. Natl. Acad. Sci. U. S. A., 2004, 101, 10979–10983.
7. C.-C. Hsieh and P. S. Doyle, Korea Aust Rheol J, 2008, 20, 127–142.
8. D. C. Schwartz, X. Li, L. I. Hernandez, S. P. Ramnarain, E. J. Huff and Y.-K. Wang, Science, 1993, 262, 110–114.
9. R. H. Meltzer, J. R. Krogmeier, L. W. Kwok, R. Allen, B. Crane, J. W. Griffis, L. Knaian, N. Kojanian, G. Malkin and M. K. Nahas, et al. , Lab Chip, 2011, 11, 863–873.
10. K. D. Dorfman, AIChE J., 2013, 59, 346–354.
11. J. F. Marko and E. D. Siggia, Macromolecules, 1995, 28, 8759–8770.
12. T. T. Perkins, D. E. Smith and S. Chu, Science, 1997, 276, 2016–2021.
13. S. R. Quake, H. Babcock and S. Chu, Nature, 1997, 388, 151–154.
14. A. Sischka, K. Toensing, R. Eckel, S. D. Wilking, N. Sewald, R. Ros and D. Anselmetti, Biophys. J., 2005, 88, 404–411.
15. C. U. Murade, V. Subramaniam, C. Otto and M. L. Bennink, Nucleic Acids Res., 2010, 38, 3423–3431.
16. M. L. Bennink, O. D. Schärer, R. Kanaar, K. Sakata-Sogawa, J. M. Schins, J. S. Kanger, B. G. de Grooth and J. Greve, Cytometry, 1999, 36, 200–208.
17. P. S. Doyle, B. Ladoux and J.-L. Viovy, Phys. Rev. Lett., 2000, 84, 4769–4772.
18. K. Günther, M. Mertig and R. Seidel, Nucleic Acids Res., 2010, 38, 6526–6532.
19. M. Reuter and D. T. Dryden, Biochem. Biophys. Res. Commun., 2010, 403, 225–229.
20. M. Maaloum, P. Muller and S. Harlepp, Soft Matter, 2013, 9, 11233–11240.
21. N. Shi and V. M. Ugaz, Small, 2014, 10, 2553–2557.
22. T. Berge, N. S. Jenkins, R. B. Hopkirk, M. J. Waring, J. M. Edwardson and R. M. Henderson, Nucleic Acids Res., 2002, 30, 2980–2986.
23. C. Murade, V. Subramaniam, C. Otto and M. L. Bennink, Biophys. J., 2009, 97, 835–843.
24. A. Larsson, C. Carlsson, M. Jonsson and B. Albinsson, J. Am. Chem. Soc., 1994, 116, 8459–8465.
25. C. Carisson, M. Johnson and B. Åkerman, Nucleic Acids Res., 1995, 23, 2413–2420.
26. C. Rivetti, M. Guthold and C. Bustamante, J. Mol. Biol., 1996, 264, 919–932.
27. P. A. Wiggins, T. Van Der Heijden, F. Moreno-Herrero, A. Spakowitz, R. Phillips, J. Widom, C. Dekker and P. C. Nelson, Nat. Nanotechnol., 2006, 1, 137–141.
28. B. Kundukad, P. Cong, J. R. van der Maarel and P. S. Doyle, Nucleic Acids Res., 2013, 41, 8280–8288.
29. P. J. Hagerman, Annu. Rev. Biophys. Biophys. Chem., 1988, 17, 265–286.
30. J. Abels, F. Moreno-Herrero, T. van der Heijden, C. Dekker and N. Dekker, Biophys. J., 2005, 88, 2737–2744.
31. A. K. Mazur and M. Maaloum, Phys. Rev. Lett., 2014, 112, 068–104.
32. F. Johansen and J. P. Jacobsen, J. Biomol. Struct. Dyn., 1998, 16, 205–222.
33. A. D. Bates and A. Maxwell, DNA topology, Oxford University press, 2005.
34. T. C. Boles, J. H. White and N. R. Cozzarelli, J. Mol. Biol., 1990, 213, 931–951.
35. G. Zuccheri, R. T. Dame, M. Aquila, I. Muzzalupo and B. Samori, Appl. Phys. A: Mater. Sci. Process., 1998, 66, S585–S589.
36. C. G. Baumann, S. B. Smith, V. A. Bloomfield and C. Bustamante, Proc. Natl. Acad. Sci. U. S. A., 1997, 94, 6185–6190.

---