Defect structures mediate the isotropic–nematic transition in strongly confined liquid crystals

Abstract

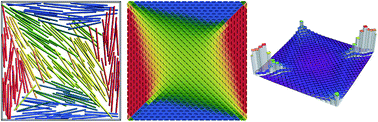
Using Monte Carlo simulations, we study rod-like lyotropic liquid crystals confined to a square slab-like geometry with lateral dimensions comparable to the length of the particles. We observe that this system develops linear defect structures upon entering the planar nematic phase. These defect structures flank a lens-shaped nematic region oriented along a diagonal of the square box. We interpret these structures as a compromise between the 2-fold order of the bulk nematic phase and the 4-fold order imposed by the lateral boundaries. A simple Onsager-type theory that effectively implements these competing tendencies is used to model the phase behavior in the center of the box and shows that the free-energy cost of forming the defect structures strongly offsets the transition-inducing effects of both the transverse and lateral confinement.