Orientational self-sorting in cuboctahedral Pd cages

Abstract

Cuboctahedral coordination cages of the general formula [Pd₁₂L₂₄]²⁴⁺ (L = low-symmetry ligand) were analyzed theoretically and experimentally. With 350 696 potential isomers, the structural space of these assemblies is vast. Orientational self-sorting refers to the preferential formation of particular isomers within the pool of potential structures. Geometric and computational analyses predict the preferred formation of cages with a cis arrangement at the metal centers. This prediction was corroborated experimentally by synthesizing a [Pd₁₂L₂₄]²⁴⁺ cage with a bridging 3-(4-(pyridin-4-yl)phenyl)pyridine ligand. A crystallographic analysis of this assembly showed exclusive cis coordination of the 3- and the 4-pyridyl donor groups at the Pd²⁺ ions.

---

Introduction

Reactions of ditopic N-donor ligands L with Pd²⁺ ions can give molecularly defined nanostructures of the general formula [Pd_nL_2n]²ⁿ⁺.¹ The structural diversity of these assemblies is remarkable, ranging from simple dinuclear [Pd₂L₄]⁴⁺ complexes^1c to interlocked [Pd₄L₈]⁸⁺ cages² and giant [Pd₃₀L₆₀]⁶⁰⁺ and [Pd₄₈L₉₆]⁹⁶⁺ spheres.³ The geometry of the ligand is a decisive factor for the assembly process, with the ligand bend angle (the relative orientation of the coordinate vectors) being of special importance.^1,4 Other factors such as ligand–ligand interactions⁵ or template effects^6,7 can also influence the self-assembly process.

In terms of applications, different directions have been explored, including the use of [Pd_nL_2n]²ⁿ⁺ complexes as receptors for ions and drugs,^8,9 as components of novel materials,¹⁰ as reactors for chemical transformations,¹¹ and as links for nanoparticles.¹²

For future advances, it is desirable to extend the library of available [Pd_nL_2n]²ⁿ⁺ nanostructures.¹³ Given the importance of the ligand in the self-assembly process, it is clear that ligand design is key for generating new [Pd_nL_2n]²ⁿ⁺ complexes. Most homoleptic [Pd_nL_2n]²ⁿ⁺ complexes are based on ligands with identical N-donor groups, and these ligands typically display C_2v symmetry.¹

The utilization of low-symmetry ligands with two distinct donor groups represents an attractive strategy to access new [Pd_nL_2n]²ⁿ⁺ structures.^13,14 A potential problem of this approach is the formation of isomers. For [Pd₂L₄]⁴⁺-type assemblies, the use of a low-symmetry ligand can give rise to four different isomers, which differ in the relative orientation of the ligands (Fig. 1).

Fig. 1 The combination of a curved ligand L with two distinct binding sites (blue/red) and Pd²⁺ ions can lead to the formation of four different [Pd₂L₄]⁴⁺ complexes. ‘Orientational self-sorting’ refers to the preferential formation of complexes with a particular relative orientation of the bridging ligand (the selective formation of the cis isomer is shown as a representative example).

Experimental studies have shown that the selective formation of one isomer with a particular relative orientation of the ligands is possible (‘orientational self-sorting’).¹⁵ However, the energy difference between the isomers is sometimes not sufficient for providing a good selectivity.^15b,c

For [Pd_nL_2n]²ⁿ⁺ assemblies based on low-symmetry ligands, the number of potential isomers quickly increases with n. Macrocyclic [Pd₃L₆]⁶⁺ assemblies can form 9 isomers, pseudo-tetrahedral [Pd₄L₈]⁸⁺ complexes can form 35 isomers, and octahedral [Pd₆L₁₂]¹²⁺ complexes can form 112 different isomers.^16,17 These numbers increase to 16, 68, and 186 if enantiomers are included.

Despite the vast number of potential isomers, orientational self-sorting is possible for high-nuclearity [Pd_nL_2n]²ⁿ⁺ assemblies, as demonstrated by a recent experimental study from our group.¹⁶ The utilization of a thiophenylene-spaced ligand with 3- and 4-pyridyl donor groups gave rise to a structurally defined [Pd₆L₁₂]¹²⁺ complex.

Below, we analyze the structures and the potential isomers of cuboctahedral [Pd₁₂L₂₄]²⁴⁺ complexes (n = 12). Geometric and computational analyses predict the preferred formation of cages with exclusive cis coordination at the metal centers. This prediction was corroborated experimentally by synthesis and structural analysis of a [Pd₁₂L₂₄]²⁴⁺ cage with a bridging 3-(4-(pyridin-4-yl)phenyl)pyridine ligand.

Results and discussion

In order to determine the number of potential isomers for M₁₂L₂₄ structures based on low-symmetry ligands, we have used a standard mathematical counting argument related to the Orbit-Stabilizer Theorem.¹⁸ Our algorithm uses the cycle decomposition of the hyperoctahedral group¹⁹ to generate the collection of configurations with non-trivial symmetries and then uses the Orbit-Stabilizer Theorem¹⁸ to obtain a relationship between the number of distinct configurations and the number of symmetries each configuration has. As the set we generate is substantially smaller than the set of all possible configurations, we are able to apply this method of counting to M₁₂L₂₄ whose number of ligands proves too large for previous (exhaustive) methods.¹⁶ A more detailed description of the procedure is given in the (ESI, Section 4†).

Our analysis revealed that M₁₂L₂₄ cages can form 350 696 potential isomers if the bridging ligands display distinct donor groups (Fig. 2). The vast majority of these isomers are chiral (99.8%). If enantiomers are taken into account, the total number of isomers increases to 700 688. The calculations show that the structural space of M_nL_2n-type assemblies increases dramatically when going from n = 6 (octahedral cages, 112 isomers) to n = 12 (cuboctahedral cages, 350 696 isomers).

Fig. 2 Potential isomers for M₁₂L₂₄ cages with low-symmetry bridging ligands. The graphic shows only one of the possible isomers.

Cuboctahedral complexes of type M₁₂L₂₄ can be deconstructed into four M₆L₆ hexagons, with the metal centers having an occupancy of 50% (Fig. 5a). Let's consider the case, which is schematically depicted in Fig. 3b. The assemblies are based on ditopic ligands having the following general characteristics: the ligand is perfectly rigid, the donor groups are chemically distinct (red/blue), the coordinate vectors form an angle of 120°, and the distance between the donor atoms and the intersection of the coordinate vectors is not equal (a ≠ b). When combined with metal ions which act as linear connectors, three different hexagons can be formed (A–C, Fig. 3b). There are three ways to build an M₁₂L₂₄ assembly from A, and the resulting complexes have O_h, S₆, and C_4h symmetry. Hexagons of type B show two types of metal centers: three M are connected to the red parts of the ligand, and three M′ are connected to the blue parts of the ligand. The three M′ are positioned closer to the center of the hexagon B than the three M. The alternation of M and M′ in hexagon B makes it impossible to combine them into an M₁₂L₂₄-type assembly. The symmetry of the hexagon C, on the other hand, allows for the construction of a distorted cuboctahedron with D_2h symmetry.

Fig. 3 (a) Deconstruction of an M₁₂L₂₄ assembly into four hexagons with a metal occupancy of 50%; (b) a ligand with two distinct donor groups (blue/red), a 120° angle between the coordinate vectors, and non-equal lengths a and b can form three different M₆L₆ hexagons (A–C). The construction of an M₁₂L₂₄ assembly can be achieved from hexagon A (three possible combinations) or from hexagon C (one possible combination), but not from B or from mixtures of A/B/C. For simplicity, the M₁₂L₂₄ isomers formed from A and C are depicted as ideal cuboctahedra with linear ligands.

The analysis suggests that for ligands with a fixed bend angle of 120° and a pronounced difference between the distances a and b, orientational self-sorting should occur. The four preferred M₁₂L₂₄ isomers are shown in Fig. 3b. Obviously, the geometric analysis is based on perfectly rigid systems, and it does not account for the conformational flexibility of real molecular systems (e.g. variable coordinate vectors).

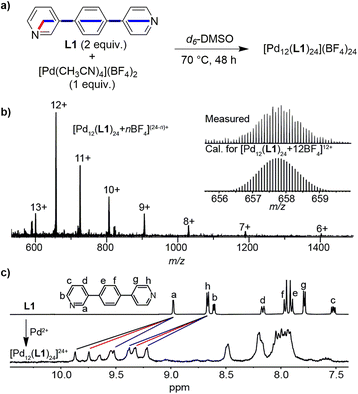
To investigate whether orientational self-sorting can be observed in [Pd₁₂L₂₄]²⁴⁺-type assemblies, we have examined the reaction between [Pd(CH₃CN)₄](BF₄)₂ (a convenient source of Pd²⁺) and ligand L1 (Fig. 4a). This ligand was chosen because it displays a fixed bend angle of 120° (rotations around the C–C single bonds do not change the bend angle), and a pronounced difference between the distances of the N-donor atoms and the intersection of the coordinate vectors.

Fig. 4 (a) Synthesis of the coordination cage [Pd₁₂(L1)₂₄](BF₄)₂₄; (b) high-resolution ESI mass spectra of [Pd₁₂(L1)₂₄ + nBF₄]^(24−n)+ (n) = 11–18); (c) ¹H NMR spectrum (d₆-DMSO) of the ligand L1 (top) and of cage [Pd₁₂(L1)₂₄](BF₄)₂₄ (bottom).

A mixture of [Pd(CH₃CN)₄](BF₄)₂ (1 equiv.) and L1 (2 equiv.) in d₆-DMSO was tempered for 48 h at 70 °C. Subsequently, the resulting solution was analyzed by high-resolution ESI mass spectrometry and by NMR spectroscopy. The MS data confirmed the expected formation of a dodecanuclear assembly (Fig. 4b). The ¹H NMR spectrum showed broad peaks (Fig. 4c), which is expected for assemblies of this size.¹ A detailed analysis by ¹H–¹H COSY and NOESY correlation spectroscopy revealed three sets of signals for the protons of ligands L1 (see the ESI, Fig. S9 and S10†). The three sets of signals have equal intensity, and the DOSY NMR spectrum (Fig. S11†) showed that they all belong to a species with the same diffusion constant. Overall, the NMR data suggested that the self-assembly process had resulted in the formation of one main species, rather than a complex mixture of isomers.

It is interesting to compare the NMR data with the results of the geometric analysis. The latter had predicted a preference for four isomers. Only two of the isomers have a ligand multiplicity of three, namely the C_4h symmetric isomer A₄-3, and the D_2h symmetric isomer C₄ (ESI, Fig. S25†).

Expanding on the geometrical analysis depicted in Fig. 3, a computational study was performed in order to evaluate the relative stabilities of the four isomers A₄-1, A₄-2, A₄-3, and C₄ of complex [Pd₁₂(L1)₂₄]²⁴⁺. Using an approach similar to recent work on Pd₂L₄ structures formed from low-symmetry ligands,²⁰ models of the four cages were constructed with the help of our Python framework, stk.^21,22 The geometry was optimized using a sequence of methods of increasing computational cost (see the ESI, Section 5†). Structures were optimized using UFF4MOF in GULP,²³ the semiempirical tight-binding method GFN2-xTB,²⁴ and, finally, density functional theory (DFT) geometry optimizations using hybrid and screened-hybrid functionals, PBE0-D3BJ²⁵ and HSE06,²⁶ respectively, with the Def2-SVP²⁷ basis set. Fig. 5 shows the relative energy of the isomers at the HSE06 level of theory, with A₄-3 being the most stable isomer. However, the energy differences between the three A₄ isomers are very small. In fact, the relative energy of the A₄ isomers changes depending on the level of theory (see the ESI, Table S3†). Therefore, the calculations do not provide a significant distinction between the A₄ isomers. However, the preference for A₄ over C₄ is apparent with an energy difference larger than 150 kJ mol⁻¹. This difference is significant, even if the limitations of our method (no solvent, no anions) are taken into account.

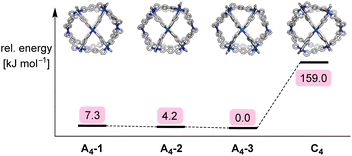Fig. 5 Schematic of the relative energy differences of the four isomers A₄-1, A₄-2, A₄-3, and C₄ of complex [Pd₁₂(L1)₂₄]²⁴⁺, along with graphics of the HSE06/Def2-SVP-optimized structures.

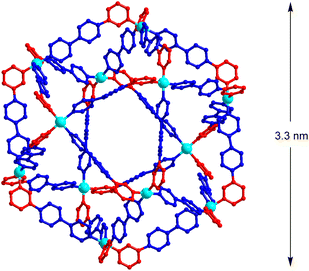
To determine the structure of cage [Pd₁₂(L1)₂₄]²⁴⁺, a crystallographic analysis was needed. Obtaining good-quality diffraction data turned out to be challenging, but we finally succeeded in using single crystals obtained by vapor diffusion of THF/Et₂O into a solution of the cage in DMSO.²⁸ Co-crystallized solvent molecules could not be located, and the solvent-mask procedure from Olex2 (ref. 29) was used to account for residual electron density. The structure of the polycationic cage could clearly be established (Fig. 6). The 12 Pd²⁺ all show a cis configuration, and the cage displays an approximate C_4h symmetry. Consequently, it is one of the four structures, which were predicted by the geometric analysis (A₄-3, Fig. 3b). The observed symmetry matches the signal multiplicity in the ¹H NMR spectrum (3 sets of signals), suggesting that the main structure in solution is the same as the one observed crystallographically. The presence of small amounts of other isomers cannot be excluded.

Fig. 6 Molecular structures of [Pd₁₂(L1)₂₄]²⁴⁺ as determined by X-ray crystallography. Hydrogen atoms and counter ions are not depicted. The color-coding of the ligand atoms aims to visualize the non-symmetric nature of the ligand.

Cuboctahedral cages of the formula [Pd₁₂L₂₄]²⁴⁺ are typically observed for ligands with a bend angle of around 120°.^1,30 Further straightening of the ligand to bend angles between 134° and 149° can give rhombicuboctahedral [Pd₂₄L₄₈]⁴⁸⁺ cages.^1,4,31 [Pd₂₄L₄₈]⁴⁸⁺ cages can form up to 5.86 trillion isomers if low-symmetry ligands are employed (ESI, Section 4†). A geometric analysis suggests that there is again a geometric preference for cages with cis-coordination at the metal centers (ESI, Section 3.3†). Preliminary attempts were made to examine orientational self-sorting in rhombicuboctahedral [Pd₂₄L₄₈]⁴⁸⁺ cages, but the available analytical data did not provide information about the selectivity of the assembly process. The interested reader can find more information in the ESI (Fig. S19–S21†).

Conclusions

Cuboctahedral coordination cages of the general formula [Pd₁₂L₂₄]²⁴⁺ (L = low-symmetry ligand) can potentially form 350 696 isomers, which differ in the relative orientation of the ligands. A geometric analysis combined with a computational study suggested the preferential formation of cages with cis coordination at the metal centers. Experimentally, we observed indeed the formation of a cage with cis-coordinated metal centers when Pd²⁺ salts were combined with 3-(4-(pyridin-4-yl)phenyl)pyridine.

The preference for cis coordination mirrors what we had observed for octahedral assemblies of type [Pd₆L₁₂]¹²⁺ (L = low-symmetry ligand).¹⁶ In fact, a geometrical analysis analogous to that shown in Fig. 3 predicts that cis coordination should be favored for [Pd₆L₁₂]¹²⁺ cages (ESI, Section 3.2†).

Overall, our results substantiate that it is possible to obtain structurally defined [Pd_nL_2n]²ⁿ⁺ cages of high nuclearity with low-symmetry ligands. A prerequisite for orientational self-sorting is the geometry of the ligand, which should display distinct distances between the donor atoms and the intersection of the coordinate vectors. Ligands of this kind will favor the formation of cages with a cis arrangement at the metal centers. We expect that the results of our analysis can be transferred to other types of coordination cages, given that donor groups of the ligands are coordinated in a square planar fashion to the metal centers.³²

Data availability

The computational data is freely available at: https://github.com/andrewtarzia/citable_data/tree/master/li_2022

Author contributions

R.-J. L. and K. S. initiated the study, R.-J. L. performed the experiments and analyzed the data, A. T., V. P. and K. E. J. performed a computational analysis of the stability of [Pd₁₂(L1)₂₄]²⁴⁺ cages, N. S. and A. M. computed the potential isomers, A. B. and G. H. C were responsible for the MS measurements of cage [Pd₂₄(L1)₄₈](BF₄)₄₈, F. F.-T. collected and processed the X-ray data, and R.-J. L. and K. S. co-wrote the manuscript. All authors discussed the results and commented on the manuscript.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The work was supported by the Swiss National Science Foundation, and by the École Polytechnique Fédérale de Lausanne (EPFL). KEJ thanks the Royal Society for a University Research Fellowship and the European Research Council through Agreement No. 758370 (ERC-StG-PE5-CoMMaD).

References

1. (a) T. Tateishi, M. Yoshimura, S. Tokuda, F. Matsuda, D. Fujita and S. Furukawa, Coord. Chem. Rev., 2022, 467, 214612; (b) N. B. Debata, D. Tripathy and H. S. Sahoo, Coord. Chem. Rev., 2019, 387, 273–298; (c) S. Saha, I. Regeni and G. H. Clever, Coord. Chem. Rev., 2018, 374, 1–14; (d) M. Han, D. M. Engelhard and G. H. Clever, Chem. Soc. Rev., 2014, 43, 1848–1860; (e) K. Harris, D. Fujita and M. Fujita, Chem. Commun., 2013, 49, 6703–6712.
2. M. Frank, M. D. Johnstone and G. H. Clever, Chem.–Eur. J., 2016, 22, 14104–14125.
3. (a) D. Fujita, Y. Ueda, S. Sato, H. Yokoyama, N. Mizuno, T. Kumasaka and M. Fujita, Chem, 2016, 1, 91–101; (b) D. Fujita, Y. Ueda, S. Sato, N. Mizuno, T. Kumasaka and M. Fujita, Nature, 2016, 540, 563–566.
4. Q.-F. Sun, J. Iwasa, D. Ogawa, Y. Ishido, S. Sato, T. Ozeki, Y. Sei, K. Yamaguchi and M. Fujita, Science, 2010, 328, 1144–1147.
5. For examples, see: (a) J. Tessarolo, H. Lee, E. Sakuda, K. Umakoshi and G. H. Clever, J. Am. Chem. Soc., 2021, 143, 6339–6344; (b) B. Chen, J. J. Holstein, S. Horiuchi, W. G. Hiller and G. H. Clever, J. Am. Chem. Soc., 2019, 141, 8907–8913; (c) B. Chen, S. Horiuchi, J. J. Holstein, J. Tessarolo and G. H. Clever, Chem.–Eur. J., 2019, 65, 14921–14927; (d) R. Zhu, W. M. Bloch, J. J. Holstein, S. Mandal, L. V. Schäfer and G. H. Clever, Chem.–Eur. J., 2018, 24, 12976–12982; (e) S. M. Jansze, G. Cecot, M. D. Wise, K. O. Zhurov, T. K. Ronson, A. M. Castilla, A. Finelli, P. Pattison, E. Solari, R. Scopelliti, G. E. Zelinskii, A. V. Vologzhanina, Y. Z. Voloshin, J. R. Nitschke and K. Severin, J. Am. Chem. Soc., 2016, 138, 2046–2054; (f) D. Preston, J. E. Barnsley, K. C. Gordon and J. D. Crowley, J. Am. Chem. Soc., 2016, 138, 10578–10585; (g) M. D. Wise, J. J. Holstein, P. Pattison, C. Besnard, E. Solari, R. Scopelliti, G. Bricogne and K. Severin, Chem. Sci., 2015, 6, 1004–1010.
6. E. G. Percástegui, Eur. J. Inorg. Chem., 2021, 4425–4438.
7. For examples, see: (a) S. Sudan, F. Fadaei-Tirani, K. E. Ebbert, G. H. Clever and K. Severin, Angew. Chem., Int. Ed., 2022, e202201823; (b) T. Tsutsui, L. Catti, K. Yoza and M. Yoshizawa, Chem. Sci., 2020, 11, 8145–8150; (c) T. Zhang, L.-P. Zhou, X.-Q. Guo, L.-X. Cai and Q.-F. Sun, Nat. Commun., 2017, 8, 15898; (d) R. Zhu, J. Lübben, B. Dittrich and G. H. Clever, Angew. Chem., Int. Ed., 2015, 54, 2796–2800; (e) S. Freye, R. Michel, D. Stalke, M. Pawliczek, H. Frauendorf and G. H. Clever, J. Am. Chem. Soc., 2013, 135, 8476–8479; (f) R. Sekiya, M. Fukuda and R. Kuroda, J. Am. Chem. Soc., 2012, 134, 10987–10997.
8. (a) G. H. Clever and P. Punt, Acc. Chem. Res., 2017, 50, 2233–2243; (b) A. Casini, B. Woods and M. Wenzel, Inorg. Chem., 2017, 56, 14715–14729.
9. For examples of drug encapsulation, see: (a) B. Woods, R. D. M. Silva, C. Schmidt, D. Wragg, M. Cavaco, V. Neves, V. F. C. Ferreira, L. Gano, T. S. Morais, F. Mendes, J. D. G. Correira and A. Casini, Bioconjugate Chem., 2021, 32, 1399–1408; (b) R. A. S. Vasdev, L. F. Gaudin, D. Preston, J. P. Jogy, G. I. Giles and J. D. Crowley, Front. Chem., 2018, 6, 563; (c) F. Kaiser, A. Schmidt, W. Heydenreuter, P. J. Altmann, A. Casini, S. A. Sieber and F. E. Kühn, Eur. J. Inorg. Chem., 2016, 5189–5196; (d) A. Schmidt, V. Molano, M. Hollering, A. Pöthig, A. Casini and F. E. Kühn, Chem.–Eur. J., 2016, 22, 2253–2256; (e) J. E. M. Lewis, E. L. Gavey, S. A. Cameron and J. D. Crowley, Chem. Sci., 2012, 3, 778–784.
10. (a) R.-J. Li, C. Pezzato, C. Berton and K. Severin, Chem. Sci., 2021, 12, 4981–4984; (b) S.-Q. Deng, D.-M. Li, X.-J. Mo, Y.-L. Miao, S.-L. Cai, J. Fan, W.-G. Zhang and S.-R. Zheng, ChemPlusChem, 2021, 86, 709–715; (c) Y. Gao, S.-Q. Deng, X. Jin, S.-L. Cai and S.-R. Zheng, Chem. Eng. J., 2019, 357, 129–139; (d) L. Zeng, Y. Xiao, J. Jiang, H. Fang, Z. Ke, L. Chen and J. Zhang, Inorg. Chem., 2019, 58, 10019–10027; (e) S. Saha, B. Holzapfel, Y.-T. Chen, K. Terlinden, P. Lill, C. Gatsogiannis, H. Rehage and G. H. Clever, J. Am. Chem. Soc., 2018, 140, 17384–17388; (f) Y. Gu, E. A. Alt, H. Wang, X. Li, A. P. Willard and J. A. Johnson, Nature, 2018, 560, 65–69; (g) J. Uchida, M. Yoshio, S. Sato, H. Yokoyama, M. Fujita and T. Kato, Angew. Chem., Int. Ed., 2017, 56, 14085–14089; (h) Y. Wang, Y. Gu, E. G. Keeler, J. V. Park, R. G. Griffin and J. A. Johnson, Angew. Chem., Int. Ed., 2017, 56, 188–192; (i) A. V. Zhukhovitskiy, M. Zhong, E. G. Keeler, V. K. Michaelis, J. E. P. Sun, M. J. A. Hore, D. J. Pochan, R. G. Griffin, A. P. Willard and J. A. Johnson, Nat. Chem., 2016, 8, 33–41; (j) A. V. Zhukhovitskiy, J. Zhao, M. Zhong, E. G. Keeler, E. A. Alt, P. Teichen, R. G. Griffin, M. J. A. Hore, A. P. Willard and J. A. Johnson, Macromolecules, 2016, 49, 6896–6902.
11. (a) R. Saha, B. Mondal and P. S. Mukherjee, Chem. Rev., 2022, 122, 12244–12307; (b) P. Howlander and M. Schmittel, Beilstein J. Org. Chem., 2022, 18, 597–630; (c) Y. Xue, X. Hang, J. Ding, B. Li, R. Zhu, H. Pang and Q. Xu, Coord. Chem. Rev., 2021, 430, 213656; (d) A. B. Grommet, M. Feller and R. Klajn, Nat. Nanotechnol., 2020, 15, 256–271; (e) A. C. H. Jans, X. Caumes and J. N. H. Reek, ChemCatChem, 2019, 11, 287–297; (f) L. J. Jongkind, X. Caumes, A. P. T. Hartendorp and J. N. H. Reek, Acc. Chem. Res., 2018, 51, 2115–2128; (g) I. Sinha and P. S. Mukherjee, Inorg. Chem., 2018, 57, 4205–4221.
12. L. Tian, C. Wang, H. Zhao, F. Sun, H. Dong, K. Feng, P. Wang, G. He and G. Li, J. Am. Chem. Soc., 2021, 143, 8631–8638.
13. S. Pullen, J. Tessarolo and G. H. Clever, Chem. Sci., 2021, 12, 7269–7293.
14. (a) C. T. McTernan, J. A. Davies and J. R. Nitschke, Chem. Rev., 2022, 122, 10393–10437; (b) J. E. M. Lewis and J. D. Crowley, ChemPlusChem, 2020, 85, 815–827.
15. (a) S. S. Mishra and D. K. Chand, Dalton Trans., 2022, 51, 11650–11657,  10.1039/d2dt01571d; (b) R. A. S. Vasdev, D. Preston, C. A. Casey-Stevens, V. Martí-Centelles, P. J. Lusby, A. L. Garden and J. D. Crowley, Inorg. Chem., 2022 DOI:10.1021/acs.inorgchem.2c00937; (c) A. Tarzia, J. E. M. Lewis and K. E. Jelfs, Angew. Chem., Int. Ed., 2021, 60, 20879–20887; (d) J. E. M. Lewis, Chem.–Eur. J., 2021, 27, 4454–4460; (e) H. Yu, J. Li, C. Shan, T. Lu, X. Jiang, J. Shi, L. Wojtas, H. Zhang and M. Wang, Angew. Chem., Int. Ed., 2021, 60, 26523–26527; (f) J. E. M. Lewis, A. Tarzia, A. J. P. White and K. E. Jelfs, Chem. Sci., 2020, 11, 677–683; (g) S. S. Mishra, S. V. K. Kompella, S. Krishnaswamy, S. Balasubramanian and D. K. Chand, Inorg. Chem., 2020, 59, 12884–12894; (h) S. Samantray, S. Krishnaswarmy and D. K. Chand, Nat. Commun., 2020, 11, 880; (i) S. K. Sen and R. Natarajan, Inorg. Chem., 2019, 58, 7180–7188; (j) D. Ogata and J. Yuasa, Angew. Chem., Int. Ed., 2019, 58, 18424–18428.
16. R.-J. Li, A. Marcus, F. Fadaei-Tirani and K. Severin, Chem. Commun., 2021, 57, 10023–10026.
17. B. Kandasamy, E. Lee, D.-L. Long, N. Bell and L. Cronin, Inorg. Chem., 2021, 60, 14772–14778.
18. D. S. Dummit and R. M. Foote, Abstract Algebra, 3rd edn, Wiley-VCH, 2003.
19. W. Y. C. Chen, SIAM J. Discret. Math., 1993, 6, 353–362.
20. A. Tarzia, J. E. M. Lewis and K. E. Kelfs, Angew. Chem., Int. Ed., 2021, 60, 20879–20887.
21. L. Turcani, A. Tarzia, F. T. Szczypiński and K. E. Kelfs, J. Chem. Phys., 2021, 154, 214102.
22. (a) https://github.com/lukasturcani/stk, version2022.1.26.0; (b) The Python code for the computational model generation is open-source at, https://github.com/andrewtarzia/big_unsymm.
23. (a) D. E. Coupry, M. A. Addicoat and T. Heine, J. Chem. Theory Comput., 2016, 12, 5215–5225; (b) M. A. Addicoat, N. Vankova, I. F. Aktera and T. Heine, J. Chem. Theory Comput., 2014, 10, 880–891; (c) J. D. Gale and A. L. Rohl, Mol. Simul., 2003, 29, 291–341; (d) J. D. Gale, J. Chem. Soc., Faraday Trans., 1997, 93, 629–637; (e) A. K. Rappe, C. J. Casewit, K. S. Colwell, W. A. Goddard III and W. M. Skiff, J. Am. Chem. Soc., 1992, 114, 10024–10035.
24. (a) C. Bannwarth, E. Caldeweyher, S. Ehlert, A. Hansen, P. Pracht, J. Seibert, S. Spicher and S. Grimme, Wires Comput. Mol. Sci., 2021, 11, e1493; (b) C. Bannwarth, S. Ehlert and S. Grimme, J. Chem. Theory Comput., 2019, 15, 1652–1671.
25. (a) S. Grimme, S. Ehrlich and L. Goerigk, J. Comput. Chem., 2011, 32, 1456–1465; (b) C. Adamo and V. Barone, J. Chem. Phys., 1999, 110, 6158–6170.
26. (a) J. Heyd, G. E. Scuseria and M. Ernzerhof, J. Chem. Phys., 2006, 124, 219906; (b) J. Heyd, G. E. Scuseria and M. Ernzerhof, J. Chem. Phys., 2006, 124, 219906.
27. F. Weigend and R. Ahlrichs, Phys. Chem. Chem. Phys., 2005, 7, 3297–3305.
28. The crystallographic analysis suggests the presence of chloride instead of nitrate counter ions. The origin of the chloride anions is not clear. Potentially, anion exchange with the glassware had occurred over the very long crystallization period (several months), or small amounts of chloride were present in the Pd(NO₃)₂ salt. For a related discussion, see: E. O. Bobylev, B. De Bruin and J. N. H. Reek, Inorg. Chem., 2021, 60, 12498–12505.
29. O. V. Dolomanov, J. L. Bourhis, R. J. Gildea, J. A. K. Howard and H. Puschmann, J. Appl. Crystallogr., 2009, 42, 339–341.
30. For examples, see: (a) R. Zaffaroni, E. O. Bobylev, R. Plessius, J. I. van der Vlugt and R. N. H. Reek, J. Am. Chem. Soc., 2020, 142, 8837–8847; (b) C.-L. Liu, E. O. Bobylev, Y. Fu, D. A. Poole III, K. Robeyns, C.-A. Fustin, Y. Garcia, J. N. H. Reek and M. L. Singleton, Chem.–Eur. J., 2020, 26, 11960–11965; (c) S. Gonell, X. Caumes, N. Orth, I. Ivanović-Burmazović and J. N. H. Reek, Chem. Sci., 2019, 10, 1316–1321; (d) S. Sato, M. Ikemi, T. Kikuchi, S. Matsumura, K. Shiba and M. Fujita, J. Am. Chem. Soc., 2015, 137, 12890–12896; (e) C. J. Bruns, D. Fujita, M. Hoshino, S. Sato, J. F. Stoddart and M. Fujita, J. Am. Chem. Soc., 2014, 136, 12027–12034; (f) C. Gütz, R. Hovorka, C. Klein, Q.-Q. Jiang, C. Bannwarth, M. Engeser, C. Schmuck, W. Assenmacher, W. Mader, F. Topíc, K. Rissanen, S. Grimme and A. Lützen, Angew. Chem., Int. Ed., 2014, 53, 1693–1698; (g) F. Jiang, N. Wang, Z. Du, J. Wang, Z. Lan and R. Yang, Chem.–Asian J., 2012, 7, 2230–2234; (h) K. Suzuki, M. Kawano, S. Sato and M. Fujita, J. Am. Chem. Soc., 2007, 129, 10652–10653; (i) T. Murase, S. Sato and M. Fujita, Angew. Chem., Int. Ed., 2007, 46, 1083–1085; (j) S. Sato, J. Iida, K. Suzuki, M. Kawano, T. Ozeki and M. Fujita, Science, 2006, 313, 1273–1276; (k) M. Tominaga, K. Suzuki, T. Murase and M. Fujita, J. Am. Chem. Soc., 2005, 127, 11950–11951; (l) M. Tominaga, K. Suzuki, M. Kawano, T. Kusukawa, T. Ozeki, S. Sakamoto, K. Yamaguchi and M. Fujita, Angew. Chem., Int. Ed., 2004, 43, 5621–5625.
31. (a) M. Han, Y. Luo, B. Damaschke, L. Gómez, X. Ribas, A. Jose, P. Peretzki, M. Seibt and G. H. Clever, Angew. Chem., Int. Ed., 2016, 55, 445–449; (b) H. Yokoyama, Y. Ueda, D. Fujita, S. Sato and M. Fujita, Chem.–Asian J., 2015, 10, 2292–2295; (c) J. Bunzen, J. Iwasa, P. Bonakdarzadeh, E. Numata, K. Rissanen, S. Sato and M. Fujita, Angew. Chem., Int. Ed., 2012, 51, 3161–3163.
32. The crystallographic analysis of a [Cu₆L₁₂]¹²⁺ cage with bridging 3-pyridinyl-triazole ligands showed also exclusive cis coordination at the six metal centers: Y. Wang, P. Cheng, Y. Song, D.-Z. Liao and S.-P. Yan, Chem.–Eur. J., 2007, 13, 8131–8138.

---

Footnote

† Electronic supplementary information (ESI) available: Containing synthetic procedures and experimental details. CCDC 2161290. For ESI and crystallographic data in CIF or other electronic format see https://doi.org/10.1039/d2sc03856k

---