Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Nature and strength of group-14 A–A′ bonds†
Daniela
Rodrigues Silva‡
 a,
Eva
Blokker‡
a,
Eva
Blokker‡
 a,
J. Martijn
van der Schuur
a,
J. Martijn
van der Schuur
 b,
Trevor A.
Hamlin
b,
Trevor A.
Hamlin
 a and
F. Matthias
Bickelhaupt
a and
F. Matthias
Bickelhaupt
 *acd
*acd
aDepartment of Chemistry and Pharmaceutical Sciences, AIMMS, Vrije Universiteit Amsterdam, De Boelelaan 1108, Amsterdam 1081 HZ, The Netherlands. E-mail: f.m.bickelhaupt@vu.nl; Web: https://www.theochem.nl
bPolymer Specialties, Nouryon, Zutphenseweg 10, Deventer 7418 AJ, The Netherlands
cInstitute of Molecules and Materials, Radboud University, Heyendaalseweg 135, Nijmegen 6525 AJ, The Netherlands
dDepartment of Chemical Sciences, University of Johannesburg, Auckland Park, Johannesburg 2006, South Africa
First published on 16th January 2024
Abstract
We have quantum chemically investigated the nature and stability of C–C and Si–Si bonds in R3A–AR3 (A = C, Si; R3 = H3, Me3, Me2Ph, MePh2, Ph3, t-Bu3) using density functional theory (DFT). Systematic increase of steric bulk of the substituents R has opposite effects on C–C and Si–Si bonds: the former becomes weaker whereas the latter becomes stronger. Only upon going further, from R = Ph to the bulkiest R = t-Bu, the R3Si–SiR3 bond begins to weaken. Our bonding analyses show how different behavior upon increasing the steric bulk of the substituents stems from the interplay of (Pauli) repulsive and (dispersion) attractive steric mechanisms. Extension of our analyses to other model systems shows that C–Si bonds display behavior that is in between that of C–C and Si–Si bonds. Further increasing the size of the group-14 atoms from C–C and Si–Si to Ge–Ge, Sn–Sn and Pb–Pb leads to a further decrease in the sensitivity of the bond strength with respect to the substituents' bulkiness. Our findings can be used as design principles for tuning A–A and A–A′ bond strengths.
Introduction
The carbon atom is not the most abundant element on earth. Yet, it plays a fundamental role in human life as it is the universal connector of organic compounds.1 The R3C–CR3 bond is remarkably strong and stable compared to other homodiatomic bonds.2 Furthermore, its strength can be easily tuned by modulation of the steric properties of the R groups. Thus, the R3C–CR3 bond is gradually weakened as the number and size of the R groups increase (e.g., from hydrogen to methyl to ethyl to isopropyl).3 However, if R becomes too bulky, the R3C˙ radicals do not dimerize because their mutual steric repulsion becomes too large in which case alternative bonding patterns occur. A remarkable example is the so-called hexaphenylethane (Ph3C–CPh3) riddle,4 where two Ph3C˙ radicals do not dimerize to form hexaphenylethane, although believed so for many decades.5 Instead, they form an unsymmetrical quinoid structure (i.e., {[4-(diphenylmethylene)cyclohexa-2,5-dien-1-yl]methane-triyl}tribenzene).6 Hexaphenylethane became over the years a theoretical construct used as a reference to explore the limits of the C–C bond length and strength.7 In a series of seminal works,8 Schreiner and coworkers showed that the C–C bond could be stabilized by increasing steric bulk in the all-meta-tert-butyl derivative of hexaphenylethane because of stabilizing dispersion interactions, also referred to as steric attraction.9Silicon is the third-period congener of, and therefore resembles in certain aspects, carbon. At the same time, silicon also exhibits vastly different bonding capacities.10 For example, silicon can readily form stable hypervalent compounds while carbon does not.11 Interestingly, contrary to the C–C bond, the Si–Si bond is strengthened in hexaphenyldisilane (Ph3Si–SiPh3) compared to sterically less congested analogs (e.g., H3Si–SiH3).12 The origin of this dichotomy has been attributed to common steric and electronic effects.13 Nevertheless, despite these efforts, the opposite behavior of the C–C versus the Si–Si bonds is still incompletely understood and lacks an overarching model that is soundly rooted in quantum mechanics.
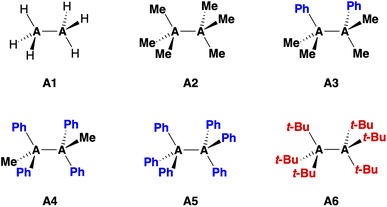
Herein, we investigate the bonding mechanism in the R3A–AR3 model systems (A = C, Si; R3 = H3, Me3, Me2Ph, MePh2, Ph3, t-Bu3; see eqn (1) and Fig. 1) as a function of the A–A bond distance using the activation strain model (ASM)14 in conjunction with Kohn–Sham molecular orbital theory (KS-MO)15 and a matching energy decomposition analysis (EDA).16
| R3A˙ + ˙AR3 → R3A–AR3 ΔH = −ΔHBDE | (1) |
We wish to understand why the C–C and Si–Si bonds behave differently upon increasing the steric bulk of the substituents. The crux turns out to be the fact that steric (Pauli) repulsion between substituents is a short-range interaction16a that is more important in the case of short bonds (i.e., C–C and C–R) whereas steric (dispersion) attraction is a long-range interaction9 that dominates in the case of longer bonds (Si–Si and Si–R). Indeed, C–Si bonds show behavior with respect to variation in the bulkiness of substituents R which is in between that of C–C and Si–Si bonds. Also, further increasing the size of the group-14 atoms along Ge–Ge, Sn–Sn and Pb–Pb shows an additional attenuation of the sensitivity of the bond strength with respect to the substituents' steric demand. The findings that emerge from our bonding analyses on a systematic set of R3A–AR3 model systems can be used as design principles for tuning the strength of A–A and A–A′ bonds.
Computational methods
Computational details
All calculations were performed using the Amsterdam Density Functional (ADF) program (ADF2019.305 for the A–A and constrained R3A–AR3 bonding analyses as well as mixed R3C–SiR3 and heavier R3A–AR3 (A = Ge, Sn, Pb) systems, whereas ADF2017.111 was used for all other computations).17 Geometries and energies were calculated at the BLYP level of the generalized gradient approximation (GGA).18 The DFT-D3(BJ) method developed by Grimme and coworkers,19 which contains the damping function introduced by Becke and Johnson,20 was used to correct for dispersion interactions. Molecular orbitals (MOs) were expanded using a large, uncontracted set of slater-type orbitals (STO): TZ2P.21 The TZ2P basis set is of triple-ζ quality, augmented by two sets of polarization functions. All electrons were treated variationally. The trends and conclusions emerging from our BLYP-D3(BJ)/TZ2P computations are nicely reproduced at the M06-2X22/TZ2P level (see Table S1 and Fig. S1†). The radical fragments were treated with a spin-unrestricted formalism. The accuracies of the fit scheme (Zlm fit)23 and the integration grid (Becke grid)24 were set to EXCELLENT. All geometry optimizations were performed in C1 symmetry without any symmetry constraint. All optimized structures were confirmed to be true minima (no imaginary frequencies) through vibrational analyses.25Thermochemistry
Bond enthalpies at 298.15 K and 1 atm (ΔH) were calculated from electronic bond energies (ΔE) and vibrational frequencies using standard thermochemistry relations for an ideal gas, according to eqn (2):26| ΔH = ΔE + ΔEtrans,298 + ΔErot,298 + ΔEvib,0 + Δ(ΔEvib,0)298 + Δ(pV) | (2) |
Activation strain model and energy decomposition analysis
In the activation strain model (ASM),14 the overall A–A bond energy ΔE [which also features in eqn (2)] between two radicals R3A˙ in R3A–AR3 is decomposed into two major components:| ΔE = ΔEstrain + ΔEint | (3) |
We further analyze the interaction energy ΔEint within the framework of the Kohn–Sham molecular orbital (KS-MO)15 model by dissecting it using our canonical energy decomposition analysis (EDA)16 scheme into electrostatic interactions, Pauli repulsion, (attractive) orbital interactions, dispersion corrections, and spin polarization:
| ΔEint = ΔVelstat + ΔEPauli + ΔEoi + ΔEdisp + ΔEspinpol | (4) |
The electrostatic energy ΔVelstat corresponds to the electrostatic interactions between the unperturbed charge distribution of the radical fragments R3A˙, which is usually attractive. The Pauli repulsion ΔEPauli comprises the destabilizing interactions between occupied orbitals and is responsible for any steric repulsion. The orbital interactions ΔEoi term accounts for electron-pair bonding (the SOMO–SOMO interaction), charge transfer (donor–acceptor interaction between an occupied orbital of one fragment with an empty orbital of the other fragment), and polarization (empty/occupied orbital mixing on one fragment due to the presence of another fragment). The dispersion energy ΔEdisp is added as a correction.19 Finally, the ΔEspinpol term refers to the spin polarization of the spin-α and spin-β electrons of the deformed unrestricted fragments and is with respect to ΔEint destabilizing (i.e., the deformed unrestricted fragments without spin polarization lie consistently 2–4 kcal mol−1 higher in energy and therefore have a too stabilizing ΔEint; see Table S2†).27 The open-shell PyFrag2019 program was used to analyze the bond dissociation as a function of the R3A–AR3 distance.28
Results and discussion
General trends in bond strength
The bond enthalpies ΔH [eqn (1)] under standard conditions (298.15 K and 1 atm) of the R3A–AR3 model systems (A = C, Si; R3 = H3, Me3, Me2Ph, MePh2, Ph3, t-Bu3) from our BLYP-D3(BJ)/TZ2P computations are collected in Table 1. The computed trends in ΔH, and hence in bond strength, agree very well with available experimental data (see Table S3†).29 We find that the Si–Si bond in Si1 is intrinsically weaker and longer than the C–C bond in C1 (ΔH = −71.4 kcal mol−1 and rSi–Si = 2.356 Å for Si1 and ΔH = −85.2 kcal mol−1 and rC–C = 1.538 Å for C1). Furthermore, the R3C–CR3 bond systematically weakens and elongates as the R groups are varied from hydrogen to methyl to phenyl, for example, from −85.2 kcal mol−1 and 1.538 Å for C1 to −4.6 kcal mol−1 and 1.738 Å for C5. The opposite trend emerges for R3Si–SiR3 along the same R series, that is, the Si–Si bond becomes slightly stronger from −71.4 kcal mol−1 for Si1 to −78.5 kcal mol−1 for Si5. The Si–Si bond length varies little and is ca. 2.36 Å in all cases. Only with the addition of the isotropically bulky tert-butyl group30 in Si6, the Si–Si bond does significantly weaken and stretch (ΔH = −41.0 kcal mol−1 and rSi–Si = 2.726 Å).31 The t-Bu groups in C6 encumber the dimerization of the t-Bu3C˙ radicals,32 which are known as persistent radicals.33| No. | Speciesb | ΔH | ΔE | ΔEstrain | ΔEint | r A–A |
|---|---|---|---|---|---|---|
| a Computed at BLYP-D3(BJ)/TZ2P at 298.15 K and 1 atm. All structures are staggered (for more details, see Table S4). b C6 does not form a stable C–C electron-pair bond. c Gauche conformation. d Anti conformation. | ||||||
| C1 | H3C–CH3 | −85.2 | −92.1 | 18.4 | −110.4 | 1.538 |
| C2 | Me3C–CMe3 | −64.0 | −69.6 | 25.0 | −94.6 | 1.597 |
C3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) c c |
PhMe2C–CMe2Ph | −42.1 | −46.8 | 39.7 | −86.5 | 1.627 |
| C4 | Ph2MeC–CMePh2 | −25.9 | −29.9 | 56.9 | −86.8 | 1.638 |
| C5 | Ph3C–CPh3 | −4.6 | −7.0 | 65.1 | −72.2 | 1.738 |
| Si1 | H3Si–SiH3 | −71.4 | −74.3 | 0.6 | −74.8 | 2.356 |
| Si2 | Me3Si–SiMe3 | −73.0 | −75.1 | 0.3 | −75.4 | 2.357 |
| Si3 | PhMe2Si–SiMe2Ph | −73.8 | −74.7 | 0.7 | −75.4 | 2.356 |
| Si4 | Ph2MeSi–SiMePh2 | −74.4 | −75.3 | 3.5 | −78.8 | 2.353 |
| Si5 | Ph3Si–SiPh3 | −78.5 | −79.8 | 1.2 | −81.0 | 2.358 |
| Si6 | t-Bu3Si–Sit-Bu3 | −41.0 | −45.8 | 15.0 | −60.8 | 2.726 |
The electronic bond energy ΔE is slightly more stabilizing than the ΔH, mainly due to zero-point vibrational energy effects in the latter, and always retains the same overall trends as the latter (Table 1). Therefore, to understand the origin of the aforementioned trends in C–C and Si–Si bond strengths, we analyze the features in the bonding mechanism that determine the trends in ΔE using the activation strain model (ASM),14 which decomposes ΔE into the strain energy ΔEstrain and the interaction energy ΔEint (eqn (3); see Computational methods for a theoretical overview). Inspection of the ASM terms in Table 1 reveals that, in all cases, the trends in bond strength ΔE emerge from the trends in the interaction energy ΔEint. For example, the Si–Si bond in Si1 is weaker than the C–C bond in C1 (ΔE = −74.3 kcal mol−1 and −92.1 kcal mol−1, respectively) because of a less stabilizing interaction between H3Si˙ radicals than between H3C˙ radicals (ΔEint = −74.8 kcal mol−1 for Si1 and −110.4 kcal mol−1 for C1). The weakening of the C–C bond from C1 to C5 (ΔE = −92.1 kcal mol−1 and −7.0 kcal mol−1, respectively) and strengthening of the Si–Si bond from Si1 to Si5 (ΔE = −74.3 kcal mol−1 and −79.8 kcal mol−1, respectively) also comes from the interaction energy that becomes less and more stabilizing, respectively (ΔEint = −110.4 kcal mol−1 for C1 and −72.2 kcal mol−1 for C5, whereas ΔEint = −74.8 kcal mol−1 for Si1 and −81.0 kcal mol−1 for Si5). Furthermore, the C–C bond strength is also determined by strain energy ΔEstrain. As will be discussed later, part of the less stabilizing ΔEint is absorbed into a more destabilizing ΔEstrain, which is associated with the pyramidalization of the R3A˙ radicals upon formation of the R3A–AR3 bond. Below, we systematically elucidate each of these features and provide their underlying physical mechanism.
Intrinsic bond strength
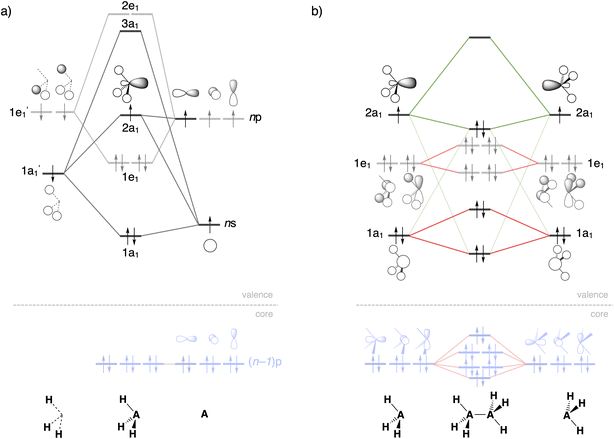
Before examining the effect of substituents, we wish to understand the difference in the intrinsic strength between the C–C and Si–Si bonds in the unsubstituted, archetypal model systems ethane C1 and disilane Si1. Why is the Si–Si bond in Si1 weaker than the C–C bond in C1, i.e., why is ΔE less stabilizing for the former than for the latter? To facilitate an equitable comparison of the studied systems to arrive at the actual causalities in their bonding mechanism, our bonding analyses are carried out as a function of the A–A bond distance from now on (Fig. 2). As will become clear in the following, our analyses reveal that the Si–Si bond in Si1 is weaker than the C–C bond in C1 due to the increase in effective atom size of Si compared to C and, thus, the increase in Pauli repulsion. This pushes the distance between the two Si atoms to a longer value and also weakens the bond compared to two C atoms. The bond weakening from C–C to Si–Si occurs despite an electron-pair bond that, at most bond distances, becomes stronger, not weaker, because of a larger bonding overlap between the spatially more extended (and diffuse) silyl SOMOs compared to the more compact methyl SOMOs. This is reminiscent of the weakening and lengthening of C–X bonds as X is varied from F to I.34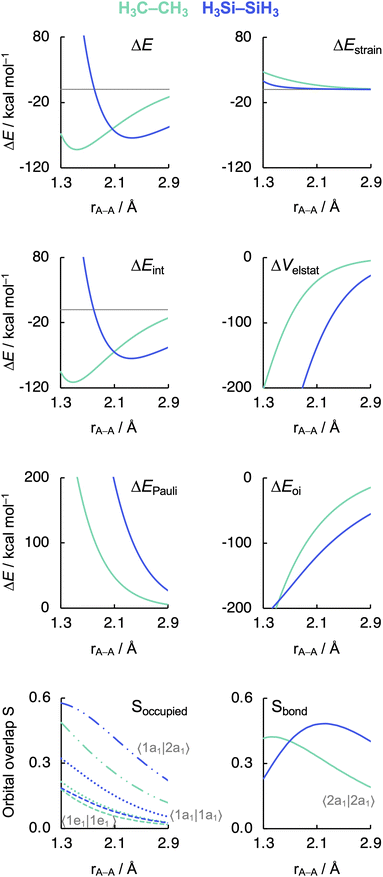 | ||
| Fig. 2 Energy decomposition analysis terms, main occupied–occupied orbital overlaps (Soccupied), and SOMO–SOMO overlap (Sbond) as a function of the A–A distance in H3A–AH3 (1, A = C, Si). The dispersion energy ΔEdisp is nearly constant and, therefore, not shown. See Fig. 3 for the schematic AH3˙ FMOs. Computed at BLYP-D3(BJ)/TZ2P. MO numbering starts at the lowest-energy valence AO combination. | ||
As can be seen in Fig. 2, the trends in the interaction energy ΔEint determine the trends in bond energy ΔE and cause the Si–Si bond to be weaker than the C–C bond. Therefore, we further analyze the bonding mechanism and the interaction energy ΔEint using quantitative Kohn–Sham MO theory15 and a matching canonical energy decomposition analysis (EDA),16 which dissects ΔEint into the electrostatic interactions ΔVelstat, Pauli repulsion ΔEPauli, and orbital interactions ΔEoi, among others (eqn (4); see Computational methods for a theoretical overview). Our quantitative MO and EDA analyses reveal a key role for the Pauli repulsion ΔEPauli behind the weaker Si–Si than C–C bond. In both cases, the main factor preventing the two atoms from coming closer than the equilibrium distance is Pauli repulsion between occupied closed-shell orbitals. The spatially more extended valence AOs of Si lead to the occurrence of a larger occupied–occupied orbital overlap at a longer A–A distance than in the case of the more compact valence AOs of C (Soccupied, see Fig. 2). In addition, Si has a larger number of closed-shell subvalence orbitals (Fig. 3). This situation gives rise to more ΔEPauli for Si–Si than for C–C, which pushes the Si–Si bond to a longer equilibrium distance, where all energy terms are weaker. Note that ΔEPauli is partially absorbed into the destabilizing strain energy ΔEstrain.35 The H3A˙ radicals pyramidalize to reduce the build-up of steric Pauli repulsion between the substituents as the A–A distance becomes shorter (see Fig. S2 and Table S5†). The ΔEstrain is always less destabilizing for Si1 than C1 because H3Si˙ is already pyramidal in its equilibrium geometry, while H3C˙ needs to pyramidalize from its planar equilibrium geometry upon formation of the C–C bond.36
Both electrostatic and orbital interactions (ΔVelstat and ΔEoi, respectively) are more stabilizing for Si1 than for C1, thus counteracting, but not overruling, the trends set by ΔEPauli. The Si atom has a large nuclear charge and electron cloud, which leads to a stronger electrostatic attraction between the electrons of one H3Si˙ fragment with the nuclei of the other H3Si˙ fragment than between two H3C˙ fragments, at any given bond distance.37 Furthermore, the spatially more extended valence AOs of Si lead to an earlier buildup of SOMO–SOMO bond overlap (Sbond) as the two fragments are approaching (see Fig. 2 and 3), although the cancelation of overlap also begins earlier. This situation results in a more stabilizing ΔEoi for Si1 than C1 at longer A–A distances. The stabilization of ΔEoi upon shortening the A–A distance below the optimum electron-pair bond overlap Sbond is due to other donor–acceptor orbital interactions (see Fig. S3†). The earlier onset of cancellation effects on the Si–Si bond overlap causes a shallower slope in the ΔEoi curve of the Si–Si bond, which therefore comes closer to, and crosses, the ΔEoi curve of the C–C bond which remains steeper at these short distances.
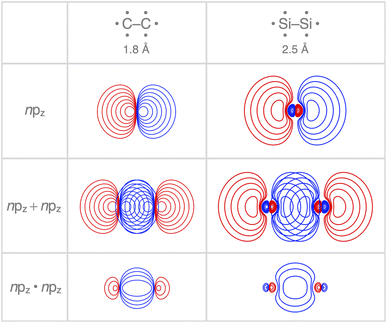
Interestingly, the maximum value of the Sbond overlap is also larger for Si than C (Sbond = 0.48 and 0.42, respectively). This effect can be traced back to the 〈npz|npz〉 overlap between the bare A–A atoms (A = C, Si; Fig. 4). Note that, at the A–A distance with maximum 〈npz|npz〉 (i.e., 1.8 Å and 2.5 Å for C–C and Si–Si, respectively), the C 2pz orbital crosses the nodal surface and begins to enter into an out-of-phase admixture with the rear lobe of the other C 2pz orbital (see red counter lines of the npz·npz overlap densities in Fig. 4). This cancellation of overlap does not occur to the same extent for Si as the radial node of the Si 3pz orbital (nonexistent in C 2pz) pushes the region of maximum amplitude of the 3pz lobe further away from the Si nucleus. This circumstance delays the out-of-phase overlap with the backside lobe of the 3pz AO of the other Si atom, resulting in a larger maximum 〈npz|npz〉 overlap and, therefore, a larger Sbond for the Si–Si bond than for the C–C bond. The trend from the C–C to the Si–Si bond continues for the heavier group-14 elements with increasingly larger maximum bond overlaps 〈npz|npz〉 at increasingly longer A–A distances going down group 14 from A = C and Si to Ge, Sn, and Pb (see Fig. S4 and S5†). The largest increase in maximum bond-overlap values, however, occurs in the step from period 2 to period 3 where, for the first time along the series, a p-core shell is introduced. Finally, note that the maximum Sbond occurs at a H3A–AH3 bond distance (A = C, Si) slightly shorter than the corresponding equilibrium bond length (Fig. 2). Again, it is the increasing Pauli repulsion at shorter distances that pushes the equilibrium bond lengths to a longer H3A–AH3 distance. Altogether, our findings once more highlight the well-known role of the Pauli repulsive orbital interactions in determining the length and strength of main-group element bonds.34,37b,38
Effect of steric hindrance
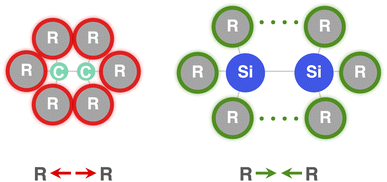
Now that we understand the difference in intrinsic R3C–CR3 and R3Si–SiR3 (R3 = H3) bonding mechanism, we evaluate the effect of changing the steric size of the R groups. All trends change systematically on going from R3 = H3 to t-Bu3. Hence, we focus our discussion mainly on R3 = H3 and Ph3 to pinpoint the origin of the opposite trends, that is, of decreasing C–C bond strength going from C1 to C5 and increasing Si–Si bond strength going from Si1 to Si5. As will become clear in the following, these opposite trends arise from the net steric interactions that are destabilizing for C (i.e., steric repulsion) and stabilizing for Si (i.e., steric attraction) because of the different A–R bond lengths (A = C, Si) and, consequently, R⋯R distances. Thus, if the R groups are in closer proximity, as in the C systems, due to the shorter C–C and C–R bond lengths, the interaction energy is dominated by the steric Pauli repulsion term, which causes a weakening of the C–C bond as R increases in size. On the other hand, when the R groups are farther apart from each other, as in the Si systems, due to the intrinsically longer Si–Si and Si–R bonds, the steric repulsion is smaller and becomes dominated by steric attraction, resulting in an overall more stabilizing interaction energy and, therefore, Si–Si bond strengthening from R3 = H3 to Ph3. Our findings support and extend earlier reports in the literature that discuss the interplay of repulsive and attractive steric effects in determining the length and strength of C–C bonds and other pheonomena.8,30The ASM and EDA terms as a function of the A–A distance for the R3A–AR3 systems (A = C, Si; R3 = H3, Ph3) are given in Fig. 5 (see Fig. S6–S8† for the complete data set). As can be seen in Fig. 5, the opposite trends, for R3Si–SiR3 compared to R3C–CR3, in bond strength ΔE going from R3 = H3 to Ph3 originates mainly from the interaction energy ΔEint. That is, from R3 = H3 to Ph3, ΔEint becomes less stabilizing for C–C (i.e., from full to dashed green lines) and more stabilizing for Si–Si (i.e., from full to dashed blue lines). The substitution of the H atoms by Ph groups in R3A–AR3 results in an increase in steric Pauli repulsion between the R3A˙ fragments. As R increases in size, the number of occupied–occupied orbital overlaps also increases, resulting in a larger ΔEPauli. As mentioned before, part of ΔEPauli is absorbed into the strain energy ΔEstrain as the R3A˙ fragments deform in response to the increasing steric repulsion (Fig. 5; see also Fig. S2 and Table S5†). In R3C–CR3, the R groups are in closer proximity due to the short C–R bonds and, therefore, this increase in steric repulsion is large enough to cause a weakening of the C–C bond going from C1 to C5 (Fig. 6). This effect is much less pronounced in R3Si–SiR3 simply because the R groups are farther removed from each other compared to R3C–CR3 (the Si–R bonds are longer than the C–R bonds; Fig. 6). If the C–C bond is artificially placed in the Si–Si geometry and vice versa, we observe that the trends in the interaction energy are reversed (Fig. S9†). That is, as R is varied from H to Ph, ΔEint becomes more stabilizing for C and less stabilizing for Si.
 | ||
| Fig. 5 Activation strain model (top row) and energy decomposition analysis (bottom row) as a function of the A–A distance in R3A–AR3 (A = C, Si; R3 = H3, Ph3) computed at BLYP-D3(BJ)/TZ2P. | ||
The much less pronounced increase in Pauli repulsion ΔEPauli going from R3 = H3 to Ph3 in R3Si–SiR3 allows for the long-range, weakly stabilizing interactions (see ΔVelstat and ΔEdisp in Fig. 5), also referred to as steric attraction,9 to take over and strengthen the Si–Si bond from Si1 to Si5. The ΔVelstat term is dominated by the nuclear–electron electrostatic attraction,37 which becomes more stabilizing as R increases in size. The same occurs for the dispersion energy ΔEdisp as larger substituent surfaces are in each other's proximity, close enough for dispersion interaction but not yet having large mutual closed-shell overlap. The only exception is the isotropically bulky tert-butyl group (i.e., R3 = t-Bu3), whose mutual closed-shell overlap and thus steric Pauli repulsion is large enough to cause a weakening even of the Si–Si bond (Fig. S7†).
Generalization
Finally, we evaluate the generality of the steric effects observed in the R3A–AR3 (A = C, Si; R3 = H3, Me3, Me2Ph, MePh2, Ph3, t-Bu3) model systems by extending our bonding analysis to mixed R3C–SiR3 (R3 = H3, Ph3, t-Bu3) and heavier R3A–AR3 (A = Ge, Sn, Pb; R3 = H3, Ph3, t-Bu3) systems. As can be seen in Fig. 7 (the complete dataset is provided in Table S6†), the C–Si bond shows behavior in between that of the C–C and the Si–Si bonds while still retaining the C–C bond sensitivity to steric hindrance. The H3C–SiH3 bond is somewhat weaker and longer (ΔH = −83.2 kcal mol−1 and rC–Si = 1.888 Å) than H3C–CH3 (ΔH = −85.2 kcal mol−1 and rC–C = 1.538 Å) while stronger and shorter than H3Si–SiH3 (ΔH = −71.4 kcal mol−1 and rSi–Si = 2.356 Å). This is again due to the increase in mutual steric Pauli repulsion between the radical fragment as one H3A˙ is varied from H3C˙ to H3Si˙ (Fig. S10†).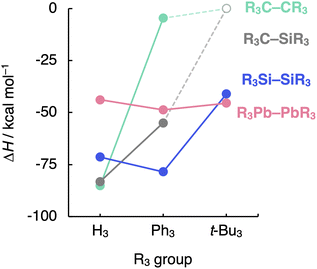 | ||
| Fig. 7 Bond enthalpies (ΔH) of the R3A–AR3 (A = C, Si, Pb; R3 = H3, Ph3, t-Bu3) systems computed at BLYP-D3(BJ)/TZ2P for A = C, Si and at ZORA-BLYP-D3(BJ)/TZ2P for A = Pb. | ||
On the other hand, the sensibility of the R3A–AR3 bond strength towards the substituents' bulkiness decreases as the central atom increases in size from C–C and Si–Si to Ge–Ge, Sn–Sn, and Pb–Pb (see Table S7† the complete dataset). Note that the R3Pb–PbR3 bond weakens by ca. 3 kcal mol−1 as R3 is varied from H3 to t-Bu3, while the R3Si–SiR3 bond weakens by almost 30 kcal mol−1 (Fig. 7). This is again due to the increase in the effective atom size of A and, therefore, the longer R⋯R distances. If the R groups are packed together, as in R3C–CR3, occupied–occupied orbital overlap is significant and the R3A–AR3 bond strength is dominated by steric repulsion between the substituents. If the R groups are further away from each other, as in R3Si–SiR3 and heavier analogs, that overlap becomes negligible, and dispersion takes over; thus, steric repulsion turns into steric attraction. But if the A–A and A–R bond becomes too long and, therefore, the R groups are too far removed from each other, both short-range repulsive and long-range attractive steric effects are weakened (see ASM and EDA terms in Fig. S11 and S12†), and the R3A–AR3 bond is almost insensitive to the size of R. The latter holds in particular for the Pb–Pb bond (see Fig. 7).
Conclusions
The Si–Si bond is intrinsically longer, weaker, and much less sensitive to substitution of H substituents for bulkier groups than the C–C bond. Thus, whereas the R3C–CR3 bond significantly weakens as R is varied from H to Me to Ph, the R3Si–SiR3 bond is somewhat strengthened along the same R series and only weakens when R is the isotropically bulky t-Bu group. This follows from our quantum chemical bonding analyses using dispersion-corrected density functional theory.The H3Si–SiH3 bond is longer and weaker than the H3C–CH3 bond because the larger number of occupied shells and the larger spatial extension of silicon's valence AOs cause an earlier onset of, and a stronger, steric Pauli repulsion that destabilizes the Si–Si bond and pushes it to a longer equilibrium distance. This trend and mechanism hold for the entire series of group-14 H3A–AH3 bonds which become weaker and longer along C–C, Si–Si, Ge–Ge, Sn–Sn, and Pb–Pb. Interestingly, this is so despite the electron-pair bonding overlap and orbital interactions becoming stronger, not weaker, along this series. The reason for the increasing bond overlap is the introduction of a radial node in the valence npz orbital from C to Si, which delays the occurrence of cancellation of bond overlap between the SOMOs, resulting in a larger maximum SOMO–SOMO overlap for Si.
When the hydrogen atoms in H3C–CH3 are replaced by larger R groups, the C–C bond is weakened due to a steep increase in steric Pauli repulsion between the R groups which is partially converted into strain energy associated with geometrical deformation of the R3C moieties in R3C–CR3. This increase in steric repulsion is less pronounced in R3Si–SiR3 as the R groups are farther removed from each other due to the longer Si–R bonds. Then, repulsive interactions are compensated by long-range attractive ones in R3Si–SiR3. Only R = t-Bu gives rise to steric repulsion that is large enough to cause a weakening of the R3Si–SiR3 bond. The sensitivity of the bond strength with respect to the substituents' bulkiness (even for R = t-Bu) further decreases going down group-14 due to the increasingly longer A–R bonds. Our findings nicely equip chemists with the rational design principles to tune the strength of A–A and A–A′ bonds at will.
Data availability
All data of this study are available in the main text and ESI.†Author contributions
FMB and JMS conceived the project, which was supervised by TAH and FMB. DRS and EB carried out the quantum chemical computations and bonding analyses. DRS also drafted the manuscript. DRS, TAH, and FMB discussed all results. All authors reviewed the manuscript.Conflicts of interest
There are no conflicts of interest to declare.Acknowledgements
This work is part of the Advanced Research Center for Chemical Building Blocks (ARC CBBC; grant 2018.019.B), which is co-founded and co-financed by the Dutch Research Council (NWO) and the Netherlands Ministry of Economic Affairs and Climate Policy. This work was carried out on the Dutch national e-infrastructure with the support of SURF Cooperative. Furthermore, we thank Dr Lucas de Azevedo Santos and Dr Pascal Vermeeren for helpful discussions.References
- J. Clayden, N. Greeves and S. G. Warren, Organic Chemistry, Oxford University Press, Oxford, 2012 Search PubMed.
- Y.-R. Luo, Comprehensive Handbook of Chemical Bond Energies, CRC Press, Boca Raton, 2007 Search PubMed.
- C. Rüchardt and H.-D. Beckhaus, Angew. Chem., Int. Ed., 1980, 19, 429 ( Angew. Chem. , 1980 , 92 , 417 ) CrossRef.
- J. M. McBride, Tetrahedron, 1974, 30, 2009 CrossRef CAS , and references cited therein.
- M. Gomberg, J. Am. Chem. Soc., 1900, 22, 757 CrossRef.
- (a) H. Lankamp, W. T. Nauta and C. MacLean, Tetrahedron Lett., 1968, 9, 249 CrossRef; (b) P. Jacobsen, Ber. Dtsch. Chem. Ges., 1905, 38, 196 CrossRef.
- (a) S. Grimme and P. R. Schreiner, Angew. Chem., Int. Ed., 2011, 50, 12639 ( Angew. Chem. , 2011 , 123 , 12849 ) CrossRef CAS PubMed; (b) Y. Uchimura, T. Takeda, R. Katoono, K. Fujiwara and T. Suzuki, Angew. Chem., Int. Ed., 2015, 54, 4010 ( Angew. Chem. , 2015 , 127 , 4082 ) CrossRef CAS; (c) S. Rösel, C. Balestrieri and P. R. Schreiner, Chem. Sci., 2017, 8, 405 RSC; (d) E. Dames, B. Sirjean and H. Wang, J. Phys. Chem. A, 2010, 114, 1161 CrossRef CAS PubMed.
- (a) P. R. Schreiner, L. V. Chernish, P. A. Gunchenko, E. Yu. Tikhonchuk, H. Hausmann, M. Serafin, S. Schlecht, J. E. P. Dahl, R. M. K. Carlson and A. A. Fokin, Nature, 2011, 477, 308 CrossRef CAS PubMed; (b) J. P. Wagner and P. R. Schreiner, Angew. Chem., Int. Ed., 2015, 54, 12274 ( Angew. Chem. , 2015 , 127 , 12446 ) CrossRef CAS; (c) S. Rösel, J. Becker, W. D. Allen and P. R. Schreiner, J. Am. Chem. Soc., 2018, 140, 14421 CrossRef PubMed; (d) E. Solel, M. Ruth and P. R. Schreiner, J. Org. Chem., 2021, 86, 7701 CrossRef CAS.
- (a) R. Hoffmann, C. C. Levin and R. A. Moss, J. Am. Chem. Soc., 1973, 95, 629 CrossRef CAS; (b) R. R. Sauers, J. Chem. Educ., 1996, 73, 114 CrossRef CAS.
- (a) M. Karni, Y. Apeloig, J. Kapp and P. von R. Schleyer, in The Chemistry of Organic Silicon Compounds, ed. Z. Rappoport and Y. Apeloig, John Wiley & Sons, 2001, pp. 1–163 Search PubMed; (b) Y. Apeloig, in The Chemistry of Organic Silicon Compounds, ed. S. Patai and Z. Rappoport, John Wiley & Sons, 1989, pp. 57–225 Search PubMed.
- S. C. A. H. Pierrefixe, C. Fonseca Guerra and F. M. Bickelhaupt, Chem.–Eur. J., 2008, 14, 819 CrossRef PubMed.
- (a) L. M. Calle and A. S Kana'an, J. Chem. Thermodyn., 1974, 6, 935 CrossRef CAS; (b) J. M. Gaidis, P. R. Briggs and T. W. Shannon, J. Phys. Chem., 1971, 75, 974 CrossRef CAS; (c) H. Gilman, R. K. Ingham and A. G. Smith, J. Org. Chem., 1953, 18, 1743 CrossRef CAS; (d) H. Gilman and T. C. Wu, J. Am. Chem. Soc., 1951, 73, 4031 CrossRef CAS.
- (a) B. Tumanskii, M. Karni and Y. Apeloig, in Organosilicon Compounds: Theory and Experiment (Synthesis), ed. V. Ya. Lee, Elsevier, 2017, pp. 231–294 Search PubMed; (b) C. Rüchardt and H.-D. Beckhaus, Synthetic organic chemistry, in Topics in Current Chemistry, Springer, Berlin, Heidelberg, 1980, vol. 130, pp. 1–22 Search PubMed.
- (a) P. Vermeeren, T. A. Hamlin and F. M. Bickelhaupt, Chem. Commun., 2021, 57, 5880 RSC; (b) P. Vermeeren, S. C. C. van der Lubbe, C. Fonseca Guerra, F. M. Bickelhaupt and T. A. Hamlin, Nat. Protoc., 2020, 15, 649 CrossRef CAS PubMed; (c) F. M. Bickelhaupt and K. N. Houk, Angew. Chem., Int. Ed., 2017, 56, 10070 ( Angew. Chem. , 2017 , 129 , 10204 ) CrossRef CAS PubMed; (d) L. P. Wolters and F. M. Bickelhaupt, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2015, 5, 324 CAS; (e) I. Fernández and F. M. Bickelhaupt, Chem. Soc. Rev., 2014, 43, 4953 RSC; (f) W.-J. van Zeist and F. M. Bickelhaupt, Org. Biomol. Chem., 2010, 8, 3118 RSC; (g) F. M. Bickelhaupt, J. Comput. Chem., 1999, 20, 114 CrossRef CAS.
- (a) R. van Meer, O. V. Gritsenko and E. J. Baerends, J. Chem. Theory Comput., 2014, 10, 4432 CrossRef CAS PubMed; (b) T. A. Albright, J. K. Burdett and W. H. Wangbo, Orbital Interactions in Chemistry, Wiley, New York, 2013 CrossRef.
- (a) T. A. Hamlin, P. Vermeeren, C. Fonseca Guerra and F. M. Bickelhaupt, in Complementary Bonding Analysis, ed. S. Grabowsky, De Gruyter, Berlin, 2021, pp. 199–212 Search PubMed; (b) F. M. Bickelhaupt and E. J. Baerends, in Reviews in Computational Chemistry, ed. K. B. Lipkowitz and D. B. Boyd, Wiley–VCH, Hoboken, 2000, pp. 1–86 Search PubMed.
- (a) ADF2017.111/2019.305, SCM, Theoretical Chemistry, Vrije Universiteit Amsterdam, The Netherlands, http://www.scm.com Search PubMed; (b) G. te Velde, F. M. Bickelhaupt, E. J. Baerends, C. Fonseca Guerra, S. J. A. van Gisbergen, J. G. Snijders and T. Ziegler, J. Comput. Chem., 2001, 22, 931 CrossRef CAS; (c) C. Fonseca Guerra, J. G. Snijders, G. te Velde and E. J. Baerends, Theor. Chem. Acc., 1998, 99, 391 Search PubMed.
- (a) A. D. Becke, Phys. Rev. A, 1988, 38, 3098 CrossRef CAS PubMed; (b) C. T. Lee, W. T. Yang and R. G. Parr, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785 CrossRef CAS PubMed.
- (a) S. Grimme, J. Antony, S. Ehrlich and H. Krieg, J. Chem. Phys., 2010, 132, 154104 CrossRef PubMed; (b) S. Grimme, S. Ehrlich and L. Goerigk, J. Comput. Chem., 2011, 32, 1456 CrossRef CAS PubMed.
- E. R. Johnson and A. D. Becke, J. Chem. Phys., 2005, 123, 024101 CrossRef.
- E. van Lenthe and E. J. Baerends, J. Comput. Chem., 2003, 24, 1142 CrossRef CAS.
- Y. Zhao and D. G. Truhlar, Theor. Chem. Acc., 2008, 120, 215 Search PubMed.
- M. Franchini, P. H. T. Philipsen, E. van Lenthe and L. Visscher, J. Chem. Theory Comput., 2014, 10, 1994 CrossRef CAS PubMed.
- M. Franchini, P. H. T. Philipsen and L. Visscher, J. Comput. Chem., 2013, 34, 1819 CrossRef CAS PubMed.
- (a) A. Bérces, R. M. Dickson, L. Fan, H. Jacobsen, D. Swerhone and T. Ziegler, Comput. Phys. Commun., 1997, 100, 247 CrossRef; (b) H. Jacobsen, A. Bérces, D. P. Swerhone and T. Ziegler, Comput. Phys. Commun., 1997, 100, 263 CrossRef CAS; (c) S. K. Wolff, Int. J. Quantum Chem., 2005, 104, 645 CrossRef CAS.
- (a) P. W. Atkins and J. de Paula, Physical Chemistry, W. H. Freeman, New York, 9th edn, 2010 Search PubMed; (b) F. Jensen, Introduction to Computational Chemistry, Wiley, 2007 Search PubMed.
- F. M. Bickelhaupt, M. Solà and C. Fonseca Guerra, Faraday Discuss., 2007, 135, 451 RSC.
- (a) W. J. van Zeist, C. Fonseca Guerra and F. M. Bickelhaupt, J. Comp. Chem., 2008, 29, 312 CrossRef CAS PubMed; (b) X. Sun, T. M. Soini, J. Poater, T. A. Hamlin and F. M. Bickelhaupt, J. Comput. Chem., 2019, 40, 2227 CrossRef CAS; (c) X. Sun, T. Soini, L. P. Wolters, W.-J. van Zeist, C. Fonseca Guerra, T. A. Hamlin and F. M. Bickelhaupt, PyFrag 2019, Vrije Universiteit Amsterdam, The Netherlands Search PubMed.
- A comparison between the ΔHBDE at BLYP-D3(BJ)/TZ2P computed herein with experimental data is provided in Table S3 of the ESI.† See, for example: (a) M. Szwarc, Proc. R. Soc. Lond. A, 1951, 207, 5 CrossRef CAS; (b) R. Walsh, Acc. Chem. Res., 1981, 14, 246 CrossRef CAS.
- L. P. Wolters, R. Koekkoek and F. M. Bickelhaupt, ACS Catal., 2015, 5, 5766 CrossRef CAS.
- The Si–Si bond in Si6 is known to be unusually long (rSi–Si = 2.687 Å by X-ray data). See: N. Wiberg, H. Schuster, A. Simon and K. Peters, Angew. Chem., Int. Ed., 1986, 25, 79 ( Angew. Chem. , 1986 , 98 , 100 ) CrossRef.
- C6 does not form a stable C–C electron-pair bond. See Table S4 in the ESI.†.
- D. Griller and K. U. Ingold, Acc. Chem. Res., 1976, 9, 13 CrossRef CAS.
- E. Blokker, X. Sun, J. Poater, J. M. van der Schuur, T. A. Hamlin and F. M. Bickelhaupt, Chem.–Eur. J., 2021, 27, 15616 CrossRef CAS PubMed.
- (a) E. Blokker, W.-J. van Zeist, X. Sun, J. Poater, J. M. van der Schuur, T. A. Hamlin and F. M. Bickelhaupt, Angew. Chem., Int. Ed., 2022, 61, e202207477 ( Angew. Chem. , 2022 , 134 , e202207477 ) CrossRef CAS PubMed; (b) W.-J. van Zeist and F. M. Bickelhaupt, Phys. Chem. Chem. Phys., 2009, 11, 10317 RSC.
- The methyl radical fragment is planar to minimize steric repulsion between the hydrogen substituents. See: F. M. Bickelhaupt, T. Ziegler and P. von Ragué Schleyer, Organometallics, 1996, 15, 1477 CrossRef CAS.
- For a detailed discussion on the behavior of ΔVelstat along the formation of a chemical bond, see: (a) G. Frenking and F. M. Bickelhaupt, in The Chemical Bond: Fundamental Aspects of Chemical Bonding, ed. G. Frenking and S. Shaik, Wiley-VCH, Weinheim, 2014, pp. 121–158 Search PubMed; (b) A. Krapp, F. M. Bickelhaupt and G. Frenking, Chem.–Eur. J., 2006, 12, 9196 CrossRef CAS PubMed.
- (a) P. Vermeeren, W.-J. van Zeist, T. A. Hamlin, C. Fonseca Guerra and F. M. Bickelhaupt, Chem.–Eur. J., 2021, 27, 7074 CrossRef CAS; (b) F. M. Bickelhaupt, R. L. DeKock and E. J. Baerends, J. Am. Chem. Soc., 2002, 124, 1500 CrossRef CAS PubMed.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d3sc06215e |
| ‡ These authors contributed equally to this work. |
| This journal is © The Royal Society of Chemistry 2024 |