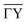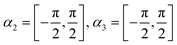Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Surface electronic corrugation of a one-dimensional topological metal: Bi(114)
Stephan J.
Schmutzler
 ab,
Adrian
Ruckhofer
ab,
Adrian
Ruckhofer
 *a,
Wolfgang E.
Ernst
*a,
Wolfgang E.
Ernst
 a and
Anton
Tamtögl
a and
Anton
Tamtögl
 *a
*a
aInstitute of Experimental Physics, Graz University of Technology, 8010 Graz, Austria. E-mail: ruckhofer@tugraz.at; tamtoegl@gmail.com
bFreie Universität Berlin, Fachbereich Physik, Arnimallee 14, 14195 Berlin, Germany
First published on 9th February 2022
Abstract
The surface of Bi(114) is a striking example where the reduced dimensionality gives rise to structural rearrangement and new states at the surface. Here, we present a study of the surface structure and electronic corrugation of this quasi one-dimensional topological metal based on helium atom scattering (HAS) measurements. In contrast to low-index metal surfaces, upon scattering from the stepped (114) truncation of Bi, a large proportion of the incident beam is scattered into higher order diffraction channels which in combination with the large surface unit cell makes an analysis challenging. The surface electronic corrugation of Bi(114) is determined, using measurements upon scattering normal to the steps, together with quantum mechanical scattering calculations. Therefore, minimisation routines that vary the shape of the corrugation are employed, in order to minimise the deviation between the calculations and experimental scans. Furthermore, we illustrate that quantum mechanical scattering calculations can be used to determine the orientation of the in- and outgoing beam with respect to the stepped surface structure.
1 Introduction
An attractive path to study systems of reduced dimensionality is to create them on the surfaces of semiconducting or semimetallic substrates. Many systems have been realised and studied in this way recently, such as metallic chains or graphene nanoribbons on semiconductors.1 In fact, such systems with reduced dimensionality, have often been the key to the discovery of fundamentally new physics. The formation of the surface itself may give rise to drastic changes, e.g., for polar semiconductors faceting is expected and unique surface orientations forming low-energy vicinal surfaces have been observed, in order to account for the unstable bulk termination.2The semimetal surfaces of Bi and Sb are prominent examples of materials where the physical and chemical properties are radically different from those of the corresponding bulk material. E.g. in both materials surface electronic states exist3–5 and in the case of Sb(111), charge density waves have been observed.6 Both Bi and Sb are also elemental building blocks of binary topological insulators with their unique electronic structure which exhibits a protected conducting surface as well as insulating bulk states.5,7–14 More importantly, stepped surfaces are particularly interesting for the realisation of quasi one-dimensional systems15 and they provide an ideal playground to study e.g. site-specific catalytic reactivity16–18 or ice formation at a highly corrugated adsorption template.19
In this work we describe a study of the stepped (114) truncation of Bi (Fig. 1), which is a quasi-one dimensional topological metal.20 The surface undergoes a (1 × 2) reconstruction at room temperature resulting in a unit cell length of 28.4 Å normal to the steps ( in Fig. 1(a)). Such a large unit cell makes any detailed quantitative analysis and modelling approaches particularly challenging. We rely on scattering experiments which are strictly surface sensitive and in addition to the surface structure provide information about the surface electronic corrugation and the atom-surface interaction potential.21–23 The latter are necessary prerequisites for any quantitative description and theoretical treatment of molecular adsorption,24 scattering approaches to chemisorption25,26 and the coordinates relevant to the reaction potential27–29 within the wide aspects of physical chemistry. We provide an in-depth analysis of the experimentally measured scattering intensities based on quantum-mechanical scattering calculations.
in Fig. 1(a)). Such a large unit cell makes any detailed quantitative analysis and modelling approaches particularly challenging. We rely on scattering experiments which are strictly surface sensitive and in addition to the surface structure provide information about the surface electronic corrugation and the atom-surface interaction potential.21–23 The latter are necessary prerequisites for any quantitative description and theoretical treatment of molecular adsorption,24 scattering approaches to chemisorption25,26 and the coordinates relevant to the reaction potential27–29 within the wide aspects of physical chemistry. We provide an in-depth analysis of the experimentally measured scattering intensities based on quantum-mechanical scattering calculations.
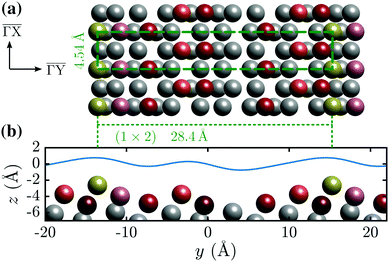 | ||
Fig. 1 (a) Top view of the (1 × 2) reconstructed Bi(114) surface. (b) Side view of the atomic structure of Bi(114) surface along the  -direction, illustrating the asymmetry upon scattering normal to the steps. An exemplary corrugation function (eqn (3)) using j = 3 terms with h = [0.435,0.43,0.14] and -direction, illustrating the asymmetry upon scattering normal to the steps. An exemplary corrugation function (eqn (3)) using j = 3 terms with h = [0.435,0.43,0.14] and  is drawn as solid blue line above the side view of the atomic structure. is drawn as solid blue line above the side view of the atomic structure. | ||
2 Experimental and computational details
2.1 Experimental details
The Bi(114) crystal was cleaned by repeated circles of Ar+-sputtering and annealing to 400 K until clear diffraction peaks during helium atom scattering (HAS) were observed. The HAS measurements were performed by scattering a nearly monochromatic beam of He (ΔE/E ≈ 2%) off the sample surface in a fixed 91.5° source-sample-detector geometry (for a detailed description refer to ref. 30). The angular diffraction scans (ϑ-scans) were produced by rotating the sample in the scattering plane to yield different incident angles. The diffraction scans were performed either at room temperature (300 K) or at cryogenic temperatures (113 K) via a thermal connection to a liquid nitrogen reservoir. -direction. The side view along
-direction. The side view along  (Fig. 1(b)) reveals the stepped structure of the (114) truncation with an additional step (protrusion) within the unit cell.
(Fig. 1(b)) reveals the stepped structure of the (114) truncation with an additional step (protrusion) within the unit cell.
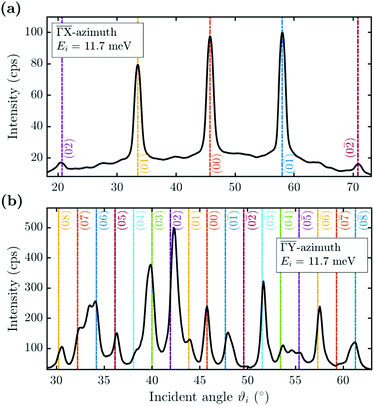
He scattering occurs from the surface electronic corrugation above the ion cores,21,32,33 as illustrated by the exemplary corrugation in Fig. 1(b). Elastic diffraction scans parallel to the steps give rise to a diffraction scan similar to the one obtained for the Bi(111) surface34 – as shown in Fig. 2(a) for the  -azimuth with the specular reflection at 45.75°. The individual diffraction peaks are labelled with the respective interacting G-vector. Additional small features in between the main diffraction peaks arise due to a dimerisation at low temperatures.1,31 This (2 × 2) reconstruction is caused by the
-azimuth with the specular reflection at 45.75°. The individual diffraction peaks are labelled with the respective interacting G-vector. Additional small features in between the main diffraction peaks arise due to a dimerisation at low temperatures.1,31 This (2 × 2) reconstruction is caused by the  intervalley electron–phonon coupling.35 From the temperature dependence of the Debye–Waller exponent of the specular intensity in
intervalley electron–phonon coupling.35 From the temperature dependence of the Debye–Waller exponent of the specular intensity in  theoretical calculations determined the electron–phonon coupling constant to be λ = 0.45 when treating the system as a 1D free-electron gas.35
theoretical calculations determined the electron–phonon coupling constant to be λ = 0.45 when treating the system as a 1D free-electron gas.35
Scattering normal to the steps  as shown in Fig. 2(b) on the other hand, gives rise to a multitude of diffraction peaks due to the large unit cell. Diffraction peaks up to 8th order were resolved at incident energies between Ei = 10–15 meV. It is noteworthy that in Fig. 2(b), some diffraction peak positions do not exactly coincide with the calculated G-vector positions. A possible explanation is the effect of the the large unit cell in combination with the kinematic and angular broadening of the individual peaks, causing some of the maxima to be obscured by the shoulders of the next-nearest peaks. Additionally, selective adsorption resonances may also cause additional peaks. Due to the large “asymmetric” corrugation along
as shown in Fig. 2(b) on the other hand, gives rise to a multitude of diffraction peaks due to the large unit cell. Diffraction peaks up to 8th order were resolved at incident energies between Ei = 10–15 meV. It is noteworthy that in Fig. 2(b), some diffraction peak positions do not exactly coincide with the calculated G-vector positions. A possible explanation is the effect of the the large unit cell in combination with the kinematic and angular broadening of the individual peaks, causing some of the maxima to be obscured by the shoulders of the next-nearest peaks. Additionally, selective adsorption resonances may also cause additional peaks. Due to the large “asymmetric” corrugation along  the diffraction scans are not symmetric around the specular reflection either. Moreover, the highest intensity is typically scattered into a different diffraction channel than the (00) channel, analogous to scattering form a blazed grating in optics.36,37 While higher ratios of scattered intensity between nonzeroth-order diffraction and the specular peak have been demonstrated to be correlated with increased surface corrugation alone,38 the emerging beam resonance effect upon scattering of atomic and molecular beams from blazed gratings has been studied by Schöllkopf and coworkers.39 For the latter effect it was shown that the ratio of intensities scattered into specific diffraction channels depends strongly on the details of the particle surface interaction, based on quantum mechanical scattering calculations.40
the diffraction scans are not symmetric around the specular reflection either. Moreover, the highest intensity is typically scattered into a different diffraction channel than the (00) channel, analogous to scattering form a blazed grating in optics.36,37 While higher ratios of scattered intensity between nonzeroth-order diffraction and the specular peak have been demonstrated to be correlated with increased surface corrugation alone,38 the emerging beam resonance effect upon scattering of atomic and molecular beams from blazed gratings has been studied by Schöllkopf and coworkers.39 For the latter effect it was shown that the ratio of intensities scattered into specific diffraction channels depends strongly on the details of the particle surface interaction, based on quantum mechanical scattering calculations.40
2.2 Computational details
Starting point for the elastic CC approach is the time-independent Schrödinger equation, where upon insertion of a Fourier series expansion of the surface potential and the wave function, a set of coupled equations for the outgoing waves is obtained. The system treats each scattering event as a separate channel, described by a surface G-vector, where channels describing measurable peaks are “open”. Though kinematically forbidden and thus not directly accessible, evanescent or “closed” channels receive significant scattering contributions and their consideration is important for numerical convergence. Thus the wavefunctions are numerically solved for in the CC-algorithm, for a finite set of these channels.42,43 Appendix B outlines the number of channels considered as well as the integration boundaries handed to the algorithm. The method of solving the equations has been widely discussed in previous publications,22,42–46 and is thus not described in the following. Before comparison with measured data, the elastic CC-calculations further need to be corrected for the Debye–Waller attenuation, using an experimentally determined Debye–Waller factor 2W.
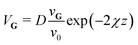 | (1) |
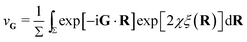 | (2) |
In this work, a one-dimensional simplification of the CC-formalism was applied, for the calculation of several diffraction scans along the  -direction, in order to make the problem computationally tractable. Following the ideas from Miret-Artes et al.,32 the corrugation function of a stepped or vicinal surface, can be modelled according to:
-direction, in order to make the problem computationally tractable. Following the ideas from Miret-Artes et al.,32 the corrugation function of a stepped or vicinal surface, can be modelled according to:
 | (3) |
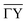 -direction.
-direction.
Fig. 1(b) shows such a function with j = 3 terms, h = [0.435,0.43,0.14] and 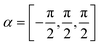 above the
above the 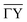 -direction of the Bi(114) surface. It can be seen that three terms are sufficient to reproduce the overall asymmetry of this particular surface, including the protrusion by the atomic row at approximately half the distance of the main modulation.
-direction of the Bi(114) surface. It can be seen that three terms are sufficient to reproduce the overall asymmetry of this particular surface, including the protrusion by the atomic row at approximately half the distance of the main modulation.
Treating terms with j > 1 as perturbation terms and inserting the resulting expression into eqn (2) yields:
 | (4) |
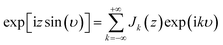 | (5a) |
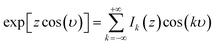 | (5b) |
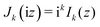 | (5c) |
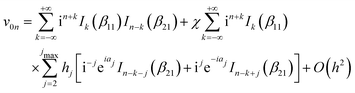 | (6) |
3 Results
A multitude of elastic diffraction scans upon scattering normal to the steps such as in Fig. 2(b) was recorded, with varying incident beam energy Ei. These angular scattering distributions measured via ϑ-scans along the -direction of the sample, are then further analysed based on quantum-mechanical scattering calculations, employing the CC-algorithm.
-direction of the sample, are then further analysed based on quantum-mechanical scattering calculations, employing the CC-algorithm.
Fig. 3(a) shows a typical diffraction scan with the coloured vertical lines denoting the interacting G-vector. In Fig. 3(b) the corresponding pseudo-Voigt profiles as the result from a best fit for the overall representation of the above scan, following the curve_fit function from the scipy.optmize package, are plotted. Due to the broadening of the elastic peaks caused by the energy spread of the helium beam and the broadening caused by the apparatus as well as the domain size of the crystal surface, the experimental peak areas, rather than the peak maxima, were used for comparison with the calculated values. Therefore, the peak areas and their respective errors were calculated (see Appendix A for details), normalised to the specular peak and corrected for the Debye–Waller attenuation finally resulting in the experimental intensities Iexp. The Debye–Waller correction was determined via the analysis of several elastic measurements of the specular intensity, I(TS), at a fixed incident energy of 11.08 meV and for various sample temperatures TS.41,48,49 The slope γ was then extracted from a plot of the natural logarithm of the intensity ln[I(TS)] versus TS. The Debye–Waller correction was applied via I(0) = I(TS)exp[2W], with the Debye–Waller factor 2W = γTS following from the experimentally determined slope γ = (−6.0 ± 0.1) × 10−3 K−1.
Earlier works showed that the position of the rainbow angle in a diffraction scan gives an estimate for the corrugation height of a surface.50–52 In fact, a peak-to-peak corrugation of about 0.5 Å yields a rainbow angle of 6.2° and would thus be consistent with the high intensities of the (03) diffraction peaks in Fig. 3(a). However, the simple assumptions made above do neither hold for the herein used scattering geometry nor the asymmetry of the surface corrugation. Moreover, such an analysis, based on a hard wall assumption, does not reflect a realistic atom-surface potential and cannot account for any beam energy dependence of the corrugation. Therefore, we employ quantum mechanical scattering calculations as outlined in the following.
3.1 Surface electronic corrugation
To reproduce the measured scattering intensities, the aim of the calculations was to find a corrugation function ξ(y) as described by eqn (3), ultimately resulting in a set of coupling parameters that determine the amount of intensity scattered into each channel. The shape of ξ(y) is determined by the terms hj and αj, resulting in six fit parameters h1–3 and α1–3. To avoid additional fit parameters,32 values for D and χ were fixed at 7.898 meV and 0.884 Å−1 respectively, as found by Kraus et al.46 for the Bi(111) surface. Since these values are largely governed by the polarisability of the surface atoms, these should therefore not differ greatly depending on the surface plane. Additionally, since the leading term of the vG coefficients can always be defined as real, by a change in origin53 which in this case is defined by α1, its value was fixed to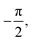 further reducing the optimisation problem to five fit parameters. Minimisation routines, as described in Appendix B, were then employed to find the optimal set of hj and αj.
further reducing the optimisation problem to five fit parameters. Minimisation routines, as described in Appendix B, were then employed to find the optimal set of hj and αj.
Four diffraction scans, at incident energies of 10.58, 11.17, 11.71 and 13.22 meV, measured for a cooled sample (sample temperature TS ≈ 113 K) were analysed. Diffraction scans at higher incident energies were also recorded, however they showed a significant decrease in resolution and the returned peak fits were unsuitable. Furthermore, not all diffraction intensities of a single scan were handed to the calculations, on the one hand to keep the computational time reasonable and on the other hand because not all experimental peaks showed an adequate fit, partly caused by the experimental uncertainties/resolution.
Fig. 4 compares the experimental intensities of four scans (with increasing beam enery Ei) with the calculated diffraction intensities for the optimised corrugation functions, as well as the respective deviation between simulation and experiment σs which was calculated via:
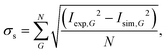 | (7) |
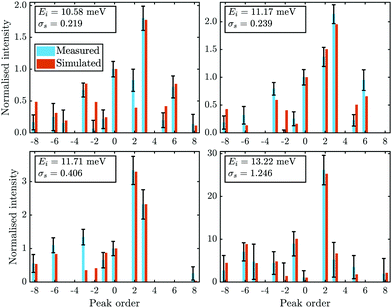 | ||
Fig. 4 Comparison of the measured (blue bars, see text for details) and the simulated peak intensities (orange bars) for the diffraction peaks along  handed to the optimisation routines (see Fig. 5 for comparison). The error bars of the measurements were calculated with eqn (9). handed to the optimisation routines (see Fig. 5 for comparison). The error bars of the measurements were calculated with eqn (9). | ||
The best fit values of hj and αj for the four scans are given in Table 1. No uncertainties are presented at this point, as the values in Table 1 are simply the values of the minimum found by the routines. However, it was found that varying h1 and h2 in the 10−4 order of magnitude resulted in an increase of σs by approximately 0.001. The minimum appeared to be more stable towards changes of h3, α2 and α3. Using these values, the experimental peak intensities are well reproduced and most of the simulated intensities are within the experimental uncertainties.
| E i /meV | h 1/Å | h 2/Å | h 3/Å | α 2/rad | α 3/rad | σ s |
|---|---|---|---|---|---|---|
| 10.58 | 0.197 | 0.070 | 0.031 | 1.678 | 2.692 | 0.219 |
| 11.17 | 0.190 | 0.073 | 0.031 | 1.557 | 3.246 | 0.239 |
| 11.71 | 0.171 | 0.087 | 0.014 | 1.855 | 3.737 | 0.406 |
| 13.22 | 0.262 | 0.063 | 0.041 | 2.547 | 2.745 | 1.246 |
In addition to the diffraction peaks handed over to the optimisation routine, Fig. 5 shows a comparison where all peaks have been calculated with the respective corrugation functions obtained above, illustrating that the scattering distributions are also well reproduced in their entirety. One should note that the resolution of the experimental scans decreased with increasing incident energy, which can also be seen by the overall increasing size of the error bars in Fig. 4 and 5. In comparison, the arithmetic mean,  of the experimental uncertainties ranged from 0.15–3.5 thus explaining the overall increase of the deviation between simulation and experiment in terms of σs(7).
of the experimental uncertainties ranged from 0.15–3.5 thus explaining the overall increase of the deviation between simulation and experiment in terms of σs(7).
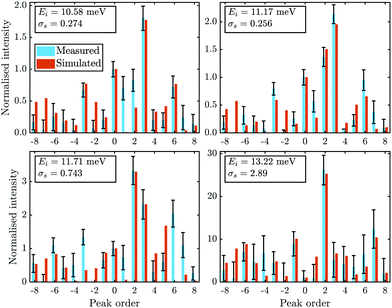 | ||
Fig. 5 Comparison of all measured intensities (blue bars, i.e. including also the ones which where not handed over to the optimisation routines due to their uncertainties) with the simulated peak intensities (orange bars) along  . The error bars for the measurements were calculated with eqn (9). The error bars for the peaks of orders −7 and 4, of the scan with Ei = 11.17 meV were omitted as they showed relative errors . The error bars for the measurements were calculated with eqn (9). The error bars for the peaks of orders −7 and 4, of the scan with Ei = 11.17 meV were omitted as they showed relative errors  of 9.5 × 106 and 9.2 × 102 respectively. of 9.5 × 106 and 9.2 × 102 respectively. | ||
The corresponding corrugation profiles as a result of the optimal set of hj and αj are displayed in Fig. 6. Visually, the corrugation functions show little deviation from one another, with the exception of the one found for the highest incident energy (purple line in Fig. 6). The latter may be due to a closer approach of the incident He atoms to the ion cores, with increasing beam energy. The overall shape of the surface profile is also well reproduced. Not only can the overall asymmetry be seen clearly but also the aforementioned protrusion is visible in all of the ξ(y), due to the value of h2 in combination with α2, which places it on the same lateral position for all four functions. The corresponding peak-to-peak corrugation height ξpp for the three lower incident energies is ξpp = 0.46 Å while for Ei = 13.22 meV the value increases to ξpp = 0.6 Å.
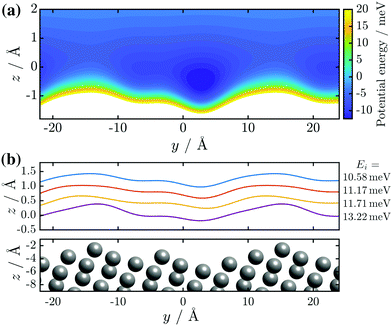 | ||
| Fig. 6 (a) Contour plot of the interaction potential, i.e. the potential energy for the best-fit corrugation function as obtained for the lowest beam energy Ei = 10.58 meV. (b) Results of the best-fit corrugation profiles ξ(y) as calculated with eqn (3) for four diffraction scans with various incident energies Ei. A consecutive offset of 0.4 Å along z was added to each profile to make them easily distinguishable, and thus the z-position of the corrugation functions is not a representation of the atoms' closest approach. | ||
Analysis on a Bi(111) surface showed peak-to-peak values of 0.21 Å,46 in good agreement when considering that the (114) surface has a much higher corrugation in terms of the ion cores. However, the electronic corrugation profiles as “seen” in HAS experiments on the Bi(114) show clearly a Smoluchowski smoothing, which is also reflected by the topography from scanning tunnelling microscopy (STM) by Wells et al.20 which shows a corrugation of ≈3.3 Å. The latter is also supported by comparison with the analysis of vicinal copper surfaces, as reported by Miret-Artes et al. which resulted in peak-to-peak values of ≈0.5 Å.32
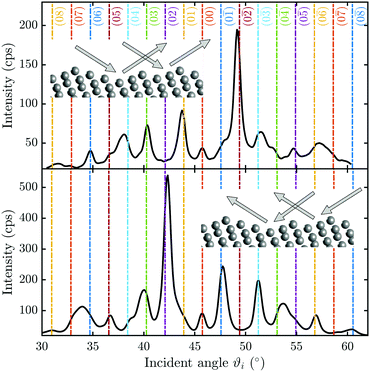
3.2 Verifying the scattering direction
From the experimental data alone, the orientation of the in- and outgoing beam with respect to the stepped surface structure, is unknown. As shown by the insets in Fig. 7, upon scattering normal to the steps, the impinging helium atom “sees” a different electronic corrugation, depending on the azimuthal rotation of the crystal. To clarify this issue, quantum-mechanical scattering calculations can be utilised to determine the orientation of the steps with respect to the incoming beam and thus the azimuthal rotation of the crystal.Fig. 7 shows two ϑ-scans with the sample at room temperature, where the azimuthal orientation of the crystal is rotated by 180° with respect to each other. From Fig. 7 it becomes evident that the peak height distribution is mirrored around the specular peak (ϑi = 45.75°), as expected. However, due to the Debye–Waller attenuation the intensities at TS = 300 K are much smaller than for the cooled scans used above, which resulted in difficulties when fitting the pseudo-Voigt profiles to the diffraction peaks. Therefore, the simulations were carried out for a cooled scan with Ei = 10.58 meV, where the mirroring of the angular intensity distribution around the specular peak was done artificially, by simply changing the sign of the interacting G-vectors from here on called quasi-mirrored scan.
It was found that shifting α2 by 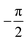 shifts the protrusion and asymmetry in ξ(y), resulting in a mirrored unit cell along y in Fig. 1(b), i.e. an azimuthal rotation by 180°. Therefore, the Nelder Mead routine (see section Appendix B) was run for this quasi-mirrored scan where the initial values of hj and αj were set to the optimal values found for that particular scan, but with α2 shifted by
shifts the protrusion and asymmetry in ξ(y), resulting in a mirrored unit cell along y in Fig. 1(b), i.e. an azimuthal rotation by 180°. Therefore, the Nelder Mead routine (see section Appendix B) was run for this quasi-mirrored scan where the initial values of hj and αj were set to the optimal values found for that particular scan, but with α2 shifted by 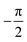 .
.
The results from the scattering calculations are plotted in Fig. 8, illustrating that the peak intensity distribution of the quasi-mirrored scan is reproduced rather well with a negative α2. The corresponding parameters of the corrugation function are given in Table 2.
| h 1/Å | h 2/Å | h 3/Å | α 2/rad | α 3/rad | |
|---|---|---|---|---|---|
| Quasi-mirrored | 0.221 | 0.093 | 0.027 | -2.044 | 2.416 |
To rule out a coincidental match, the un-mirrored scan was simulated again with the now negative value of α2, which clearly cannot reproduce the peak distribution of the former diffraction scan. It suggests that the method is in fact capable of determining the azimuthal rotation of the crystal, i.e. the orientation of the steps with respect to the incoming beam. The classical blazed optical reflection grating shows in fact the same property by shifting the maximum intensity from the positive nth order to the negative nth order upon changing the step inclination to its mirror image.36
For a firm validation scans with the mirrored sample, with the same or at least similar conditions to those in the previous section, should be analysed and compared. Another interesting qualitative comparison with an optical reflection grating arises when the step inclination is estimated which would be necessary to arrive at a maximum diffraction order for the second and third orders for wavelengths between 1.15 to 1.25 Å. The corresponding angle with respect to the grating plane should be around 4°, not far from the averaged angle obtained for the lattice corrugation of 3.3 Å and the step length of 28.4 Å.
4 Conclusion and outlook
Vicinal surfaces provide an attractive path to study systems of reduced dimensionality and here we have reported a study of the quasi one-dimensional topological metal Bi(114), based on atom-surface scattering experiments. In general, diffraction scans of “asymmetrically” stepped surfaces in combination with the large unit cell, make both a peak separation experimentally difficult and the analysis challenging. We have provided a foundation for more accurate representations of atom-surface interaction potentials and the surface electronic corrugation of such vicinal surfaces.As outlined, the surface electronic corrugation of Bi(114) upon scattering normal to the steps, can be determined together with quantum mechanical scattering calculations. By varying the shape of the corrugation function, elastic scattering measurements performed on a Bi(114) surface are reproduced to a good degree of accuracy. The resulting corrugation profiles, determined as the best fit from minimisation routines, show that the atomic asymmetry along the 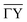 -direction of the sample is also represented in the surface electronic corrugation. The latter is further underlined by demonstrating that it is in principle possible, to determine the orientation of the in- and outgoing beam with respect to the stepped surface structure, based on scattering calculations. The peak-to-peak amplitudes of the determined surface electronic corrugation profiles are larger than compared to the low-index Bi(111) surface, but are still much smoother compared to the position of the ion cores, as also additionally reflected in comparison with the topography from STM measurements.
-direction of the sample is also represented in the surface electronic corrugation. The latter is further underlined by demonstrating that it is in principle possible, to determine the orientation of the in- and outgoing beam with respect to the stepped surface structure, based on scattering calculations. The peak-to-peak amplitudes of the determined surface electronic corrugation profiles are larger than compared to the low-index Bi(111) surface, but are still much smoother compared to the position of the ion cores, as also additionally reflected in comparison with the topography from STM measurements.
It should also be noted, that in order to develop the full He–Bi(114) interaction potential other potential parameters of the potential, such as the well depth and its stiffness need to be considered. It cannot be said with certainty that the (114) surface exhibits the same parameters as the (111) surface of bismuth as studies have shown that they may in fact vary depending on the surface plane.54 A precise experimental determination of these parameters would therefore be of interest in order to further develop and refine the interaction potential. Finally, for a better representation of the surface one could also consider constructing a three dimensional corrugation function and calculating two dimensional coupling terms and coefficients in the form vmn. This would allow for the consideration of scattering contributions into out of plane channels and therefore for more accurate simulations of the experiments.
Author contributions
S. J. S. performed the quantum mechanical scattering calculations and carried out most of the data analysis. A. T. and A. R. performed the experimental measurements while S. J. S., A. R. and A. T. developed the physical interpretation of the data. All authors discussed the results and contributed to writing the manuscript.Data and code availability
The datasets generated and analysed during the current study are available from the TU Graz repository, with the identifier https://doi.org/10.3217/yetw4-ahr29. An implementation of the CC algorithm is available from https://repository.tugraz.at/records/cd0y0-xa478 under the GNU General Public License v3.0.Conflicts of interest
There are no conflicts to declare.Appendix
A Experimental peak areas
The peak areas were calculated by fitting a scaled pseudo-Voigt profile to the measured data, using the curve_fite function from the scipy.optmize package. The profile is a combination of a Gaussian and a Lorentzian profile which are mixed according to a mixing parameter η. The area A of such a profile is calculated via: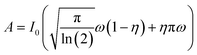 | (8) |
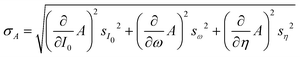 | (9) |
B Minimisation routines
The first major routine to be discussed is the Differential Evolution (DE) algorithm, based on the ideas of Storn and Price.55 This is a stochastic global optimisation routine, which, depending on the test function is well suited for minimisation of non-linear and non-differentiable continuous space functions.55 In this case, the objective function was defined as the absolute difference/error between the measured and simulated peak intensities: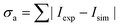 | (10) |
| xmut = xr1 + F × (xr2 − xr3) | (11) |
Following the rules for the usage of DE as formulated by Storn (see ref. 56) the absolute error was used rather than the sum of error squares as the objective function, since the latter has the potential to hide the path to the global minimum.56 Since the absolute error can however yield more local minima, larger values of F = 0.8 and CR = 0.7 were chosen to increase the search radius and the chance of “leaving” a local minimum.
Bounds for the search in hj and αj were also introduced and set to:
| h1 = [0,0.435], h2 = [0,0.43], h3 = [0,0.14] |
While it may seem arbitrary, the bounds were chosen by comparing the resulting corrugation function ξ(y) to the atomic representation as seen in Fig. 1. The bounds for α2 were set to the above values, since these cover the functional shapes of possible corrugation profiles for both  scattering directions.
scattering directions.
The upper bounds of hj were chosen as above, to ensure that even very extreme corrugation profiles would be searched, and additionally to guarantee that convergence could still be reached with a reasonable number of closed channels. Thus, for the DE routine, all open channels together with 165 closed channels were used for the calculations, with the integration bounds along the z-direction set to [−6,8] Å and with 28 steps per minimum observed scattering channel wavelength.
As it is often the case, a so called polishing routine was then used for the first set of optimal parameters found by the DE algorithm. For this the Nelder-Mead method, as part of the scipy.optmize package, was applied. The algorithm as described by Nelder and Mead (see ref. 57) uses a simplex, which is a N + 1 dimensional construct in the space of the N-dimensional objective function. Each vertex of the simplex thus constitutes a set of values for the fit parameters. It then adapts itself to the local landscape, elongating down long inclined planes, changing direction on encountering a valley at an angle, and contracting in the neighbourhood of a minimum.57 The routine was then fed the optimal values as found by the DE algorithm as initial values, to give rise to a further improvement. Furthermore, the more conventional σs, as described in the main text, was used as the objective function. The next step was to use the improved values as starting points for the next scans. As for the number of channels and integration parameters, they were chosen according to the corrugation amplitude resulting from the DE routine. They were ultimately set to 50 closed channels, z = [−8,9] Å and 35 steps per minimum wavelength for all of the scans that were analysed.
Acknowledgements
We would like to thank G. Benedek for teaching us the theoretical foundations of atom-surface scattering and the initiation as well as continuation of various collaborative efforts over the last years. This research was funded by the Austrian Science Fund (FWF) [P29641-N36 & P34704] and NAWI Graz. For the purpose of open access, the author has applied a CC BY public copyright licence to any Author Accepted Manuscript version arising from this submission. We would like to thank M. Pusterhofer for helpful discussions and Ph. Hofmann, M. Bremholm and M. Bianchi for providing us with a Bi(114) sample as well as for many helpful discussions.References
- P. Hofmann, M. M. Ugeda, A. Tamtögl, A. Ruckhofer, W. E. Ernst, G. Benedek, A. J. Martínez-Galera, A. Stróżecka, J. M. Gómez-Rodríguez, E. Rienks, M. F. Jensen, J. I. Pascual and J. W. Wells, Phys. Rev. B, 2019, 99, 035438 CrossRef CAS.
- H. Zheng, M. Gruyters, E. Pehlke and R. Berndt, Phys. Rev. Lett., 2013, 111, 086101 CrossRef PubMed.
- P. Hofmann, Prog. Surf. Sci., 2006, 81, 191–245 CrossRef CAS.
- F. Schindler, Z. Wang, M. G. Vergniory, A. M. Cook, A. Murani, S. Sengupta, A. Y. Kasumov, R. Deblock, S. Jeon, I. Drozdov, H. Bouchiat, S. Guéron, A. Yazdani, B. A. Bernevig and T. Neupert, Nat. Phys., 2018, 14, 918–924 Search PubMed.
- H. Hirayama, Adv. Phys. X, 2021, 6, 1845975 Search PubMed.
- A. Tamtögl, P. Kraus, M. Mayrhofer-Reinhartshuber, G. Benedek, M. Bernasconi, D. Dragoni, D. Campi and W. E. Ernst, npj Quantum Mater., 2019, 4, 28 Search PubMed.
- Y. L. Chen, J. G. Analytis, J.-H. Chu, Z. K. Liu, S.-K. Mo, X. L. Qi, H. J. Zhang, D. H. Lu, X. Dai, Z. Fang, S. C. Zhang, I. R. Fisher, Z. Hussain and Z.-X. Shen, Science, 2009, 325, 178–181 CrossRef CAS PubMed.
- M. Z. Hasan and C. L. Kane, Rev. Mod. Phys., 2010, 82, 3045–3067 CrossRef CAS.
- J. E. Moore, Nature, 2010, 464, 194–198 CrossRef CAS PubMed.
- X.-L. Qi and S.-C. Zhang, Rev. Mod. Phys., 2011, 83, 1057–1110 CrossRef CAS.
- A. Bansil, H. Lin and T. Das, Rev. Mod. Phys., 2016, 88, 021004 CrossRef.
- B. Bradlyn, L. Elcoro, J. Cano, M. G. Vergniory, Z. Wang, C. Felser, M. I. Aroyo and B. A. Bernevig, Nature, 2017, 547, 298–305 CrossRef CAS PubMed.
- A. Tamtögl, M. Sacchi, N. Avidor, I. Calvo-Almazán, P. S. M. Townsend, M. Bremholm, P. Hofmann, J. Ellis and W. Allison, Nat. Commun., 2020, 11, 278 CrossRef PubMed.
- G. Benedek, S. Miret-Artés, J. R. Manson, A. Ruckhofer, W. E. Ernst and A. Tamtögl, J. Phys. Chem. Lett., 2020, 11(5), 1927–1933 CrossRef CAS PubMed.
- C. Tegenkamp, J. Phys.: Condens. Matter, 2008, 21, 013002 CrossRef PubMed.
- L. A. Miccio, M. Setvin, M. Müller, M. Abadía, I. Piquero, J. Lobo-Checa, F. Schiller, C. Rogero, M. Schmid, D. Sánchez-Portal, U. Diebold and J. E. Ortega, Nano Lett., 2016, 16, 2017–2022 CrossRef CAS PubMed.
- R. van Lent, S. V. Auras, K. Cao, A. J. Walsh, M. A. Gleeson and L. B. F. Juurlink, Science, 2019, 363, 155–157 CrossRef CAS PubMed.
- S. V. Auras, R. van Lent, D. Bashlakov, J. M. Piñeiros Bastidas, T. Roorda, R. Spierenburg and L. B. F. Juurlink, Angew. Chem., Int. Ed., 2020, 59, 20973–20979 CrossRef CAS PubMed.
- C. Lin, G. Corem, O. Godsi, G. Alexandrowicz, G. R. Darling and A. Hodgson, J. Am. Chem. Soc., 2018, 140, 15804–15811 CrossRef CAS PubMed.
- J. W. Wells, J. H. Dil, F. Meier, J. Lobo-Checa, V. N. Petrov, J. Osterwalder, M. M. Ugeda, I. Fernandez-Torrente, J. I. Pascual, E. D. L. Rienks, M. F. Jensen and P. Hofmann, Phys. Rev. Lett., 2009, 102, 096802 CrossRef CAS PubMed.
- A. Tamtögl, A. Ruckhofer, D. Campi, W. E. Ernst and W. Allison, Phys. Chem. Chem. Phys., 2021, 23, 7637–7652 RSC.
- A. Ruckhofer, A. Tamtögl, M. Pusterhofer, M. Bremholm and W. E. Ernst, J. Phys. Chem. C, 2019, 123, 17829–17841 CrossRef CAS PubMed.
- A. Tamtögl, M. Pusterhofer, M. Bremholm, E. M. Hedegaard, B. B. Iversen, P. Hofmann, J. Ellis, W. Allison, S. Miret-Artés and W. E. Ernst, Surf. Sci., 2018, 678, 25–31 CrossRef.
- L. W. Bruch, R. D. Diehl and J. A. Venables, Rev. Mod. Phys., 2007, 79, 1381–1454 CrossRef CAS.
- O. Bünermann, H. Jiang, Y. Dorenkamp, A. Kandratsenka, S. M. Janke, D. J. Auerbach and A. M. Wodtke, Science, 2015, 350, 1346 CrossRef PubMed.
- H. Jiang, M. Kammler, F. Ding, Y. Dorenkamp, F. R. Manby, A. M. Wodtke, T. F. Miller, A. Kandratsenka and O. Bünermann, Science, 2019, 364, 379–382 CrossRef CAS PubMed.
- G.-J. Kroes and C. Díaz, Chem. Soc. Rev., 2016, 45, 3658–3700 RSC.
- G. B. Park, B. C. Krüger, D. Borodin, T. N. Kitsopoulos and A. M. Wodtke, Rep. Prog. Phys., 2019, 82, 096401 CrossRef CAS PubMed.
- Y. Alkoby, H. Chadwick, O. Godsi, H. Labiad, M. Bergin, J. T. Cantin, I. Litvin, T. Maniv and G. Alexandrowicz, Nat. Commun., 2020, 11, 3110 CrossRef CAS PubMed.
- A. Tamtögl, M. Mayrhofer-Reinhartshuber, N. Balak, W. E. Ernst and K. H. Rieder, J. Phys.: Condens. Matter, 2010, 22, 304019 CrossRef PubMed.
- D. Leuenberger, H. Yanagisawa, S. Roth, J. H. Dil, J. W. Wells, P. Hofmann, J. Osterwalder and M. Hengsberger, Phys. Rev. Lett., 2013, 110, 136806 CrossRef CAS PubMed.
- S. Miret-Artés, J. P. Toennies and G. Witte, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 5881–5892 CrossRef PubMed.
- G. Bracco, L. Vattuone, M. Smerieri, G. Carraro, L. Savio, G. Paolini, G. Benedek, P. M. Echenique and M. Rocca, J. Phys. Chem. Lett., 2021, 12, 9894–9898 CrossRef CAS PubMed.
- M. Mayrhofer-Reinhartshuber, A. Tamtögl, P. Kraus, K. H. Rieder and W. E. Ernst, J. Phys.: Condens. Matter, 2012, 24, 104008 CrossRef CAS PubMed.
- G. Benedek, J. R. Manson, S. Miret-Artés, A. Ruckhofer, W. E. Ernst, A. Tamtögl and J. P. Toennies, Condens. Matter, 2020, 5(4), 79, DOI:10.3390/condmat5040079.
- W. Demtröder, Experimentalphysik 2: Elektrizität und Optik, Springer-Verlag: Berlin, Heidelberg, 2013 Search PubMed.
- M. Debiossac, P. Pan and P. Roncin, Phys. Chem. Chem. Phys., 2021, 23, 7615–7636 RSC.
- K. J. Nihill, J. D. Graham and S. J. Sibener, Phys. Rev. Lett., 2017, 119, 176001 CrossRef PubMed.
- Z. B. Suk, Z. Weiqing and S. Wieland, Sci. Adv., 2016, 2, e1500901 CrossRef PubMed.
- G. Rojas-Lorenzo, J. Rubayo-Soneira, S. Miret-Artés and E. Pollak, Phys. Rev. A, 2018, 98, 063604 CrossRef CAS.
- D. Farías and K.-H. Rieder, Rep. Prog. Phys., 1998, 61, 1575 CrossRef.
- A. Sanz and S. Miret-Artés, Phys. Rep., 2007, 451, 37–154 CrossRef.
- P. Kraus, A. Tamtögl, M. Mayrhofer-Reinhartshuber, G. Benedek and W. E. Ernst, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87, 245433 CrossRef.
- M. Mayrhofer-Reinhartshuber, P. Kraus, A. Tamtögl, S. Miret-Artés and W. E. Ernst, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 88, 205425 CrossRef.
- P. Kraus, M. Mayrhofer-Reinhartshuber, C. Gösweiner, F. Apolloner, S. Miret-Artés and W. E. Ernst, Surf. Sci., 2014, 630, 208–215 CrossRef CAS.
- P. Kraus, A. Tamtögl, M. Mayrhofer-Reinhartshuber, F. Apolloner, C. Gösweiner, S. Miret-Artés and W. E. Ernst, J. Phys. Chem. C, 2015, 119, 17235–17242 CrossRef CAS PubMed.
- M. Abramowitz and I. A. Stegun, Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables, 1972 Search PubMed.
- A. Tamtögl, P. Kraus, N. Avidor, M. Bremholm, E. M. J. Hedegaard, B. B. Iversen, M. Bianchi, P. Hofmann, J. Ellis, W. Allison, G. Benedek and W. E. Ernst, Phys. Rev. B, 2017, 95, 195401 CrossRef.
- A. Tamtögl, E. Bahn, J. Zhu, P. Fouquet, J. Ellis and W. Allison, J. Phys. Chem. C, 2015, 119, 25983–25990 CrossRef PubMed.
- G. Boato, P. Cantini, U. Garibaldi, A. C. Levi, L. Mattera, R. Spadacini and G. E. Tommei, J. Phys. C: Solid State Phys., 1973, 6, L394–L398 CrossRef CAS.
- S. Miret-Artés and E. Pollak, Surf. Sci. Rep., 2012, 67, 161–200 CrossRef.
- G. Anemone, M. Garnica, M. Zappia, P. C. Aguilar, A. A. Taleb, C.-N. Kuo, C. S. Lue, A. Politano, G. Benedek, A. L. V. de Parga, R. Miranda and D. Farías, 2D Mater., 2020, 7, 025007 CrossRef CAS.
- J. F. Annett, Phys. Rev. B: Condens. Matter Mater. Phys., 1987, 35, 7826–7834 CrossRef CAS PubMed.
- D. Gorse, B. Salanon, F. Fabre, A. Kara, J. Perreau, G. Armand and J. Lapujoulade, Surf. Sci., 1984, 147, 611–646 CrossRef CAS.
- R. Storn and K. Price, J. Global Optimization, 1997, 11, 341–359 CrossRef.
- R. Storn, Proceedings of North American Fuzzy Information Processing, 1996 Search PubMed.
- J. Nelder and R. Mead, Comput. J., 1965, 7, 308–313 CrossRef.
| This journal is © the Owner Societies 2022 |