Monolayer CuBr-based gas sensor to detect habitat and industry-relevant molecules with high sensitivity and selectivity: a first-principles study

Abstract

The adsorption characteristics of different environmental gas molecules such as HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂ on the surface of a CuBr monolayer have been studied using DFT+U calculations with Grimme scheme DFT-D2 for accurate description of the long-range interactions (van der Waals). Our findings indicate that the CuBr monolayer (ML) exhibits high sensitivity to CO, SO₂, H₂S, NH₃, NO and NO₂, as evidenced by their strong adsorption energies and significant charge transfer. In contrast, HF and CO₂ molecules show weak adsorption on the CuBr ML, due to their low adsorption energies and minimal charge transfer. High diffusion energy barriers for gas molecules (CO, CO₂, NH₃ and NO₂) indicate that they are less mobile and tend to remain stable at their adsorption sites. Conversely, low diffusion energy barriers (HF, SO₂, H₂S and NO) suggest that a lesser amount of energy needs to be expended and gases can move easily across the surface of the substrate. The band structure and partial density of states calculations reveal that the electronic properties of the CuBr ML are altered due to the contributions of the orbitals of the gas molecules (C-p and O-p of CO, F-p of HF, O-p of CO₂, S-p of H₂S, N-p of NH₃, S-p and O-p of SO₂, N-p and O-p of NO and NO₂) and CuBr ML (Cu-p, Cu-d, Br-p). The charge density difference and Bader charge analysis indicate that the gas molecules (CO, HF, SO₂, CO₂, NO and NO₂) either act as charge acceptors or donors (H₂S and NH₃). The work function variations of the CuBr ML before and after adsorption and significant changes in the conductivity verify the high sensitivity of CO, SO₂, H₂S, NH₃, NO and NO₂ with the CuBr ML. The band gap variations (before and after adsorption) are small for HF, CO, CO₂, H₂S and NH₃ whereas large variations in band gap for SO₂, NO and NO₂ reveal that the CuBr ML is quite selective to these three gases. The recovery time for gas molecules desorption from CuBr ML is reduced to a reasonable recovery time by increasing the temperature from ambient to 500 K with UV exposure. Thus our theoretical results indicate that the CuBr ML is a promising candidate as a gas sensor for sensing applications of CO, SO₂, H₂S, NH₃ NO and NO₂ with high sensitivity and selectivity.

---

1. Introduction

Two-dimensional (2D) materials have garnered a great deal of attention because of their fascinating electrical, chemical and physical characteristics, their incredible thinness, availability of active sites, large surface-to-volume ratio, suitable carrier density and other appealing quantum properties.^1–3 2D nano devices have applications in many fields.^4,5 2D materials are highly promising for use as high-efficiency nanosensors as the gas molecule’s adsorption on the surface of a 2D nanomaterial results in a significant change in the electrical conductivity with little change in the carrier concentration.^6,7 Emission of hazardous and toxic gases have raised severe concerns about the quality and quantity regulation of these harmful gases in both indoor and outdoor areas. Exposure to these harmful gases has negative impacts on the ecosystem and human health.^8,9 Thus, a significant amount of work has been done for the precise identification and detection of harmful gases in the environment.¹⁰ Gas sensors are devices that use materials that sense gases in the surrounding air to determine the composition and concentration of gases. High sensitivity, high selectivity, performance stability, fast response, low working temperature and low power consumption are the fundamental standards and performance metrics for a gas sensor.¹¹ Unfortunately, a few intrinsic drawbacks of common 2D materials, like graphene’s zero band gap,¹² MoS₂’s low carrier mobility and α-p’s low chemical stability¹³ severely restrict their widespread application. This has spurred ongoing research into finding additional 2D materials with characteristics that could result in particular enhanced performance. The graphene family,¹⁴ 2D metal oxides,¹⁵ transition metal dichalcogenides (TMDCs)¹⁶ such as WS₂,¹⁷ WSe₂,¹⁸ MoS₂,¹⁹ MXenes,²⁰ single element materials, such as black phosphorous,²¹ arsenene,²² antimonene²³ and so on, are examples of emerging 2D materials used in gas sensing.

2D metal halide monolayers can be made by simply mechanically exfoliating 3D bulk phases. Among the different metal halides, 2D semiconductor cuprous bromide (CuBr) possesses a variety of applications in various fields. It is a very promising material for usage in UV photodetectors, photocatalysis, light-emitting devices and quantum cutting.^24,25 It belongs to the group of tetrahedrally coordinated binary compounds. These compounds are of great interest to researchers and those who study the fundamentals of semiconductor physics. Several groups have explored the fundamental structures, electrical characteristics and stability for copper(I/II) bromide surfaces using DFT.^25,26 Single-layer CuBr has a cleavage energy value of 0.291 J m⁻² (ref. 26) which is less than graphene’s (0.37 J m⁻²).²⁷ This suggests that the CuBr monolayer can be easily manufactured by mechanical exfoliation. Calculations of the phonon spectrum are used to assess the dynamic stability of the CuBr ML.²⁶ The absence of an imaginary mode in the CuBr monolayer implies that it is dynamically stable and could potentially exist as an independent 2D crystal and it is also thermally and mechanically stable with a formation energy of −0.27 eV.²⁶ The electron mobility (∼1153.54 cm² V⁻¹ s⁻¹) of the CuBr ML²⁶ is similar to phosphorene (∼1000 cm² V⁻¹ s⁻¹)²⁸ and greater than other 2D semiconductors, including MoS₂ (∼200 cm² V⁻¹ s⁻¹)²⁹ and BN (∼487 cm² V⁻¹ s⁻¹).³⁰ The CuBr ML possesses a band gap of 3.198 eV and Γ(0, 0, 0) is the location of the valence band maximum and conduction band minimum.²⁶

Pervaiz et al.³¹ studied in detail the adsorption of CO and HF gas molecules on a CuCl ML using the DFT+U method. Different parameters like adsorption energy, band gap energy, nudged elastic band, charge density difference, work function, conductivity and recovery time were calculated to study the sensitivity and selectivity of the CuCl ML for these gases. Likewise previous research studies show adsorption of toxic gas molecules on various materials including InN, phosphorene, Janus Te₂Se, graphene (doped), ZnS, Ti-doped hBN, TMDCs, MoSi₂N₄, graphene, Ti₂CO₂, arsenene and defective tellurine.^32–43

In this research work, founded on computations from first principles, we inclusively investigated a wide range of gas molecules on the CuBr ML, including combustible (CO, H₂S, NH₃), non-combustible (HF), inert gases (CO₂, SO₂) and oxidizers (NO, NO₂) which have not been presented before. The properties of the adsorption system (gas–CuBr), such as the adsorption energy (E_ads), distance (d, between gas molecule and ML), charge shifted (ΔQ), total and partial density of states (DOS/PDOS), band gap energy, work function, conductivity and recovery time were calculated to investigate the sensitivity and selectivity of a CuBr sensor with these toxic gas molecules. The nudged elastic band (NEB) model and the charge density difference (CDD) were also used to investigate the potential adsorption mechanism and interaction. The findings show that the electronic and magnetic characteristics of the CuBr ML are influenced by the adsorption of various molecules, which makes it suitable to use as a gas sensing material. As far as we are aware, there hasn’t been any research on how various molecules adsorb on the CuBr monolayer or how this affects the monolayer’s characteristics. Therefore, this paper’s goal is to fill in the knowledge gap in this area.

2. Computational details

The quantum espresso package⁴⁴ is the ab initio code utilized in our research work for structural optimization and electronic properties calculation. To relax the structures, the plane-wave basis set using generalized gradient approximation, as indicated by the Perdew–Burke–Ernzerhof functional,⁴⁵ is applied. Ultra-soft pseudo-potentials⁴⁶ were used to express the valence electrons and ionic core interaction. Furthermore, for a precise representation of van der Waals interactions between the monolayer and gas molecules, the Grimme scheme DFT-D2 is applied⁴⁷ as DFT produced accurate results in the previous studies.^48–51 The Coulomb repulsion interaction between the electrons in the Cu:3d and Br:4p orbitals is considered utilizing the DFT+U approach, which is also utilized for the correction of self-interaction error in the s, p, d, and f states.⁵² So, the PBE functional includes the Hubbard parameter (U),^53,54 obtained via the ACBN0 technique⁵⁵ chosen as U (Cu:3d) = 6.3 eV and U (Br:4p) = 6.3 eV, in accordance with Mehta et al.⁵⁶

A CuBr monolayer consisting of 18 atoms (nine Cu and nine Br atoms) is utilized, with a 3 × 3 × 1 supercell having lattice constants of a = b = 12.150 Å. A vacuum of 20 Å is set in order to separate neighboring repeated images of the supercell. Each atom is subjected to a force of less than 0.005 eV Å⁻¹ for structural relaxation. A cutoff of 650 eV in kinetic energy for the wavefunction and 10⁻⁸ eV energy convergence is employed. A 4 × 4 × 1 k-mesh is taken to depict the first Brillouin zone using the Monkhorst–Pack technique.⁵³ For the precision of electronic computations, Brillouin zone sampling employs a denser k-mesh of 12 × 12 × 1.

Bader charge analysis is applied to look into the charge transformation between the CuBr ML and gas molecule.⁵⁴ The following relationship is utilized to assess the gas molecule’s adsorption phenomenon strength on the CuBr ML:³¹

E_ads = E_total − (E_CuBr + E_{gas molecule}) (1)

where E_ads is adsorption energy, E_total is the combined system energy (gas–CuBr), E_CuBr is the pristine CuBr ML’s energy and E_{gas molecule} is the gaseous molecule energy.

The climbing image nudged elastic band approach is employed for tracing the lowest energy pathway (between the beginning and final coordinates) during adsorption of molecules. The transition state search is done for this reason. One can find the DEB as follows:³¹

E_DEB = E_TS − E_IS (2)

where E_IS denotes the initial state energy and E_TS, the transition state energy (final transition state in the case of more than one transition).

The charge density difference (CDD) for the monolayer–molecule systems is determined using the following formula:³²

Δρ = ρ_total(r) − ρ_CuBr(r) − ρ_gas(r) (3)

where the charge distribution of the gas-adsorbed CuBr ML is represented by ρ_total(r). In the absence of gas adsorption, the charge distribution of the free CuBr ML is ρ_CuBr(r), and the free gas molecule’s distribution of charges is represented by ρ_gas(r). The gas molecule and the free CuBr have the same coordinates as when they are adsorbed.

The work function is the minimum energy required for the ejection of electrons from the Fermi level to infinity for the adsorbed gas, which can be computed as:³¹

ϕ = V(ϕ) − E_Fermi (4)

where ϕ stands for work function, V(ϕ) for electrostatic potential and E_Fermi for the Fermi energy of the CuBr ML.

The equation below relates to the conductivity of the CuBr ML, which varies as a result of the gas molecules’ adsorption phenomena:⁵⁵

σ = A e^−E_g/k_BT (5)

In this case, σ stands for electrical conductivity, A is the proportionality constant, T is absolute temperature, E_g denotes the band gap energy and k_B denotes the Boltzmann constant. The sensitivity can be defined by the change in conductivity, which can be written as:⁵⁵

In order to evaluate the reusability of a gas sensor, its recovery time is calculated using the following equation:³¹

In this case E_ads stands for adsorption energy, v₀ (= 10¹² s⁻¹)⁵⁵ for the frequency of the molecular attempt, and k_B (= 8.617 × 10⁻⁵ eV K⁻¹) is the Boltzmann constant.

3. Results and discussion

The dynamic stability of the CuBr ML has been evaluated by examining the phonon dispersion plot, as illustrated in Fig. 1. Nearly all of the modes in the phonon dispersion plot of CuBr show positive frequency in the range of the Γ–Γ high symmetry k-point, which is in accordance with the previous theoretical research on the CuBr ML,²⁶ so it is implied that the CuBr monolayer is dynamically stable and may exist as an independent 2D crystal as it does not exhibit an imaginary mode.

Fig. 1 Phonon dispersion spectrum of the CuBr monolayer.

The different gas molecules are initially positioned above the CuBr ML with varying orientations for determining the most energetically stable configurations of adsorption with the lowest energy. The gaseous molecules HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂ were initially positioned at a distance of 3.0 Å above the adsorption sites and the system was completely relaxed after adsorption. For the gas molecules’ adsorption on the CuBr ML, we first took into consideration a number of typical adsorption sites as shown in Fig. 2 namely, center (above hexagon center), Cu (above Cu atom), Br (above Br atom), and bridge (above Cu–Br link). Additionally on the CuBr surface, many typical orientations of gas molecules are also taken into consideration. As an example of SO₂ molecule adsorption, the SO₂ molecule is first positioned vertically (CuBr↔O–SO, and CuBr↔O₂–S) or in parallel (CuBr↔O₂–S, and CuBr↔S–O₂) on the surface of the CuBr ML for each and every adsorption site under study. So all the orientations of the SO₂ molecule are taken into account. It is clear that distinct gas molecules prefer different adsorption sites and configurations within the same substrate. Furthermore, it is evident that several gas molecules, including CO, CO₂, SO₂, NH₃, H₂, NO and NO₂, caused buckling effects and structural deformation, which are produced in the monolayer due to adsorption of these gas molecules compared to the pristine CuBr monolayer as shown in Fig. 2. It is also observed that after adsorption, variation in the bond length and bond angle of the gas molecules has occurred as Fig. 3 shows the configurations of the gas molecules after adsorption.

Fig. 2 Top and side views (most favorable configurations with different buckling heights for adsorption on the CuBr ML) of (a) CO, (b) H₂S, (c) NH₃, (d) HF, (e) CO₂, (f) SO₂, (g) NO and (h) NO₂. Green, yellow, red, black, gray and orange balls represent H, F, O, C, S, and N atoms respectively.

Fig. 3 Configuration of adsorbed gas molecules of (a) CO, (b) H₂S, (c) NH₃, (d) HF, (e) CO₂, (f) SO₂, (g) NO and (h) NO₂ on the CuBr ML. Green, yellow, red, black, gray and orange balls represent H, F, O, C, S, and N atoms respectively.

Table 1 lists the adsorption energy values (E_ads) on different sites (center, Cu, Br and bridge), D, closest distance (between gas molecule and the CuBr ML), and Q, total charge transfer between the CuBr ML and gas molecules. For most of the adsorption systems of CuBr with gas molecules (HF, CO, CO₂, SO₂, NO, NO₂), it is concluded that the adsorption energy absolute values rise as the distance of adsorbed molecule decreases. Eqn (1) is used for the calculation of adsorption energy. The charge transfer between the gas molecules and the CuBr ML is provided by Bader charge analysis.⁵⁴

Table 1 Adsorption sites, adsorption energy (E_ads), adsorption distance (D), charge transfer (Q) and molecular nature (donor/acceptor) on the CuBr ML for CO, H₂S, NH₃, HF, SO₂, CO₂ NO and NO₂

Model	Site	Eads (eV)	D (Å)	Q (e^−)	Molecule on CuBr
CO	Bridge	−1.284	1.798	−0.097	Donor
	Cu	−1.285	1.796	−0.111
	Br	−0.136	3.519	−0.014
	Center	−0.262	2.111	−0.063
H2S	Bridge	−1.037	3.326	0.098	Acceptor
	Cu	−1.124	3.252	0.111
	Br	−1.172	2.203	0.027
	Center	−0.859	2.747	0.047
NH3	Bridge	−1.287	1.850	0.117	Acceptor
	Cu	−1.280	1.901	0.115
	Br	−0.363	3.343	0.012
	Center	−0.332	2.578	0.019
HF	Bridge	−0.222	2.183	−0.032	Donor
	Cu	−0.075	2.266	−0.060
	Br	−0.188	2.205	−0.059
	Center	−0.229	1.648	−0.056
SO2	Bridge	−0.591	2.140	−0.188	Donor
	Cu	−0.632	2.071	−0.158
	Br	−0.297	3.335	−0.110
	Center	−0.316	2.751	−0.158
CO2	Bridge	−0.248	3.108	−0.040	Donor
	Cu	−0.277	3.294	−0.034
	Br	−0.253	3.106	−0.042
	Center	−0.224	2.762	−0.014
NO	Bridge	−0.739	1.805	−0.261	Donor
	Cu	−0.791	1.775	−0.244
	Br	−0.187	3.231	−0.077
	Center	−0.852	0.952	−0.528
NO2	Bridge	−0.965	1.930	−0.521	Donor
	Cu	−0.850	1.942	−0.518
	Br	−1.429	1.910	−0.519
	Center	−1.754	1.233	−0.812

---

In the case of combustible gases, CO, H₂S and NH₃, all these gases show strong adsorption on the CuBr monolayer having E_ads absolute values of 1.285, 1.172 and 1.287 eV with charge transfer of −0.111|e|, 0.027|e| and 0.117|e| respectively. These combustible gas molecules have different optimal sites on the CuBr ML as shown in Table 1. CO and NH₃ molecules have small adsorption distances of 1.796 and 1.850 Å respectively, whereas the adsorption distance for H₂S is 2.203 Å. All the three combustible gas molecules show physisorption on CuBr on the basis of E_ads, adsorption distance and small charge transfer values. These results demonstrate that the CuBr ML exhibits significant potential in the sensing of these combustible gases. Consequently it is evaluated that the CuBr ML shows very good adsorption for these combustible gases when comparing with E_ads values on other 2D materials which have been previously studied, like InN (for CO, H₂S and NH₃), phosphorene (for CO and NH₃), Janus Te₂Se (for CO, H₂S and NH₃), graphene (for CO and NH₃), ZnS monolayer (for CO) as well as pristine hBN (for CO, H₂S) and Ti doped hBN (for CO).^32–37

For the non-combustible HF gas molecule, the center and bridge sites are the optimal configurations on the CuBr ML as the adsorption energies (−0.222 and −0.229 eV) on these sites are not much different with small Q = −0.032 and −0.056|e| respectively. The adsorption distances for HF molecules on center and bridge sites are 2.183 and 1.648 Å respectively. It is observed that there is very little interaction between the CuBr and the HF molecule. The HF molecule also shows physisorption on CuBr due to small E_ads and charge transfer values.

In the case of inert gases SO₂ and CO₂, a good interaction is observed for the SO₂ gas molecule as compared to CO₂ (weak adsorption) with E_ads value of −0.632 and −0.277 eV on Cu sites respectively. Both these inert gas molecules show different adsorption behaviour on different sites as shown in Table 1. Similarly for the SO₂ molecule more charge transfer is observed (−0.158|e|) as obvious from its greater adsorption energy compared with the small charge transfer for CO₂ (−0.014|e|) with adsorption distances of 2.071 and 2.762 Å respectively. These two inert gas molecules also show physisorption on the CuBr ML on the basis of E_ads value, adsorption distance and small charge transfer values. The CuBr ML showed a significantly good adsorption energy value for SO₂ and CO₂ molecules when comparing with E_ads values on other substrates like Janus Te₂Se-based ML (for SO₂),³⁴ hBN (for CO₂),³⁷ MoSi₂N₄ (for SO₂ and CO₂),³⁹ pristine graphene (for CO₂),⁴⁰ Ti₂CO₂ (for CO₂)⁴¹ and pristine arsenene (for SO₂).⁴²

Regarding the adsorption of oxidizer gases NO and NO₂, their nitrogen end, often known as the nitro configuration, is attached to the monolayer surface similar to the previous studies.⁴¹ This nitro configuration with the center site is the most optimal site for both the molecules. Both oxidizer molecules show strong adsorption with E_ads values of about −0.852 and −1.754 eV with small adsorption distances of 0.952 and 1.233 Å respectively. It is also observed that a huge charge of about −0.528|e| and −0.812|e| is transferred from the CuBr ML to the NO and NO₂ molecules respectively. Both the gas molecules show chemisorption on the CuBr ML on the basis of large E_ads, small adsorption distance and large charge transfer values. It is evaluated that CuBr shows good adsorption affinity for NO and NO₂ molecules as compared to other 2D monolayers like InN (for both NO and NO₂),³² Janus Te₂Se (for both NO and NO₂),³⁴ graphene (for NO and NO₂),³⁵ pristine hBN (for NO),³⁷ tellurine (for NO and NO₂)⁴³ and phosphorene (for NO₂).³³

The adsorption of all gaseous molecules on the CuBr ML is energy favourable and occurs through an exothermic process, as indicated by the negative sign of the adsorption energy. Fig. 4 gives a comparison of the adsorption energy values of the different molecules (HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂) at the most stable site (center, Cu, Br, bridge).

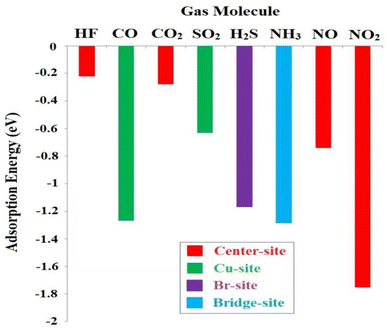Fig. 4 Comparison of adsorption energy values of different molecules on the CuBr ML at the most stable site.

Moreover a transition state search is executed to gain more understanding of the optimal configuration. The NEB calculations are used to identify the least energy path between the reactants and products. The results of different gas molecules are displayed in Fig. 5. The accuracy of the adsorption energy calculations is confirmed by the reaction path’s energy relationship, which demonstrates that the best location for adsorption is indeed the site with the lowest energy. In NEB calculations, the Initial State (IS), Transition State (TS), and Final State (FS) are key points along the minimum Energy Path, which describes the reaction or adsorption process and scenarios of how the gases move on the CuBr surface. These states are used to determine the diffusion energy barrier (DEB) for adsorption of gas molecules on the CuBr ML. The IS state is the starting configuration where the adsorbate gas molecule is positioned at an initial adsorption site before migration. In this state, the interaction between the molecule and the surface is minimal. As the system (CuBr–gas) progresses along the reaction pathway, it reaches the TS, which corresponds to the highest energy point along the minimum Energy Path and represents the reaction or diffusion barrier. At the TS, partial bond formation occur, and the gas molecule is in an unstable or metastable configuration. This state is crucial for determining the DEB, which is the energy difference between the TS and IS as given in eqn (2). Finally, the system reaches the FS, which represents the most stable configuration after the transition. At the FS, the gas molecule is fully adsorbed at a preferred site on the surface. The total energy of the system at the FS is lower than or comparable to the IS, indicating a more stable configuration. The adsorption energy at this state is minimized, confirming strong interactions between the gas molecule and the CuBr ML. The NEB method interpolates between the IS and FS through the TS using “images” to capture intermediate states and is plotted when gas molecules are migrating from one optimal site to another optimal site on the monolayer surface.^56,57 For CO and CO₂ adsorption on the CuBr monolayer, the most stable optimal adsorption site (minimum energy site) is the Cu site, shown in Table 1, which is designated as the IS. The neighboring Cu site is considered the FS, while the transition states (TS1 and TS2) occur via the Br (or near Br) site and the bridge site, as depicted in Fig. 5. Our calculations indicate that the Cu–Br–bridge–Cu pathway is the most favorable diffusion route of minimum energy for CO and CO₂ adsorption on the CuBr ML. Similarly, for H₂S adsorption, the molecule migrates from the Br site (IS), which is the optimal site to the adjacent Br site (FS) through the Cu site as the TS, encountering a diffusion barrier of 0.62 eV given in Table 2. For NH₃ adsorption, the IS is the bridge site, the transition states (TS1 and TS2) occur at the center site, and the FS returns to the bridge site. For SO₂, the IS, TS and FS states are Cu, Br and Cu respectively. Similarly, for HF, NO, and NO₂ adsorption on the CuBr monolayer, the center site is identified as the most stable adsorption site and is designated as the IS. The neighboring center site serves as the FS, with the TS occurring via the bridge site. Our calculations verify that the center–bridge–center route is the most preferred diffusion path with the least energy for these gas molecules. Therefore by accurately defining the IS, TS and FS and using interpolated images between them, NEB calculations provide an accurate estimation of the energy barrier, adsorption stability, and reaction kinetics for surface processes on the CuBr ML.

Fig. 5 Diffusion energy barrier with diffusion pathway and configurations of initial, transition and final states of (a) CO, (b) H₂S, (c) NH₃, (d) HF, (e) CO₂, (f) SO₂, (g) NO and (h) NO₂. Green, yellow, red, black, gray and orange balls represent H, F, O, C, S, and N atoms respectively.

Table 2 Band gap energy (E_g), sensitivity of the CuBr ML towards various gas molecules, work function (ϕ) and diffusion energy barrier (DEB) values of the pristine CuBr ML and with gas molecules adsorption. (↑/↓) shows spin up and spin down band gap

Model	Eg (eV)	Φ (eV)	DEB (eV)	Sensitivity
CuBr ML	3.198	4.21	—	—
CO–CuBr	3.050	4.77	1.51	16.50
H2S–CuBr	3.316	4.38	0.62	0.90
NH3–CuBr	3.276	4.98	0.91	0.78
HF–CuBr	3.191	5.13	0.014	0.14
SO2–CuBr	1.536	4.64	0.44	9.12 × 10^13
CO2–CuBr	3.193	4.39	0.81	0.10
NO–CuBr	0↑/1.92↓	5.56	0.55	7.28 × 10^26
NO2–CuBr	2.06↑/0.11↓	5.17	1.02	3.62 × 10^9

---

There are two observed transition states in the cases of CO, CO₂ and NH₃ designated as TS1 and TS2, with TS2 being used for DEB calculations. An intermediate stage between TS1 and TS2 is also observed. During the course of motion of gas molecules from the starting state to the optimal final state, an intermediate state exists, which is an unstable reaction in adsorption. Eqn (2) is used to compute the DEB by considering Fig. 5, where the DEB values for CO, H₂, NH₃, HF, CO₂, SO₂, NO and NO₂ are given in Table 2. The higher value of the energy barrier for CO, CO₂, NH₃ and NO₂ when these molecules are migrating from one optimal site to another suggests that these gases are less mobile and tend to remain stable at their adsorption sites, while the small value of DEB for HF, SO₂, H₂S and NO indicates that a lesser amount of energy needs to be expended and these gases can move easily across the surface of the CuBr ML as according to the previous study on DEB calculations.⁵⁸

To evaluate the impact of gas adsorption on the electronic properties of the CuBr ML in more detail, we examined the total DOS, PDOS and band structures as shown in Fig. 6 and 7. These calculations reveal that the electronic properties of the CuBr monolayer are altered due to the orbital contributions from the gas molecules near the Fermi level as compared to pristine CuBr ML. The band gap energies of the CuBr ML without and with the adsorption of different gaseous molecules (CO, H₂S, NH₃, SO₂, CO₂, NO, and NO₂) on the surface of the CuBr ML are given in Table 2. The band gap of the pristine CuBr ML is 3.198 eV, which is in accordance with the previous DFT studies.²⁶

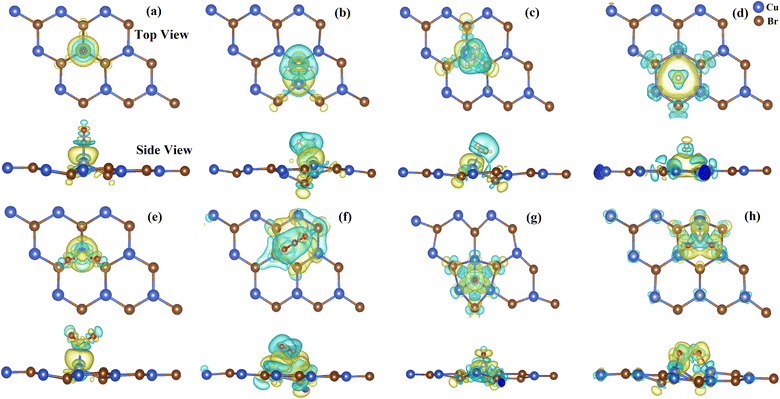
Fig. 6 The total and partial densities of states (PDOS) and band structures of (a) CO, (b) H₂S, (c) NH₃, (d) HF, (e) CO₂, (f) SO₂ adsorbed on the CuBr ML.

Fig. 7 The total and partial densities of states (PDOS) and band structures of paramagnetic (a) NO and (b) NO₂ molecules adsorbed on the CuBr ML.

The adsorption of combustible gases CO, H₂S and NH₃ does not change the semiconducting feature due to the large band gap after adsorption. In the case of CO, the band gap E_g is reduced to 3.050 eV as compared to the pristine CuBr ML. The CO molecule acts as a charge donor and after adsorption new peaks are noticed due to C-p and O-p orbitals of the CO molecule at about 1.988 eV in the conduction band (CB) near the Fermi level. The PDOS of CO adsorption shows strong hybridization of orbitals as shown in Fig. 6(a), which is due to the high value of the adsorption energy of the CO molecule. On the other hand, the H₂S and NH₃ adsorptions show small increases in the E_g values to 3.316 and 3.276 eV respectively. The H₂S and NH₃ adsorptions show strong adsorption energy values but still no significant difference is observed in the DOS of the combined CuBr after adsorption of these molecules and pristine CuBr ML close to the Fermi level, which implies the advantage and sensitivity of the CuBr ML for the adsorption of these combustible gases. A small contribution from the H₂S electronic states to the total DOS of the H₂S–CuBr system is localized in the valence band (VB), away from the Fermi level (about −2.924 eV) due to S-p orbitals as given in Fig. 6(b). The contribution of NH₃ electronic states to the total DOS of NH₃–CuBr is localized in the VB, near the Fermi level (about −1.675 eV) due to N-p orbitals as given in Fig. 6(c).

In the case of non-combustible HF gas molecule adsorption, it is observed that the energy band gap (E_g) is slightly reduced to 3.191 eV for the HF–CuBr system. The PDOS of HF adsorption plotted in Fig. 6(d), shows that CuBr after adsorbing HF keeps maintaining the semiconducting feature with a large band gap. The HF electronic levels’ contribution to the overall DOS of the whole system is concentrated in the valence bands (VB), away from the Fermi level, at a location of −4.262 eV due to F-p orbitals. Near the Fermi level, however no major difference is observed between the DOS of the intrinsic CuBr ML and the HF–CuBr system. Also no strong hybridization of orbitals is observed in the PDOS because of the smaller adsorption energy of HF gas molecules.

In the case of adsorption of inert gases CO₂ and SO₂, a very small difference is observed in the band gap of the CuBr ML after adsorption of CO₂ molecule, whereas a significant decrease in band gap (1.536 eV) is observed in the case of SO₂ but keeping the semiconducting feature after adsorption in both cases. For CO₂, the electronic levels’ contribution to the total DOS of the CO₂–CuBr system is localized in the VB, far from the Fermi level, at a location of about −4.938 eV due to O-p orbitals as shown in Fig. 6(e). Therefore close to the Fermi level, there is no notable variation between the DOS of the CO₂–CuBr and CuBr ML and no hybridization of orbitals is observed in the PDOS because of the smaller adsorption energy, indicating the physisorption phenomenon in CO₂ adsorption. The SO₂ electronic levels’ contribution to the total DOS of the combined systems (SO₂–CuBr) is localized in the CB, close to the Fermi level, at a location of about 0.740 eV due to S-p and O-p orbitals as shown in Fig. 6(f).

In contrast, the oxidizer gases NO and NO₂ are paramagnetic and their adsorption introduces spin-polarization, due to which an asymmetry is introduced between the spin up/down (↑/↓) states. In Fig. 7(a) and (b), it is observed that a significant doping impact is produced by the spin-induced magnetic moment, where NO and NO₂ gas molecules act as charge acceptors with high values of charge transfer (−0.528|e| and −0.812|e|) respectively. The magnetic moment could also be the major cause for the strong adsorption energy (−0.852 eV for NO, −1.754 eV for NO₂) and band gap reduction. For NO the band gap is reduced to 0.0 eV (spin up) and 1.92 eV (spin down) and for NO₂, the band gap is decreased to 2.06 eV (spin up) and 0.11 eV (spin down) upon adsorption of the NO₂ gas molecule on the CuBr monolayer.

Furthermore, the CDD is demonstrated to provide additional understanding of the transfer of charge between gas molecules and the CuBr ML. Eqn (3) is used to determine the CDD, and the resulting diagrams are shown in Fig. 8. The charge depletion is represented by the cyan region, and the charge gain is shown by the yellow region. For the combustible gases, the CO molecule acts as a charge acceptor. Whereas H₂S and NH₃ act as a charge donor with small charge transfer from these gas molecules to the CuBr ML showing the physisorption phenomenon in the case of these combustible gases. In the case of the non-combustible HF gas molecule adsorption, the charge is accumulated on the F of the HF molecule, indicating that the HF molecule behaves as a charge acceptor. It may be due to high electronegativity of the F atom. Here also a small charge is lost by the Br atom of the CuBr ML to the HF showing physisorption. In the case of inert gases SO₂ and CO₂, both the gas molecules behave as charge acceptors due to the high electronegativity of the oxygen atoms and charge is transferred from the CuBr ML to the molecule. In this case the charge accumulation is observed between two O atoms, and charge depletion is seen between Cu and Br atoms of the CuBr ML. For the adsorption of oxidizer gases NO and NO₂, charge accumulation is observed on the molecule side. This may be due to the electron-accepting nature of the N and O atoms of the NO and NO₂ molecules that show high values for their adsorption energy upon the CuBr ML. High values of charge transmission of −0.582|e| and −0.812|e| are observed from the CuBr ML to these molecules, indicating the chemisorption between NO/NO₂ and the CuBr ML.

Fig. 8 Charge density difference (CDD) of (a) CO, (b) H₂S, (c) NH₃, (d) HF, (e) SO₂, (f) CO₂, (g) NO and (h) NO₂ adsorbed on the CuBr monolayer. Green, yellow, red, black, gray and orange balls represent H, F, O, C, S, and N atoms respectively.

In our research work, the calculation of the work function is done by using eqn (4), to further examine the impact of gas adsorption on the CuBr ML. It indicates the least energy needed to eliminate an electron from the ML surface. Work function is commonly used to assess a material’s sensitivity when a gas sensor based on the Kelvin method is used and in this approach measurement of ϕ is done before and after gas exposure on sensing material using a Kelvin oscillator instrument.⁵⁹ Variations in ϕ during gas exposure would have an impact on the sensitivity of the sensor. The work function varies in response to variations in the charge concentration, so it is connected to conductivity. Fig. 9 shows the ϕ values of the CuBr ML without and with adsorption of the gas molecules. The work function of the pristine CuBr ML (4.21 eV) increases as a result of the adsorption of all gas molecules, suggesting that this adsorption is preventing electrons from moving to the vacuum level. Consequently, the work function can be greatly impacted by the adsorption of a specific gas molecule and the sensitivity of each molecule upon the CuBr ML depends on the percentage variations of ϕ (before and after adsorption), whose values in response to HF, CO, SO₂, NH₃, NO and NO₂ gas adsorptions are 21.8, 13.3, 10.2, 16.1, 32.0 and 22.8%. Therefore the CuBr ML is quite sensitive to all six of these gases, as evidenced by the variations in their work functions after adsorption.

Fig. 9 Calculated work function of (a) CuBr-ML, (b) CO–CuBr, (c) H₂S–CuBr, (d) NH₃–CuBr, (e) HF–CuBr, (f) CO₂–CuBr, (g) SO₂–CuBr, (h) NO–CuBr and (i) NO₂–CuBr.

The sensitivity and selectivity of a monolayer for specific gas molecules also depends on the variation of the band gap. Eqn (5) indicates that the conductivity is proportional to the exponential of band gap, which reflects that a change in the band gap width results in a change in the conductivity. The sensitivity of a monolayer for a molecule can be defined by the change in conductivity as given by eqn (6).⁵⁹ The calculated sensitivity of the CuBr ML towards different gas molecules is given in Table 2, which shows that SO₂, NO and NO₂ gas molecules have greater sensitivity due to the greater change in conductivity as compared to other gas molecules, and similarly the selectivity of these molecules depends on the percentage change in band gap whose values for HF, CO, CO₂, H₂S and NH₃ are small which indicates that the CuBr ML cannot distinguish between the sensing of these molecules. On the contrary, the variations in band gap for SO₂, NO and NO₂ are 52, 100↑/40↓ and 35.6↑/96.6↓% respectively. The (↑/↓) shows spin up and spin down band gaps. The results show that the CuBr ML is quite selective to these three gas molecules.

Application feasibility of a high performing and practically efficient gas sensor depends upon the fact that the gas adsorption mechanism must allow the gas to remain on the sensor surface long enough to be detected and then removed without damaging it. The gas molecule needs to stay adsorbed on the gas sensing material for the first instance at ambient temperature (300 K). When gas is successfully detected, the gas sensor should quickly return to its initial state. Table 3 shows the estimated recovery times with the exposure to visible light (attempt frequency v₀ = 10¹² s⁻¹)⁵⁵ at different temperatures of 300 K, 400 K, and 500 K. Also for CO, NH₃ and NO₂ gas molecules the recovery time is calculated in the presence of UV light (v₀ = 10¹⁵ s⁻¹).³⁷ We have examined the CuBr monolayer’s room temperature (300 K) gas sensing capability in order to confirm its feasibility for real-time applications. For determining the reusability of a gas sensor, its recovery time is calculated using eqn (7). The recovery period for HF, CO₂ and SO₂ is very small, therefore the CuBr ML can thus be utilized frequently for detection of these gas molecules at room temperature, since these gas molecules can be readily desorbed from the CuBr surface. The recovery time of H₂S and NO at 300 K is high, which can be further reduced by increasing the temperature. The full desorption of CO, NH₃ and NO₂ gas molecules from the CuBr ML at 500 K requires long recovery times. Eqn (7) indicates that if the adsorption energy E_ads is kept the same, then a shortened recovery time can be obtained by either a higher temperature or increasing attempt frequency (UV light exposure) or both because the UV light helps the sensor to recover quickly, therefore the recovery time of these gas molecules at a high temperature of 500 K in the presence of ultraviolet light can be reduced to an acceptable value. As a result the CuBr monolayer is predicted to be a promising material for gas sensing of HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂ gases.

Table 3 Recovery time of the CuBr monolayer for HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂ gas desorption

Material	Recovery time (τ)
	300 K	400 K	500 K	500 K
	Visible light	Visible light	Visible light	UV light
CO	3.84 × 10^9 s	1.55 × 10^4 s	8.97 s	8.97 × 10^−3 s
H2S	4.71 × 10^7 s	9.4 min	0.64 s	—
NH3	7.00 × 10^9 s	1.58 × 10^4 s	9.38 s	9.38 × 10^−3 s
HF	7.04 × 10^−9 s	7.68 × 10^−10 s	2.03 × 10^−10 s	—
SO2	3.95 × 10^−2 s	8.86 × 10^−5 s	2.35 × 10^−6 s	—
CO2	4.44 × 10^−8 s	2.98 × 10^−9 s	6.01 × 10^−10 s	—
NO	3.23 min	5.44 × 10^−2 s	3.86 × 10^−4 s	—
NO2	2.79 × 10^17 s	1.15 × 10^10 s	4.69 × 10^5 s	7.81 min

---

4. Conclusion

In summary, the results obtained through first-principles calculations demonstrate that the CuBr monolayer (ML) shows different adsorption behaviors when exposed to common and contaminating gas molecules (HF, CO, CO₂, SO₂, H₂S, NH₃, NO and NO₂). The CuBr ML reveals a strong adsorption affinity for CO, SO₂, H₂S, NH₃, NO and NO₂ molecules as compared to HF and CO₂. The band structure and PDOS reveal that the electronic properties of the CuBr ML are altered due to the orbital contributions of the gas molecules (C-p and O-p of CO, F-p of HF, O-p of CO₂, S-p of H₂S, N-p of NH₃, S-p and O-p of SO₂, N-p and O-p of NO and NO₂) and the CuBr ML (Cu-p, Cu-d, Br-p) The gas molecules CO, CO₂, NH₃ and NO₂ have large DEB values as compared to the other molecules. The CDD and Bader charge analysis indicate that the gas molecules act as charge acceptors or donors. The work function variation of the CuBr ML after adsorption and significant changes in the conductivity verify the sensitivity of these gas molecules. The percentage band gap variation for SO₂, NO and NO₂ is observed to be greater as compared to the other molecules showing that the CuBr monolayer is quite selective for these molecules. The recovery time for HF, CO₂ and SO₂ is very small at 300 K, therefore the CuBr ML can be utilized frequently for detection of these molecules at room temperature. However the recovery time for the other molecules can be significantly reduced by using high temperature with exposure to UV light. Thus our theoretical results indicate that monolayer CuBr is a promising candidate for gas sensing applications with high sensitivity and selectivity for CO, H₂S, NH₃, SO₂, NO and NO₂ gas molecules.

Data availability

The data supporting this article has been included as part of this manuscript.

Conflicts of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

The authors would like to thank the Researchers Supporting Project Number (RSP2025R511), King Saud University, Riyadh, Saudi Arabia. The author Y. Saeed would like to thank the Higher Education Commission (HEC) of Pakistan for providing a grant under NRPU-15844.

Notes and references

1. Z. Xie, F. Yang, X. Xu, R. Lin and L. Chen, Front. Chem., 2018, 6, 430.
2. K. Rasool, R. P. Pandey, P. A. Rasheed, S. Buczek, Y. Gogotsi and K. A. Mahmoud, Mater. Today, 2019, 30, 80.
3. B. Wang, Y. Gu, L. Chen, L. Ji, H. Zhu and Q. Sun, Nanotechnology, 2022, 33, 252001.
4. X. Xie and N. Zhang, Adv. Funct. Mater., 2020, 30, 2002528.
5. J. Pang, R. G. Mendes, A. Bachmatiuk, L. Zhao, H. Q. Ta, T. Gemming, H. Liu, Z. Liu and M. H. Rummeli, Chem. Soc. Rev., 2019, 48, 72.
6. H. Qin, C. Feng, X. Luan and D. Yang, Nanoscale Res. Lett., 2018, 13, 264.
7. B. Cho, M. G. Hahm, M. Choi, J. Yoon, A. R. Kim, Y.-J. Lee, S.-G. Park, J.-D. Kwon, C. S. Kim and M. Song, et al., Sci. Rep., 2015, 5, 8052.
8. S.-Y. Cho, K.-H. Woo, J.-S. Kim, S.-Y. Yoon, J.-Y. Na, J.-H. Yu and Y.-B. Kim, Ann. Occup. Environ. Med., 2013, 25, 36.
9. N. Nasiri and C. Clarke, Biosensors, 2019, 9, 43.
10. G. Panneerselvam, V. Thirumal and H. M. Pandya, Arch. Acoust., 2018, 43, 357.
11. K. S. Novoselov, D. Jiang, F. Schedin, T. Booth, V. Khotkevich, S. Morozov and A. K. Geim, Proc. Natl. Acad. Sci. U. S. A., 2005, 102, 10451.
12. H. Suzuki, N. Ogura, T. Kaneko and T. Kato, Sci. Rep., 2018, 8, 11819.
13. C. Chen, M. Wang, J. Wu, H. Fu, H. Yang, Z. Tian, T. Tu, H. Peng, Y. Sun and X. Xu, et al., Sci. Adv., 2018, 4, eaat8355.
14. C. Shen and S. O. Oyadiji, Mater. Today Phys., 2020, 15, 100257.
15. A. P. Dral and J. E. ten Elshof, Sens. Actuators, B, 2018, 272, 369.
16. R. Kumar, N. Goel, M. Hojamberdiev and M. Kumar, Sens. Actuators, A, 2020, 303, 111875.
17. P. Bhattacharyya and D. Acharyya, IEEE Sens. J., 2021, 21, 22414.
18. H. Mi, Q. Zhou and W. Zeng, Appl. Surf. Sci., 2021, 563, 150329.
19. X. Zhang, Z. Lai, C. Tan and H. Zhang, Angew. Chem., Int. Ed., 2016, 55, 8816.
20. E. Lee and D.-J. Kim, J. Electrochem. Soc., 2020, 167, 037515.
21. S. Sinha, Y. Takabayashi, H. Shinohara and R. Kitaura, 2D Mater., 2016, 3, 035010.
22. M. S. Khan, A. Srivastava and R. Pandey, RSC Adv., 2016, 6, 72634.
23. J. Wu, Y. Wei, W. Shen, Y. Xiong, C. Lin, Y. Gao, A. AL-Ammari, K. Liu, T. Ma and J. Chen, et al., Appl. Phys. Lett., 2020, 116, 261903.
24. C. Gong, J. Chu, C. Yin, C. Yan, X. Hu, S. Qian, Y. Hu, K. Hu, J. Huang and H. Wang, et al., Adv. Mater., 2019, 31, 1903580.
25. M. Altarawneh, A. Marashdeh and B. Z. Dlugogorski, Phys. Chem. Chem. Phys., 2015, 17, 9341.
26. X. Huang, L. Yan, Y. Zhou, Y. Wang, H.-Z. Song and L. Zhou, J. Phys. Chem. Lett., 2021, 12, 525.
27. R. Zacharia, H. Ulbricht and T. Hertel, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 69, 155406.
28. H. Liu, A. T. Neal, Z. Zhu, Z. Luo, X. Xu, D. Tománek and P. D. Ye, ACS Nano, 2014, 8, 4033.
29. Y. Cai, G. Zhang and Y.-W. Zhang, J. Am. Chem. Soc., 2014, 136, 6269.
30. S. Bruzzone and G. Fiori, Appl. Phys. Lett., 2011, 99, 222108.
31. S. Pervaiz, M. U. Saeed, S. Khan, B. Asghar, Y. Saeed, H. O. Elansary and A. Bacha, RSC Adv., 2024, 14, 16284.
32. X. Sun, Q. Yang, R. Meng, C. Tan, Q. Liang, J. Jiang, H. Ye and X. Chen, Appl. Surf. Sci., 2017, 404, 291.
33. L. Kou, T. Frauenheim and C. Chen, J. Phys. Chem. Lett., 2014, 5, 2675.
34. B. Zhu, K. Zheng, X. Chen, J. Qiu, H. Guo, F. Zhang, L. Lang, J. Yu and J. Bao, Phys. Chem. Chem. Phys., 2021, 23, 1675.
35. Y.-H. Zhang, Y.-B. Chen, K.-G. Zhou, C.-H. Liu, J. Zeng, H.-L. Zhang and Y. Peng, Nanotechnology, 2009, 20, 185504.
36. L. Chhana, B. Lalroliana, R. C. Tiwari, B. Chettri, L. Pachuau, S. Gurung, L. Vanchhawng, D. P. Rai, L. Zuala and R. Madaka, ACS Omega, 2022, 7, 40176.
37. B. A. Kalwar, W. Fangzong, A. M. Soomro, M. R. Naich, M. H. Saeed and I. Ahmed, RSC Adv., 2022, 12, 34185.
38. V. Babar, H. Vovusha and U. Schwingenschlögl, ACS Appl. Nano Mater., 2019, 2, 6076.
39. A. Bafekry, M. Faraji, M. Fadlallah, A. A. Ziabari, A. B. Khatibani, S. Feghhi, M. Ghergherehchi and D. Gogova, Appl. Surf. Sci., 2021, 564, 150326.
40. N. Tit, K. Said, N. M. Mahmoud, S. Kouser and Z. H. Yamani, Appl. Surf. Sci., 2017, 394, 219.
41. X.-f. Yu, Y.-c. Li, J.-b. Cheng, Z.-b. Liu, Q.-z. Li, W.-z. Li, X. Yang and B. Xiao, ACS Appl. Mater. Interfaces, 2015, 7, 13707.
42. X.-P. Chen, L.-M. Wang, X. Sun, R.-S. Meng, J. Xiao, H.-Y. Ye and G.-Q. Zhang, IEEE Electron Device Lett., 2017, 38, 661.
43. Z. Xu, Z. Shi, M. Wang, R. Song, X. Zhang, G. Liu and G. Qiao, Sens. Actuators, A, 2021, 328, 112766.
44. P. Giannozzi, S. Baroni, N. Bonini, M. Calandra, R. Car, C. Cavazzoni, D. Ceresoli, G. L. Chiarotti, M. Cococcioni and I. Dabo, et al., J. Phys.: Condens. Matter, 2009, 21, 395502.
45. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
46. D. Vanderbilt, Phys. Rev. B: Condens. Matter Mater. Phys., 1990, 41, 7892.
47. S. Grimme, J. Comput. Chem., 2006, 27, 1787.
48. U. Khan, M. U. Saeed, H. O. Elansary, I. M. Moussa and Y. Saeed, et al., RSC Adv., 2024, 14, 4844.
49. F. A. Khan, M. U. Saeed, U. Khan, H. O. Elansary, A. Z. Dewidar and Y. Saeed, et al., Solid State Sci., 2024, 153, 107554.
50. Z. Ali, A. Razzaq, S. M. Ali, M. U. Saeed, H. O. Elansary, I. M. Moussa, M. A. El-Sheikh, A. U. R. Bacha and Y. Saeed, J. Electron. Mater., 2024, 53, 3834.
51. S. M. Ali, M. U. Saeed, H. O. Elansary and Y. Saeed, RSC Adv., 2024, 14, 3178.
52. H. J. Kulik, M. Cococcioni, D. A. Scherlis, and N. Marzari, arXiv, 2006, preprint, arXiv:cond-mat/0608285,  DOI:10.48550/arXiv.cond-mat/0608285.
53. H. J. Monkhorst and J. D. Pack, Phys. Rev. B, 1976, 13, 5188.
54. G. Henkelman, A. Arnaldsson and H. Jónsson, Comput. Mater. Sci., 2006, 36, 354.
55. R. Shahriar, O. Hassan and M. K. Alam, RSC Adv., 2022, 12, 16732.
56. V. Mehta, H. S. Saini, S. Srivastava, M. K. Kashyap and K. Tankeshwar, J. Phys. Chem. C, 2019, 123, 25052.
57. V. Mehta, H. S. Saini, S. Srivastava, M. K. Kashyap and K. Tankeshwar, Mater. Today Commun., 2021, 26, 102100.
58. A. Sengupta, Appl. Surf. Sci., 2018, 451, 141.
59. D. Chidambaram, R. Kalidoss, K. Pushparaj, V. J. Surya and Y. Sivalingam, Mater. Sci. Eng., B, 2022, 280, 115694.

---