Coalescence of electrically charged liquid marbles
Zhou
Liu
ab,
Xiangyu
Fu
a,
Bernard P.
Binks
c and
Ho Cheung
Shum
*ab
aMicrofluidics & Soft Matter Group, Department of Mechanical Engineering, University of Hong Kong, Pokfulam Road, Hong Kong, China. E-mail: ashum@hku.hk
bHKU-Shenzhen Institute of Research and Innovation, Shenzhen, Guangdong 518000, China
cDepartment of Chemistry, University of Hull, Hull, HU6 7RX, UK
First published on 4th May 2016
Abstract
In this work, we investigated the coalescence of liquid water marbles driven by a DC electric field. We have found that two contacting liquid marbles can be forced to coalesce when they are charged by a sufficiently high voltage. The threshold voltage leading to the electro-coalescence sensitively depends on the stabilizing particles as well as the surface tension of the aqueous phase. By evaluating the electric stress and surface tension effect, we attribute such coalescence to the formation of a connecting bridge driven by the electric stress. This liquid bridge subsequently grows and leads to the merging of the marbles. Our interpretation is confirmed by the scaling relation between the electric stress and the restoring capillary pressure. In addition, multiple marbles in a chain can be driven to coalesce by a sufficiently high threshold voltage that increases linearly with the number of the marbles. We have further proposed a simple model to predict the relationship between the threshold voltage and the number of liquid marbles, which agrees well with the experimental results. The concept of electro-coalescence of liquid marbles can be potentially useful in their use as containers for chemical and biomedical reactions involving multiple reagents.
Introduction
Liquid water marbles are composed of water droplets in air encapsulated by a shell of particles.1–5 They can be easily generated by depositing and rolling the water droplets on a layer of hydrophobic particles either manually1 or automatically.6 Similar to the particle adsorption in Pickering emulsions,7–9 these hydrophobic particles can also irreversibly absorb on the liquid–air interfaces to minimize surface energy,1 making droplets non-wetting and non-sticky with the substrate. Therefore, liquid marbles are excellent carriers for transporting and handling small volumes of liquids in air.5,10–14 They have been shown to be a promising platform for different droplet-based applications, including water pollution detection,15 gas sensing,16 as micro-pumps,14 for optical absorbance detection12 and for fabrication of color pigments.17 In particular, liquid marbles are advantageous when used as miniaturized reactors for chemical reaction11,12 and bioassays.18–20 The amount of reagents as well as the reaction time can be significantly reduced due to the small volume of liquids involved.11 In these micro-reactor applications, each liquid marble encapsulates one type of reagent or ingredient. By manipulating them to coalesce, the encapsulated reagents can mix, and the corresponding reactions or tests can be initiated.11,18 Currently, most of these concepts and ideas are demonstrated by manually pushing the liquid marbles to move or coalesce. However, manual manipulation of liquid marbles is inefficient and tedious when a large quantity of sample is involved, hence limiting the realization of the potential of liquid marble-based liquid handling. Therefore, on-demand and fast coalescence of liquid marbles controlled by external forces is highly desired.13 For instance, a magnetic field has been applied to initiate the coalescence between marbles coated by magnetic particles.11,12 However, this approach is only applicable to liquids that can be coated by specific magnetic particles. With the exception of a recently reported method that achieves the opening and closing of liquid marbles using acoustic levitation,21 general approaches that do not rely on the unique properties of the coating particles or liquids for manipulating the liquid marbles in a controlled manner are rarely explored.In this work, we propose to manipulate the coalescence of liquid marbles by applying an electric field. An electric field has been demonstrated previously as an efficient tool for deforming the liquid interface,22–25 for demulsifying emulsions stabilized by surfactants26,27 or colloidal particles28 as well as for actuating liquid marbles.2,13,29–31 A systematic study of the coalescence of liquid marbles driven by an electric stress is reported here for the first time. We found that two contacting liquid marbles can be forced to coalesce efficiently upon applying a voltage above a threshold value. We have systematically studied the dependence of this threshold voltage on various parameters and found it to be significantly influenced by the diameter of the coating particles and the surface tension of the liquid core. We attribute the electro-coalescence of liquid marbles to the electric stress that deforms the liquid interfaces to form a bridge and initiate coalescence. This interpretation is confirmed by evaluating the magnitude of the electric stress as well as the surface tension stress. In addition, we have found that a sufficiently high voltage can also lead to the coalescence of multiple liquid marbles. By treating a chain of liquid marbles as a series of electric capacitors, we propose a simple model to predict the dependence of the threshold voltage on the number of liquid marbles; the model predictions agree well with the experimental results. Our approach of imposing an electric stress offers a general, robust and feasible way to manipulate the coalescence of liquid marbles, which is a breakthrough potentially needed to trigger liquid marble-based applications such as chemical or biomedical reactions.
Experimental
Materials
Liquid marbles were prepared by coating aqueous solutions with particles. The electrical conductivity of the liquid κ was tuned by adding different concentrations (≤10−1 M) of sodium chloride (AR, Aladdin, China) in deionized water (Direct-Q, Merck Millipore, Germany) and measured using an electrical conductivity meter (CONT 610, EUtech Inc.). The viscosity of the liquid η was varied by dissolving different concentrations (≤2 wt%) of sodium alginate (BioReagent, Aladdin, China) in water and measured using a viscosity meter (μVISC, Pheosense Inc.). The surface tension of the liquids γ was changed by dissolving different concentrations (≤8 × 10−3 M) of sodium dodecyl sulfate, SDS (AR, Aladdin, China) in water and measured using the pendant drop method.32,33 The coating particles included PTFE grains (diameter = 35 μm, contact angle between water and particle = 110°,34,35 Sigma-Aldrich, USA), silicone particles (diameter = 2.0 μm, 4.5 μm or 6.0 μm, contact angle between water and particle = 91°,36 Momentive Performance Material Inc., Japan) as well as silica particles (diameter = 92 μm, Qinhuang Glass Microsphere, China), the latter being rendered hydrophobic (contact angle = 112°, measured between water and a glass slide that was treated under the same conditions as silica particles) using trimethoxy(octadecyl)silane (technical grade, Sigma-Aldrich, USA) following the protocol in ref. 37.Liquid marble formation
To form liquid marbles, we deposited water droplets on a glass substrate covered by a layer of particles. The volume V of the liquid marble (from 3.6 μL to 16.1 μL) was precisely controlled by dispensing droplets vertically through nozzles with various diameters.38 The droplets were then rolled around five times to ensure a full coverage of particles on the surface. After formation, these liquid marbles were transported to a PDMS substrate (Dow Corning, USA). Two marbles were pushed to contact each other for subsequent experiments.Electro-coalescence of liquid marbles
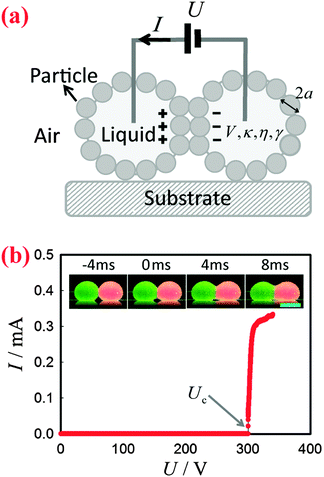
To apply a DC voltage, we inserted electrodes made of metal wires into the liquid marbles to charge them using a high-voltage supply (Tianjin Dongwen, China), as shown schematically in Fig. 1(a). The applied voltage U was gradually increased until the liquid marbles coalesced (they are in contact before application of the field). The corresponding current I was monitored by an electrochemical workstation (660E, CH Instrument, USA). In the meantime, the morphology of the marbles was recorded by a high-speed camera (Phantom v9.1, USA) coupled with a commercial lens (AF Nikkor, Nikon, Japan). The frame rate was set to 500 fps. All the experiments were carried out at a temperature of 24 ± 1 °C and at a relative humidity of 50 ± 5%. For any pair of marbles, the experiments were repeated at least 10 times to determine Uc.Results and discussion
Electro-coalescence of two liquid marbles
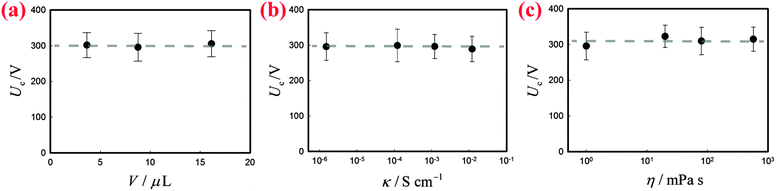
Two bare liquid droplets will coalesce into a single one following an initial contact, seeking to minimize the surface energy. When two liquid marbles contact each other, however, such coalescence may be avoided since the presence of two particle layers prevents the direct contact of the liquid surfaces. To trigger the coalescence, we apply an increasing DC voltage to two contacting liquid marbles. When the applied voltage is small, the two contacting liquid marbles remain stable (Fig. 1(b), inset: −4 ms). Such a stable state is also indicated by the nil current in the electric circuit shown in Fig. 1(b). Interestingly, when the applied voltage is increased above a critical value Uc, the two liquid marbles coalesce into a single one. For instance, two water marbles coated with PTFE particles coalesce within several milliseconds at a threshold voltage of 300 V, as shown in Fig. 1(b), insets: 0–8 ms. The coalescence event is also reflected by a significant increase in current, also shown in Fig. 1(b). The critical voltage for electro-coalescence of liquid marbles is much larger than that for w/o emulsions stabilized by surfactant, which is typically on the scale of 1 V.26,27,39 After electro-coalescence, the surface area of the marble is significantly reduced, leading to a more densely packed particle layer than in the two original marbles. Therefore, the coalesced liquid marble remains in the non-spherical shape for a long time as the relaxation of the liquid surfaces is resisted by the particle layer.In order to understand the origin of the threshold voltage responsible for the electro-coalescence of liquid marbles, we first investigate the influence of the volume V and the electrical conductivity κ of the liquid core on the threshold voltage Uc. Considering that both V and κ can directly affect the electrical resistance of the liquid core, we expect Uc may change accordingly. Surprisingly, the results indicate that for the liquid marble with a volume V ranging from 3.6 μL to 16 μL, the critical voltage Uc is more or less constant, as shown in Fig. 2(a). Similarly, the critical voltage remains identical when the electrical conductivity of water is significantly increased from 1.5 × 10−6 S cm−1 to 1.2 × 10−2 S cm−1, as shown in Fig. 2(b). We attribute these results to the extremely high electrical resistance of particle layers compared to that of the water core considering that air40 (κair = 3–8 × 10−15 S cm−1) is much less conductive than water41 (κdeionized water = 5.5 × 10−6 S cm−1). As such, the applied voltage mainly falls on the particle layers that separate the marbles rather than the liquid cores of the marbles. Therefore, any change in electrical resistance of the liquid core cannot affect the threshold voltage significantly. These results imply that it is the voltage drop across the particle layer (through air) that contributes to the electro-coalescence of liquid marbles. Moreover, we have studied the effect of the liquid viscosity η on the critical voltage Uc. The results also show that the critical voltage is independent of the liquid viscosity as Uc does not change considerably when η increases from 1 mPa s to 576 mPa s (Fig. 2(c)). This suggests that the dynamic drainage of liquid does not contribute significantly during the electro-coalescence events.
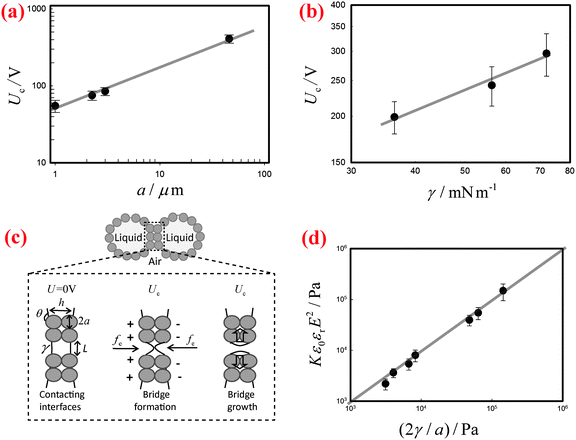
By contrast, the threshold voltage Uc depends markedly on the radius a of the stabilizing particles as well as on the surface tension γ of the liquid. We systematically measure Uc of the liquid marbles fabricated by coating the surfaces of water droplets with silicone particles of various sizes and with hydrophobic silica particles of one large size. The results indicate that Uc increases significantly as the size of the particles increases, as shown in Fig. 3(a). In addition, when the surface tension γ of water is varied by the addition of SDS surfactants, the corresponding Uc decreases with decreasing γ (Fig. 3(b)).
To understand the threshold voltage Uc responsible for the electro-coalescence of liquid marbles, we analyze the stresses on the particle-stabilized interfaces by referring to recent work on Pickering emulsions.28 When a voltage U is applied between the two contacting liquid marbles, the opposing particle-stabilized liquid interfaces are oppositely charged, with most of the applied voltage drop occurring across them, as shown schematically in Fig. 3(c). The charging induces an electric stress fe on the liquid interfaces, which scales as ε0εrE2, where ε0εr is the permittivity of the liquid and E is the local electric field strength defined as U/h. The separation distance h between liquid interfaces can be estimated as h ∼ 2a(1 − cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ), with a being the radius of the particles and θ being the contact angle between particle and water. Such an electric stress would deform the liquid interface to form a conical tip within the particle shells. When the electro-deformation is pronounced enough, a liquid bridge would form and subsequently grow to cause coalescence,28,42 as shown schematically in Fig. 3(c). However, the electro-deformation of the liquid interfaces is resisted by a restoring capillary pressure that scales as 2γ/L, where L is the local radius of the formed tips (Fig. 3(c)). Typically, the coating particles are closely packed at the liquid–air interfaces in a hexagonal pattern.43 Recent work on Pickering emulsions indicates that some defects exist randomly at the interfaces,28 with a size on the same scale as the particle diameter. Such defects are the most likely places where the coalescence starts. Similarly, these defects also exist in our formed liquid marbles. By inputting L ∼ a, and values of εr, Uc, a, and γ, we can evaluate the competition between the electric stress ε0εrE2 and the restoring capillary pressure 2γ/a. Following this, we re-plot the data in Fig. 3(a) and (b) with axes of Kε0εrE2 and 2γ/a in Fig. 3(d), where K = 0.30 is a dimensionless pre-factor. Indeed, the electric stress and the restoring capillary pressure are of the same order of magnitude, and all the results collapse onto a line with a slope of unity, in agreement with our hypothesis. Considering our scaling relation is modified from the work of Chen et al.28 by including the role of contact angle, we have also compared the dimensionless pre-factor between liquid marbles and Pickering emulsions. Using typical values of the relevant variables in Pickering emulsions (εr = 78.5, Uc = 80 V, a = 3 μm, θ = 133° and γ = 30 mN m−1)28 in our modified scaling relation, we deduce K = 0.24, which is close to the value obtained for liquid marbles. Moreover, following the scaling relationship ε0εrE2 ∼ 2γ/a, we can deduce the dependence of the threshold voltage on the particle diameter and surface tension as Uc ∝ a0.5 and Uc ∝ γ0.5, respectively. Indeed, these two predicted relations agree well with our experimental data, as indicated by the solid lines in Fig. 3(a) and (b). Therefore, we conclude that it is the electro-driven deformation of the liquid interface at the defects that triggers the electro-coalescence between two touching liquid marbles.
θ), with a being the radius of the particles and θ being the contact angle between particle and water. Such an electric stress would deform the liquid interface to form a conical tip within the particle shells. When the electro-deformation is pronounced enough, a liquid bridge would form and subsequently grow to cause coalescence,28,42 as shown schematically in Fig. 3(c). However, the electro-deformation of the liquid interfaces is resisted by a restoring capillary pressure that scales as 2γ/L, where L is the local radius of the formed tips (Fig. 3(c)). Typically, the coating particles are closely packed at the liquid–air interfaces in a hexagonal pattern.43 Recent work on Pickering emulsions indicates that some defects exist randomly at the interfaces,28 with a size on the same scale as the particle diameter. Such defects are the most likely places where the coalescence starts. Similarly, these defects also exist in our formed liquid marbles. By inputting L ∼ a, and values of εr, Uc, a, and γ, we can evaluate the competition between the electric stress ε0εrE2 and the restoring capillary pressure 2γ/a. Following this, we re-plot the data in Fig. 3(a) and (b) with axes of Kε0εrE2 and 2γ/a in Fig. 3(d), where K = 0.30 is a dimensionless pre-factor. Indeed, the electric stress and the restoring capillary pressure are of the same order of magnitude, and all the results collapse onto a line with a slope of unity, in agreement with our hypothesis. Considering our scaling relation is modified from the work of Chen et al.28 by including the role of contact angle, we have also compared the dimensionless pre-factor between liquid marbles and Pickering emulsions. Using typical values of the relevant variables in Pickering emulsions (εr = 78.5, Uc = 80 V, a = 3 μm, θ = 133° and γ = 30 mN m−1)28 in our modified scaling relation, we deduce K = 0.24, which is close to the value obtained for liquid marbles. Moreover, following the scaling relationship ε0εrE2 ∼ 2γ/a, we can deduce the dependence of the threshold voltage on the particle diameter and surface tension as Uc ∝ a0.5 and Uc ∝ γ0.5, respectively. Indeed, these two predicted relations agree well with our experimental data, as indicated by the solid lines in Fig. 3(a) and (b). Therefore, we conclude that it is the electro-driven deformation of the liquid interface at the defects that triggers the electro-coalescence between two touching liquid marbles.
Electro-coalescence of multiple liquid marbles
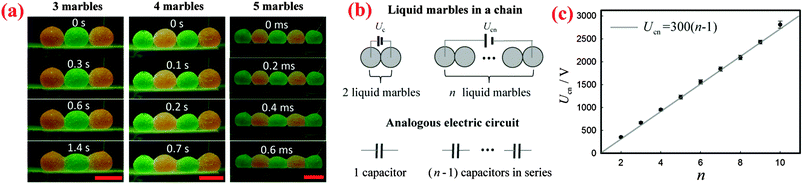
Apart from two marbles, a sufficiently large voltage can cause the coalescence of a chain of multiple marbles. By simply applying a sufficiently high voltage to the liquid marbles at the two ends of the chain, we can trigger the electro-coalescence of 3, 4 and 5 marbles as shown in Fig. 4(a). We find that the threshold voltage required for coalescence simply increases with the number of marbles: Ucn = 664 ± 23 V for 3 marbles, Ucn = 950 ± 26 V for 4 marbles and Ucn = 1224 ± 43 V for 5 marbles, respectively.To interpret the dependence of threshold voltage Ucn on the number of marbles n, we first investigate the voltage drop for each pair of liquid marbles. When a voltage is applied across two liquid marbles, most of the voltage drop is across the air gap between the liquid surfaces. Therefore, we can treat the air gap that exists between each pair of neighboring marbles as a capacitor. Based on this, when n liquid marbles in a chain are charged by a voltage U, the corresponding analogous circuit is (n − 1) capacitors in series connected to the power source, as shown schematically in Fig. 4(b). For each capacitor, the corresponding voltage drop is U/(n − 1). The liquid marbles start to coalesce when the voltage drop on each capacitor exceeds the threshold voltage of two marbles: U/(n − 1) > Uc. Therefore, the critical voltage leading to the coalescence of multiple marbles can be written as Ucn = (n − 1)Uc. To vindicate this simple model, we measured the threshold voltages for different numbers of water marbles. Indeed, the results show that the critical voltage Ucn increases linearly with the number of the marbles n as seen in Fig. 4(c), which agrees well with our model. Moreover, the slope of the line is around 300 V, which is equal to the threshold voltage Uc of two marbles, in excellent agreement with our prediction.
Conclusions
In this work, we have investigated the electrically controlled coalescence of two and multiple liquid marbles in a chain. We have found that two oppositely charged liquid marbles coalesce above a threshold applied voltage. We show that such coalescence occurs when the electric stress exceeds the surface tension and deforms the liquid interface to form a liquid bridge connecting the liquid marbles. This bridge subsequently grows and eventually leads to the coalescence of the marbles. Moreover, we have extended our approach of the electro-coalescence of two marbles to multiple marbles. By treating a chain of liquid marbles as a series of electrical capacitors, we put forward a simple model to predict the threshold voltage for electro-coalescence based on the number of liquid marbles, with excellent agreement with experiment. Our approach as well as understanding of the electro-assisted coalescence of liquids marbles facilitates a more widespread use of a large number of liquid marbles as containers for chemical and biomedical reactions where controllable mixing of liquid reagents represents a current limitation.Acknowledgements
This research was supported by the Early Career Scheme (HKU 707712P) and the General Research Fund (HKU 719813E, 17304514 and 17306315) from the Research Grants Council of Hong Kong, as well as the General Program (21476189/B060201) from the National Natural Science Foundation of China.References
- P. Aussillous and D. Quéré, Nature, 2001, 411, 924–927 CrossRef CAS PubMed
.
- P. Aussillous and D. Quéré, Proc. – R. Soc. Edinburgh, Sect. A: Math., 2006, 462, 973–999 CrossRef CAS
.
- G. McHale and M. I. Newton, Soft Matter, 2015, 11, 2530–2546 RSC
.
- E. Bormashenko, Soft Matter, 2012, 8, 11018–11021 RSC
.
- G. McHale and M. I. Newton, Soft Matter, 2011, 7, 5473–5481 RSC
.
- Y. Liu, C. P. Hugentobler and H. C. Shum, J. Colloid Sci. Biotechnol., 2013, 2, 350–354 CrossRef CAS
.
-
Colloidal Particles at Liquid Interfaces, ed. B. P. Binks and T. S. Horozov, Cambridge University Press, Cambridge, 2006 Search PubMed
.
- S.-H. Kim, A. Abbaspourrad and D. A. Weitz, J. Am. Chem. Soc., 2011, 133, 5516–5524 CrossRef CAS PubMed
.
- I. Akartuna, A. R. Studart, E. Tervoort, U. T. Gonzenbach and L. J. Gauckler, Langmuir, 2008, 24, 7161–7168 CrossRef CAS PubMed
.
- E. Bormashenko, Curr. Opin. Colloid Interface Sci., 2011, 16, 266–271 CrossRef CAS
.
- Y. Xue, H. Wang, Y. Zhao, L. Dai, L. Feng, X. Wang and T. Lin, Adv. Mater., 2010, 22, 4814–4818 CrossRef CAS PubMed
.
- Y. Zhao, Z. Xu, H. Niu, X. Wang and T. Lin, Adv. Funct. Mater., 2015, 25, 437–444 CrossRef CAS
.
- C. H. Ooi and N.-T. Nguyen, Microfluid. Nanofluid., 2015, 19, 483–495 CrossRef CAS
.
- E. Bormashenko, R. Balter and D. Aurbach, Appl. Phys. Lett., 2010, 97, 091908 CrossRef
.
- E. Bormashenko and A. Musin, Appl. Surf. Sci., 2009, 255, 6429–6431 CrossRef CAS
.
- J. Tian, T. Arbatan, X. Li and W. Shen, Chem. Commun., 2010, 46, 4734–4736 RSC
.
- H. Gu, B. Ye, H. Ding, C. Liu, Y. Zhao and Z. Gu, J. Mater. Chem. C, 2015, 3, 6607–6612 RSC
.
- T. Arbatan, L. Li, J. Tian and W. Shen, Adv. Healthcare Mater., 2012, 1, 80–83 CrossRef CAS PubMed
.
- T. Arbatan, A. Al-Abboodi, F. Sarvi, P. P. Y. Chan and W. Shen, Adv. Healthcare Mater., 2012, 1, 467–469 CrossRef CAS PubMed
.
- F. Sarvi, T. Arbatan, P. P. Y. Chan and W. Shen, RSC Adv., 2013, 3, 14501–14508 RSC
.
- D. Zang, J. Li, Z. Chen, Z. Zhai, X. Geng and B. P. Binks, Langmuir, 2015, 31, 11502–11507 CrossRef CAS PubMed
.
- D. A. Saville, Annu. Rev. Fluid Mech., 1997, 29, 27–64 CrossRef
.
- A. R. Thiam, N. Bremond and J. Bibette, Phys. Rev. Lett., 2009, 102, 188304 CrossRef PubMed
.
- W. D. Ristenpart, J. C. Bird, A. Belmonte, F. Dollar and H. A. Stone, Nature, 2009, 461, 377–380 CrossRef CAS PubMed
.
- T. Kong, Z. Liu, L. Wang and H. C. Shum, Phys. Rev. Appl., 2015, 3, 034010 CrossRef
.
- X. Niu, F. Gielen, A. J. demello and J. B. Edel, Anal. Chem., 2009, 81, 7321–7325 CrossRef CAS PubMed
.
- C. Priest, S. Herminghaus and R. Seemann, Appl. Phys. Lett., 2006, 89, 134101 CrossRef
.
- G. Chen, P. Tan, S. Chen, J. Huang, W. Wen and L. Xu, Phys. Rev. Lett., 2013, 110, 064502 CrossRef PubMed
.
- M. I. Newton, D. L. Herbertson, S. J. Elliott, N. J. Shirtcliffe and G. McHale, J. Appl. Phys., 2006, 40, 20 Search PubMed
.
- G. McHale, D. L. Herbertson, S. J. Elliott, N. J. Shirtcliffe and M. I. Newton, Langmuir, 2007, 23, 918–924 CrossRef CAS PubMed
.
- E. Bormashenko, R. Pogreb, R. Balter, O. Gendelman and D. Aurbach, Appl. Phys. Lett., 2012, 100, 151601 CrossRef
.
- B. Song and J. Springer, J. Colloid Interface Sci., 1996, 184, 64–76 CAS
.
- B. Song and J. Springer, J. Colloid Interface Sci., 1996, 184, 77–91 CAS
.
-
Polymer interface and adhesion, ed. S. Wu and M. Dekker, New York, 1982 Search PubMed
.
- B. Jańczuk and T. Białlopiotrowicz, J. Colloid Interface Sci., 1989, 127, 189–204 CrossRef
.
- K. Matsukawa, M. Watanabe, T. Hamada, T. Nagase and H. Naito, Int. J. Polym. Sci., 2012, 2012, 852063 Search PubMed
.
- S. A. Kulkarni, S. B. Ogale and K. P. Vijayamohanan, J. Colloid Interface Sci., 2008, 318, 372–379 CrossRef CAS PubMed
.
- Z. Liu, X. Fu, B. P. Binks and H. C. Shum, Langmuir, 2015, 31, 11236–11242 CrossRef CAS PubMed
.
- Z. Liu, S. T. Chan, H. A. Faizi, R. C. Roberts and H. C. Shum, Lab Chip, 2015, 15, 2018–2024 RSC
.
- S. D. Pawar, P. Murugavel and D. M. Lal, J. Geophys. Res., 2009, 114, 02205 CrossRef
.
- R. M. Pashley, M. Rzechowicz, L. R. Pashley and M. J. Francis, J. Phys. Chem. B, 2005, 109, 1231–1238 CrossRef CAS PubMed
.
- J. C. Bird, W. D. Ristenpart, A. Belmonte and H. A. Stone, Phys. Rev. Lett., 2009, 103, 164502 CrossRef PubMed
.
- D. Vella, P. Aussillous and L. Mahadevan, Europhys. Lett., 2004, 68, 212–218 CrossRef CAS
.
| This journal is © The Royal Society of Chemistry 2017 |