Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
The effect of dissolved chlorides on the photocatalytic degradation properties of titania in wastewater treatment†
Maicon
Delarmelina
 *ab,
Mbongiseni W.
Dlamini
*ab,
Mbongiseni W.
Dlamini
 bc,
Samuel
Pattisson
bc,
Samuel
Pattisson
 c,
Philip R.
Davies
c,
Philip R.
Davies
 bc,
Graham J.
Hutchings
bc,
Graham J.
Hutchings
 bc and
C. Richard A.
Catlow
bc and
C. Richard A.
Catlow
 abd
abd
aSchool of Chemistry, Cardiff University, Cardiff, CF10 3AT, UK. E-mail: DelarmelinaM@cardiff.ac.uk
bUK Catalysis Hub, Research Complex at Harwell, STFC Rutherford Appleton Laboratory, Didcot, Oxfordshire OX11 0FA, UK
cMax Planck-Cardiff Centre on the Fundamentals of Heterogeneous Catalysis FUNCAT, Cardiff Catalysis Institute, School of Chemistry, Cardiff University, Main Building, Park Place, Cardiff, CF10 3AT, UK
dDepartment of Chemistry, University College London, 20 Gordon St., London WC1 HOAJ, UK
First published on 6th January 2023
Abstract
We investigate the effect of chlorides on the photocatalytic degradation of phenol by titania polymorphs (anatase and rutile). We demonstrate how solubilised chlorides can affect the hydroxyl radical formation on both polymorphs with an overall effect on their photodegradative activity. Initially, the photocatalytic activity of anatase and rutile for phenol degradation is investigated in both standard water and brines. With anatase, a significant reduction of the phenol conversion rate is observed (from a pseudo-first-order rate constant k = 5.3 × 10−3 min−1 to k = 3.5 × 10−3 min−1). In contrast, the presence of solubilised chlorides results in enhancement of rutile activity under the same reaction conditions (from 2.3 × 10−3 min−1 to 4.8 × 10−3 min−1). Periodic DFT methods are extensively employed and we show that after the generation of charge separation in the modelled titania systems, adsorbed chlorides are the preferential site for partial hole localisation, although small energy differences are computed between partially localised hole densities over adsorbed chloride or hydroxyl. Moreover, chlorides can reduce or inhibit the ability of r-TiO2 (110) and a-TiO2 (101) systems to localise polarons in the slab structure. These results indicate that both mechanisms – hole scavenging and the inhibition of hole localisation – can be the origin of the effect of chlorides on photocatalytic activity of both titania polymorphs. These results provide fundamental insight into the photocatalytic properties of titania polymorphs and elucidate the effect of adsorbed anions over radical formation and oxidative decomposition of organic pollutants.
1. Introduction
Dissolved organic compounds are one of the main components of polluted wastewater. They can originate from non-biodegradable pesticides, pharmaceuticals, and food additives, amongst other industrial compounds and by-products.1–3 Extensive work has been undertaken during the last decades to eliminate these contaminants prior to reutilisation or the return of the effluent to nature.4,5 In this context, the photodegradation of organic pollutants by heterogeneous catalysts under visible-UV light has shown very promising results for oxidation and mineralisation of such organic materials, with the additional benefit of eliminating the need to use hazardous materials, such as hypochlorite, peroxide, and ozone, in these processes.6Titania-based photocatalytic materials have been widely employed in such applications and are currently considered to be one of the most effective materials for environmental applications.1 In addition to its photocatalytic properties, TiO2 is low-cost, has high chemical and biological stability, and low toxicity, all of which make the material ideal for wastewater treatment.1,7 Although TiO2 has shown promise in the degradation of organic compounds dissolved in standard water solutions, the performance of such photocatalysts was observed to decrease drastically when investigating wastewater solutions containing a high content of dissolved salts.8,9 This depletion of titania's photoactivity is often attributed to blockage of surface sites, impeding the adsorption of hydroxyl anions and formation of hydroxyl radicals, or, hole trapping/scavenging, inhibiting the formation of active radicals responsible for the oxidation of organic pollutants.8
Conversely, the opposite effect has also been reported when halide anions were present in a wide range of experimental conditions. Pre-treatment of titania catalysts by HF or HCl, for instance, have been demonstrated to enhance the decomposition rate of organic derivatives such as benzene, phenol, and toluene,10,11 and the HCl pre-treated systems were shown to be active for the decomposition of branched aromatics.11 A similar enhancement was reported for the photodecomposition of Reactive Brilliant Orange K–R in NaCl solutions,12 during the decomposition of halogenated organic derivatives (perchloroethylene),13 and after chlorination of titania surface by CHCl3 decomposition or HCl impregnation.14,15 Although these distinct observations of depletion/enhancement of activity seem contradictory, in principle they could be rationalized by comparison of the distinct chemical system and reaction conditions employed in each case (pH, substrate, hydrogenation of the catalyst surface, etc.) Nevertheless, there is a need for a more thorough appraisal and rationalisation of the halide effect over the degradative photocatalytic activity of titania, which can be key for the proposal of new mitigation approaches for reduced photoactivity performance of TiO2-based catalysts in contaminated waters.
In this work, we compare periodic DFT based calculations of the potential role of solubilised chlorides on the photocatalytic activity of anatase and rutile with the experimentally observed effect of chlorides on the photocatalytic activity of the two TiO2 polymorphs for phenol decomposition. Blockage of surface sites and changes in the polaronic self-trapping ability of the different titania surfaces by adsorbed chlorides are considered in detail.
2. Methodology
Materials
All chemical reagents were used without further purification, which are listed as follows; titanium(IV) oxide-anatase (99.8%, SA: 9 m2 g−1, Merck), titanium(IV) oxide-rutile (99.99%, SA: 4 m2 g−1, Merck), sodium chloride (analytical grade reagent, Fisher Scientific), acetonitrile (HPLC grade, Fisher Scientific) and 1,4-benzoquinone (standard reference material, Aldrich).Photocatalysis experiments
The effect of chloride ions on the photocatalytic activity of anatase and rutile photocatalysts was evaluated in a batch reactor using phenol as the model organic compound.16 In a typical experiment, phenol (60 mg L−1, 110 mL) was mixed with the selected photocatalytic powder (1 g L−1) and all experiments performed under stirring in a double-walled open-top reactor coated with aluminium foil paper. Before illumination, the photocatalyst-phenol solution was stirred in the dark for 30 min to achieve an absorption–desorption equilibrium. The photocatalyst-phenol solution was irradiated with light for 4 h under constant stirring using a 300 W Xe arc lamp (1000 W m−2). During the reaction, 2 mL sample aliquots were withdrawn at specific time intervals, diluted and then centrifuged to remove the photocatalyst. Analysis for residual phenol and intermediate species formed during the reaction was performed by high-performance liquid chromatography (HPLC) and UV-Vis spectroscopy. HPLC analysis was performed on an Agilent Technologies 1200 series instrument, equipped with an Agilent C18 column, using a stepwise gradient elution mode with a mixture of acidified water (phosphoric acid) and acetonitrile as the mobile phase. The temperature of the Agilent C18 column was maintained at 30 °C during analysis. To prepare the hypersaline brines, NaCl (100 g L−1 Cl−) was added to the reaction mixture while the phenol (60 mg L−1) and photocatalyst (1 g L−1) concentrations were maintained.Computational methods
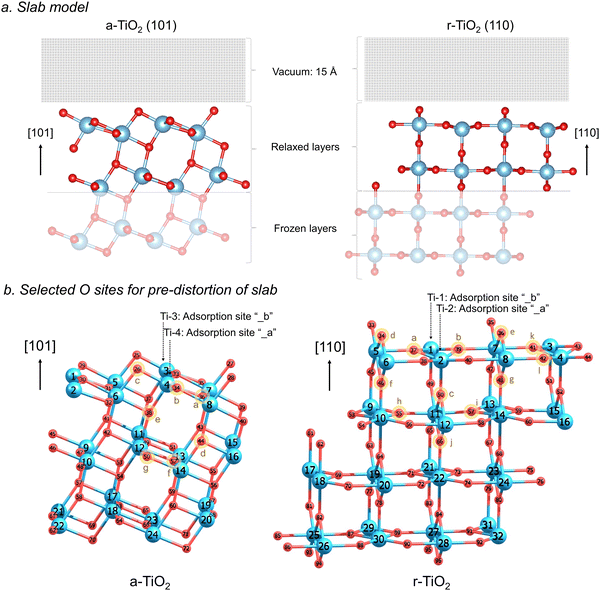
All calculations were performed using the Vienna ab initio simulation package (VASP)17–20 within the framework of periodic density functional theory (DFT). The electronic structure of all the systems modelled employed the RPBE21 functional combined with Grimme's semiclassical D3 dispersion correction22,23 and Coulomb repulsive interaction (U = 4.5 eV) for the d orbitals of Ti, in accordance with preliminary evaluation of the relative stability of the titania polymorphs investigated24 (Fig. S1, ESI†) and evaluation of the effect of distinct U values (U = 2.5 eV, 4.5 eV, and 6 eV) on the charge localisation in these systems (Table S1 and Fig. S2, S3, ESI†). The electron–ionic core interaction was represented by the projector-augmented-wave (PAW) potentials25,26 and the cut-off energy was selected after extensive benchmarking and set to 550 eV (Fig. S5 and S6, ESI†). The Ti 3d34s1 and O 2s22p4 orbitals were explicitly included as valence electrons. Brillouin zone sampling was performed using the Monkhorst-Pack scheme with a k-point grid of 7 × 7 × 1 together with a Gaussian smearing broadening of 0.02 eV. Forces and electronic SCF convergence were set at 10−2 eV Å−1 and 10−5 eV, respectively. Dipole corrections were additionally used during all calculations, according to the method by Makov & Payne27 and Neugebauer & Scheffler.28 The optimized lattice constants obtained at this level of theory were used in this work to construct the investigated surface models.The most stable surfaces of anatase and rutile were selected for this investigation, namely a-TiO2 (101) and r-TiO2 (110). The slab model for a-TiO2 (101) surface used a 2 × 1 × 3 supercell containing 24 titanium and 48 oxygen atoms, whereas for r-TiO2 (110), a 2 × 2 × 4 supercell containing 32 titanium and 64 oxygen atoms was used. In a-TiO2 (101) model, the 8 top titanium ions and 20 oxygen ions were allowed to relax, whereas for r-TiO2 (110) model, 16 top titanium ions and 32 oxygen ions were allowed to relax (see illustration in Fig. 1a). A vacuum gap of 15 Å in the z direction was added to the surface in order to avoid undesired interactions with slab images.
In order to simulate excited state electronic structures, the difference between electron spins up and down was set to 2 (N = 2), formally resulting in a triplet state. For simulation of anionic species (OH− and/or Cl−) adsorbed on the investigated surface, one excess electron was added to the system. In these cases, a neutralizing background charge was assumed to compensate the resulting negative charge over the slab. For the hole- and excess electron-containing electronic structures, one electron was removed or added, respectively, to the system. Similarly, a neutralizing background charge is assumed and the difference between electron spins up and down was set to 1 (N = 1), formally resulting in a doublet state. It is worth noting, however, the well-known limitations to this methodology due to divergence of Coulomb energy when distinct vacuum widths are inserted between slab images.29,30 Nevertheless, alternative tests performed in the presence of counterions in order to avoid the use of charged slabs resulted in unrealistic solutions of the electronic structure of the systems studied. More details are given in Tables S2–S4 and Fig. S8, in the ESI.† Therefore, utilisation of neutralizing background charge was chosen as a more appropriate approach to investigate self-trapping of polarons in this work.
For all simulations involving the triplet state (N = 2), or hole and electron-containing structures (N = 1), the ionic structure of the surfaces underwent pre-optimisations to produce local lattice distortions around selected oxygen sites, facilitating the formation of a O−–Ti3+ charge localised systems within the slab model employed in our calculations. Such pre-distortion of the ionic structure was performed by temporarily replacing one oxygen atom by one fluorine atom in a selected lattice site (Fig. 1b) and allowing the ions around it to relax. The lower charge of the fluorine/fluoride results in an outward relaxation of the surrounding titanium ions and thereby produces an appropriate geometry for charge localisation when the oxygen atom was returned to the structure. The procedure follows an approach developed by Grau-Crespo et al. for localising electrons in CeO2.31,32 Twelve distinct pre-distortions of lattice sites were considered for all calculations involving r-TiO2 (110), and seven for a-TiO2 (101), as illustrated in Fig. 1b. Additional tests of pre-distortion around Ti sites were conducted (Fig. S9 and S10, ESI†) by temporarily replacing one titanium by a scandium atom, leading to results very similar to those obtained for structures with biased O sites.
3. Results and discussion
3.1 Photocatalytic oxidation of phenol in standard water and brine solutions: anatase and rutile activities
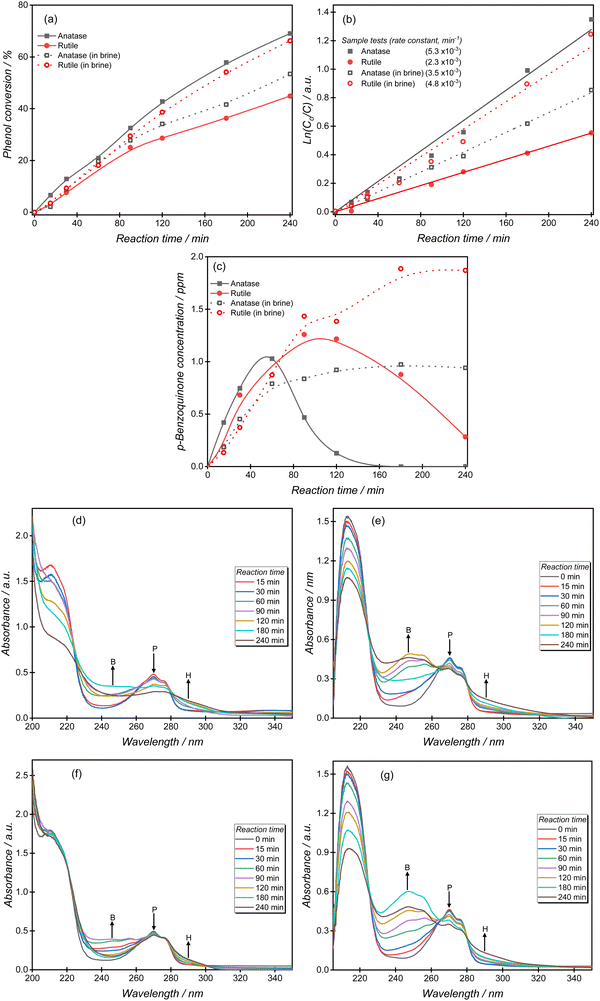
The photocatalytic oxidation of phenol is an attractive reaction to compare catalyst structure and reaction conditions, because of the relatively rapid rate and reproducibility of results. The phenol oxidation reaction is approximately twice as fast over anatase than rutile, with a pseudo-first-order rate constant of 5.3 × 10−3 min−1 calculated for the anatase reaction (Fig. 2a and b). In the presence of chloride ions, the rate of phenol oxidation over the anatase surface decreased significantly giving an apparent first-order rate constant k of 3.5 × 10−3 min−1, Fig. 2a and b. A very different effect was observed over rutile however, with the photocatalytic activity in brines accelerated by a factor of more than two, with the pseudo-first-order rate constant increasing from 2.3 × 10−3 min−1 to 4.8 × 10−3 min−1. In contrast to some other reported systems,9,33–36 the higher phenol oxidation rates observed in brines over rutile suggests that there are alternative reaction pathways offered by the presence of chlorine radicals.To study the chloride effect on reaction intermediates, we monitored the formation and subsequent oxidation of p-benzoquinone (BQ), a primary phenol oxidation product. Over the anatase surface, BQ achieves a maximum concentration of 1 ppm after 60 min and then declines until it is undetectable by HPLC analysis at the end of the reaction (Fig. 2c). BQ production over rutile follows a similar trend but a steady decline in total concentration is observed due to the slower degradation kinetics over this TiO2 polymorph. The brine experiments resulted in a constantly high BQ concentration; an effect attributed to a reduced generation of reactive oxygen species. Notably higher BQ concentrations were measured over rutile than anatase, consistent with the phenol oxidation trend observed in brine solutions.
It is generally accepted that phenol degradation occurs via the initial hydroxylation of the aromatic ring to form benzoquinone, hydroquinone and catechol, followed by aromatic ring opening to produce short-chain aliphatic acids such as maleic acid, formic acid and oxalic acid, which are subsequently mineralized to carbon dioxide and water.37–40Fig. 2d–g shows the transformation of phenol over anatase and rutile comparing experiments performed in water or in brines. For experiments performed in water, the phenol absorption peaks (210, 270 nm) decreased significantly over anatase relative to rutile, in agreement with many studies about the superior photo-activity of anatase. During these experiments the intensities of both p-benzoquinone (246 nm) and hydroquinone (288 nm) increased, with the former intermediate gradually decreasing as the reaction progressed. Several differences were noted when the reactions were performed in brines. First, the phenol peak decreased significantly over rutile indicating an unusually improved phenol degradation compared to experiments performed in aqueous solutions. Secondly, higher p-benzoquinone signal intensities were observed over both TiO2 polymorphs indicating the accumulation of the intermediate as the reaction progressed. The UV-Vis spectra agree with the HPLC results discussed earlier.
In any of these cases, the effect of chlorides can originate from interference with the OH radical generation during UV irradiation. In an aqueous medium, after the photogeneration of charge carriers in the system, hydroxyl radicals can be expected to be generated via two mechanisms: (i) from H2O2 decomposition produced after O2 reacts with an electron in the conduction band, forming solubilised hydroxyl radicals, and, (ii) from concerted H2O deprotonation/oxidation by a hole in the valence band, generating adsorbed hydroxyl radicals (OH˙ads).5 In principle, chlorides could interfere with both mechanisms; however, the latter is considered to be the most relevant pathway for the degradation of organic molecules, and it was selected for further evaluation by DFT methods.5,41 Nevertheless, the many complex processes expected to occur in solution could also have a key role in the rationalisation of the chloride effect. However, they will be explored in a future investigation.
Chloride interference in OH˙ads generation has been proposed to occur by at least two mechanisms, blockage of surface sites and/or hole trapping/scavenging.8 In the following subsection, we describe an investigation of the competitive adsorption of Cl− and OH− anions over two titania model surfaces – a-TiO2 (101) and r-TiO2 (110). Subsequently, the self-trapping ability of Cl− and OH− containing systems is analysed.
3.2 Competitive adsorption of chloride and hydroxyl anions over a-TiO2 (101) and r-TiO2 (110)
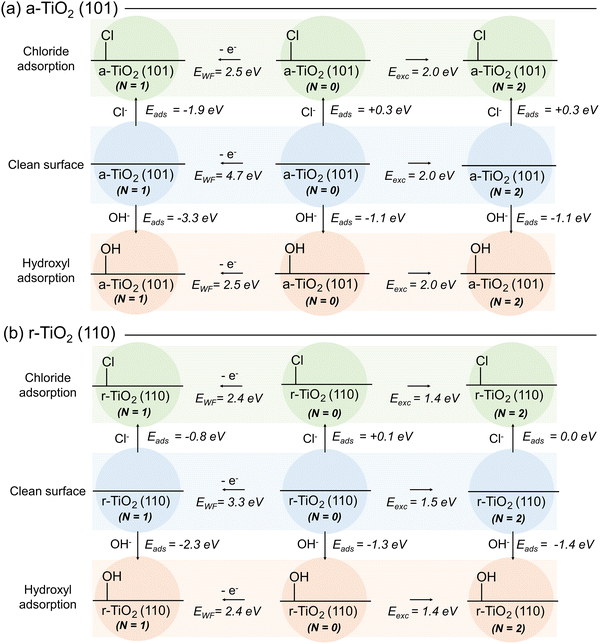
The role of competitive adsorption of chloride and hydroxyl anions over model titania systems was initially investigated by DFT methods. For this, we took into consideration three distinct electronic states of these systems: (i) the ground (singlet) state, identified in Fig. 3 as N = 0, (in which N is the difference between electron with spin up and down in the system); (ii) the excited (triplet, N = 2) state and the doublet state (N = 1) for hole-containing structures. For each of these cases, the adsorption energy (Eads) of chloride and hydroxyl anions was computed considering a-TiO2 (101) and r-TiO2 (110) surfaces (Fig. 3a and b, respectively). Additionally, the excitation energy (Eexc) for the triplet state was calculated as the energy difference between the ground and excited state structure, and the work function (EWF) was computed as the energy difference between ground state and the hole-containing structure (Fig. 3). Although the computed work function and excitation energies may diverge from experimental values, comparisons made at the same theory level can still provide relevant insights into the trends of these systems. Limitations of these methods with regard to the intrinsic aspects of the electronic structure are well known, including the ambiguities about the reference energy in periodic methods, and alternative approaches may be required for computing values directly comparable to experiment.42 However, such limitations should only have a small effect on the comparative analysis in this and the following subsections.For all cases presented in Fig. 3, the adsorption of chlorides is less energetically favourable than the adsorption of hydroxyl anions, regardless of surface or the electronic state of the system. Whilst the calculated adsorption energies of the chloride vary between −1.9 and +0.3 eV, for the hydroxyl anion the values range between −3.3 and −1.1 eV. These results indicate that the putative competitive adsorption of chloride and hydroxyl anion would result in the latter being favoured, at least over the surfaces selected for this investigation. Such a behaviour suggests that inhibition of oxidative photoactivity of titania catalysts by blockage of adsorption sites by chlorides is unlikely to occur. Arguably, competitive adsorption over ground state surface would be better described if the calculated adsorption energies of water molecules and chloride were compared, instead. However, the Eads for water calculated at the same level of theory are similar to that of hydroxyl anions: −1.1 and −1.0 eV over a-TiO2 (101) and r-TiO2 (110), respectively (Fig. S11, ESI†). Once again, these results confirm that adsorption of the hydroxyl anion (or water itself) is still favoured in chloride-containing aqueous solutions. Nevertheless, these results do not eliminate the possibility of small amounts of chloride being adsorbed over the surface of the photocatalyst and acting as hole scavengers, as previously suggested.8,9 To investigate such a possibility, in the next stage of this work, we compare the ability of such systems to self-trap holes and electrons in their structure.
3.3 Charge localisation on clean, hydroxylated, and chlorinated surfaces
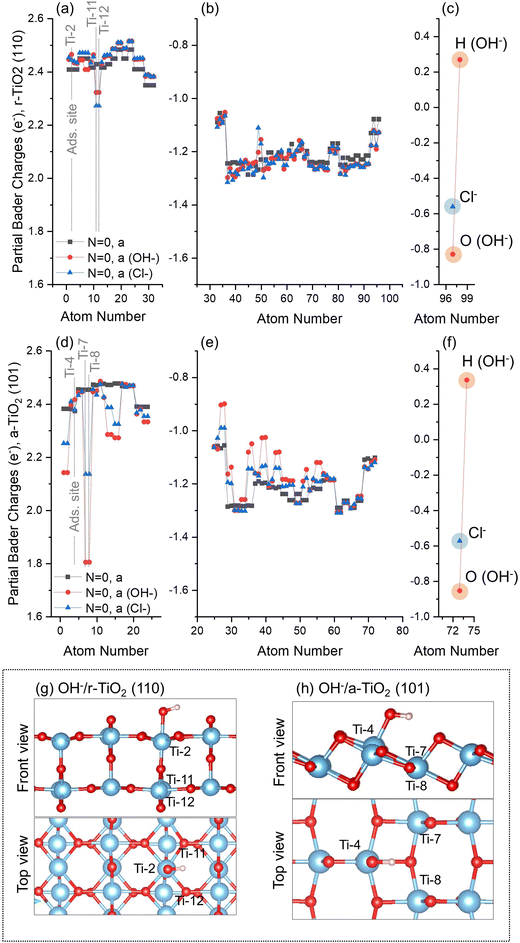
This stage of our investigation was divided into four steps: investigation of atomic charges in a-TiO2 (101) and r-TiO2 (110) surfaces, before and after adsorption of hydroxyl and chloride anions (Subsection 3.3.a); Investigation of atomic charges over surface atoms of clean a-TiO2 (101) and r-TiO2 (110) surfaces when simulating ground state and triplet states, as well as hole-containing and excess electron-containing electronic structures (Subsection 3.3.b); the variation of atomic charges in hydroxyl- and chloride-containing surfaces (Subsections 3.3.c and 3.3.d) when considering triplet states and hole-containing electronic structures; and, finally, the co-adsorption of hydroxyl and chloride anions over a-TiO2 (101) and r-TiO2 (110) surfaces (Subsection 3.3.e).After adsorption of hydroxyl or chloride anions, the charges over surface atoms of r-TiO2 (110) remain roughly unchanged, including the adsorption site Ti-2 (Fig. 4a and g). The only exceptions are the cations Ti-11 and Ti-12 located below the adsorption sites of r-TiO2 (110) (Fig. 4g), which have a slight reduction of their positive charges when compared to the case of the clean surface (Δq ∼ −0.15e). Similar behaviour was observed for a-TiO2 (101) (Fig. 4d); however, in this case the cations with larger charge variation after adsorption of hydroxyl or chloride anions were Ti-7 and Ti-8 (see Fig. 4h), with computed Δq of −0.65e for OH−/a-TiO2 (101) and −0.31e for Cl−/a-TiO2 (101) when compared to the clean surface.
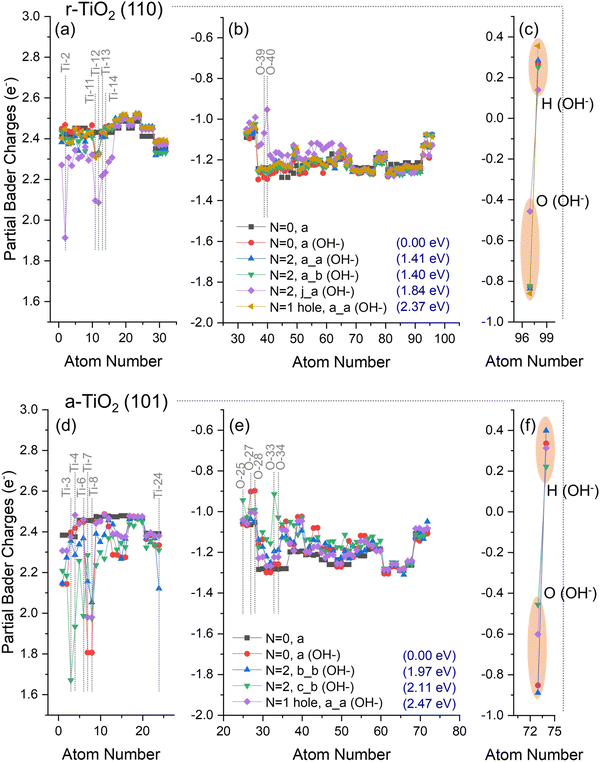
No significant differences are observed between the calculated charges of hydroxyl or chloride on the surfaces of the two polymorphs (Fig. 4c and f). Adsorbed Cl and O(H) atoms on r-TiO2 (110) have partial charges of −0.56e and −0.83e, respectively (Fig. 4c). On a-TiO2 (101) these species have charges of −0.57e and −0.86e for Cl and O(H) atoms, respectively (Fig. 4f). For the adsorbed hydroxyl anion, the total charges of this group are −0.57e for r-TiO2 (110) and −0.52e for a-TiO2 (101).
Overall, only small variations of charge were computed for a-TiO2 (101) and r-TiO2 (110) surfaces after adsorption of chloride or hydroxyl anions. The most significant changes occurred at surface and subsurface Ti sites neighbouring the adsorption site in a-TiO2 (101) and r-TiO2 (110), respectively. Additionally, charges over adsorbates were comparable for both polymorphs, varying between −0.57e and −0.57e for chloride and from −0.57e to −0.52e for hydroxyl anion.
For the hole-containing structure, only one ionic structure was obtained for both cases and no significant charge localisation was observed over the O atoms of TiO2 systems. Similar behaviour was observed regarding hole localisation when considering triplet state systems, which is consistent with previous investigations using DFT hybrid functional (HSE06 and PBE0-TC-LRC) and ab initio embedded cluster approaches,43–46 in which hole trapping is not observed for r-TiO2 (110) systems, except in the presence of adsorbed/dissociated water or hydroxyl species on the surface of the catalyst. Such behaviour is also the case here, as will be further discussed in Subsection 3. 3.c. For a-TiO2 (101), on the other hand, previous investigations have shown this system as capable of hole trapping.43,47–53 In our approach, however, such hole trapping could only be observed when in the presence of hydroxyl species (see Subsection 3.3.c) – similar behaviour to that observed for rutile.
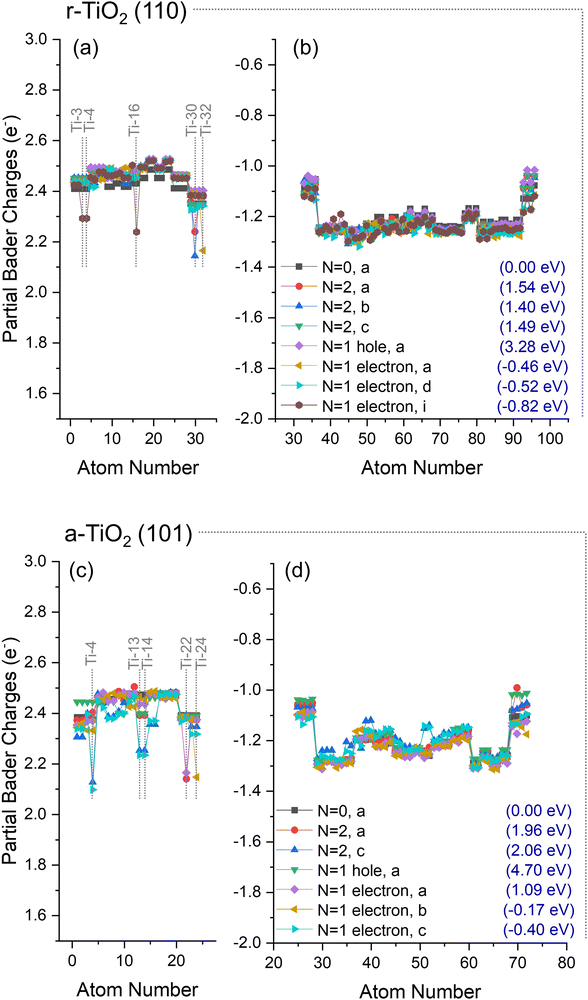
A slight increase of the electron density over Ti cations was observed for rutile at Ti-32 (N = 1 electron, a, Fig. 5a), Ti-30 (N = 2, a and b, Fig. 5a), or simultaneously over Ti-3, Ti-4, and Ti-16 (N = 1 electron, i, Fig. 5a). Ti-32 and Ti-30 are 5-fold titanium ions localised at the bottom of the slab (Fig. 1), whereas Ti-3 and Ti-4 are localised at the surface and directly over Ti-16 (Fig. 1). Such observations are consistent with previous investigations, in which electron trapping in r-TiO2 (110) systems was reported to occur either in the subsurface or surface of the catalyst, with only small energy differences between these possibilities.5,43,47,54–61
For anatase, the most significant electron localisations were observed at Ti-22 (N = 2, a, and, N = 1 electron, a, Fig. 5c), Ti-24 (N = 1 electron, b, Fig. 5c), and simultaneously over Ti-13, Ti-14, and Ti-4 (N = 2, c, and, N = 1 electron, c, Fig. 5c). Ti-22 and Ti-24 are 5-fold titanium ions localised at the bottom of the slab (Fig. 1), whereas Ti-4 is localised at the surface, and sites Ti-13 and Ti-14 are localised in the subsurface directly below Ti-4 (Fig. 1). Previous work has proposed that only in the presence of water molecules, a-TiO2 (101) systems would be able to trap electrons.43,47,49,55 However, as discussed above, at least five distinct structures with partial localisation of electrons in clean anatase were identified.
These results show that no hole localisation is found when clean r-TiO2 (110) and a-TiO2 (101) surfaces are considered. However, distinct electron localisations were obtained for both systems at surface and subsurface Ti sites, not requiring the presence of adsorbates. Next, the changes in the polaronic self-trapping ability of r-TiO2 (110) and a-TiO2 (101) surfaces due to adsorbed anionic species are investigated.
No significant hole or electron localisation was observed for OH−/r-TiO2 (110) systems, except for the triplet state “N = 2, j_a (OH−)” (Fig. 6a–c), which exhibited significant charge variation over both titanium (Ti-11 and Ti-12: Δq = −0.24e; Ti-13 and Ti-14: Δq = −0.21e; and Ti-2: Δq = −0.56e, Fig. 6a) and oxygen ions (O-39: Δq = +0.2e; O-40: Δq = +0.3e, Fig. 6b) when compared to ground state OH−/r-TiO2 (110) system (N = 0, a, Fig. 4a). Ti-11, Ti12, Ti-13, and Ti-14 are located at the subsurface of the slab, whereas Ti-2 is the hydroxyl adsorption site in the “N = 2, j_a (OH−)” system (Fig. 1). Charges over the O(H) atom of the absorbate were roughly the same in most of the alternative structures considered for OH−/r-TiO2 (110), with partial Bader charges circa −0.8e. The only exception was, again, “N = 2, j_a (OH−)” structure (Fig. 6c), which showed a significant reduction of electron density over the O atom of the hydroxyl, with a calculated partial charge of −0.46e. When charges over both O and H atoms of the adsorbate are summed up, the hydroxyl total charge for “N = 2, j_a (OH−)” is −0.3e, which represents a Δq ∼ +0.2e if compared to the ground state OH−/r-TiO2 (110) system (N = 0, a, Fig. 4c). For all the other cases considered here, the total charge of the hydroxyl varied between −0.6e and −0.5e, revealing no significant charge variation when compared to the ground state system.
Similarly, no significant hole localisation was observed over O ions in the surface of OH−/a-TiO2 (101) systems (Fig. 6e). In this case, the largest decrease of electron density over O ions was observed for O-25, O-33, O-34 (Δq = +0.1e, +0.2e, and +0.3e). Moreover, only the triplet states in OH−/a-TiO2 (101) systems led to partial electron localisation over Ti ions (Ti-3: Δq = −0.7e; Ti-24: Δq = −0.2e; Ti-6: Δq = −0.4e; and Ti-4: Δq = −0.5e). Ti-4 and Ti-3 are adsorption sites considered for “N = 2, b_b (OH−)” and N = 2, c_b (OH−), respectively; Ti-6 is next to the adsorption site on the surface; and Ti-24 is located at the bottom of the slab. For Ti-7 and Ti-8 (both neighbouring the adsorption sites on the surface) a charge reduction was computed over these ions when compared to ground state OH−/a-TiO2 (101) system (N = 0 (OH−), a, Fig. 4f): Ti-7, Δq = 0.2–0.5e; Ti-8, Δq = 0.2e. Considering the charge of the O atom of the absorbate, a partial charge of −0.46e was obtained for “N = 2, c_b (OH−)” structure, −0.60e for the hole-containing structure (N = 1 hole, a), and partial Bader charges circa −0.8e over O(H) atom for all other cases. When the total charge over the hydroxyl was calculated, these values were −0.2e for “N = 2, c_b (OH−)”, −0.3e for hole-containing structure (N = 1 hole, a), and −0.5e for all other cases. For these first two cases, this represents a reduction of the calculated charges of Δq ∼ 0.2–0.3e if compared to the ground state system.
Analysis of the energy differences between the structures obtained can give insight into the thermodynamic aspect of photogenerated charges.62 When compared to the ground state energy of OH−/r-TiO2 (110), the energy cost to form the triplet state structures a_a, a_b, and j_b (Fig. 6a–c) was 1.41, 1.40, and 1.84 eV, respectively. The same analysis reveals a higher energy cost for forming the triplet states structures b_b and c_b (1.97 and 2.11 eV, Fig. 6d–f) from ground state OH−/a-TiO2 (101), as would be expected due to the intrinsic differences in band gap energy for these polymorphs.63 The calculated energy cost to form the triplet state structures in the clean surfaces varied between 1.40–1.54 eV and 1.96–2.06 eV for a-TiO2 (101) and r-TiO2 (110), respectively.
Despite these observations, it is well known that formation of adsorbed hydroxyl radicals over rutile has a higher energy barrier than over anatase.64 Geng et al. investigated the photoinduced water dissociation on a-TiO2 (101) surface by laser surface photolysis technique.64 The authors showed that at high water coverages, the water dissociation on r-TiO2 (110) is largely inhibited by the strong hydrogen bonding network between solvent molecules, whereas the same process over a-TiO2 (101) is considerably more efficient due to the much weaker interactions between these molecules.50 The same effect can be expected to occur under the reaction conditions tested here (Subsection 3.1) since anatase presents a higher rate of phenol degradation than rutile.
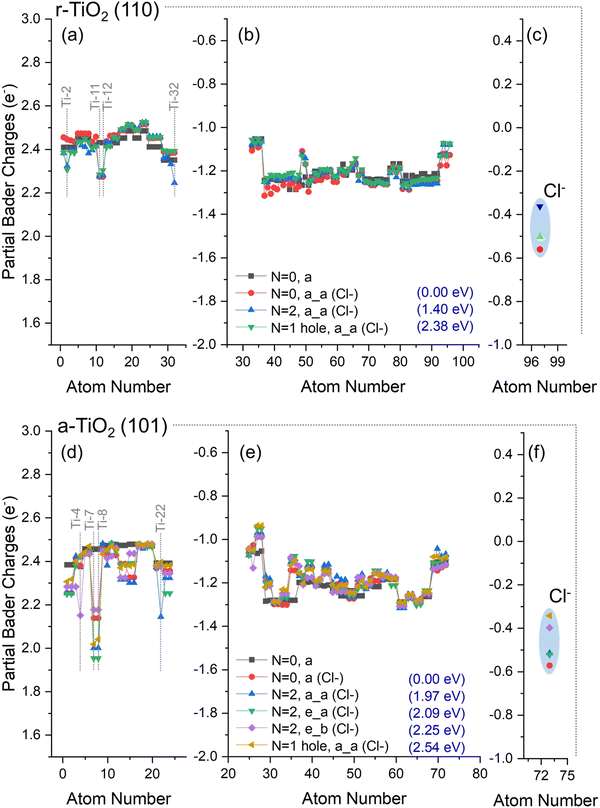
For calculations using Cl−/a-TiO2 (101), three distinct structures for triplet state configurations were obtained (N = 2, a_a (Cl−), N = 2, e_a (Cl−), and N = 2, e_b (Cl−), Fig. 7e); however, the magnitudes of the localised charges over the surface atoms were significantly reduced when compared to the results from OH−/a-TiO2 (101) (Fig. 6e). The hole-containing system (N = 1 hole, a_a (Cl−)) gave a partial Bader charge for the Cl atom of −0.34e, whereas the triplet “e–b” structure had a charge of −0.40e. For all other cases, the charge over Cl atom varied between −0.6e and −0.5e.
These results show that the presence of chloride over r-TiO2 (110) and a-TiO2 (101) reduces the ability of these systems to localise charges in their structure when compared to hydroxylated surfaces, indicating a chloride induced destabilisation of separated charges in titania and, consequently, a reduction in the formation of adsorbed hydroxyl radicals could be expected. In contrast, partial hole localisation over adsorbed chloride is highly favoured, confirming the hole sink character of this species. Although such observations can be used to rationalise the decrease of activity of anatase in brines, it does not explain why rutile activity was enhanced under the same conditions (Subsection 3.1). This point will be further discussed in the following subsection.
For Cl−/r-TiO2 (110), the energy difference between triplet and ground state structures was 1.40 eV (Fig. 7a–c), whereas for Cl−/a-TiO2 (101) this difference ranged between 1.97–2.25 eV (Fig. 7d–f). Interestingly, the energy to form triplet states structures from ground state titania in chloride-containing systems was similar to that of hydroxyl-containing systems for both anatase and rutile (Fig. 6). Consequently, the stability of adsorbed hydroxyl and chloride radicals are predicted to be comparable in both titania phases. This result is also confirmed when co-adsorption of hydroxyl and chloride anions is considered, as will be discussed next.
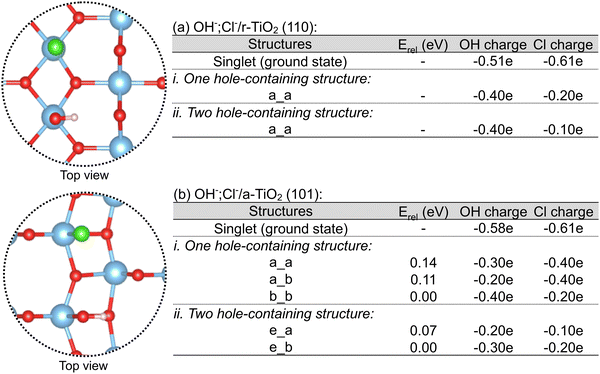
As shown in Fig. 8a, only one distinct structure was obtained for rutile in each of these cases, in which the hole density over chloride was always larger than that of the co-adsorbed hydroxyl. Additionally, when these charges are compared to the singlet (ground state) OH−,Cl−/r-TiO2 (110) system, it is clear that there is a larger reduction of electron density (or increase of hole density) over chloride than over hydroxyl when holes are added to the electronic structure. For anatase, three and two distinct structures were obtained for the calculation considering one and two hole-containing structures (Fig. 8b), respectively. The most stable structures were those in which chloride bears higher hole density than hydroxyl. However, the calculated energy differences are small, ranging between 0.07 and 0.14 eV. Such a small difference suggests that adsorbed hydroxyl and chlorine radicals have comparable stabilities, as proposed in Subsection 3.3 d.
It is worth noting, however, that the formation of adsorbed hydroxyl radicals over titania is not thermodynamically, but kinetically controlled. As proposed by Geng et al.,64 the energy barrier for forming adsorbed hydroxyl radical derives from the energy cost for concerted deprotonation and oxidation of adsorbed water molecules. These authors showed that rutile presents a high energy barrier for adsorbed hydroxyl radical formation, caused by the inhibition of proton transfer due to a strong hydrogen bonding network between solvent molecules, resulting in lower activity.64 The same effect is not observed for a-TiO2 (101), as weaker interactions between solvent molecules are expected.64 This factor is one of a number of possible justifications for the distinct photocatalytic activities of anatase and rutile previously published5,41,64–69 and, as mentioned in Subsection 3.2 c it is in agreement with our experimental results (Subsection 3.1). In contrast, formation of adsorbed chlorine radicals from chloride is a much simpler process and would not be hindered by solvent molecules. The energy cost for forming such a species can be expected to be very similar to that of creating charge separation in the system, as estimated in Fig. 7. In this case Cl˙/r-TiO2 (110) could be formed at a lower energy cost than Cl˙/a-TiO2 (101). In other words, rutile requires a higher energy to form an adsorbed hydroxyl radical than anatase; however, formation of adsorbed chlorine radical from adsorbed chloride could be kinetically favoured. This is a key observation to rationalise the enhancement of the photocatalytic activity of rutile in brines.
It has been reported by different authors that chloride radicals can decompose branched aromatics but would be ineffective for non-branched aromatic decomposition due to the endothermicity of this reaction, which was confirmed by Lewandowski et al. for hydrogen abstraction from benzene.11 Interestingly, the slight endothermicity of hydrogen abstraction from phenol by chlorine radicals is a strong indicator that it could be surmounted due to the exothermicity of the overall degradation process.
All these factors lead us to hypothesise that the enhancement of the photocatalytic activity of rutile in brine could be due to the low concentration of adsorbed hydroxyl radicals formed in standard water and the relative higher surface concentration of adsorbed chlorine radicals in brine. In both polymorphs, adsorbed chlorides will act as hole scavengers (as shown by an increase of hole density over chlorides in contrast to hydroxyl) and by inhibition of charge localisation (as demonstrated by there being only one solution for the hole-containing structure, despite the multiple preliminary lattice distortion considered here). However, formation of adsorbed chlorine radicals over anatase would inhibit the formation of a hydroxyl radical rich surface, whereas for rutile a hydroxyl radical poor surface would be replaced by one with a relative high chlorine radical concentration. Although chlorine is slightly less reactive than hydroxyl radicals, the overall effect would still be an increase in the activity of this photocatalyst. These observations are in agreement with the observed changes in phenol degradation rate observed for these polymorphs in brine, as described in Subsection 3.1. It is worth mentioning, however, that future investigations will be required to confirm the proposed mechanism, in which kinetic effects for the formation of radicals should be taken in consideration, as well as their reactivity towards the target model molecule.
4. Summary and conclusions
In summary, we have investigated the changes in the photocatalytic activity of two titania polymorphs (anatase and rutile) during phenol degradation in standard water and brines. Under such conditions, we observed a significant reduction of the phenol conversion rate over anatase but an enhancement of rutile activity under the same reaction conditions. The origin of such diverging effects was investigated by periodic DFT methods, comparing the possibility of competitive adsorption of hydroxyl and chloride, as well as analysing the polaronic self-trapping ability of these systems.Our calculations of adsorption at a-TiO2 (101) and r-TiO2 (110) surfaces showed that regardless of the model surface or its electronic state, adsorption of the hydroxyl anion is always more favourable than the chloride. Therefore, inhibition of oxidative photoactivity of these systems by blockage of surface sites is unlikely to occur. Nevertheless, the chloride might still be adsorbed in small amounts, resulting in the observed changes of titania activity.
The ability of chlorides to act as hole scavengers was also investigated. According to our approach, a-TiO2 (101) and r-TiO2 (110) systems are unable to localise holes in the absence of adsorbates. On the other hand, weak electron localisation was observed for both surfaces when considering triplet states or excess electrons in these systems. For the hydroxylated surfaces, OH−/r-TiO2 (110) and OH−/a-TiO2 (101), hole localisation was observed mainly on the hydroxyl, but also in the surrounding surface oxygen. Electron localisation was also present in both hydroxylated surfaces when considering triplet states; however, this effect was more significant in anatase than rutile.
Chlorinated surfaces – Cl−/r-TiO2 (110) and Cl−/a-TiO2 (101) – showed a significant reduction in their ability to localise holes and electrons, when compared to the hydroxylated system. For rutile, only delocalised charges were obtained during our calculations, except when the hole-containing electronic structure was considered, in which case the adsorbed chloride showed an increase in its hole density. When anatase was investigated, although distinct structures were found as solutions for the triplet state structure, the localisation of electron density to Ti ions was less significant than that observed for the hydroxylated surface. Additionally, partial hole localisation was also observed at the adsorbed chloride over a-TiO2 (101). Overall, our results indicate that adsorbed chlorides are the preferred hole traps, even in the presence of co-adsorbed hydroxyl. Moreover, this anion can reduce or inhibit the ability of r-TiO2 (110) and a-TiO2 (101) systems to localise polarons in its structure. These results indicate that both mechanisms – hole scavenging and the inhibition of hole localisation – can lead to the observed chloride effect on titania photocatalytic activity. Interestingly, while the formation of adsorbed chlorines on anatase results in a reduction of its activity, the phenol decomposition rate over rutile is proposed to be enhanced due to its higher energy barrier to form adsorbed hydroxyl radicals in standard water and more facile adsorbed chlorines formation in brines. As noted, although chlorines are less reactive than hydroxyl radicals towards phenol degradation, the high concentration of the former in brines and low concentration of the latter in standard water could result in an overall increase of the observed activity of this polymorph.
Overall, our results reveal a decrease of anatase activity in brine, originating from hole scavenging and inhibition of hole localisation cause by adsorbed chlorides. Conversely, rutile exhibited an enhancement of phenol conversion rate in brine, which we hypothesise to occur due to high energy barriers for formation of hydroxyl radicals over rutile with a more facile formation of chlorine radicals, and phenol degradation being initiated by reaction of chlorine radicals. Nevertheless, further investigation of the energy barriers for the formation of radicals and their reactivity towards the target model molecule will be required to confirm the proposed Cl− effect over rutile photoactivity. These findings provide fundamental insights into the photocatalytic properties of titania polymorphs and associated mechanisms for radical formation and oxidative decomposition of organic pollutants.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work used the computing facilities provided by ARCCA at Cardiff University (HPC Wales), GW4 Isambard ‘‘Phase 2’’ – ARM XC50 at Bristol University, and Cirrus UK National Tier-2 HPC Service at EPCC (https://www.cirrus.ac.uk) funded by the University of Edinburgh and EPSRC (EP/P020267/1). Additionally, via our membership of the UK's HEC Materials Chemistry Consortium, which is funded by EPSRC (EP/R029431), this work used the ARCHER2 UK National Supercomputing Service (https://www.archer2.ac.uk) and the UK Materials and Molecular Modelling Hub for computational resources, MMM Hub, which is partially funded by EPSRC (EP/T022213). UK Catalysis Hub is kindly thanked for resources and support provided via our membership of the UK Catalysis Hub Consortium and funded by EPSRC grant: EP/R026939/1, EP/R026815/1, EP/R026645/1, EP/R027129/1. XP spectra in the SI were acquired by EPSRC National Facility for Photoelectron Spectroscopy (HarwellXPS), operated by Cardiff University and UCL under contract number PR16195.References
- S. Kanan, M. A. Moyet, R. B. Arthur and H. H. Patterson, Catal. Rev.: Sci. Eng., 2020, 62, 1–65 CrossRef CAS.
- D. Kanakaraju, B. D. Glass and M. Oelgemöller, J. Environ. Manage., 2018, 219, 189–207 CrossRef CAS PubMed.
- D. J. Lapworth, N. Baran, M. E. Stuart and R. S. Ward, Environ. Pollut., 2012, 163, 287–303 CrossRef CAS PubMed.
- Q. Guo, Z. Ma, C. Zhou, Z. Ren and X. Yang, Chem. Rev., 2019, 119, 11020–11041 CrossRef CAS PubMed.
- Q. Guo, C. Zhou, Z. Ma and X. Yang, Adv. Mater., 2019, 31, 1–26 Search PubMed.
- C. McCullagh, N. Skillen, M. Adams and P. K. J. Robertson, J. Chem. Technol. Biotechnol., 2011, 86, 1002–1017 CrossRef CAS.
- D. Friedmann, C. Mendive and D. Bahnemann, Appl. Catal., B, 2010, 99, 398–406 CrossRef CAS.
- M. Krivec, R. Dillert, D. W. Bahnemann, A. Mehle, J. Štrancar and G. Dražić, Phys. Chem. Chem. Phys., 2014, 16, 14867–14873 RSC.
- E. Bouleghlimat, D. Bethell and P. R. Davies, Chemosphere, 2020, 251, 126469 CrossRef CAS PubMed.
- C. Minero, G. Mariella, V. Maurino, D. Vione and E. Pelizzetti, Langmuir, 2000, 16, 8964–8972 CrossRef CAS.
- M. Lewandowski and D. F. Ollis, J. Catal., 2003, 217, 38–46 CAS.
- W. Zhang, T. An, M. Cui, G. Sheng and J. Fu, J. Chem. Technol. Biotechnol., 2005, 80, 223–229 CrossRef CAS.
- N. Petit, A. Bouzaza, D. Wolbert, P. Petit and J. Dussaud, Catal. Today, 2007, 124, 266–272 CrossRef CAS.
- R. Yuan, T. Chen, E. Fei, J. Lin, Z. Ding, J. Long, Z. Zhang, X. Fu, P. Liu, L. Wu and X. Wang, ACS Catal., 2011, 1, 200–206 CrossRef CAS.
- R. Yuan, S. Fan, H. Zhou, Z. Ding, S. Lin, Z. Li, Z. Zhang, C. Xu, L. Wu, X. Wang and X. Fu, Angew. Chem., Int. Ed., 2013, 52, 1035–1039 CrossRef CAS PubMed.
- G. J. Hutchings, P. R. Davies, S. Pattisson, T. E. Davies, D. J. Morgan and M. W. Dlamini, Catal. Commun., 2022, 169, 106480 CrossRef CAS.
- G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15–50 CrossRef CAS.
- G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 47, 558–561 CrossRef CAS PubMed.
- G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 49, 14251–14269 CrossRef CAS PubMed.
- G. Kresse and J. Furthmüller, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169–11186 CrossRef CAS PubMed.
- B. Hammer, L. B. Hansen and J. K. Nørskov, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 7413–7421 CrossRef.
- S. Grimme, J. Antony, S. Ehrlich and H. Krieg, J. Chem. Phys., 2010, 132, 154104 CrossRef PubMed.
- S. Grimme, J. Comput. Chem., 2006, 27, 1787–1799 CrossRef CAS PubMed.
- D. Mahlberg, S. Sakong, K. Forster-Tonigold and A. Groß, J. Chem. Theory Comput., 2019, 15, 3250–3259 CrossRef CAS PubMed.
- P. E. Blöchl, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953–17979 CrossRef PubMed.
- D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758–1775 CrossRef.
- G. Makov and M. C. Payne, Phys. Rev. B: Condens. Matter Mater. Phys., 1995, 51, 4014–4022 CrossRef CAS PubMed.
- J. Neugebauer and M. Scheffler, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 46, 16067–16080 CrossRef CAS PubMed.
- C. Freysoldt, J. Neugebauer and C. G. Van De Walle, Phys. Rev. Lett., 2009, 102, 1–4 CrossRef PubMed.
- J. Hafner, J. Comput. Chem., 2008, 29, 2044–2078 CrossRef CAS PubMed.
- N. C. Hernández, R. Grau-Crespo, N. H. De Leeuw and J. F. Sanz, Phys. Chem. Chem. Phys., 2009, 11, 5246–5252 RSC.
- M. M. Branda, N. J. Castellani, R. Grau-Crespo, N. H. De Leeuw, N. C. Hernandez, J. F. Sanz, K. M. Neyman and F. Illas, J. Chem. Phys., 2009, 131, 094702 CrossRef PubMed.
- Y. Nosaka and A. Nosaka, ACS Energy Lett., 2016, 1, 356–359 CrossRef CAS.
- B. Tryba, M. Toyoda, A. W. Morawski, R. Nonaka and M. Inagaki, Appl. Catal., B, 2007, 71, 163–168 CrossRef CAS.
- H. Park, H. Kim, G. Moon and W. Choi, Energy Environ. Sci., 2016, 9, 411–433 RSC.
- W. Kim, T. Tachikawa, G. Moon, T. Majima and W. Choi, Angew. Chem., Int. Ed., 2014, 53, 14036–14041 CrossRef CAS PubMed.
- C. Prasse, B. Ford, D. K. Nomura and D. L. Sedlak, Proc. Natl. Acad. Sci. U. S. A., 2018, 115, 2311–2316 CrossRef CAS.
- R. Alnaizy and A. Akgerman, Adv. Environ. Res., 2000, 4, 233–244 CrossRef.
- G. Darabdhara, P. K. Boruah, P. Borthakur, N. Hussain, M. R. Das, T. Ahamad, S. M. Alshehri, V. Malgras, K. C. W. Wu and Y. Yamauchi, Nanoscale, 2016, 8, 8276–8287 RSC.
- R. Su, R. Tiruvalam, Q. He, N. Dimitratos, L. Kesavan, C. Hammond, J. A. Lopez-Sanchez, R. Bechstein, C. J. Kiely, G. J. Hutchings and F. Besenbacher, ACS Nano, 2012, 6, 6284–6292 CrossRef CAS PubMed.
- Y. Nam, J. H. Lim, K. C. Ko and J. Y. Lee, J. Mater. Chem. A, 2019, 7, 13833–13859 RSC.
- D. O. Scanlon, C. W. Dunnill, J. Buckeridge, S. A. Shevlin, A. J. Logsdail, S. M. Woodley, C. R. A. Catlow, M. J. Powell, R. G. Palgrave, I. P. Parkin, G. W. Watson, T. W. Keal, P. Sherwood, A. Walsh and A. A. Sokol, Nat. Mater., 2013, 12, 798–801 CrossRef CAS PubMed.
- A. R. Elmaslmane, M. B. Watkins and K. P. McKenna, J. Chem. Theory Comput., 2018, 14, 3740–3751 CrossRef CAS PubMed.
- D. Wang, H. Wang and P. Hu, Phys. Chem. Chem. Phys., 2015, 17, 1549–1555 RSC.
- S. Kerisit, N. Aaron Deskins, K. M. Rosso and M. Dupais, J. Phys. Chem. C, 2008, 112, 7678–7688 CrossRef CAS.
- V. Shapovalov, E. V. Stefanovich and T. N. Truong, Surf. Sci., 2002, 498, 1–6 CrossRef.
- J. J. Carey and K. P. McKenna, J. Phys. Chem. C, 2018, 122, 27540–27553 CrossRef CAS.
- J. J. Carey, J. A. Quirk and K. P. McKenna, J. Phys. Chem. C, 2021, 125, 12441–12450 CrossRef CAS PubMed.
- C. Di Valentin and A. Selloni, J. Phys. Chem. Lett., 2011, 2, 2223–2228 CrossRef CAS.
- N. M. Dimitrijevic, Z. V. Saponjic, B. M. Rabatic, O. G. Poluektov and T. Rajh, J. Phys. Chem. C, 2007, 111, 14597–14601 CrossRef CAS.
- J. M. Coronado, A. Javier Maira, A. Martínez-Arias, J. C. Conesa and J. Soria, J. Photochem. Photobiol. A Chem., 2002, 150, 213–221 CrossRef CAS.
- T. Berger, M. Sterrer, O. Diwald, E. Knözinger, D. Panayotov, T. L. Thompson and J. T. Yates, J. Phys. Chem. B, 2005, 109, 6061–6068 CrossRef CAS PubMed.
- T. Berger, M. Sterrer, O. Diwald and E. Knözinger, Chem. Phys. Chem., 2005, 6, 2104–2112 CrossRef CAS PubMed.
- S. K. Wallace and K. P. Mckenna, J. Phys. Chem. C, 2015, 119, 1913–1920 CrossRef CAS.
- S. Selcuk and A. Selloni, Nat. Mater., 2016, 15, 1107–1112 CrossRef CAS PubMed.
- C. C. Mercado, F. J. Knorr, J. L. McHale, S. M. Usmani, A. S. Ichimura and L. V. Saraf, J. Phys. Chem. C, 2012, 116, 10796–10804 CrossRef CAS.
- M. Chiesa, M. C. Paganini, S. Livraghi and E. Giamello, Phys. Chem. Chem. Phys., 2013, 15, 9435–9447 RSC.
- P. Zawadzki, J. Phys. Chem. C, 2013, 117, 8647–8651 CrossRef CAS.
- M. Setvin, X. Hao, B. Daniel, J. Pavelec, Z. Novotny, G. S. Parkinson, M. Schmid, G. Kresse, C. Franchini and U. Diebold, Angew. Chem., Int. Ed., 2014, 53, 4714–4716 CrossRef CAS PubMed.
- N. A. Deskins, R. Rousseau and M. Dupuis, J. Phys. Chem. C, 2009, 113, 14583–14586 CrossRef CAS.
- C. J. Calzado, N. C. Hernández and J. F. Sanz, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 1–10 CrossRef.
- G. Di Liberto, S. Tosoni and G. Pacchioni, Phys. Chem. Chem. Phys., 2019, 21, 21497–21505 RSC.
- J. Schneider, M. Matsuoka, M. Takeuchi, J. Zhang, Y. Horiuchi, M. Anpo and D. W. Bahnemann, Chem. Rev., 2014, 114, 9919–9986 CrossRef CAS PubMed.
- Z. Geng, X. Chen, W. Yang, Q. Guo, C. Xu, D. Dai and X. Yang, J. Phys. Chem. C, 2016, 120, 26807–26813 CrossRef CAS.
- S. Tan, H. Feng, Y. Ji, Y. Wang, J. Zhao, A. Zhao, B. Wang, Y. Luo, J. Yang and J. G. Hou, J. Am. Chem. Soc., 2012, 134, 9978–9985 CrossRef CAS PubMed.
- A. Migani and L. Blancafort, J. Am. Chem. Soc., 2017, 139, 11845–11856 CrossRef CAS PubMed.
- Y. Ji, B. Wang and Y. Luo, J. Phys. Chem. C, 2014, 118, 21457–21462 CrossRef CAS.
- A. Y. Ahmed, T. A. Kandiel, T. Oekermann, C. Günnemann and D. Bahnemann, ACS Appl. Energy Mater., 2019, 2, 5308–5318 CrossRef CAS.
- T. Luttrell, S. Halpegamage, J. Tao, A. Kramer, E. Sutter and M. Batzill, Sci. Rep., 2015, 4, 1–8 Search PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d2cp03140j |
| This journal is © the Owner Societies 2023 |