Dynamics of polymer chains confined to a periodic cylinder: molecular dynamics simulation vs. Lifson–Jackson formula

Abstract

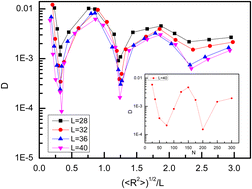
The dynamics of polymer chains confined to a periodic cylinder is explored using molecular dynamics simulation and theoretical analysis. The cylinder is divided into two cavities in one periodicity: one cavity consists of a channel of length L₁ and diameter D₁ and another cavity is a channel of length L₂ and diameter D₂. For L₁ = L₂ = L/2, the diffusion coefficient D of a single confined polymer chain decreases rapidly with increase in periodicities L. For a fixed periodicity with L = L₁ + L₂ = constant, the diffusion coefficient D of a single confined polymer chain shows strong dependence on L₁ (or L₂). Moreover, for a multi-chain system with L₁ = L₂, the diffusion coefficient D shows strong non-monotonic dependence on the chain monomer density ρ, and the confined polymer chains diffuse fastest for ρ = 0.068, in which there are three polymer chains in two periodicities as well as two opposing effects: one is that the excluded volume effect between polymer chains can reduce the free energy barrier, and another is that when the chain monomer density ρ increases further, the entanglement effect increases, which leads to that the diffusion coefficient D decreases as ρ increases. Finally, we found that the diffusion coefficient D has a similar oscillation relationship with the ratio of R/L for different chain lengths N and different periodicity L, and the oscillation amplitude decreases gradually as R/L increases; here R is the mean end-to-end distance of a single confined polymer chain, i.e., 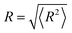. From the view of free energy potential, both the width of the free energy potential well and the height of the free energy potential barrier govern simultaneously the diffusion behavior of confined polymer chains. According to the mean force potential (PMF) based on the weighted histogram analysis method (WHAM), we found that our results agree very well with the theoretical analysis using the Lifson–Jackson formula. Our investigation may help us understand the dynamics of particles in a periodic medium, which is one of the interesting problems in many different fields of science, such as physics, chemistry and biology.