Combined influences of viscous fingering and solvent effect on the distribution of adsorbed solutes in porous media
Abstract
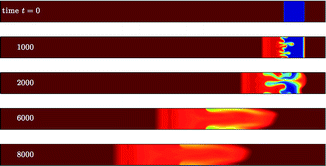
The displacement of two fluids in a porous medium can be affected by a viscous fingering instability (VF) that arises at the interface between the fluids when their viscosities are different. In parallel, one of the fluids may contain solutes that reversibly adsorb on the porous matrix at a rate that depends on the composition of the two-fluid mixture, a so-called solvent strength effect. In some systems encountered for instance in liquid chromatographic columns or in underground flows in environmental applications, both VF and solvent strength effects may combine to influence the spatio-temporal distribution of solutes. Here, a computational investigation of such dynamics is performed. The distribution of the solute in the porous medium is affected by the combined effects of VF and solvent strength. A three component system (displacing fluid, sample solvent and solute) is modeled using Darcy's law for the fluid flow velocity coupled to a convection–diffusion equation for the sample solvent and a mass balance equation for the solute in the mobile and stationary phases. The sample solvent is assumed to have a larger solvent strength than the displacing fluid, in which the retention parameter due to the linear adsorption of the solute depends exponentially on the concentration of the sample solvent. A parametric study of the influences of the factors controlling the VF and solvent strength effects on the displacement velocity of the fronts of solute zone and on its width along the porous medium has been performed by direct numerical simulation of the governing equations. While each of the two effects (VF and solvent strength effects) distorts and significantly increases the broadening of the solute zone, the simulations reveal that, when they are acting in combination, these solute zone perturbations are reduced.

 Please wait while we load your content...
Please wait while we load your content...