Contrasting impact of coordination polyhedra and site symmetry on the electronic energy levels in nine-coordinated Eu(III) and Sm(III) crystals structures determined from single crystal luminescence spectra

Abstract

Lanthanide luminescence is characterised by “forbidden” 4f–4f transitions and a complicated electronic structure. Our understanding of trivalent lanthanide(III) ion luminescence is centered on Eu³⁺ because absorbing and emitting transitions in Eu³⁺ occur from a single electronic energy level. In Sm³⁺ both absorbing and emitting multiplets have a larger multiplicity. A band arising in transitions from the first emitting state multiplet to the ground state multiplet will have nine lines for a Sm³⁺ complex. In this study, high-resolution emission and excitation spectra were used to determine the electronic energy levels for the lowest multiplet and first emitting multiplet in four Sm³⁺ compounds with either tricapped trigonal prismatic TTP or capped square antiprismatic cSAP coordination polyhedra but different site symmetry. This was achieved by the use of Boltzmann distribution population analysis and experimentally determined transition probabilities from emission and excitation spectra. Using this analysis it was possible to show the effect of changing three oxygen atoms with three nitrogen atoms in the donor set for two compounds with the same coordination polyhedra and site symmetry. This work celebrates the 40^th anniversary of Kirby and Richardson's first report of [Eu(ODA)₃]³⁻ luminescence.

---

Introduction

Lanthanide luminescence fascinates as it is characterised by sharp bands in the luminescence spectra and forbidden transitions with long luminescent lifetimes.^2–9 Similar properties are explained by the similar electron configuration of the lanthanide(III) ions,^2,3 and these are exploited in applications including bioassay, bioimaging, and anti-counterfeiting technologies.^5,6,10–16 The applications rely on our ability to understand the complicated molecular and electronic structure of the lanthanide(III) ions.^4,13,17–22 While the electronic splitting caused by the strong spin–orbit coupling, observed as bands in the luminescence spectra, has been extensively studied for the lanthanide(III) ions,^5,6,23,24 the spectral fine structure arising from the crystal field splitting remains a puzzle. The fine structure occurs when individual lines are resolved. Each line is from the transition between the electronic states (m_J levels for integer J, and double degenerate Kramers levels for half-integer J) of the spin–orbit multiplets (^SL_J terms).^8,9,25 The energy of each electronic state is defined by how the crystal field splits the spin–orbit multiplet.

The crystal field is defined by the coordination polyhedra modulated by differences in the type and position of donor atoms and actual positioning—potentially simplified by molecular symmetry. The crystal field defining the symmetry of a lanthanide(III) we determine as the site symmetry. The crystal field splitting we observe is defined by the site symmetry and the nature of the donor atoms.²⁶ In lanthanide luminescence we consider the site symmetry as low, when all states are be non-degenerate. Historically, we have observed this when more lines are resolved in the luminescence spectra. In lanthanide(III) ions, higher site symmetry does not lift the degeneracy of the spin–orbit multiplet, and fewer lines are observed, see below.^27–30

The knowledge of trivalent lanthanide(III) ion photophysics is centered around Eu³⁺.^{13,23,27,31–33} For Eu³⁺ the transition from the ground state (⁷F₀) to the first emitting state (⁵D₀) can be observed as a single line, as both states—indeed both spin–orbit multiplets—are non-degenerate.^34,35 This makes the interpretation of europium luminescence spectra simpler than for other lanthanide(III) ions.^27,36 The non-degenerate ground and emitting states combined with the fact that the Eu³⁺ ion has been comprehensively studied, is the starting point for a broader understanding of other trivalent lanthanide(III) ions.^{13,23,27,31–33}

In contrast to Eu³⁺, Sm³⁺ has ⁶H_5/2 as the low energy spin–orbit multiplet and ⁴G_5/2 as the emitting spin–orbit multiplet.^{5,28–30,36–38} Both multiplets contain three Kramers levels. For Eu³⁺ the site symmetry has been evaluated by counting the number of observed lines in the emission spectrum.^{23,27,31,39,40} For Sm³⁺—and all lanthanide(III) ions with an uneven number of electrons—the maximum splitting of each spin–orbit multiplet is expected. That is (2J + 1)/2 Kramers levels for each spin–orbit multiplet in the half-integer J Kramers lanthanide(III) ions.^9,37 For the high energy band in the Sm³⁺ emission spectrum e.g.⁴G_5/2 → ⁶H_5/2, nine lines should be expected.³⁸ Resolving high numbers of spectral fine structure can be difficult, and Sm³⁺ luminescence remains less explored.^{37,38,41–44} The other bands in the Sm³⁺ luminescence spectrum have twelve, fifteen, and eighteen lines for ⁴G_5/2 → ⁶H_7/2, ⁴G_5/2 → ⁶H_9/2, and ⁴G_5/2 → ⁶H_11/2 respectively.

As the multiplets of Sm³⁺ contain more than one level, there will be a difference in the population between these states. The population of each state is given by the Boltzmann distribution,⁴⁹ which depends on the energy between the states and the temperature. The spectra can be simplified using cryogenic temperatures as fewer states in ⁴G_5/2 and ⁶H_5/2 will be populated. In this case, the number of resolved lines can be counted, but we show that a more robust analysis relying on the Boltzmann distribution can be used to resolve the electronic structure.^{9,37,38,45–48}

To investigate the electronic structure of Sm³⁺, four compounds were prepared and crystallised, see Fig. 1. To be able to contrast and compare, the corresponding Eu³⁺ compounds were also crystallised. By using what is known for Eu³⁺ as a symmetry probe,^{23,27,31,39,40} the Eu³⁺ model system allows us to expand the knowledge on Sm³⁺.

Fig. 1 Polyhedra depicting coordination around the Sm³⁺ ion and molecular structure of the compounds: Na₃[Sm(ODA)₃]·7H₂O, Cs₃[Sm(DPA)₃]·9H₂O, Na[Sm(DOTA)H₂O]·4H₂O, and Na[Sm(EDTA)(H₂O)₃]·5H₂O.

The series is composed of two compounds that have been assigned a Trigonal Tricapped Prismatic TTP coordination polyhedra, Na₃[Ln(ODA)₃]·7H₂O and Cs₃[Ln(DPA)₃]·9H₂O, but with different donor sets. (H₂ODA = 2,2′-oxydiacetic acid/diglycolic acid, H₂DPA = 2,6,dipicolinic acid or pyridine-2,6-dicarboxylic acid). The Na₃[Ln(ODA)₃]·7H₂O compound has a donor set consisting of nine oxygen atoms. The Cs₃[Ln(DPA)₃]·9H₂O compound has a donor set with three nitrogen atoms in the capping layer and six oxygen atoms in the two trigonal layers.^17,18,49,50

The other half of the series is two compounds which have been assigned a capped square-antiprismatic cSAP geometry polyhedra with different donor sets: Na[Ln(DOTA)H₂O]·4H₂O and Na[Ln(EDTA)(H₂O)₃]·5H₂O (H₄DOTA = 1,4,7,10-tetraazacyclododecane-N,N′,N′′,N′′′-tetraacetate, H₄EDTA = (2,2′,2′′,2′′′-(ethane-1,2-diyldinitrilo))tetraacetic acid). The Na[Ln(DOTA)H₂O]·4H₂O compound has a donor set consisting of four nitrogen atoms, four oxygen atoms, and a capping oxygen, and Na[Ln(EDTA)(H₂O)₃]·5H₂O compound has a mixed oxygen/nitrogen donor set without any apparent symmetry. The crystal structures were determined, and luminescence spectra were recorded from single crystals and microcrystalline powders. To quantify the site symmetry of each compound, deviations from ideal coordination polyhedra and site symmetry were computed.⁵¹ While the compounds have geometries close to the idealised coordination polyhedra they have previously been assigned, we show that the site symmetry is lower than expected. None of the four compounds were found to have the previously empirically predicted number of lines in the luminescence spectra.³⁶

Experimental section

Synthesis

All chemicals were used as received without further purification.

0.2 M Eu(CF₃SO₃)₃ stock solution

Eu(CF₃SO₃)₃ (2.40 g, 4.00 mmol) (98% from STREM Chemicals) was used to make a 0.20 M stock solution by dissolving the salt in water making a solution with a volume of 20.0 ± 0.04 ml.

Na₃[Eu(ODA)₃]·8H₂O crystallisation

H₂ODA (0.538 g, 4.01 mmol) (H₂ODA 98% from Sigma Aldrich), H₂ODA = 2,2′-oxydiacetic acid/diglycolic acid was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a volume of 20 ± 0.04 ml with pH = 5 using NaOH (1.0 M). Na₃[Eu(ODA)₃]·9H₂O was made by adding 0.5 ml of the 0.20 M Eu(CF₃SO₃)₃ to a sample vial with 1.5 ml of the 0.20 M H₂ODA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 3 days crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Cs₃[Eu(DPA)₃]·9H₂O crystallisation

H₂DPA (0.669 g, 4.01 mmol) (H₂DPA from Riedel-De Haën Seelze-Hannover), H₂DPA = pyridine-2,6-dicarboxylate was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 7 using NaOH (1.0 M).

Cs₂CO₃ (0.326 g, 1.00 mmol) (Cs₂CO₃ 99% from Sigma Aldrich) was used to make a 0.10 M stock solution by dissolving the salt in the water to make a solution with a volume of 10 ± 0.06 ml.

Cs₃[Eu(DPA)₃]·9H₂O was made by adding 0.5 ml of the 0.20 M Eu(CF₃SO₃)₃ stock to a sample vial with 1.5 ml of the 0.20 M H₂DPA stock and 2.0 ml of the 0.20 M Cs₂CO₃ stock. The sample was filtered through a Q-Max RR syringe filter from Frisinette and transferred to a sample vial. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 3 days crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Na[Eu(DOTA)H₂O]·4H₂O crystallisation

H₄DOTA (0.80918 g, 2.001 mmol), (H₄DOTA from CheMatech) H₄DOTA = 1,4,7,10-tetraazacyclododecane-N,N′,N′′,N′′′-tetraacetate was used to make a 0.10 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 8 using NaOH (1.0 M). Na[Eu(DOTA)H₂O]·9H₂O was made by adding 1.0 ml of the 0.20 M Eu(CF₃SO₃)₃ stock to a sample vial with 2.0 ml of the 0.1 M H₄DOTA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 1 day crystals had formed. The procedure was followed 3 times to ensure reproducibility of the crystal growth.

Na[Eu(EDTA)(H₂O)₃]·5H₂O crystallisation

H₄EDTA (0.26888 g, 0.8045 mmol) (H₄EDTA 98% from STREM Chemicals), H₄EDTA = (2,2′,2′′,2′′′-(ethane-1,2-diyldinitrilo))tetraacetic acid was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 8 using NaOH (1.0 M). Na[Eu(EDTA)(H₂O)₃]·5H₂O was made by adding 1.0 ml of the 0.2 M Eu(CF₃SO₃)₃ stock to a sample vial with 1.0 ml of the 0.20 M H₄EDTA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 1 day crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

0.2 M Sm(CF₃SO₃)₃ stock solution

Sm(CF₃SO₃)₃ (2.39 g, 4.00 mmol) (98% from ABCR) was used to make a 0.20 M stock solution by dissolving the salt in water making a solution with a volume of 20.0 ± 0.04 ml.

Na₃[Sm(ODA)₃]·7H₂O crystallisation

H₂ODA (0.538 g, 4.01 mmol) (H₂ODA 98% from Sigma Aldrich), H₂DPA = 2,2′-dioxyacetic acid was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 5 using NaOH (1.0 M). Na₃[Sm(ODA)₃]·7H₂O was made by adding 0.5 ml of the 0.20 M Sm(CF₃SO₃)₃ to a sample vial with 1.5 ml of the 0.20 M H₂ODA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 3 days crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Cs₃[Sm(DPA)₃]·9H₂O crystallisation

H₂DPA (0.669 g, 4.01 mmol) (H₂DPA from Riedel-De Haën Seelze-Hannover), H₂DPA = pyridine-2,6-dicarboxylate was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 7 using NaOH (1.0 M).

Cs₂CO₃ (0.326 g, 1.00 mmol) (Cs₂CO₃ 99% from Sigma Aldrich) was used to make a 0.10 M stock solution by dissolving the salt in the water to make a solution with a volume of 10 ± 0.06 ml.

Cs₃[Sm(DPA)₃]·9H₂O was made by adding 0.5 ml of the 0.20 M Sm(CF₃SO₃)₃ stock to a sample vial with 1.5 ml of the 0.20 M H₂DPA stock and 2.0 ml of the 0.20 M Cs₂CO₃ stock. The sample was filtered through a Q-Max RR syringe filter from Frisinette and transferred to a sample vial. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 3 days crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Na[Sm(DOTA)H₂O]·4H₂O crystallisation

H₄DOTA (0.80918 g, 2.001 mmol), (H₄DOTA from CheMatech) H₄DOTA = 1,4,7,10-tetraazacyclododecane-N,N′,N′′,N′′′-tetraacetate was used to make a 0.10 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 8 using NaOH (1.0 M). Na[Sm(DOTA)H₂O]·4H₂O was made by adding 1.0 ml of the 0.20 M Sm(CF₃SO₃)₃ stock to a sample vial with 2.0 ml of the 0.1 M H₄DOTA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 1 day crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Na[Sm(EDTA)(H₂O)₃]·5H₂O crystallisation

H₄EDTA (0.26888 g, 0.8045 mmol) (H₄EDTA 98% from STREM Chemicals), H₄EDTA = (2,2′,2′′,2′′′-(ethane-1,2-diyldinitrilo))tetraacetic acid was used to make a 0.20 M stock solution by dissolving the acid in water to make a solution with a with a volume of 20 ± 0.04 ml with pH = 8 using NaOH (1.0 M). Na[Sm(EDTA)(H₂O)₃]·5H₂O was made by adding 1.0 ml of the 0.2 M Sm(CF₃SO₃)₃ stock to a sample vial with 1.0 ml of the 0.20 M H₄EDTA stock. The sample was heated at 80 °C for 1 h. The sample vial was placed in a container with acetone placing a lid on top and left for acetone diffusion. After 1 day crystals had formed. The procedure was repeated 3 times to ensure reproducibility of the crystal growth.

Single crystal X-ray diffraction

Single-crystal X-ray diffraction data was collected using a Bruker D8 Venture diffractometer with a PHOTON 100 CMOS detector, a Mo Kα high brilliance IμS X-ray tube (λ = 0.71073 Å), a multilayer X-ray mirror, and the temperature kept at 100 K using an Oxford Cryo system.

The structures were solved using Olex2⁵² with the ShelXT program⁵³ using intrinsic phasing. Refinement was done using the ShelXL refinement package with least square minimisation. Aromatic hydrogens were added automatically using a riding model all other hydrogens were added manually to residual peaks. All other atoms were refined anisotropically.

Deviation between coordination polyhedra and ideal polyhedra

The deviation between coordination polyhedra and ideal reference polyhedra was calculated using AlingIt⁵¹ after manual rotational optimisation in Mercury.⁵⁴ The geometry in lanthanide(III) complexes was compared to the ideal polyhedra and the values were scaled with the average bond length in the coordinating lanthanide(III) polyhedral. The coordination number (in this case N = 9 for all lanthanide(III) complexes) were used to normalise the results. AlignIt implements eqn (1), after manual rotational optimisation in Mercury, to calculate a deviation value, σ_ideal:⁵¹where P is a point in the ideal reference structure, and Q is a point in the distorted structure. Q₀ is the origin of the distorted model. For two identical structures, the symmetry deviation value will be zero, σ_ideal = 0.⁵¹

Point group symmetry analysis¹

In addition to the geometrical deviation analysis, the fit and structural deviations to symmetry point groups were evaluated.¹ The deviation from ideal point group symmetry was evaluated with eqn (2).where σ_O(Q, Ô_SQ) is the geometry deviation defined in eqn (1) between the coordinate set of structure Q and the same set of coordinates that has been operated with the specific symmetry operation Ô_S. If the structure Q perfectly operates within the symmetry represented by the symmetry operation Ô_S the two structures are identical and σ_O(Q, Ô_SQ) = 0. The sum is over all N symmetry operations in a point group G and σ_sym(Q, G) is the averaged deviation from all symmetry operations in the group.

Crystal powders

For both PXRD and Optical spectroscopy the crystals that precipitated in each sample, were collected by vacuum filtration. The crystals were removed from the filter and crushed to a powder.

Powder X-ray diffraction

The samples for PXRD were filtered crystals crushed to a powder. Data was collected using a Bruker D8 Advance diffractometer using a Cu Kα source (λ = 1.5406 Å) at 293 K with an integration time of 1 s and a resolution of 1500. Samples were measured using a low background silica sample holder.

Spectroscopy for crystals crushed to a powder

After recording PXRD diffractograms, the crystal powders were added to a 5.0 mm diameter NMR quartz tube from Bruker loaded with 2-methyltetrahydrofuran. The resulting suspensions were cooled using liquid nitrogen and measured in a quartz dewar.

Solid-state single crystal spectroscopy

Solid-state single crystal spectroscopy was measured for Eu³⁺ complexes at 293 K. The spectroscopy settings described before were used to measure emission and excitation, respectively. A single crystal was mounted on a crystal mounting loop. The crystal mounting loop was then placed inside the spectrometer. The setup is the same as in our Eu(DPA) paper.⁵⁵

Optical spectroscopy for Eu³⁺ samples

Emission and excitation spectra were measured with a xenon arc lamp as the excitation source on a PTI QuantaMaster8075 from Horiba Scientific. For emission spectroscopy, an excitation wavelength at 394 nm (25 400 cm⁻¹) was used. Emission was detected from 575 nm (17 400 cm⁻¹) to 765 nm (14 000 cm⁻¹). The emission slits were kept at 1.0 nm for the two outermost slits and 5.0 nm for the middle slit for all samples. The excitation slits were all kept at 4.0 nm for the powder in a glass setup, and 8.0 nm when using single crystals. The voltage bias was kept at 3.200 V for the reference detector. The integration time was kept at 1 s with a step size of 0.1 nm. For excitation spectroscopy, an emission wavelength at 614 nm (16 900 cm⁻¹) was used. Excitation was detected from 310 nm (32 300 cm⁻¹) to 585 nm (17 100 cm⁻¹). Emission slits were all kept at 3.0 nm and excitation slits were kept at 1.0 nm for the two outermost slits and 5.0 nm for the middle slit. These settings were used for both the powder in a glass setup and single crystals. The voltage bias was kept at 6.800 V for the reference detector. The integration time was kept at 1 s with a step size of 0.1 nm.

Optical spectroscopy for Sm³⁺ samples

For all samples used for optical spectroscopy and luminescence lifetimes, crystals were crushed to a powder and added to a 5.0 mm diameter NMR quartz cylinders from Bruker with 2-methyl tetrahydrofuran glass.

Emission and excitation on a PTI QuantaMaster8075 from Horiba scientific

Emission and excitation spectra were measured with a xenon arc lamp as the excitation source on a PTI QuantaMaster8075 from Horiba Scientific. For this set-up spectra were recorded at 77 K using liquid nitrogen to cool the samples and measured using a quartz dewar. For emission spectroscopy, an excitation wavelength at 401 nm (24 900 cm⁻¹) was used. Emission was detected from 550 nm (18 200 cm⁻¹) to 760 nm (13 200 cm⁻¹). The emission slits were kept at 1.0 nm for the two outermost slits and 5.0 nm for the middle slit for all samples. The excitation slits were all kept at 8.0 nm. The voltage bias was kept at 3.200 V for the reference detector. The integration time was kept at 1 s with a step size of 0.1 nm. For excitation spectroscopy, an emission wavelength at 598 nm (16 700 cm⁻¹) was used. Excitation was detected from 250 nm (40 000 cm⁻¹) to 590 nm (16 900 cm⁻¹). Emission slits were all kept at 8.0 nm and excitation slits were kept at 1.0 nm for the two outermost slits and 5.0 nm for the middle slit. The voltage bias was kept at 6.800 V for the reference detector. The integration time was kept at 1 s with a step size of 0.1 nm. Emission and excitation spectra measured using this setup can be found in ESI.†

Emission and excitation on a custom build spectrometer with a supercontinuum laser

Emission and excitation spectra were measured using a custom build spectrometer,⁵⁶ equipped with a supercontinuum laser (NKT SuperK Fianium FIU-15) coupled to a tunable band pass filter (NKT LLTF Contrast VIS/SWIR HP8). Samples were measured both at 298 K and cooled to 77 K using liquid nitrogen to cool the samples and measured in a quartz dewar. For emission spectroscopy, an excitation wavelength at 463 nm (21 600 cm⁻¹) was used with 90% laser power and a maximum repetition rate (78 MHz). A 500 nm (20 000 cm⁻¹) long pass filter was used. The center wavelength was set to 640 nm (15 600 cm⁻¹). The integration time was kept at 1 s, with 10 exposures per frame and a 0.54 nm resolution. The slit was kept at 5 μm. The intensity was calibrated using the procedure described in ref. 56 Excitation spectra were measured by scanning the excitation source at 100% laser power from 520 nm (19 200 cm⁻¹) to 580 nm (17 200 cm⁻¹) through a Python code that connects to the tuneable band pass filter (NKT LLTF Contrast VIS/SWIR HP8) with an 880 nm (11 400 cm⁻¹) centre wavelength. A 750 nm (13 300 cm⁻¹) long pass filter was used.

The exposure time per data point was 2 s with 1 exposure per frame, and the step size was set to 0.25 nm. The slit was kept at 15 μm. The setup is described in more detail in ref. 48 and 56.

Luminescence lifetimes for Eu³⁺ samples

The luminescence lifetimes were determined using a PTI QuantaMaster8075 from Horiba Scientific. The excitation wavelength was 394 nm (25 400 cm⁻¹), and the emission wavelength was 598 nm (17 100 cm⁻¹). The slits were all kept at 5 nm for both emission and excitation. The time window was from 200 μs to 3000 μs with an integration time of 28 μs. The luminescence lifetimes were fitted using a mono-exponential decay function using the software Origin Lab Pro's ExpDec1 function. These settings were used for both the powder in a glass setup and single crystals.

Luminescence lifetimes for Sm³⁺ samples

The luminescence lifetimes were determined using a TCSPC FluoTime300 from PicoQuant. The excitation wavelength was 405 nm (24 700 cm⁻¹), and the emission wavelength was 600 nm (16 700 cm⁻¹). The luminescence lifetimes were fitted using a mono-exponential decay function using the PicoQuant EasyTau 2 software.

Deconvoluting the electronic energy levels in the Sm³⁺ compounds using Boltzmann population analysis

To determine which transitions originated from which electronic energy levels (m_J) in the ground state multiplet (⁶H_5/2) and the first emitting state multiplet (⁴G_5/2) in samarium(III), we used our Boltzmann analysis procedure.⁴⁸ Briefly summarised: the calculated populations from the Boltzmann distribution were compared to the observed difference in emission and excitation intensity of each line. The observed relative transition intensities for emission and excitation were extracted from the area of Voigt functions that were fitted to the data using a least square minimiser. The relative emission intensity for a transition from j to i is assumed to be proportional to the population of the state, j, at a given temperature and the relative emission transition probability. The same is assumed for the excitation intensity. A_i→j is the absorption transition probability for from i to j, and B_i←j is the emission transition probability for a from j to i. It is assumed that the ratio of transition probability between two levels i and j is the same for both emission and excitation. For Sm³⁺ specifically, the observed thermal population for the ground state multiplet, ⁶H_5/2, and the emitting state multiplet, ⁴G_5/2, can be expressed as (eqn (3) and (4)):⁴⁸

and

This observed thermal population is compared to the calculated Boltzmann distribution (eqn (5)):⁴⁸

where E_i is the energy of the i states in either the ground state multiplet, ⁶H_5/2, or emitting state multiplet, ⁴G_5/2. If each line in the emission and excitation spectra corresponds to a single electronic transition, the calculated and observed thermal population should be the same.⁴⁸ The observed population is evaluated by the use of a loss function. The model for the electronic energy levels described by the lowest loss value describes the system the best.

This was used to resolve ambiguities in the interpretation of the splitting of the energy levels determined from the spectra. Here the loss function is used to determine which of the different calculated populations agree with the observed population the best.

Results and discussion

To ensure reproducible results, all compounds were prepared in triplicates. For all crystals used in luminescence experiments the unit cell was determined, see the ESI for details.†

Crystal structure and coordination geometry

For all eight Eu³⁺ and Sm³⁺ compounds the single crystal structure was determined. Crystallographic information can be found in the ESI.†

Na₃[Eu(ODA)₃]·9H₂O crystallises in the monoclinic C2/c space group (CCDC deposition number 2291817†) and was found to be isostructural to previously reported compound (CCDC entries DOFXIF 1143446 and DOFIX01 209363).^57,58 The Na₃[Sm(ODA)₃]·7H₂O compound crystallises in the monoclinic Cc space group. Na₃[Sm(ODA)₃]·7H₂O (CCDC deposition number 2291821†) was found to be isostructural to the Na₃[Ln(ODA)₃]·7H₂O compounds, which has previously been reported for Eu³⁺ and Gd³⁺ (CCDC entries DOFXIF 1143446, DOFIX01 209363, and QUMYON 721017).^57–59Fig. 2a illustrates the nine-coordinated oxygen-only [ODA]²⁻ donor set reported with TTP coordination polyhedra.^57,58Fig. 2b shows that for both Na₃[Eu(ODA)₃]·8H₂O and Na₃[Sm(ODA)₃]·7H₂O three [ODA]²⁻ ligands coordinate to the central lanthanide(III) ion, and Fig. 2c illustrates the four Na₃[Sm(ODA)₃]·7H₂O complexes placed within the unit cell.

Fig. 2 (a) Polyhedra depicting coordination around the Sm³⁺ ion in Na₃[Sm(ODA)₃]·7H₂O. (b) Molecular structure of Na₃[Sm(ODA)₃]·7H₂O. (c) The unit cell for the Na₃[Sm(ODA)₃]·7H₂O crystal structure. (d) Polyhedra depicting coordination around the Sm³⁺ ion in Cs₃[Sm(DPA)₃]·9H₂O. (e) Molecular structure of Cs₃[Sm(DPA)₃]·9H₂O. (f) The unit cell for the Cs₃[Sm(DPA)₃]·9H₂O crystal structure. Colour code: Sm = dark blue, N = light blue, C = grey, O = red, and Cs = purple. Hydrogen atoms were omitted for clarity. Thermal ellipsoids are at 50% probability.

The Cs₃[Eu(DPA)₃]·9H₂O crystallises in the orthorhombic C222₁ space group. The crystal grown following the procedure outlined in the Experimental section was found to be twinned, and the structure could not be determined. The Bravais lattice and unit cell agreed with the previously reported structure,⁵⁰ and this crystal was used for single crystal luminescence measurements. However, the structure analysis was done using the crystal structure by Brayshaw et al. in 1995 (CCDC entry YOZRUA 1306070).⁵⁰ The Cs₃[Sm(DPA)₃]·9H₂O crystallises in the orthorhombic C222₁ space group (CCDC deposition number 2291822†). Cs₃[Sm(DPA)₃]·9H₂O was found to be isostructural to the Cs₃[Ln(DPA)₃]·9H₂O compounds, which has previously been reported for Ce³⁺, Eu³⁺, Tb³⁺, and Yb³⁺ (CCDC entries FEMPUL, YOZRUA 1306070 1497223, HOYTEV 622323, and HOYTIZ 623104).^49,50,60Fig. 2d illustrates the [DPA]²⁻ donor set that consists of three nitrogen atoms placed in the capping layer, and six oxygen atoms in the two trigonal layers in the assumed nine coordinated TTP coordination polyhedra. Fig. 2e shows three [DPA]²⁻ ligands coordinate to the central lanthanide(III) ion.^{34,50,61–64}Fig. 2f illustrates the four Cs₃[Sm(DPA)₃]·9H₂O complexes placed within the unit cell.

The Na[Eu(DOTA)H₂O]·4H₂O (CCDC deposition number 2291807†) and Na[Sm(DOTA)H₂O]·4H₂O (CCDC deposition number 2291819†) compounds were found to be isostructural. They crystallise in the trigonal P1̄ space group as previously reported.^65,66 The compounds are reported to have the cSAP coordination polyhedra.^65–67 In the DOTA compounds, the donor set consists of four nitrogen atoms in the lower square antiprism plane, and five oxygen atoms, with four originating from the [DOTA]⁴⁻ in the upper square antiprism layer and a single water molecule in the capping position.

Na[Eu(EDTA)(H₂O)₃]·5H₂O (CCDC deposition number 2291811†) and Na[Sm(EDTA)(H₂O)₃]·5H₂O (CCDC deposition number 2291820†) were found to be isostructural and crystallises in the orthorhombic Fdd2 space group. They are isostructural to previously reported compounds with cSAP coordination polyhedra.^68–73 The lanthanide(III) donor set consists of two nitrogen atoms, with one occupying the capping position and the other being placed in the first square antiprism layer, and seven oxygen atoms, with four originating from [EDTA]⁴⁻ and three from water molecules. As the structures of the eight compounds were determined, the coordination polyhedra, site symmetry, and molecular symmetry of all eight nine-coordinated Ln³⁺ compounds could then be examined.

Evaluation of coordination polyhedra and site symmetry

The site symmetry and donor atoms determine the crystal field splitting of a lanthanide complex. We first consider the coordination polyhedral of the eight compounds using σ_ideal from the AlignIt methodology.⁵¹ The method does not distinguish between atoms, and we thus move on to determine the site symmetry and molecular symmetry using σ_sym.

Table 1 compares experimental coordination polyhedral to the relevant ideal TTP and cSAP polyhedra.^74,75 Additional ideal polyhedra can be found in ESI.† A cursory inspection of Table 1 shows that Na₃[Eu(ODA)₃]·8H₂O and, Cs₃[Eu(DPA)₃]·9H₂O are best described by the TTP structure.^{34,50,61–64} The ODA σ_ideal values are lower than that of the DPA complexes. As the Ln–N and Ln–O bond distances differ by 0.3 Å this is in good agreement with the more symmetric all oxygen donor set.^50,64 The DOTA and EDTA complexes are best described by the cSAP polyhedral, with DOTA closer to an ideal cSAP structure than EDTA.^67,73

Table 1 Geometry deviation values, σ_ideal, from AlignIt. The deviation between the coordination polyhedra and ideal polyhedra considers only the coordination geometry. Values are shown for Eu³⁺ compounds and the Sm³⁺ compounds in parenthesis

---

To analyse the site symmetry of the lanthanide ions, the point group symmetry deviation value or σ_sym was used. This method is derived from the continuous symmetry measure and evaluates how well a point group matches to a structure by computing the distance between the structures, before and after it has been operated by all symmetry operations within the point group. This takes both coordination geometry and the difference in donor atoms into account.¹ Further, the analysis can be done for the coordination polyhedra alone and for the entire compound. Table 2 shows the σ_sym-values calculated for the six most relevant point groups, as described in eqn (2) in the Experimental section.

Table 2 Symmetry deviation values (σ_sym)¹ for the real coordination polyhedra with mixed atom donor sets and the molecular structure for the Eu³⁺ compounds and the Sm³⁺ in parenthesis. Light pink indicates the point group with the highest symmetry that describes the system

---

For the two compounds conventionally assigned to a TTP structure, Na₃[Ln(ODA)₃]·8H₂O and Cs₃[Ln(DPA)₃]·9H₂O, the site symmetry is best described by the D₃ point group. For D_3h, which should be the case for if the compound had an ideal TTP structure, a higher σ_sym-value was found. This indicates that the compounds do not contain a horizontal mirror plane. Interestingly, Cs₃[Ln(DPA)₃]·9H₂O has a perfect σ_sym value against D₃. If we expand the evaluation to include the ligand backbone, we find that the molecular symmetry of [ODA]²⁻ complex is lower than that of the [DPA]²⁻ complex. The [ODA]²⁻ ligands are disordered, while the rigid [DPA]²⁻ ligand maintains high symmetry even at the molecular structure level.

The site symmetry of Na[Ln(DOTA)H₂O]·4H₂O was found to be well-described by the C₄ point group, and it could be argued that the coordination polyhedra has C_4v symmetry. The molecular symmetry is C₄ as the molecular structure does not contain the vertical mirror planes as they are not present in the [DOTA]⁴⁻ ligand backbone. Na[Ln(EDTA)(H₂O)₃]·5H₂O has very little symmetry, and all σ_sym values in Table 2 are high. A single vertical mirror plane is possible, but the site symmetry of the compound does not have this symmetry element and belongs to the C₁ point group. This clearly illustrates the difference in analysing coordination polyhedral and site symmetry.

The analysis shows that the Na₃[Ln(ODA)₃]·8H₂O and, Cs₃[Ln(DPA)₃]·9H₂O compounds have TTP coordination polyhedra with D₃ site symmetry. And that Na[Ln(DOTA)H₂O]·4H₂O has a cSAP coordination polyhedra with C₄ site symmetry, while Na[Ln(EDTA)(H₂O)₃]·5H₂O has a cSAP coordination polyhedra with no (C₁) site symmetry.

Luminescence spectra

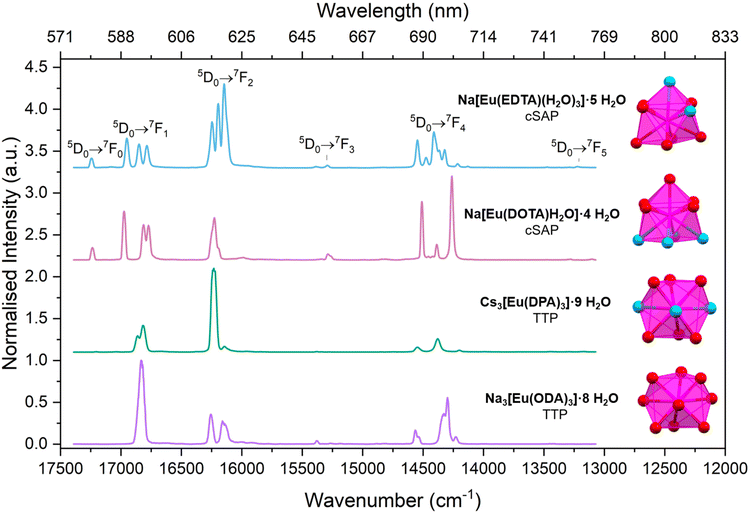
To examine the effect of the crystal field on the electronic structure of the central lanthanide ion, emission and excitation spectra and luminescence lifetimes were recorded for single crystals at 298 K and microcrystalline powders at 77 K.

Eu³⁺ compounds. Table 3 shows the number of lines predicted in each band of the europium emission spectrum as a function of site symmetry.^26,48 The splitting of each ⁷F_J multiplet is related to the site symmetry of the compound,^{13,23,27,31–33} although it must be recognised that most of our analytical framework is empiric in nature.^23,27,39 The Eu³⁺ compounds are useful model systems as both the ground state (⁷F₀) and emitting state (⁵D₀) are non-degenerate,^34,35 but counting of lines in a spectrum is a subjective method.³⁸Table 3 shows that Tanner and Kirby report a different number of lines of the [ODA]²⁻ complex of europium. When we tried to count the lines in the europium emission spectra in Fig. 3, we arrived at three different results. Thus we chose not to include our results in Table 3, but we encourage the reader to count the lines in the Na₃[Eu(ODA)₃]·8H₂O and compare with the literature values. Additional high-resolution spectra are available in the ESI.†

Fig. 3 Normalised single crystal emission at (excitation at 394 nm) for Na₃[Eu(ODA)₃]·8H₂O, Cs₃[Eu(DPA)₃]·9H₂O, Na[Eu(DOTA)H₂O]·4H₂O and Na[Eu(EDTA)(H₂O)₃]·5H₂O at 77 K. Spectra normalised to the highest peak. Polyhedral depicts coordination around the Eu³⁺ ion.

Table 3 The number of bands empirically observed in the ⁵D₀ → ⁷F_J emission for different point group symmetries.²³ The table is from ref. 27. The table also includes the number of observed bands in two different ODA compounds with the same coordination by Kirby et al. and Tanner et al.^27,76

^5D0 → ^7FJ	J = 0	J = 1	J = 2	J = 3	J = 4	J = 5
Nm	580	590	617	650	690	750
C 4v and C 4	1	2	4	5	7	8
D 3h	1	2	3	5	6	7
D 3, C3vand C3	1	2	3	5	6	7
C 2	1	3	5	7	9	11
Kirby et al. ODA ^76	0	1	3	4	4	not reported
Tanner ODA ^27	0	2	2	4	4	not reported

---

The two Eu compounds with cSAP coordination polyhedra, Na[Eu(DOTA)H₂O]·4H₂O and Na[Eu(EDTA)(H₂O)₃]·5H₂O, were analysed first. The symmetry analysis revealed C₄ and C₁ site symmetry for the two compounds, respectively. In the luminescence spectra, shown in Fig. 3, we count one line for the ⁵D₀ → ⁷F₀ band for both of the two cSAP species. This is predicted in Table 3 for these symmetries and also indicates that only one compound is present in the samples.²⁷ For the ⁵D₀ → ⁷F₁ band we count three lines, which is higher than what is predicted for C₄. We are investigating crystalline samples at 77 K with modern equipment, which could explain why the empirical predictions fail.^23,27,39

For the ⁵D₀ → ⁷F₂ band only three lines were resolved for Na[Eu(DOTA)H₂O]·4H₂O and Na[Eu(EDTA)(H₂O)₃]·5H₂O. This is a lower than what is expected cf.Table 3, and that coincidental degeneracy also has a role to play. Another issue, if you rely strongly on counting lines, is that you rely on spectral resolution, which is limited by both equipment and temperature.³⁸

Due to low intensity, the ⁵D₀ → ⁷F₃ and ⁵D₀ → ⁷F₅ bands are seldom included in an analysis. Here, we count two lines in the ⁵D₀ → ⁷F₃ band for both cSAP species, and we count two lines in the ⁵D₀ → ⁷F₅ band for Na[Eu(DOTA)H₂O]·4H₂O and three lines for Na[Eu(EDTA)(H₂O)₃]·5H₂O. The resolution and total splitting of these multiplets make it hard to differentiate between real and coincidental/experimental degeneracy.

Finally, the ⁵D₀ → ⁷F₄ band has five lines in the Na[Eu(DOTA)H₂O]·4H₂O spectra and seven in the Na[Eu(EDTA)(H₂O)₃]·5H₂O spectra. Also, here the number of lines is lower than what is predicted by Table 3, but we note that fewer high-intensity lines are seen in the more symmetric DOTA complex compared to the less symmetric EDTA complex.

Single crystals of Na[Eu(DOTA)H₂O]·4H₂O have previously been analysed by Thomsen et al. and Parker et al.^77–80 Our spectra are consistent with their spectra. The failure of the empirical model and the predictions in Table 3 have been described previously.^{27,29,51,77–81} We often find these descriptors only work for some multiplets in a spectrum, and that the symmetry reported by each multiplet in a spectrum can be different.

Both compounds with TTP coordination polyhedra have D₃ site symmetry. For both Na₃[Eu(ODA)₃]·8H₂O and Cs₃[Eu(DPA)₃]·9H₂O the ⁵D₀ → ⁷F₀ transition was not observed, see Fig. 3. This line is often barely detectable for compounds with TTP coordination polyhedra. The lack of at ⁵D₀ → ⁷F₀ band is often explained by the horizontal mirror plane in the D_3h point group.²⁷ Even though the compounds were found to have D₃ symmetry, σ_sym-values < 2 for the D_3h symmetry could explain that no ⁵D₀ → ⁷F₀ transitions were observed.

Continuing the analysis of the TTP compounds, we move to the ⁵D₀ → ⁷F₁ band. Here, we count two lines for Cs₃[Eu(DPA)₃]·9H₂O indicating a splitting of m_J = ±1 and m_J = 0. In contrast, only one (broad) line was observed in the ⁵D₀ → ⁷F₁ band of Na₃[Eu(ODA)₃]·8H₂O.

If we rely only on counting, this indicates that all the electronic energy levels are degenerate, which is not predicted for any symmetries lower than cubic. The splitting of m_J = ±1 and m_J = 0 should occur in compounds with D₃ symmetry, but the splitting of these levels is not resolved in the data for Na₃[Eu(ODA)₃]·8H₂O.^{9,23,27,39,76} An alternative explanation is that transitions from the m_J = 0 level are forbidden, but there is limited precedence for assuming that single m_J-states are pure states.^9,23,27,39 With the level of theory used in creating Table 3, the ⁷F₁ and ⁷F₂m_J-states can be pure states in D₃ symmetry, and with m_J = 0 forbidden, only degenerate bands for m_J = ±1 and m_J = 2 would be observed. However, the ⁷F₃, ⁷F₄, and ⁷F₅ bands cannot contain a pure m_J = 0 state as it must mix with the m_J = ±3 states. That is the theory predicts m_J mixing when Δm_J ≥ 3.^8,66,80,82 In modern computational chemistry m_J mixing is seen in all multiplets.

For Cs₃[Eu(DPA)₃]·9H₂O two and three lines were counted for the ⁵D₀ → ⁷F₂ and ⁵D₀ → ⁷F₄ band, respectively. This indicates a lower splitting than what is predicted in Table 3 for D₃ point group symmetry.^9,23,27,39 Even though the two compounds, Na₃[Eu(ODA)₃]·8H₂O and Cs₃[Eu(DPA)₃]·9H₂O, are both best described by a TTP coordination polyhedra and both have D₃ site symmetry, the observed splitting in the emission spectra are different. A possible explanation for a larger number of lines in the ⁵D₀ → ⁷F₂ and ⁵D₀ → ⁷F₄ bands in Na₃[Eu(ODA)₃]·8H₂O compared to Cs₃[Eu(DPA)₃]·9H₂O might be ascribed to the lower molecular symmetry for Na₃[Eu(ODA)₃]·8H₂O (C₂).

Overall, we cannot reproduce the prediction in Table 3 for the number of lines we should be able to count in the Eu³⁺ model compounds. To do so we must fit all bands in the spectra and compare them to computational data that remains exceedingly difficult to obtain for Eu³⁺ complexes. Instead we determine the electronic structure of the Sm³⁺ compounds and compare the properties of the two ions.

Sm³⁺ compounds. Before we scrutinise the samarium(III) luminescence spectra in Fig. 4, we consider the differences between Eu³⁺ and Sm³⁺. Samarium(III) is a Kramers ion and both the ⁶H_5/2 low energy spin–orbit multiplet and the ⁴G_5/2 emitting spin–orbit multiplet consists of 3 double degenerate energy levels. At low temperature emission spectra we expect three lines in the ⁶H_5/2 band. At ambient temperature we expect to count nine lines in the ⁴G_5/2 → ⁶H_5/2 band, as all three states in emitting ⁴G_5/2 multiplet are populated at 298 K.

Fig. 4 Normalised emission (excitation at 463 nm) for Na₃[Sm(ODA)₃]·7H₂O, Cs₃[Sm(DPA)₃]·9H₂O, Na[Sm(DOTA)H₂O]·4H₂O and Na[Sm(EDTA)(H₂O)₃]·5H₂O at 77 K and 298 K. Normalised to the maximum in the ⁴G_5/2 → ⁶H_5/2 band. Polyhedra depicts coordination around the Sm³⁺ ion.

For Sm³⁺ we do not expect to see any simplifications due to set symmetry. The predictions shown for Eu³⁺ in Table 3 only predict degenerate electronic energy levels for Sm³⁺ in cubic symmetry.³⁸ Even so, we start by considering the number of lines we can count in Fig. 4 before we move to a more robust method of determining the electronic structure of the ⁴G_5/2 and ⁶H_5/2 states in the four samarium(III) compounds.

A cursory inspection of the spectra in Fig. 4 shows that the two Sm³⁺ compounds with cSAP coordination polyhedra contain fewer lines than the two compounds with TTP coordination polyhedra. A more detailed analysis of the Sm³⁺ compounds with cSAP coordination polyhedra, Na[Sm(DOTA)H₂O]·4H₂O and Na[Sm(EDTA)(H₂O)₃]·5H₂O, shows that this may be due to a larger splitting of the emitting multiplet in these two compounds. For Na[Sm(DOTA)H₂O]·4H₂O we count three lines in the ⁴G_5/2 → ⁶H_5/2 band at 77 K. We see the three Kramers levels of ⁶H_5/2 and only one of the levels in ⁴G_5/2, which indicates a large crystal field splitting in ⁴G_5/2. Counting the number of lines in the ⁴G_5/2 → ⁶H_7/2, ⁴G_5/2 → ⁶H_9/2, and ⁴G_5/2 → ⁶H_11/2 bands become increasingly difficult, but the number of lines is lower than what we would see with more than one emittinglevel in ⁴G_5/2.³⁸ This supports the assumption of a large crystal field splitting in ⁴G_5/2.

For Na[Sm(EDTA)(H₂O)₃]·5H₂O we count four lines in the ⁴G_5/2 → ⁶H_5/2 band. And we count more lines in the ⁴G_5/2 → ⁶H_7/2, ⁴G_5/2 → ⁶H_9/2, and ⁴G_5/2 → ⁶H_11/2 bands than we did for the DOTA complex. This indicates that more levels in ⁴G_5/2 are populated at 77 K, which suggests a smaller crystal field splitting in the ⁴G_5/2 multiplet. As site symmetry should not influence the number of observed lines, we cannot use the difference between C₁ and C₄ to rationalise the observations.

For the two Sm³⁺ compounds with TTP coordination polyhedra, Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O, have the same problem as we did for Eu³⁺. When we consider Fig. 4 we arrive at different numbers of lines when each author counts the number of lines. We all count three lines in the ⁴G_5/2 → ⁶H_5/2 band of Cs₃[Sm(DPA)₃]·9H₂O, while we can count both two and three lines in the ⁴G_5/2 → ⁶H_5/2 band of Na₃[Sm(ODA)₃]·7H₂O. We leave it to the reader to decide how many lines they can resolve in Fig. 4.

For both compounds, we count more lines in the ⁴G_5/2 → ⁶H_7/2 and ⁴G_5/2 → ⁶H_9/2 bands than can be explained with the maximum splitting of ⁶H_7/2 and ⁶H_9/2 alene. The maximum number of levels in the two states are four and five respectively. This indicates that two or more of the states in ⁴G_5/2 are thermally populated to a degree where we easily observe transitions from both levels.^37,38,47 While the spectra at 298 K show how the thermal population of the states changes as well as a thermal broadening of the peaks, see Fig. 4, the complexity of the spectra does not allow us to extract more information without fitting. Nevertheless, the Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O spectra suggest degeneracy in both the ⁶H_5/2 and ⁴G_5/2 multiplet. For a Kramers ion such as Sm³⁺ the crystal field splitting in these multiplets should result in three Kramers levels in all symmetries lower than cubic.^9,37,45–47 This is not what we see.

Deconvoluting the electronic energy levels of Sm³⁺

As counting is not enough, see above, a robust method to deconvolute the spectra and determine electronic energy levels must be used.^{8,9,24,37,38,41–43,45,47,83–85} We can determine the electronic energy levels through a Boltzmann distribution analysis. This requires excitation and emission spectra, see Fig. 5, and can be supported by all the bands in the emission spectra. Further, the method relies on good spectral resolution. The analysis can be made simpler by having the spectra at different temperatures, as the population of the electronic energy levels change with temperature. Here, we record the spectra at 77 K and 298 K, see Fig. 4.

Fig. 5 Normalised emission (excitation at 463 nm) recorded at 77 K normalised to the ⁴G_5/2 → ⁶H_5/2 band. Normalised powdered excitation (emission scan from 520 nm to 580 nm) recorded at 77 K normalised to the ⁴G_5/2 ← ⁶H_5/2 band. The spectra were normalised to the transition from the lowest m_J state in the ground state multiplet (⁶H_5/2) to the lowest m_J state in the first emitting state multiplet (⁴G_5/2).

The analysis uses that the lines in the ⁴G_5/2 → ⁶H_5/2 band are recovered from emission spectra, while the lines in the ⁴G_5/2 ← ⁶H_5/2 band are recovered from the excitation spectra, see Fig. 5. Each band was fitted to a sum of Voigt functions (see ESI for details†), where each non-degenerate line is described by a single Voigt function. In the fit, the Gaussian width applied accounts for the instrumental broadening plus structural fluctuations, and the Lorentzian broadening is associated with the electronic transition. Fixing the Gaussian width was assumed to be a valid assumption for these samples.⁴⁸ These fits are used in a Boltzmann population analysis for Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O from which the electronic energy levels in the two Sm³⁺ compounds were obtained. The fit alone was enough to resolve the electronic structure of Na[Sm(DOTA)H₂O]·4H₂O and Na[Sm(EDTA)(H₂O)₃]·5H₂O.

In the Boltzmann analysis, the best solution was found using a Loss function. This method relies on the intensities being representative of the transition probability, which could be an issue in the case of different emitting species. As the powders were monophasic, and as only one species was seen in the Eu³⁺ compounds, we assume that only one emitting species is present in the samples. Further, in the case of Na₃[Sm(ODA)₃]·7H₂O, the spectra were identical when measured on a single crystal and a single crystal crushed to a powder, see ESI.†

The deconvolution process for Na₃[Sm(ODA)₃]·7H₂O is shown in Fig. 6. Briefly, two lines are observed in the emission spectrum. Thus, we assume that only one of the three levels in ⁴G_5/2 is significantly populated. The observed lines are to the three levels in ⁶H_5/2. Since only two lines are observed, three different electronic structures of the ⁶H_5/2 multiplet are possible: (i) only two energy levels exist in ⁶H_5/2 (0,1). (ii) The two lowest energy levels in ⁶H_5/2 are degenerate ⁶H_5/2 (0,0,1). And (iii) The two highest energy levels in ⁶H_5/2 are degenerate ⁶H_5/2 (0,1,1). To select the correct electronic structure a Loss number is calculated, see Fig. 6. For more details, we refer the reader to the detailed description of the method in ref. 49.

Fig. 6 Top left: normalised powdered emission (excitation at 463 nm) recorded of Na₃[Sm(ODA)₃]·7H₂O at 77 K normalised to the most intense line in ⁴G_5/2 → ⁶H_5/2 band. The spectra has been fitted with two Voigt functions where the splitting between the two lines are the splitting of the levels in ⁶H_5/2. Bottom left: normalised powdered excitation (emission scan from 520 nm to 580 nm) recorded of Na₃[Sm(ODA)₃]·7H₂O at 77 K where the spectra has been fitted with Voigt functions with shared parameters. Right top: from the spectral intensities an observed population (P) is determined from the relative intensities (I) and the relative transition probability (A,B). Bottom right: evaluation of three different models for the electronic structure of the ⁶H_5/2 multiplet (1), (2), and (3). The black and red bars shows the calculated Boltzmann population at 77 K (the red bar) and 298 K (the black bar) are shown along the calculated Loss number (L) for each model is shown.

This analysis computes state populations, compares models, and clearly shows that model (iii) is the correct electronic structure for Na₃[Sm(ODA)₃]·7H₂O. Based on this model all transitions in the excitation spectra can now also be rationalised. Fitting the spectrum with three sets of two Voigt functions reveals the electronic splitting of the ⁴G_5/2 term. The fitting is done with the splitting in ⁶H_5/2 optimised as a global parameter.

The resulting electronic energy levels are shown in Fig. 7. The determined crystal field splitting for Sm³⁺ in the four compounds, confirms the initial qualitative assignments done based on the spectra alone, but we now have a figure of merit and can trust the assignments.

Fig. 7 Dieke diagram for the energy levels from the ground state multiplet (⁶H_5/2) and first emitting state multiplet (⁴G_5/2) for the Sm³⁺ compounds resolved at 77 K. Symmetry deviation values (σ_sym)¹ are shown above. σ_sym-inner indicates the symmetry deviation value for the inner sphere coordination polyhedral and σ_sym-mol indicates the symmetry deviation value for the molecular symmetry.

Fig. 7 shows that a larger total splitting occurs in the two compounds with cSAP coordination polyhedra, Na[Sm(DOTA)H₂O]·4H₂O and Na[Sm(EDTA)(H₂O)₃]·5H₂O, compared to the two compounds with TTP coordination polyhedra and D₃ site symmetry, Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O. The splitting pattern of ⁶H_5/2 Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O are similar, but in ⁴G_5/2 it is reversed.

If ⁶H_5/2 does not have the maximum splitting e.g. show three Kramers level, this would indicate higher symmetry than D₃. The data reported here show that D₃ site symmetry does not lift the degeneracy in the ⁶H_5/2 multiplet. This is in agreement with the observations done for the Eu³⁺ model compounds. We cannot say why the crystal field symmetry has these effects on the Sm³⁺ electronic energy levels, yet.

Conclusions

In this study, four Sm³⁺ compounds were made and compared to four isostructural Eu³⁺ model compounds. Two new compounds were reported: Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O. They were both found to have TTP coordination polyhedra, quantified with the AlignIt method, and they were shown to have D₃ site symmetry by calculating the σ_sym-values. The shape and symmetry were evaluated for all eight compounds, and while the coordination polyhedra alone does not rationalise the data, the site symmetry is determining for the observed electronic structure of Eu³⁺ and Sm³⁺.

To evaluate the electronics structure, the luminescent properties of the compounds were characterised at 77 K and 298 K. By using a Boltzmann population analysis, the electronic energy levels in the Sm³⁺ compounds were determined. Generally, a smaller splitting was observed in the ⁶H_5/2 low energy spin–orbit multiplet for the two compounds with D₃ site symmetry compounds, Na₃[Sm(ODA)₃]·7H₂O and Cs₃[Sm(DPA)₃]·9H₂O, compared to the two compounds with just a single rotational axis, Na[Sm(DOTA)H₂O]·4H₂O and Na[Sm(EDTA)(H₂O)₃] ·5H₂O. We note that the splitting of the ⁶H_5/2 spin–orbit multiplet is largest for Na[Sm(DOTA)H₂O]·4H₂O (C₄), while the splitting of the ⁴G_5/2 emitting spin–orbit multiplet is similar for all four compounds. This takes the first steps towards understanding the crystal field splitting in Sm³⁺ compounds.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

We thank the Villum Foundation, the Carlsberg Foundation, and the University of Copenhagen for financial support.

References

1. V. R. M. Nielsen, B. Le Guennic and T. J. Sørensen, ChemRxiv, 2024, preprint,  DOI:10.26434/chemrxiv-2024-qf84c.
2. V. Balaram, Geosci. Front., 2019, 10, 1285–1303.
3. S. Cotton, Lanthanide and actinide chemistry, John Wiley & Sons, 2013.
4. J.-C. G. Bünzli and S. V. Eliseeva, Chem. Sci., 2013, 4, 1939–1949.
5. W. Carnall, P. Fields and K. Rajnak, J. Chem. Phys., 1968, 49, 4424–4442.
6. W. T. Carnall, P. Fields and B. Wybourne, J. Chem. Phys., 1965, 42, 3797–3806.
7. F. A. Cotton, G. Wilkinson and P. L. Gaus, Basic inorganic chemistry, John Wiley & Sons, 1995.
8. A. de Bettencourt-Dias, Luminescence of lanthanide ions in coordination compounds and nanomaterials, John Wiley & Sons, 2014.
9. S. V. Eliseeva and J.-C. G. Bünzli, Chem. Soc. Rev., 2010, 39, 189–227.
10. C. N. Dansholm, A. K. R. Junker, L. G. Nielsen, N. Kofod, R. Pal and T. J. Sørensen, ChemPlusChem, 2019, 84, 1778–1788.
11. I. Hemmilä, S. Dakubu, V.-M. Mukkala, H. Siitari and T. Lövgren, Anal. Biochem., 1984, 137, 335–343.
12. G. Vereb, E. Jares-Erijman, P. R. Selvin and T. M. Jovin, Biophys. J., 1998, 74, 2210–2222.
13. W. D. Horrocks Jr and D. R. Sudnick, Acc. Chem. Res., 1981, 14, 384–392.
14. X. Zhu, X. Wang, H. Zhang and F. Zhang, Angew. Chem., 2022, 134, e202209378.
15. M. Wang, C. Hu and Q. Su, Biosensors, 2022, 12, 131.
16. R. Arppe and T. J. Sørensen, Nat. Rev. Chem., 2017, 1, 1–13.
17. S. J. Butler, M. Delbianco, L. Lamarque, B. K. McMahon, E. R. Neil, R. Pal, D. Parker, J. W. Walton and J. M. Zwier, Dalton Trans., 2015, 44, 4791–4803.
18. S. J. Butler, L. Lamarque, R. Pal and D. Parker, Chem. Sci., 2014, 5, 1750–1756.
19. P. Caravan, J. J. Ellison, T. J. McMurry and R. B. Lauffer, Chem. Rev., 1999, 99, 2293–2352.
20. J. Luzon and R. Sessoli, Dalton Trans., 2012, 41, 13556–13567.
21. P. Zhang, L. Zhang, C. Wang, S. Xue, S.-Y. Lin and J. Tang, J. Am. Chem. Soc., 2014, 136, 4484–4487.
22. D. Parker, Coord. Chem. Rev., 2000, 205, 109–130.
23. K. Binnemans, Coord. Chem. Rev., 2015, 295, 1–45.
24. G. H. Dieke, Spectra and Energy Levels of Rare Earth Ions in Crystals, John Wiley and Sons, New York, USA, 1968.
25. J. M. Clemente-Juan, E. Coronado and A. Gaita-Ariño, Lanthanides Actinides Mol. Magn., 2015, 12, 27–59.
26. P. R. Nawrocki and T. J. Sørensen, Phys. Chem. Chem. Phys., 2023, 25, 19300–19336.
27. P. A. Tanner, Chem. Soc. Rev., 2013, 42, 5090–5101.
28. T. Cheisson and E. J. Schelter, Science, 2019, 363, 489–493.
29. D. Parker, in Handbook on the Physics and Chemistry of Rare Earths, Elsevier, 2016, vol. 50, pp. 269–299.
30. B. G. Wybourne, J. Alloys Compd., 2004, 380, 96–100.
31. J. C. G. Bünzli and G. O. Pradervand, J. Chem. Phys., 1986, 85, 2489–2497.
32. W. D. Horrocks Jr and D. R. Sudnick, Science, 1979, 206, 1194–1196.
33. G. B. Jean-claude, Inorg. Chim. Acta, 1987, 139, 219–222.
34. P. R. Nawrocki, N. Kofod, M. Juelsholt, K. M. Jensen and T. J. Sørensen, Phys. Chem. Chem. Phys., 2020, 22, 12794–12805.
35. N. Kofod, P. Nawrocki, M. Juelsholt, T. L. Christiansen, K. M. Jensen and T. J. Sørensen, Inorg. Chem., 2020, 59, 10409–10421.
36. C. Görller-Walrand and K. Binnemans, Handb. Phys. Chem. Rare Earths, 1996, 23, 121–283.
37. X. Chen, M. Jensen and G. Liu, J. Phys. Chem. B, 2005, 109, 13991–13999.
38. S. S. Mortensen, M. A. Marciniak Nielsen, P. Nawrocki and T. J. Sørensen, J. Phys. Chem. A, 2022, 126, 8596–8605.
39. K. Binnemans and C. Görller-Walrand, Chem. Phys. Lett., 1995, 245, 75–78.
40. W. Carnall, P. Fields and K. Rajnak, J. Chem. Phys., 1968, 49, 4450–4455.
41. B.-L. An, M.-L. Gong, M.-X. Li and J.-M. Zhang, J. Mol. Struct., 2004, 687, 1–6.
42. S. Mohan, S. Kaur, D. Singh and P. Kaur, Opt. Mater., 2017, 73, 223–233.
43. O. Chukova, S. Nedilko, Z. Moroz and M. Pashkovskyi, J. Lumin., 2003, 102, 498–503.
44. I. Kebaïli and M. Dammak, J. Lumin., 2012, 132, 2092–2097.
45. A. Lupei, C. Tiseanu, C. Gheorghe and F. Voicu, Appl. Phys. B: Lasers Opt., 2012, 108, 909–918.
46. R. Skaudzius, S. Sakirzanovas and A. Kareiva, J. Electron. Mater., 2018, 47, 3951–3956.
47. S. Sakirzanovas, A. Katelnikovas, H. Bettentrup, A. Kareiva and T. Jüstel, J. Lumin., 2011, 131, 1525–1529.
48. V. R. M. Nielsen, P. R. Nawrocki and T. J. Sørensen, J. Phys. Chem. A, 2023, 127(16), 3577–3590.
49. G. Kervern, A. d'Aléo, L. Toupet, O. Maury, L. Emsley and G. Pintacuda, Angew. Chem., Int. Ed., 2009, 48, 3082–3086.
50. P. A. Brayshaw, J.-C. G. Buenzli, P. Froidevaux, J. M. Harrowfield, Y. Kim and A. N. Sobolev, Inorg. Chem., 1995, 34, 2068–2076.
51. M. S. Thomsen, A. S. Anker, L. Kacenauskaite and T. J. Sørensen, Dalton Trans., 2022, 51, 8960–8963.
52. O. V. Dolomanov, L. J. Bourhis, R. J. Gildea, J. A. Howard and H. Puschmann, J. Appl. Crystallogr., 2009, 42, 339–341.
53. G. M. Sheldrick, Acta Crystallogr., Sect. C: Struct. Chem., 2015, 71, 3–8.
54. C. F. Macrae, I. Sovago, S. J. Cottrell, P. T. Galek, P. McCabe, E. Pidcock, M. Platings, G. P. Shields, J. S. Stevens and M. Towler, J. Appl. Crystallogr., 2020, 53, 226–235.
55. S. S. Mortensen and T. J. Sørensen, Eur. J. Inorg. Chem., 2023, e202300159.
56. P. R. Nawrocki, V. R. Nielsen and T. J. Sørensen, Methods Appl. Fluoresc., 2022, 10, 045007.
57. M. Albin, R. R. Whittle and W. D. Horrocks Jr, Inorg. Chem., 1985, 24, 4591–4594.
58. F. A. Cotton and P. Huang, Inorg. Chim. Acta, 2003, 346, 223–226.
59. A. Lennartson and M. Håkansson, CrystEngComm, 2009, 11, 1979–1986.
60. S. M. Elahi and M. V. Rajasekharan, ChemistrySelect, 2016, 1, 6515–6522.
61. D. Zhou, C. Huang, K. Wang and G. Xu, Polyhedron, 1994, 13, 987–991.
62. J.-G. Kim, S.-K. Yoon, Y. Sohn and J.-G. Kang, J. Alloys Compd., 1998, 274, 1–9.
63. M. Autillo, M. A. Islam, J. Jung, J. Pilmé, N. Galland, L. Guerin, P. Moisy, C. Berthon, C. Tamain and H. Bolvin, Phys. Chem. Chem. Phys., 2020, 22, 14293–14308.
64. J. Salaam, I. N’Dala-Louika, C. Balogh, I. Suleimanov, G. Pilet, L. Veyre, C. Camp, C. Thieuleux, F. Riobé and O. Maury, Eur. J. Inorg. Chem., 2022, 2022, e202200412.
65. F. Benetollo, G. Bombieri, S. Aime and M. Botta, Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1999, 55, 353–356.
66. M. Briganti, E. Lucaccini, L. Chelazzi, S. Ciattini, L. Sorace, R. Sessoli, F. Totti and M. Perfetti, J. Am. Chem. Soc., 2021, 143, 8108–8115.
67. S. Aime, A. Barge, F. Benetollo, G. Bombieri, M. Botta and F. Uggeri, Inorg. Chem., 1997, 36, 4287–4289.
68. D. W. Engel, F. Takusagawa and T. F. Koetzle, Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1984, 40, 1687–1693.
69. R. J. Holmberg, I. Korobkov and M. Murugesu, RSC Adv., 2016, 6, 72510–72518.
70. K. Nakamura, T. Kurisaki, H. Wakita and T. Yamaguchi, Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1995, 51, 1559–1563.
71. L. Templeton, D. Templeton, A. Zalkin and H. Ruben, Acta Crystallogr., Sect. B: Struct. Crystallogr. Cryst. Chem., 1982, 38, 2155–2159.
72. J. Wang, P. Hu, B. Liu, L. Q. Zhang, G. X. Han, R. Xu and X. D. Zhang, Russ. J. Inorg. Chem., 2010, 55, 1567–1573.
73. R. Ragul and B. Sivasankar, Synth. React. Inorg., Met.-Org., Nano-Met. Chem., 2013, 43, 382–389.
74. J. J. Thomson, The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 1904, vol. 7, pp. 237–265.
75. M. G. Drew, Coord. Chem. Rev., 1977, 24, 179–275.
76. A. F. Kirby and F. S. Richardson, J. Phys. Chem., 1983, 87, 2557–2563.
77. M. S. Thomsen, H. O. B. Andersen and T. J. Sørensen, Dalton Trans., 2022, 51, 14118–14124.
78. M. S. Thomsen, A. O. Madsen and T. J. Sorensen, Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 2021, 77, 354–364.
79. M. Woods, S. Aime, M. Botta, J. A. K. Howard, J. M. Moloney, M. Navet, D. Parker, M. Port and O. Rousseaux, J. Am. Chem. Soc., 2000, 122, 9781–9792.
80. D. Parker, E. A. Suturina, I. Kuprov and N. F. Chilton, Acc. Chem. Res., 2020, 53, 1520–1534.
81. R. S. Dickins, D. Parker, J. I. Bruce and D. J. Tozer, Dalton Trans., 2003, 1264–1271.
82. J. D. Rinehart and J. R. Long, Chem. Sci., 2011, 2, 2078–2085.
83. W. Carnall, G. Goodman, K. Rajnak and R. Rana, J. Chem. Phys., 1989, 90, 3443–3457.
84. S. K. Gupta, P. S. Ghosh, N. Pathak, A. Arya and V. Natarajan, RSC Adv., 2014, 4, 29202–29215.
85. S. K. Gupta, N. Pathak, S. K. Thulasidas and V. Natarajan, J. Lumin., 2016, 169, 669–673.

---

Footnote

† Electronic supplementary information (ESI) available: The following files are available free of charge. Emission and excitation spectra, time-resolved emission decay profiles, PXRD, and crystallographic information (PDF-file and CIF-files). CCDC 2291807, 2291811, 2291817 and 2291819–2291822. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d4dt00157e

---