Hosting of diamantane alcohols in water and hydrogen-bonded organic solvents: the (non-)classical hydrophobic effect

Abstract

Understanding the forces governing hydrophobically driven inclusion provides a path for aimed utilization of non-polar synthons and provides insights into the related hydration thermodynamics. To shed light on the factors that determine the stability of complexes with large, rigid guests, we studied the temperature and the solvent effect on the hosting of diamantane alcohols with heptameric and octameric cyclodextrins and cucurbiturils. The smaller cyclodextrin was a more efficient binder of the explored guests, while inclusion within γ-CD was observed solely in water. The higher stability of β-CD complexes in this solvent (298 K) was due to the strongly exothermic, entropically opposed inclusion, whereas endothermic hosting of alcohols by γ-CD was observed in all cases except for diamantan-1-ol. The entropically more demanding dehydration of the β-CD cavity hence masks the positive entropy changes accompanying the removal of guest-hydrating water. A strong decrease in Δ_rH°(T) for all studied systems was noticed in water. In the case of cyclodextrins, the phenomenon shifts the driving force from completely or predominantly classical towards non-classical. Conversely, due to the particularly poor structuring of cucurbituril-confined water, the binding remained essentially non-classical over the explored temperature range. Unlike complexation in water, the complexation in formamide and ethylene glycol was entirely enthalpy-driven and weakly temperature-dependent.

---

Introduction

Water still has some surprises left for us despite its familiarity and ubiquity. The small size of the H₂O molecule, its bent shape, and the difference in electronegativity of the constituent atoms enable the assembly of complex three-dimensional, hydrogen-bonded networks that are responsible for unique and remarkable solvation properties.^1–4 These are perhaps most evident in the case of hydrophobic species, whose introduction into water leads to elaborate solvent reorganization.^1,5–8 Two different types of hydrophobic hydration have been recognized over the years, the classical and the non-classical. The former is related to the exothermic, entropically unfavorable dissolution of simple gases and hydrocarbons in water,^9–11 which is typically rationalized by the formation of ordered hydration shells around the non-polar species.¹² The latter concerns the endothermic hydration of non-polar pockets and cavities which contain hydrogen-bond deficient water.^13–16 Thus, hydrogen bond patterns realized in contact with non-polar functionalities proved to be of considerable importance for supramolecular chemistry since they can be a powerful driving force for hydrophobic association. Based on this, it has been established that the association of aliphatic chains (micellization) in water at ambient temperature (≈298 K) usually bears the blueprint of the classical hydrophobic effect (endothermic process),^17–20 whereas the inclusion of hydrophobic species within suitable receptors is predominantly a non-classical hydrophobic effect process type.^21,22

Turning our attention to the host molecules of interest, natural cyclodextrins (CDs) are the most frequently explored receptors for hydrophobic species in water,^21,23,24 while cucurbiturils (CB[n]s) seem to be the most efficient ones.^15,25–30 Compared to cyclodextrins that consist of an equal number of monomers, rigid cucurbiturils contain more water molecules in the inner cavity, which are also less associated.¹⁵ The inclusion of non-polar moieties within CB[n] (n = 5–8) is therefore far more enthalpically favorable, which results in significantly higher complex stabilities.^15,22,28,31 Among the smaller homologues in both receptor classes, the heptamers (CB[7] and β-CD) are finely tuned in terms of both the number of cavity H₂O molecules and their “frustration”, resulting in the most pronounced non-classical hydrophobic effect.¹⁵ Namely, smaller family members contain less associated water molecules but their number is rather low, while the opposite holds true for macrocycles consisting of more than seven monomers.

Another important difference between CB[n]s and CDs should be mentioned. The electron-rich portals of cucurbiturils can serve as efficient cation binding sites,³² so that the presence of positive group(s) on a hydrophobic backbone of the guest leads to higher complex stability^33–37 when compared to neutral analogues.³⁸ The opposite appears to hold true for cyclodextrins, which contain poorer electron-donating hydroxyl groups. Strong hydration of the directly attached charged functionality usually reduces the inclusion depth of hydrophobic subunits within these receptors, thus lowering the guest binding affinity.^21,39–43

Rigid diamantanes^44,45 seem to be almost custom-tailored for constrained, barrel-shaped cavities of CB[n]s. This fact, combined with peculiar hydration of the host cavities and the guest, leads to remarkable complex stability in water. For example, log K ≥ 7 for hosting of neutral diamantane-based guests with both CB[7] and CB[8] has been reported.^38,46 While the exact influence of neutral guest-solubilizing groups on complex stability remains elusive, their structural analogues containing two positively charged solubilizing functionalities (e.g., tetraalkylammonium groups) generate complexes with even higher stability (log K ≥ 16).^33,47 As expected, the smaller CB[7] more readily accommodates apical diamantane derivatives, while the larger CB[8] prefers the medially substituted scaffolds. As far as cyclodextrins are concerned, β-CD is more compatible with adamantane-based guests, whereas γ-CD prefers the larger diamantane derivatives.^38,40,48,49 Somewhat surprisingly, both β- and γ-CD did not bind the permethylated 4,9-diammonium diamantane derivative⁴⁰ for which the octameric, and particularly, the heptameric cucurbiturils exhibited especially high affinity.^33,47

The properties of γ-CD receptor are particularly intriguing with respect to the influence of guest dehydration on the complexation equilibrium. Compared to smaller cyclodextrins and cucurbiturils (especially CB[7]), its binding thermodynamics at 298 K seems to be most consistent with the classical hydrophobic effect (endothermic, entropy-driven inclusion).^14,15,21 Since complexation is accompanied by a reduction in the translational entropy of the system and since the investigations of cyclodextrin-confined water indicate that the included solvent is both enthalpy- and entropy-rich,¹³ the positive Δ_rS° accompanying the inclusion can be ascribed to the release of the guest-hydrating water. Intriguingly, our investigations of the temperature effect on the hosting of adamantane-based guests with β-CD revealed that the driving force of complexation for this bulky guest shifts from predominantly classical towards non-classical as temperature increases (the reversal of Δ_rS° at T ≈ 305 K was observed).⁵⁰ Considering that small positive heat capacities accompany the expulsion of cyclodextrin-confined water⁵¹ as well as the establishment of dispersive host–guest interactions,⁵² the pronounced Δ_rH°(T) decrease must be predominantly due to the gradual disordering of guest hydrating water. However, negative reaction heat capacities for hosting of smaller cyclic and linear aliphatic compounds by both α- and β-CD have been reported from the mid-1990s onwards.^22,53–55 The Δ_rH°(T) dependence was found to be far less pronounced compared to those of adamantane-based guests, and the authors concluded that the negative 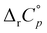is consistent with the temperature dependence of the enthalpies for the transfer of aliphatic chains from water to a hydrocarbon environment (weak contribution of van der Waals interactions to reaction heat capacities was confirmed by Olvera⁵² in 2008). Almost simultaneously with our research, Schönbeck et al. reported similar for the inclusion of adamantane derivatives within β-CD.⁵⁶ To answer the question of whether the inclusion of non-polar species is a strictly water-limited phenomenon, we also explored the inclusion of adamantane-based guests within β-CD in organic hydrogen-bonded and weakly associated solvents (e.g., ethylene glycol, formamide, and N-methylformamide).⁵⁰ Although the inclusion was observed in all solvents whose molecules form a network of hydrogen bonds, the cyclodextrin affinity for the guests was considerably lower compared to water. Furthermore, the binding was entropically unfavorable throughout the explored temperature range (278–338 K) and the temperature dependence of the standard thermodynamic complexation parameters was weak. Considering the larger size of ethylene glycol and formamide molecules, it remains to be answered whether the inclusion of larger hydrophobic moieties could lead to a stronger Δ_rH°(T) dependence, perhaps revealing the classical solvation of the guests in organic solvents. In addition, to our knowledge, the influence of temperature on the hosting of guests larger than adamantane by cyclodextrins and by cucurbiturils in water remained unexplored.

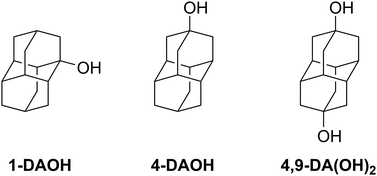
Neutral, diamantane-based compounds are arguably the perfect guests to address these questions for two main reasons. First, we wanted to avoid all contributions to complexation thermodynamics apart from those associated with the hydrophobic effect as much as possible. Second, these guests are structurally highly compatible with heptameric and octameric cyclodextrins and cucurbiturils, which is reasonably expected to result in extensive dehydration of rather large hydrophobic subunits and the receptor cavities. We therefore embarked on studying the temperature and solvent effect on the binding of the rigid diamantane alcohols 1-DAOH, 4-DAOH and 4,9-DA(OH)₂ (Fig. 1) as well as adamantan-1-ol (1-AdOH) with β- and γ-CD in water, formamide and ethylene glycol. Their complexation (apart from 1-DAOH) with CB[7] and CB[8] in aqueous medium was recently reported by Grimm et al. at 298 K.³⁸ It was found that the affinities of both cucurbiturils for diamantane alcohols were rather similar (log K ≈ 7); however, the position of the OH group affected the Δ_rH° and Δ_rS° values considerably. The highest complex stability constant was obtained for 1-AdOH·CB[7], which the authors attributed to the thermodynamically unfavorable dehydration of carbonyl portals concomitant with the inclusion of larger diamantane-based alcohols. It is also noteworthy that the binding thermodynamics did not follow the general Rebek and Mecozzi packing coefficient rule.⁵⁷

Fig. 1 Structures of diamantane alcohols used in this study.

Experimental

The guest molecules 1-DAOH, 4-DAOH and 4,9-DA(OH)₂ were synthesized and purified according to a previously published procedure.⁵⁸1-AdOH (99%), β-CD (HPLC grade, ≥98%), γ-CD (>99.9%), and CB[7] (hydrate) were purchased from Sigma Aldrich and used as received. CB[8] was synthesized according to the previously published procedure.⁵⁹ Apart from water (MiliQ), formamide (FMD, Sigma Aldrich, spectrophotometric grade, ≥99%) and ethylene glycol (EG, Sigma Aldrich, 99%) were used without further purification.

Microcalorimetric investigations

ITC measurements were performed using Microcal VP-ITC (V_cell = 1.45 mL) and PEAQ-ITC (V_cell = 0.205 mL) calorimeters. All titrations were carried out by stepwise addition of the host solution to a solution of the prepared diamantane derivatives. The concentration (c₀ from 3 × 10⁻⁴ to 3 × 10⁻² mol dm⁻³) and volume (V_addition from 10 to 30 μL (VP-ITC), 1.0 to 2.6 μL (PEAQ-ITC)) of the host (titrant) were varied depending on the concentration of the guest (titrand) solution (c₀ from 1 × 10⁻⁵ to 5 × 10⁻⁴ mol dm⁻³). The only exception is the reverse titrations (titration of the host solution with guests) conducted in the case of sparsely soluble CB[8]. For these experiments, the concentration of the host was kept constant (2 × 10⁻⁵ mol dm⁻³), whereas the guest concentrations ranged from 2 × 10⁻⁴ to 4 × 10⁻⁴ mol dm⁻³. Constant stirring was applied, and depending on the temperature and solvent, the time between additions varied from 300 to 600 s (VP-ITC) or 100 to 200 s (PEAQ-ITC). Blank experiments were carried out for each experiment and the heats of the titrant dilution were subtracted from those measured in the titration experiments.

Microcal OriginPro 7.0, Microcal PEAQ-ITC Control Software, and Microcal PEAQ-ITC Analysis Software, all supplied by the manufacturer, were used for data acquisition and processing. The experimental data were fitted to a 1 : 1 (host:guest) complex stoichiometry. All ITC titrations were conducted at least in triplicate and the determined thermodynamic parameters are reported as mean values with the standard errors of the mean provided as a measure of uncertainty. The reactants and the products were neutral species and the concentrations of the titrand and titrant solutions were low in all experiments, so that the values of determined equilibrium constants correspond to K°. Isobaric reaction heat capacities () were determined by weighted linear regression analysis of Δ_rH° vs. T dependence.

The calorimeters were calibrated electrically, and their reliability was assessed according to Briggner and Wadsö.⁶⁰ The thermodynamic complexation parameters for the reaction of 18-crown-6 (18C6, Sigma Aldrich, 99%) with BaCl₂ (Sigma Aldrich, 99.9%) at 298 K, obtained using Microcal VP-ITC (Δ_rH° = −32.19 kJ mol⁻¹; −TΔ_rS° = 10.73 kJ mol⁻¹; Δ_rG° = −21.45 kJ mol⁻¹; K = 5738 mol⁻¹ dm⁻³) and PEAQ-ITC (Δ_rH° = −31.70 kJ mol⁻¹; −TΔ_rS° = 10.17 kJ mol⁻¹; Δ_rG° = −21.47 kJ mol⁻¹; K = 5772 mol⁻¹ dm⁻³), were in excellent agreement with the literature values (Δ_rH° = −31.42 kJ mol⁻¹; −TΔ_rS° = −9.90 kJ mol⁻¹; Δ_rG° = −21.52 kJ mol⁻¹; K = 5900 mol⁻¹ dm⁻³).

NMR investigations

NMR experiments were performed in deuterated water (D₂O) at 25 °C using a Bruker AV600 NMR spectrometer equipped with a 5 mm diameter probe. The chemical shifts (δ/ppm) in the ¹H spectra were referred to as the D₂O signal (¹H: δ = 4.80 ppm). The structure of the complexes was investigated using 2D ROESY NMR spectra with water suppression (Bruker pulse program: roesygpph19.2) with 2k data points in f2 dimension, 256 increments, 32 scans, 500 ms mixing time and a relaxation delay of 2 s.

Computational investigations

In addition to experimental investigations, the packing coefficients for the optimized complex geometries were computed using the Conformer-Rotamer Ensemble Sampling Tool (CREST) based on the GFN methods^61,62 by applying iterative meta-dynamic sampling for noncovalently bound complexes, clusters or aggregates (NCI-iMTD mode). The analytical linearized Poisson–Boltzmann (ALPB) solvation model was used to account for the implicit influence of water in the xTB computations. The packing coefficients were assessed using the approach of Zhao et al.⁶³ and the CD cavity volumes from the work of Szejtli.⁶⁴

Results and discussion

The hosting of diamantane alcohols in water

The temperature and solvent effects on the complexation of diamondoid alcohols with cyclodextrins were studied using ITC (Fig. 2 and Fig. S1–S31, ESI†). This enabled a complete thermodynamic characterization of the binding event, i.e., the determination of all standard thermodynamic complexation parameters. The titration curves were processed by a 1 : 1 (host:guest) binding model, resulting in a very good agreement between experimental and fitted data. In the case of reactions with β-CD as a host (e.g., Fig. 2a), the complex stoichiometry was also evident from a clear break in the titration curve at the equimolar reactant ratio. The microcalorimetrically determined Δ_rX° (X = G, H, S) and log K° values for all studied complexations in water at 298 K are listed in Table 1.

Fig. 2 Microcalorimetric titration of 4,9-DA(OH)₂ (c₀ = 1 × 10⁻⁴ mol dm⁻³) with (a) β-CD (c = 3 × 10⁻³ mol dm⁻³) and (b) γ-CD (c = 5 × 10⁻³ mol dm⁻³) in H₂O at 298 K.

Table 1 Thermodynamic parameters for complexation of diamondoid alcohols with β-CD and γ-CD in H₂O at 298 K^a

Host	Guest	log K	ΔrG°/kJ mol^−1	ΔrH°/kJ mol^−1	−TΔrS°/kJ mol^−1
a Uncertainties of the last digit are given in parentheses as standard errors of the mean (N = 3–5). b From ref. 50.
β-CD	1-AdOH	4.66(1)	−26.38(3)	−21.86(6)	−4.53(5)
	1-DAOH	4.91(1)	−28.02(6)	−37.2(2)	9.2(2)
	4-DAOH	5.54(1)	−31.61(1)	−36.0(1)	4.4(2)
	4,9-DA(OH)2	5.02(1)	−28.67(4)	−34.5(2)	5.8(2)
γ-CD	1-AdOH	2.59(1)	−14.75(6)	11.5(3)	−26.3(3)
	1-DAOH	4.48(1)	−25.57(5)	−10.8(2)	−14.7(2)
	4-DAOH	4.32(5)	−24.6(3)	1.36(9)	−26.0(2)
	4,9-DA(OH)2	3.64(3)	−20.8(2)	2.76(7)	−23.5(1)

---

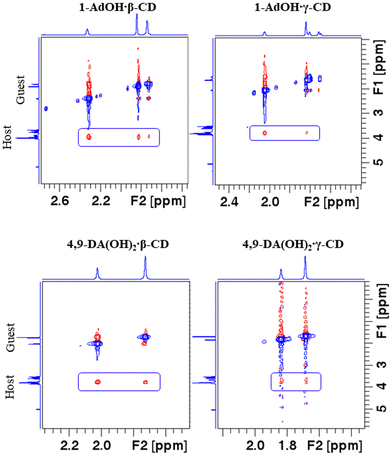
The inclusion within β-CD was enthalpically considerably more favorable in all cases while the opposite holds for the accompanying entropy changes. This resulted in partial enthalpy–entropy compensation, thus lowering the differences in stability constants among the β-CD and γ-CD complexes. Despite this fact, γ-CD was an inferior host for all examined hydrophobic alcohols. As can be seen from the data, β-CD preferred the diamantane-based alcohols over 1-AdOH due to considerably more favorable complexation energetics (Δ(Δ_rH°) ≈ −(12–15) kJ mol⁻¹). Given the fact that the number and position of the hydroxyl group(s) on diamantane alcohols rather weakly influences the complexation thermodynamics with β-CD, the binding is predominantly due to the hydrophobic effect, i.e., the inclusion of non-polar moieties within the receptor cavity. We can therefore ascribe the enthalpically least favorable inclusion of 1-AdOH to the shallower inclusion of the adamantyl moiety in the cyclodextrin and weaker host–guest dispersive interactions. The correlations between the ¹H signals of the guest and host cavities in the ROESY spectra of the mixtures containing 1-AdOH/4,9-DA(OH)₂ and β-/γ-CD (Fig. 3 and Fig. S32, S33, S38, S39, ESI†) are in line with these conclusions. Although the results of computational studies (including the ALPB solvation model to account for the implicit influence of water) of β-CD complexes with diamondoid alcohols most likely somewhat exaggerate the importance of host–guest hydrogen bonds for the studied hosting reaction, the minimized geometries of the products (Fig. 4 and Fig. S40, ESI†) are consistent with the results of spectroscopic and ITC investigations.

Fig. 3 Partial contour plot of the 2D ROESY NMR spectra of mixtures containing guest (1-AdOH or 4,9-DA(OH)₂) and host (β- or γ-CD) at 298 K in D₂O. The 600 MHz ¹H NMR spectrum is shown partially at the top and fully at the left edge.

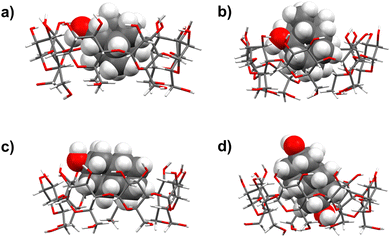Fig. 4 Representations of the minimized geometries of the studied β-CD complexes with diamondoid alcohols (side view): (a) 1-AdOH·β-CD; (b) 1-DAOH·β-CD; (c) 4-DAOH β-CD; and (d) 4,9-DA(OH)₂·β-CD.

Note that the binding of 1-AdOH by β-CD was the only entropically favorable reaction with this host. As far as entropy changes accompanying the inclusion are concerned, the association of host and guest molecules results in a strong decrease in translational entropy. Likewise, the entropy of poorly associated β-CD cavity water was reported to be higher compared to the bulk solvent at 298 K.¹³ Consequently, the positive Δ_rS° for the reaction of 1-AdOH with β-CD seems to be a consequence of the dehydration of the adamantyl subunit. This finding is in line with the exothermic and entropy-opposed (classical) hydration of linear and cyclic hydrocarbons (up to six carbon atoms) at 298 K.¹¹ The negative Δ_rS° for the complexation of diamantane-based guest can be rationalized by their bulkiness. Namely, the ability of water to organize around the guest should decrease with the size of the hydrophobic solute,^1,4,6,8,65 so the dehydration of diamantanols could result in lower entropy changes (hence lower Δ_rS°) compared to 1-AdOH even though their hydration spheres contain more water molecules.

In contrast to reactions with β-CD, the hosting of all guests with γ-CD was accompanied by positive entropy changes, whereby the binding of 1-AdOH, 4-DAOH and 4,9-DA(OH)₂ was endothermic. The higher complexation enthalpies with diamantane-based alcohols can be explained by a stronger association of the water in the γ-CD cavity. According to MD investigations,¹³ each molecule within β-CD realizes an average of 1.9 hydrogen bonds, whereas this number amounts to 2.2 in the case of γ-CD. In comparison, the water bulk is more strongly associated (3.6 hydrogen bonds per water molecule) at 25 °C.^2,15 The poorer organization of the solvent inside β-CD therefore leads to an enthalpically more favorable binding of all guests, even though γ-CD contains more water molecules that can be released (especially in the case of diamantane alcohols).

The position of the OH group(s) on a diamantyl scaffold affected the binding thermodynamics with γ-CD more than in the case of β-CD. With this respect, the exothermic inclusion of 1-DAOH can be explained by the complete burial of its hydrophobic subunit within γ-CD, resulting in the most favorable host–guest interactions, while the OH alcohol group protrudes from the cavity into the bulk. In contrast, the endothermic complexation of 4-DAOH and 4,9-DA(OH)₂, accompanied by rather similar Δ_rH° and Δ_rS° values, suggests a different orientation of the included diamantane subunit. More specifically, the hydrophobic part of the guest is buried within the cavity while the apical hydroxyl guest group(s) are situated at the receptor rim(s). The strong cross-peaks between the equatorial protons of the 4,9-DA(OH)₂ and inward-oriented protons of the host (Fig. 3 and Fig. S39, ESI†) are in line with these conclusions, as are the minimized geometries of the γ-CD complexes with diamantane-based alcohols (Fig. 5d) and Fig. S40h (ESI†).

Fig. 5 Representations of the minimized geometries of the studied γ-CD complexes with diamondoid alcohols (side view): (a) 1-AdOH·γ-CD; (b) 1-DAOH·γ-CD; (c) 4-DAOH·γ-CD; and (d) 4,9-DA(OH)₂·γ-CD.

The enthalpically favorable complexation of 1-DAOH serves as a clear evidence that the dispersion interactions, when optimized (large contact surface area and host–guest size compatibility), stabilize the product considerably. In fact, judging by the enthalpies of condensation of hydrocarbons,⁶⁶ the realized host–guest interactions are expected to be rather favorable for size compatible host–guest systems; however, their contribution to complexation enthalpy is more or less compensated by the endothermic dehydration of the guest. For instance, the enthalpy of vaporization of cyclohexane (the disruption of the corresponding dispersion interactions) is 33.05 kJ mol⁻¹ and its enthalpy of hydration (the dissolution of gas in water) is −33.2 kJ mol⁻¹ at 298 K.¹⁰ The removal of cyclohexane from water and its placement inside a non-polar receptor (roughly equal to −Δ_vapH°−Δ_hydH°) is therefore nearly isoenthalpic. However, compared to the cyclohexyl group, the bulky hydrophobic subunit of 1-DAOH can realize substantially more contacts with the γ-CD cavity atoms which, combined with the enthalpically beneficial removal of frustrated water, leads to its exothermic hosting.

The predominantly or completely entropically driven hosting by γ-CD can be rationalized by the entropically beneficial release of water surrounding the adamantyl and diamantyl subunits, i.e., their classical hydration. As discussed earlier, Priya et al.¹³ reported that dehydration of the cyclodextrin cavity is accompanied by negative entropy changes, whereby the entropic penalty per released water molecule decreases with the size of the macrocycle. Consequently, if present, the classical hydration of guests should be most evident in the case of γ-CD which contains the most bulk-resembling solvent. Our experimental findings support the results of the above-mentioned computational studies, thereby revealing the classical (Δ_hydS° < 0) hydration of adamantyl and diamantyl subunits at 298 K.

The least entropically beneficial, exothermic binding of 1-DAOH indicates that the most favorable host–guest interactions are realized at the expense of entropy. Such a relationship between Δ_rH° and Δ_rS° can be explained by the induced fit of the guest causing the entropically unfavorable conformational changes of the macrocycle and the restricted mobility of the included diamantyl subunit. Moreover, the Δ_rS° for binding of 1-AdOH and apical diamantane alcohols is rather similar even though the inclusion of the latter guests results in more extensive dehydration of the reactants. This finding, combined with solely positive Δ_rS° for hosting of 1-AdOH by smaller β-CD, indeed suggests that the entropic favorability of guest dehydration decreases with their size.

The temperature effect on the binding of 1-AdOH and diamantane alcohols with both cyclodextrins is particularly strong (Fig. 6 and Fig. S5, S10, S15, S20, S27, Tables S1, S2, ESI†). The Δ_rH° for the binding of 1-DAOH with γ-CD decreased by an astonishing 40 kJ mol⁻¹ from 278 K to 338 K ( = −715 J K⁻¹ mol⁻¹). Still, even in this case, the opposing temperature influence on Δ_rH° and Δ_rS° resulted in almost complete entropy-enthalpy compensation (the Δ_rG° decreased only slightly over the studied temperature range). As mentioned in the Introduction, negative 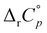values of cyclodextrin inclusion reactions have long been associated with the transfer of non-polar surfaces from the aqueous medium to the hydrocarbon environment (receptor interior).^54,67 Since small and positive values of the reaction heat capacities accompany the realization of host–guest dispersive interactions and dehydration of the cyclodextrin cavity,⁵² a sharp decrease in Δ_rH°(T) (therefore Δ_rS°(T)) is primarily a consequence of the influence of temperature on the organization of the guest-hydrating water.⁵⁰ The reason why the complexation thermodynamics of the studied alcohols is so severely affected by the temperature-induced disordering of the guest hydrating water lies in the bulkiness of the corresponding non-polar subunits (large number of hydrating water molecules). Namely, the so far carried out studies of cyclodextrin complexation properties revealed that the corresponding 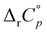values decrease with the size of included hydrophobic moieties.^53–55 However, most of the investigated inclusion reactions involved linear guests and α-CD whose binding was characterized by weaker Δ_rH°(T) and Δ_rS°(T) dependence over the examined (and rather narrow) temperature range.^53–55 In contrast, the complexation of 4-DAOH (Fig. S27, ESI†) and 4,9-DA(OH)₂ (Fig. 6b)) with γ-CD shifts from completely classical (endothermic) in low-temperature towards non-classical (exothermic and accompanied by small Δ_rS°) in high-temperature water (Tables S2 and S3, ESI†). As expected, the 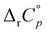values for the inclusion of diamantane-based alcohols within β-CD are higher compared to analogous reactions with γ-CD due to lower extent of guest dehydration in the case of a smaller receptor. On the other hand, the values for binding of 1-AdOH by both receptors were highly similar. This strongly supports the conclusion that the pronounced Δ_rH°(T) dependence is primarily associated with the removal of guest hydrating water⁵⁰ (the contributions arising from dispersive interactions⁵² and the cavity dehydration⁵¹ are rather low). The reaction heat capacities for binding of diamantane-based alcohols with γ-CD are informative with respect to the orientation of the included hydrophobic moiety within the cavity. Namely, the lowest 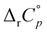for the binding of 1-DAOH with this cyclodextrin indicates the most extensive burial of its non-polar subunit within the receptor. Such findings strongly support the aforementioned conclusions regarding the different orientations of apical and equatorial diamantane-based alcohols within the larger cyclodextrin (Fig. 5).

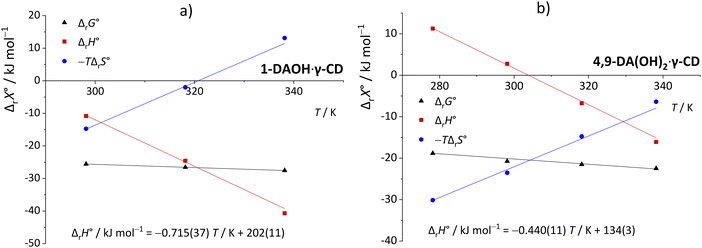Fig. 6 The temperature dependence of standard complexation parameters of (a) 1-DAOH and (b) 4,9-DA(OH)₂ with γ-CD in H₂O.

As stated in the Introduction, Grimm et al.³⁸ recently studied the complexation of 1-AdOH, 4-DAOH and 4,9-DA(OH)₂ with CB[7] and CB[8] at 298 K. The cucurbiturils exhibited larger affinities for all investigated guests compared to β-CD and γ-CD due to far more favorable complexation energetics (Fig. S41–S64 and Tables S3, S4, ESI†). On the other hand, they were entropically inferior hosts to cyclodextrins. This is to be expected considering that the entropic penalty of cyclodextrin cavity dehydration per included solvent molecule decreases as the included water molecules become more associated, i.e., from α- to γ-CD.¹³ Namely, the water within cucurbiturils is particularly hydrogen bond deficient,¹⁵ so its expulsion into the bulk should be more entropically unfavorable compared to the analogous process involving cyclodextrins. The more exothermic but also more entropically unfavorable hosting of all alcohols with smaller CB[7] (containing more frustrated water than CB[8]) is also in line with this rationale. It therefore seems that the differences in the thermodynamic potential of water confined within heptameric and octameric macrocycles are, on a relative scale, preserved in both receptor classes. Specifically, the expulsion of more ordered or bulk-resembling water out of larger family members results in higher complexation entropies compared to smaller receptors. It should also be noted that both cyclodextrins exhibited a larger affinity for diamantane-based alcohols, whereas a considerable preference of CB[7] for 1-AdOH over all other guests was observed (Δ log K° > 4). As in the case of cyclodextrins, the differences in Δ_rH° and Δ_rS° for the complexation of 4-DAOH and 4,9-DA(OH)₂ were low, suggesting a weak involvement of the OH groups in the complexation process.

Generally, the herein determined 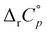values for the inclusion within cucurbiturils were lower than for cyclodextrins consisting of an equal number of subunits, especially in the case of diamantane alcohols. The exceptions were the reaction of 4-DAOH with heptameric receptors for which the associated standard deviations were substantial, and the binding of 1-AdOH with octameric receptors where the low reaction heats with CB[8] prevented the reliable determination of 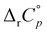. The observed difference in 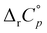values for reactions involving two macrocyclic classes is in accord with the higher compatibility of barrel-shaped cucurbiturils and diamantyl subunits (i.e. more extensive dehydration of the guest in the case of cucurbiturils). Still, in contrast to cyclodextrins, the inclusion remained predominantly enthalpy-driven over the entire temperature range. This clearly indicates that the removal of high-energy water is the main driving force for the inclusion of hydrophobic moieties within cucurbiturils irrespective of temperature, at least for those composed of seven and eight subunits.

Lastly, the obtained values of packing coefficients for complexes with β- and γ-CD range between 43 and 58% (Table S5, ESI†) and are seemingly in line with the 55 ± 9% Rebek and Mecozzi packing coefficient rule.⁵⁷ However, it was previously demonstrated that packing coefficients, and consequently, their indirect measure of host–guest interactions, are not always a straightforward way to assess high-affinity binding (e.g., CB[n] complexes with diamondoid alcohols as guests).³⁸ When comparing the measured binding parameters and the calculated packing coefficients for the analogous β- and γ-CD complexes studied here, one does not find a straightforward correlation between them, leading us to again conclude that the most extensive dehydration coupled with sufficiently strong host–guest interactions leads to the most stable complexes.

The hosting of diamantanes in formamide and ethylene glycol

The binding of hydrophobic alcohols with γ-CD in formamide (FMD) and ethylene glycol (EG) was not observed calorimetrically; however, the titrations of guests with β-CD (Fig. S65–S71, S73–S75, S77–S82, S84–S86, ESI†) over the 278–338 K temperature range resulted in measurable enthalpy changes in both explored solvents. The experimental data could be satisfactorily processed according to a 1 : 1 binding model, which yielded the complex stability constants and the Δ_rH° and Δ_rS° values (Tables S6 and S7, ESI†). The stabilities of complexes with β-CD in studied solvents and the standard thermodynamic reaction quantities at 298 K are compared in Fig. 7. Water was obviously the most efficient complexation medium, followed by FMD and EG. The complex stability in formamide decreased as follows: 4-DAOH > 4,9-DA(OH)₂ > 1-AdOH > 1-DAOH, while in ethylene glycol it amounted to: 4-DAOH > 4,9-DA(OH)₂ > 1-DAOH. The heptameric cyclodextrin exhibited a rather similar affinity for studied guests in both solvents. In agreement with our previous studies,⁵⁰ the hosting was accompanied by far lower (negative) entropy changes but was more enthalpically favorable compared to water. The only exception is the binding of 1-DAOH which was more exothermic in aqueous medium than in formamide. The highly energetically beneficial and entropy-opposed inclusion of guests in organic solvents can be explained by the chaotropic behavior of both the cavity and the explored guests. Namely, formamide and ethylene glycol form stronger hydrogen bonds than water (Δ_vapH(H₂O, 298 K) = 43.99 kJ mol⁻¹,⁶⁸ Δ_vapH(FMD, 298 K) = 62.2 kJ mol⁻¹,⁶⁹ Δ_vapH(EG, 298 K) = 65.6 kJ mol^−1 70), whereas their ability to organize around the solutes is far lower compared to water. For instance, the dissolution of aliphatic hydrocarbons (n-hexane, cyclohexane and n-heptane) in formamide at 298 K is considerably more endothermic than in water.^71,72 Still, their introduction into FMD is entropically favorable,^71,72 whereas the opposite holds true for water, leading to their overall better solvation in formamide. In contrast to water, this leaves the guest- and the cavity-solvating molecules hydrogen-bond deficient which, combined with the decrease in translational entropy, results in negative Δ_rH° and Δ_rS° at 298 K.

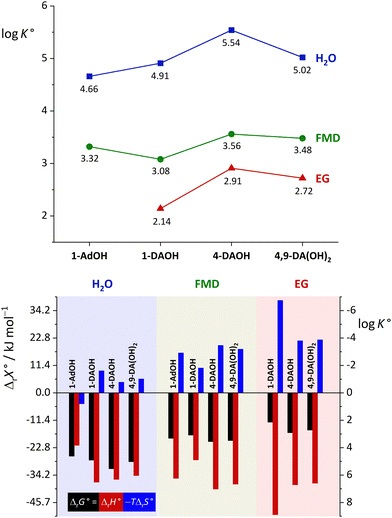Fig. 7 Thermodynamic parameters for complexation of 1-AdOH and diamantane alcohols with β-CD in water (H₂O), formamide (FMD), and ethylene glycol (EG) at 298 K.

Unlike in water, the Δ_rH°(T) (hence Δ_rS°(T)) dependence in FMD and EG was rather weak. Such behavior was also reported for the binding of adamantane-based guest molecules with β-CD.⁵⁰ Apparently, the decrease in temperature cannot induce the energetically favorable organization of these solvents, even in the case of larger diamondoids, which in principle could be wrapped in organized shells more readily than a smaller adamantane. This is clear evidence that the small size and shape of water molecules results in truly unique solvation properties. Consequently, the solvophobic effect in H₂O bears a different thermodynamic signature than in other strongly hydrogen bonded solvents, resulting in considerably larger complex stabilities in aqueous media.

Finally, a few words about the classical hydration of the guest non-polar moieties. Although the results of thermodynamic investigations undoubtedly reveal exothermic, entropically unfavorable hydration of lower hydrocarbons and simple, non-polar gases in ambient and sub-ambient temperature water, this phenomenon is still the subject of many investigations. According to some researchers, thermodynamic,^1,73–75 spectroscopic^76–81 as well as many computational results^1,79,82–84 indicate that water molecules form more ordered tetrahedral networks around spherical and linear hydrophobic functionalities in low-temperature water. In agreement with the particularly strong temperature dependence of the enthalpy and entropy of hydration (Δ_hydC_p > 0),^9,85 the probability of their formation diminishes with temperature, eventually leading to complete disordering of hydration water. However, it has been pointed out that the negative hydration entropies could be primarily due to the excluded volume effect (the reduction in translational degrees of freedom of the solvent due to the introduction of non-polar moieties), enhanced by the small size of a water molecule.^86–88 Recent investigations indicate that this may indeed be so, further revealing that the enhanced hydrogen bonding of the hydrating water occurs in the secondary rather than the primary hydration sphere of the lipophilic functionalities.^8,89,90 Conversely, in quite a few investigations very weak or no ordering of the hydrating water was observed, either experimentally^89–91 or computationally.^83,92 Perhaps the reason lies in the literal interpretation of the classical iceberg model. A relatively large number of water molecules are involved in the hydration of bulky hydrophobic solutes, e.g., approx. 20 in the case of adamantane. Given the large enthalpy of vaporization of water, Δ_vapH(H₂O, 298 K) = 43.99 kJ mol⁻¹,⁶⁸ and that water forms on an average of 3.62 per molecule at this temperature,¹⁵ it is sufficient that each hydrating molecule forms on average 0.1 hydrogen bonds more compared to bulk to result in a remarkable increase in the enthalpy of complexation by 24 kJ mol⁻¹. In other words, the more pronounced stratification of guest-hydrating water compared to the bulk must be rather subtle and, most likely, unobservable using most experimental methods. In line with that, a particularly strong temperature dependence of cyclodextrin binding thermodynamics can provide valuable information regarding the organization of water around non-polar moieties.

Conclusions

More efficient binding of diamondoid alcohols by smaller β-CD was a consequence of enthalpically more favorable inclusion within this receptor, whereas the opposite holds true as far as complexation entropies are concerned. This can be explained by poorer organization of the cavity water, which also accounts for the lower complexation entropies compared to inclusions with γ-CD. In other words, energy-rich cavity water is also entropy-rich water, more so in the case of the smaller receptor. In contrast, the completely entropically driven complexation of all guests with γ-CD, with the exception of 1-DAOH, indicates that removal of guest-hydrating water is accompanied by positive entropy changes. This can be explained by the more pronounced organization of water molecules around the non-polar functionalities of the guests (classical hydrophobic effect). The solely exothermic, entropically unfavorable hosting of 1-DAOH can serve as a reminder of how important dispersion interactions can be for complexes involving larger guests and macrocycles. These are however realized at the expense of entropy, most likely due to the conformational changes of the macrocycle that accompany the complete inclusion of the diamantyl subunit.

The temperature effect on the binding thermodynamics was particularly strong due to the large dehydrated hydrophobic surface. In line with our previous findings,⁵⁰ large negative reaction heat capacities indicate that the guest hydrating water experiences gradual disordering with temperature, thereby shifting the driving force from more (or completely) classical at 278 K towards predominantly non-classical at 338 K. In contrast to cyclodextrins, the hosting of diamondoid alcohols by analogous cucurbiturils was predominantly non-classical over the 278–338 K range. Moreover, the cucurbiturils were entropically inferior hosts compared to cyclodextrins, meaning that dehydration of their cavities results in considerably lower entropy changes.

Author contributions

The manuscript was written through contributions from all authors. All authors have given approval to the final version of the manuscript.

Conflicts of interest

The authors declare no competing financial interest.

Acknowledgements

These materials are based on work funded by the Croatian Science Foundation (HRZZ, UIP-2017-05-9653 and IP-2019-04-9560). The computations were performed using the resources of the computer cluster Isabella based in SRCE – University of Zagreb, University Computing Centre.

References

1. D. Chandler, Interfaces and the driving force of hydrophobic assembly, Nature, 2005, 437, 640–647.
2. T. Urbic and K. A. Dill, Water Is a Cagey Liquid, J. Am. Chem. Soc., 2018, 140, 17106–17113.
3. Y. Marcus, Ion Properties, Taylor & Francis, New York, 1997.
4. A. Ben-Naim, Water and Aqueous Solutions, Springer, New York, NY, 1974.
5. L. R. Pratt and D. Chandler, Theory of the hydrophobic effect, J. Chem. Phys., 2008, 67, 3683–3704.
6. D. M. Huang and D. Chandler, Temperature and length scale dependence of hydrophobic effects and their possible implications for protein folding, Proc. Natl. Acad. Sci. U. S. A., 2000, 97, 8324–8327.
7. S. Strazdaite, J. Versluis, E. H. G. Backus and H. J. Bakker, Enhanced ordering of water at hydrophobic surfaces, J. Chem. Phys., 2014, 140, 054711.
8. K. Lum, D. Chandler and J. D. Weeks, Hydrophobicity at Small and Large Length Scales, J. Phys. Chem. B, 1999, 103, 4570–4577.
9. H. S. Frank and M. W. Evans, Free Volume and Entropy in Condensed Systems III. Entropy in Binary Liquid Mixtures; Partial Molal Entropy in Dilute Solutions; Structure and Thermodynamics in Aqueous Electrolytes, J. Chem. Phys., 1945, 13, 507–532.
10. S. J. Gill, N. F. Nichols and I. Wadsö, Calorimetric determination of enthalpies of solution of slightly soluble liquids II. Enthalpy of solution of some hydrocarbons in water and their use in establishing the temperature dependence of their solubilities, J. Chem. Thermodyn., 1976, 8, 445–452.
11. S. J. Gill and I. Wadsö, An equation of state describing hydrophobic interactions, Proc. Natl. Acad. Sci. U. S. A., 1976, 73, 2955–2958.
12. R. D. Wauchope and R. Haque, Aqueous Solutions of Nonpolar Compounds. Heat-Capacity Effects, Can. J. Chem., 1972, 50, 133–138.
13. A. A. Sandilya, U. Natarajan and M. H. Priya, Molecular View into the Cyclodextrin Cavity: Structure and Hydration, ACS Omega, 2020, 5, 25655–25667.
14. F. Biedermann, V. D. Uzunova, O. A. Scherman, W. M. Nau and A. De Simone, Release of High-Energy Water as an Essential Driving Force for the High-Affinity Binding of Cucurbit[n]urils, J. Am. Chem. Soc., 2012, 134, 15318–15323.
15. F. Biedermann, W. M. Nau and H.-J. Schneider, The Hydrophobic Effect Revisited—Studies with Supramolecular Complexes Imply High-Energy Water as a Noncovalent Driving Force, Angew. Chem., Int. Ed., 2014, 53, 11158–11171.
16. A. J. Metherell, W. Cullen, N. H. Williams and M. D. Ward, Binding of Hydrophobic Guests in a Coordination Cage Cavity is Driven by Liberation of “High-Energy” Water, Chem. Eur. J., 2018, 24, 1554–1560.
17. G. C. Kresheck and W. A. Hargraves, Thermometric titration studies of the effect of head group, chain length, solvent, and temperature on the thermodynamics of Micelle formation, J. Colloid Interface Sci., 1974, 48, 481–493.
18. S. Paula, W. Sues, J. Tuchtenhagen and A. Blume, Thermodynamics of Micelle Formation as a Function of Temperature: A High Sensitivity Titration Calorimetry Study, J. Phys. Chem., 1995, 99, 11742–11751.
19. J. Lah, M. Bešter-Rogač, T.-M. Perger and G. Vesnaver, Energetics in Correlation with Structural Features:  The Case of Micellization, J. Phys. Chem. B, 2006, 110, 23279–23291.
20. E. Fisicaro, C. Compari and A. Braibanti, Entropy/enthalpy compensation: hydrophobic effect, micelles and protein complexes, Phys. Chem. Chem. Phys., 2004, 6, 4156–4166.
21. M. V. Rekharsky and Y. Inoue, Complexation Thermodynamics of Cyclodextrins, Chem. Rev., 1998, 98, 1875–1918.
22. A. Usenik, K. Leko, V. Petrović Peroković, Ž. Car, R. Ribić, K. Pičuljan, M. Hanževački, J. Draženović and J. Požar, Hydrophobically driven hosting – What about the guest?, J. Mol. Liq., 2023, 388, 122774.
23. K. A. Connors, The Stability of Cyclodextrin Complexes in Solution, Chem. Rev., 1997, 97, 1325–1358.
24. Cyclodextrins and Their Complexes: Chemistry, Analytical Methods, Applications, ed. H. Dodziuk, John Wiley & Sons, 2006.
25. W. M. Nau, M. Florea and K. I. Assaf, Deep Inside Cucurbiturils: Physical Properties and Volumes of their Inner Cavity Determine the Hydrophobic Driving Force for Host–Guest Complexation, Isr. J. Chem., 2011, 51, 559–577.
26. C. Márquez, R. R. Hudgins and W. M. Nau, Mechanism of Host−Guest Complexation by Cucurbituril, J. Am. Chem. Soc., 2004, 126, 5806–5816.
27. D.-S. Guo, V. D. Uzunova, K. I. Assaf, A. I. Lazar, Y. Liu and W. M. Nau, Inclusion of neutral guests by water-soluble macrocyclic hosts – a comparative thermodynamic investigation with cyclodextrins, calixarenes and cucurbiturils, Supramol. Chem., 2016, 28, 384–395.
28. S. J. Barrow, S. Kasera, M. J. Rowland, J. del Barrio and O. A. Scherman, Cucurbituril-Based Molecular Recognition, Chem. Rev., 2015, 115, 12320–12406.
29. J. Murray, K. Kim, T. Ogoshi, W. Yao and B. C. Gibb, The aqueous supramolecular chemistry of cucurbit[n]urils, pillar[n]arenes and deep-cavity cavitands, Chem. Soc. Rev., 2017, 46, 2479–2496.
30. E. Masson, X. Ling, R. Joseph, L. Kyeremeh-Mensah and X. Lu, Cucurbituril chemistry: a tale of supramolecular success, RSC Adv., 2012, 2, 1213–1247.
31. M. V. Rekharsky and Y. Inoue, Thermodynamics of Cucurbituril Complexation in Aqueous Solutions, Calorim. Therm. Anal., 2007, 34, 232–243.
32. S. Zhang, L. Grimm, Z. Miskolczy, L. Biczók, F. Biedermann and W. M. Nau, Binding affinities of cucurbit[n]urils with cations, Chem. Commun., 2019, 55, 14131–14134.
33. D. Sigwalt, M. Šekutor, L. Cao, P. Y. Zavalij, J. Hostaš, H. Ajani, P. Hobza, K. Mlinarić-Majerski, R. Glaser and L. Isaacs, Unraveling the Structure–Affinity Relationship between Cucurbit[n]urils (n = 7, 8) and Cationic Diamondoids, J. Am. Chem. Soc., 2017, 139, 3249–3258.
34. D. King, T. Šumanovac, S. Murkli, P. R. Schreiner, M. Šekutor and L. Isaacs, Cucurbit[8]uril forms tight inclusion complexes with cationic triamantanes, New J. Chem., 2023, 47, 5338–5346.
35. X. Ling, S. Saretz, L. Xiao, J. Francescon and E. Masson, Water vs. cucurbituril rim: a fierce competition for guest solvation, Chem. Sci., 2016, 7, 3569–3573.
36. S. G. Kulkarni, Z. Prucková, M. Rouchal, L. Dastychová and R. Vícha, Adamantylated trisimidazolium-based tritopic guests and their binding properties towards cucurbit[7]uril and β-cyclodextrin, J. Inclusion Phenom. Macrocyclic Chem., 2016, 84, 11–20.
37. E. Babjaková, P. Branná, M. Kuczyńska, M. Rouchal, Z. Prucková, L. Dastychová, J. Vícha and R. Vícha, An adamantane-based disubstituted binding motif with picomolar dissociation constants for cucurbit[n]urils in water and related quaternary assemblies, RSC Adv., 2016, 6, 105146–105153.
38. L. M. Grimm, S. Spicher, B. Tkachenko, P. R. Schreiner, S. Grimme and F. Biedermann, The Role of Packing, Dispersion, Electrostatics, and Solvation in High-Affinity Complexes of Cucurbit[n]urils with Uncharged Polar Guests, Chem. Eur. J., 2022, 28, e202200529.
39. A. Štimac, M. Tokić, A. Ljubetič, T. Vuletić, M. Šekutor, J. Požar, K. Leko, M. Hanževački, L. Frkanec and R. Frkanec, Functional self-assembled nanovesicles based on β-cyclodextrin, liposomes and adamantyl guanidines as potential nonviral gene delivery vectors, Org. Biomol. Chem., 2019, 17, 4640–4651.
40. M. Alešković, S. Roca, R. Jozepović, N. Bregović and M. Šekutor, Unravelling binding effects in cyclodextrin inclusion complexes with diamondoid ammonium salt guests, New J. Chem., 2022, 46, 13406–13414.
41. P. D. Ross and M. V. Rekharsky, Thermodynamics of hydrogen bond and hydrophobic interactions in cyclodextrin complexes, Biophys. J., 1996, 71, 2144–2154.
42. M. V. Rekharsky, M. P. Mayhew, R. N. Goldberg, P. D. Ross, Y. Yamashoji and Y. Inoue, Thermodynamic and Nuclear Magnetic Resonance Study of the Reactions of α- and β-Cyclodextrin with Acids, Aliphatic Amines, and Cyclic Alcohols, J. Phys. Chem. B, 1997, 101, 87–100.
43. M. V. Rekharsky and Y. Inoue, Complexation and Chiral Recognition Thermodynamics of 6-Amino-6-deoxy-β-cyclodextrin with Anionic, Cationic, and Neutral Chiral Guests:  Counterbalance between van der Waals and Coulombic Interactions, J. Am. Chem. Soc., 2002, 124, 813–826.
44. H. Schwertfeger, A. A. Fokin and P. R. Schreiner, Diamonds are a Chemist's Best Friend: Diamondoid Chemistry Beyond Adamantane, Angew. Chem., Int. Ed., 2008, 47, 1022–1036.
45. M. A. Gunawan, J.-C. Hierso, D. Poinsot, A. A. Fokin, N. A. Fokina, B. A. Tkachenko and P. R. Schreiner, Diamondoids: functionalization and subsequent applications of perfectly defined molecular cage hydrocarbons, New J. Chem., 2014, 38, 28–41.
46. S. Moghaddam, C. Yang, M. Rekharsky, Y. H. Ko, K. Kim, Y. Inoue and M. K. Gilson, New Ultrahigh Affinity Host−Guest Complexes of Cucurbit[7]uril with Bicyclo[2.2.2]octane and Adamantane Guests: Thermodynamic Analysis and Evaluation of M2 Affinity Calculations, J. Am. Chem. Soc., 2011, 133, 3570–3581.
47. L. Cao, M. Šekutor, P. Y. Zavalij, K. Mlinarić-Majerski, R. Glaser and L. Isaacs, Cucurbit[7]uril·guest pair with an attomolar dissociation constant, Angew. Chem., Int. Ed., 2014, 53, 988–993.
48. J. Voskuhl, M. Waller, S. Bandaru, B. A. Tkachenko, C. Fregonese, B. Wibbeling, P. R. Schreiner and B. J. Ravoo, Nanodiamonds in sugar rings: an experimental and theoretical investigation of cyclodextrin–nanodiamond inclusion complexes, Org. Biomol. Chem., 2012, 10, 4524–4530.
49. F. Schibilla, J. Voskuhl, N. A. Fokina, J. E. P. Dahl, P. R. Schreiner and B. J. Ravoo, Host–Guest Complexes of Cyclodextrins and Nanodiamonds as a Strong Non-Covalent Binding Motif for Self-Assembled Nanomaterials, Chem. Eur. J., 2017, 23, 16059–16065.
50. K. Leko, M. Hanževački, Z. Brkljača, K. Pičuljan, R. Ribić and J. Požar, Solvophobically Driven Complexation of Adamantyl Mannoside with β-Cyclodextrin in Water and Structured Organic Solvents, Chem. – Eur. J., 2020, 26, 5208–5219.
51. L.-E. Briggner and I. Wadsö, Heat capacities of maltose, maltotriose, maltotetrose and α-, β-, and γ-cyclodextrin in the solid state and in dilute aqueous solution, J. Chem. Thermodyn., 1990, 22, 1067–1074.
52. Á. Olvera, S. Pérez-Casas and M. Costas, Heat Capacity Contributions to the Formation of Inclusion Complexes, J. Phys. Chem. B, 2007, 111, 11497–11505.
53. I. Gómez-Orellana, D. Hallén and M. Stödeman, Microcalorimetric titration of α-cyclodextrin with some straight-chain α,ω-dicarboxylates in aqueous solution at different temperatures, J. Chem. Soc., Faraday Trans., 1994, 90, 3397–3400.
54. D. Hallén, A. Schön, I. Shehatta and I. Wadsö, Microcalorimetric titration of α-cyclodextrin with some straight-chain alkan-1-ols at 288.15, 298.15 and 308.15 K, J. Chem. Soc., Faraday Trans., 1992, 88, 2859–2863.
55. M. Bastos, L. E. Briggner, I. Shehatta and I. Wadsö, The binding of alkane-α,ω-diols to α-cyclodextrin. A microcalorimetric study, J. Chem. Thermodyn., 1990, 22, 1181–1190.
56. C. Schönbeck and R. Holm, Exploring the Origins of Enthalpy–Entropy Compensation by Calorimetric Studies of Cyclodextrin Complexes, J. Phys. Chem. B, 2019, 123, 6686–6693.
57. S. Mecozzi and J. J. Rebek, The 55% Solution: A Formula for Molecular Recognition in the Liquid State, Chem. – Eur. J., 1998, 4, 1016–1022.
58. N. A. Fokina, B. A. Tkachenko, A. Merz, M. Serafin, J. E. P. Dahl, R. M. K. Carlson, A. A. Fokin and P. R. Schreiner, Hydroxy Derivatives of Diamantane, Triamantane, and [121]Tetramantane: Selective Preparation of Bis-Apical Derivatives, Eur. J. Org. Chem., 2007, 4738–4745.
59. W.-H. Huang, S. Liu and L. Isaacs, in Modern Supramolecular Chemistry, ed. F. Diederich, P. J. Stang and R. R. Tykwinski, Wiley-VCH, 2008, pp. 113–142.
60. L.-E. Briggner and I. Wadsö, Test and calibration processes for microcalorimeters, with special reference to heat conduction instruments used with aqueous systems, J. Biochem. Biophys. Methods, 1991, 22, 101–118.
61. S. Grimme, Exploration of Chemical Compound, Conformer, and Reaction Space with Meta-Dynamics Simulations Based on Tight-Binding Quantum Chemical Calculations, J. Chem. Theory Comput., 2019, 15, 2847–2862.
62. P. Pracht, F. Bohle and S. Grimme, Automated exploration of the low-energy chemical space with fast quantum chemical methods, Phys. Chem. Chem. Phys., 2020, 22, 7169–7192.
63. Y. H. Zhao, M. H. Abraham and A. M. Zissimos, Fast Calculation of van der Waals Volume as a Sum of Atomic and Bond Contributions and Its Application to Drug Compounds, J. Org. Chem., 2003, 68, 7368–7373.
64. J. Szejtli, Introduction and General Overview of Cyclodextrin Chemistry, Chem. Rev., 1998, 98, 1743–1754.
65. M. B. Hillyer and B. C. Gibb, Molecular Shape and the Hydrophobic Effect, Annu. Rev. Phys. Chem., 2016, 67, 307–329.
66. J. W. E. Acree and J. S. Chickos, in NIST Chemistry WebBook, NIST Standard Reference Database Number 69, ed. P. J. Linstrom and W. G. Mallard, National Institute of Standards and Technology, Gaithersburg MD, 20899, 2023.
67. M. Stodeman and I. Wadsö, Scope of microcalorimetry in the area of macrocyclic chemistry, Pure Appl. Chem., 1995, 67, 1059–1068.
68. W. M. Haynes, CRC Handbook of Chemistry and Physics, CRC Press, Hoboken, 2014, 95th edn.
69. V. N. Emel’yanenko, S. P. Verevkin, M. A. Varfolomeev, V. V. Turovtsev and Y. D. Orlov, Thermochemical Properties of Formamide Revisited: New Experiment and Quantum Mechanical Calculations, J. Chem. Eng. Data, 2011, 56, 4183–4187.
70. S. P. Verevkin, Determination of vapor pressures and enthalpies of vaporization of 1,2-alkanediols, Fluid Phase Equilib., 2004, 224, 23–29.
71. D. Berling and G. Olofsson, Solvation of small hydrophobic molecules in formamide: A calorimetric study, J. Solution Chem., 1994, 23, 911–923.
72. I. A. Sedov, M. A. Stolov and B. N. Solomonov, Thermodynamics of solvation and solvophobic effect in formamide, J. Chem. Thermodyn., 2013, 64, 120–125.
73. N. T. Southall, K. A. Dill and A. D. J. Haymet, A View of the Hydrophobic Effect, J. Phys. Chem. B, 2002, 106, 521–533.
74. G. Graziano, On the temperature dependence of hydration thermodynamics for noble gases, Phys. Chem. Chem. Phys., 1999, 1, 1877–1886.
75. E. Wilhelm, R. Battino and R. J. Wilcock, Low-pressure solubility of gases in liquid water, Chem. Rev., 1977, 77, 219–262.
76. Y. Ishihara, S. Okouchi and H. Uedaira, Dynamics of hydration of alcohols and diols in aqueous solutions, J. Chem. Soc., Faraday Trans., 1997, 93, 3337–3342.
77. D. Laage, G. Stirnemann and J. T. Hynes, Why Water Reorientation Slows without Iceberg Formation around Hydrophobic Solutes, J. Phys. Chem. B, 2009, 113, 2428–2435.
78. C. Petersen, K. J. Tielrooij and H. J. Bakker, Strong temperature dependence of water reorientation in hydrophobic hydration shells, J. Chem. Phys., 2009, 130, 214511.
79. A. A. Bakulin, M. S. Pshenichnikov, H. J. Bakker and C. Petersen, Hydrophobic Molecules Slow Down the Hydrogen-Bond Dynamics of Water, J. Phys. Chem. A, 2011, 115, 1821–1829.
80. J. G. Davis, K. P. Gierszal, P. Wang and D. Ben-Amotz, Water structural transformation at molecular hydrophobic interfaces, Nature, 2012, 491, 582–585.
81. J. Grdadolnik, F. Merzel and F. Avbelj, Origin of hydrophobicity and enhanced water hydrogen bond strength near purely hydrophobic solutes, Proc. Natl. Acad. Sci. U. S. A., 2017, 114, 322–327.
82. D. Ben-Amotz, Hydration-Shell Vibrational Spectroscopy, J. Am. Chem. Soc., 2019, 141, 10569–10580.
83. N. Galamba, Water Tetrahedrons, Hydrogen-Bond Dynamics, and the Orientational Mobility of Water around Hydrophobic Solutes, J. Phys. Chem. B, 2014, 118, 4169–4176.
84. J. Grabowska, A. Kuffel and J. Zielkiewicz, Revealing the Frank–Evans “Iceberg” Structures within the Solvation Layer around Hydrophobic Solutes, J. Phys. Chem. B, 2021, 125, 1611–1617.
85. W. Blokzijl and J. B. F. N. Engberts, Hydrophobic Effects. Opinions and Facts, Angew. Chem., Int. Ed. Engl., 1993, 32, 1545–1579.
86. G. Graziano, Contrasting the hydration thermodynamics of methane and methanol, Phys. Chem. Chem. Phys., 2019, 21, 21418–21430.
87. G. Graziano, Shedding light on the hydrophobicity puzzle, Pure Appl. Chem., 2016, 88, 177–188.
88. G. Graziano, Hydration entropy of polar, nonpolar and charged species, Chem. Phys. Lett., 2009, 479, 56–59.
89. F. Böhm, G. Schwaab and M. Havenith, Mapping Hydration Water around Alcohol Chains by THz Calorimetry, Angew. Chem., Int. Ed., 2017, 56, 9981–9985.
90. V. Conti Nibali, S. Pezzotti, F. Sebastiani, D. R. Galimberti, G. Schwaab, M. Heyden, M. P. Gaigeot and M. Havenith, Wrapping Up Hydrophobic Hydration: Locality Matters, J. Phys. Chem. Lett., 2020, 11, 4809–4816.
91. P. Buchanan, N. Aldiwan, A. K. Soper, J. L. Creek and C. A. Koh, Decreased structure on dissolving methane in water, Chem. Phys. Lett., 2005, 415, 89–93.
92. L. Rossato, F. Rossetto and P. L. Silvestrelli, Aqueous Solvation of Methane from First Principles, J. Phys. Chem. B, 2012, 116, 4552–4560.

---

Footnote

† Electronic supplementary information (ESI) available: Analytical details on binding studies, computational details, and copies of NMR spectra. See DOI: https://doi.org/10.1039/d3nj03097k

---