SPICY: a method for single scan rotating frame relaxometry

Abstract

T _1ρ is an NMR relaxation mode that is sensitive to low frequency molecular motions, making it an especially valuable tool in biomolecular research. Here, we introduce a new method, SPICY, for measuring T_1ρ relaxation times. In contrast to conventional T_1ρ experiments, in which the sequence is repeated many times to determine the T_1ρ time, the SPICY sequence allows determination of T_1ρ within a single scan, shortening the experiment time remarkably. We demonstrate the method using ¹H T_1ρ relaxation dispersion experiments. Additionally, we combine the sequence with spatial encoding to produce 1D images in a single scan. We show that T_1ρ relaxation times obtained using the single scan approach are in good agreement with those obtained using the traditional experiments.

---

1. Introduction

Nuclear magnetic resonance (NMR) experiments provide versatile information on time-dependent dynamic processes of molecules. NMR relaxation experiments give information on molecular rotational motions and environments. Most often, NMR relaxation studies utilize longitudinal T₁ and transverse T₂ relaxation. T₁ is sensitive to molecular motions with frequencies close to the Larmor frequency, ω₀ = γB₀, which is determined using gyromagnetic ratio γ and the strength of the static magnetic field B₀.¹ To probe a range of molecular motion frequencies, B₀ needs to be changed,² which is not possible with conventional NMR instruments.

However, there exists a third relaxation process called T_1ρ, or spin–lattice relaxation in the rotating frame, where the nuclear magnetization returns to equilibrium under the influence of continuous wave spin lock (SL) field B₁.^1,3,4 Since the ω₁ frequency can be changed, typically between 1 and 6 kHz, T_1ρ can be utilized to probe motional processes over a wide dynamic timescale.⁴ The T_1ρ relaxation time extends between T₂ and T₁ as the SL field increases, and this increase of T_1ρ is called T_1ρ relaxation dispersion.^4–7

The other common method for measuring relaxation dispersion is T₂ dispersion in which the effective transverse relaxation time is measured as a function of the time between the refocusing pulses in the Carr–Purcell–Meiboom–Gill (CPMG) pulse sequence.^8,9 However, in conventional NMR instruments, T₂ relaxation dispersion measurements are limited to lower frequency ranges than T_1ρ dispersion.^4,10

Relaxation dispersion measurements have been shown to be a powerful tool for characterizing micro- and millisecond timescale biomolecular motions, such as chemical exchange and protein dynamics^{4–7,11–16} and biomechanical properties of objects like rat tissues^17,18 and articular cartilage of various origins.^19–22 Articular cartilage, which is a connective tissue possessing very specialized biomechanical properties, is composed of cartilage cells which are surrounded by an extracellular matrix, principally consisting of collagen, proteoglycans and water.²³T_1ρ relaxation and dispersion studies have been utilized in both NMR spectroscopy and MRI to track biochemical changes of the cartilage extracellular matrix.^{19–22,24–27} An additional use of T_1ρ measurements is T_1ρ-weighted magnetic resonance imaging (MRI).²⁸T_1ρ preparation is often paired with MRI to obtain T_1ρ image contrast which provides higher resolution to some pathological changes and disease progression than only with T₁ and T₂ weighted imaging. For example, T_1ρ is sensitive to proteoglycan loss in articular cartilage as osteoarthritis progresses and T_1ρ mapping can detect early articular cartilage degradation with more sensitivity than T₂ mapping.^29–32 Furthermore, T_1ρ has shown potential to probe diseases such as spinal disc degeneration,^33,34 Alzheimer's disease,^35,36 Parkinson's disease³⁷ and ischemia.³⁸

The conventional T_1ρ pulse sequence consists of a continuous wave (CW) radiofrequency (RF) pulse followed by the spin lock period and signal acquisition.^39–41 Alternatively, the T_1ρ experiments can be performed using adiabatic spin locking.^42–44 The conventional experiments must be repeated with incremented spin lock times, such that the total experiment time ranges from minutes to days depending on the number of repetitions. Furthermore, T_1ρ dispersion experiments are more time consuming since the experiments must be repeated with several SL frequencies. Anoardo et al. have presented an off-resonance technique (SLOAFI)⁴⁵ for determining T_1ρ relaxation dispersion in a shorter time, but to our knowledge, no methods for acceleration of the T_1ρ preparation portion of the on-resonance rotating frame relaxation experiment have been reported. To circumvent the long experiment times, several rapid T_1ρ imaging methods have been developed. For example, Duvvuri et al.⁴⁶ combined a fast spin echo sequence with T_1ρ preparation, Li et al.⁴⁷ developed a T_1ρ imaging sequence based on spiral k-space readout, Liimatainen and Gröhn⁴⁸ acquired T_1ρ decay of one k-space line during single adiabatic pulse train, and Bothakur et al.⁴⁹ introduced a pulse sequence for spin locked echo planar imaging (SLEPI). There are also accelerated T_1ρ methods based on gradient echoes and steady state free precession,^50–53 and reconstruction of undersampled k-space data.^54–60 The development of rapid imaging methods is advantageous not only for reducing patient imaging time, but also for allowing imaging of dynamic objects like a cardiovascular system.^54–57 In addition, the short experiment time of SLEPI has expanded the application of T_1ρ to functional MRI.⁶¹

Here, we introduce a novel single scan method for rotating frame relaxometry. In this method, the T_1ρ is produced by the SPIn lock CYcle (SPICY), in which a single RF excitation pulse is followed by a loop of spin locking and signal acquisition lobes. This allows for the determination of T_1ρ within a single scan, similar to the time domain Carr–Purcell–Meiboom–Gill (CPMG)⁸ acquisition, which measures T₂. The total scan time is from a few seconds to minutes. We perform ¹H T_1ρ dispersion experiments with both the conventional and the SPICY T_1ρ sequences on liquid samples and protein hydrogels prepared from the main constituents of the articular cartilage extracellular matrix, and we show that the relaxation time information obtained with these methods is in good agreement. In addition, we show that the SPICY sequence can be combined with spatial encoding to produce 1D images in a single scan.

2. Experimental

2.1. Samples

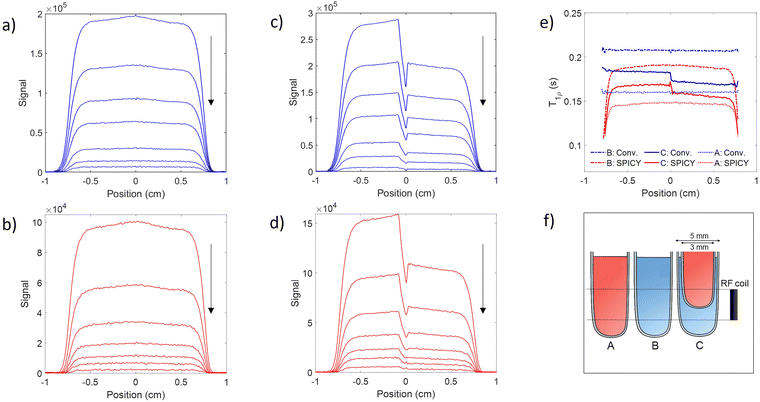
Three liquid samples, A, B and C, were used. The samples were made by dissolving CuSO₄·5H₂O in water (10% H₂O, 90% D₂O), with the sample A containing 0.013 wt% and sample B containing 0.04 wt% CuSO₄. The solutions were placed in 5 mm NMR tubes. Sample C represents a two-compartment sample of liquids A and B made by placing the 0.04 wt% solution to the bottom of a 5 mm tube inside which a 3 mm tube containing the 0.13 wt% solution was placed. The tubes were placed in the RF coil in such a way that the liquid B covered the bottom half and liquids A and B the top half of the coil. The illustration of the samples is shown in Fig. 3f.

A hydrogel sample containing 40 mg g⁻¹ collagen type II, 10 mg g⁻¹ chondroitin sulfate and phosphate-buffered saline (PBS) was used. 10 mL of collagen solution (rat tail, ∼6 mg mL⁻¹ in 0.01 M acetic acid, Merck KGaA, Darmstadt, Germany) was added to a sample tube together with 0.15 mL 10× PBS. Stock solution of CS (50–80 mg mL⁻¹ CS powder from bovine trachea, Merck KGaA, Darmstadt, Germany in double distilled water) was added to the collagen solution and the mixture was diluted with double distilled water to give a total volume of 6 mL. The sample was thoroughly mixed and pH adjusted to basic (pH: 8–9) using 1 M sodium hydroxide (NaOH) and 0.5 M hydrochloric acid (HCl). The sample tube was placed for 45–60 min in a water bath at 37 °C to solidify the mixture into a gel. The solid was removed from the sample tube, transferred into a plastic cell culture dish, and dried at 40 °C in a laminar flow oven until the mass of the sample was reduced to 1.50 ± 0.03 g. Finally, the sample was placed in a 5 mm NMR tube in the middle of the RF coil.

2.2. Pulse sequence design

The conventional T_1ρ pulse sequence³⁹ (Fig. 1a) consists of a 90° excitation pulse, two CW spin lock pulses separated by a 180° pulse, and an acquisition period in which the free induction decay (FID) is recorded. To encode for T_1ρ, the sequence is repeated with incremented SL pulse durations. Contrary to the conventional T_1ρ pulse sequence, the SPICY pulse sequence (Fig. 1b) utilizes a loop structure which repeats the spin locking of constant length and signal acquisition blocks n times during one scan. To accomplish this, the acquisition period acquires the signal intensity of the spin echo. While in the conventional experiment T_1ρ is determined from the FID signal, in the SPICY, the T_1ρ fit is obtained from the echo intensities corresponding to different echo numbers. The 1D imaging versions of the conventional T_1ρ and the SPICY sequences are shown in Fig. 1c and d. In the SPICY imaging sequence, the third 180° pulse and the dephasing gradient block are needed to return to the center of the k-space before the beginning of the next loop. An 8-step phase cycle is used in the SPICY sequences (see the ESI†).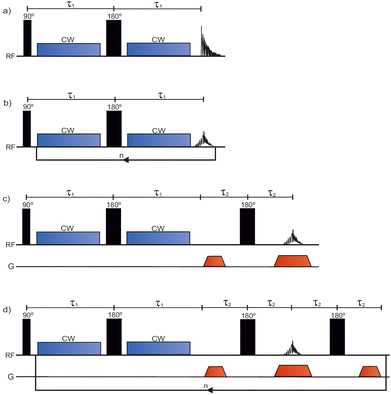

Fig. 1 The pulse sequences for (a) conventional T_1ρ, (b) SPICY, (c) conventional 1D T_1ρ imaging and (d) SPICY 1D imaging.

2.3. Experiments

The T_1ρ experiments were performed on a spectrometer with 600 MHz ¹H operating frequency equipped with a 5 mm QXI probe with a gradient strength of 0.6 T m⁻¹ and TopSpin 3.6.2 software (Avance III, Bruker Biospin, MA, USA). The probe temperature was set to 290 K during the experiments. In all the experiments, the 90° excitation pulse had a length of P₁ ≈ 9.5 μs, and the 180° pulse had P₂ ≈ 19 μs. The relaxation delay in all the experiments was 15 s. In all T_1ρ experiments, the SL-pulses were applied on-resonance. In the conventional T_1ρ experiments (Fig. 1a and c), the SL-pulse length was incremented between 30 and 480 ms in 16 steps. In the non-imaging SPICY measurements (Fig. 1b) of the water samples, the SL pulse duration was 30 ms and the number of echoes n was 16. The signal at the center of the echo was acquired for 50 μs with 12 points and the total SL interruption time during a single loop including all the SL gaps was 130 μs. In the SPICY measurements of hydrogels, the SL pulse duration was 40 ms, the number of echoes was 12, the signal acquisition time was 25 μs, the number of points was 6 and the total SL interruption time was 100 μs. In the SPICY imaging sequence (Fig. 1d), the signal at the center of the echo was acquired for 0.82 ms with 512 points and the total SL interruption time was 8 ms. In both imaging sequences, the read gradient length was 0.82 ms, the gradient ramp time was 100 μs, and the gradient strength was 0.3 T m⁻¹. The length of the dephasing gradients was half of the read gradients. The non-imaging sequences were performed with SL frequencies of 2–11 kHz and the imaging experiments with 7 kHz. The number of scans was 16 for the water samples and 32 for the hydrogel sample. The experiment time of one scan was 4 min 12 s for the conventional and 16 s for the SPICY sequences.

2.4. Data analysis

To find T_1ρ relaxation times, the equation was fitted to the experimentally obtained signal decay. In the analysis of the SPICY data, the signal was summed across all the points acquired for each echo to perform T_1ρ fitting while the area under the spectra was used in the conventional T_1ρ analysis. The 1D images were obtained by Fourier transform. The data analysis was done using MATLAB (Mathworks, R2020b, Natick, MA, USA).

3. Results and discussion

To determine the accuracy of the single scan acquisition method, the T_1ρ relaxation times recorded using the SPICY method were compared to the T_1ρ relaxation times recorded using the two-dimensional conventional acquisition scheme (Fig. 2). The T_1ρ values were measured for three aqueous solutions A, B and C with variable SL fields in the range of 2–11 kHz (Fig. 2a). The T₂ values were measured for all samples with SPICY sequence by turning off the CW pulses. All samples show stable T_1ρ values over the whole frequency range, and the difference between conventional and SPICY T_1ρ values is less than 2% for all three aqueous solutions, which indicates that the SPICY sequence is precise and works under different conditions.

Fig. 2 (a) T_1ρ measured with different spin lock frequencies for the water samples A, B and C. (b) T_1ρ dispersion measured for the hydrogel sample. Blue color indicates the conventional T_1ρ and red SPICY. The T_1ρ value measured with 0 kHz SL frequency is the T₂ of the sample.

To test the applicability of the SPICY sequence on a biochemically more relevant sample, T_1ρ dispersion was measured for the hydrogel sample containing collagen and chondroitin sulfate which acts as a model of the cartilage extracellular matrix (Fig. 2b). The SL frequency was varied between 2–12 kHz leading the T_1ρ values to increase between 0.12–0.17 s. The difference between the SPICY and reference T_1ρ values is only 1–2% showing that the SPICY method is applicable to T_1ρ dispersion studies of more complicated samples as well. The SPICY sequence was further tested with imaging such that 1D read gradients were used to record 1D signal intensity data (Fig. 1d and 3). The 1D images of the samples A and C measured with the conventional and the SPICY readouts are shown in Fig. 3a–d. The image profiles obtained with both methods are consistent. The T_1ρ profiles measured for all three samples are shown in Fig. 3e. The T_1ρ values obtained with SPICY imaging are about 8% smaller than the values measured with the conventional imaging sequence. The small difference is expected to arise predominantly from the signal decay caused by molecular diffusion in the presence of gradients, because the SPICY imaging experiment includes more gradient pulses due to the additional rephasing gradient and loop structure.⁶² The difference can be minimized by minimizing the gradient strength and length. Both methods give stable profiles, however the T_1ρ values measured with SPICY show decreased T_1ρ values at the edges of the coil. This is a consequence of a decrease of the B₁ field close to the edges of the coil and much higher number of 180° pulses in the SL gaps during the SPICY experiment. One of the major differences between the principles of SPICY and conventional sequences is the T₂ decay occurring during the SL gaps during the SPICY experiment. For that reason, the delays between the SL pulses and the signal acquisition period should be set as short as possible. Especially samples with strong dispersion can be very sensitive to non-locked periods, which is why T₂ relaxation should be considered when performing the SPICY measurements. In the SPICY imaging sequence, which includes the longer acquisition period due to the imaging gradients, the T₂ decay affects the signal more than in the non-imaging experiments. The T₂ effect can be reduced either by shortening the non-locked periods or by reducing the number of echoes and increasing the SL pulse length. Since the acquisition time of the echo signal should be short, the SPICY sequence cannot be used to resolve spectral lines by Fourier transform.

Fig. 3 (a–d) 1D MR images of the samples A (left; a and b) and C (right; c and d) measured with the conventional (blue; a and c) and the SPICY T_1ρ (red; b and d) sequences. The arrow indicates the direction of increasing spin lock time (a and c) or echo number (b and d). (e) T_1ρ profiles of samples A (dotted line), B (dashed-dotted line) and C (solid line) measured with the conventional (blue) and the SPICY sequence (red). (f) Illustration of samples A, B and C with respect to the RF coil.

The SPICY method has a lot of potential for future applications in both spectroscopy and imaging. The SPICY method could be applied to accelerate the T_1ρ relaxation dispersion studies of biomacromolecules and to determine, for example, the rates of chemical exchange or conformational changes.^{4–7,11–22} We showed dispersion measurements with SL fields of 2–11 kHz, however, a wider range of SL fields could be applicable. The SPICY sequence could be further developed for accelerating multidimensional T_1ρ imaging by adding additional spatial encoding dimensions, for example, in the same way as in the RARE imaging.⁶³ Because SPICY is much faster and the spin locking does not produce more energy deposition compared to the conventional method, it could have a lot of potential in clinical MRI. The higher number of 180° pulses may cause more heating compared to the standard method, however, the problem could be avoided by replacing the image readout part by gradient echo. The T_1ρ values of the SPICY imaging remain slightly smaller than the reference values due to the higher number of gradient pulses and longer SL gap during signal acquisition, but it must be noted that in clinical imaging, the contrast between tissues is generally more important than the quantitative T_1ρ values. Also, the decreased T_1ρ values at the edges of the RF coil can be managed by placing the imaged object in the middle of the sensitive area of the coil. In the same way as the SLEPI sequence,^49,60 SPICY could be applied to imaging of dynamic processes. It might be possible to use adiabatic spin locking in the SPICY sequence.^42–44 Because the SPICY allows signal readout in a similar manner to the CPMG,⁸ it could be added to multidimensional NMR or Laplace NMR experiments⁶⁴ to correlate T_1ρ with the other relaxation or diffusion parameters. Furthermore, the 1D imaging version of SPICY could replace the CPMG readout part in multidimensional ultrafast NMR⁶⁵ or Laplace NMR (LNMR) experiments.^62,66–69 The single scan approach also significantly facilitates the use of nuclear spin hyperpolarization techniques^70–73 to boost the sensitivity of the experiments by several orders of magnitude. For example, recently Qi et al.⁷⁴ boosted T₂ relaxation dispersion measurements by dissolution dynamic nuclear polarization (dDNP) to study protein–ligand binding. Similarly, SPICY could be exploited in hyperpolarized studies as well.

4. Conclusions

In summary, we introduced a novel single-scan method called SPICY for measuring T_1ρ relaxation times. We demonstrated that the SPICY method can be successfully used to measure T_1ρ dispersions with spin locks of 2–11 kHz. SPICY shortens the scan time about an order of magnitude while producing less RF energy deposition compared to the conventional T_1ρ sequence. We showed that the T_1ρ values obtained from the SPICY sequence are in good agreement with those of the conventional method. We also combined the SPICY with one dimensional spatial encoding and showed that the method is applicable to single scan 1D imaging as well. Overall, the single scan T_1ρ method presented in this work has a lot of potential for future applications in rotating frame relaxation studies, MR imaging, ultrafast multidimensional NMR and Laplace NMR studies, as well as in hyperpolarize NMR and MRI experiments.

Author contributions

K. Tolkkinen was responsible for conceptualization, methodology, validation, investigation, resources, and writing – original draft. S. Mailhiot was responsible for conceptualization, methodology, validation, investigation, and writing – review and editing. A. Selent was responsible for conceptualization, methodology, and writing – review and editing. O. Mankinen was responsible for support in the final measurements, and writing – review and editing. H. Henschel, M. Nieminen and M. Hanni were responsible for planning and preparing the hydrogel sample, and writing – review and editing. A. M. Kantola was responsible for supervision, and writing – review and editing. T. Liimatainen was responsible for supervision, and writing – review and editing. V.-V. Telkki was responsible for conceptualization, supervision, and writing – review supervision, and writing – review and editing.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The authors acknowledge the financial support from the European Research Council (grant number 772110), the Academy of Finland (grant numbers 340099, 340761 and 896824), the Marie Sklodowska-Curie Actions (grant number 896824), KAUTE foundation and the University of Oulu Kvantum Institute. Part of this work was carried out with the support from the Centre for Material Analysis, the University of Oulu, Finland. The authors thank Olli-Pekka Aro for his contribution in the preparation of the protein gel sample.

References

1. J. Keeler, Understanding NMR spectroscopy, John Wiley & Sons, Chichester, UK, 2nd edn, 2010.
2. E. Anoardo, G. Galli and G. Ferrante, Appl. Magn. Reson., 2001, 20, 365–404.
3. A. G. Redfield, Phys. Rev., 1955, 98, 1787–1809.
4. A. G. Palmer and F. Massi, Chem. Rev., 2006, 106, 1700–1719.
5. A. Rangadurai, E. S. Szymaski, I. J. Kimsey, H. Shi and H. M. Al-Hashimi, Prog. Nucl. Magn. Reson. Spectrosc., 2019, 112–113, 55–102.
6. P. Neudecker, P. Lundström and L. E. Kay, Biophys. J., 2009, 96, 2045–2054.
7. D. F. Hansen, P. Vallurupalli and L. E. Kay, J. Biomol. NMR, 2008, 41, 113–120.
8. S. Meiboom and D. Gill, Rev. Sci. Instrum., 1958, 29, 688–691.
9. J. P. Loria, M. Rance and A. G. Palmer, J. Am. Chem. Soc., 1999, 121, 2331–2332.
10. J. G. Reddy, S. Pratihar, D. Ban, S. Frischkorn, S. Becker, C. Griesinger and D. Lee, J. Biomol. NMR, 2018, 70, 1–9.
11. D. M. Korzhnev, V. Y. Orekhov and L. E. Kay, J. Am. Chem. Soc., 2005, 127, 713–721.
12. F. Massi, E. Johnson, C. Wang, M. Rance and A. G. Palmer, J. Am. Chem. Soc., 2004, 126, 2247–2256.
13. A. G. Palmer, J. Magn. Reson., 2014, 241, 3–17.
14. J. G. Cobb, J. Xie and J. C. Gore, Magn. Reson. Med., 2011, 66, 1563–1571.
15. M. Marušič, J. Schlagnitweit and K. Petzold, ChemBioChem, 2019, 20, 2685–2710.
16. P. Lundström and M. Akke, J. Biomol. NMR, 2005, 32, 163–173.
17. S. K. Koskinen, P. T. Niemi, S. A. Kajander and M. E. Komu, Magn. Reson. Imaging, 2006, 24, 295–299.
18. S. K. Koskinen, A. M. Virta, P. T. Niemi, S. A. Kajander and M. E. Komu, Magn. Reson. Imaging, 1999, 17(7), 1043–1047.
19. E. Rössler, C. Mattea and S. Stapf, Magn. Reson. Med., 2015, 73, 2005–2014.
20. U. Duvvuri, A. D. Goldberg, J. K. Kranz, L. Hoang, R. Reddy, F. W. Wehrli, A. J. Wand, S. W. Englander and J. S. Leigh, Proc. Natl. Acad. Sci. U. S. A., 2001, 98, 12479–12484.
21. N. Wang and Y. Xia, J. Magn. Reson., 2011, 212, 124–132.
22. H. Elsayed, J. Karjalainen, M. J. Nissi, J. Ketola, A. W. Kajabi, V. Casula, Š. Zbýň, M. T. Nieminen and M. Hanni, Magn. Reson. Imaging, 2023, 97, 91–101.
23. A. J. Sophia Fox, A. Bedi and S. A. Rodeo, Sports Health, 2009, 1(6), 461–468.
24. P. Wang, J. Block and J. C. Gore, Magn. Reson. Imaging, 2015, 33(1), 38–42.
25. E.-N. Salo, T. Liimatainen, S. Michaeli, S. Mangia, J. Ellermann and M. Nieminen, T1rho dispersion in constituent-specific degradation models of articular cartilage with correlation to biomechanical properties, Intl. Soc. Magn. Reson. Med., Elbourne, Australia, 2012.
26. K. E. Keenan, T. F. Besier, J. M. Pauly, R. L. Smith, S. L. Delp, G. S. Beaupre and G. E. Gold, Cartilage, 2015, 6(2), 113–122.
27. U. Duvvuri, R. Reddy, S. D. Patel, J. H. Kaufman, J. B. Kneeland and J. S. Leigh, Magn. Reson. Med., 1997, 38(6), 863–867.
28. R. W. Brown, Y. N. Cheng, E. M. Haacke, M. R. Thompson and R. Venkatesan, Magnetic Resonance Imaging - Physical Principles and Sequence Design, John Wiley & Sons, Hoboken, New Jersey, 2nd edn, 2014.
29. S. V. Akella, R. R. Regatte, A. J. Gougoutas, A. Borthakur, E. M. Shapiro, J. B. Kneeland, J. S. Leigh and R. Reddy, Magn. Reson. Med., 2001, 46, 419–423.
30. U. Duvvuri, S. Kudchodkar, R. Reddy and J. S. Leigh, Osteoarthritis Cartilage, 2002, 10, 838–844.
31. R. R. Regatte, S. V. Akella, J. H. Lonner, J. B. Kneeland and R. Reddy, J. Magn. Reson. Imaging., 2006, 23, 547–553.
32. X. Li, C. Benjamin, Ma, T. M. Link, D. D. Castillo, G. Blumenkrantz, J. Lozano, J. Carballido-Gamio, M. Ries and S. Majumdar, Osteoarthritis Cartilage, 2007, 15, 789–797.
33. M. A. Yoon, S. J. Hong, C. H. Kang, K. S. Ahn and B. H. Kim, Magn. Reson. Imaging, 2016, 34, 932–939.
34. Y. X. Wang, F. Zhao, J. F. Griffith, G. S. Mok, J. C. Leung, A. T. Ahuja and J. Yuan, Eur. Radiol., 2013, 23, 228–234.
35. A. Borthakur, M. Sochor, C. Davatzikos, J. Q. Trojanowski and C. M. Clark, NeuroImage, 2008, 41, 1199–1205.
36. M. Haris, E. McArdle, M. Fenty, A. Singh, C. Davatzikos, J. Q. Trojanowski, E. R. Melhem, C. M. Clark and A. Borthakur, J. Magn. Reson. Imaging, 2009, 29, 1008–1012.
37. I. Nestrasil, S. Michaeli, T. Liimatainen, C. E. Rydeen, C. M. Kotz, J. P. Nixon, T. Hanson and P. J. Tuite, J. Neurol., 2010, 257, 964–968.
38. M. I. Kettunen, O. Gröhn, M. Penttonen and R. A. Kauppinen, Magn. Reson. Med., 2001, 46, 565–572.
39. W. R. Witschey, A. Borthakur, M. A. Elliot, E. Mellon, S. Niyogi, D. J. Wallman, C. Wang and R. Reddy, J. Magn. Reson., 2007, 186, 75–85.
40. R. E. Sepponen, J. A. Pohjonen, J. T. Sipponen and J. I. Tanttu, J. Comput. Assisted Tomogr., 1985, 9, 1007–1011.
41. I. A. Gilani and R. Sepponen, NMR. Biomed., 2016, 29, 841–861.
42. T. Liimatainen, D. J. Sorce, R. O'Connell, M. Garwood and S. Michaeli, Magn. Reson. Med., 2010, 64(4), 983–994.
43. J. Rautiainen, M. J. Nissi, T. Liimatainen, W. Herzog, R. K. Korhonen and M. T. Nieminen, Osteoarthritis Cartilage, 2014, 22(10), 1444–1452.
44. N. E. Hänninen, T. Liimatainen, M. Hanni, O. Gröhn, M. T. Nieminen and M. J. Nissi, Sci. Rep., 2022, 12(1), 12155.
45. E. Anoardo, C. Hauser and R. Kimmich, J. Magn. Reson., 2000, 142, 372–378.
46. U. Duvvuri, S. R. Charagundla, S. B. Kudchodkar, J. H. Kaufman, J. B. Kneeland, R. Rizi, J. S. Leigh and R. Reddy, Radiology, 2001, 220, 822–826.
47. X. Li, E. T. Han, D. Newitt and S. Majumdar, Conf. Proc. IEEE. Eng. Med. Biol. Soc., 2004, 2004, 1032–1035.
48. T. Liimatainen and O. Gröhn, A fast, quantitative T1ρ imaging method, Int. Soc. Magn. Reson. Med., Stockholm, Sweden, 2010.
49. A. Borthakur, J. Hulvershorn, E. Gualtieri, A. J. Wheaton, S. Charagundla, M. A. Elliott and R. Reddy, J. Magn. Reson. Imaging, 2006, 23, 591–596.
50. A. Sharafi, M. V. W. Zibetti, G. Chang, M. Cloos and R. R. Regatte, NMR. Biomed., 2022, 35, e4800.
51. P. Schuenke, D. Paech, C. Koehler, J. Windschuh, P. Bachert, M. E. Ladd, H. P. Schlemmer, A. Radbruch and M. Zaiss, Sci. Rep., 2017, 7, 42093.
52. W. R. Witschey, A. Borthakur, M. A. Elliott, M. Fenty, M. A. Sochor, C. Wang and R. Reddy, J. Magn. Reson. Imaging., 2008, 28, 744–754.
53. S. Zbyn, M. J. Nissi, H. Elsayed, V. Casula, M. Hanni, T. Liimatainen and M. T. Nieminen, Accelerated T1ρ Dispersion Data Acquisition for Correlation Time Mapping of Cartilage at 3T, Proc. Intl. Soc. Mag. Reson. Med., Paris, France, 2018.
54. S. Kamesh Iyer, B. Moon, E. Hwuang, Y. Han, M. Solomon, H. Litt and W. R. Witschey, J. Cardiovasc. Magn. Reson., 2019, 21, 5.
55. M. Gram, D. Gensler, P. Winter, M. Seethaler, P. A. Arias-Loza, J. Oberberger, P. M. Jakob and P. Nordbeck, MAGMA, 2022, 35, 325–340.
56. H. Qi, A. Bustin, T. Kuestner, R. Hajhosseiny, G. Cruz, K. Kunze, R. Neji, R. M. Botnar and C. Prieto, J. Cardiovasc. Magn. Reson., 2020, 22, 12.
57. H. Qi, Z. Lv, J. Hu, J. Xu, R. Botnar, C. Prieto and P. Hu, Magn. Reson. Med., 2022, 88, 2520–2531.
58. Y. Zhu, Q. Zhang, Q. Liu, Y. X. Wang, X. Liu, H. Zheng, D. Liang and J. Yuan, Magn. Reson. Med., 2015, 73, 263–272.
59. M. Hanhela, A. Paajanen, M. J. Nissi and V. Kolehmainen, J. Imaging, 2022, 8, 157.
60. H. Li, M. Yang, J. H. Kim, C. Zhang, R. Liu, P. Huang, D. Liang, X. Zhang, X. Li and L. Ying, Magn. Reson. Med., 2023, 89, 64–76.
61. J. Hulvershorn, A. Borthakur, L. Bloy, E. E. Gualtieri, R. Reddy, J. S. Leigh and M. A. Elliott, Magn. Reson. Med., 2005, 54, 1155–1162.
62. V.-V. Telkki, M. Urbańczyk and V. Zhivonitko, Prog. Nucl. Magn. Reson. Spectrosc., 2021, 126–127, 101–120.
63. J. Hennig, A. Nauerth and H. Friedburg, Magn. Reson. Med., 1986, 3, 823–833.
64. P. T. Callaghan, Translational Dynamics and Magnetic Resonance: Principles of Pulsed Gradient Spin Echo NMR, Oxford University Press, Oxford, 2011.
65. A. Tal and L. Frydman, Prog. Nucl. Magn. Reson. Spectrosc., 2010, 57, 241–292.
66. S. Ahola and V.-V. Telkki, ChemPhysChem, 2014, 15, 1687–1692.
67. S. Ahola, V. Zhivonitko, O. Mankinen, G. Zhang, A. M. Kantola, H. Y. Chen, C. Hilty, I. V. Koptyug and V.-V. Telkki, Nat. Commun., 2015, 6, 8363.
68. O. Mankinen, V. Zhivonitko, A. Selent, S. Mailhiot, S. Komulainen, N. L. Prisle, S. Ahola and V.-V. Telkki, Nat. Commun., 2020, 11, 3251.
69. S. Ullah, O. Mankinen, V. Zhivonitko and V.-V. Telkki, Phys. Chem. Chem. Phys., 2022, 24, 22109–22114.
70. J. H. Ardenkjær-Larsen, B. Fridlund, A. Gram, G. Hansson, L. Hansson, M. H. Lerche, R. Servin, M. Thaning and K. Golman, Proc. Natl. Acad. Sci. U. S. A., 2003, 100, 10158.
71. S. R. Bowers, Sensitivity enhancement utilizing parahydrogen, in Encyclopedia of Nuclear Magnetic Resonance, John Wiley & Sons, Chichester, 2007.
72. R. W. Adams, J. A. Aguilar, K. D. Atkinson, M. J. Cowley, P. I. Elliott, S. B. Duckett, G. G. Green, I. G. Khazal, J. López-Serrano and D. C. Williamson, Science, 2009, 323, 1708–1711.
73. B. M. Goodson, J. Magn. Reson., 2002, 155, 157–216.
74. C. Qi, Y. Wang and C. Hilty, Angew. Chem., Int. Ed., 2021, 60, 24018–24021.

---

Footnote

† Electronic supplementary information (ESI) available: Pulse sequences for the T_1ρ experiments described. See DOI: https://doi.org/10.1039/d2cp05988f

---