Structural stability enables high thermoelectric performance in room temperature Ag2Se†
Abstract
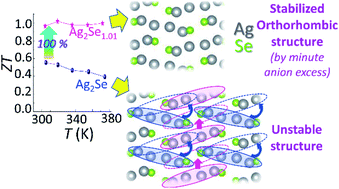
Ag2Se is considered as an attractive candidate for use in room-temperature thermoelectric applications owing to its unique transport properties, such as glass-like thermal conductivity and good electrical conductivity. However, understanding the correlation between composition (Ag/Se ratio), defect structure, and transport properties is an important prerequisite to optimize its figure of merit (ZT). Using in-depth microscopic analysis, this study reveals the coexistence of a metastable and the main orthorhombic crystal structure in stoichiometric Ag2Se. The formation of the metastable structure was found to be detrimental to the transport properties of bulk Ag2Se. We were able to successfully inhibit its formation and stabilize the main orthorhombic structure via small anion (Se and S) excess. The compositions Ag2SeChy (y ≤ 0.01; Ch = Se, S) yielded 40–70% rise in carrier mobility with a value of 2510 cm2 V−1 s−1 at 300 K and extremely low lattice-thermal-conductivity (0.2–0.1 W m−1 K−1 over 300–375 K). This combination of transport properties yielded a room-temperature power factor of 3.2 mW m−1 K−2 and a nearly flat ZT value of ∼1.0 over the 300–375 K temperature range. Additionally, a record-high conversion efficiency (ηmax) of 3.7% was theoretically obtained for single-leg Ag2Se for a small temperature gradient of ∼80 K.


 Please wait while we load your content...
Please wait while we load your content...