Open Access Article
Open Access ArticleGeometry underlies the mechanical stiffening and softening of an indented floating film†
Monica M.
Ripp
 ab,
Vincent
Démery
ab,
Vincent
Démery
 *cd,
Teng
Zhang
*cd,
Teng
Zhang
 *be and
Joseph D.
Paulsen
*be and
Joseph D.
Paulsen
 *ab
*ab
aDepartment of Physics, Syracuse University, Syracuse, NY 13244, USA. E-mail: jdpaulse@syr.edu
bBioInspired Syracuse: Institute for Material and Living Systems, Syracuse University, Syracuse, NY 13244, USA
cGulliver, CNRS, ESPCI Paris, PSL Research University, 10 rue Vauquelin, 75005 Paris, France. E-mail: vincent.demery@espci.psl.eu
dUniv Lyon, ENS de Lyon, Univ Claude Bernard Lyon 1, CNRS, Laboratoire de Physique, F-69342 Lyon, France
eDepartment of Mechanical and Aerospace Engineering, Syracuse University, Syracuse, NY 13244, USA. E-mail: tzhang48@syr.edu
First published on 31st March 2020
Abstract
A basic paradigm underlying the Hookean mechanics of amorphous, isotropic solids is that small deformations are proportional to the magnitude of external forces. However, slender bodies may undergo large deformations even under minute forces, leading to nonlinear responses rooted in purely geometric effects. Here we study the indentation of a polymer film on a liquid bath. Our experiments and simulations support a recently-predicted stiffening response [D. Vella and B. Davidovitch, Phys. Rev. E, 2018, 98, 013003], and we show that the system softens at large slopes, in agreement with our theory that addresses small and large deflections. We show how stiffening and softening emanate from nontrivial yet generic features of the stress and displacement fields.
1 Introduction
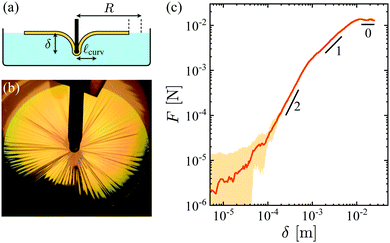
A major challenge in the mechanics of materials and structures is bridging the gap between a system's local material response and its global stiffness. This connection from microscopic to macroscopic scales is often complicated by subtle geometric effects.1 One conceptually simple example is a buckled elastic rod, which drastically changes its shape in response to loading. Such elastica problems captured the attention of Galileo, the Bernoullis, and Euler, and variations on them continue to fascinate and push our understanding of slender bodies today.2–5 Particular attention surrounds the behaviors of two-dimensional sheets, which may carry tensile loads in one direction while buckling or wrinkling in a perpendicular direction, leading to large anisotropies in the equilibrium stresses and deformations.6–10 Understanding such geometrically-nonlinear behaviors is important to a wide range of applications, from stretchable electronics11 to large-scale inflatable structures.12Here we study the indentation of a polymer film on a liquid bath [Fig. 1a and b] using experiments and simulations spanning four decades in indentation depth, and theory that addresses small and large deflections. This model system shows a remarkably rich response under continual loading—it first stiffens (i.e., F/δ increases) and then subsequently softens [Fig. 1c]. Whereas stiffening was predicted by a recent detailed theoretical study,13 softening occurs at large slopes where a direct analysis of the Föppl–von Kármán equations is prohibitive. We harness a simple geometric model that treats the sheet as inextensible but with zero bending cost,14 which allows us to understand both the force and the film profile at large amplitude.
Particular interest in the indentation of thin films surrounds the observation that wrinkles significantly modify the stress field in the sheet and the force transmitted to the indenter,13,15,16 which has practical importance for indentation assays used to measure film properties. Fundamental interest is bolstered by the recent discovery of a novel wrinkled morphology that is nearly isometric to the original undeformed state.15,17 Such “asymptotically isometric mechanics” arises in the dual limit of weak applied tension and vanishing bending modulus. Experiments on ultrathin polymer films measuring the extent of wrinkles,15 the vertical profile of the film,18 and the force response19 support this picture. Yet, apart from this particular regime, direct measurements of forces in wrinkled interfacial films are scarce. Here we confirm the entire predicted force evolution for an indented floating film,13,15 and we demonstrate an additional regime at large amplitude with two surprising features: A force plateau and a limiting interface shape that is independent of the indentation depth. Our results establish the maximal load-bearing capacity of a floating film under point loading, and our theoretical approach for small and large deflections can readily be adapted to different geometries and loading conditions. We close by highlighting analogous behaviors that arise from similar mechanisms in model fiber networks, due to a separation of energy scales between bending and stretching deformations that favors nearly-isometric wrinkled deformations in a sheet and the buckling of individual fibers in a network.
2 Model system
We work in a geometry previously investigated by Holmes and Crosby19 and Huang.20 We use spin-coated, ultrathin polystyrene sheets (thickness 58 < t < 490 nm, Young's modulus E = 3.4 GPa) of circular shape (radius 11 < R < 44 mm), that are floated on a liquid bath of density ρ = 1000 kg m−3 and surface tension γ = 72 mN m−1. We indent the films a vertical distance δ as illustrated in Fig. 1a, using a custom force probe mounted on a computer-controlled vertical translation stage, following ref. 19. To access an even larger dynamic range of indentation depths, we perform numerical simulations in the molecular dynamics software LAMMPS.21 We use a triangular lattice model for the sheet22 and springs with zero rest length for the liquid.4 This approach allows us to use parallel computing methods to address this inherently multiscale problem where the sheet thickness, wrinkle wavelength, and sheet radius occupy separate lengthscales.Our films fall in the doubly-asymptotic limit of weak tension, γ/Y < 10−3, and negligible bending stiffness, ε = Bρg/γ2 < 10−4, where Y = Et is the stretching modulus, B is the bending modulus, g is the gravitational acceleration, and ε is the inverse bendability.6 Such films can bare only a minute level of compression before buckling out of plane, and they exhibit an approximately linear stress–strain response under tensile loading, all the way up to fracture.23 We work in the regime R ≫ ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c, where
c, where 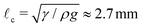 is the gravity–capillary length, which defines the lateral scale on which a bare liquid interface would be disturbed due to a point deflection.
is the gravity–capillary length, which defines the lateral scale on which a bare liquid interface would be disturbed due to a point deflection.
As the sheet is indented beyond a threshold δ, wrinkles form in an annular region and then expand radially towards the indenter and the edge of the film,15,18 eventually covering the film as in Fig. 1b. Fig. 1c shows the measured force versus displacement for an experiment with t = 213 nm and R = 44 mm. The data show a complex response with multiple distinct scaling regimes. At the largest δ probed, the force levels off to a constant value. For a bulk material, such a plateau would suggest plasticity or failure, but as we will show, here the coupling of the geometry of the sheet and the transmission of forces allows such a response within the Hookean regime of the material. At smaller δ the force F(δ) is linear in δ, but this is not the conventional linear response often obtained at infinitesimal amplitude. At yet smaller δ the force is markedly nonlinear and proportional to δ2. For δ ≲ 10−4 m, our experimental uncertainties are too large to extract a clear scaling; here our simulations are able to resolve a transition to an additional linear regime for very small δ [Fig. 4b]. We thus observe four distinct scaling regimes for the normal force.
This phenomenology is consistent with a recent detailed theoretical analysis of indentation,13 although that work was limited to small slopes and did not anticipate the force plateau at large δ. We begin by reviewing key results from previous theory and then develop a complementary theoretical approach that is valid for arbitrary slopes and explains the observed behavior at large amplitude.
3 Theory
3.1 Previous results
Progress on understanding the mechanical response of thin floating films to indentation has relied on a far-from-threshold (FT) framework that was developed in a series of recent works.6,24–26 This theory is based on the observation that for sufficiently thin films, out-of-plane buckling completely relaxes the compressive stress in one direction. In this framework, complex wrinkle patterns emerge from at least two distinct competitions: (i) in-plane stretching energy determines the extent of the wrinkled zone; (ii) inside this region, the wrinkle wavelength comes from balancing bending of the sheet with an effective substrate stiffness.18,27 (Studies of ordering on other mesoscopic lengthscales are ongoing.28)In the present problem, the indenter performs work that is transmitted to a combination of elastic stretching of the sheet, gravitational energy of the displaced fluid, and surface energy due to exposing liquid surface area as the sheet retracts radially inward [dashed lines in Fig. 1a]. At small δ, the indenter probes the stress state of the film, which is set by the interfacial tension pulling at its edge. Thus, in the incipient regime:
 | (1) |
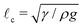 .‡
.‡
At larger δ the deflections in the sheet become nonlinear and the force departs from this scaling. Eventually, azimuthal stresses are compressive in an annular region, leading to the formation of wrinkles that qualitatively modify the global stress field. When wrinkles cover a finite fraction of the sheet, the force is predicted to be:
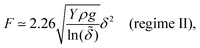 | (2) |
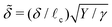 is a dimensionless indentation depth that compares geometric and mechanical strain.15 Note that eqn (2) is a stiffening response because the effective spring constant F/δ (hereafter called the stiffness) is an increasing function of δ.
is a dimensionless indentation depth that compares geometric and mechanical strain.15 Note that eqn (2) is a stiffening response because the effective spring constant F/δ (hereafter called the stiffness) is an increasing function of δ.
At still larger δ, wrinkles reach the edge of the sheet. This event gives rise to a so-called “asymptotic isometry” where elastic energies in the sheet become negligible. In this regime, the vertical profile of the sheet decays over an emergent lateral scale ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv =
curv = ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c2/3R1/3 to minimize the sum of gravitational and surface energies.15,18 A precise calculation yields:
c2/3R1/3 to minimize the sum of gravitational and surface energies.15,18 A precise calculation yields:
| F ≃ 4.58(γR)2/3(ρg)1/3δ (regime III). | (3) |
We note that regime I can be understood with standard linear response theory, whereas regime III arises from distinct geometric effects,13,15 yet the force is proportional to δ in both regimes.
The transitions between these regimes are governed by the evolution of the stresses in the film.13 A broad transition to regime II is predicted at indentation depth:
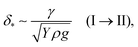 | (4) |
 | (5) |
With the exception of eqn (3), the above predictions have not been tested by experiments or simulations;§ we do so in Section 4. Moreover, the behaviors at large slopes are completely unexplored. As we will show, the force plateau at large amplitude constitutes another distinct scaling regime, which we define as regime IV.
3.2 Stress field and indentation force
Here we outline a set of general arguments based on force balance that are applicable to indentation at both small and large slopes. This treatment allows us to describe the fundamental mechanisms for the previously-predicted stiffening at small slopes,13 while elucidating a novel softening regime at large slopes.We consider axially-symmetric vertical deflections of the sheet. Radial force balance in the sheet reads: 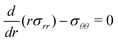 , where σrr and σθθ are the radial and azimuthal stress components, with the boundary condition σrr(R) = γ at the edge. This equation has two simple solutions: In the undeformed state, the stress field is uniform, corresponding to the first solution: σrr = σθθ = γ. At large indentation (regimes III and IV), radial wrinkles cover the entire sheet to avoid azimuthal compression that would have otherwise been induced by the contraction of circles, so that σθθ = 0.13 Thus, in the second solution:
, where σrr and σθθ are the radial and azimuthal stress components, with the boundary condition σrr(R) = γ at the edge. This equation has two simple solutions: In the undeformed state, the stress field is uniform, corresponding to the first solution: σrr = σθθ = γ. At large indentation (regimes III and IV), radial wrinkles cover the entire sheet to avoid azimuthal compression that would have otherwise been induced by the contraction of circles, so that σθθ = 0.13 Thus, in the second solution:
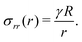 | (6) |
Fig. 2a shows the radial tensile stress σrr measured in our simulations. As δ increases, the data go away from the uniform solution towards the second solution that transmits larger stresses to the center of the sheet. This occurs as the hoop stress σθθ drops from γ towards 0 over the majority of the film [Fig. 2a, lower panel].
The radial tensile stress is linked to the normal force on the indenter, F, by vertical force balance:  , where α is the angle between the horizontal and the sheet at r = 0, as drawn in Fig. 2b. We denote the axisymmetric height of the sheet by ζ(r), which neglects the undulations due to small-amplitude wrinkles, so
, where α is the angle between the horizontal and the sheet at r = 0, as drawn in Fig. 2b. We denote the axisymmetric height of the sheet by ζ(r), which neglects the undulations due to small-amplitude wrinkles, so 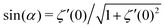 . In regimes III and IV we may use eqn (6) for the radial stress, leading to:
. In regimes III and IV we may use eqn (6) for the radial stress, leading to:
F = 2πRγ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/i_char_2009.gif) sin(α). sin(α). | (7) |
These basic considerations get at the essence of the observed stiffening and softening responses. Reduction of the hoop stress due to radial retraction leads to larger radial stresses; this growing anisotropy of stresses causes stiffening. Then at large slopes, the force saturates as sin(α) ≃ 1 [viaeqn (7)], which causes softening. To substantiate this qualitative picture, we now consider the response of the film in more detail, starting from small indentation.
3.3 Stiffening due to growing anisotropy of stresses
We begin by estimating the force on the indenter within the linear response of the system at infinitesimal indentation. Here the stress in the film is given by the simple isotropic solution, namely, σrr = σθθ = γ. We suppose that the indenter will deform a finite region of the sheet, so that the stress and displacement are modified only within a core of some finite radius![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) (where it is natural to assume that
(where it is natural to assume that ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) ∝
∝ ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c since there is no other available length). The force transmitted to the indenter is then estimated by 2π
c since there is no other available length). The force transmitted to the indenter is then estimated by 2π![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) γ
γ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/i_char_2009.gif) sin(α), in analogy with eqn (7) but with sin(α) ≃ δ/
sin(α), in analogy with eqn (7) but with sin(α) ≃ δ/![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) . Thus, in the initial regime, the force on the indenter should scale as F ∼ γδ, independent of
. Thus, in the initial regime, the force on the indenter should scale as F ∼ γδ, independent of ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) so long as
so long as ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) < R. This scaling is in agreement with eqn (1).
< R. This scaling is in agreement with eqn (1).
Intuitively, moving from an approximately uniform stress field¶ to an increasingly anisotropic stress field causes the observed stiffening, as forces are transmitted more effectively to the indenter in the latter case. This qualitative picture is supported by a recent far-from-threshold analysis of the Föppl–von Kármán equations,13,15 summarized above. At larger δ, wrinkles reach the edge of the sheet, giving rise to a so-called “asymptotic isometry”13,15 where elastic energies in the sheet become negligible.
3.4 Softening due to large slopes
We now show how large slopes give rise to a previously-unanticipated softening response. We begin by considering the case where wrinkles cover the sheet, yet the vertical deflections are still within the small-slopes limit. Under these conditions, the vertical profile is given by: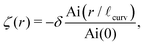 | (8) |
![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv =
curv = ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c2/3R1/3. This profile may be derived by solving for the stresses and deflections everywhere in the sheet15 or by minimizing the surface and gravitational energy using the geometric model described in Section 3.5. Using this result, the slope at the origin is ζ′(0) = −(δ/
c2/3R1/3. This profile may be derived by solving for the stresses and deflections everywhere in the sheet15 or by minimizing the surface and gravitational energy using the geometric model described in Section 3.5. Using this result, the slope at the origin is ζ′(0) = −(δ/![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) curv)Ai′(0)/Ai(0) ≃ 0.729(δ/
curv)Ai′(0)/Ai(0) ≃ 0.729(δ/![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) curv), so that small slopes are obtained when δ ≪
curv), so that small slopes are obtained when δ ≪ ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv. Eqn (7) for the force then reduces to:
curv. Eqn (7) for the force then reduces to:| F = 2πRγζ′(0) ≃ 4.58(γR)2/3(ρg)1/3δ (regime III). | (9) |
Our approach can also address large slopes, which correspond to δ ≳ ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv. The asymptotic force is obtained by setting sin(α) = 1 in eqn (7), yielding:
curv. The asymptotic force is obtained by setting sin(α) = 1 in eqn (7), yielding:
| F = 2πRγ (regime IV). | (10) |
This is a softening response because the stiffness, F/δ, is a decreasing function of δ. The crossover occurs when the expressions (3) and (10) are comparable:
δ*** ≃ 1.37![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv (III → IV). curv (III → IV). | (11) |
Interestingly, eqn (10) for the force in regime IV is the same maximal force that a rigid disc of radius R can support before sinking29—despite the drastically different interfacial geometries they produce, neither is significantly better at staying afloat.
3.5 Geometric model and localization transition
Here we show that in regimes III and IV, the profile ζ(r) can be obtained from purely geometric arguments. In regime III, this approach recovers the profile found in ref. 13; in regime IV, we show that softening is accompanied by localization of ζ(r).We treat the sheet as inextensible but with zero bending cost. Such a treatment was used to explain the wrapping of a droplet in a thin sheet14 and the folding of an annular sheet submitted to different inner and outer surface tensions,30 and it is motivated by the weak lateral tension and negligible bending stiffness of these films (γ/Y → 0 and ε → 0). In this asymptotic regime, the only relevant energies are due to gravity and surface tension:
| U = Ugravity + γ(ΔAfree) | (12) |
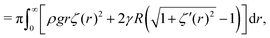 | (13) |
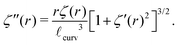 | (14) |
In the small-slope limit, eqn (14) reduces to ζ′′(r) = rζ(r)/![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) curv3, which is solved by an Airy function [see eqn (8) above]. For arbitrary slope we solve eqn (14) numerically, yielding the height profiles shown in Fig. 3. At large indentation, ζ(r) becomes increasingly localized. (One way to see this is observing the deflection at r/
curv3, which is solved by an Airy function [see eqn (8) above]. For arbitrary slope we solve eqn (14) numerically, yielding the height profiles shown in Fig. 3. At large indentation, ζ(r) becomes increasingly localized. (One way to see this is observing the deflection at r/![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv = 1, which saturates for large δ.) Moreover, the profiles appear to approach a universal curve as δ increases. By analyzing the asymptotic behavior of eqn (14), we find that ζ(r) ∼ −[3
curv = 1, which saturates for large δ.) Moreover, the profiles appear to approach a universal curve as δ increases. By analyzing the asymptotic behavior of eqn (14), we find that ζ(r) ∼ −[3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) log(1/r)/2]2/3 as r → 0, in good agreement with the numerical solution (see Fig. S1, ESI†). As the profile approaches this curve, the volume of fluid lifted by the sheet reaches a plateau, so that the gravitational energy eventually becomes negligible compared to surface energy. The total energy at large amplitude is thus U ∼ 2πγRδ, which accounts for the interfacial area of the bath that is exposed as the boundary of the film is pulled inwards. This energy scaling recovers eqn (10) for the force in regime IV.
log(1/r)/2]2/3 as r → 0, in good agreement with the numerical solution (see Fig. S1, ESI†). As the profile approaches this curve, the volume of fluid lifted by the sheet reaches a plateau, so that the gravitational energy eventually becomes negligible compared to surface energy. The total energy at large amplitude is thus U ∼ 2πγRδ, which accounts for the interfacial area of the bath that is exposed as the boundary of the film is pulled inwards. This energy scaling recovers eqn (10) for the force in regime IV.
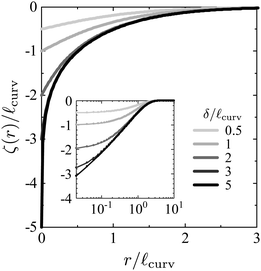 | ||
| Fig. 3 Localization at large slopes. Vertical profile of the film, ζ(r), computed by numerically minimizing eqn (13). The profile becomes increasingly localized at large δ. Inset: Same curves on semilogarithmic axes, showing the approach to a universal curve. | ||
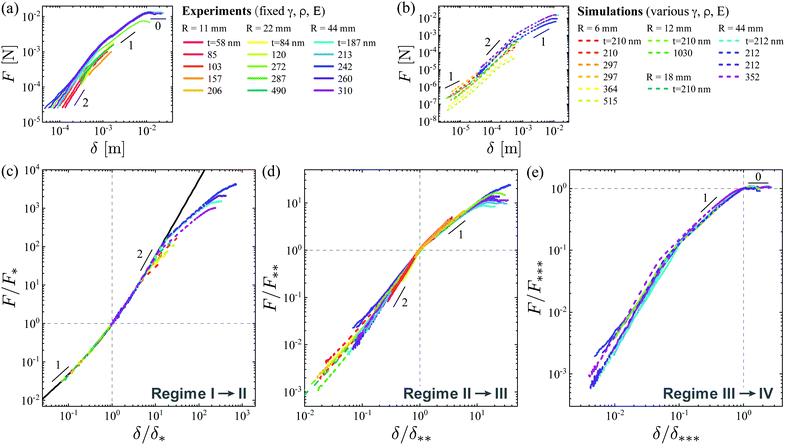 | ||
Fig. 4 Normal force measurements showing four scaling regimes. (a and b) Force versus indentation depth for (a) experiments and (b) simulations at several film radii and a wide range of thicknesses. (Values of γ, ρ, and E are listed in the legend to Fig. 5.) (c) The data at small indentation (simulation curves) are collapsed by rescaling the vertical and horizontal axes by δ* and F* for each film. The data are described well by the empirical form: 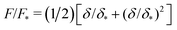 (black line), until they peel away at large δ. (d) A different rescaling by δ** and F** collapses the data at larger indentation in simulations and experiments. (e) A transition to a plateau in the force occurs at δ*** in simulations and experiments. (black line), until they peel away at large δ. (d) A different rescaling by δ** and F** collapses the data at larger indentation in simulations and experiments. (e) A transition to a plateau in the force occurs at δ*** in simulations and experiments. | ||
 | ||
Fig. 5 Comparison between experiments, simulations, and theory for the magnitude of the force in regimes I–IV (a–d) and the transitions between the regimes (e–g). We varied the sheet and liquid parameters over a wide range, as shown in the legend. Panels (a) and (e) include only simulations; all other panels include simulations and experiments. In all panels, the solid lines show the theoretical predictions given in the main text with no free parameters [eqn (1)–(5) from ref. 13 and 15 and eqn (10)–(11) predicted by our work]. The prediction for panel (b) was computed using ![[small delta, Greek, tilde]](https://www.rsc.org/images/entities/i_char_e125.gif) = 6, which is in the middle of the range of = 6, which is in the middle of the range of ![[small delta, Greek, tilde]](https://www.rsc.org/images/entities/i_char_e125.gif) for the measurements. The star in panel (c) shows the value obtained from previous experiments in regime III, reported in ref. 19. The dashed lines in panel (e) show eqn (4) with the lowest and highest theoretically-predicted prefactors for the simulation parameters used here. The inset to panel (e) shows the dimensionless indentation depth for the measurements. The star in panel (c) shows the value obtained from previous experiments in regime III, reported in ref. 19. The dashed lines in panel (e) show eqn (4) with the lowest and highest theoretically-predicted prefactors for the simulation parameters used here. The inset to panel (e) shows the dimensionless indentation depth 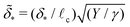 versus bendability, ε−1 = γ2/(Bρg), and the solid line connects points predicted by ref. 13. versus bendability, ε−1 = γ2/(Bρg), and the solid line connects points predicted by ref. 13. | ||
4 Comparison to experiments and simulations
We show the measured force curves in experiments and simulations in Fig. 4a and b, respectively, where the properties of the film and bath were varied while staying in the highly bendable yet inextensible limit (ε < 10−3 and γ/Y < 10−3). We varied the radius and thickness of the film over a wide range, as shown in the legend. In the simulations, we additionally varied the Young's modulus of the film (0.05 < E < 3.4 GPa) as well as the liquid density (909 < ρ < 4545 kg m−3) and surface tension (3.9 < γ < 77 mN m−1).4.1 Collapsing the force curves
The data over a wide range of parameters may be organized into four distinct scaling regimes, which correspond to regimes I–IV described above. At small δ we can collapse the data by rescaling the axes, δ → δ/δ* and F → F/F* as shown in Fig. 4c, where δ* and F* are selected for each curve to produce the best collapse. Here we rely solely on the simulations to characterize the normal force, as the experiments could not definitively resolve the incipient regime. We find an excellent fit to the empirical form: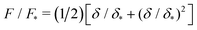 , consistent with a broad transition from F ∝ δ to F ∝ δ2 with increasing δ. (There is at present no theoretical explanation for this particular form of the crossover between these two regimes.)
, consistent with a broad transition from F ∝ δ to F ∝ δ2 with increasing δ. (There is at present no theoretical explanation for this particular form of the crossover between these two regimes.)
At intermediate δ, we observe a transition from F ∝ δ2 to F ∝ δ in the simulations and experiments, which coincides with wrinkles reaching the edge of the sheet [Fig. 6]. We can collapse all the data in the neighborhood of this transition at δ** by rescaling the axes, δ → δ/δ** and F → F/F**, as shown in Fig. 4d. At larger δ, the force reaches a plateau; we collapse the curves around this third transition by selecting δ*** and F*** for each curve [Fig. 4e].
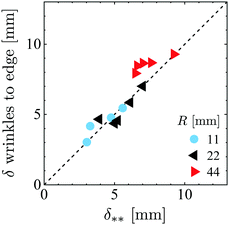 | ||
| Fig. 6 Morphological transition marking the start of regime III. Indentation depth where wrinkles reach the edge of the film, versus δ** extracted from the force measurements. These two transitions are found to coincide, as predicted in ref. 13 and shown by the dashed line with slope 1. Data are from experiments using three different film radii. | ||
4.2 Magnitude of the force
This observed sequence of scalings of the normal force with δ follows the theoretically-predicted progression given by regimes I–IV [eqn (1)–(3) from ref. 13, 15 and eqn (10) from the present work]. We now show that the data also follow the predicted dependence of the force on the other system parameters, namely, R, t, E, γ, and ρ. To reveal this dependence, in Fig. 5(a–d) we plot the magnitudes of F/δ, F/δ2, F/δ, and F measured in regimes I–IV, respectively, which were obtained by measuring the coefficients of these scalings for each sheet. (We also include the value measured by ref. 19 in regime III.) We are able to resolve regimes II–IV in experiments, and we find reasonable agreement with the theoretical predictions with no free parameters (solid lines). The experimental data in regime II show a systematically lower coefficient than the prediction, whereas the agreement in regime III is excellent. We note that in regime IV, the sheet contacts itself over an appreciable area, due to the formation of radial folds. Because of this additional adhesion energy that we do not include in the geometric model, we expect the predictions to overestimate the indentation force at large δ, in agreement with observations.Our simulations greatly expand the range of the tested parameters, as they allow us to vary γ and ρ over a wide range. The simulations can also resolve regime I and measure its coefficient [Fig. 5a]. The data are in reasonable agreement with the prediction, eqn (1), but with a somewhat higher numerical prefactor. The coefficient in regime II is again lower than the prediction, although the simulation data suggest that the discrepancy is limited to the numerical prefactor in eqn (2), as the open symbols are shifted down by a constant factor from the solid curve. We again find excellent agreement with the prediction in regime III, and good agreement in regime IV.
4.3 Transitions between the regimes
Fig. 5e shows the indentation depth δ* where we observe the transition from regime I to II, obtained by collapsing the simulation data in Fig. 4c. To compare with theory, we note that ref. 13 predicted a numerical prefactor for the scaling in eqn (4) that varies slowly with the bendability, ε−1. For the physical parameters used here, this prefactor varies from 1.25 to 1.6. We show this range with a purple band. The data are in reasonable agreement with the prediction. To further examine the variation of the numerical prefactor in eqn (4), the inset to Fig. 5e shows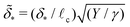 versus the bendability, ε−1. The measurements are systematically higher than the prediction obtained from ref. 13, which has no free parameters. Both show a weak dependence on ε−1.
versus the bendability, ε−1. The measurements are systematically higher than the prediction obtained from ref. 13, which has no free parameters. Both show a weak dependence on ε−1.
Fig. 5f shows the measured indentation depth δ**, obtained by collapsing the data in Fig. 4d. The data are in good agreement with the theoretical prediction, eqn (5), over a wide range of parameters. This transition into regime III is predicted to be brought on by the change in the stresses when wrinkles reach the edge of the film,13 leading to the eqn (6) for σrr(r). Our experiments provide simultaneous optical, force, and depression measurements, so we can test this scenario directly. Fig. 6 compares the indentation depth where wrinkles first reach the edge of the film, with the indentation depth where we see a crossover from a quadratic to a linear scaling in the normal force, i.e., δ = δ**. The data follow the dashed line of slope 1, indicating that these two events indeed coincide.
Fig. 5g shows the measured transition depth δ*** marking the onset of regime IV, obtained by collapsing the data in Fig. 4e. Only a subset of our experiments and simulations were carried out to large amplitude, so we have fewer measurements of this transition. Nevertheless, we find good agreement between experiments, simulations, and our theory with no fitting parameters [eqn (11)].
Fig. 7 assembles regimes I–III into a phase diagram using the dimensionless parameters 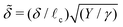 and
and ![[scr R, script letter R]](https://www.rsc.org/images/entities/char_e531.gif) = R/
= R/![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c. Regime IV is not included in the diagram, because the III → IV transition is determined by a different dimensionless group,
c. Regime IV is not included in the diagram, because the III → IV transition is determined by a different dimensionless group, 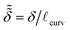 . Nevertheless, our predictions imply that δ*** > δ** as long as Y/γ ≫ (R/
. Nevertheless, our predictions imply that δ*** > δ** as long as Y/γ ≫ (R/![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) c)2/3, which is the case in our studies (as well as most experimentally-accessible scenarios in the inextensible regime, Y/γ ≫ 1).
c)2/3, which is the case in our studies (as well as most experimentally-accessible scenarios in the inextensible regime, Y/γ ≫ 1).
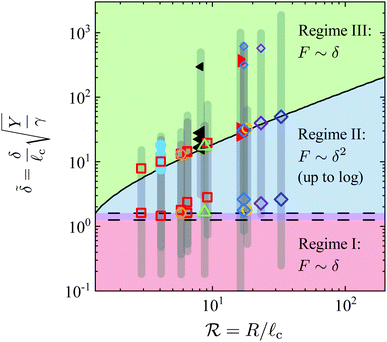 | ||
| Fig. 7 Phase diagram for regimes I–III. Dashed lines: Phase boundary for the I → II transition, given by eqn (4) for δ* with numerical prefactors of 1.25 and 1.6, corresponding to the range of ε−1 in the data. Solid line: Phase boundary for the II → III transition, given by eqn (5) for δ**. The predictions are supported by our experiments (filled symbols) and simulations (open symbols) over a wide range of parameters (see Fig. 5 for symbol legend). Small symbols mark the observed transition into regime IV. | ||
4.4 Softening and localization
To examine regime IV in more detail, Fig. 8a shows F(δ) on linear axes for a set of experiments with R = 44 mm. At large displacement, the force reaches a plateau value that shows no trend with sheet thickness. We mark the predicted transition at δ*** ≃ 9.4 mm with a dashed line, which is in excellent agreement with the data. The force is predicted to saturate at ∼20 mN from eqn (10); this is the order of magnitude of the observed plateau, but other effects such as folding19 should play a role in determining the value of the saturation force, as discussed above.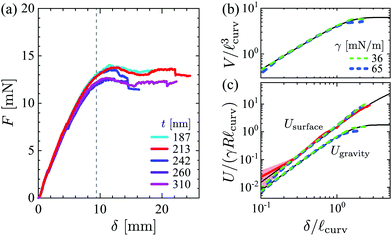 | ||
Fig. 8 Softening and localization at large slopes. (a) Normal force versus displacement in experiments with R = 44 mm and a range of thicknesses. At large δ, the force reaches a plateau that shows no trend with thickness. This transition to regime IV is captured by eqn (11) for δ*** (dashed line). (b) The volume of the fluid that is lifted by the film, V, plateaus to a value proportional to ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv3. Solid line: Axisymmetric theory. Dashed lines: Simulations with t = 212 nm, R = 44 mm, and two different values of surface tension. (c) Surface energy becomes dominant at large δ when the lifted volume saturates. The axisymmetric predictions for Usurface and Ugravity (black curves) are in excellent agreement with our simulations (dashed lines) and experiments (solid red line: t = 213 nm, R = 44 mm). curv3. Solid line: Axisymmetric theory. Dashed lines: Simulations with t = 212 nm, R = 44 mm, and two different values of surface tension. (c) Surface energy becomes dominant at large δ when the lifted volume saturates. The axisymmetric predictions for Usurface and Ugravity (black curves) are in excellent agreement with our simulations (dashed lines) and experiments (solid red line: t = 213 nm, R = 44 mm). | ||
Fig. 8b shows the volume displaced by the film in two simulations carried out to large amplitude with differing values of the surface tension. The curves are collapsed by plotting the volume scaled by ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv3 and the indentation depth scaled by
curv3 and the indentation depth scaled by ![[small script l]](https://www.rsc.org/images/entities/char_e146.gif) curv. The quantitative evolution is described extremely well by our geometric model, including the plateau predicted at large δ, which results from the localization of the vertical profile at large amplitude. As the displaced volume saturates, our theory predicts that the surface energy, Usurface = γ(ΔAfree), becomes dominant over the gravitational energy, Ugravity defined in eqn (12) and (13). This progression is supported by Fig. 8c, where we show Usurface and Ugravity measured in the same simulations, in agreement with our theory. We also obtain Usurface in experiment by measuring the shape of the boundary of the sheet in top-view images, further corroborating this picture.
curv. The quantitative evolution is described extremely well by our geometric model, including the plateau predicted at large δ, which results from the localization of the vertical profile at large amplitude. As the displaced volume saturates, our theory predicts that the surface energy, Usurface = γ(ΔAfree), becomes dominant over the gravitational energy, Ugravity defined in eqn (12) and (13). This progression is supported by Fig. 8c, where we show Usurface and Ugravity measured in the same simulations, in agreement with our theory. We also obtain Usurface in experiment by measuring the shape of the boundary of the sheet in top-view images, further corroborating this picture.
4.5 Crumples and folds
Two additional morphologies are observed in the experiments: stress-focusing “crumples” that consist of repeated buckled structures terminating at sharp tips,31,32 and radial folds where the film contacts itself.19 Remarkably, crumples do not affect the force on the probe (see Fig. S5, ESI†), whereas a fold elicits a small drop in the force. According to the geometric model, crumples should only affect the force on the probe if they alter the gross shape of the film from the optimal axisymmetric shape. In contrast, folds may contribute an additional energy due to self-contact, which is beyond the scope of this work.5 Conclusion
Despite the simplicity of the poking protocol, we have shown that it gives rise to two distinct geometric nonlinearities. Stiffening is controlled by the dimensionless number: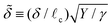 , which compares geometry-induced strain to the mechanical strain imposed by surface tension, as predicted by ref. 13. Our results at large amplitude have identified a softening response that is controlled by the dimensionless number:
, which compares geometry-induced strain to the mechanical strain imposed by surface tension, as predicted by ref. 13. Our results at large amplitude have identified a softening response that is controlled by the dimensionless number: 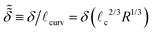 , which approximates the maximal slope of the sheet. Fig. 9 summarizes our results by showing the evolution of the stiffness, F/δ, using simulation, experiment, and theory, for a film with t = 213 nm. The stiffness may be seen transitioning through the four regimes as the indentation depth is increased.
, which approximates the maximal slope of the sheet. Fig. 9 summarizes our results by showing the evolution of the stiffness, F/δ, using simulation, experiment, and theory, for a film with t = 213 nm. The stiffness may be seen transitioning through the four regimes as the indentation depth is increased.
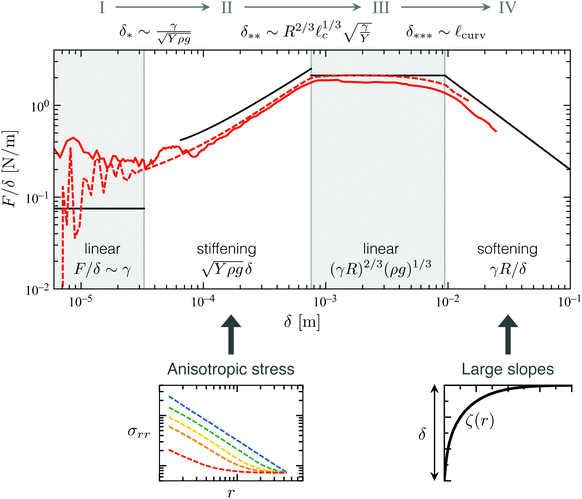 | ||
| Fig. 9 Stiffening and softening of a single film. Stiffness, F/δ, versus displacement, measured in experiment (solid red line) and simulation (dashed line) for R = 44 mm, t = 213 nm, E = 3.4 GPa, γ = 72 mN m−1, and ρ = 1000 kg m−3. Previous work13,15 predicted the stiffness in regimes I–III via the Föppl–von Kármán equations [black lines given by eqn (1)–(3)]. Our geometric model reproduces the result in regime III and predicts a new regime with softening behavior [rightmost black line: eqn (10)]. Vertical lines show values of δ*, δ** predicted by ref. 13 [eqn (4) and (5)] and δ*** predicted by our work [eqn (11)]. The figure contains no adjustable parameters. As a reference, scalings for F/δ and transitions are written up to logarithmic corrections. | ||
The mechanism for the observed stiffening is the growing anisotropy of stresses in the sheet as loading progresses. The resulting hoop compression eventually leads to wrinkles, which for very thin films cause the compressive stresses in the azimuthal direction to vanish, (σθθ = 0), so that radial forces are passed directly through the wrinkled region, rather than being carried evenly by the radial and hoop components. This leads to a long-range decay of the radial stress, σrr(r) ∝ 1/r. The basic physical ingredients leading to this enhanced force propagation are quite modest, so that similar behaviors occur in other materials. For instance, the same stress field arises in the mechanics of fiber networks, which make up paper, textiles, and the structural components of tissues and cells.33 There, the buckling of fibers sets a threshold for the maximum compressive stresses that may be endured, which is a natural analogue to the formation of wrinkles in thin sheets subjected to small compressional loads. This applies to both two- and three-dimensional networks; in d dimensions, eqn (6) becomes σrr(r) ∝ r−(d−1).34 (The stress field for forcing at multiple sites does not obey superposition, so the behavior will differ at high density of forcing sites.35)
Softening is not related to the stress field but rather to the gross shape—it arises when there are large deviations from the original slopes on the surface of the object. Seen in this way, one may identify similarities with other interfacial problems. For instance, when a thin film is laterally compressed on a liquid bath, the confining force softens as it undergoes a wrinkle-to-fold transition with large slopes.36,37 The energy functional [eqn (13)] is qualitatively similar to that of a liquid meniscus in 1D and 2D, where the profile also localizes at large displacements.38
Recent work has proposed using elastic sheets to tailor the mechanical, chemical, or optical properties of droplets and interfaces,39 in analogy with molecular or particulate surfactants.40 Here we have shown how a simple geometric model—originally developed to understand shape selection14,30—can also predict forces, including at large displacements where conventional approaches may fail. In particular, eqn (12) may be used to determine the energy-minimizing configurations of a sheet as a function of a continuously-varying control parameter (here, the indentation depth δ), which then determines the force. This general approach is suitable for other forms of loading such as displacements applied at the edge of a sheet, and it applies to intrinsically curved sheets and curved liquid surfaces. This versatile method thus opens the way for understanding the mechanics of sheet-laden interfaces in general settings.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
We are grateful to Benny Davidovitch and Dominic Vella. We thank Douglas Holmes for sharing his force probe design. Simulations were performed at the Triton Shared Computing Cluster at the San Diego Supercomputer Center and the Comet cluster (Award no. TG-MSS170004 to T. Z.) in the Extreme Science and Engineering Discovery Environment. Funding support from National Science Foundation Grants No. IGERT-1068780 (M. M. R.), No. DMR-CAREER-1654102 (M. M. R. and J. D. P.), and No. CMMI-CAREER-1847149 (T. Z.) is gratefully acknowledged.Notes and references
- J. D. Paulsen, Annu. Rev. Condens. Matter Phys., 2019, 10, 431–450 CrossRef CAS.
- J. E. Flaherty, J. B. Keller and S. Rubinow, SIAM J. Appl. Math., 1972, 23, 446–455 CrossRef.
- B. Audoly and Y. Pomeau, Elasticity and geometry: from hair curls to the nonlinear response of shells, Oxford University Press, 2010 Search PubMed.
- L. Giomi and L. Mahadevan, Proc. R. Soc. London, Ser. A, 2012, 1851–1864 CrossRef.
- B. Davidovitch, Y. Sun and G. M. Grason, Proc. Natl. Acad. Sci. U. S. A., 2019, 116, 1483–1488 CrossRef CAS PubMed.
- B. Davidovitch, R. D. Schroll, D. Vella, M. Adda-Bedia and E. A. Cerda, Proc. Natl. Acad. Sci. U. S. A., 2011, 108, 18227–18232 CrossRef CAS PubMed.
- B. Li, Y.-P. Cao, X.-Q. Feng and H. Gao, Soft Matter, 2012, 8, 5728–5745 RSC.
- J. Hure, B. Roman and J. Bico, Phys. Rev. Lett., 2012, 109, 054302 CrossRef PubMed.
- P. Bella and R. V. Kohn, Communications on Pure and Applied Mathematics, 2014, 67, 693–747 CrossRef.
- J. Chopin and T. Romildo Filho, Phys. Rev. E, 2019, 99, 043002 CrossRef CAS PubMed.
- J. A. Rogers, T. Someya and Y. Huang, Science, 2010, 327, 1603–1607 CrossRef CAS PubMed.
- M. Pagitz, Philos. Trans. R. Soc., A, 2007, 3003–3017 CrossRef PubMed.
- D. Vella and B. Davidovitch, Phys. Rev. E, 2018, 98, 013003 CrossRef CAS PubMed.
- J. D. Paulsen, V. Démery, C. D. Santangelo, T. P. Russell, B. Davidovitch and N. Menon, Nat. Mater., 2015, 14, 1206–1209 CrossRef CAS PubMed.
- D. Vella, J. Huang, N. Menon, T. P. Russell and B. Davidovitch, Phys. Rev. Lett., 2015, 114, 014301 CrossRef PubMed.
- D. Vella and B. Davidovitch, Soft Matter, 2017, 13, 2264–2278 RSC.
- D. Vella, H. Ebrahimi, A. Vaziri and B. Davidovitch, EPL, 2015, 112, 24007 CrossRef.
- J. D. Paulsen, E. Hohlfeld, H. King, J. Huang, Z. Qiu, T. P. Russell, N. Menon, D. Vella and B. Davidovitch, Proc. Natl. Acad. Sci. U. S. A., 2016, 113, 1144–1149 CrossRef CAS PubMed.
- D. P. Holmes and A. J. Crosby, Phys. Rev. Lett., 2010, 105, 038303 CrossRef PubMed.
- J. Huang, PhD thesis, University of Massachusetts, Amherst, 2010.
- S. Plimpton, J. Comput. Phys., 1995, 117, 1–19 CrossRef CAS.
- H. Seung and D. R. Nelson, Phys. Rev. A: At., Mol., Opt. Phys., 1988, 38, 1005 CrossRef PubMed.
- Y. Liu, Y.-C. Chen, S. Hutchens, J. Lawrence, T. Emrick and A. J. Crosby, Macromolecules, 2015, 48, 6534–6540 CrossRef CAS.
- B. Davidovitch, R. Schroll and E. Cerda, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2012, 85, 066115 CrossRef CAS PubMed.
- R. Schroll, M. Adda-Bedia, E. Cerda, J. Huang, N. Menon, T. Russell, K. Toga, D. Vella and B. Davidovitch, Phys. Rev. Lett., 2013, 111, 014301 CrossRef CAS PubMed.
- J. Chopin, V. Démery and B. Davidovitch, J. Elasticity, 2015, 119, 137–189 CrossRef.
- E. Cerda and L. Mahadevan, Phys. Rev. Lett., 2003, 90, 074302 CrossRef CAS PubMed.
- O. Tovkach, J. Chen, M. M. Ripp, T. Zhang, J. D. Paulsen and B. Davidovitch, Proc. Natl. Acad. Sci. U. S. A., 2020, 117, 3938–3943 CrossRef CAS PubMed.
- D. Vella, Annu. Rev. Fluid Mech., 2015, 47, 115–135 CrossRef.
- J. D. Paulsen, V. Démery, K. B. Toga, Z. Qiu, T. P. Russell, B. Davidovitch and N. Menon, Phys. Rev. Lett., 2017, 118, 048004 CrossRef PubMed.
- H. King, R. D. Schroll, B. Davidovitch and N. Menon, Proc. Natl. Acad. Sci. U. S. A., 2012, 109, 9716–9720 CrossRef CAS PubMed.
- Y. Timounay, R. De, J. L. Stelzel, Z. S. Schrecengost, M. M. Ripp and J. D. Paulsen, Phys. Rev. X, 2020, accepted.
- D. A. Fletcher and R. D. Mullins, Nature, 2010, 463, 485 CrossRef CAS PubMed.
- P. Rosakis, J. Notbohm and G. Ravichandran, J. Mech. Phys. Solids, 2015, 85, 16–32 CrossRef.
- P. Ronceray, C. P. Broedersz and M. Lenz, Proc. Natl. Acad. Sci. U. S. A., 2016, 113, 2827–2832 CrossRef CAS PubMed.
- L. Pocivavsek, R. Dellsy, A. Kern, S. Johnson, B. Lin, K. Y. C. Lee and E. Cerda, Science, 2008, 320, 912–916 CrossRef CAS PubMed.
- V. Démery, B. Davidovitch and C. D. Santangelo, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2014, 90, 042401 CrossRef PubMed.
- M. L. Anderson, A. P. Bassom and N. Fowkes, Proc. R. Soc. London, Ser. A, 2006, 3645–3656 CrossRef.
- D. Kumar, J. D. Paulsen, T. P. Russell and N. Menon, Science, 2018, 359, 775–778 CrossRef CAS PubMed.
- B. P. Binks, Curr. Opin. Colloid Interface Sci., 2002, 7, 21–41 CrossRef CAS.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/d0sm00250j |
| ‡ There are logarithmic corrections to eqn (1) for a finite-size indenter; see for example ref. 16. |
| § Eqn (3) was compared with the experiments of Holmes & Crosby19 in the ESI to ref. 15. |
| ¶ In the approximately uniform solution, there is nevertheless a stretched core region where the stress is transmitted to the indenter.13 |
| This journal is © The Royal Society of Chemistry 2020 |