Nanostructured tungsten sulfides: insights into precursor decomposition and the microstructure using X-ray scattering methods†
Abstract
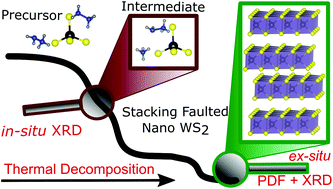
Herein we present an in-depth study of precursor derived tungsten sulfides, with a focus on their micro- and local structures. We prepared a new tetrathiotungstate based precursor (N2H5)2WS4 and unveiled the details of its unique decomposition mechanism by a combination of in situ and ex situ analytical techniques. Upon heating the precursor, a new compound with composition (NH4)(N2H5)WS4 is formed by the decomposition of one hydrazinium molecule. Above ∼190 °C, (NH4)2WS4 crystallized as the second crystalline intermediate followed by successive decomposition to WS2via amorphous WS3 upon increasing the temperature. Using X-ray diffraction, total scattering data and pair distribution function (PDF) analyses we are able to develop a detailed picture of the microstructure of nanosized WS2 samples obtained by the thermal decomposition of the precursor. The microstructure is described by global optimization of the stacking pattern of WS2 slabs in a supercell containing a large number of layers. The results clearly demonstrate that both stacking faults and random shifts of the WS2 layers contribute to the disorder in the material. This is significantly distinct to bulk materials, where solely stacking faults with no turbostratic disorder components were found.


 Please wait while we load your content...
Please wait while we load your content...