A systematic examination of classical and multi-center bonding in heteroborane clusters

Abstract

This paper presents a systematic study of multicenter and classical bonding on a broad series of experimentally known heteroboranes covering closo, nido, arachno and hypho types of cages with incorporated tetrel, pnictogen or chalcogen heterovertices up to the third-row elements. The nature of bonding is studied using a novel quantum-chemical tool, the intrinsic atomic/bond orbital (IAO/IBO) approach, which provides a direct connection between quantum chemistry and chemical concepts. We also discuss how the computed IBO properties are related to molecular observables such as interatomic distances, molecular electrostatic potential surfaces and dipole moments.

---

Introduction

Bonding in boron clusters (boron hydrides, boranes) is predominantly of multicenter nature, and usually referred to as 3-center-2-electron (3c2e) bonding.^1–4 Two types of 3c2e bonds, B–B–B and B–H–B triangles, occur in boranes. The bridging B–H–B triangles of B₂H₆ led W. N. Lipscomb to formulate the concept of multicenter bonding. The Nobel Prize was awarded to him for the explanation of the differences between boranes and analogous organic compounds. The geometries of boranes and hydrocarbons are not compatible even in the case of simple B₂H₆ and C₂H₆ molecules. B₂H₆ with D_2h symmetry differs from the ethane structure with D_3d symmetry. Conceivably, B₂H₆ could be fitted to the electron diffraction data only when a hydrogen-bridge-based model of D_2h symmetry is considered.⁵

Multicenter bonding is not limited to boranes. It has been shown by using high-angle X-ray diffraction that the bonding in the trimethylaluminium dimer (Al₂Me₆) has multicenter character.⁶ Multicenter bonding was also proposed for heteroborane clusters. W. N. Lipscomb et al. studied the nature of bonding in closo-carboranes at the semiempirical quantum mechanical level (the partial retention of diatomic differential overlap/PRDDO/method⁷).⁸ In Lipscomb's classical view of 3c2e bonding, n atomic orbitals are combined into 3c2e bonds by forming only n/3 bonding orbitals. Extra electrons would have to occupy antibonding orbitals. A valence pattern for a given number of atoms is thus restricted to a few well-defined possibilities. Further attention has been paid to smaller cages such as closo-1,2-Y₂B₃H₅ (Y = CH, N, P), where it has been known that both 3c2e and 2c2e alternatives are possible.⁹ Since the effort to study the bonding of heteroroboranes has focused mainly on smaller closo-carboranes, the knowledge of bonding in other heteroborane architectures is very limited.

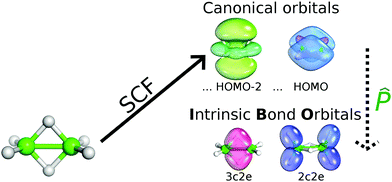
Recently, it has also been possible to analyze bonding patterns using a novel quantum-chemical tool, the intrinsic atomic/bond orbital (IAO/IBO) method.¹⁰ This method provides a direct connection between quantum chemistry and intuitive chemical concepts. It helps in determining the nature of chemical bonding from first principles calculations and computes the nature and shape of chemical bonds in terms of connecting quantitative SCF wave functions to a qualitative chemical picture (see Scheme 1).

Scheme 1 Simplification of the intrinsic bond orbital (IBO) methodology.

Generally speaking, the bonding character illustrated (e.g. in the form of not assumed Lewis structures) thus naturally emerges. This procedure thus goes beyond the standard natural bond orbital (NBO) approach,^11a which assumes a spherical symmetry of AOs and Lewis bonding patterns. Boldyrev has also introduced a tool known as adaptive natural density partitioning (AdNP),^11b a method that analyzes the first-order reduced density matrix, which inherently contains some assumptions. However, this approach works well for various boron rings such as boron molecular Wankel motors, boron fullerenes and 2D boron sheets.^11c The wave function used for IBOs is initially calculated using canonical molecular orbitals (MOs) from the SCF procedure, and their subsequent unitary transformation yields the desired IBOs, which clarifies the nature of the corresponding bonding pattern. The point is that basis set functions are not associated with any atom. In the case of expanding MOs over a minimal basis set of free-atom AOs (known from any textbook of general chemistry), the resulting wave function can easily be interpreted. However, such a wave-function would be imprecise because the free-atom AOs do not undergo any polarization from the corresponding surroundings. On that basis, an accurate wave function is calculated and a set of polarized AOs is formed in terms of splitting the free-atom AOs into contributions corresponding to a depolarized occupied space and its complement by the corresponding projection.

We have applied the novel IBO approach to a broad series of experimentally known heteroboranes covering closo, nido, arachno and hypho types of cages with incorporated tetrel, pnictogen or chalcogen heterovertices up to the third-row elements in order to study the nature of bonding in heteroborane clusters.

Classical and multicenter bonding patterns are reflected in observable molecular properties. Firstly, the different bonding arrangements of various bonding types result in various interatomic distances that are slightly longer in multicenter bonds, as exemplified further. An interatomic distance close to the sum of the covalent radii (Σr_cov)^1c of both atoms can thus be considered as an indication of classical bonding. However, it might be questionable whether a larger interatomic distance necessarily indicates multicenter bonding. It might also be a consequence of electron deficiency in the case of electron unsaturated boranes. In this study, the obtained IBO results are compared with the interatomic distances refined either from the experimental electron diffraction or from the combined ab initio/GIAO/NMR approach. Secondly, classical bonding follows the established electronegativity concept, which is not true for multicenter bonding.^1b We have thus computed and analyzed the molecular electrostatic potential (ESP) surfaces and the dipole moments of selected representative molecules.

Methods

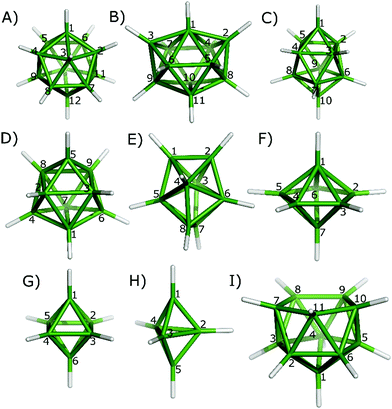
In the first step, the IBO method was validated on a series of organic and inorganic compounds with well described either covalent or multicenter bonding: ethane, elemental white phosphorus P₄ and hydrogen disulfide (containing classical, 2c2e bonding), trimethylaluminium dimer,⁶ diborane,¹²closo-B₁₂H₁₂²⁻,¹³nido-B₁₁H₁₁⁴⁻,¹⁴arachno-B₁₀H₁₄²⁻,¹⁵closo-B₁₀H₁₀²⁻,^13bcloso-B₈H₈²⁻,¹⁶closo-B₇H₇²⁻,¹⁷closo-B₆H₆²⁻,¹⁸closo-B₅H₅²⁻,¹⁹ and tetra-tert-butyltetrabora-tetrahedrane.²⁰

In the second step, we used the IBO method to investigate the nature of bonding in a series of experimentally known heteroboranes containing only up to third-row elements, specifically: closo-1-CB₁₁H₁₂⁻,²¹closo-1,2-C₂B₁₀H₁₂,²²closo-1,7-C₂B₁₀H₁₂,²²closo-1-NB₁₁H₁₂,²³closo-1,2-P₂B₁₀H₁₀,²⁴closo-1,7-P₂B₁₀H₁₀,²⁵closo-1-SB₁₁H₁₁,²⁶closo-2-CB₁₀H₁₁⁻,²⁷closo-2,3-C₂B₉H₁₁,²⁸closo-1-CB₉H₁₀⁻,²⁹closo-1,2-C₂B₈H₁₀,³⁰closo-1,6-C₂B₈H₁₀,³¹closo-1,10-C₂B₈H₁₀,³²closo-1-NB₉H₁₀,³³closo-2,1-PCB₈H₉,³⁴closo-6,1-PCB₈H₉,³⁴closo-1-SB₉H₉,³⁵closo-4-CB₈H₉⁻,³⁶closo-1,7-C₂B₇H₉,³⁷closo-1-CB₇H₈⁻,³⁸closo-1,2-C₂B₆H₈,³⁹closo-1,7-C₂B₆H₈,⁴⁰closo-1,6-C₂B₆H₈,³⁹closo-2-CB₆H₇⁻,⁴¹closo-2,4-C₂B₅H₇,⁴²closo-1,2-C₂B₄H₆,⁴³closo-1,6-C₂B₄H₆,⁴²closo-1,5-C₂B₃H₅,⁴²closo-2,3-C₂B₃H₅,⁴²closo-1,2-C₂B₃H₅,⁴²nido-2,9-C₂B₉H₁₂⁻,⁴⁴nido-2,7-C₂B₉H₁₂⁻,⁴⁵nido-7,8-C₂B₉H₁₂⁻,⁴⁶nido-7,9-C₂B₉H₁₂⁻,⁴⁴nido-7,8,10-C₂SB₈H₁₀,⁴⁷nido-9,11,7,8-P₂C₂B₇H₉,⁴⁸nido-7,8,9,10-P₂C₂B₇H₉,⁴⁸nido-7,8,9,10-P₃CB₇H₈,⁴⁹nido-7,9,8,10-P₂C₂B₇H₉,⁴⁸nido-7,8,9,11-P₂C₂B₇H₉,⁴⁸nido-6,7-C₂B₇H₉²⁻,⁴⁹arachno-1,8,11-NC₂B₈H₁₃,⁵⁰arachno-6,9-CSB₈H₁₂,⁵¹arachno-4,6,5-C₂SB₆H₁₀,⁵²hypho-2,5,12-C₃B₈H₁₅⁻,⁵³hypho-7,8-C₂B₆H₁₃⁻,⁵⁴hypho-7,8-NSB₆H₁₁,⁵⁴hypho-7,8-S₂B₆H₉⁻,⁵⁴ and hypho-7,8-CSB₆H₁₁⁻.⁵⁴ Because of the hypho vs. nido conflict present, the classification of such compounds as hypho should be treated with caution.⁵⁴ The numbering of the studied cages is shown in Fig. 1.

Fig. 1 The numbering scheme for the following studied cages: closo-B₁₂H₁₂²⁻ (A), closo-B₁₁H₁₁²⁻ (B), closo-B₁₀H₁₀²⁻ (C), closo-B₉H₉²⁻ (D), closo-B₈H₈²⁻ (E), closo-B₇H₇²⁻ (F), closo-B₆H₆²⁻ (G), closo-B₅H₅²⁻ (H) and nido-B₁₁H₁₁⁴⁻ (I).

All the calculations were performed at the DFT/B3-LYP/def2-TZVPP level of theory using the Turbomole6.6⁵⁵ and Gaussian09⁵⁶ program packages. The outputs were examined using the IBOview program¹⁰ to visualize the IBOs (threshold of 60%) and render it. Furthermore, the electrostatic potential and the dipole moments were computed at the HF/cc-pVDZ level using the Gaussian09⁵⁶ and Molekel4.3⁵⁷ program packages.

Results and discussion

Benchmarks

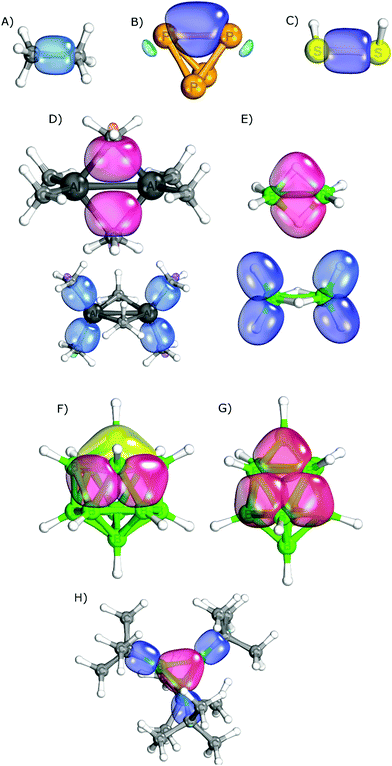
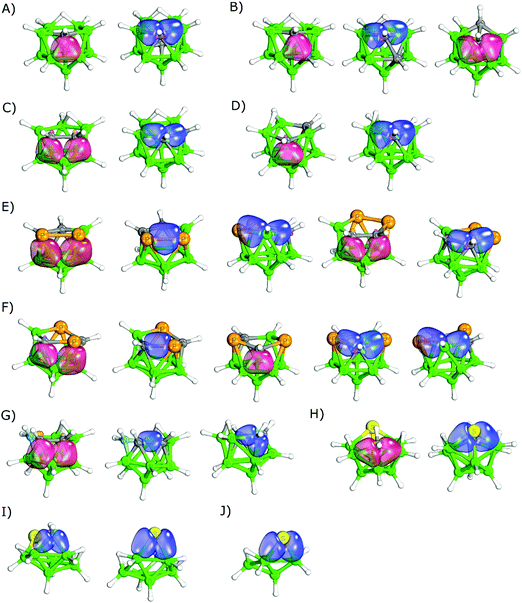
To validate the IBOs and illustrate the difference between the 2c2e and 3c2e types of bonding, we first applied the IBO approach to a series of organic/inorganic compounds with 2c2e bonding and closo-borane clusters with 3c2e bonding. In addition, we considered the bridging Al–C bonds in Al₂(CH₃)₆ because the multicenter bonding of this molecule had been confirmed experimentally.⁶

The IBOs for the benchmark organic/inorganic compounds and the selected boron clusters are summarized in Fig. 2. The IBOs clearly demonstrated classical bonding in ethane, elemental white tetraphosphorus P₄ and disulfide hydrogen (Fig. 2A–C). The IBOs also confirmed multicenter bonding in Al₂(CH₃)₆ (Fig. 2D) in accordance with the experimental data. In addition, the hydrogen bridging in diborane was also shown to be of multicenter nature (Fig. 2E). The interatomic distances are slightly elongated in the shown multicenter bonds. The B–H, B–B and C–Al separations are 4–14% longer than the sum of the covalent radii (Σr_cov)^1c of both atoms, i.e. distances of 1.334, 1.770⁵ and 2.125⁶ Å, respectively. For comparison, the classical 2c2e B–H and C–Al bonds of the same molecules are closer to Σr_cov, i.e. 1.187⁵ and 1.953⁶ Å, which correspond to 101.5 and 97.2% of Σr_cov, respectively. In addition, the terminal (exosceletal) H atoms involved in the classical 2c2e B–H bonds have hydridic character due to the low electronegativity of B atoms. In contrast to the terminal H atoms, the bridging H atoms of diborane have partial positive atomic charges.⁵⁸

Fig. 2 The visualized IBOs for selected organic/inorganic compounds (A–C), trimethylaluminium (D), diborane (E) closo-B₁₂H₁₂ (F), closo-B₁₀H₁₀ (G) and tetra-tert-butyl-tetrabora-tetrahedrane (H). The orbital color coding is as follows: dark blue/purple – classical 2c2e bonding; red/pink – multicenter 3c2e bonding; yellow – multicenter 4c2e bonding.

In the series of various borane clusters, the presence of multicenter bonding was confirmed (Fig. 2F and G). Besides the 3c2e bonds, this approach also revealed 4-center-2-electron (4c2e)⁵⁹ bonds of the B–B–B–B type in closo-B_nH_n²⁻ (n = 12, 10 and 8), nido-B₁₁H₁₁⁴⁻ and arachno-B₁₀H₁₄²⁻. This unique 4c2e bonding has already been known for some borane compounds.^60,61 However, this bonding has only been reported as 4c2e B–B–B–H with no evidence for 4c2e B–B–B–B bonding. The presence of the 4c2e bond in closo-B₁₂H₁₂²⁻ is shown in Fig. 2F. The number of 4c2e bonds increased with the size of the cluster (e.g. closo-B₁₀H₁₀²⁻ and closo-B₁₂H₁₂²⁻ had one and three 4c2e bonds, respectively).

In the case of tetra-tert-butyl-tetrabora-tetrahedrane, B₄(t-Bu)₄ (see Fig. 2H), the IBO approach clearly indicated the presence of four multicenter 3c2e bonds (B–B–B triangles). The other bonds, including the B–C, C–C and C–H bonds, were confirmed by the IBOs to be of classical 2c2e nature. The observed presence of 3c2e bonding corresponded well with the published study on B₄H₄ and B₄(CH₃)₄.⁶²

Bonding in heteroboranes

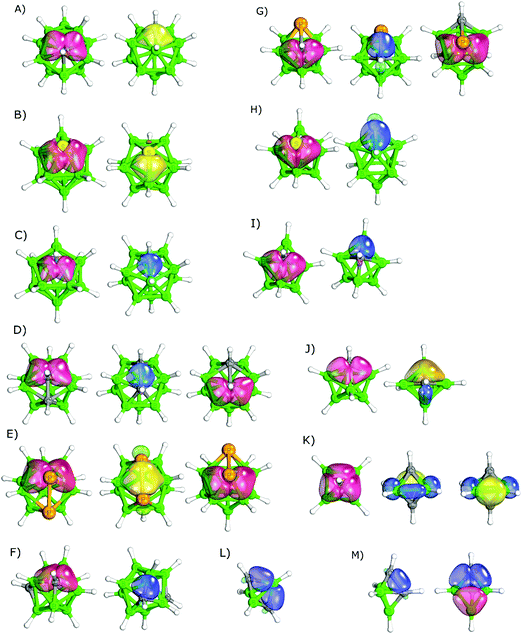
It is important to consider the differences between various boron architectures when analyzing the results for multidimensional boron compounds – e.g. closo-1-SB₁₁H₁₁ differs in many respects from closo-1-SB₉H₉.⁶³ The IBO results of icosahedral heteroboranes are summarized in Table 1. Furthermore, the IBOs of selected closo-heteroborane clusters are shown in Fig. 3. When the icosahedral cluster only contained a single heteroatom, the heteroatom was mostly part of multicenter bonding. For instance, the C atom of closo-1-CB₁₁H₁₂⁻ participated in three bonds, one of 4c2e nature (B–B–B–C) and two of 3c2e nature (B–B–C, see Fig. 3A). This bonding was thus analogous to the parent closo-B₁₂H₁₂²⁻, which also contained one 4c2e and two 3c2e bonds in the same location. Similarly, the S atom of closo-1-SB₁₁H₁₁ (see Fig. 3B) took part in two multicenter (3c2e) bonds of B–S–B type and one bond of B–B–S–B type of 4c2e nature. Such a bonding pattern corresponded to a B–S distance of 2.010 Å (106.9% of Σr_cov).^26a More interestingly, it could also help in explaining the highly positive σ-holes (V_S,max of 28.2 kcal mol⁻¹) on the S atom, which are known to be important for its crystal packing.⁶⁴ This V_S,max value is unusually high considering the low electronegativity of boron atoms. closo-1-NB₁₁H₁₂ contained two 3c2e B–N–B bonds and one 2c2e B–N bond. However, it should be mentioned that the 2c2e bonding was debatable in the case of closo-1-NB₁₁H₁₂ (see Fig. 3C). The bond evaluated as a classical covalent bond was less localized than a typical 2c2e bond (Fig. 2) and could also be seen as a crossing between the 2c2e and 4c2e bonds. Such a bonding pattern would also better correspond to a B–N distance of 1.716 Å (110.0% of Σr_cov).²³

Table 1 The summarized IBO data for the icosahedral heteroboranes. For the sake of clarity, the B–B–H and B–B–B multicenter bonds are not included

Compound	Bonding
	Multicenter	Classical
12-vertex
closo-1-CB11H12^−	2 × B–C–B; 1 × B–C–B–B	—
closo-1-SB11H11	1× B–S–B; 1 × B–S–B–B	—
closo-1-NB11H12	2 × B–N–B	1 × B–N
closo-1,7-C2B10H12	6 × B–C–B	—
closo-1,7-P2B10H10	3 × B–P–B; 3 × B–B–P–B	—
closo-1,2-C2B10H12	4 × B–C–B	1 × C–C
closo-1,2-P2B10H10	4 × B–P–B; 1 × B–P–P–B	—

---

Fig. 3 Calculated IBOs for the following heteroboranes: closo-1-CB₁₁H₁₂⁻ (A), closo-1-SB₁₁H₁₁ (B), closo-1-NB₁₁H₁₂ (C), closo-1,2-C₂B₁₀H₁₂ (D), closo-1,2-P₂B₁₀H₁₀ (E), closo-2,3-C₂B₉H₁₁ (F), closo-2,1-PCB₈H₉ (G), closo-1-SB₉H₉ (H), closo-1-CB₇H₈⁻ (I), closo-2-CB₆H₇⁻ (J), closo-1,6-C₂B₄H₆ (K), closo-1,5-C₂B₃H₅ (L) and closo-1,2-C₂B₃H₅ (M). The orbitals that are not shown in this figure are analogous to those rendered. The orbital color coding is as follows: dark blue/purple – classical 2c2e bonding; red/pink – multicenter 3c2e bonding; yellow – multicenter 4c2e bonding.

closo-1,7-C₂B₁₀H₁₂ and closo-1,7-P₂B₁₀H₁₀ have two heteroatoms that are not adjacent. They can thus be considered as a crossing between heteroboranes with one and more heterovertices. In contrast to closo-1-CB₁₁H₁₂⁻, the C atoms of closo-1,7-C₂B₁₀H₁₂ did not form 4c2e B–C–B–B bonds. Instead, they only formed B–C–B 3c2e multicenter bonds. closo-1,7-P₂B₁₀H₁₀, however, formed both 3c2e and 4c2e bonds. closo-1,2-C₂B₁₀H₁₂ has two adjacent C atoms, which form 3c2e B–C–B bonds and a classical C–C bond (see Fig. 3D). This pair of adjacent C–C atoms is known to act as an electron donor and becomes the center of the partial positive charge in the molecule. This has already been determined experimentally.⁶⁵ It might thus be considered important experimental evidence supporting the 3c2e B–C–B bonding. The C–C bond of closo-1,2-C₂B₁₀H₁₂ was the only classical bond found by the IBO approach among the icosahedral heteroboranes besides the debatable case of closo-1-NB₁₁H₁₂ described above. Similar to the B–N bond of closo-1-NB₁₁H₁₂, the C–C bond of closo-1,2-C₂B₁₀H₁₂ was less localized and longer (1.624 Å) than the C–C classical bonds in the validation data set, e.g. in ethane (1.540 Å).²² It also corresponds to LMO results obtained by Lipscomb et al.⁶⁶ The studied icosahedral cluster with adjacent P atoms, closo-1,2-P₂B₁₀H₁₀ (see Fig. 3E), did not form a classical bond between the P atoms; instead, it formed a multicenter B–P–P–B 4c2e bond. This bonding could be compared to B–B–B–B obtained in the parent closo-B₁₂H₁₂²⁻. The P–P distance in closo-1,2-P₂B₁₀H₁₀ is 2.310 Å (104.1% of Σr_cov).²⁴ The most positive molecular ESP surface is located between the two P atoms (V_S,max = 28.2 kcal mol⁻¹),⁶⁷ which is analogous to closo-1,2-C₂B₁₀H₁₂.

The bonding patterns of the 11-vertex closo-carboranes were similar to those of the 12-vertex cages (compare Table 1 and Table S1, ESI†). The IBO results did not reveal any difference in bonding between closo-2-CB₁₀H₁₁⁻ and the above-discussed closo-1-CB₁₁H₁₂⁻. However, some difference was found between closo-2,3-C₂B₉H₁₁ and closo-1,7-C₂B₁₀H₁₂ (separated C atoms in both clusters, numbering shown in Fig. 1). The C atoms of closo-2,3-C₂B₉H₁₁ (see Fig. 3F) participated in a classical B–C bond besides the two B–C–B 3c2e bonds. On the other hand, closo-1,7-C₂B₁₀H₁₂ only had 3c2e B–C–B bonds.

The bonding patterns obtained in the 10-vertex closo-heteroboranes (see Table 2) were also overall similar to those in the icosahedral cages. closo-1-CB₉H₁₀⁻ and closo-1,2-C₂B₈H₁₀ had analogous bonding patterns to closo-1-CB₁₁H₁₂⁻ and closo-1,2-C₂B₁₀H₁₂, respectively. It should be mentioned, however, that the C–C bond of closo-1,2-C₂B₈H₁₀ is shorter than that bond in closo-1,2-C₂B₁₀H₁₂, (1.538 vs. 1.624 Å).³⁰ In addition, the bonding in closo-2,1-PCB₈H₉ (see Fig. 3G) was similar to closo-1,2-C₂B₁₀H₁₂. Besides closo-1,2-C₂B₈H₁₀, two other isomers of closo-C₂B₁₀H₁₂ were studied, both with non-adjacent C atoms. Both clusters only formed multicenter bonds of either 3c2e or 4c2e nature. The fact that these molecules formed a 4c2e bond corresponded to the parent closo-B₁₀H₁₀²⁻, in which a 4c2e bond occurred as well. The analysis of closo-1-NB₉H₁₀ revealed very similar results to the above-discussed closo-1-NB₁₁H₁₂. Both of these molecules had a single 2c2e B–N bond. This might be in accordance with closo-1-SB₉H₉, where two multicenter B–S–B bonds and one classical B–S bond were found. When closo-1-SB₉H₉ (see Fig. 3H) was compared to closo-1-SB₁₁H₁₁, one classical B–S bond was formed instead of a B–S–B–B 4c2e bond. This finding might help to rationalize the less positive σ-holes (22.4 vs. 28.2 kcal mol⁻¹)⁶⁷ and the shorter S–B distance (1.939^35avs. 2.010^26a Å) in closo-1-SB₉H₉.

Table 2 The summarized IBO data for various closo-heteroboranes. For the sake of clarity, B–B–H and B–B–B multicenter bonds are not included

Compound	Bonding
	Multicenter	Classical
10-vertex
closo-1-CB9H10^−	2 × B–C–B; 1 × B–C–B–B	—
closo-1,2-C2B8H10	4 × B–C–B	1 × C–C
closo-1,6-C2B8H10	5 × B–C–B; 1 × B–C–B–B	—
closo-1,10-C2B8H10	5 × B–C–B; 1 × B–C–B–B	—
closo-1-NB9H10	2 × B–N–B	1 × B–N
closo-2,1-PCB8H9	2 × B–P–B; 2 × B–C–B	1 × C–P
closo-6,1-PCB8H9	3 × B–C–B; 2 × B–P–B; 1 × B–P–B–B	—
closo-1-SB9H9	2 × B–S–B	1 × B–S
8-vertex
closo-1-CB7H8^−	2 × B–C–B	1 × B–C
closo-1,2-C2B6H8	4 × B–C–B	1 × C–C
closo-1,7-C2B6H8	4 × B–C–B; 2 × B–C–B–B	—
closo-1,6-C2B6H8	4 × B–C–B; 2 × B–C–B–B	—

---

Two closo-carborane cages with 9 vertices were considered (Table S1, ESI†): closo-4-CB₈H₉⁻ and closo-1,7-C₂B₇H₉. Only B–C–B 3c2e bonds were found in these molecules. The absence of 4c2e bonding in closo-4-CB₈H₉⁻ could be caused by differences in the architecture of the cage. Otherwise, the data were in agreement with the results obtained for the larger closo-cages, discussed above.

The bonding patterns observed across the 8- and 7-vertex closo-carbaboranes were overall similar to the bigger ones (see Tables 1 and 2). However, one significant difference was found. While the IBOs did not show any C–B classical bonding in the bigger closo-CB_n−1H_n⁻ (n = 9–12) cages, the C atom of closo-CB_n−1H_n⁻ (n = 7–8) formed a classical 2c2e B–C bond as well as multicenter bonds.

The 6- and 5-vertex closo-dicarbaboranes showed different trends from the bigger ones. Classical bonding was still more frequent in the smallest cages. In addition to the C–C and B–C bonds, the classical B–B bond was also found in closo-1,6-C₂B₄H₆ and closo-2,3-C₂B₃H₅ (see Table 3 and Fig. 3). Furthermore, the IBOs even disproved the presence of any multicenter bonding in closo-1,5-C₂B₃H₅. All bonds appeared to be B–C classical bonds. Such a bonding pattern would correspond to the localized molecular orbital (LMO) structures,⁸ which were already used to question the presence of the multicenter nature of the C–B–C bonding in closo-1,5-C₂B₃H₅.⁶⁸ Note that the small cluster dimensions are problematic cases in terms of ¹¹B NMR computations.^20b In addition, the interatomic distances and charge distribution should reflect the classical bonding pattern. The short C–B separation (1.553 Å,^20b 97.1% of Σr_cov) supports the classical bonding pattern. It should also be mentioned that the B–B separation in these molecules is 1.844 Å^20b (108.5% of Σr_cov) and the IBO did not show any bonding between these boron atoms. In contrast to the other closo carboranes, the most positive ESP of closo-1,5-C₂B₃H₅ is not on the top of the CH vertex; instead, it is on the B atom (see Fig. 4). This finding shows that the classical electronegativity concept is valid in this molecule, which supports classical bonding in this molecule. The IBO results of closo-1,2-C₂B₄H₆ did not differ from the IBO results of the bigger closo-1,2-C₂B_n−2H_n (n = 12, 10, 8), in contrast to the other smaller compounds. The C atom participated in four B–C–B bonds and one C–C bond in all of these closo-1,2-C₂B_n−2H_n (n = 12, 10, 8, 6) molecules. The IBO results of closo-1,2-C₂B₄H₆ nicely resembled the LMO structures on the PRDDO level obtained using the Boys criteria.⁸

Table 3 The summarized IBO data for various closo-heteroboranes. For the sake of clarity, B–B–H and B–B–B multicenter bonds are not included

Compound	Bonding
	Multicenter	Classical
6-vertex
closo-1,2-C2B4H6	4 × B–C–B	1 × C–C
closo-1,6-C2B4H6	6 × B–C–B; 1 × C–B–B–C	3 × B–B
5-vertex
closo-1,5-C2B3H5	—	6 × B–C
closo-2,3-C2B3H5	2 × B–C–B; 1 × B–C–C–B	2 × B–C; 2 × B–B
closo-1,2-C2B3H5	2 × B–C–B	1 × C–C; 2 × B–C

---

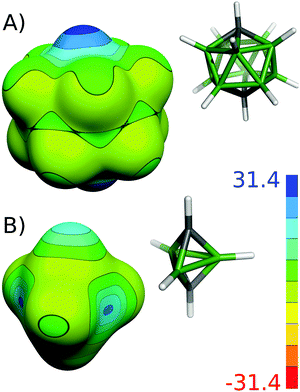Fig. 4 The molecular diagrams and electrostatic potential on the 0.001 a.u. molecular surface for closo-1,12-C₂B₁₀H₁₁ (A) and closo-1,5-C₂B₃H₅ (B). The color range of the ESP is in kcal mol⁻¹.

The bonding results for nido- are summarized in Table 4. The calculated IBOs for the selected heteroborane clusters are included in Fig. 5 to illustrate their bonding. The results show that the nature of bonding is heavily dependent on the position of the heteroatom in the nido-cages. Covalent bonds were found exclusively in the open face. For example, nido-2,9-C₂B₉H₁₂ and nido-2,7-C₂B₉H₁₂, which had one C atom located in the open face and one in the closed face, only formed the classical B–C bonds on the open belt, whereas the carbon in the closed face only participated in bonding of 3c2e nature. Moreover, although nido-2,7-C₂B₉H₁₂ had adjacent carbon atoms, the classical C–C bonding did not occur. Instead, C–C–B bonding of 3c2e nature was formed. On the other hand, when a nido-cage contained at least one heteroatom in the open pentagonal belt, evidence for classical covalent bonding was found. nido-Carboranes with two C atoms in the face formed B–C–B, B–C and C–C bonds. nido-7,8-C₂B₉H₁₂ contained adjacent C atoms and thus formed one C–C and two B–C bonds of classical 2c2e nature. nido-7,9-C₂B₉H₁₂ with separated C atoms formed four B–C bonds of 2c2e nature, all in the open face. The 3c2e bonds were formed in B–C–B triangles, when both B atoms were located under the C atom.

Table 4 The summarized IBO data for various nido-, arachno- and hypho-heteroboranes. For the sake of clarity, the multicenter B–B–H and B–B–B bonds are not included

Compound	Bonding
	Multicenter	Classical
nido-
nido-2,9-C2B9H12^−	4 × B–C–B	2 × B–C
nido-2,7-C2B9H12^−	1 × B–C–C; 2 × B–C–B	2 × B–C
nido-7,8-C2B9H12^−	2 × B–C–B	1 × C–C; 2 × B–C
nido-7,9-C2B9H12^−	2 × B–C–B	4 × B–C
nido-7,8,10-C2SB8H10	1 × B–S–B	2 × B–S; 2 × B–C; 1 × C–C
nido-9,11,7,8-P2C2B7H9	2 × B–C–B; 2 × B–P–B	2 × B–P; 2 × C–P; 1 × C–C
nido-8,9,7,11-P2C2B7H9	2 × B–P–B; 2 × B–C–B	1 × P–P; 1 × C–C; 1 × C–P; 1 × B–C; 1 × P–B
nido-7,8,9,10-P3CB7H8	3 × B–P–B; 1 × B–C–B	2 × P–P; 1 × P–C; 1 × B–C; 1 × B–P
nido-7,9,8,10-P2C2B7H9	2 × B–P–B; 2 × B–C–B	3 × P–C; 1 × B–P; 1 × B–C
nido-7,8,9,11-P2C2B7H9	2 × B–P–B; 2 × B–C–B	1 × P–P; 2 × P–C; 2 × B–C
nido-6,7-C2B7H9^2−	2 × B–C–B	1 × C–C; 2 × B–C
arachno-
arachno-1,8,11-NC2B8H13	3 × B–C–B	1 × C–C; 2 × N–B
arachno-6,9-CSB8H12	2 × B–C–B	2 × B–S
arachno-4,6,5-C2SB6H10	4 × B–C–B	2 × B–S
hypho-
hypho-C3B8H15^−	4 × B–C–B	2 × B–C
hypho-7,8-C2B6H13^−	—	4 × B–C
hypho-7,8-NSB6H11	—	2 × B–S; 2 × N–B
hypho-7,8-S2B6H9^−	—	4 × S–B
hypho-7,8-SCB6H11^−	—	2 × B–S; 2 × B–C

---

Fig. 5 Calculated IBOs for the following heteroboranes: nido-2,9-C₂B₉H₁₂⁻ (A), nido-2,7-C₂B₉H₁₂⁻ (B), nido-7,8-C₂B₉H₁₂⁻ (C), nido-7,9-C₂B₉H₁₂⁻ (D), nido-7,8,9,10-P₂C₂B₇H₉ (E), nido-7,9,8,10-P₂C₂B₇H₉ (F), arachno-1,8,11-NC₂B₈H₁₃ (G), arachno-6,9-CSB₈H₁₂ (H), hypho-7,8-NSB₆H₁₁ (I) and hypho-7,8-S₂B₆H₉⁻ (J). The orbitals that are not shown in this figure are analogous to those rendered. The orbital color coding is as follows: dark blue/purple – classical 2c2e bonding; red/pink – multicenter 3c2e bonding; yellow – multicenter 4c2e bonding.

The bonding patterns of the nido-cages with other incorporated heteroatoms were very similar to the already-described nido-carboranes. Any heteroatom incorporated into the open face was a source of classical bonding. This resulted in a variety of bonding. For example, the open belts of nido-7,8,10-C₂SB₈H₁₀, nido-7,8,9,10-P₃CB₇H₈, nido-7,8,9,10-P₂C₂B₇H₉ and nido-9,11,7,8-P₂C₂B₇H₉ were formed exclusively via the classical covalent bonds. There was evidence for S–B, P–P, C–C, C–P, B–C and P–B classical bonds. The length of these bonds ranged from 98.3 to 102.0% of Σr_cov, thus supporting the classical bonding pattern.^47–49 Besides the classical bonds in the open face, the nido-heteroboranes also contained multicenter bonds of 3c2e nature with the participating heteroatom in agreement with the above-described nido-carboranes. In nido-8,9,7,11-P₂C₂B₇H₉, the 3c2e B–P–B bonds were formed in the triangles 3–4–8 and 4–5–9 (the numbering of this cage is shown in Fig. 1I). Similarly, B–C–B 3c2e bonds were formed in the 2–6–11 and 2–3–7 triangles. The interatomic distances ranged from 103.9 to 108.7% of Σr_cov in these triangles.⁴⁸nido-9,11,7,8-P₂C₂B₇H₉ had an analogous bonding pattern even though the P atoms were not adjacent.

When the two heteroatoms were adjacent to each other, two 3c2e bonds of B–Z–B (Z = various heteroatoms) were formed. Here, one boron atom was shared by both of the triangles. This one lay on the under-belt under the middle of the Z–Z covalent bonding. When the heteroatoms were not located adjacent to each other, the 3c-2e triangles were still formed. The heteroatoms and boron atoms lying under them are involved in this kind of bonding. The computed dipole moments and molecular ESP surfaces of the representative nido clusters show that the heterovertices located in the open pentagonal face act as an electron donor and become the center of a partial positive charge of the molecule (Fig. 6), which supports the existence of the B–Z–B 3c2e bonds. Moreover, the crystal packing of nido-7,8,9,11-Sb₂C₂B₇H₉ is predominantly dictated by the very strong Sb₂⋯H–B σ-hole interaction.^48b

Fig. 6 The visualization of the calculated dipole moment and electrostatic potential (ESP) on the 0.001 a.u. molecular surface of nido-7,8,10-C₂SB₈H₁₀ (A), nido-9,11,7,8-P₂C₂B₇H₉ (B), nido-8,9,7,11-P₂C₂B₇H₉ (C) and nido-7,8,9,11-P₂C₂B₇H₉ (D). The color range of the ESP is in kcal mol⁻¹.

We also applied the IAO method to nido-6,7-C₂B₇H₉ as a representative of the 9-vertex nido-heteroboranes. Two B–C–B bonds of 3c2e nature and B–C and C–C 2c2e bonds suggested that bonding in the 9-vertex is not different from the 11-vertex nido-clusters.

The IBO results of the arachno-clusters are summarized in Table 4 and some of them have also been selected for Fig. 5, where the IBO results are illustrated. The results were similar to those of the nido-clusters. The C atoms were bonded via 3c2e B–C–B bonds in the arachno-clusters. In the case of arachno-1,8,11-NC₂B₈H₁₃ with adjacent carbon atoms, the classical C–C bond was formed. At the same time, two B–C–B multicenter bonds were formed as well (see Fig. 5G). The other heteroatoms only formed classical bonds, e.g. the S atom of arachno-4,6,5-C₂SB₆H₁₀ or the N atom of arachno-1,8,11-NC₂B₈H₁₃.

The calculated IBOs for hypho-clusters are summarized in Table 4. Their IBOs are shown in Fig. 5. Bonding of the heteroatoms was dominated by classical bonding in the studied hypho-clusters. With the exception of hypho-C₃B₈H₁₅, the IBOs did not reveal any bonds of multicenter nature with the exception of the B–B–B and B–B–H bonds. Each of the heteroatoms formed two covalent bonds with a boron atom of 2c2e nature.

In contrast to closo-1-SB₁₁H₁₁, the S atom of hypho-7,8-NSB₆H₁₁ is incorporated into the hypho-cluster network exclusively via classical B–S bonds and consequently acts as an electron acceptor. This S atom thus has a negative electrostatic potential (ESP) surface without areas of positive ESP (see Fig. 7).

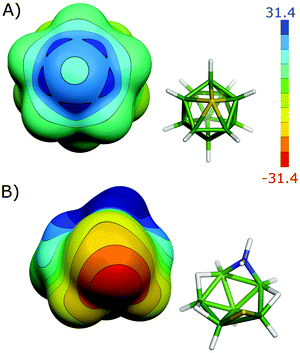Fig. 7 The molecular diagrams and electrostatic potential on the 0.001 a.u. molecular surface of closo-1-SB₁₁H₁₁ (A) and hypho-7,8-NSB₆H₁₁ (B). The color range of the ESP is in kcal mol⁻¹.

Table S2 (ESI†) shows the results for a selected series of representative compounds obtained by the most widely used orbital localization scheme, the NBO methodology. The NBO and IBO results are overall similar with many small differences. The majority of the differences are due to the different localization techniques used. While IBO only minimizes the spread of bond orbitals over the atoms (i.e. maximizes their localization), the NBOs explicitly search for the predefined Lewis structures most closely matching the given wave function. Consequently, 4c2e bonds naturally emerged in the IBO analysis of many heteroborane clusters. On the other hand, the NBO analysis was limited to 2c2e and 3c2e bonds. Other nonsystematic differences could be found between the IBO and NBO methods. However, an exhaustive comparison of the IBO with other methods is beyond the scope of this study.

Conclusions

The nature of bonding has been systematically studied on a broad series of heteroboranes using a novel quantum chemical tool, the intrinsic bond orbital (IBO) approach. The results have shown that the bonding of heteroatoms in icosahedral clusters is mainly of multicenter nature. The role of classical bonding increases with the decreasing size of the closo-heteroborane cages. An extreme case is closo-1,5-C₂B₃H₅, where the IBOs have disproved the presence of any multicenter bonding. The nature of bonding in the nido- and arachno-cages heavily depends on the position of the heteroatom, and covalent bonds have been found exclusively in the open face in nido- and arachno-cages. However, classical bonds are more common in clusters with open faces than in closo-heteroboranes.

It has also been shown in the studied architectures that the nature of bonding is reflected in the molecular observables such as the molecular electrostatic potential surface. Roughly speaking, where electron distribution is opposed to the classical electronegativity complex, such an electron distribution might be attributed to multicenter bonding. On the other hand, the classical bonding indicates the classical electronegativity concept. Vector analyses of the experimental and computed dipole moments support such an observation.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was supported by the research project RVO 61388963 of the Czech Academy of Sciences. We acknowledge the financial support from the Czech Science Foundation (17-08045S).

Notes and references

1. (a) W. N. Lipscomb, Boron Hydrides, Benjamin, New York, 1963; (b) more recent examples are outlined in D. Hnyk and D. A. Wann, Boron – the Fifth Element, in Challenges and Advances in Computational Chemistry and Physics, ed. D. Hnyk and M. McKee, Springer, Heidelberg, New York, Dordrecht and London, 2015, ch. 2, vol. 20; (c) the nature of a chemical bond is reflected in bond lengths, see P. Pykkö and M. Atsumi, Chem. – Eur. J., 2009, 15, 186–197.
2. R. C. Bochicchio, R. Ponec and F. Uhlík, Inorg. Chem., 1997, 36, 5363–5368.
3. R. M. Lobayan, R. C. Bochicchio, A. Torre and L. Lain, J. Chem. Theory Comput., 2009, 5, 2030–2043.
4. K. A. Lyssenko, M. Y. Antipin and V. N. Lebedev, Inorg. Chem., 1998, 37, 5834–5843.
5. K. Hedberg and V. Schomaker, J. Am. Chem. Soc., 1951, 73, 1482–1487.
6. H. Stammler, S. Blomeyer, R. J. F. Berger and N. W. Mitzel, Angew. Chem., Int. Ed., 2015, 54, 13816–13820.
7. Although PRDDO keeps a partially differential overlap and does not neglect it completely unlike CNDO, this approach is still empirically-based.
8. D. A. Dixon, D. A. Kleier, T. A. Halgren, J. H. Hall and W. N. Lipscomb, J. Am. Chem. Soc., 1977, 99, 6226–6237.
9. (a) P. Schleyer, G. Subramanian and A. Dransfeld, J. Am. Chem. Soc., 1996, 118, 9988–9989; (b) G. D. Graham, D. S. Marynick and W. N. Lipscomb, J. Am. Chem. Soc., 1980, 102, 2939–2945; (c) E. D. Jemmis, J. Am. Chem. Soc., 1982, 104, 7017–7020; (d) R. F. W. Bader and D. A. Legare, Can. J. Chem., 1992, 70, 657–676; (e) E. D. Jemmis, G. Subramanian, I. H. Srivastava and S. R. Gadre, J. Phys. Chem., 1994, 98, 6445–6451; (f) K. Takano, M. Izuho and H. Hosoya, J. Phys. Chem., 1992, 96, 6962–6969; (g) E. D. Jemmis and G. Subramanian, J. Phys. Chem., 1994, 98, 9222–9226; (h) Y. Sahin, C. Präsang, M. Hofmann, G. Geiseler, W. Massa and A. Berndt, Angew. Chem., 2005, 117, 1670–1673; (i) D. R. Armstrong, M. A. Fox and K. Wade, J. Organomet. Chem., 2012, 721, 130–136; (j) V. Dyczmons, M. Horn, P. Botschwina and A. Meller, THEOCHEM, 1998, 1, 137–149; (k) R. M. Lobayan, R. C. Bochicchio, A. Torre and L. Lain, J. Chem. Theory Comput., 2011, 7, 979–987; (l) A. Torre, L. Lain, R. Bochicchio and R. Ponec, J. Comput. Chem., 1999, 20, 1085–1090.
10. (a) G. Knizia, J. Chem. Theory Comput., 2013, 9, 4834–4843; (b) G. Knizia and J. E. M. N. Klein, Angew. Chem., Int. Ed., 2015, 54, 5518–5522.
11. (a) E. Reed, R. B. Weinstock and F. Weinhold, J. Chem. Phys., 1985, 83, 735; (b) D. Yu Zubarev and A. Boldyrev, Phys. Chem. Chem. Phys., 2008, 10, 5207–5217 . This work references other “empirically-based” bonding tools; (c) I. A. Popov and A. I. Boldyrev, Boron – the Fifth Element, in Challenges and Advances in Computational Chemistry and Physics, ed. D. Hnyk and M. McKee, Springer, Heidelberg, New York, Dordrecht and London, 2015, ch. 1, vol. 20.
12. S. H. Bauer, J. Am. Chem. Soc., 1937, 59, 1096–1103.
13. (a) I. B. Sivaeva, V. I. Bregadze and S. Sjöberg, Collect. Czech. Chem. Commun., 2002, 67, 679–727 and the references therein; (b) W. H. Knoth, H. C. Miller, J. C. Sauer, J. H. Balthis, Y. T. Chia and E. L. Muetterties, Inorg. Chem., 1964, 3, 159–167.
14. For the structure of B₁₁H₁₄⁻ with three hydrogen bridges, see: J. Fritchie, Inorg. Chem., 1967, 6, 1199–1203.
15. M. Hofmann and P. Schleyer, Inorg. Chem., 1998, 37, 5557–5565.
16. J. W. Bausch, G. K. S. Prakash and R. E. Williams, Inorg. Chem., 1992, 31, 3763–3768 ; see also the references therein.
17. F. Schlüter and E. Bernhardt, Inorg. Chem., 2011, 50, 2580–2589.
18. T. Schaper and Wilhelm Preetz, Inorg. Chem., 1998, 37, 363–365.
19. D. J. Wales, Encyclopedia of Inorganic Chemistry, Electronic Structure of Clusters, Wiley, Berlin, 2006.
20. (a) D. Hnyk, Polyhedron, 1997, 16, 603–606; (b) M. Bühl, J. Gauss, M. Hofmann and P. Schleyer, J. Am. Chem. Soc., 1993, 115, 12385–12390.
21. J. Pecyna, B. Ringstrand, S. Domagała, P. Kaszyński and K. Woźniak, Inorg. Chem., 2014, 53, 12617–12626.
22. A. R. Turner, H. E. Robertson, K. B. Borisenko, D. W. H. Rankin and M. A. Fox, Dalton Trans., 2005, 1310–1318.
23. D. Hnyk, M. Bühl, P. Schleyer, H. V. Volden, S. Gundersen, J. Müller and P. Paetzold, Inorg. Chem., 1993, 32, 2442–2445.
24. R. McLellan, N. M. Boag, K. Dodds, D. Ellis, S. A. Macgregor, D. McKay, S. L. Masters, R. Noble-Eddy, N. P. Platt, D. W. H. Rankin, H. E. Robertson, G. M. Rosaira and A. J. Welch, Dalton Trans., 2011, 40, 7181–7192.
25. J. L. Little, J. G. Kester, J. C. Huffman and L. J. Todd, Inorg. Chem., 1989, 28, 1087–1091.
26. (a) D. Hnyk, E. Vajda, M. Bühl and P. R. Schleyer, Inorg. Chem., 1992, 31, 2464; (b) H. Møllendal, S. Samdal, J. Holub and D. Hnyk, Inorg. Chem., 2003, 42, 3043–3046.
27. Of the five possible isomers of closo-CB₁₀H₁₁⁻, only closo-2-CB₁₀H₁₁⁻ exists: W. H. Knoth, J. Am. Chem. Soc., 1967, 89, 1274–1275 . This was also analyzed in P. R. Schleyer and K. Najafian, Inorg. Chem., 1998, 37, 3454–3470.
28. I. D. Mackie, H. E. Robertson, D. W. H. Rankin, M. A. Fox and J. M. Malget, Inorg. Chem., 2004, 43, 5387–5392.
29. B. Ringstranda, D. Batemana, R. K. Shoemaker and Z. Janoušek, Collect. Czech. Chem. Commun., 2009, 74, 419–431.
30. D. Hnyk, D. W. H. Rankin, H. E. Robertson, M. Hofmann, P. v. R. Schleyer and M. Bühl, Inorg. Chem., 1994, 33, 4781–4786.
31. P. M. Garrett, G. S. Ditta and M. F. Hawthorne, Inorg. Chem., 1970, 9, 947–1948.
32. J. Holub, T. Jelínek and Z. Janoušek, Collect. Czech. Chem. Commun., 2002, 67, 949–952.
33. L. Schneider, U. Englert and P. Paetzold, Z. Anorg. Allg. Chem., 1994, 620, 1191–1193.
34. J. Holub, M. Bakardjiev, B. Štíbr, D. Hnyk, O. L. Tok and B. Wrackmeyer, Inorg. Chem., 2002, 41, 2817–2819.
35. (a) D. Hnyk, D. A. Wann, J. Holub, S. Samdal and D. W. H. Rankin, Dalton Trans., 2011, 40, 5734–5737; (b) H. Møllendal, S. Samdal, J. Holub and D. Hnyk, Inorg. Chem., 2002, 41, 4574–4578; (c) J. MacCurtain, P. Brint and T. R. Spalding, J. Chem. Soc., Dalton Trans., 1985, 2591–2594.
36. T. Jelínek, B. Štíbr, J. Holub, M. Bakardjiev, D. Hnyk, D. L. Ormsby, C. A. Kilner, M. Thornton-Pett, H. J. Schanz, B. Wrackmeyer and J. D. Kennedy, Chem. Commun., 2001, 1756–1757.
37. B. M. Gimarc, B. Dai and J. J. Ott, J. Comput. Chem., 1989, 10, 14–16.
38. T. Jelínek, B. Štíbr, J. Plešek, J. D. Kennedy and M. Thornton-Pett, J. Chem. Soc., Dalton Trans., 1995, 431–437.
39. B. M. Gimarc and J. J. Ott, J. Am. Chem. Soc., 1987, 109, 1388–1392.
40. G. B. Dunks and M. F. Hawthorne, Inorg. Chem., 1968, 7, 1038–1039.
41. B. Štíbr, O. L. Tok, W. Milius, M. Bakardjiev, J. Holub, D. Hnyk and B. Wrackmeyer, Chem. – Eur. J., 2008, 14, 6529–6533.
42. E. D. Jemmis, E. G. Jayasree and P. Parameswaran, Chem. Soc. Rev., 2006, 35, 157–168 and the references therein.
43. R. M. Minyaev, V. I. Minkin, T. N. Gribanova and A. G. Starikov, Russ. Chem. Bull., Int. Ed., 2004, 53, 1159–1167.
44. M. A. Fox, A. E. Goeta, A. K. Hughes and A. L. Johnson, J. Chem. Soc., Dalton Trans., 2002, 2132–2141.
45. F. A. Kiani and M. Hofmann, Inorg. Chem., 2004, 43, 8561–8571.
46. M. A. Fox, A. E. Goeta, J. A. K. Howard, A. K. Hughes, A. L. Johnson, D. A. Keen, K. Wade and Ch. C. Wilson, Inorg. Chem., 2001, 40, 173–175.
47. D. Hnyk, M. Hofmann, P. Schleyer, M. Bühl and D. Rankin, J. Phys. Chem., 1996, 100, 3435–3440.
48. (a) J. Holub, T. Jelínek, D. Hnyk, Z. Plzák, I. Císařová, M. Bakardjiev and B. Štíbr, Chem. – Eur. J., 2001, 7, 1546–1554; (b) J. Holub, P. Melichar, Z. Růžičková, J. Vrána, D. A. Wann, J. Fanfrlík, D. Hnyk and A. Růžička, Dalton Trans., 2017, 46, 13714–13719.
49. M. Bakardjiev, J. Holub, B. Štíbr, D. Hnyk and B. Wrackmeyer, Inorg. Chem., 2005, 44, 5826–5832.
50. J. Plešek, B. Štíbr, D. Hnyk, T. Jelínek, S. Heřmánek, J. D. Kennedy, M. Hofmann and P. R. Schleyer, Inorg. Chem., 1998, 37, 3902–3909.
51. D. Hnyk, J. Holub, S. A. Hayes, M. F. Robinson, D. A. Wann, H. E. Robertson and D. W. H. Rankin, Inorg. Chem., 2006, 45, 8442–8446.
52. D. Hnyk, M. Hofmann and P. R. Schleyer, Collect. Czech. Chem. Commun., 1999, 64, 993–1000.
53. M. G. S. Londesborough, Z. Janoušek, B. Štíbr, D. Hnyk, J. Plešek and I. Císařová, Dalton Trans., 2007, 1221–1228.
54. J. P. F. Nunes, J. Holub, D. W. H. Rankin, D. A. Wann and D. Hnyk, Dalton Trans., 2015, 44, 11819–11826.
55. TURBOMOLE Version 6.6, 2014, a development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007, TURBOMOLE GmbH, since 2007.
56. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, G. A. Petersson, H. Nakatsuji, X. Li, M. Caricato, A. Marenich, J. Bloino, B. G. Janesko, R. Gomperts, B. Mennucci, H. P. Hratchian, J. V. Ortiz, A. F. Izmaylov, J. L. Sonnenberg, D. Williams-Young, F. Ding, F. Lipparini, F. Egidi, J. Goings, B. Peng, A. Petrone, T. Henderson, D. Ranasinghe, V. G. Zakrzewski, J. Gao, N. Rega, G. Zheng, W. Liang, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, K. Throssell, J. A. Montgomery Jr., J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, T. Keith, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, J. M. Millam, M. Klene, C. Adamo, R. Cammi, J. W. Ochterski, R. L. Martin, K. Morokuma, O. Farkas, J. B. Foresman and D. J. Fox, Gaussian 09, Revision A.02, Gaussian, Inc., Wallingford CT, 2016.
57. (a) P. Flükiger, H. P. Lüthi, S. Portmann and J. Weber, MOLEKEL 4.3, Swiss Center for Scientific Computing, Manno (Switzerland), 2000; (b) S. Portmann and H. P. Lüthi. MOLEKEL: CHIMIA, 2007, vol. 28, 555.
58. J. Fanfrlík, A. Pecina, J. Řezáč, R. Sedlak, D. Hnyk, M. Lepšík and P. Hobza, Phys. Chem. Chem. Phys., 2017, 19, 18194–18200.
59. J. S. Miller and J. J. Novoa, Acc. Chem. Res., 2007, 40, 189–196.
60. H. Jacobsen, Dalton Trans., 2009, 4252–4258.
61. M. L. McKee, M. Bühl, O. P. Charkin and P. R. Schleyer, Inorg. Chem., 1993, 32, 4549–4554.
62. J. E. D. Bene, I. Alkorta and J. Elguero, J. Phys. Chem. A, 2016, 120, 5745–5751.
63. J. Fanfrlik and D. Hnyk, CrystEngComm, 2016, 18, 8982–8987.
64. J. Fanfrlík, A. Přáda, Z. Padělková, A. Pecina, J. Macháček, M. Lepšík, J. Holub, A. Růžička, D. Hnyk and P. Hobza, Angew. Chem., Int. Ed., 2014, 53, 10139–10142.
65. D. Hnyk, V. Všetečka, L. Drož and O. Exner, Collect. Czech. Chem. Commun., 2001, 66, 1375–1379.
66. W. N. Lipscomb, in Boron Hydride Chemistry, ed. E. L. Muetterties, Academic Press, New York, 1975.
67. A. Pecina, M. Lepsik, D. Hnyk, P. Hobza and J. Fanfrlík, J. Phys. Chem. A, 2015, 119, 1388–1395.
68. T. Kar and K. Jug, Int. J. Quantum Chem., 1995, 53, 407–412.

---

Footnote

† Electronic supplementary information (ESI) available: The summarized IBO data for 7-, 9-, and 11-vertex closo-heteroboranes and the NBO results for selected representative boranes. See DOI: 10.1039/c7cp07422k

---