Dendritic polyelectrolytes revisited through the Poisson–Boltzmann–Flory theory and the Debye–Hückel approximation

Abstract

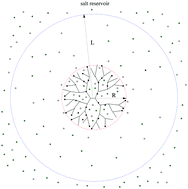
The properties of a dendritic polyelectrolyte in equilibrium with a reservoir of monovalent salts are investigated using the cell model and the Poisson–Boltzmann–Flory theory. Within this approach we use the Debye–Hückel approximation to solve the Poisson–Boltzmann equation and minimize the semi-grand potential of the system with respect to the size of the molecule which enables us to inspect its conformations as well as the electric field, the ionic density profile, the overall charge density, the effective charge of the dendrimer and the osmotic pressure based on their response to the salt concentration and the dendrimer charge. The model predicts pronounced trapping of salt ions, a local charge neutrality and a zero electric field in the volume of the molecule as well as oscillations of the density profiles and the electric field in the vicinity of the dendrimer–bulk interface. As a result of ion trapping and screening of Coulomb interactions monovalent salts are found to have a minor effect on the size of the dendrimer. Specifically, the dendrimer exists in slightly swollen states as compared to the neutral molecule which indicates that the conformational properties of the polyelectrolyte depend weakly on monovalent salts. These observations harmonise with the equilibrium behavior of the dendrimer pressure, the internal pressure and the bulk pressure, respectively.