The role of hierarchical design and morphology in the mechanical response of diatom-inspired structures via simulation
Alejandro
Gutiérrez
a,
Metin G.
Guney
b,
Gary K.
Fedder
bc and
Lilian P.
Dávila
 *a
*a
aMaterials Science and Engineering, School of Engineering, University of California Merced, 5200 N. Lake Road, Merced, CA 95343, USA. E-mail: ldavila@ucmerced.edu
bElectrical and Computer Engineering, College of Engineering, Carnegie Mellon University, USA
cThe Robotics Institute, Carnegie Mellon University 5000 Forbes Ave., Pittsburgh, PA 15213, USA
First published on 6th November 2017
Abstract
Diatoms are microscopic algae with intricate shell morphologies and features ranging from the nanometer to the micrometer scale, which have been proposed as templates for drug delivery carriers, optical devices, and metamaterials design. Several studies have found that diatom shells show unique mechanical properties such as high specific strength and resilience. One hypothesis is that these properties stem from the structural arrangement of the material at the nanometer and micrometer length scales, challenging the concept between what constitutes a “material” versus a “structure”. In this work, we have conducted a systematic simulation-prototyping study to shed light on the mechanics of diatom-inspired hierarchical microstructures. The Finite Element Method (FEM) was used to replicate three-dimensional diatom shells under compressive forces. The intricate hierarchical shell structure of the Coscinodiscus sp. diatom frustule observed in nature was reproduced in detail. Simulation parameters were selected to reproduce compression experiments, with a force distributed on the surface of the diatom shell. A frustule diameter of 50 μm was used with pore diameters ranging from 0.25 to 1.2 μm across different layers. A “unit cell” FEM model was also created to focus on the basic structural element of a diatom frustule. Both of these models were used to elucidate the relation between morphology and mechanical response. Additionally, select designs were prototyped using 3D Direct Laser Writing (DLW) lithography to evaluate the feasibility of manufacturing diatom-inspired devices at the micro-scale. Distinct correlations between pore size in each frustule layer, or pore shape in the basal layers, and the mechanical response of the diatom shell were established in this study. The 3D-DLW prototypes exhibit a similar level of intricate morphological traits observed in real diatoms, opening the possibility of a simulation-based process for the design and fabrication of diatom-inspired microdevices. This research helps explain how morphology features are central to the mechanical performance of hierarchically arranged structures and biomaterials in general, and it represents a step toward the manufacture of emerging metamaterials and microarchitectures.
Introduction
Manipulating materials at the nanometer scale has been consistently proposed as a grand challenge of science,1 with implications on the fabrication of materials and structures with unique properties.2 One path towards nanoscale manipulation is focusing on biomaterials, taking advantage of the results of millions of years of evolution to develop effective solutions. Specifically, this work focuses on one type of biostructure: diatoms. These microscopic algae have intricate porous shell morphologies and features3 ranging from the nanometer to the micrometer scale. Diatoms have been proposed4–8 as templates for drug delivery carriers, optical devices, and metamaterials design. Several studies9–13 have found diatom shells show unique mechanical properties such as high specific strength and resilience. Indeed, silica biostructures produced by microbial diatoms or radiolarians have complex morphologies tailored by evolution that appear to optimize specific functions such as protection from predators or filtering.14A diatom shell (called frustule) consists of two overlapping valves joined by one or more girdle bands as shown in Fig. 1, giving the shell a resemblance to a Petri dish. Frustules can vary in size from a few microns to over a millimeter3 and often exhibit features (e.g. pores, slits, ribs) as small as a few nanometers. There are two general types of diatoms depending on symmetry:3centric diatoms are often circular and have radial symmetry while pennate diatoms are elongated and often have bilateral symmetry. In particular, the Coscinodiscus sp. frustule diameters vary from 50–300 μm, and the basic structure of each valve comprises three interlinked silica layers hierarchically arranged, each layer with different thickness, pore sizes and pore distributions.3
The morphology and mechanical behavior of diatom frustules have been studied using micro- and nanomechanical testing as well as atomic force microscopy (AFM), scanning electron microscopy (SEM) and the finite element method (FEM). Hamm et al.10 investigated the potential of diatom frustules as armor against predators based on microneedle loading tests, discovering an inverse relation between frustule size and mechanical strength up to the point of fracture. The researchers further elaborated on the relation between the structural components of the frustule and the mechanical loads that diatoms experience in their natural environment using both experiments and simulations. Almqvist et al.11 used AFM to measure elasticity and hardness in the frustules of the pennate diatom species Navicula pelliculosa and found the values of Young's modulus E varied from the tens to the hundreds of GPa depending on the specific point over the frustule on which the tests were performed. The authors also observed a similar situation for hardness values, H varying from 1 to 12 GPa. Subhash et al.12 reported notoriously localized behavior over the centric frustules of Coscinodiscus sp., including differences in the failure mode of these frustules depending on loading orientation. Later, Losic et al.15 used SEM to characterize the morphology of Coscinodiscus sp. and performed AFM nanoindentation tests on the frustules, finding significant variations of the elastic modulus and hardness measurements depending on frustule morphology and location of the indentation, pointing towards highly localized properties. Recently, Aitken et al.16 sought to isolate the material effects by cutting square sections out of a Coscinodiscus sp. frustule and performing nanomechanical three-point bending tests on these squares. Their results show a brittle fracture of the samples and their measurements of toughness corroborate the diatom's high strength-density ratio. The authors hypothesized this mechanical performance is not only related to the biomaterial itself but also to the morphology of the frustule, which is the focus of the present work.
Computer simulation is an increasingly favored approach to study biomaterials, especially when there is a need to investigate and compare different hierarchically arranged microarchitectures. Atomistic principles, such as molecular dynamics (MD) represent one possible alternative towards this goal. An important study by Garcia et al.9 involved MD simulations of diatom-inspired nanoporous materials to study the effect of nanoporous layers in mechanical behavior under tension. This study found higher levels of hierarchy improve fracture toughness due to a crack arrest mechanism. Although MD can provide valuable insights to the structure–property relations of biomaterials, the method is too computationally expensive to be practical in the study of complex models. An alternative technique is the Finite Element Method (FEM), such as in the study by Hamm et al.,10 where FEM was used to simulate a valve of the diatom species Fragilariopsis kerguelensis under mechanical stresses caused by a mandible bite. Similarly, Lu et al.17 focused on the mechanics of a Coscinodiscus sp. frustule, modeling it as a cellular structure and analyzing the response of its basic hexagonal cell under compression. Special attention was given to the walls of the toroidal cavities that form in between layers with different pore sizes. More recently, Diaz et al.18 combined FEM and nanoindentation experiments to study the mechanical response of Coscinodiscus sp. and F. kerguelensis frustules under uniform compression, finding a correlation between mechanical properties and porosity.
Meanwhile the technology for fabricating complex structures at the micrometer, and even nanometer, scale has been developing rapidly for applications in MEMS, microfluidics and bioengineering.19–21 Though traditional methods of 2D photolithography22 and casting23 are still widely used, their application to the fabrication of bio-inspired microarchitectures is difficult. In conventional 2D photolithography,24 it remains challenging to achieve the level of intricacy characteristic of biological structures like diatom frustules without resorting to complex and expensive processes involving several lithographic steps with different photo masks, increasing the required time and effort to fabricate prototypes. In the case of casting, a high level of structural detail can be achieved for microfluidics applications.25 Indeed, casting techniques used by Belegratis et al.26 replicated the geometry of centric Cyclotella sp. frustules with a high level of detail. Drawbacks to this technique are: (a) the manufacturing process requires significant time in the cleanroom, and (b) the final shapes are limited to the initial templates available.
A more recently developed technique is 3D direct laser writing (DLW) lithography. This method is based on the two-photon absorption of a photosensitive material. The resulting polymerization is confined into a 3D volume around the focal spot of the laser with high resolution, allowing the creation of intricate 3D shapes in the photosensitive volume up to a scale of hundreds of nanometers.27 Jang et al.28 used DLW to fabricate hollow scaffolds mimicking the length scales and a single-layer hierarchical structure of diatom shells. Similarly, Belegratis et al.26 involved 3D-DLW to create single-layered diatom-inspired templates to drive their fabrication process.
In this work, the relation between structure and mechanics of diatom shells was studied using FEM and 3D-DLW. First, a detailed hierarchical model for a centric frustule was created based on SEM characterization data. This model was then used to study the mechanical response of a diatom shell under uniform compression, with emphasis on the effect of distinct hierarchical structural features in the overall mechanical response of the frustule. Upon systematic simulations, a series of correlations are defined crucial for the hierarchical design of diatom-inspired devices. A group of diatom-inspired polymeric geometries was fabricated using high-resolution 3D-DLW to show the potential of this approach in the design of bio-inspired materials and structures. The hypothesis is that key morphological features (e.g. pores, layers, I-beams) have dominant roles in the mechanical response (e.g. resilience, total deformation energy) of diatom frustules, with insights for the manufacture of bio-inspired microstructures. To the authors’ knowledge, these findings on the Coscinodiscus sp. frustules and fabrication of layered hierarchical prototypes inspired by them are reported here for the first time.
Methodology
Hierarchical CAD model
This study focuses on the mechanical response of centric frustules for two reasons: (i) the radial symmetry in these species simplifies computational modeling as well as visualization, and (ii) experimental data on the mechanics of diatom shells is more plentiful for centric species. More specifically, the Coscinodiscus sp. was chosen as a representative diatom due to its abundance in nature3 and well studied hierarchical morphology.15 Based on SEM data,15 a fully three-dimensional domed CAD model of a radial section (one sixth) of a single valve was created using Creo Parametric 3.0 (PTC, Needham, MA). Due to symmetry, the mechanics of the complete valve can be described by modeling only a radial section by imposing the proper boundary conditions, as explained in the next section. The intricate hierarchical structure of the frustule was reproduced as shown in Fig. 2. Experiments15 have established the Coscinodiscus sp. frustule is composed of three layers: the top layer (Cribellum) is the thinnest and softest and exhibits the smallest pore size; the middle layer (Cribrum) has hexagonal pore arrays; and the bottom layer (Areola) is the thickest and strongest with large toroidal cavities. In Fig. 2, color bars are used to show the dimensions of specific sections within the three layers. The dimensions for the thickness of each layer, different pore sizes, and diameter of the valve were taken from the Coscinodiscus sp. characterization reported elsewhere.15 The average pore diameter in each layer was 45 nm (Cribellum), 192 nm (Cribrum), and 1150 nm (Areola).15 From this initial design, features (e.g. pore size per layer, pore shape) can be modified in a systematic way to study correlations between morphology and mechanical response.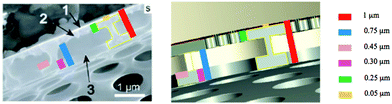 | ||
| Fig. 2 SEM micrograph (left) of a real Coscinodiscus sp. frustule15 and an image (right) of an associated CAD model used for FEM simulations in this study. Labels 1, 2 and 3 denote top, middle and bottom layers characteristic in this frustule.15 Colored scale bars show the dimensions of layers (top: 0.05 μm, middle: 0.25 μm, bottom: 0.3 μm) and associated morphological features (e.g. I-beams) and other sections within the three layers. The average pore diameters in each layer of the frustule model resemble the dimensions reported in experiments.15 | ||
FEM simulation
The CAD models were imported in COMSOL Multiphysics 5.2 (COMSOL, Inc., Burlington, MA) in order to simulate their behavior under compression. The main input variables for these simulations were experimental data gathered from previous works.18 In particular, an isotropic material with a Young's modulus E = 3.1 GPa was used to describe the frustule models. This value was taken from prior experimental studies18 and was used to create an elastic model.The domain was discretized using a fine mesh of tetrahedral elements, taking care to avoid convergence errors by successive refinements of the mesh until stress convergence was reached under a generic load. A fixed boundary condition was imposed at the base of the valve (see Fig. 3) to mimic adhesion to the substrate, a common feature in diatom nanomechanical experiments.16 Symmetry boundary conditions were applied to all the side surfaces to account for the radial symmetry of the valve. Finally, a uniform compression was applied to the top surface of the valve (see left-hand side of Fig. 3) in the vertical direction. The total force of F = 10 μN was evenly distributed over the top surface along the z-direction.
Additionally, a second model was created to study local effects. Taking advantage of the diatom frustule cellular structure, a hexagonal “unit cell” was created (see right-hand side of Fig. 3) as a representative sample of the complete structure. The diatom frustule can thus be considered as an assembly of an arbitrary number of these unit cells.15,17 The reason to study this sub-structure is that it makes it easier to focus on localized phenomena such as stress concentration. In this case, a fixed boundary condition was imposed along the outer edge of the unit cell. The same compression F = 10 μN was applied as before, uniformly distributed over the top surface.
3D prototyping
Diatom-inspired hierarchical CAD models were prepared for prototyping to test the fabrication potential at this scale. Fabrication was achieved using high-precision 3D-DLW, a sophisticated lithographic patterning technology. A Photonic Professional GT system (Nanoscribe GmbH, Eggenstein-Leopoldshafen, Germany)29 was used for printing selected CAD models in IP-Dip photoresist (Nanoscribe GmbH) using “dip-in” lithography, where the optical objective is immersed in the resist during printing. The system utilizes a 780 nm wavelength erbium-doped fiber laser that generates 100 fs pulses with 80 MHz repetition rate, which gets focused through a high numerical aperture (N.A. = 1.4) objective. For the fabrication of our prototypes, the laser beam was scanned with galvo mirrors at 1000 μm s−1 scan speed and 15 mW laser power. For this work, a total of nine different samples were prototyped on a Si substrate in about 24 hours.Results and discussion
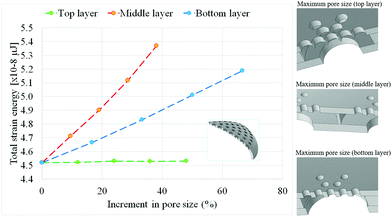
The two layered frustule models (Fig. 3) were used for independent simulations in this study. First, the dome-shaped multilayer symmetry model (left-hand side, Fig. 3) was used to investigate the effect of morphology over the stiffness of the entire diatom frustule. Fig. 4 shows the strain energy results after successive compression tests using the same parameters (boundary conditions, material properties, applied loads) while changing the pore diameter of a single layer. This was done for each layer separately, to isolate the effects of pore size in each case over the complete structure. The inset (right-hand side, Fig. 4) illustrates three extreme cases: the maximum pore size at the top, middle, and bottom layers respectively, with the other layers maintaining their initial pore size. The initial pore sizes for all three layers were chosen to most closely resemble the actual diatom frustule found in nature,15 shown in Fig. 3. The initial pore size was kept constant in other layers while changing it for one specific layer at a time.The total elastic strain energy is inversely related to the overall frustule stiffness. As seen in Fig. 4, successive increases in pore diameter correspond to a linear decrease in frustule stiffness (or increase in strain energy) when these changes occur in the bottom or middle layer of the frustule hierarchical structure. In particular, the middle layer was found to be the main contributor to the frustule stiffness. In the case of the top layer, the variation in strain energy for different pore sizes is negligible. This arises from the comparatively small pore size in this layer with respect to the other two aforementioned (middle and bottom) layers, defined in the Methodology section. The correlation of stiffness (strain energy) with changes in the frustule layer thickness was not included in this study, and future work can build on correlation trends previously reported.18,30
Results in Fig. 4 show the top layer in this model plays a small role in the overall stiffness of the diatom frustule, regardless of pore size. This is mainly due to the top layer being comparatively thinner than its lower counterparts. Indeed, while both the middle and bottom layers play significant roles in stiffness, the middle layer, being thickest, is the dominant one. However, it should be noticed that the proportional relation between strain energy and pore size is more pronounced (slope is higher, see Fig. 4) in the case of the middle layer. Since this layer contains vertical elements (known as I-beams17) resulting from the change in pore size, this difference could be the result of an additional stiffening effect resulting from these I-beam features. It should be noted this I-beam resemblance is simply the result of seeing the cross section of the toroidal cavities created by the bottom and middle layers (Fig. 2). These findings are in good agreement with the possible adaptations to outside forces discussed by Hamm et al.13 and with the mechanisms shown numerically in the work of Lu et al.,17 implying the basal layers are the main structural features giving the frustule its strength. A similar conclusion was reached by Aitken and collaborators16 through three-point bending tests. These results and those from prior studies16,17 imply the mechanical design of diatom-inspired microdevices can focus on the basic structure of the basal layers leaving aside the thin top layer.
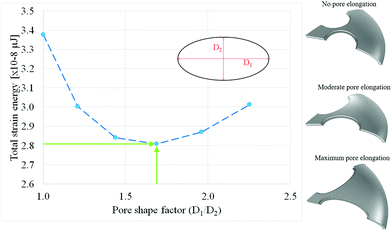
To further investigate the effect of pore geometry on the overall stiffness, the pore shape of the basal (bottom and middle) layers was systematically modified keeping other variables constant. In this sense, a pore shape factor was defined as D1/D2 where D1 and D2 are the major and minor axes of an ellipse. The circular pore commonly found in nature has a shape factor of 1, and for subsequent models, the D1, D2 pairs were chosen to keep the area of the pore constant. Thus the total surface area subject to compressive loads does not change in each simulation. Fig. 5 shows there is at first a stiffening effect (or decreased strain energy) created by elongating the pore as D1 increases, but eventually this tendency is reversed when the pore shape factor D1/D2 is greater than 1.6. One possible explanation for this is that the mechanism of stress distribution changes as the pore elongates, with the initial asymmetry allowing for slightly larger stress concentration at both ends of the major axis. Eventually, however, the elongation is such that local buckling phenomena could appear along the pore walls, decreasing stiffness (i.e. increasing total strain energy), as shown in Fig. 5.
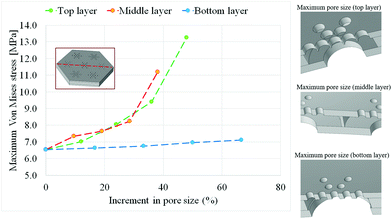
Next, the diatom “unit cell” layered frustule model (right-hand side, Fig. 3) was used to evaluate localized phenomena. This unit cell represents an arbitrary portion of the diatom frustule when treated as a cellular structure. In this sense, the entire frustule would be the repetition of the unit cell over a dome-shaped surface. Fig. 6 shows the maximum von Mises stress for different pore sizes in each layer. As before, the simulations were performed in a systematic fashion changing only the pore size in one single layer each time. It can be seen that pore size at the bottom layer has little effect on the maximum equivalent stress. Pore size in the middle and top layers has a much more noticeable effect, with the top layer being the dominant element. This effect was expected since the load was applied on the top layer, which is also the thinnest layer.
In all cases studied above, the stress concentration was found to be at the top layer, with the pores acting as stress concentrators in a manner according with standard structural mechanics. In general, the maximum stress concentration was found along the center line of the model (Fig. 6).
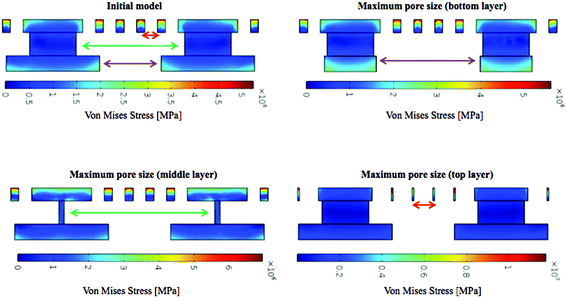
Further results in Fig. 7 illustrate the cut plane defined by the line of maximum stress for the three most extreme cases studied (maximum pore size for each layer). It can be seen (Fig. 7) that the stress distributions in the frustule layers remain qualitatively the same in each case, with only the maximum stress value changing.
Results in Fig. 6 and 7 indicate the top layer is the main feature subject to stress and hence the dominant feature defining stress concentration. Since the uniform compression load is applied over this layer, the pores in this case act as stress concentrators. Increasing the diameter of the pores implies that a comparatively small surface (see Fig. 7 lower-right) will carry the load. While there is a modest transfer of stress towards the lower layers when decreasing their pore sizes (as shown in Fig. 7), the top layer remains the critical factor. Generally, stress concentration is an undesirable phenomenon from a structural perspective, so the fact that the outside layer favors this effect could indicate an adaptation towards isolating stresses on the external layers and avoiding them in the inside of the frustule. This stress concentration phenomenon can help explain the “ridges and valleys” morphology observed in characterization studies (such as that of Losic et al.15) in adaptive terms: the stress concentration around the pore rims pushes an increase in shell thickness during morphogenesis. This opens the possibility of a new area of study: that of controlled growth of diatom shells in the laboratory by introducing mechanical forces during the silica addition phase of diatom valve formation.3
In addition, some of the geometries studied were manufactured using 3D-DLW to test the feasibility of this manufacturing technique to produce diatom-inspired microdevices. The main reason to pursue these prototypes was to demonstrate the geometries simulated are indeed feasible for fabrication at a diatom scale, instead of mere conceptual studies. Due to the resolution of the 3D-DLW equipment,29 structures were enlarged by a scale factor of 5![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, thus the resulting polymeric prototypes have an outer diameter of 250 μm and pore diameters ranging from 0.75 μm to 6 μm. Fig. 8 presents the results of this prototyping via SEM micrographs. In addition to the “natural” Coscinodiscus sp. geometries (Fig. 2 and 4), some supplementary hierarchical structures were manufactured to explore different potential design directions. As shown in Fig. 8, the detailed features of diatom frustules were successfully reproduced, even in the case of the small basal pores. This high-resolution technology is thus capable of reproducing the intricate features of frustules (e.g. pore sizes, pore shapes, and basal layers) and potentially facilitating the fabrication of diatom-inspired microdevices.
1, thus the resulting polymeric prototypes have an outer diameter of 250 μm and pore diameters ranging from 0.75 μm to 6 μm. Fig. 8 presents the results of this prototyping via SEM micrographs. In addition to the “natural” Coscinodiscus sp. geometries (Fig. 2 and 4), some supplementary hierarchical structures were manufactured to explore different potential design directions. As shown in Fig. 8, the detailed features of diatom frustules were successfully reproduced, even in the case of the small basal pores. This high-resolution technology is thus capable of reproducing the intricate features of frustules (e.g. pore sizes, pore shapes, and basal layers) and potentially facilitating the fabrication of diatom-inspired microdevices.
The prototypes in Fig. 8 indicate that 3D-DLW technology replicates diatom geometries with great accuracy. While a scale of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5 was necessary to guarantee reproduction of the small pores in the top layer, the prototypes have dimensions sufficiently small for their mechanical behavior to reasonably correspond to their natural counterparts. The capacity to fabricate diatom-inspired devices at this scale is of great value to biomedical applications. One promising example is the design of diatom-inspired drug delivery implants5,15 that use the hierarchical pore arrangements to regulate diffusive drug release. The geometries studied in this work are a promising starting point to the fabrication of such devices, potentially achievable via biocompatible materials.
5 was necessary to guarantee reproduction of the small pores in the top layer, the prototypes have dimensions sufficiently small for their mechanical behavior to reasonably correspond to their natural counterparts. The capacity to fabricate diatom-inspired devices at this scale is of great value to biomedical applications. One promising example is the design of diatom-inspired drug delivery implants5,15 that use the hierarchical pore arrangements to regulate diffusive drug release. The geometries studied in this work are a promising starting point to the fabrication of such devices, potentially achievable via biocompatible materials.
The simulation results (Fig. 5) showing the effect of pore shape on overall frustule stiffness infer that prototypes with varied pore shapes are in principle feasible to fabricate. In particular, layered prototypes with pore factors of D1/D2 > 1.6 (elongated pores) are predicted to exhibit decreasing frustule stiffness values, which are not readily measurable experimentally. Candidate prototyping materials and resolution requirements would involve soft materials (e.g. polymers) and high-resolution technology. Therefore, predictive simulations and geometries (Fig. 5) led to the successful fabrication of diatom-inspired layered structures with tailored pore sizes and pore shapes (Fig. 8) using sub-micron 3D-DLW lithography. Future experiments will be necessary to perform actual measurements in layered prototypes and to advance cellular materials research.
The level of precision achieved in the 3D-DLW prototypes is encouraging and could open the option to finely tuned geometries for specific applications. For example, starting from the relationship between pore shape and mechanical response (Fig. 5), a diatom-inspired microdevice can be fabricated to behave in a desired way when subject to external stimuli. Given the small scale of these geometries, microfluidic effects, micromechanical devices, or even photonic devices6 can be fabricated following the principles presented in this work.
Conclusions
Overall findings from this study indicate that morphological features such as pores and layers have significant effects on the mechanical behavior of Coscinodiscus sp. frustules under compression. Specifically, basal layers contribute distinctively to the overall mechanical response of the frustule. While the very small pores in the top layer seem to be subject to stress concentration, the geometry of the middle layer contributes most to the overall frustule stiffness. This suggests the pores in the top layer could act as initial flaws and control crack propagation, which would agree with the results presented by Aitken et al.16 To validate this hypothesis, further studies are necessary to determine whether it is valid to assume all three layers have similar mechanical properties. If, for example, the top layer was significantly softer, there would be no stress concentration on its pores and the mechanical performance of the frustule would only depend on the basal layers. Future directions regarding simulations will include investigating the effect of layer thickness on frustule stiffness and applying optimization methods to identify desirable combination of mechanical properties (e.g. high strength, high toughness).This work presents a promising path towards the design and fabrication of diatom-inspired devices. The correlations between hierarchical features and mechanical behavior opens the door to tailored diatom-inspired microdevices relying on structure–property relations as a working principle. According to the results obtained, stiffness-oriented applications should focus on the geometry of the bottom and middle layers, while the top layer should be the main feature in devices where stress distribution is a limiting factor. The feasibility of 3D-DLW technology for the fabrication of diatom-inspired devices is shown in this work. While a 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 scale for these prototypes remains to be achieved (i.e. for prototypes including small features in the top layer), the fidelity of the resulting geometries is promising for future experimental mechanical studies.
1 scale for these prototypes remains to be achieved (i.e. for prototypes including small features in the top layer), the fidelity of the resulting geometries is promising for future experimental mechanical studies.
The simulations showing the effect of pore shape on overall frustule stiffness infer that prototypes with varied pore shapes are feasible to fabricate. Specifically, layered prototypes with elongated pore shapes (D1/D2 > 1.6) are predicted to show decreasing frustule stiffness values. The connection of predictive simulations and pore geometries (Fig. 5) with the sub-micron 3D prototyping of diatom-inspired layered structures with tailored pore sizes and pore shapes (Fig. 8) was accomplished. Advanced experiments will be needed in the future to obtain measurements from layered porous prototypes and accelerate cellular materials research further. An immediate continuation of this work would be the fabrication of diatom-inspired prototypes using different materials. 3D-DLW lithography utilizes photosensitive resins (photo-resist materials), resulting in soft polymer-based prototypes. Future work will focus on pursuing mechanical tests of the Nanoscribe samples (e.g. via AFM) and investigating different photosensitive resins containing a percentage of silica, which potentially would result in harder prototypes that can also be subject to mechanical testing. The exploration of such photo-resist materials will depend on the successful polymerization using a 3D-DLW system such as the Photonic Professional GT. Another possibility is using the current soft prototypes as templates to fabricate hard replicas using atomic layer deposition or similar techniques. Ultimately, the combination of simulation, prototyping and experimentation will likely provide an integrated framework for the design of diatom-inspired human-made structures and devices.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
The authors would like to thank Mary Ann Tiffany (San Diego State University, retired) for offering her high-resolution SEM micrographs and M. Dunlap (University of California, Merced) for technical assistance. The SEM used to image the prototypes is located in the Imaging and Microscopy Facility at the University of California, Merced. This work was performed under the auspices of an internal grant, which supported a co-author (AG). In addition, a research award from the Academic Senate Committee on Research at the University of California, Merced provided funds for research supplies and conference expenses. Dedicated to the memory of Luis Dávila Negrón, a legacy of love and authenticity (father of co-author LD).References
- G. Fleming and M. Ratner, Grand challenges in basic energy sciences, Phys. Today, 2008, 61(7), 28 CrossRef.
- NSF, Dear Colleague Letter: Design Materials to Revolutionize and Engineer our Future (DMREF), [Online]. Available: http://www.nsf.gov/pubs/2011/nsf11089/nsf11089.jsp.
- F. Round, R. Crawford and D. Mann, The Diatoms, Biology & Morphology of the Genera, Cambridge University Press, Cambridge, 1990 Search PubMed.
- R. Drum and R. Gordon, Star Trek Replicators and Diatom Nanotechnology, Trends Biotechnol., 2003, 21(8), 325–328 CrossRef CAS PubMed.
- R. Gordon, D. Losic, M. Tiffany, S. Nagy and F. A. S. Sterrenburg, The Glass Menagerie: diatoms for novel applications in nanotechnology, Trends Biotechnol., 2009, 27(2), 116–127 CrossRef CAS PubMed.
- A. Parker and H. Townley, Biomimetics of Photonic Nanostructures, Nat. Nanotechnol., 2007, 2(6), 347–353 CrossRef CAS PubMed.
- N. Kroger and N. Poulsenn, Diatoms: From Cell Wall Biogenesis to Nanotechnology, Annu. Rev. Genet., 2008, 42, 83–107 CrossRef CAS PubMed.
- L. De Stefano, I. Rendina, M. De Stefano, A. Bismuto and P. Maddalena, Marine Diatoms as Optical Chemical Sensors, Appl. Phys. Lett., 2005, 87(23), 2339021–2339023 CrossRef.
- A. Garcia, D. Sen and M. Buehler, Hierarchical Silica Nanostructures Inspired by Diatom Algae Yield Superior Deformability, Toughness, and Strength, Metall. Mater. Trans. A, 2011, 42(13), 3889–3897 CrossRef CAS.
- C. Hamm, R. Merkel, O. Springer, P. Jurkojc, C. Maier, K. Prechtel and V. Smetacek, Architecture and material properties of diatom shells provide effective mechanical protection, Nature, 2003, 421, 841–843 CrossRef CAS PubMed.
- N. Almqvist, Y. Delamo, B. L. Smith, N. H. Thomson, Å. Bartholdson, R. Lal, M. Brzezinski and P. K. Hansma, Micromechanical and structural properties of a pennate diatom investigated by atomic force microscopy, J. Microsc., 2001, 202(3), 518–532 CrossRef CAS PubMed.
- G. Subhash, S. Yao, B. Bellinger and M. Gretz, Investigation of mechanical properties of diatom frustules using nanoindentation, J. Nanosci. Nanotechnol., 2005, 5(1), 50–56 CrossRef CAS PubMed.
- C. Hamm, The evolution of advanced mechanical defenses and potential technological applications of diatom shells, J. Nanosci. Nanotechnol., 2005, 5(1), 108–119 CrossRef CAS PubMed.
- P. Pondaven, Grazing-induced changes in cell wall silicification in a marine diatom, Protist, 2007, 158(1), 21–28 CrossRef CAS PubMed.
- D. Losic, G. Rosengarten, J. G. Mitchell and N. H. Voelcker, Pore Architecture of Diatom Frustules: Potential Nanostructured Membranes for Molecular and Particle Separations, J. Nanosci. Nanotechnol., 2006, 6(4), 1–8 CrossRef.
- Z. Aitken, S. Luo, S. Reynolds, C. Thaulow and J. Greer, Microstructure provides insights into evolutionary design and resilience of Coscinodiscus sp. frustule, Proc. Natl. Acad. Sci. U. S. A., 2016, 113(8), 2017–2022 CrossRef CAS PubMed.
- J. Lu, C. Sun and J. Wang, Mechanical Simulation of a Diatom Frustule Structure, J. Bionic Eng., 2015, 12(1), 98–108 CrossRef.
- M. Diaz Moreno, K. Ma, J. Schoenung and L. P. Dávila, An integrated approach for probing the structure and mechanical properties of diatoms: toward engineered nanotemplates, Acta Biomater., 2015, 25, 313–324 CrossRef PubMed.
- A. Razul, A review of micro-manufacturing, micro-forming, and their key issues, Procedia Eng., 2013, 53, 665–672 CrossRef.
- J. Liow, Mechanical micromachining: a sustainable micro-device manufacturing approach?, J. Cleaner Prod., 2009, 17(7), 662–667 CrossRef.
- S. Giannitelli, P. Mozetic, M. Trombetta and A. Rainer, Combined additive manufacturing approaches in tissue engineering, Acta Biomater., 2015, 24, 1–11 CrossRef CAS PubMed.
- C. Dixon and O. Curtines, Nanotechnology: Nanofabrication, patterning, and self-assembly, Nova Science Publishers Incorporated, 2009 Search PubMed.
- J. McDonald and G. Whitesides, Poly(dimethylsiloxane) as a material for fabricating microfluidic devices, Acc. Chem. Res., 2002, 35, 491–499 CrossRef CAS PubMed.
- M. Walsh, F. Zhang, R. Menon and H. Smith, Maskless photolithography, in Nanolithography, Woodhead publishing, 2014, pp. 179–193 Search PubMed.
- Y. Hwang, O. Paydar and R. Candler, 3D printed molds for non-planar PDMS microfluidic channels, Sens. Actuators, A, 2015, 226, 137–142 CrossRef CAS.
- M. Belegratis, V. Schmidt, D. Nees, B. Stadlober and P. Hartmann, Diatom-inspired templates for 3D replication: natural diatoms versus laser written artificial diatoms, Bioinspiration Biomimetics, 2014, 9(1), 0160041–01600411 Search PubMed.
- W. Xiong, L. Jiang, T. Baldacchini and Y. Lu, Laser additive manufacturing using nanofabrication by integrated two-photon polymerization and multiphoton ablation, in Laser Additive Manufacturing, Woodhead Publishing, 2017, pp. 237–256 Search PubMed.
- D. Jang, L. Meza, F. Greer and J. Greer, Fabrication and deformation of three-dimensional hollow ceramic nanostructures, Nat. Mater., 2013, 12, 893–898 CrossRef CAS PubMed.
- Nanoscribe, High-precision 3D-LW, June 2015. [Online]. Available: http://www.nanoscribe.de/en/.
- A. Gutierrez, R. Gordon and L. P. Davila, Deformation modes and structural response of diatom frustules, J. Mater. Sci. Eng. Adv. Technol., 2017, 15, 105–134 Search PubMed.
| This journal is © The Royal Society of Chemistry 2018 |