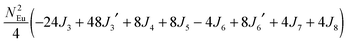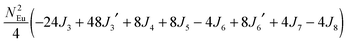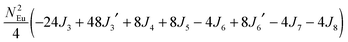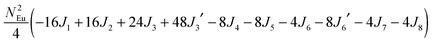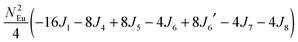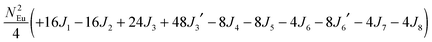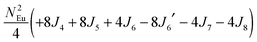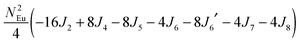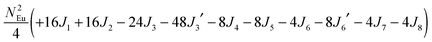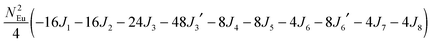DOI:
10.1039/C7QM00057J
(Research Article)
Mater. Chem. Front., 2017,
1, 1563-1572
Synthesis, crystal and electronic structures, physical properties and 121Sb and 151Eu Mössbauer spectroscopy of the alumo-antimonide Zintl-phase Eu5Al2Sb6†
Received
7th February 2017
, Accepted 8th March 2017
First published on 16th March 2017
Abstract
Eu5Al2Sb6 was synthesized from the elements in niobium ampoules. It crystallizes in the orthorhombic crystal system (a = 1027.55(7), b = 1200.28(6), c = 1324.22(7) pm) with space group Pnma (no. 62), isostructural to Sr5Al2Sb6, and can be described as a Zintl phase. The Al atoms are tetrahedrally surrounded by Sb atoms, forming branched strands. Besides Sb3− anions, antimony Sb24− dumbbells can also be found in the crystal structure. Eu5Al2Sb6 [≡(Eu2+)5(Al3+)2(Sb3−)4(Sb24−)] exhibits three magnetic ordering phenomena at T1 = 12.3(1), T2 = 10.6(1) and T3 = 3.0(1) K, obtained by heat capacity measurements. While T3 is of antiferromagnetic nature, T1 and T2 correspond to canted antiferromagnetic transitions. Resistivity investigations indicate that the title compound is a semiconductor, in line with the band structure calculations. Spin exchange parameters, calculated using mapping analysis, confirm that the magnetic phase transition at TN = 3.5(1) is associated with the ordering of Eu1 atoms. The divalent character has been confirmed by 151Eu Mössbauer spectroscopic studies. Measurements conducted at 5 K show a hyperfine field splitting for two of the three Eu sites, underlining the magnetic data. Additionally, 121Sb Mössbauer spectroscopic studies have been conducted on Eu5Al2Sb6 and isostructural Sr5Al2Sb6.
Introduction
Zintl phases are a class of materials that can be positioned between intermetallic compounds and insulating valence compounds from an electronic perspective.1 Usually they contain an electropositive element from group 1 or 2 which, in a formal sense, transfers its electrons to an electronegative element from group 13, 14, or 15. A more concise definition of a Zintl compound is that they are materials in which both ionic and covalent bonding is found. If the anionic component of the structure has sufficient electrons, they will form isolated anions, isoelectronic to the noble gases. An example would be Mg2Si, which adopts an anti-CaF2 type structure with isolated Si4− anions.2 In cases of insufficient electrons, the main group elements form regular covalent bonds (2c–2e bonds), e.g. CaSi2 where the Si22− anion forms corrugated layers analogous to grey As.3 Besides the ‘classical’ binary Zintl phases numerous ternary compounds, also transition metal containing ones, have been found.4 Usually Zintl compounds are considered to be semiconductors, although compounds that are metallic in nature have also been found. The semiconducting properties make Zintl compounds an important class of materials for, as an example, thermoelectric applications.5 The aforementioned Mg2Si is a good candidate for automotive waste heat recovery systems due to the low density and abundance of the constituent elements.6,7 Yb14MnSb118,9 and Yb9Mn4.2Sb910 are examples of antimony-containing high ZT thermoelectric materials. While in the ternary system Sr–Al–Sb three compounds are known (Sr5Al2Sb6,11,12 Sr14AlSb1113 and Sr3AlSb314) and have been characterized with respect to their properties, no compounds have been reported for the related system Eu–Al–Sb. Due to the comparable ionic radii of Eu2+ (117 pm) and Sr2+ (127 pm)15 known strontium representatives are a good starting point for the synthesis and characterization of isostructural europium compounds. Amongst the alkaline-earth metal group 13 antimonides several compounds with 5–2–6 composition are found. They can be grouped with respect to their prototypes, the Ca5Ga2As6 (Pnma, no. 62) or Ca5Al2Bi6 (Pbam, no. 55) type structures. For the latter one, the Yb5M2Sb616–20 (M = Al, Ga, In) series is found, while for the Ca5Ga2As6 type structure, e.g. Ca5Al2Sb6,21 Ca5Ga2Sb6,22,23 Ca5In2Sb6,22 Ca5Al2−xInxSb6,24 Sr5In2Sb6,22,25 Eu5In2Sb626,27 and Ba5In2Sb611 have been reported. Both compounds exhibit [MSb4] tetrahedra and Sb24− units; only the packing of the structural elements is slightly different. All of the aforementioned compounds exhibit intriguing thermoelectric properties. Here we report on the first compound in the Eu–Al–Sb system, Eu5Al2Sb6. We have investigated its crystal structure, the physical properties (magnetism and electrical resistivity) along with 121Sb and 151Eu Mössbauer spectroscopic investigations of the bulk material. Theoretical calculations have been used to elucidate the electronic and magnetic structures of Eu5Al2Sb6 and Bader charges in comparison with the isotypic strontium compound.
Experimental
Synthesis
The starting materials for the syntheses of the Eu5Al2Sb6 and Sr5Al2Sb6 samples were europium ingots (Alfa Aesar, 99.9%), strontium pieces (Sigma Aldrich, 99.0%), aluminium turnings (Koch Chemicals, 99.99%) and antimony pieces (ChemPur, 99.999%). The europium ingots and strontium pieces were stored under argon; the surface oxides were removed prior to the synthesis. The argon was purified over molecular sieves, silica gel and a titanium sponge (873 K). The elements were weighed in the ideal atomic ratio and arc-welded28 in niobium tubes in a dried argon atmosphere of about 800 mbar. The metal tubes were subsequently placed in the water-cooled sample chamber of a high-frequency furnace (Hüttinger Elektronik, Freiburg, type TIG 1.5/300) under flowing argon.29 The temperature was controlled by a Sensor Therm Methis MS09 pyrometer with an accuracy of ±50 K. The samples were rapidly heated to 1273 K, after 10 min the temperature was reduced to 1123 K and the samples were annealed for two hours. After the annealing step, the samples were cooled to room temperature within two hours. The samples were obtained as brittle polycrystalline pieces with metallic lustre and could easily be separated from the crucible material. No reaction with the containers was observed. The compounds showed moderate sensitivity to moisture and were therefore stored under dry argon atmosphere.
EDX data
Semiquantitative EDX analysis of the single crystal that has been investigated on the diffractometer was carried out by use of a Zeiss EVO® MA10 scanning electron microscope in variable pressure mode with EuF3, Al2O3 and Sb as standards. The experimentally observed composition (39(2) at% Eu: 12(2) at% Al: 49(2) at% Sb) was close to the theoretical (38.5 at% Eu: 15.4 at% Al: 46.1 at% Sb) and refined one and no impurity elements heavier than sodium (detection limit of the instrument) were detected.
Powder X-ray diffraction
The polycrystalline sample was characterized by the Guinier powder pattern using CuKα1 radiation and α-quartz (a = 491.30 and c = 540.46 pm) as an internal standard. The Guinier camera was equipped with an imaging plate (Fuji film, BAS-READER 1800). Least-squares refinements led to the lattice parameters listed in Table 1. To ensure correct indexing, the experimental pattern was compared to the calculated one using the refined atomic positions (vide infra) obtained from single crystal X-ray diffraction.30 The diffraction pattern is depicted in Fig. S1 (ESI†).
Table 1 Crystallographic data and details of the room temperature single crystal structure refinement of Eu5Al2Sb6 (Sr5Al2Sb6 type, Pnma, no. 62, Z = 4)
| Lattice parameter, (pm) |
a = 1027.55(7) |
|
b = 1200.28(6) |
|
c = 1324.22(7) |
| Volume, V (nm3) |
1.628(1) |
| Molar mass (g mol−1) |
1544.3 |
| Calc. density, Dx (g cm−3) |
6.30 |
| Abs. coeff. μ (mm−1) |
28.8 |
| F(000) (e) |
2588 |
| Crystal size (μm3) |
90 × 50 × 45 |
| Transmission ratio (min/max) |
0.074/0.292 |
| Detector distance (mm) |
70 |
| Exposure time (min) |
10 |
| Integr. parameters A/B/EMS |
13.0/2.7/0.015 |
|
θ range (deg) |
2.3–36.3 |
| Range in hkl |
±17; ±14; ±18 |
| Total no. reflections |
26![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 749 749 |
| Independent reflections/Rint |
2570 (0.0626) |
| Reflections with I ≥ 3σ(I)/Rσ |
2192 (0.0112) |
| Data/parameters |
2570/71 |
| Goodness-of-fit on F2 |
1.68 |
|
R
1/wR2 for I ≥ 3σ(I) |
0.0244/0.0514 |
|
R
1/wR2 for all data |
0.0330/0.0531 |
| Extinction coefficient |
124(10) |
| Largest diff. peak/hole (e Å−3) |
+1.52/−1.88 |
Single crystal X-ray diffraction
Single crystals were isolated from the crushed samples. The crystal fragments were glued to thin quartz fibres using beeswax and investigated by Laue photographs on a Buerger camera (white Mo radiation, Fujifilm imaging plate, BAS-1800) in order to check their quality for intensity data collection. The intensity data set was collected at room temperature by using a Stoe IPDS II (graphite-monochromatized MoKα radiation; oscillation mode) diffractometer. Numerical absorption correction was applied to the data set. Details about the data collection and the crystallographic parameters are summarized in Tables 1–3.
Table 2 Atomic coordinates and isotropic displacement parameters Ueq (pm2) for Eu5Al2Sb6 (Sr5Al2Sb6 type, space group Pnma, Z = 4). Standard deviations are given in parentheses
| |
Wyckoff site |
x
|
y
|
z
|
U
eq
|
| Eu1 |
4c |
0.16073(4) |
1/4 |
0.68713(3) |
165(1) |
| Eu2 |
8d |
0.08106(2) |
0.02636(3) |
0.26332(2) |
161(1) |
| Eu3 |
8d |
0.33297(3) |
0.52005(3) |
0.46349(2) |
192(1) |
| Al1 |
4c |
0.4226(2) |
1/4 |
0.0655(2) |
152(6) |
| Al2 |
4c |
0.3128(2) |
1/4 |
0.2982(2) |
141(6) |
| Sb1 |
4c |
0.35383(5) |
1/4 |
0.87598(4) |
158(1) |
| Sb2 |
8d |
0.32703(3) |
0.05337(4) |
0.16923(3) |
144(1) |
| Sb3 |
4c |
0.15063(5) |
1/4 |
0.43721(4) |
158(1) |
| Sb4 |
4c |
0.45364(5) |
1/4 |
0.62173(4) |
157(1) |
| Sb5 |
4c |
0.00550(5) |
1/4 |
0.09135(4) |
145(1) |
Table 3 Interatomic distances (in pm) in the structure of Eu5Al2Sb6 (Sr5Al2Sb6 type, space group Pnma, Z = 4). Standard deviations are smaller or equal to ±0.1 pm. All distances <400 pm are listed
| Eu1 |
1 |
Sb4 |
313.0 |
Eu2 |
1 |
Sb2 |
277.6 |
Eu3 |
1 |
Sb2 |
320.5 |
Al1 |
1 |
Sb3 |
234.2 |
| 1 |
Sb1 |
319.0 |
1 |
Sb2 |
283.4 |
1 |
Sb1 |
355.2 |
1 |
Sb1 |
260.5 |
| 1 |
Sb1 |
326.0 |
1 |
Sb5 |
360.1 |
1 |
Eu3 |
359.6 |
2 |
Sb2 |
289.9 |
| 1 |
Sb4 |
330.4 |
1 |
Sb3 |
360.5 |
1 |
Sb5 |
363.5 |
1 |
Al2 |
327.9 |
| 1 |
Sb3 |
330.8 |
1 |
Al2 |
361.4 |
1 |
Sb4 |
369.8 |
2 |
Eu2 |
386.9 |
| 2 |
Sb2 |
364.7 |
1 |
Sb1 |
369.4 |
1 |
Eu2 |
374.4 |
|
|
|
|
|
|
|
1 |
Eu3 |
374.4 |
1 |
Sb3 |
375.6 |
|
|
|
|
|
|
|
1 |
Sb4 |
382.3 |
1 |
Sb5 |
376.1 |
|
|
|
|
|
|
|
1 |
Al1 |
386.9 |
1 |
Al2 |
391.3 |
|
|
|
|
|
|
|
1 |
Al2 |
392.9 |
1 |
Eu2 |
397.6 |
|
|
|
|
|
|
|
1 |
Eu3 |
397.6 |
1 |
Sb2 |
399.2 |
|
|
|
|
|
| Al2 |
1 |
Sb5 |
246.0 |
Sb1 |
1 |
Al1 |
260.5 |
Sb2 |
1 |
Eu2 |
277.6 |
Sb3 |
1 |
Al1 |
234.2 |
| 1 |
Sb3 |
248.2 |
1 |
Eu1 |
319.0 |
1 |
Eu2 |
283.4 |
1 |
Al2 |
248.2 |
| 2 |
Sb2 |
291.4 |
1 |
Eu1 |
326.0 |
1 |
Al1 |
289.9 |
1 |
Eu1 |
330.8 |
| 1 |
Al1 |
327.9 |
1 |
Sb4 |
351.7 |
1 |
Al2 |
291.4 |
2 |
Eu2 |
360.5 |
| 2 |
Eu2 |
361.4 |
2 |
Eu3 |
355.2 |
1 |
Eu3 |
320.5 |
1 |
Sb5 |
366.3 |
| 2 |
Eu3 |
391.3 |
2 |
Eu2 |
369.4 |
1 |
Eu1 |
364.7 |
2 |
Eu3 |
375.6 |
| 2 |
Eu2 |
392.9 |
1 |
Sb3 |
392.4 |
1 |
Eu3 |
399.2 |
1 |
Sb1 |
392.4 |
|
|
|
|
|
|
|
|
|
|
1 |
Sb4 |
395.5 |
|
|
| Sb4 |
1 |
Sb5 |
286.9 |
Sb5 |
1 |
Al2 |
246.0 |
|
|
|
|
|
|
|
|
| 1 |
Eu1 |
313.0 |
1 |
Sb4 |
286.9 |
|
|
|
|
|
|
| 1 |
Eu1 |
330.4 |
2 |
Eu2 |
360.1 |
|
|
|
|
|
|
| 1 |
Sb1 |
351.7 |
2 |
Eu3 |
363.5 |
|
|
|
|
|
|
| 2 |
Eu3 |
369.8 |
1 |
Sb3 |
366.3 |
|
|
|
|
|
|
| 2 |
Eu2 |
382.3 |
2 |
Eu3 |
376.1 |
|
|
|
|
|
|
| 1 |
Sb3 |
395.5 |
|
|
|
|
|
|
|
|
|
Physical property measurements
The powdered samples of Eu5Al2Sb6 and Sr5Al2Sb6 were packed in polyethylene (PE) capsules and attached to the sample holder rod of a Vibrating Sample Magnetometer unit (VSM) for measuring the magnetization M(T,H) in a Quantum Design physical property measurement system (PPMS). The samples were investigated in the temperature range of 2.5–300 K with magnetic fields up to 80 kOe.
For the heat capacity measurement of Eu5Al2Sb6, one piece of the sample was fixed to a pre-calibrated heat capacity puck using Apiezon N grease and investigated in the temperature range of 2.0 to 100 K.
A cold pressed tablet (∅ 4.2 mm) of Eu5Al2Sb6 was annealed at 773 K for 4 weeks. The sintered material (Archimedes density 90(1)%) was used for the resistivity experiments. The measurements were carried out in the AC transport mode31 of a Quantum-Design physical property measurement system (PPMS). The ACT puck was modified by a van-der-Pauw press contact assembly purchased from Wimbush Science & Technology. The probes are spring contacts, gold plated over nickel; the distance between the pins was set to 2 mm. The resistivity was measured between 2–300 K with a data point every 0.1 K up to 20 K and one data point every 1 K up to 300 K. The AC frequency was set to 17 Hz with a measurement time of 1 s. The recorded data of channel 1 & 2 was converted according to the van-der-Pauw equation given in the Quantum Design Application Note 1076-304.
Mössbauer spectrosopy
The 21.53 keV transition of 151Eu with an activity of 130 MBq (2% of the total activity of a 151Sm:EuF3 source) and a Ba121mSnO3 source were used for the 151Eu and 121Sb Mössbauer spectroscopic experiments, which were conducted in the usual transmission geometry. The measurements were performed with a commercial helium-bath cryostat and a liquid nitrogen bath cryostat. The temperature of the absorber was set to 5 and 78 K, while the source was kept at room temperature. The temperature was controlled by a resistance thermometer (∼0.5 K accuracy). The sample was enclosed in a small PVC container, and the required sample mass was calculated based on the work by Long et al.32 Fitting of the spectrum was performed with the NORMOS-90 program system.33
Theoretical methodology
Electronic structure calculations on Eu5Al2Sb6 and Sr5Al2Sb6 were performed using the projector augmented wave method (PAW) of Blöchl34,35 coded in the Vienna ab initio simulation package (VASP).36,37 All VASP calculations employed the generalized gradient approximation (GGA) with exchange and correlation treated by Perdew–Burke–Enzerhoff (PBE).38 To describe the electron correlation associated with Eu 4f states, an on-site Hubbard repulsion U parameter39 was applied with an effective Ueff = 6 eV on the Eu atom. The cutoff energy for the plane wave calculations was set to 500 eV and the Brillouin zone integration was carried out using 6 × 7 × 5 Γ-centered k-point meshes. The Bader charge analysis was based on VASP calculations with subsequent calculations with the Bader program developed by the Henkelman group.40–42
Results and discussion
Crystal chemistry
Eu5Al2Sb6 crystallizes in the orthorhombic crystal system with the space group Pnma (no. 62) and lattice parameters of a = 1027.55(7), b = 1200.28(6), c = 1324.22(7) pm, isostructural to Sr5Al2Sb6, which forms the respective prototype.11 The structure can be considered as the Zintl phase according to (Eu2+)5(Al3+)2(Sb3−)4(Sb24−) with Sb24− being covalently bound dumbbells. An extended view of the crystal structure of Eu5Al2Sb6 is shown in Fig. 1. The Al and Sb atoms form a polyanionic [Al2Sb6]10− framework, while the Eu cations are found in cavities. The polyanion can be described as a branched strand of distorted edge- and corner-sharing aluminium centred tetrahedra running along the a axis (Fig. 2, top). Two Sb2 atoms form the shared edge while the Sb3 atoms are corner-shared. The Sb1 and (Sb4–Sb5) dumbbells finally complete the coordination environments (Fig. 2, top). The shortest interatomic distances can be found between Al and Sb (264–274 pm), which are in the range of the sum of the covalent radii (125 + 141 = 266 pm43). In addition Sb–Sb (289 pm) contacts can be found which are in line with the sum of the covalent radii (2 × 141 = 282 pm43) of the element and with an antimony-antimony single bond observed in other Zintl phases e.g., BaSb2 (CaSb2 type: 291 pm;44 BaSb2 type: 291–296 pm45) or EuSb2 (CaSb2 type: 293 pm46). The coordination environment of the Sb24− anion is depicted in Fig. 2 (bottom). In between the polyanion three crystallographically independent Eu atoms are found with coordination numbers of seven and eight and interatomic distances from 320 to 397 pm (Fig. 3).
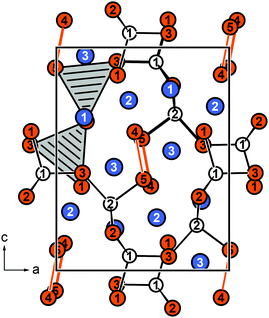 |
| | Fig. 1 Extended view of the crystal structure of Eu5Al2Sb6 along [010]. The bonding within the polyanion is highlighted along with the tetrahedral coordination environments surrounding the Al atoms. Eu atoms are depicted in blue, Al atoms as open white circles and Sb atoms in orange. | |
 |
| | Fig. 2 (top) Branched strand-like [Al2Sb6]10− polyanion of the Zintl phase Eu5Al2Sb6 running along [100]; (bottom) coordination environment and interatomic distances, given in pm, of the Sb24− anion; the Sb–Sb bond is highlighted. | |
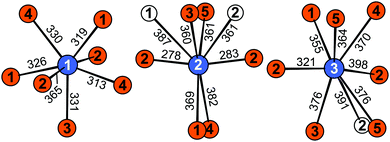 |
| | Fig. 3 Coordination environments surrounding the three crystallographically independent Eu atoms in Eu5Al2Sb6. Interatomic distances are given in pm. Eu atoms are depicted in blue, Al atoms as open white circles and Sb atoms in orange. | |
Magnetic measurements
A high-field susceptibility measurement of Eu5Al2Sb6 conducted in ZFC mode at 10 kOe (Fig. 4, top panel) indicates magnetic ordering at low temperatures and paramagnetic behaviour above the Curie-temperature. A Curie–Weiss fit of the reciprocal magnetic susceptibility in the paramagnetic region (50–300 K) was used to determine the effective magnetic moment (μeff = 8.32(1) μB per Eu atom) and the Weiss constant (θp = −1.8(1) K). The magnetic moment is slightly higher than the calculated moment of a Eu2+ ion (μcalc = 7.94 μB) but still indicates that the europium atoms are divalent. To obtain more information about the magnetic ground state ZFC/FC (zero-field-cooled/field-cooled) measurements at 100 Oe were conducted. Fig. 4 (middle panel) depicts the measurement indicating at least two anomalies: an antiferromagnetic transition at TN = 3.5(1) K and a second transition at T = 12.5(1) K. Heat capacity measurements (depicted in red in Fig. 4, middle panel) reveal the presence of three intrinsic magnetic ordering phenomena at T1 = 12.3(1), T2 = 10.6(1) and T3 = 3.0(1) K. While T1 and T3 are in line with the ZFC/FC experiment, T2 must correspond to the change of slope between 8–12 K, visible in the 100 Oe susceptibility measurements. The intensity of the features in the CP curve characterizes them all as transitions corresponding to the material itself. While the transition at TN = 3.5(1) K is undoubtedly of antiferromagnetic nature, T1 and T2 are not as clear to interpret. Since the susceptibility rises below T = 12.5(1) K a second antiferromagnetic transition (AFM) can be ruled out. A ferromagnetic transition (FM) on the other hand would be more dominant in nature. Mössbauer spectroscopic studies (vide infra) revealed that the AFM transition at TN = 3.5(1) K has to be associated with the Eu1 site (4c). Getting back to the magnitude of the transition – since both plain AFM and FM transitions can be ruled out with respect of the magnitude of the magnetic transitions, a canted antiferromagnetic state is the only explanation remaining. The net moment of the Eu2 and Eu3 sites is small causing only a small rise in the low field susceptibility measurements. Finally magnetization isotherms were recorded at 3, 10, 25 and 50 K (Fig. 4, bottom panel). The isotherm at 50 K shows a linear field dependency in line with paramagnetic behaviour. The isotherms at 10 and 25 K show a curved trace without any tendency for saturation. The 3 K isotherm finally exhibits an “s-shaped” character caused by a spin-reorientation also called metamagnetic step, underlining the antiferromagnetic ground state. The critical field was determined to be Hcrit = 20.2(1) kOe from the derivative of the 3 K isotherm. The saturation magnetization is μsat = 5.4(1) μB per Eu atom at 3 K and 80 kOe. This is lower than the expected saturation magnetization of 7 μB according to gJ × J.
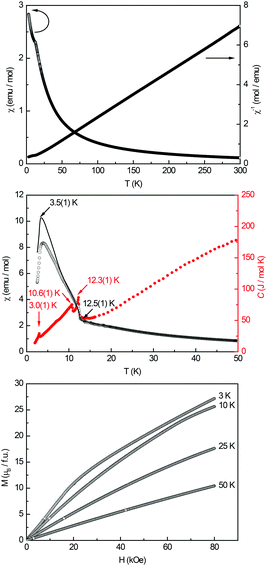 |
| | Fig. 4 Magnetic properties of Eu5Al2Sb6: (top) temperature dependence of the magnetic susceptibility (χ and χ−1 data) measured at 10 kOe; (middle) magnetic susceptibility in zero-field-cooled/field-cooled (ZFC/FC) mode, measured at 100 Oe in the low-temperature range, and in red the heat capacity measurements in zero-field between 2–50 K are shown; (bottom) magnetization isotherms recorded at 3, 10, 25 and 50 K. | |
Sr5Al2Sb6 in contrast exhibits diamagnetic behaviour (Fig. S2, ESI†), as expected for a semiconducting material with only small amounts of charge carriers available up to room temperature. The susceptibility at 300 K is χ = −480(5) × 10−6 emu mol−1, which is in good agreement with the sum of the diamagnetic increments of χ(Sr5Al2Sb6) = −599 × 10−6 emu mol−1 using the tabulated values for the respective ions (χ(Sr2+) = −19 × 10−6 emu mol−1; χ(Al3+) = −2 × 10−6 emu mol−1).47 The values for Sb3− and Sb24− are not available and have been estimated with respect to the neighbouring anions Te2− and I− (χ(Sb3−) = −90 × 10−6 emu mol−1; χ(Sb24−) = −140 × 10−6 emu mol−1).
Electrical resistivity
The temperature dependence of the electrical resistivity of Eu5Al2Sb6 is depicted in Fig. 5. The measured data exhibits the behaviour of the semiconductor. In region (I), at low temperatures, only a limited amount of charge carriers can overcome the band gap. With increasing temperature (II), ‘saturation’ or ‘exhaustion’ occurs, since the doped (extrinsic) charge carriers get promoted, causing a temperature independent plateau. Finally, the intrinsic charge carriers start to take over (III), however, the distinct behaviour of the materials itself is seen only at even higher temperatures (vide infra).48,49 Analysis of the data between 150–300 K using the relation ρ ∝ eEg/2kT for intrinsic semiconductors yielded a band gap of 0.10(2) eV. From the electronic structure, a band gap of 0.52 eV was determined. The band gaps of Eu5Al2Sb6 are smaller compared to Sr5Al2Sb6 (experimental: 0.5 eV; calculated 0.8 eV),12 which were determined at temperatures >500 K. These differences between isotypic compounds are not unusual as seen from the M2CdAs2 (M = Ca, Eu) systems, where the Ca-compound exhibits a band gap of ∼1 eV, while Eu2CdAs2 has a band gap of ∼0.3 eV.50 This feature can be attributed to the mixing of Eu 4f–5d states, as seen in many optical materials.51–53 A closer look at the resistivity data reveals that the region between 150–300 K, used to determine the band gap, might not be fully ‘intrinsic’. Assuming a linear trend, extrapolation of the data to high temperatures would cause the resistivity to cut the temperature axis at ∼450 K. Therefore a kink of the resistivity at higher temperatures is very likely, similar to what has been observed for Sr5Al2Sb6.12 When the resistivity of Eu5Al2Sb6 is compared to isotypic Sr5Al2Sb6, it is evident that both compounds exhibit similar resistivities at 300 K (Sr5Al2Sb6: ρ(300 K) ∼ 1.8 Ω cm; Eu5Al2Sb6: ρ(300 K) = 1.4(1) Ω cm).12 The observed resistivity is also in line with other non-isotypic Zintl compounds, e.g. Ca5Ga2Sb6 (ρ(300 K) ∼ 0.1 Ω cm),23 Sr5In2Sb6 (ρ(300 K) = 10 Ω cm)25 or Eu5In2Sb6 (ρ(300 K) ∼ 0.085 Ω cm).27
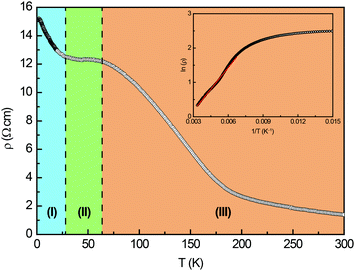 |
| | Fig. 5 Temperature dependence of the electrical resistivity of Eu5Al2Sb6. The different regions are highlighted by colours; for explanation see text. The inset depicts the determination of the band gap. | |
121Sb and 151Eu Mössbauer spectroscopy
To further prove the presence of Eu2+ in Eu5Al2Sb6, 151Eu Mössbauer spectra were recorded at 78 K and 5 K (Fig. 6, top and bottom). The obtained fitting parameters are listed in Table 4. The 78 K spectrum could be fitted with two signals, and the 5 K spectrum with four signals. The two signals in the 78 K spectrum could be attributed to Eu2+ and Eu3+; the latter one contributed ∼1%, hence it can be considered to be an Eu3+ impurity (most likely surface oxidation). The main signal shows an isomer shift of δ = −11.35(1) mm s−1, and the impurity signal is found at δ = +1.2(1) mm s−1. The four signals at 5 K can be attributed to the three crystallographic Eu positions in Eu5Al2Sb6 and again to the small Eu3+ impurity. The main signals show isomer shifts of δ = −11.23(6), −11.41(4) and −11.47(4) mm s−1, being in line with Eu2+ and the magnetic data discussed above, and the impurity signal is found at δ = −0.2(2) mm s−1. As a consequence of the non-cubic site symmetry of the europium atom, the signals of the 5 K spectra show quadrupole splittings of ΔEQ = −1.0(2) mm s−1, −4.4(7) mm s−1 and −1.9(3) mm s−1, respectively. The experimental line widths of Γ = 3.2(1), 3.1(1) and 2.8(1) mm s−1 are within the usual range.54–58 Of the three signals at 5 K, two signals exhibit significant magnetic hyperfine field splitting (Bhf = 23.1(1) and 20.0(1) T) along with the third signal showing only a small hyperfine field splitting of Bhf = 3.2(1) T. The observed hyperfine fields are in line with other intermetallic europium compounds.54–58 The areas of the three signals were fixed with a 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2
2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 ratio, corresponding to the respective site multiplicities of the crystal structure (4c and 2 × 8d). The signal with the small hyperfine field can be therefore attributed to the Eu1 site (4c), which at 5 K is not magnetically ordered (but shows at least some short-range order), whereas the Eu2 and Eu3 sites (both 8d) show a full hyperfine field splitting, in line with their magnetic ordering phenomena at temperatures >5 K. This fits well to the magnetic data, where the antiferromagnetic ordering occurs at TN = 3.5(1) K, below the 5 K used to record the Mössbauer spectra. The small difference between TN and the measurement temperature is causing a minor hyperfine field for the Eu1 site. The 121Sb Mössbauer spectra of Sr5Al2Sb6 measured at 78 K and Eu5Al2Sb6, (78 and 5 K) are depicted in Fig. 7 along with the respective fits. The crystal structure contains three crystallographic sites for the Sb3− anions (2 × 4c and 8d) and two sites forming the Sb42− anion (2 × 4c), therefore the spectrum was fitted using two signals, one for the sum of the Sb3− and one for the sum of the ‘Sb2−’ anions. The area ratio of the two signals was fixed in a 2
2 ratio, corresponding to the respective site multiplicities of the crystal structure (4c and 2 × 8d). The signal with the small hyperfine field can be therefore attributed to the Eu1 site (4c), which at 5 K is not magnetically ordered (but shows at least some short-range order), whereas the Eu2 and Eu3 sites (both 8d) show a full hyperfine field splitting, in line with their magnetic ordering phenomena at temperatures >5 K. This fits well to the magnetic data, where the antiferromagnetic ordering occurs at TN = 3.5(1) K, below the 5 K used to record the Mössbauer spectra. The small difference between TN and the measurement temperature is causing a minor hyperfine field for the Eu1 site. The 121Sb Mössbauer spectra of Sr5Al2Sb6 measured at 78 K and Eu5Al2Sb6, (78 and 5 K) are depicted in Fig. 7 along with the respective fits. The crystal structure contains three crystallographic sites for the Sb3− anions (2 × 4c and 8d) and two sites forming the Sb42− anion (2 × 4c), therefore the spectrum was fitted using two signals, one for the sum of the Sb3− and one for the sum of the ‘Sb2−’ anions. The area ratio of the two signals was fixed in a 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 ratio (Sb3−
1 ratio (Sb3−![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ‘Sb2−’). While for the ‘Sb2−’ signal shifts of δ ∼ −10 mm s−1 were found, the Sb3− signal exhibits shifts of δ ∼ −8 mm s−1 (Table 4). For the latter one, the observed isomer shift is in line with other antimonides containing formally Sb3− anions, e.g. the binary alkali metal antimonides Li3Sb,59 and A3Sb (A = Na, K, Rb),60 the III–V semiconductors AlSb, GaSb and InSb,61 the MT2Sb2 (M = Eu, Yb; T = Mn, Zn) series,62 the antimonide oxides RETSbO (RE = La–Sm, Gd; T = Mn, Zn)63 or the half-Heusler phases YbTSb (T = Ni, Pd, Pt, Cu, Ag, Au)64 and ScTSb (T = Ni, Pd, Pt)65 all with isomer shifts around δ ∼ −8 mm s−1. Antimonide dumbbells in contrast are rather rare with respect to 121Sb Mössbauer spectroscopic investigations. One example that can be found in the literature is α-Zn13Sb10, which can be formulated as (Zn2+)13(Sb3−)6(Sb24−)2. The Mössbauer spectroscopic data was also fitted using two signals in the respective fixed ratio of 3
‘Sb2−’). While for the ‘Sb2−’ signal shifts of δ ∼ −10 mm s−1 were found, the Sb3− signal exhibits shifts of δ ∼ −8 mm s−1 (Table 4). For the latter one, the observed isomer shift is in line with other antimonides containing formally Sb3− anions, e.g. the binary alkali metal antimonides Li3Sb,59 and A3Sb (A = Na, K, Rb),60 the III–V semiconductors AlSb, GaSb and InSb,61 the MT2Sb2 (M = Eu, Yb; T = Mn, Zn) series,62 the antimonide oxides RETSbO (RE = La–Sm, Gd; T = Mn, Zn)63 or the half-Heusler phases YbTSb (T = Ni, Pd, Pt, Cu, Ag, Au)64 and ScTSb (T = Ni, Pd, Pt)65 all with isomer shifts around δ ∼ −8 mm s−1. Antimonide dumbbells in contrast are rather rare with respect to 121Sb Mössbauer spectroscopic investigations. One example that can be found in the literature is α-Zn13Sb10, which can be formulated as (Zn2+)13(Sb3−)6(Sb24−)2. The Mössbauer spectroscopic data was also fitted using two signals in the respective fixed ratio of 3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2. Isomer shifts of −8.17(2) and −9.73(2) mm s−1 were derived,66 which are in line with the data presented herein. In contrast to the zinc phase (ΔEQ = 0 mm s−1), the dumbbells in Sr5Al2Sb6 and Eu5Al2Sb6 exhibit asymmetric coordination environments (Fig. 2, bottom), therefore a rather large quadrupole splitting is observed (ΔEQ = +2.9 and −8.9 as well as +3.6 and −9.9 mm s−1) for the Sb24− signals in both compounds. The low temperature measurements of Eu5Al2Sb6, conducted at 5 K, at first glimpse show an increase of the line width, in line with a transferred hyperfine field from the Eu atoms. The data can be fitted with this increase of the line width or alternatively with fixed isomer shifts and line widths taken from the 78 K measurements and freely refined quadrupole splitting and hyperfine field parameters. The respective parameters are listed in Table 4, the fit depicted in Fig. 7 corresponds to the variant with increased line widths and no refined magnetic hyperfine field.
2. Isomer shifts of −8.17(2) and −9.73(2) mm s−1 were derived,66 which are in line with the data presented herein. In contrast to the zinc phase (ΔEQ = 0 mm s−1), the dumbbells in Sr5Al2Sb6 and Eu5Al2Sb6 exhibit asymmetric coordination environments (Fig. 2, bottom), therefore a rather large quadrupole splitting is observed (ΔEQ = +2.9 and −8.9 as well as +3.6 and −9.9 mm s−1) for the Sb24− signals in both compounds. The low temperature measurements of Eu5Al2Sb6, conducted at 5 K, at first glimpse show an increase of the line width, in line with a transferred hyperfine field from the Eu atoms. The data can be fitted with this increase of the line width or alternatively with fixed isomer shifts and line widths taken from the 78 K measurements and freely refined quadrupole splitting and hyperfine field parameters. The respective parameters are listed in Table 4, the fit depicted in Fig. 7 corresponds to the variant with increased line widths and no refined magnetic hyperfine field.
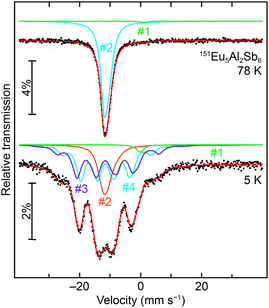 |
| | Fig. 6 Experimental (data points) and simulated (continuous lines) 151Eu Mössbauer spectra of Eu5Al2Sb6 at 78 and 5 K. The red line corresponds to the sum of the different signals used for fitting; they are labeled according to Table 4, and the green signals (#1) are the Eu3+ impurities. | |
Table 4 Fitting parameters for 121Sb and 151Eu Mössbauer spectroscopic measurements of Eu5Al2Sb6. δ: isomer shift; Γ: experimental line width; ΔEQ: quadrupole splitting; Bhf: magnetic hyperfine field; A: signal area ratio
| |
T (K) |
# |
Ion |
δ (mm s−1) |
Γ (mm s−1) |
ΔEQ (mm s−1) |
B
hf (T) |
A (%) |
Value fixed in the last cycle.
Ratio of signals #1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #2 fixed to 2 #2 fixed to 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1.
Ratio of signals #2 1.
Ratio of signals #2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #3 #3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #4 fixed to 1 #4 fixed to 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) : :![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2, ratio of #1 to Σ(#2 + #3 + #4) refined. 2, ratio of #1 to Σ(#2 + #3 + #4) refined.
|
| Eu5Al2121Sb6 |
78 |
1 |
Sb3− |
−8.3(1) |
3.1(2) |
+2.9(1) |
— |
66.67b |
| 2 |
Sb24− |
−9.7(2) |
3.0(2) |
−8.9(1) |
— |
33.33b |
| No Bhf |
5 |
1 |
Sb3− |
−8.3(1) |
3.5(1) |
+4.4(3) |
— |
66.67b |
| 2 |
Sb24− |
−9.8(1) |
3.1(1) |
−9.9(4) |
— |
33.33b |
| With Bhf |
5 |
1 |
Sb3− |
−8.3a |
3.1a |
+2.9(2) |
3.1(1) |
66.67b |
| 2 |
Sb24− |
−9.7a |
3.0a |
−10.7(3) |
1.3(1) |
33.33b |
|
|
| Sr5Al2121Sb6 |
78 |
1 |
Sb3− |
−8.2(1) |
3.8(2) |
+3.6(3) |
— |
66.67b |
| 2 |
Sb24− |
−9.9(2) |
3.6(2) |
−9.9(2) |
— |
33.33b |
|
|
|
151Eu5Al2Sb6 |
78 |
1 |
Eu3+ |
+1.2(1) |
3.0a |
— |
— |
1.1 |
| 2 |
Eu2+ |
−11.35(1) |
2.7(1) |
−4.4(1) |
— |
98.9 |
| 5 |
1 |
Eu3+ |
−0.2(2) |
3.0a |
— |
— |
1.9c |
| 2 |
Eu2+ |
−11.23(6) |
3.2(1) |
−4.4(7) |
3.6(3) |
19.6c |
| 3 |
Eu2+ |
−11.41(4) |
3.1(1) |
−1.9(3) |
23.1(1) |
39.25c |
| 4 |
Eu2+ |
−11.47(4) |
2.8(1) |
−1.0(2) |
20.0(1) |
39.25c |
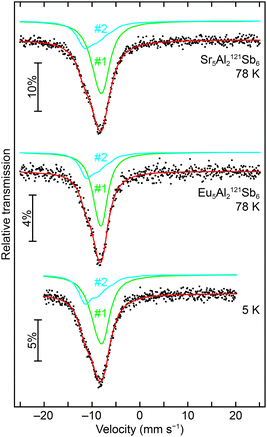 |
| | Fig. 7 Experimental (data points) and simulated (continuous lines) 121Sb Mössbauer spectra of Sr5Al2Sb6 at 78 K and Eu5Al2Sb6 at 78 and 5 K. The red line corresponds to the sum of the different signals used for fitting; they are labeled according to Table 4. | |
Theoretical calculations
To gain more insights into the possible magnetic exchange interactions and magnetic orderings in Eu5Al2Sb6, the electronic structure and total energies of eleven magnetically ordered models (see Fig. 8) were examined: they included one ferromagnetic (FM) model, two antiferromagnetic (AFM1, AFM2) models and eight ferrimagnetic (Fi1–Fi8) models. Due to the overall structural complexity, the repeating unit of each model was limited to one crystallographic unit cell, that is, a total of 4 × Eu1, 8 × Eu2 and 8 × Eu3 atoms. The results of this analysis are summarized in Fig. 8 and Table 5. The spin-polarized density of states (DOS) curve for the FM state is plotted in Fig. 9. There is a small band gap (ca. 0.52 eV from the most stable AFM1 model) at the Fermi level, consistent with the semiconducting behaviour found experimentally. The Eu majority spin states are all occupied while its minority spin states are all unoccupied, indicating a high spin f7 (Eu2+) configuration. Ten spin exchange parameters within Eu5Al2Sb6 were evaluated by performing a mapping analysis67–69 based on the eleven magnetic models (FM, Fi1–Fi8, AFM1 and AFM2) shown in Fig. 8. The ten spin exchange paths include the Eu1–Eu2 interactions (J1), the Eu1–Eu3 interactions (J2), two types of Eu2–Eu3 interactions (J3 and J3′), Eu2–Eu2 interactions (J4), three types of Eu3–Eu3 interactions (J5, J6, J6′) and two types of Eu1–Eu1 interactions (J7 and J8). The relative energies, per unit cell (u.c.), of the eleven magnetic models determined from our VASP calculations are summarized in Fig. 8. The energies of these models can also be approximated in terms of the spin Hamiltonian,
in which, Jij is the spin exchange parameter for the interaction between the spin sites i and j. By applying this energy expression for spin dimers with N unpaired spins per spin site, the total spin exchange energy expression for each of the eleven models are summarized in Table 5. By mapping the relative energies of the eleven models determined by VASP calculations onto the corresponding relative energies determined from the above exchange energies, we obtained the values of Jij listed in Table 5. These parameters are really small compared with other rare-earth compounds with high ordering temperatures,70,71 but they are consistent with the experimentally found small Weiss constant (weak interactions) and low ordering temperatures. Most of the interactions (seven) are antiferromagnetic except for J1, J3 and J6. Based on the calculated spin exchange parameters, one antiferromagnetic state (AFM1) has the lowest energy, but this energy is only slightly lower than those of AFM2 and Fi1 (see Fig. 8), whereas all other ferrimagnetic models are at least 36 meV u.c.−1 higher in energy. The only difference between AFM1, AFM2 and Fi1 is the spin arrangement on the four Eu1 atoms in the unit cell (see Fig. 8, top three models), as all other atoms have the same spin arrangements, that is intra-chain antiferromagnetic interactions in Eu2 and Eu3 chains and ferromagnetic Eu2 and Eu3 layers. In addition, the energy differences between these models are very small (less than 1.5 meV u.c.−1). Therefore, the Eu1 spin arrangements within the unit cell may fluctuate between these three cases. Below TN = 3.5(1) K, antiferromagnetic states (AMF1 or AFM2) prevail and the magnetic phase transition related to the ordering of Eu1 atoms is observed. The magnetic ordering at 12.5 K is more complicated but both ferromagnetic and antiferromagnetic interactions exist based on the calculated spin exchange parameters. So, the magnetic ordering at 12.5 K should be either ferrimagnetic or canted antiferromagnetic.
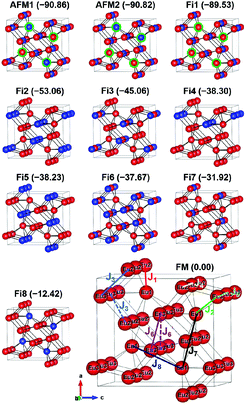 |
| | Fig. 8 Eleven magnetic structure models of Eu5Al2Sb6 constructed using the crystallographic unit cell with their relative energies (in meV u.c.−1) in parentheses. Only Eu atoms are shown in each model. Red and blue colors indicate oppositely oriented magnetic moments of Eu. The ten spin exchange paths in Eu5Al2Sb6 (J1–J8, J3′ and J6′) are shown in the FM model. The green circles highlight the Eu1 atoms. | |
Table 5 Total energies and spin exchange energy expressions for the eleven magnetic structure models of Eu5Al2Sb6. NEu = 7
| Models |
Energya (meV u.c.−1) |
Spin exchange energy expression (meV) |
|
Total energy relative to model FM from VASP calculation.
|
| AFM1 |
−90.86 |
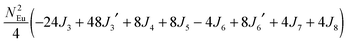
|
| AFM2 |
−90.82 |
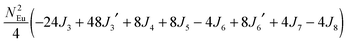
|
| Fi1 |
−89.53 |
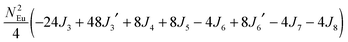
|
| Fi2 |
−53.06 |
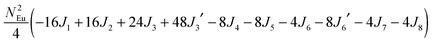
|
| Fi3 |
−45.06 |
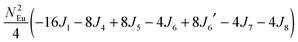
|
| Fi4 |
−38.30 |

|
| Fi5 |
−38.23 |
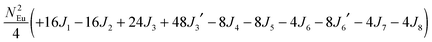
|
| Fi6 |
−37.67 |
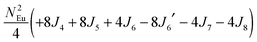
|
| Fi7 |
−31.92 |
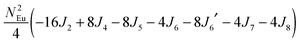
|
| Fi8 |
−12.42 |
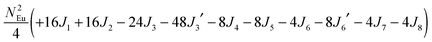
|
|
|
| FM |
0.00 |
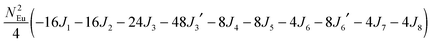
|
|
|
|
J (meV) |
|
J
1 = +0.0031, J2 = −0.035, J3 = +0.021, J3′ = −0.044, J4 = −0.065, J5 = −0.015, J6 = +0.040, J6′ = −0.080, J7 = −0.013, J8 = −0.00041 |
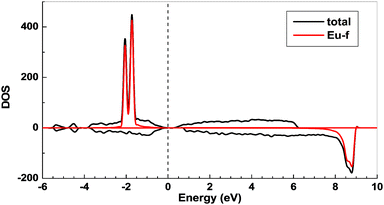 |
| | Fig. 9 Total and partial spin polarized density of states (DOS) of Eu5Al2Sb6 (ferromagnetic model). The Fermi level is denoted by the dashed line. | |
The calculated Bader effective charges for Eu5Al2Sb6 and Sr5Al2Sb6 are listed in Table 6. In both Eu5Al2Sb6 and Sr5Al2Sb6, the Eu, Sr and Al atoms have positive effective charges while Sb atoms have negative effective charges. The effective charges found on the Eu atoms are all between +1 and +2, which indicates that Eu3+ can be ruled out, in accordance with the 151Eu Mössbauer result that found Eu2+. However, the fact that the effective charges on Eu atoms are less than +2 indicates the presence of some partial covalent bonding interactions between Eu and Sb (or Al).
Table 6 Bader charges in Eu5Al2Sb6 and Sr5Al2Sb6
| Eu5Al2Sb6 |
Atom |
Eu1 |
Eu2 |
Eu3 |
Sb1 |
Sb2 |
Sb3 |
Sb4 |
Sb5 |
Al1 |
Al2 |
| Charge |
+1.18 |
+1.18 |
+1.24 |
−1.59 |
−1.68 |
−1.63 |
−1.08 |
−1.30 |
+1.51 |
+1.44 |
|
|
| Sr5Al2Sb6 |
Atom |
Sr1 |
Sr2 |
Sr3 |
Sb1 |
Sb2 |
Sb3 |
Sb4 |
Sb5 |
Al1 |
Al2 |
| Charge |
+1.32 |
+1.31 |
+1.36 |
−1.71 |
−1.78 |
−1.71 |
−1.21 |
−1.39 |
+1.49 |
+1.43 |
Conclusions
The Zintl phase Eu5Al2Sb6, which crystallizes isostructural to Sr5Al2Sb6 (Pnma, no. 62, a = 1027.55(7), b = 1200.28(6), c = 1324.22(7) pm), was synthesized from the elements in niobium ampoules and characterized by powder and single crystal X-ray diffraction. In the orthorhombic crystal structure, the Al and Sb atoms form branched strands, consisting of Al3+, Sb3− and Sb24− dumbbells. The three Eu sites are located in cavities. Three magnetic ordering phenomena at T1 = 12.3(1), T2 = 10.6(1) and T3 = 3.0(1) K were observed in the susceptibility measurements and confirmed by heat capacity measurements. 151Eu Mössbauer spectroscopic studies confirmed the divalent oxidation state of all three Eu atoms. Measurements at 5 K, therefore below T1 and T2, showed a hyperfine field splitting for two of the three Eu sites due to magnetic ordering. The calculated densities of states using an antiferromagnetic model indicate the presence of a band gap in accordance with the semiconducting behavior found by electrical conductivity. Based on the spin exchange parameters calculated using mapping analysis, the spin exchange interactions in Eu5Al2Sb6 are really small compared with other magnetically ordered rare-earth compounds. In addition, the magnetic phase transition at TN = 3.5(1) is related to the ordering of the Eu1 atoms. Bader charge analysis confirms the divalent oxidation state of Eu, but also indicates significant covalent interactions involving the Eu atoms.
Acknowledgements
We thank Dipl.-Ing. U. Ch. Rodewald for the single crystal intensity data collection. Boniface P. T. Fokwa and Yuemei Zhang thank San Diego Supercomputer Center for providing computing resources.
References
-
S. M. Kauzlarich, Chemistry, Structure, and Bonding of Zintl Phases and Ions, VCH Publishers, 1996 Search PubMed.
- K. A. Bol'shakov, N. A. Bul'onkov, L. N. Rastorguev and M. S. Tsirlin, Russ. J. Inorg. Chem., 1963, 8, 1418–1421 Search PubMed.
- J. Böhm and O. Hassel, Z. Anorg. Allg. Chem., 1927, 160, 152–164 CrossRef.
-
O. Janka and S. M. Kauzlarich, in Encyclopedia of Inorganic and Bioinorganic Chemistry, John Wiley & Sons, Ltd, 2013 Search PubMed.
- G. J. Snyder and E. S. Toberer, Nat. Mater., 2008, 7, 105–114 CrossRef CAS PubMed.
- S. K. Bux, M. T. Yeung, E. S. Toberer, G. J. Snyder, R. B. Kaner and J.-P. Fleurial, J. Mater. Chem., 2011, 21, 12259–12266 RSC.
- O. Janka, J. V. Zaikina, S. K. Bux, H. Tabatabaifar, H. Yang, N. D. Browning and S. M. Kauzlarich, J. Solid State Chem., 2017, 245, 152–159 CrossRef CAS.
- S. R. Brown, S. M. Kauzlarich, F. Gascoin and G. J. Snyder, Chem. Mater., 2006, 18, 1873–1877 CrossRef CAS.
- J.-A. Paik, B. Brandon, T. Caillat, R. Ewell and J. P. Fleurial, Proc. Nucl. Emerging Technol. Space, 2011, 2011(paper 3695), 1–10 Search PubMed.
- S. K. Bux, A. Zevalkink, O. Janka, D. Uhl, S. M. Kauzarich, J. G. Snyder and J.-P. Fleurial, J. Mater. Chem. A, 2014, 2, 215–220 CAS.
- G. Cordier and M. Stelter, Z. Naturforsch., 1988, 43b, 463–466 Search PubMed.
- A. Zevalkink, Y. Takagiwa, K. Kitahara, K. Kimura and G. J. Snyder, Dalton Trans., 2014, 43, 4720–4725 RSC.
- S. L. Brock, L. J. Weston, M. M. Olmstead and S. M. Kauzlarich, J. Solid State Chem., 1993, 107, 513–523 CrossRef CAS.
- G. Cordier, H. Schäfer and M. Stelter, Z. Naturforsch., 1987, 42b, 1268–1272 Search PubMed.
- R. D. Shannon, Acta Crystallogr., 1976, A32, 751–767 CrossRef CAS.
- S.-J. Kim, J. R. Ireland, C. R. Kannewurf and M. G. Kanatzidis, J. Solid State Chem., 2000, 155, 55–61 CrossRef CAS.
- M. L. Fornasini and P. Manfrinetti, Z. Kristallogr. - New Cryst. Struct., 2009, 224, 345–346 CAS.
- I. Todorov, D. Y. Chung, L. Ye, A. J. Freeman and M. G. Kanatzidis, Inorg. Chem., 2009, 48, 4768–4776 CrossRef CAS PubMed.
- U. Subbarao, S. Sarkar, V. K. Gudelli, V. Kanchana, G. Vaitheeswaran and S. C. Peter, Inorg. Chem., 2013, 52, 13631–13638 CrossRef CAS PubMed.
- U. Aydemir, A. Zevalkink, A. Ormeci, H. Wang, S. Ohno, S. Bux and G. J. Snyder, Dalton Trans., 2015, 44, 6767–6774 RSC.
- D. Cordier, E. Czech, M. Jakowski and H. Schäfer, Rev. Chim. Miner., 1981, 18, 9–18 Search PubMed.
- D. Cordier, H. Schäfer and M. Stelter, Z. Naturforsch., 1985, 40b, 5–8 Search PubMed.
- S. I. Johnson, A. Zevalkink and G. J. Snyder, J. Mater. Chem. A, 2013, 1, 4244–4249 CAS.
- A. Zevalkink, J. Swallow, S. Ohno, U. Aydemir, S. Bux and G. J. Snyder, Dalton Trans., 2014, 43, 15872–15878 RSC.
- S. Chanakian, A. Zevalkink, U. Aydemir, Z. M. Gibbs, G. Pomrehn, J.-P. Fleurial, S. Bux and G. J. Snyder, J. Mater. Chem. A, 2015, 3, 10289–10295 CAS.
- S.-M. Park, E. S. Choi, W. Kang and S.-J. Kim, J. Mater. Chem., 2002, 12, 1839–1843 RSC.
- S. Chanakian, U. Aydemir, A. Zevalkink, Z. M. Gibbs, J.-P. Fleurial, S. Bux and G. Jeffrey Snyder, J. Mater. Chem. C, 2015, 3, 10518–10524 RSC.
- R. Pöttgen, T. Gulden and A. Simon, GIT Labor-Fachz., 1999, 43, 133–136 Search PubMed.
- D. Kußmann, R.-D. Hoffmann and R. Pöttgen, Z. Anorg. Allg. Chem., 1998, 624, 1727–1735 CrossRef.
- K. Yvon, W. Jeitschko and E. Parthé, J. Appl. Crystallogr., 1977, 10, 73–74 CrossRef.
- L. J. van der Pauw, Philips Res. Rep., 1958, 13, 1–9 Search PubMed.
- G. J. Long, T. E. Cranshaw and G. Longworth, Moessbauer Eff. Ref. Data J., 1983, 6, 42–49 Search PubMed.
-
R. A. Brand, NORMOS Mössbauer Fitting Program, Universität Duisburg, Duisburg, Germany, 2007 Search PubMed.
- P. E. Blöchl, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953–17979 CrossRef.
- G. Kresse and D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758–1775 CrossRef CAS.
- G. Kresse and J. Furthmüller, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169–11186 CrossRef CAS.
- G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15–50 CrossRef CAS.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868 CrossRef CAS PubMed.
- S. L. Dudarev, G. A. Botton, S. Y. Savrasov, C. J. Humphreys and A. P. Sutton, Phys. Rev. B: Condens. Matter Mater. Phys., 1998, 57, 1505–1509 CrossRef CAS.
- E. Sanville, S. D. Kenny, R. Smith and G. Henkelman, J. Comput. Chem., 2007, 28, 899–908 CrossRef CAS PubMed.
- G. Henkelman, A. Arnaldsson and H. Jónsson, Comput. Mater. Sci., 2006, 36, 354–360 CrossRef.
- W. Tang, E. Sanville and G. Henkelman, J. Phys.: Condens. Matter, 2009, 21, 084204 CrossRef CAS PubMed.
-
J. Emsley, The Elements, Clarendon Press, Oxford University Press, Oxford, New York, 1998 Search PubMed.
- B. Eisenmann, C. Gieck and U. Rößler, Z. Kristallogr. - New Cryst. Struct., 2001, 216, 36 CAS.
- F. Emmerling, N. Längin, F. Pickhard, M. Wendorff and C. Röhr, Z. Naturforsch., 2004, 59b, 7–16 Search PubMed.
- F. Hulliger and R. Schmelczer, J. Solid State Chem., 1978, 26, 389–396 CrossRef CAS.
- G. A. Bain and J. F. Berry, J. Chem. Educ., 2008, 85, 532 CrossRef CAS.
-
A. R. West, Solid State Chemistry and its Applications, John Wiley & Sons Ltd, New York, Toronto, Singapore, 1st edn, 1990 Search PubMed.
-
R. Gross and A. Marx, Festkörperphysik, Oldenbourg Verlag, München, 2012 Search PubMed.
- J. Wang, M. Yang, M.-Y. Pan, S.-Q. Xia, X.-T. Tao, H. He, G. Darone and S. Bobev, Inorg. Chem., 2011, 50, 8020–8027 CrossRef CAS PubMed.
- N. Yamashita, J. Electrochem. Soc., 1993, 140, 840 CrossRef CAS.
- Y. Nakao, J. Phys. Soc. Jpn., 1980, 48, 534–541 CrossRef CAS.
- S. Seidel, T. Dierkes, T. Justel, C. Benndorf, H. Eckert and R. Pottgen, Dalton Trans., 2016, 45, 12078–12086 RSC.
- R. Müllmann, B. D. Mosel, H. Eckert, G. Kotzyba and R. Pöttgen, J. Solid State Chem., 1998, 137, 174–180 CrossRef.
- D. Kußmann, R. Pöttgen, U. C. Rodewald, C. Rosenhahn, B. D. Mosel, G. Kotzyba and B. Künnen, Z. Naturforsch., 1999, 54b, 1155–1164 Search PubMed.
- S. Seidel, O. Niehaus, S. F. Matar, O. Janka, B. Gerke, U. C. Rodewald and R. Pöttgen, Z. Naturforsch., 2014, 69b, 1105–1118 Search PubMed.
- J.-P. Schmiegel, T. Block, B. Gerke, T. Fickenscher, R. S. Touzani, B. P. T. Fokwa and O. Janka, Inorg. Chem., 2016, 55, 9057–9064 CrossRef CAS PubMed.
- I. Schellenberg, M. Eul and R. Pöttgen, Monatsh. Chem., 2011, 142, 875–880 CrossRef CAS.
- P. E. Lippens, J.-C. Jumas and J. Olivier-Fourcade, Hyperfine Interact., 2004, 156, 327–333 CrossRef.
- K. Kitadai, M. Takahashi and M. Takeda, J. Radioanal. Nucl. Chem., 2003, 255, 311–314 CrossRef CAS.
- P. E. Lippens, Solid State Commun., 2000, 113, 399–403 CrossRef CAS.
- I. Schellenberg, M. Eul, W. Hermes and R. Pöttgen, Z. Anorg. Allg. Chem., 2010, 636, 85–93 CrossRef CAS.
- I. Schellenberg, T. Nilges and R. Pöttgen, Z. Naturforsch., 2008, 63b, 834–840 Search PubMed.
- M. Ratikanta, R. Pöttgen, R.-D. Hoffmann, T. Fickenscher, M. Eschen, H. Trill and B. D. Mosel, Z. Naturforsch., 2002, 57b, 1215–1223 Search PubMed.
- T. Harmening, H. Eckert and R. Pöttgen, Solid State Sci., 2009, 11, 900–906 CrossRef CAS.
- R. P. Hermann, F. Grandjean, T.-C. Chen, D. E. Brown, C. E. Johnson, G. J. Snyder and G. J. Long, Inorg. Chem., 2007, 46, 767–770 CrossRef CAS PubMed.
- D. Dai and M.-H. Whangbo, J. Chem. Phys., 2001, 114, 2887–2893 CrossRef CAS.
- D. Dai and M.-H. Whangbo, J. Chem. Phys., 2003, 118, 29–39 CrossRef CAS.
- M.-H. Whangbo, H.-J. Koo and D. Dai, J. Solid State Chem., 2003, 176, 417–481 CrossRef CAS.
- C. Lee, E. Kan, H. Xiang, R. K. Kremer, S.-H. Lee, Z. Hiroi and M.-H. Whangbo, Inorg. Chem., 2012, 51, 6890–6897 CrossRef CAS PubMed.
- Q. Lin, V. Taufour, Y. Zhang, M. Wood, T. Drtina, S. L. Bud'ko, P. C. Canfield and G. J. Miller, J. Solid State Chem., 2015, 229, 41–48 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available: Powder X-ray diffraction data of Eu5Al2Sb6 and susceptibility data of Sr5Al2Sb6. See DOI: 10.1039/c7qm00057j |
|
| This journal is © the Partner Organisations 2017 |
Click here to see how this site uses Cookies. View our privacy policy here.  *ac
*ac
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 749
749![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2
2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 ratio, corresponding to the respective site multiplicities of the crystal structure (4c and 2 × 8d). The signal with the small hyperfine field can be therefore attributed to the Eu1 site (4c), which at 5 K is not magnetically ordered (but shows at least some short-range order), whereas the Eu2 and Eu3 sites (both 8d) show a full hyperfine field splitting, in line with their magnetic ordering phenomena at temperatures >5 K. This fits well to the magnetic data, where the antiferromagnetic ordering occurs at TN = 3.5(1) K, below the 5 K used to record the Mössbauer spectra. The small difference between TN and the measurement temperature is causing a minor hyperfine field for the Eu1 site. The 121Sb Mössbauer spectra of Sr5Al2Sb6 measured at 78 K and Eu5Al2Sb6, (78 and 5 K) are depicted in Fig. 7 along with the respective fits. The crystal structure contains three crystallographic sites for the Sb3− anions (2 × 4c and 8d) and two sites forming the Sb42− anion (2 × 4c), therefore the spectrum was fitted using two signals, one for the sum of the Sb3− and one for the sum of the ‘Sb2−’ anions. The area ratio of the two signals was fixed in a 2
2 ratio, corresponding to the respective site multiplicities of the crystal structure (4c and 2 × 8d). The signal with the small hyperfine field can be therefore attributed to the Eu1 site (4c), which at 5 K is not magnetically ordered (but shows at least some short-range order), whereas the Eu2 and Eu3 sites (both 8d) show a full hyperfine field splitting, in line with their magnetic ordering phenomena at temperatures >5 K. This fits well to the magnetic data, where the antiferromagnetic ordering occurs at TN = 3.5(1) K, below the 5 K used to record the Mössbauer spectra. The small difference between TN and the measurement temperature is causing a minor hyperfine field for the Eu1 site. The 121Sb Mössbauer spectra of Sr5Al2Sb6 measured at 78 K and Eu5Al2Sb6, (78 and 5 K) are depicted in Fig. 7 along with the respective fits. The crystal structure contains three crystallographic sites for the Sb3− anions (2 × 4c and 8d) and two sites forming the Sb42− anion (2 × 4c), therefore the spectrum was fitted using two signals, one for the sum of the Sb3− and one for the sum of the ‘Sb2−’ anions. The area ratio of the two signals was fixed in a 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 ratio (Sb3−
1 ratio (Sb3−![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ‘Sb2−’). While for the ‘Sb2−’ signal shifts of δ ∼ −10 mm s−1 were found, the Sb3− signal exhibits shifts of δ ∼ −8 mm s−1 (Table 4). For the latter one, the observed isomer shift is in line with other antimonides containing formally Sb3− anions, e.g. the binary alkali metal antimonides Li3Sb,59 and A3Sb (A = Na, K, Rb),60 the III–V semiconductors AlSb, GaSb and InSb,61 the MT2Sb2 (M = Eu, Yb; T = Mn, Zn) series,62 the antimonide oxides RETSbO (RE = La–Sm, Gd; T = Mn, Zn)63 or the half-Heusler phases YbTSb (T = Ni, Pd, Pt, Cu, Ag, Au)64 and ScTSb (T = Ni, Pd, Pt)65 all with isomer shifts around δ ∼ −8 mm s−1. Antimonide dumbbells in contrast are rather rare with respect to 121Sb Mössbauer spectroscopic investigations. One example that can be found in the literature is α-Zn13Sb10, which can be formulated as (Zn2+)13(Sb3−)6(Sb24−)2. The Mössbauer spectroscopic data was also fitted using two signals in the respective fixed ratio of 3
‘Sb2−’). While for the ‘Sb2−’ signal shifts of δ ∼ −10 mm s−1 were found, the Sb3− signal exhibits shifts of δ ∼ −8 mm s−1 (Table 4). For the latter one, the observed isomer shift is in line with other antimonides containing formally Sb3− anions, e.g. the binary alkali metal antimonides Li3Sb,59 and A3Sb (A = Na, K, Rb),60 the III–V semiconductors AlSb, GaSb and InSb,61 the MT2Sb2 (M = Eu, Yb; T = Mn, Zn) series,62 the antimonide oxides RETSbO (RE = La–Sm, Gd; T = Mn, Zn)63 or the half-Heusler phases YbTSb (T = Ni, Pd, Pt, Cu, Ag, Au)64 and ScTSb (T = Ni, Pd, Pt)65 all with isomer shifts around δ ∼ −8 mm s−1. Antimonide dumbbells in contrast are rather rare with respect to 121Sb Mössbauer spectroscopic investigations. One example that can be found in the literature is α-Zn13Sb10, which can be formulated as (Zn2+)13(Sb3−)6(Sb24−)2. The Mössbauer spectroscopic data was also fitted using two signals in the respective fixed ratio of 3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2. Isomer shifts of −8.17(2) and −9.73(2) mm s−1 were derived,66 which are in line with the data presented herein. In contrast to the zinc phase (ΔEQ = 0 mm s−1), the dumbbells in Sr5Al2Sb6 and Eu5Al2Sb6 exhibit asymmetric coordination environments (Fig. 2, bottom), therefore a rather large quadrupole splitting is observed (ΔEQ = +2.9 and −8.9 as well as +3.6 and −9.9 mm s−1) for the Sb24− signals in both compounds. The low temperature measurements of Eu5Al2Sb6, conducted at 5 K, at first glimpse show an increase of the line width, in line with a transferred hyperfine field from the Eu atoms. The data can be fitted with this increase of the line width or alternatively with fixed isomer shifts and line widths taken from the 78 K measurements and freely refined quadrupole splitting and hyperfine field parameters. The respective parameters are listed in Table 4, the fit depicted in Fig. 7 corresponds to the variant with increased line widths and no refined magnetic hyperfine field.
2. Isomer shifts of −8.17(2) and −9.73(2) mm s−1 were derived,66 which are in line with the data presented herein. In contrast to the zinc phase (ΔEQ = 0 mm s−1), the dumbbells in Sr5Al2Sb6 and Eu5Al2Sb6 exhibit asymmetric coordination environments (Fig. 2, bottom), therefore a rather large quadrupole splitting is observed (ΔEQ = +2.9 and −8.9 as well as +3.6 and −9.9 mm s−1) for the Sb24− signals in both compounds. The low temperature measurements of Eu5Al2Sb6, conducted at 5 K, at first glimpse show an increase of the line width, in line with a transferred hyperfine field from the Eu atoms. The data can be fitted with this increase of the line width or alternatively with fixed isomer shifts and line widths taken from the 78 K measurements and freely refined quadrupole splitting and hyperfine field parameters. The respective parameters are listed in Table 4, the fit depicted in Fig. 7 corresponds to the variant with increased line widths and no refined magnetic hyperfine field.

![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #2 fixed to 2
#2 fixed to 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1.
c Ratio of signals #2
1.
c Ratio of signals #2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #3
#3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) #4 fixed to 1
#4 fixed to 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2
2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2, ratio of #1 to Σ(#2 + #3 + #4) refined.
2, ratio of #1 to Σ(#2 + #3 + #4) refined.