Temperature dependence of poly(lactic acid) mechanical properties

Abstract

The mechanical properties of polymers are not only determined by their structures, but also related to the temperature field in which they are located. The yield behaviors, Young's modulus and structures of injection-molded poly(lactic acid) (PLA) samples after annealing at different temperatures were measured during stretching within 30–150 °C. The in situ photographs were recorded with a camera to observe deformation behavior during stretching. The less ordered α′ form crystal of PLA can be formed in the un-annealed PLA samples and those that were annealed at 70 °C with low crystallinity. The crystallinity increases with increasing annealing temperature and α form crystal is formed when the annealing temperature is higher than 100 °C. The stretched samples with low crystallinity show the first yield at draw temperatures below the glass transition temperature (T_g) and the second yield above T_g. For the samples annealed between 80 and 120 °C, a peculiar double yield appears when stretched within 50–60 °C and only the first or the second yield can be found at the lower and higher draw temperatures. The yield strain and yield stress together with Young's modulus were obtained and discussed in terms of the effects of the draw temperature and crystalline structure of PLA samples.

---

1. Introduction

Unlike the crystallization of small molecule materials, polymers crystallize partially, which therefore presents the co-existence of crystalline and amorphous phases with an alternate arrangement of crystalline lamellae and amorphous layers.¹ The mechanical properties of crystalline polymers are determined by the crystalline phase, including the crystallinity, crystal form, and crystalline morphology.^2–4 As an important mechanical property of polymer products, the Young's modulus, E, of crystalline polymers is mainly based on their structures. Generally, the E of crystalline polymers increases with crystallinity, irrespective of the annealing process.³ For crystalline PLA, not only the crystallinity but also the crystal form composition has an effect on the E. A higher E can be observed for a PLA sample containing α form, which is due to the more ordered and more densely packed crystal structure of α form than α′ form.² By modeling crystalline polymers as a two-phase composite, the modulus of crystalline polymers is not only dependent on the volumetric fraction but also the geometry properties of crystalline phase.⁵ Furthermore, the dynamic tensile modulus of oriented crystalline polymers cannot be determined solely by the crystalline phase but by the mechanical coupling of the crystalline and the amorphous regions.⁶

On the other hand, a peculiar double yield behavior occurring in one deformation process can be observed for polyethylene and ethylene copolymers under favorable deformation conditions.^7–12 Brooks et al.⁷ investigated the double yield point by the tensile and compressive experiments of polyethylene. The results showed that the first yield point marks the onset of temporary plastic deformation, which is either partially or totally recoverable when removing the external stress, while the second yield point marks the onset of permanent plastic deformation and is associated with sharp necking, therefore being totally irrecoverable. Seguela et al.⁹ reported the same phenomenon of double yield in the nominal stress–strain curves of polyethylene and ethylene copolymers under tensile deformation. The double yield points was suggested to be a general feature of all the studied materials, regardless of crystallinity, and was discussed in terms of two thermally activated rate processes of plastic deformation under various draw temperatures and strain rates. Xu et al.¹¹ revealed that the composition distribution has a great influence on the double yield behavior of ethylene copolymers. The more homogenous the composition distribution of the copolymer, the more marked the double yield phenomenon. The deformation mechanisms responsible for double yield points of polyethylene and ethylene copolymers was investigated based on the variation of crystalline structure. Takayanagi et al.¹³ suspected that the two processes are related to the paracrystalline structure of crystallites. Brooks et al.⁸ revealed that the first yield point marks the onset of the recoverable reorientation process of the lamellae within spherulites, leading to the lamellae oriented predominantly at approximately 45° to the draw direction with little or no destruction of lamellae themselves, while the second yield point is associated with the destruction of the lamellae lying at 45° to the draw direction by c shear. Young et al.¹⁴ and Schrauwen et al.¹² assigned the first and second yield process to the fine slip and coarse slip of crystalline lamellae, respectively. On the basis of mosaic block structure of crystalline lamellae, Gaucher-Miri et al.¹⁰ revealed that the double yield processes can be ascribed to two theoretical models. These are the homogeneous crystal slip and heterogeneous crystal slip, i.e., the uniform shear of crystal blocks and crystal blocks sliding through the defective block boundaries, which is supported by small-angle and wide-angle X-ray diffractions and infrared measurements. Based on the two models, the first and the second yield stress can be calculated.^10,12 The calculated values of both yield stress fairly agree with the measured values and were all proportional to the lamellar thickness. On the other hand, both the first and the second yield stress decrease with increasing draw temperature and the first one is much more temperature-dependent than the second one.¹⁰

The different crystallization temperatures can impart to PLA samples the distinct crystal form composition, i.e., only α′ form of PLA can be formed at crystallization temperatures (T_c) below 100 °C, α′ and α forms coexist in the range of 100 °C < T_c < 120 °C, and only α form appears at T_cs ≥ 120 °C,^15–17 which consequently affects the properties of PLA samples. Cocca et al.² revealed that not only the crystallinity but also the content ratio between α′ and α form in PLA film can affect its mechanical properties and permeability to water vapor. The higher the content of α form in PLA film, the higher Young's modulus and the lower elongation at break and permeability to water vapor, due to the tighter molecular packing and the more ordered chain conformation of α form with compared to α′ form. Furthermore, the phase transition of α′ to α form with increasing crystallization temperature up to 120 °C can lead to the anomalous variations of the lamellar thickness with crystallization temperature, i.e., it has a minimum at 120 °C and increases for both lower and higher crystallization temperatures.^18,19 The relatively larger thickness of PLA lamellae obtained below 120 °C can be attributed to the disordering of the crystal lattice of the α′ form. With the same degree of crystallinity, the PLA samples with the thinner lamellae can obtain the larger elongation and more oriented crystals but with the less perfection, while more lamellar blocks are preserved in the samples with the thicker lamellae, hindering the further deformation and then resulting in the lower elongation at break.⁴

To the best of our knowledge, there are few reports published for investigating the yield behavior of crystalline PLA under stretching. In present work, the yield behaviors of the annealed PLA samples were investigated over a wide stretching temperature range. The peculiar double yield behavior of crystalline PLA was for the first time observed and discussed based on the mosaic block structure model of crystalline lamellae. Furthermore, the variation of Young's modulus of crystalline PLA depending on draw temperatures and annealing-induced crystalline structure was also discussed in terms of the mechanical network containing both the amorphous and crystalline phases.

2. Experimental section

2.1. Material and sample preparation

Poly(lactic acid) (PLA) of the study is the Ingeo 3001D grade material from NatureWorks (USA). The melt flow rate (MFR) is 22 g/10 min at 210 °C under 2.16 kg. The glass transition temperature (T_g) and the melting point (T_m) are in the range of 55–60 °C and 155–170 °C, respectively. The decomposition temperature is 250 °C.

The PLA granules were dried at 50 °C in vacuum oven for 24 hours, and then were used to prepare the dumbbell-shaped sample bars by the Babyplast Injection machine with the mold dimensions of 26.0 mm (gauge length) × 3.0 mm (neck length) × 2.0 mm (neck width) × 0.5 mm (neck thickness). The mold temperature during injection is 25 °C. The temperatures of the feeding section, compression section and nozzle are 175, 215 and 200 °C, respectively. The injection pressure is 103 kPa. The obtained sample bars were annealed at 70, 80, 90, 100, 120, and 150 °C in vacuum oven for 24 hours, the un-annealed PLA and the PLA samples annealed are labeled as UnaPLA, A70PLA, A80PLA, A90PLA, A100PLA, A120PLA, and A150PLA, respectively.

2.2. Wide angle X-ray diffraction measurements (WAXD)

The WAXD measurements of the un-annealed and annealed PLA bars were carried out at room temperature by PANalytical EMPYREAN diffractometer equipped with anode Cu Kα radiation source (λ = 0.154 nm) at 45 kV and 40 mA and the detector of PIXcel^1D (A Medipix2 collaboration). The scanning speed is 2.5° min⁻¹ over the range of 5–30°.

2.3. Differential scanning calorimetry (DSC)

The DSC measurements on un-annealed and annealed PLA samples were performed with DSC (DSC-Q2000, from TA Company). The calibrations of temperature and heat flow were performed with high purity indium according to standard procedures. Samples of about 5 mg were measured under nitrogen flow purge with a heating rate of 10 °C min⁻¹ from 20 °C to 200 °C. The degree of crystallinity (X_c) was calculated by subtracting the enthalpy of cold crystallization (if presented) from the total melting enthalpy and dividing by the enthalpy of fusion of 100% crystalline PLA, which was 93 J g⁻¹.²⁰

2.4. Tensile measurements

The Linkam TST350 tensile hot stage (Linkam Scientific Instruments, Ltd., U.K., with precise controls of temperature, heating or cooling rates and stretching rate), was applied to stretch the un-annealed and annealed PLA bars uniaxially within 30–150 °C, below and above T_g of PLA, at a stretching rate of 4 μm s⁻¹ for obtaining engineering stress–strain curves. The PLA bars were heated to the desired stretching temperatures quickly with the heating rate of 30 °C min⁻¹, followed by temperature equilibration for 1 minute, and then were stretched until breakage. The real time photographs of PLA sample bars during stretching were recorded via high-pixel camera to investigate the deformation behaviors.

3. Results and discussion

3.1. The annealing temperature effects on crystalline structure of PLA samples

The un-annealed and annealed PLA samples were characterized by WAXD and DSC measurements and the obtained results were presented in Fig. 1. As shown in Fig. 1(a), the un-annealed PLA possesses very low crystallinity as evidenced by the quite weak (200/110) reflection and the lack of the other reflections at higher 2θ values. Upon annealing, the intensity of (200/110) reflection increases gradually and the (203) and (210) reflections appear successively with increasing the annealing temperature, indicating the gradual increase of crystallinity and crystalline ordering degree. The DSC heating traces in Fig. 1(b) exhibit the differences in the crystallinity and the crystalline regularity in the un-annealed and annealed PLA samples. The appearance of the broad cold crystallization peak (P_cc) of the UnaPLA and A70PLA samples reveals the low original crystallinity. Moreover, the small exothermic peak (P_exo) prior to melting, related to the transition of α′ to α form,^16,17 can be observed in all of the samples except for A150PLA, which indicates that the α′ form exists in all of the samples apart from A150PLA.

Fig. 1 (a) The WAXD profiles of PLA samples with and without annealing; (b) the DSC heating traces; (c) the magnified view of the (203) reflection of various PLA samples in (a); and (d) the crystallinity, X_c, calculated from DSC measurements and the content ratio of α form to total crystals, R_α, estimated from deconvolution of the (203) reflection of the PLA samples. The label “UnaPLA” refers to the un-annealed PLA sample, and the “AiPLA” refers to the PLA sample annealed at “i” °C for 24 hours. The “P_cc” and the “P_exo” in (c) refer to the cold crystallization peak and the small exothermic peak just prior to the melting peak, respectively.

To view in more detail the variation of crystalline regularity (the fraction of ordered α form) with annealing temperature, the magnified images of the (203) reflection were illustrated in Fig. 1(c). For the UnaPLA and A70PLA samples, the (203) reflection was hardly observed, meaning the disordered crystal structure. With increasing annealing temperature, the (203) reflection appears and the position shifts towards to the higher 2θ values, indicating the closer chain packing and therefore the enhanced crystalline regularity.¹⁶ It is known, only α′ form of PLA can be formed at crystallization temperatures (T_c) below 100 °C, α′ and α forms coexist in the range of 100 °C < T_c < 120 °C, and only α form appears at T_cs ≥ 120 °C.^15–17 It can be found that the content of α′ form decreases and that of α form increases gradually in the annealed PLA samples with increasing annealing temperature, as shown in Fig. 1(c). For PLA samples crystallized between 100 and 120 °C, the (203) reflection can be resolved into two separated components originating from the α′ and α forms.^2,16,17 Therefore, the content ratio of α form to total crystals (R_α) in annealed PLA samples can be calculated based on deconvolution of (203) reflection.² The obtained R_α values are shown in Fig. 1(d) together with the crystallinity (X_c) obtained from the DSC measurements. It pronounces that the X_c and R_α increase substantially in the different ranges of annealing temperature, i.e., the X_c increases mainly at annealing temperatures not above 80 °C while the R_α increases predominantly at temperatures higher than 100 °C.

As mentioned above, the annealing temperatures impart to PLA samples the distinct crystallinity and crystalline regularity. Correspondingly, it can affect the mechanical properties of PLA samples under stretching conditions, which will be in detail discussed.

3.2. Yield behaviors of the PLA samples at different temperatures

The engineering stress–strain curves of the UnaPLA and A70PLA samples stretched within 30–150 °C are shown in Fig. 2. As shown in Fig. 2(a), the UnaPLA samples present two different yields in terms of the yield strain with increasing stretching temperature. Upon stretching at 30 °C, the first yield (Yield-1) can be observed with a high yield stress and a sharp strain softening after yield point. The yield stress and the amplitude of strain softening decrease dramatically with increasing draw temperature. Particularly at 60 °C (just at T_g), the yield stress becomes very low and the strain softening disappears, allowing the strain hardening to occur directly after yield point. When stretching temperature goes above T_g, the first yield becomes hardly to identify at 70 °C (just above T_g) due to the low yield stress, and then the first yield disappears and the second yield (Yield-2) with larger yield strain and again increased yield stress is observed at 80 and 90 °C. The UnaPLA samples exhibit a brittle fracture when being stretched above 90 °C, leading to the disappearance of the yield phenomenon, which could be ascribed to the rapid increase of crystallinity and crystalline regularity during stretching. The variation of yield behavior of the A70PLA samples with stretching temperature is similar to that of the UnaPLA samples due to the comparable crystallinity and the same crystal form composition. The sole difference in yield behavior between both samples is observed at 70 °C, i.e., the UnaPLA shows a weak first yield while the A70PLA exhibits a stronger second yield, which can be attributed to the relatively lower crystallinity of the UnaPLA and the low rate of strain-induced cold crystallization at 70 °C.²¹

Fig. 2 The engineering stress–strain curves of (a) UnaPLA and (b) A70PLA samples stretched at different temperatures with the draw rate of 4 μm s⁻¹. The early yield with smaller yield strain is named as the first yield, Yield-1, and the late one with larger yield strain as the second yield, Yield-2.

To investigate the different yield behaviors in more detail, the photographs of the A70PLA samples before and after the yield points were taken during stretching at different temperatures, as demonstrated in Fig. 3. It is known that the stress in heterogeneous system is not homogeneously distributed. The stress is prone to concentrated on sites with a misfit of mechanical properties, whose value may increase locally manyfold and therefore trigger the nucleation of cavities. In the case of crystalline polymers, such sites are located on the defective points in the amorphous phase between the crystals. Moreover, the nucleation and growth of cavities is related to the plastic resistance of polymer crystals. For polymers with crystals of higher plastic resistance, the cavitation is prone to occur. In contrast, the plastic deformation of crystal is easier to take place and the cavitation is retarded and weakened in polymers with crystals of lower plastic resistance.^22–24 Drawn at 30 °C, the significant stress whitening appears after the first yield, which can be attributed to the nucleation and growth of massive cavities in the amorphous phase due to the high plastic resistance of crystals at this temperature.^22,24 It reveals that the first yield is initiated by a heterogeneous deformation process. Moreover, the nucleation and growth of cavities is responsible for strain softening behavior,^24–26 therefore the large amplitude of strain softening can be observed after yield point. With increasing draw temperature up to 50 °C, the stress whitening phenomenon also occurs after the first yield point but with much weaker intensity. It indicates that much less cavities is formed after yield point due to the decreased plastic resistance of crystals, therefore leading to the much weaker strain softening process, as shown in Fig. 2(b). When stretched at 80 °C (above T_g), the first yield is replaced by the second one. The stress whitening phenomenon cannot be observed after yield point, even at larger strain values, indicating that the second yield is initiated by a homogeneous deformation mechanism with no cavitation due to the lower plastic resistance of crystals.

Fig. 3 The photographs taken at specific strains during the stretching of A70PLA samples at (a) 30 °C, (b) 50 °C, and (c) 80 °C with the draw rate of 4 μm s⁻¹. The white numbers inserted in the pictures are the strain values. The red scale bar in figure is 10 mm.

For the PLA samples annealed within 80–120 °C, the crystallinity and the crystalline regularity increase significantly as compared with the UnPLA and A70PLA samples. Consequently, the corresponding yield behavior exhibits a distinct variation with the draw temperature. As shown in Fig. 4, the first yield with high yield tress and sharp strain softening can be observed when stretched at low temperatures (30 and 40 °C). Particularly, the A100PLA and A120PLA samples show a brittle fracture at 30 °C due to their high crystallinity, crystalline regularity and the low stretching temperature. Upon stretching just below T_g (50–60 °C), a peculiar yield behavior can be found, i.e., double yield points appearing in one tensile deformation process. With strain increasing, the first yield point appears after the elastic deformation, followed by no strain softening process but the further increase of stress. After the completion of the first yield, the second yield occurs with a moderate strain softening process until to fracture. In addition, for a given draw temperature, the first yield point becomes less pronounced with increasing crystallinity of samples. With draw temperatures above T_g (70–90 °C), the first yield disappears and the second one arises at higher yield strains with a slow and long strain softening process. When stretched at the high draw temperatures (100–150 °C), none of the yields can be expressed due to their brittle fracture.

Fig. 4 The engineering stress–strain curves of (a) A80PLA, (b) A90PLA, (c) A100PLA and (d) A120PLA samples stretched at different temperatures with the draw rate of 4 μm s⁻¹. The early yield with smaller yield strain is named as the first yield, Yield-1, and the late one with larger yield strain as the second yield, Yield-2.

The photographs of the A80PLA samples taken before and after the yield points during stretching at different temperatures are represented in Fig. 5 to observe the above peculiar yield behaviors. Drawn at 40 °C, the stress whitening phenomenon initiates after the first yield point and becomes significant with strain increasing. This can be ascribed to the pronounced cavitation due to the high plastic resistance of crystals, which induces the sharp strain softening.^24,26 During the double yield process at 60 °C, the stress whitening cannot be observed during the first yield process but appears immediately after the second yield point, becoming pronounced with strain increasing. This reveals that the first yield process is dominated by the homogeneous deformation while the second yield is related to the heterogeneous deformation, which can be ascribed to the retarded cavitation because of the decreased plastic resistance of crystals. When stretched at 80 °C, there is no stress whitening appeared after the second yield point, even at higher strains of the strain softening process, which strongly supports that the second yield is induced by a homogeneous deformation mechanism.

Fig. 5 The photographs taken at specific strains during the stretching of A80PLA samples at (a) 40 °C, (b) 60 °C, and (c) 80 °C with the draw rate of 4 μm s⁻¹. The white numbers inserted in the pictures are the strain values. The red scale bar in figure is 10 mm.

The A150PLA sample has the highest crystallinity and crystalline regularity among all the studied PLA samples, as discussed above, which makes it much stiffer and more difficult to deform plastically, even at temperatures higher than T_g. Therefore, the A150PLA samples drawn within 30–150 °C show the brittle fracture and the yield behavior cannot be detected, as shown in Fig. 6.

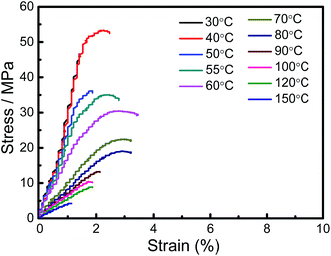Fig. 6 The engineering stress–strain curves of A150PLA samples stretched at different temperatures with the draw rate of 4 μm s⁻¹.

As it was discussed, the yield behavior of PLA samples with different crystallinity and crystalline regularity varies with draw temperature in two manners. In the first case of the UnaPLA and A70PLA samples with low crystallinity and crystalline regularity, the first yield is observed at lower yield strains and the strain softening becomes weaker and even disappears with increasing draw temperature up to 60 °C, and then the second yield replaces the first one at larger yield strains upon stretching at 70–90 °C (above T_g). The second case of the A80PLA to A120PLA samples with high crystallinity and crystalline regularity is that the first yield with sharp strain softening can be observed when drawing at 30 and 40 °C, the peculiar double yield appears in the range of 50–60 °C, and only the second yield is preserved above T_g (70–90 °C).

Comparing the double yield behavior of the PLA samples with high crystallinity and crystalline regularity (A80PLA to A120PLA samples) with that of polyethylene and its copolymers upon stretching at various temperatures,^7–12 one can find that the double yield of PLA samples occurs at temperatures just below T_g while that of polyethylene and its copolymers takes place well above T_g. Unlike the polyethylene and copolymers, the crystalline lamellae of PLA is difficult to orientate at about 45° to the draw direction due to the glassy state of amorphous phase and the crystal block is difficult for homogeneously shearing due to its high stiffness at temperatures below T_g. Moreover, the double yield of the PLA samples disappears and only the second one maintains at larger yield strains with draw temperature above T_g. Therefore, the deformation mechanisms proposed for the double yield of polyethylene and copolymers,^8,10,12,14 as described in the Introduction section, seem to be unsuitable for the present case of PLA samples. Based on the multiple-step crystallization process via mesomorphic phase of polymers proposed by Strobl et al.,²⁷ the mosaic block structure of crystalline lamellae consisting of the crystal block and the intermosaic block region^28–36 is applied to discuss the yield behavior of A80PLA to A120PLA samples, as shown in Fig. 7(a). The intermosaic block region here may represent some kind of disordered region,^28,37 which is easier to deform than crystal blocks and therefore being the defective position within the crystalline lamellae. Upon stretching at low temperatures (30 and 40 °C), the lamellae are stretched to brittle fragmentation through the intermosaic block region into small crystal blocks due to the very low plasticity of lamellae, which can be induced the significant cavitation in amorphous phase and also the sharp first yield with the large strain softening,^23,25 as shown in Fig. 4 and 5a. With draw temperature just below T_g (50–60 °C), the plasticity of lamellae increases and consequently the intermosaic block region can be stretched to shear homogenously after the elastic deformation, leading to the initiation of the first yield with no cavitation and therefore no strain softening. When the intermosaic block region shears largely enough, the crystal blocks within the lamellae start to slide each other through the intermosaic block region, initiating the second yield with significant cavitation and therefore pronounced strain softening. When stretched above T_g (70–90 °C), the plasticity of lamellae increases significantly, the mobility of chain segments in the intermosaic block region is almost same as that in amorphous phase. Therefore, the homogenous shear of the intermosaic block region becomes much easier and can occur concurrently with the stretching of amorphous phase, making the first yield point disappeared. Meanwhile, the crystal blocks within the lamellae can also more or less get sheared homogeneously due to the high mobility of chain segments of blocks. As the shear of the intermosaic block region and crystal blocks within lamellae reaches the maximum limit, the crystal blocks sliding occurs slowly through the intermosaic block region, inducing the second yield with a slow strain softening process but no cavitation.

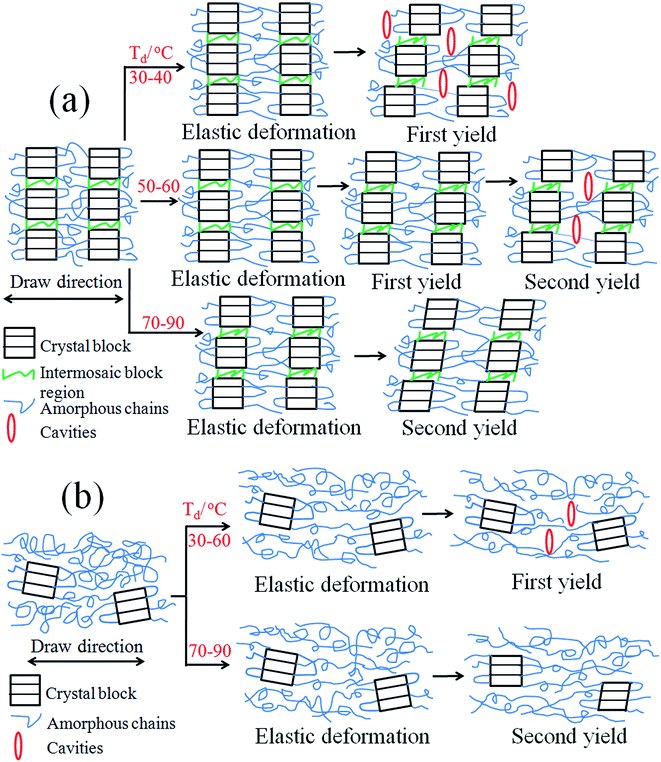Fig. 7 The mechanisms of the yield behavior of (a) the A80PLA to A120PLA samples and (b) the UnaPLA and A70PLA samples stretched at 30–90 °C.

However, for the PLA samples with low crystallinity and crystalline regularity (UnaPLA and A70PLA samples), there is no double yield occurred in one deformation process at all the drawing temperatures. With increasing drawing temperature, only the first yield appears below T_g (30–60 °C) and then the second yield occurs with a larger yield strain above T_g (70–90 °C). We considered that the intermosaic block region in the mosaic block structure of crystalline lamellae plays an important role in the occurrence of the double yield for the A80PLA to A120PLA samples stretched within 50–60 °C. However, due to the low crystallinity and crystalline regularity of the UnaPLA and the low chain segments mobility during annealing at 70 °C, the merging of crystal blocks into the lamellae is difficult to occur. Therefore, there may be no intermosaic block region and correspondingly no crystalline lamellae but only the crystal blocks or granular crystals existing in the UnaPLA and A70PLA samples, as shown in Fig. 7(b). Upon stretching below T_g (30–60 °C), the crystal blocks are with high plastic resistance at lower draw temperature, therefore can induce significant cavitation in amorphous phase, leading to the first yield with the obvious strain softening.²³ With draw temperature increasing, the plastic resistance of crystal blocks becomes lower, leading to the gradual decrease of cavitation in amorphous phase and the smaller strain softening. With draw temperature above T_g (70–90 °C), the mobility of chain segments in amorphous phase increases, the plasticity of crystal blocks becomes much higher. Therefore, the amorphous phase can be stretched homogeneously to large extent and no cavitation forms. After elastic deformation stage, the crystal blocks initiate to orientate and shear along drawing direction, leading to the onset of the second yield.

The yield points of the PLA samples stretched within 30–90 °C were determined by a method of intersecting lines from the initial modulus and the almost linear region past the yield point.^7,12 The obtained values of yield strain and yield stress are illustrated in Fig. 8. As shown in Fig. 8(a), the first yield strain, ε_y-1, of all the samples decreases gradually with increasing draw temperature, which may be due to that the first yield can be activated by the higher temperature, irrespective of the yield mechanism, and therefore needs the lower yield strain. Conversely, the second yield strain, ε_y-2, of all the samples increases with increasing draw temperature, which is attributed to the homogeneous extending of amorphous region and the homogeneous shear of the intermosaic block region and crystal blocks before the second yield increase with increasing draw temperature. On the other hand, for the double yield of the A80PLA to A120PLA samples stretched within 50–60 °C, both ε_y-1 and ε_y-2 show a slight decrease with increasing annealing temperature. As well known, the PLA lamellar thickness decreases with increasing annealing temperature up to 120 °C due to the increased crystalline regularity.^18,19 Therefore the width of intermosaic block region within the lamellae becomes smaller. The narrower intermosaic block region of the lamellae in the samples annealed at higher temperatures will start to deform at lower strain and has a smaller shear deformation, leading to the lower ε_y-1 and ε_y-2.

Fig. 8 (a) The first and second yield strain, ε_y-1 and ε_y-2 and (b) the first and second yield stress, σ_y-1 and σ_y-2, of different temperature annealed PLA samples stretched at different temperatures, T_d, with the draw rate of 4 μm s⁻¹.

For all of the samples, both the first yield stress, σ_y-1, and the second one, σ_y-2, are decreased with increasing draw temperature due to the increased chain segments mobility, as shown in Fig. 8(b). The σ_y-1 of the UnaPLA and A70PLA samples decreases more rapidly than that of the A80PLA to A120PLA samples with increasing draw temperature up to 60 °C (T_g). The first yield of the former case is mainly controlled by the amorphous phase while that of the latter one is determined by the intermosaic block region of lamellae. Therefore, the σ_y-1 of the former one is more easily influenced by high temperature and decreases more drastically. On the other hand, for the A80PLA to A120PLA samples with double yield stretched within 50–60 °C, both the σ_y-1 and σ_y-2 decrease slightly with increasing annealing temperature, which could be also due to the decreased width of intermosaic block region of lamellae with increasing annealing temperature, being consistent with the relationship between the σ_y-1, σ_y-2 and the lamellar thickness proposed by the Gaucher-Miri et al.¹⁰ and Schrauwen et al.¹²

3.3. Young's modulus of the PLA samples at different temperatures

The Young's modulus, E, of different PLA samples was plotted as a function of draw temperature, T_d, and shown in Fig. 9. All of the PLA samples have the highest Young's modulus at the low draw temperature (30 °C). With increasing draw temperature up to 70 °C (just above T_g), the E value of all the PLA samples decreases rapidly and, especially, it shows the highest decrease rate within the temperature range of 50–70 °C (including T_g). At draw temperatures higher than 70 °C, the decrease of the E becomes much slower and even a slight increase appears for the UnaPLA and A70PLA samples. The crystalline polymers are consisting of at least two distinct material regions by definition heterogeneous materials,⁵ i.e., the crystalline and the amorphous regions. The modulus of crystalline polymers is dependent on not only the crystalline but also the amorphous region, namely, the mechanical coupling of both regions.⁶ The structure of crystalline polymers can be viewed as a mechanical network by treating the stiff crystallites as multifunctional crosslink units.⁵ In the present study, the Young's modulus of crystalline PLA can be considered as the resistance to stretching of the mechanical network composed of the amorphous and the crystalline phases within the elastic deformation. Upon stretching at low temperature (30 °C), both the amorphous and the crystalline phases are in deeply glassy state and difficult to deform under tensile stress. Therefore, the resistance to stretching of the mechanical network is high, leading to the high E. With increasing draw temperature up to 70 °C, although the crystalline phase is almost not affected owing to its high thermal stability, the amorphous phase is activated significantly due to the substantial devitrification. The resistance to stretching of the mechanical network decreases significantly, making the E show a rapid decrease with increasing draw temperature. The fastest decreasing of the E within 50–70 °C is attributed to the great activation of amorphous phase induced by the transition from glassy state to high-elastic state. With draw temperature higher than 70 °C, the amorphous phase is in the high-elastic state. Its chain segments mobility is high and only has a little increasing with increasing draw temperature. The crystalline phase is still in ordered state because of its high melting point and continues to act as the physical cross-linking point of the mechanical network. The resistance to stretching of mechanical network has a much slower decrease with increasing draw temperature, leading to the much slower decrease of the E. For the UnaPLA and A70PLA samples, because of their low crystallinity and crystalline regularity, the new crystallites can be formed and the originally disordered crystals can also be improved during temperature equilibration for draw temperatures above 70 °C, which leads to the stronger physical cross-linking of mechanical network. Therefore, the E of these two samples shows a slight increase with draw temperature higher than 70 °C.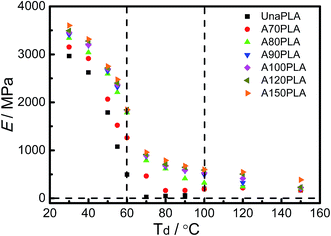

Fig. 9 The Young's modulus, E, of different temperature annealed PLA samples stretched at different temperatures, T_d, with the draw rate of 4 μm s⁻¹.

The reported studies revealed that the E of crystalline polymers is related not only to the crystallinity³ or the volumetric fraction of crystalline region⁵ but also to the crystal form composition.² The higher crystallinity or the more crystals with high regularity can lead to the higher E due to the stronger physical cross-linking of mechanical network. Upon stretching at a fixed temperature, the E of PLA samples increases gradually with increasing annealing temperature, but the amplitude of increase of the E is distinct for different samples. For the UnaPLA, A70PLA and A80PLA samples, the crystal form composition is the same (only the disordered α′ crystal) but the crystallinity increases significantly, which leads to the significant increase of the E. For the A80PLA to A150PLA samples, although the fraction of the more ordered α crystal increases substantially, their crystallinity are comparable, which leads to only a slight increase of the E. Based on the above discussion, we may speculate that the crystallinity of PLA is the major factor to determine the E and the fraction of the more ordered α crystal is the secondary one. It may be due to that the resistance to stretching of mechanical network is dependent more on the density of physical cross-linking point than on the strength of physical cross-linking point. Furthermore, at draw temperatures above 70 °C, the discrepancy of the E between the samples with low crystallinity (the UnaPLA and A70PLA) and those with high crystallinity (the A80PLA to A150PLA) becomes smaller with increasing draw temperature, which is due to the slight increase of the E of the former two samples with increasing draw temperature, as discussed above.

4. Conclusions

The injection-molded PLA samples were annealed at different temperatures and obtained the distinct crystallinity (X_c) and crystalline regularity (R_α). The UnaPLA and A70PLA samples possess the lower crystallinity and the disordered α′ crystal. For the A80PLA to A150PLA samples, the crystallinity is high and the fraction of ordered α crystal increases substantially with annealing temperature.

The different crystallinity and crystalline regularity of PLA samples can lead to the different yield behaviors. For the UnaPLA and A70PLA samples, the first yield occurs for draw temperatures below T_g and then the second yield replaces the first one when draw temperature above T_g. Considering that only the small crystal blocks rather than the crystalline lamellae can be formed in these samples, the first yield is induced by the strain-induced cavitation in amorphous phase, and the second yield is related to the orientation and shear of the small crystal blocks. For the samples annealed from 80 to 120 °C, the peculiar double yield appears upon stretching within 50–60 °C and only the first or the second yield occurs for the lower and higher draw temperatures. Based on the mosaic block structure of crystalline lamellae formed in these samples, the first yield occurred within 30–40 °C is related to the fragmentation of lamellae through the intermosaic block region, the first and the second yield within 50–60 °C is induced by the homogeneous shear of intermosaic block region and the sliding of crystal blocks within lamellae through intermosaic block region, respectively, and the second yield within 70–90 °C is initiated by the slow sliding of crystal blocks after the maximum extent of concurrent deformation of amorphous phase and intermosaic block region. The A150PLA sample exhibits the brittle fragmentation behavior and therefore no obvious yield at all the draw temperatures due to its highest X_c and R_α.

The ε_y-1 decreases while the ε_y-2 increases with increasing draw temperature for all of the PLA samples. Both the σ_y-1 and σ_y-2 of all the PLA samples decrease with increasing draw temperature, but exhibit the different decrease rates for different samples due to the different yield mechanisms. For the double yield of the samples annealed from 80 to 120 °C and stretched within 50–60 °C, both the ε_y-1, ε_y-2 and the σ_y-1, σ_y-2 show a slight decrease with increasing annealing temperature, which is due to the decrease of the width of the intermosaic block region within lamellae with increasing annealing temperature.

The E of all the PLA samples decreases with increasing draw temperature and shows the highest decrease rate within 50–70 °C, which is due to that the resistance to stretching of mechanical network composed of the amorphous and crystalline phases decreases with increasing draw temperature. The E increases with increasing X_c and R_α and is more dependent on X_c. For draw temperatures above 70 °C, the E of the UnaPLA and A70PLA samples shows a slight increase due to their low crystallinity and crystalline regularity, leading to the discrepancy of the E between them and the highly crystalline samples becomes smaller.

Acknowledgements

This work is supported by the National Natural Science Foundation of China (51573131 and 21374077) and the China Scholarship Council (CSC).

References

1. C. De Rosa, F. Auriemma and O. R. de Ballesteros, Phys. Rev. Lett., 2006, 96(167801), 1–4.
2. M. Cocca, M. L. Di Lorenzo, M. Malinconico and V. Frezza, Eur. Polym. J., 2011, 47, 1073–1080.
3. H. Tsuji and Y. Ikada, Polymer, 1995, 36, 2709–2716.
4. J. Y. Liu, S. J. Zhang, L. Y. Zhang, L. Z. Liu and Y. Q. Bai, Eur. Polym. J., 2014, 61, 83–92.
5. J. C. Halpin and J. L. Kardos, J. Appl. Phys., 1972, 43, 2235–2241.
6. M. Takayanagi, K. Imada and T. Kajiyama, J. Polym. Sci., Part C: Polym. Symp., 1966, 15, 263–281.
7. N. W. Brooks, R. A. Duckett and I. M. Ward, Polymer, 1992, 33, 1872–1880.
8. N. W. J. Brooks, A. P. Unwin, R. A. Duckett and I. M. Ward, J. Macromol. Sci., Part B: Phys., 1995, 34, 29–54.
9. R. Seguela and O. Darras, J. Mater. Sci., 1994, 29, 5342–5352.
10. V. Gaucher-Miri and R. Séguéla, Macromolecules, 1997, 30, 1158–1167.
11. X. R. Xu, J. T. Xu and L. X. Feng, Polym. Int., 2002, 51, 458–463.
12. B. A. Schrauwen, R. P. Janssen, L. E. Govaert and H. E. Meijer, Macromolecules, 2004, 37, 6069–6078.
13. M. Takayanagi and T. Kajiyama, J. Macromol. Sci., Part B: Phys., 1973, 8, 1–27.
14. R. J. Young, P. B. Bowden, J. M. Ritchie and J. G. Rider, J. Mater. Sci., 1973, 8, 23–36.
15. J. M. Zhang, Y. X. Duan, H. Sato, H. Tsuji, I. Noda, S. K. Yan and Y. Ozaki, Macromolecules, 2005, 38, 8012–8021.
16. J. M. Zhang, K. Tashiro, H. Tsuji and A. J. Domb, Macromolecules, 2008, 41, 1352–1357.
17. S. Y. Huang, H. F. Li, S. C. Jiang, X. S. Chen and L. J. An, Polymer, 2011, 52, 3478–3487.
18. T. Y. Cho and G. Strobl, Polymer, 2006, 47, 1036–1043.
19. J. M. Zhang, K. Tashiro, A. J. Domb and H. Tsuji, Macromol. Symp., 2006, 242, 274–278.
20. J. Mulligan and M. Cakmak, Macromolecules, 2005, 38, 2333–2344.
21. C. B. Zhou, H. F. Li, W. Y. Zhang, J. Q. Li, S. Y. Huang, Y. F. Meng, J. C. Christiansen, D. H. Yu, Z. H. Wu and S. C. Jiang, Polymer, 2016, 90, 111–121.
22. A. Pawlak and A. Galeski, Macromolecules, 2008, 41, 2839–2851.
23. A. Pawlak and A. Galeski, Macromolecules, 2005, 38, 9688–9697.
24. S. Castagneta, S. Girault, J. L. Gacougnollea and P. Dang, Polymer, 2000, 41, 7523–7530.
25. K. Schneider, S. Trabelsi, N. E. Zafeiropoulos, R. Davies, C. Riekel and M. Stamm, Macromol. Symp., 2006, 236, 241–248.
26. C. B. Zhou, H. F. Li, Y. Zhang, F. F. Xue, S. Y. Huang, H. Y. Wen, J. Q. Li, J. C. Christiansen, D. H. Yu, Z. H. Wu and S. C. Jiang, CrystEngComm, 2015, 17, 5651–5663.
27. G. Strobl, Eur. Phys. J. E: Soft Matter Biol. Phys., 2000, 3, 165–183.
28. T. Kajiyama, T. Okada and M. Takayanagi, J. Macromol. Sci., Part B: Phys., 1974, 9, 35–69.
29. Y. F. Men, J. Rieger and G. Strobl, Phys. Rev. Lett., 2003, 91(9), 095502.
30. Y. F. Men, J. Rieger, H. F. Endeler and D. Lie, Macromolecules, 2003, 36, 4689–4691.
31. Y. F. Men, J. Rieger and K. Hong, J. Polym. Sci., Part B: Polym. Phys., 2005, 43, 87–96.
32. Z. Y. Jiang, Y. J. Tang, Y. F. Men, H. F. Enderle, D. Lilge, S. V. Roth, R. Gehrke and J. Rieger, Macromolecules, 2007, 40, 7263–7269.
33. Z. Y. Jiang, Y. J. Tang, J. Rieger, H. F. Enderle, D. Lilge, S. V. Roth, R. Gehrke, Z. H. Wu, Z. H. Li and Y. F. Men, Polymer, 2009, 50, 4101–4111.
34. S. Patlazhan and Y. Remond, J. Mater. Sci., 2012, 47, 6749–6767.
35. Z. Y. Jiang, Y. J. Tang, J. Rieger, H. F. Enderle, D. Lilge, S. V. Roth, R. Gehrke, W. Heckmann and Y. F. Men, Macromolecules, 2010, 43, 4727–4732.
36. Y. Wang, B. Na, Q. Zhang, H. Tan, Y. Xiao, L. B. Li and Q. Fu, J. Mater. Sci., 2005, 40, 6409–6415.
37. R. Seguela and F. Rietsch, J. Mater. Sci. Lett., 1990, 9, 46–47.

---