Effect of nano structures on the nucleus wetting modes during water vapour condensation: from individual groove to nano-array surface
Abstract
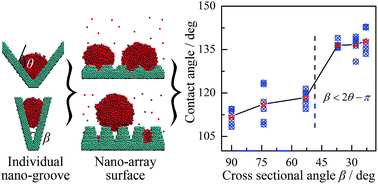
The geometrical structures of surfaces are important to the formation and growth of nuclei during water vapor condensation and the related heat and mass transfer performances. In the present research, the nucleus wetting modes on individual grooves and nano-array surfaces were investigated by molecules dynamics simulations. The results proposed a criterion that the nucleus wetting modes on a V-shaped groove are determined by the intrinsic contact angle θ and the cross sectional angle β. As the cross sectional angle decreases to β < 2θ − π, the nuclei can suspend in the groove center and the suspending height increases with decreasing β. For the nano-array surfaces, the nucleus can wet the grooves between adjacent nano arrays during the initial nucleation stage as the initial nuclei are very small and the nucleus surface are fluctuating frequently. The wetting mode may change as nucleation continuous and the nucleus can depart from the groove bottom to form a Cassie mode nucleus on a surface with β < 2θ − π. The apparent contact angle of nucleus also increases sharply with the wetting mode transition. Moreover, the dynamic behaviors of nucleating droplets were also observed on a nano-array superhydrophobic surface that meets the criterion. The droplets on this surface can recover the spherical shape after coalescence and the droplet jumping occurs, indicating a lower surface stiction. The results provide an insight of the interfacial phenomenon between the nucleus and the geometrical structures and propose a guideline to construct nano-array surfaces in the aim of promoting the Cassie mode nucleus.

 Please wait while we load your content...
Please wait while we load your content...