Hydrodynamics of a cocurrent downward liquid–liquid extraction column

Abstract

The present study focuses on the hydrodynamic characteristics of a cocurrent liquid–liquid downward system with perforated distributor. Hydrodynamic variables like pressure drop, phase holdup, drop size distribution and flow regimes are experimentally studied using a kerosene–water system. Two major flow regimes – kerosene continuous and kerosene dispersed – are identified. The effect of water and kerosene velocities on pressure drop and phase holdup in these two regimes is studied. Drop size distribution variation with water velocity is also studied. An empirical correlation is proposed for the dispersed phase holdup, which satisfactorily predicts the experimental data.

---

1. Introduction

Liquid–liquid extraction (LLE), an important industrial unit of operation, can be carried out using mixer-settlers, pulsed columns, rotary disc contactors, fluidized beds, etc. In LLE, transfer of one of the components is achieved by contacting the feed and the solvent as a dispersed or continuous phase. It is well known that countercurrent operations are more efficient than cocurrent operations in chemical engineering systems. However, in a countercurrent liquid–liquid extraction (CCLLE) column, the dispersed phase holdup is usually low. Further, wider distribution of drop size is observed. It is hypothesized that in cocurrent downward operation, higher holdup with almost uniform drop size distribution can be obtained. This may be expected because of the interplay of drag of continuous phase and buoyancy of dispersed phase.

Hydrodynamic studies on liquid–liquid vertical flow through pipes have been carried out in the literature in both cocurrent upward and downward modes. Hu and Angeli experimentally measured the holdup, pressure drop and drop size distribution for concurrent upward and downward flow of water and oil to explain the phenomenon of phase inversion.¹ The phase inversion velocity was measured using a conductivity method. The frictional pressure gradient was found to be minimum at the phase inversion point. The phase inversion point was different for the two modes of operation.

Flow regimes, holdup and pressure drop were experimentally studied by Jana et al. in cocurrent upflow through vertical pipes using oil and water.² The holdup and pressure drop were predicted using homogeneous, drift flux and two fluid model in the dispersed bubbly, bubbly & churn turbulent and core annular regime respectively. It is observed from the literature that most of the studies so far have focussed on small diameter pipe flows with high velocities, generally not encountered in extraction columns.

Very limited literature is available on cocurrent liquid–liquid downward flow. Babu et al. studied the hydrodynamics of liquid–liquid dispersion in a downflow column with a nozzle-ejector assembly.³ They reported the pressure drop across the column and dispersed phase holdup. Dimensional analysis was used and the data was correlated in terms of dimensionless numbers to predict pressure drop and holdup of dispersed phase. However, the geometric details like diameter and height of the column, ejector and divergent diffuser are not available. Gosh et al. reported pressure drop characteristics and flow patterns for liquid–liquid (oil–water) downflow through a 12 mm pipe with a coaxially placed nozzle jet to create core annular flow to minimize pressure drop.⁴ While the separated flow model was used to predict the pressure drop in the core annular regime, homogenous and drift flux models were used for slug and dispersed flow regimes. Empirical correlations have been proposed to better predict the data because of mismatch in the model prediction.

Based on the literature review it can be seen that the information on liquid–liquid downflow with conventional perforated distributor is not available to the best of our knowledge. The use of conventional distributor requires less energy compared to ejector type and leads to simpler design and operation. This type of distributor is also expected to result in higher dispersed phase holdup (compared to countercurrent upflow) with narrow drop size distribution. This is expected because of the cocurrent downward flow of both the phases and the interplay of drag and buoyancy.

The two studies mentioned above use ejectors to create dispersed phases in the system. The hydrodynamics of these systems starting from flow regimes, would be different from the proposed mode of operations in the present work. Hence, in the present study, it is planned to collect experimental data on the hydrodynamics of two-phase cocurrent downward liquid–liquid system with conventional perforated distributor. Hydrodynamic characteristics studied include pressure drop, phase holdups, flow regimes and drop size distribution.

2. Experimental details

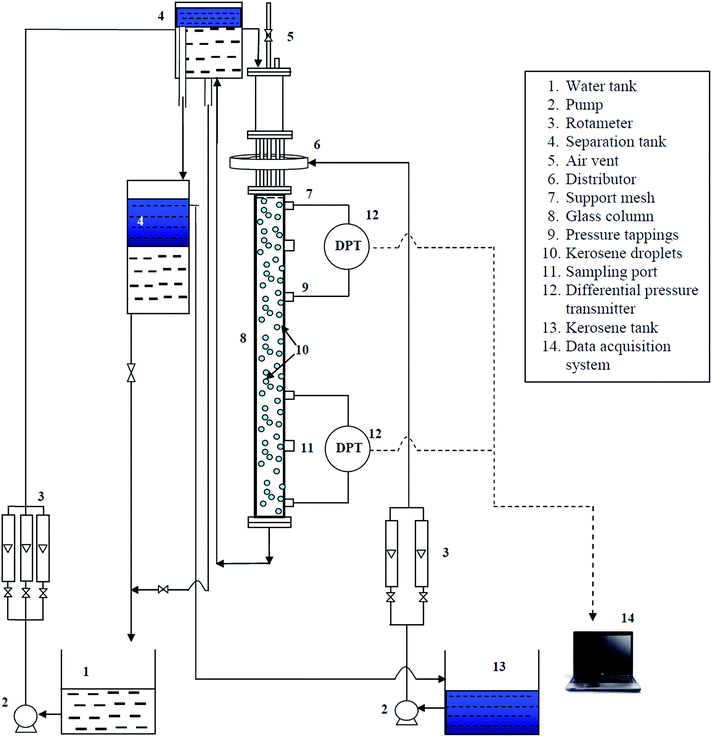
The schematic of the experimental setup used in the present study is shown in Fig. 1. The column was made of glass with an ID of 60 mm and OD of 70 mm with top and bottom sections made of acrylic for inlet and exit of the liquid streams. The test section (glass tube) has a total height of 2 m. The top section is used to distribute the two liquid streams as continuous and dispersed phase using a heat exchanger type distributor. The dispersed phase enters shell side whereas the continuous phase enters through tube side. The dispersed phase gets distributed through the perforations (2 mm holes on 5 mm pitch) at the bottom of the shell side of the distributor. The bottom section serves as exit to both the streams. The streams leaving the column flow through an overflow weir and are separated into two liquids using a series of separation tanks. Pressure taps are provided at an interval of 10 cm along the length of the column. Pressure tappings are connected to differential pressure transmitters (Rosemount 1151DP) and differential pressure data is acquired online using data acquisition system (Agilent 34792 0A). Some of the pressure tappings also serve as sampling ports for measurement of holdup of dispersed phase.

Fig. 1 Schematic of cocurrent downward liquid–liquid extraction column.

In the present study, kerosene and water are used as dispersed and continuous phases respectively. The properties of the liquids are given in Table 1. Kerosene was pumped from a storage tank through calibrated rotameters to the shell side of heat exchanger type distributor at the top. Water was also pumped from another storage tank through calibrated rotameters to the tube side of the distributor. Different rotameters were used to cover wide range of kerosene and water flowrates. The water and kerosene mixture exiting from the column at the bottom enters through the weir and through a series of separation tanks. After separation, kerosene and water flow back to the respective storage tanks.

Table 1 Properties of liquids

Sl. No.	Liquid	Density (kg m^−3)	Viscosity (Pa s)	Surface tension (mN m^−1)
1	Water	1000	0.000894	72.97
2	Kerosene	800	0.00166	25.44

---

In the present work, experiments were planned to collect data on pressure drop across the column, holdup, flow regimes and drop size distribution. The steady state was confirmed by constant pressure difference as indicated by differential pressure transmitters. After steady state was reached, the pressure drop was measured online using the data acquisition system at a sampling frequency of 1 Hz for 5 minutes. After pressure drop data were taken, samples of kerosene–water mixture were collected through sampling ports using quick opening and closing valve mechanism. The collected samples were allowed to separate and the fraction of the phases was measured. This procedure was repeated for various flowrates of kerosene and water. Flow regimes were studied by keeping water flowrate constant and varying the kerosene flowrate. In a typical experiment, after choosing particular water and kerosene flowrates, the changes in the column were visually observed and the corresponding flow regimes were noted after steady state was reached.

The drop size distribution was measured using the photographic technique.⁵ A small test section of ID 60 mm and height 26 cm was fitted in the middle of the test section. This test section was covered by a box made of glass of dimensions (20 cm × 20 cm × 15 cm). The box was filled with water to avoid deflection of light due to change in refractive index. Photographs of the section of the column covered by the glass box were taken using high-speed camera (AOS technologies, model: X-PRI) with 500 frames per s. Since the concentration of drops was very high, image processing tools could not be used to determine the drop size from the photographs. The size of the drops were manually measured by analyzing 175–200 drops from the photographic image (in Adobe Photoshop) by drawing parallel and perpendicular lines enveloping the droplets. The data obtained are analyzed for the drop size distribution. For all the above hydrodynamic experiments, the range of flowrates of kerosene and water used in the present study are 0–200 l h⁻¹ (0.10–1.97 cm s⁻¹) and 60–1000 l h⁻¹ (0.59–9.83 cm s⁻¹) respectively.

3. Results and discussion

In the present study, liquid–liquid cocurrent downflow contacting pattern, achieved using a perforated distributor is chosen for hydrodynamic studies on pressure drop, holdup, flow regimes and drop size distribution.

3.1 Pressure drop

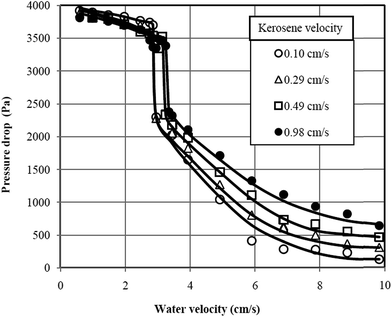
The cumulative pressure drop profile along the length of the column (from top to bottom) is shown in Fig. 2 for various water velocities at a constant velocity of kerosene. As expected, pressure drop increases along the length of the column. The pressure drop varies linearly along the length of the column, for a given set of kerosene and water flowrates indicating uniform pressure gradient. With increase in water flowrate, the pressure drop along the length of the column decreases due to more displacement of kerosene out of the system.

Fig. 2 Cumulative pressure drop profile along the height of the column.

The total pressure drop across the column with increase in water velocity is shown in Fig. 3 for various kerosene velocities. At a particular flowrate of kerosene, with increase in water flowrate, the total pressure drop decreases since kerosene is pushed out of the column. At low flowrates of water (<3 cm s⁻¹), the decrease in pressure drop is less. At low water flowrates, kerosene is in continuous phase and water flows in the form of streaks and strands. These streaks and strands of water are unable to exert sufficient drag to take away the kerosene from the system. This results in lower reduction in pressure drop. At high water flowrates (beyond 3 cm s⁻¹), kerosene is already in dispersed phase, exhibiting more surface area on which water can exert sufficient drag. This causes the kerosene droplets to be carried out of the column, thus decreasing the total pressure drop across the column drastically. In Fig. 3, one can observe a sudden decrease in the pressure drop, which indicates the transition from kerosene continuous to kerosene dispersed regime. With increase in kerosene flowrate, the amount of kerosene present in the column increases, thus increasing the total pressure drop.

Fig. 3 Variation of total pressure drop with water velocity for different kerosene velocities.

3.2 Phase holdup

In the present study, the holdup of phases is measured through sampling method as mentioned in experimental section. It is generally observed in multiphase systems, the fluidization equation

ΔP = (1 − ε_w)(ρ_w − ρ_k)gH (1)

is used to calculate the holdup of the dispersed phase.⁶ In eqn (1), ΔP, ρ_w, ρ_k, ε_w and H represent pressure drop, density of water, density of kerosene, holdup of water and height of test section respectively. In the present work also, eqn (1) is used to calculate the holdup of the dispersed phase. The comparison between holdup calculated from eqn (1) and sampling method is shown in Fig. 4. The kerosene holdup calculated using eqn (1) is higher than that measured using sampling method by 8.5% on the average. This may be due to size distribution of the kerosene droplets. As mentioned in the section on “Pressure drop”, at low flowrates, water is not dispersed properly and flows in the form of streaks and strands. Under these conditions the comparison of water holdup calculated from pressure drop measurements (eqn (1)) and sampling technique is poor as can be seen from Fig. 4.

Fig. 4 Comparison of dispersed phase holdup by sampling and pressure drop methods.

The variation of dispersed phase holdup with water velocity is presented in Fig. 5 for different kerosene velocities. At low water velocities, kerosene is in continuous phase and water flows in streaks and strands as dispersed phase. In the present work, it is observed that continuous kerosene phase changes to dispersed phase in the form of droplets at water velocities between 3–3.5 cm s⁻¹. The holdup shown in Fig. 5 before these velocities refers to the holdup of water as dispersed phase and after these velocities refers to the holdup of kerosene as dispersed phase.

Fig. 5 Variation of dispersed phase holdup with water velocity.

With increase in water velocity, the holdup of dispersed phase (water) gradually increases in low range of water velocities (∼<3.5 cm s⁻¹). The gradual increase in holdup of dispersed water phase is due to increase in water quantity. As mentioned earlier, between 3–3.5 cm s⁻¹ of water velocity the continuous kerosene phase transforms into dispersed kerosene phase. When this transition occurs, droplets of kerosene are very high in number contributing to high holdup of kerosene as dispersed phase. With further increase in water velocity beyond transition, the holdup of kerosene decreases since more drag of water on kerosene droplets carries away the kerosene phase from the column contributing to lower and lower holdup.

In the continuous kerosene flow regime, the effect of kerosene flowrate on holdup of water as dispersed phase is not very clear, even though the holdup of water increases with increase in water velocity. The reason for ambiguous trend with respect to kerosene flowrate is due to the heterogeneous structure of water in the column under these conditions. In kerosene dispersed regime, the effect of flowrate of kerosene can be seen clearly, with kerosene holdup increasing with increase in kerosene flowrate. It is obvious that the amount of kerosene increases with increase in its flowrate.

3.3 Prediction of phase holdup

The holdup of kerosene in the kerosene dispersed regime is empirically correlated using the equation

ε_k = au_w^b, u_w in m s⁻¹ (2)

where a = 32.9u_k^1.74 and b = 0.467 ln(u_k) + 0.945 with u_k in m s⁻¹. The constants a and b are first obtained by fitting ε_k vs. u_w as a power law and then a and b are correlated in terms of u_k. The comparison between the experimental and predicted kerosene holdup is shown in Fig. 6a. The empirical equation predicts the experimental data satisfactorily with an RMS error of 4.1%. The variation of kerosene holdup with water velocity for different kerosene velocities as predicted by the correlation (eqn (2)) is compared with the experimental data in Fig. 6b. As can be seen, the correlation predicts the experimental trend satisfactorily.

Fig. 6 Comparison of experimental and predicted holdup.

3.4 Flow regimes

In multiphase systems, one should know the boundaries of possible operational regimes, known as flow regimes of any equipment, usually in terms of phase velocities. Generally, the flow regimes are identified by careful visual observations or analysis of pressure drop/voidage signals by methodically changing the phase velocities. In the present work on liquid–liquid cocurrent downward system, careful, repeated and reproducible visual observations were used to identify the flow regimes by systematic variation of kerosene and water flowrates in small increments.

For a given kerosene flowrate, as the water flowrate is increased the flow regime changes from kerosene continuous to kerosene dispersed at a particular water flowrate. At low water flowrates, water flows in streaks and strands amidst continuous kerosene phase. With further increase in water flowrate a slow breakup of continuous kerosene phase into droplets is observed. Just before kerosene breaking into droplets, aggregates of size of 5–7 cm are observed with a structure of honeycomb. Careful visual observations revealed that the aggregate structure is made up of water droplets encapsulated by a film of kerosene. A slight increase in water flowrate breaks up the honeycomb structure and splits it into continuous water phase and dispersed kerosene phase. At this moment, it is observed that water being heavy slips through the outer film of kerosene leaving the kerosene film to form droplets of kerosene. At this point, the droplets of kerosene are nearly uniform (6–8 mm). Further increase in water flowrate decreases the droplet size maintaining nearly uniform size.

It is known in multiphase systems, that one of the extremes in flow regimes is flooding and this regime is absent in cocurrent systems. Hence, in the present system, the possible flow regimes are kerosene continuous (water dispersed) or kerosene dispersed (water continuous). These regimes are shown in Fig. 7 based on experimental observations. It is mentioned in the above paragraph that there is a velocity at which aggregates of honeycomb structure form just before kerosene breaks into droplets. This means that there is a combination of water and kerosene velocities to form honeycomb structure and there is a pair of water and kerosene velocities at which the honeycomb structure breaks into kerosene droplets. These velocities are shown in Fig. 7 as lines 1 and 2 respectively. The regime left to line 1 is kerosene continuous and the regime to the right of line 2 is kerosene dispersed or water continuous. The region sandwiched between lines 1 and 2 is honeycomb regime. As the kerosene velocity increases, the holdup of kerosene increases leading to more number of aggregates, which requires more amount of water. Similarly, more amount of water is required to break the structure into droplets. The formation of honeycomb structure as well as breaking into droplets requires a small change in water velocity and large change in kerosene velocity as can be seen from Fig. 7. This may be due to higher density of water, which can easily break the lighter kerosene into droplets.

Fig. 7 Flow regime map.

The holdup of kerosene at the point of breaking of honeycomb structure into kerosene droplets (line 2 in Fig. 7), corresponding to transition from kerosene continuous to kerosene dispersed regime is shown in Fig. 8. The holdup increases with increase in kerosene and water velocity (shown at the top of the graph) and reaches a constant holdup of around 0.6, which corresponds to the maximum packing fraction in a packed bed for spheres. At low velocities of kerosene, the holdup of kerosene is less and at the point of breaking into droplets, the droplets will not pack to the maximum extent (0.6) and hence the holdup is less than 0.6. The holdup obtained for any combination of water and kerosene flowrates in kerosene dispersed regime lie below the curve shown in Fig. 8.

Fig. 8 Kerosene holdup along the regime transition boundary.

3.5 Drop size distribution

Drop size and drop size distribution are important parameters for the design of any multiphase apparatus. As mentioned in the introduction, the droplets are expected to be nearly uniform. In the present work, with cocurrent downward operation of both the phases, large droplets of size 6–8 mm were observed immediately after the continuous kerosene phase transformed to dispersed kerosene phase. Immediately after transition velocity, with an increase in continuous phase (water) velocity, these large spherical drops were broken into smaller droplets due to higher drag exerted by the continuous phase on the dispersed (kerosene) phase. The smaller droplets were carried away by the continuous phase out of the system, which is also one of the reasons for decrease in holdup in the column with increase in water velocity. Photographic images of drops and drop size distribution obtained as discussed earlier, are shown in Fig. 9a and b respectively for different water flowrates at a given kerosene flowrate. It can be concluded from the figures that, for the cocurrent downward operation (i) drop size distribution at any water flowrate almost follows normal distribution (ii) droplets are more narrowly distributed at higher water flowrates than at low water flowrates and (iii) the decrease in average drop size with water flowrate is less (Sautermean diameters are 2.79, 2.48 and 2.44 mm for water velocity of 5.9, 7.9 and 9.8 cm s⁻¹ respectively).

Fig. 9 (a) Photographs of kerosene droplets for different water velocities at 0.20 cm s⁻¹ kerosene velocity. (b) Variation of drop size distribution with water velocities.

4. Conclusions

Hydrodynamics of cocurrent liquid–liquid downflow system with conventional perforated distributor has been studied in the present work. Experimental studies have been carried out to determine pressure drop, phase holdup, drop size distribution and flow regimes. Two major flow regimes are observed viz. kerosene continuous at lower water velocities and kerosene dispersed at higher water velocities. The kerosene flow rate required for this phase transition increases with increase in water velocity. While the pressure drop does not decrease much in the kerosene continuous regime, it decreases rapidly in the kerosene dispersed regime with a steep fall at the phase transition velocity. Increase in kerosene velocity increases the pressure drop. With increases in water velocity, the water hold up increases marginally in the kerosene continuous regime and the kerosene holdup decreases significantly in the kerosene dispersed regime. The effect of kerosene flowrate on the water holdup is not clear in the kerosene continuous regime. However in the kerosene dispersed regime, kerosene holdup increases with kerosene flowrate. The kerosene holdup at the regime transition velocity varies from ∼0.55 at lower velocities to ∼0.6 for higher velocities. Narrow size distribution of droplets has been observed in the cocurrent downward kerosene–water system. In the kerosene dispersed regime, the average droplet size (sauter mean diameter) decreases marginally with increase in continuous phase velocity (water).

References

1. B. Hu and P. Angeli, Can. J. Chem. Eng., 2006, 84, 94–107.
2. A. K. Jana, P. Ghoshal, G. Das and P. K. Das, Chem. Eng. Technol., 2007, 30, 920–925.
3. P. S. K. C. Babu, G. Kundu and D. Mukherjee, Indian J. Chem. Technol., 1999, 6, 243–246.
4. S. Ghosh, G. Das and P. K. Das, J. Fluids, 2011, 133, 011202.
5. M. H. I. Baird and S. J. Lane, Chem. Eng. Sci., 1973, 28, 947–957.
6. L. Lapidus and J. C. Elgin, AIChE J., 1956, 3, 63–68.

---