Enhancement of the oxygen evolution reaction in Mn³⁺-based electrocatalysts: correlation between Jahn–Teller distortion and catalytic activity

Abstract

We systematically studied the catalytic activity of the oxygen evolution reaction (OER) for the tetragonal spinel oxide Mn_3−xCo_xO₄ (0 ≤ x < 1 and 1 < x ≤ 1.5). The OER catalytic activity of Mn_3−xCo_xO₄ (0 ≤ x < 1) dramatically improved with an increase in Co content. We found that the OER activity of Mn_3−xCo_xO₄ (0 ≤ x < 1) increased linearly with the suppression of the Jahn–Teller distortion. We therefore propose that the Jahn–Teller distortion plays an important role in the OER activities of compounds containing Mn³⁺. Mn_3−xCo_xO₄ (0 ≤ x < 1) provides a rare case for directly studying the effect of the Jahn–Teller distortion on OER activity.

---

1 Introduction

The material design of low cost and highly active catalysts for energy storage applications is crucial for solving global energy problems. The oxygen evolution reaction (OER) is used for charging rechargeable metal-air batteries^1,2 and in direct solar water splitting.^3,4 Although precious metal-based catalysts with high OER activities (such as RuO₂ and IrO₂ (ref. 5)) facilitate the reaction, the kinetics of the OER are rather sluggish due to its multistep proton coupled electron transfer.⁴ It is therefore important to explore the mechanism of the OER and to discover and develop a highly active catalyst for the process.

As demonstrated by Suntivich et al.,⁶ when the number of electrons in the e_g orbital is close to unity for transition metals, perovskite oxides exhibit maximum OER activity. In other words, Mn³⁺ (t³_2g e¹_g for both surface and bulk), Co³⁺ (t⁵_2g e¹_g for surface), and Ni³⁺ (t⁶_2g e¹_g for both surface and bulk) are OER active sites for Mn³⁺, Co³⁺, and Ni³⁺-based perovskite oxides.^6–9 However, LaMnO₃ exhibits a significantly lower specific OER activity compared with LaCoO₃ and LaNiO₃ (∼6% of LaNiO₃ at 1.8 V vs. RHE).¹⁰ It is therefore important to explore what causes degradation of the OER activity of Mn³⁺-based (t³_2g e¹_g) compounds to improve their catalytic activity. Among such compounds, Mn₃O₄ (Mn²⁺[Mn³⁺]₂O₄) is abundant in nature as the mineral hausmannite,¹¹ and is an inexpensive and effective catalyst for limiting the emission of carbon monoxide and NO_x.^12,13 Mn₃O₄ adopts a tetragonally distorted spinel structure (Fig. 1) under ambient conditions^14,15 due to the Jahn–Teller active Mn³⁺ (t³_2g e¹_g) occupying the octahedral site. The Jahn–Teller distorted Mn³⁺O₆ octahedra consist of four shorter Mn–O bonds (0.192 nm) and two longer Mn–O bonds (0.228 nm).¹⁵ Although Mn₃O₄ contains the OER active site (i.e. Mn³⁺) previous studies report a low specific OER activity (∼40% of Mn₂O₃ at 1.8 V vs. RHE¹⁶) similar to LaMnO₃.

Fig. 1 Schematic image of the crystal structure of Mn_3−xCo_xO₄ nanoparticles.

We herein attempt to improve the performance of Mn₃O₄ by controlling the degradation of its OER activity. To directly compare the OER activities of Mn³⁺-based spinel oxides, Mn³⁺ concentrations at the octahedral site and at the tetragonally distorted structure must be maintained. Mn_3−xCo_xO₄ (0 ≤ x < 1), a series of tetragonally distorted spinel compounds, provide such an opportunity, as their octahedral sites remain occupied by only Mn³⁺ ions.^17,18 Although the OER activities of Mn₂CoO₄ (ref. 19 and 20) (usually tetragonal phase) and Mn_yCo_3−yO₄ (0 ≤ y ≤ 1)^19,21,22 (usually cubic phase) have been previously studied, the OER activities of Mn_3−xCo_xO₄ (0 ≤ x < 1) have not been studied. We therefore chose to systematically study the OER performance of tetragonal Mn_3−xCo_xO₄ (0 ≤ x < 1 and 1 < x ≤ 1.5). Nanoparticles were prepared at ∼20 °C to minimize the influence of geometric factors (e.g., morphology and surface area) and to minimize the influence of the statistical error for catalytic activity per unit mass.

2 Experimental

2.1 Preparation and characterization

Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) nanoparticles were synthesized at room temperature according to the following liquid phase synthetic process. Mn(CH₃COO)₂·4H₂O (Wako, 99.9%) and Co(CH₃COO)₂·4H₂O (Wako, 99.9%) (total 2 mmol) in a stoichiometric molar ratio were dissolved in a mixture of ethanol (17 mL) and distilled water (5 mL). The solution was mixed in air for 10 min at ∼20 °C to give a homogenous solution. NH₃·H₂O (9 mL, Wako, 25–28 wt%) was then added, and mixed under air for 20 min at ∼20 °C to evenly disperse the particles in the solution. The resulting mixture was centrifuged for 15 min at 8000 rpm, and the supernatant removed. The obtained wet product was washed twice with excess ethanol, and dried at 70 °C for 24 h to remove any residual ethanol and water.

The prepared Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) nanoparticles were investigated by powder X-ray diffraction (XRD) using a Rigaku SmartLab diffractometer with Cu Kα radiation (λ = 1.5418 Å, 45 kV, 200 mA, step size = 0.02° s⁻¹). GSAS software was used for Rietveld refinement of the crystal structure.²³ The value of x in the Mn_3−xCo_xO₄ nanoparticles were determined on the basis of lattice constants, specifically the Jahn–Teller distortion indicator: c/√2a which decreases with the increase of the Co content. First, the values of x were calculated using the initial molar ratio in the starting material. Then, the validity of x in the nanoparticles was checked by comparing the values of c/√2a in this study with those obtained by neutron diffraction studies in Bordeneuve et al.¹⁷ (Fig. S3(d)†).

Transmission electron microscopy (TEM) was conducted on selected nanoparticle samples (x = 0, 0.3, 0.6, 0.9) using an H-9000 NAR (Hitachi Ltd.) with an acceleration voltage of 300 kV. Nitrogen Brunauer–Emmett–Teller (BET) surface area measurements were conducted for the Mn_3−xCo_xO₄ (0 ≤ x ≤ 0.9) nanoparticles at 77 K with a conventional high vacuum static system.

2.2 Electrochemical measurement of the OER activity

The catalyst inks of the nanoparticle samples for electrochemical measurement were prepared referring to the methods reported by Suntivich et al.^6,24 and Jung et al.²⁵ K⁺ ion-exchanged Nafion® was used as an immobilizing binder, enabling smooth transport of dissolved O₂ to the surface of the catalysts. A 3.33 wt% K⁺ ion-exchanged Nafion® suspension was first obtained from a mixture of 5 wt% proton-type Nafion® suspension (Sigma-Aldrich) and a 0.1 M aqueous KOH solution in a 2 : 1 (v/v) ratio. This process increased the pH of the initial 5 wt% proton-type Nafion® suspension from 1–2 to 11. The catalyst inks of Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) nanoparticle samples were prepared from a mixture of the as-prepared samples (25 mg), acetylene black (AB, Denka, 5 mg), and 3.33 wt% K⁺ ion-exchanged Nafion® suspension (1.5 mL). The total ink volumes were adjusted to 5 mL by the addition of tetrahydrofuran (THF, Sigma Aldrich), to give final concentrations of 5 mg sample/mL, 1 mg AB/mL, and 10 mg Nafion/mL in the ink. A sample of the ink (6.4 μL) was then drop-casted on a rotating ring-disk electrode composed of a glassy carbon (GC) disk (0.2 × 0.2 × π cm²) (BAS Inc., Japan) and a Pt ring (ϕ_inner = 0.5 cm, ϕ_outer = 0.7 cm) (BAS Inc., Japan), which was used as the working electrode after mirror polishing with 0.05 μg alumina slurry (BAS Inc., Japan). The rotating ring-disk electrode bearing the Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) ink was then dried overnight under vacuum at room temperature.

Electrochemical measurements were conducted using a rotating ring disk electrode rotator (RRDE-3A, BAS Inc., Japan) at 1600 rpm, in combination with a bipotentiostat (ALS Co., Ltd, Japan). In addition, a Pt wire counter electrode, and an Hg/HgO reference electrode (International Chemistry Co., Ltd., Japan) filled with 0.10 M KOH (Nacalai Tesque, Inc., Japan) were used. Electrochemical measurements were conducted with O₂ saturation (30 min bubbling O₂ gas through the solution) at ∼25 °C, where the equilibrium potential of the O₂/H₂O redox couple was fixed at 0.304 V vs. Hg/HgO (or 1.23 V vs. RHE). During OER current density measurements (100 cycles for x = 0, 0.3, 0.6, 0.9, 1.5, and 10 cycles for x = 1.2) for each Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) sample, the potential of the sample-modified GC was controlled from 0.3–0.9 V vs. Hg/HgO (1.226–1.826 V vs. RHE) at 10 mV s⁻¹. For all measurements, the OER current density was iR-corrected (R = ∼43 Ω) using the measured solution resistance, and capacitance-corrected by averaging the anodic and cathodic scans⁶ to remove the influence of the current related to the formation of an electrical double layer. For comparison, specific OER activities, Tafel slopes,^26,27 and overpotentials of the Mn_3−xCo_xO₄ (0 ≤ x ≤ 1.5) samples were obtained from the OER current density data (up to 100 cycles).

3 Results and discussion

XRD patterns (Fig. S1†) demonstrate that the prepared Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9, 1.2, 1.5) samples are single-phase. All XRD peaks were indexed by the distorted spinel structure with the tetragonal space group I4₁/amd. The morphologies of the Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9) samples were then examined by TEM (Fig. 2). As shown in Fig. 2, the TEM images show nanoparticles with an isotropic morphology, exhibiting average particle sizes of ∼17 nm for x = 0 and ∼15 nm for x = 0.3–0.9.

Fig. 2 Typical transmission electron microscopy (TEM) images of Mn_3−xCo_xO₄ nanoparticles for x = 0, 0.3, 0.6, and 0.9. All scale bars equal 100 nm.

In terms of site occupancy, the tetragonally distorted Mn_3−xCo_xO₄ spinel (x = 0, 0.3, 0.6, 0.9, 1.2, 1.5) can be divided into two groups, i.e. 0 ≤ x < 1 and 1 < x ≤ 1.5. The octahedral site of the former group is occupied only by the Mn³⁺ ions (Fig. S2(a)†),¹⁷ while the octahedral site of the latter group is composed of a mixture of Mn³⁺, Co³⁺, Mn⁴⁺, and Co²⁺ ions.¹⁷ To determine the key factor that determines the OER catalytic performance of the Mn_3−xCo_xO₄ spinel materials, we first chose to focus on the Mn_3−xCo_xO₄ (0 ≤ x < 1) group.

Fig. 3(a) shows the capacitance-corrected voltammetry curves of the oxygen evolution reaction (OER) for Mn_3−xCo_xO₄ (0 ≤ x < 1). The higher the OER current density is, the higher the OER activity of the catalyst is. Therefore, Fig. 3(a) demonstrates that the OER activity of Mn_3−xCo_xO₄ (0 ≤ x < 1) elevates with the increase in Co content. Fig. 3(b) shows the linear correlation between the logarithmic OER current density and the iR-corrected potential for Mn_3−xCo_xO₄ (0 ≤ x < 1), known as the Tafel plot.^26,27 The data used in Fig. 3(b) were extracted from Fig. 3(a). The smaller the slope of the Tafel plot (called Tafel slope) is, the higher the OER activity of the catalyst is. Fig. 3(b) exhibits the elevation of the OER activity with the increase in Co content. Fig. 3(c) shows the correlation between the OER current density at 1.76 V vs. RHE (specific OER activity) and the Jahn–Teller distortion indicator: c/√2a. Specific OER activities were compared at 1.76 V vs. RHE to minimize the influence of the statistical error. Fig. 3(d) shows the durability of Mn_3−xCo_xO₄ (0 ≤ x < 1) as OER catalysts. Evaluation of durability is important when putting catalysts into application for metal-air batteries. The ratio of Tafel slope of cycle 100 towards cycle 1 was used as an indicator of the durability. Longer term stability as an OER catalyst was observed for Co-enriched members (Fig. 3(d)). All these figures (Fig. 3) demonstrate that the OER performance of Mn_3−xCo_xO₄ (0 ≤ x < 1) improves with the increase in Co content. In particular, Mn_2.1Co_0.9O₄ exhibited an excellent OER performance with a high specific OER activity (1700% of Mn₃O₄ at 1.76 V vs. RHE), along with long-term stability over 100 cycles (>3.3 h). As recently reported for perovskite oxides,^6–9 when the number of electrons in the e_g orbital is close to unity for transition metal ions, they play the role of OER active sites. In the case of Mn_3−xCo_xO₄ (0 ≤ x < 1), Mn³⁺ (t³_2g e¹_g) at the octahedral site forms an antibonding e_g orbital with the oxygen-related adsorbates (O₂²⁻ and O²⁻). The antibonding Mn³⁺ e_g orbital has the strongest overlap with oxygen since it is at a higher energy level relative to the bonding t_2g orbitals of Mn³⁺ and the antibonding t_2g orbitals of Mn²⁺ (e²_g t³_2g)/Co²⁺ (e⁴_g t³_2g) at the tetrahedral site (Fig. 4). Therefore, Mn³⁺ becomes the OER active site for Mn_3−xCo_xO₄ (0 ≤ x < 1). Since the number of Mn³⁺ e_g electrons remained constant with an increase in Co content (only Mn³⁺ ions occupied the octahedral site), the higher OER activities of Co-enriched members could not be explained by the number of e_g electrons. In addition, as the surface areas of Mn_3−xCo_xO₄ (x = 0.3, 0.6, 0.9) are ∼1.2 times that of Mn₃O₄,²⁸ this small variation of surface area cannot be used to explain the different OER activities for Mn_3−xCo_xO₄ (0 ≤ x < 1). Furthermore, the influence of electrical conductivity can be neglected as it remains almost constant for 0 ≤ x < 1 (Fig. S2(b)†). Thus, it is necessary to find alternative explanations for the higher OER activities of the Co-enriched species.

Fig. 3 (a) Linear sweep voltammetry curves of the OER (cycle 10) for Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9) in 0.10 M aqueous KOH at 10 mV s⁻¹. (b) Tafel plots and the variation of Tafel slopes (cycle 10) for Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9). (c) Variation of the specific OER activities (cycles 1–10) for Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9) towards the Jahn–Teller distortion indicator: c/√2a. (d) The OER durability of Mn_3−xCo_xO₄ (x = 0, 0.3, 0.6, 0.9). The ratio of the Tafel slope of cycle 100 towards cycle 1 (%) was used as an indicator of the OER durability.

Fig. 4 Schematic view of the Mn³⁺ 3d orbital energy levels at the octahedral site and the Mn²⁺/Co²⁺ 3d orbitals at the tetrahedral site for Mn_3−xCo_xO₄ (0 ≤ x < 1). Triply degenerated Mn³⁺ t_2g orbitals and doubly degenerated Mn³⁺ e_g orbitals split into two energy levels to lower the total energy of the Mn³⁺ 3d electrons (Jahn–Teller effect). The Mn³⁺O₆ octahedra then became distorted (Jahn–Teller distortion). The vertical dotted lines represent the energy levels of Mn³⁺ t_2g and e_g orbitals in the absence of the Jahn–Teller effect. The Mn³⁺ e_g orbital (occupied by a single electron) is at a higher energy level than the Mn²⁺/Co²⁺ t_2g orbitals, due to the larger crystal field splitting in octahedral symmetry. As c/√2a (indicator of Mn³⁺O₆ distortion) decreases with an increase in Co content, the octahedral distortion is suppressed (Mn³⁺ e_g orbital splitting becomes smaller) and the single electron occupying the Mn³⁺ e_g orbital shifts to a higher energy level.

In this context, we chose to examine whether the electronic state of Mn³⁺ changes in favor of enhancing the OER activities for Mn_3−xCo_xO₄ (0 ≤ x < 1). Since lattice, spin and orbital degrees of freedom are strongly coupled for the Mn³⁺-based tetragonally distorted spinel species,^29–31 the electronic state of Mn³⁺ can be probed by the crystal structure. Therefore, we examined the electronic state of Mn³⁺ focusing on the Jahn–Teller distortion of Mn³⁺O₆ octahedra. When 3d transition metals (M) are situated at the center of the MO₆ octahedra, their 3d orbitals split into triply degenerated t_2g orbitals and doubly degenerated e_g orbitals. As Mn³⁺ has four 3d electrons, the Mn³⁺ 3d orbitals split into two energy levels to lower the total energy of the Mn³⁺ 3d electrons (Fig. 4). When the Jahn–Teller distortion of Mn³⁺O₆ octahedra is suppressed (equivalent to c/√2a decreasing) due to the increase in Co content (Fig. S3†), the splitting of the Mn³⁺ e_g orbitals becomes smaller and the electron occupying the Mn³⁺ e_g orbital shifts to a higher energy level (Fig. 4). Thus, the overlap of the antibonding Mn³⁺ e_g orbitals with the O 2p orbitals of the oxygen adsorbate becomes stronger. The OER activity of Mn_3−xCo_xO₄ should therefore be enhanced due to the stronger binding of OER intermediates to the catalytic surface. This prediction was experimentally supported by the linear correlation between the indicator of the Jahn–Teller distortion (c/√2a) and the OER activity (Fig. 3(d)). The linear correlation becomes more pronounced when the OER activity is divided by the normalized BET surface area (Fig. S4(d)†). We could therefore conclude that a correlation exists between the suppression of the Jahn–Teller distortion and the OER activity. For compounds containing Mn³⁺, not only the number of e_g electrons but also the Jahn–Teller distortion plays a crucial role in their OER activities. Minimizing the Jahn–Teller distortion (without changing the number of e_g electrons) could further improve the OER activities of Mn³⁺ based compounds. Since the suppression of the Jahn–Teller distortion (by increasing the Co content) is physically equivalent to raising the temperature of Mn₃O₄ (Mn₃O₄ becomes cubic at 1170 °C (ref. 32)), our result suggests a future application of Mn_3−xCo_xO₄ (0 ≤ x < 1) as an energy saving catalyst for metal-air batteries.

In contrast, the OER performance of Mn_3−xCo_xO₄ decreased with an increase in Co content for 1 < x ≤ 1.5 (Fig. 5). Lower specific OER activities (Fig. S5(a)†), slightly larger Tafel slopes (Fig. S5(b)†), and relatively constant overpotentials (Fig. S5(c)†) were observed for Mn_3−xCo_xO₄ (x = 1.2, 1.5) when compared to Mn_2.1Co_0.9O₄. This OER behavior can be explained in terms of the electrons in the e_g orbital and the Jahn–Teller distortion, as was the case for Mn_3−xCo_xO₄ (0 ≤ x < 1). When the Co content increased above x > 1, the octahedral site was occupied by a mixture of Mn³⁺, Co³⁺, Mn⁴⁺, and Co²⁺ ions.¹⁷ This is in contrast with Mn_3−xCo_xO₄ (0 ≤ x < 1) where the octahedral site is occupied only by Mn³⁺ (Fig. S2(a)†). When the number of electrons in the e_g orbital changes from unity for transition metal ions, they cannot remain as the OER highly active sites. Therefore, the increase of Mn⁴⁺ (t³_2g e⁰_g) and Co²⁺ (t⁵_2g e²_g) concentration (at the epical center of the octahedra) with the increase in Co content degrades the OER activity of Mn_3−xCo_xO₄ (1 < x ≤ 1.5). On the other hand, the suppression of Jahn–Teller distortion with the increase in Co content (Fig. S3†) elevates the OER activity. These two effects compete with each other, and the OER activities of x = 1.2 and 1.5 drop slightly compared with that of x = 0.9. The reduction in Mn³⁺ (t³_2g e¹_g) concentration degrades the OER activity, but the increase of Co³⁺ (OER active site: t⁵_2g e¹_g for surface) compensates this effect. It should be noted that maintaining the concentration of cations with a single e_g electron (Mn³⁺ and Co³⁺ at the octahedral site) is essential to improve the OER activity. Therefore, in summary, the OER performance of Mn_3−xCo_xO₄ (1 < x ≤ 1.5) does not contradict with the explanation for the OER performance of Mn_3−xCo_xO₄ (0 ≤ x < 1), thus strongly supporting a correlation between the suppression of Jahn–Teller distortion and the OER activity of the material.

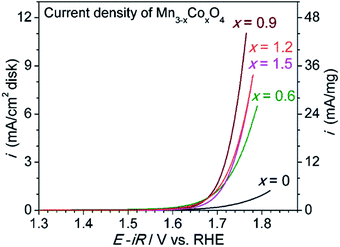Fig. 5 Linear sweep voltammetry curves of the OER (cycle 10) for Mn_3−xCo_xO₄ (x = 0, 0.6, 0.9, 1.2, 1.5).

4 Conclusion

We systematically studied the OER performance of Mn_3−xCo_xO₄ (0 ≤ x < 1) nanoparticles for the first time. Nanoparticles were prepared at room temperature to minimize the influence of geometric factors. With an increase in Co content, a significant improvement in the OER activity was observed for Mn_3−xCo_xO₄ (0 ≤ x < 1). More specifically, Mn_2.1Co_0.9O₄ exhibited a high specific OER activity (1700% of Mn₃O₄ at 1.76 V vs. RHE) and long-term stability over 100 cycles. The OER activities of Mn_3−xCo_xO₄ (0 ≤ x < 1) increased linearly with the suppression of the Jahn–Teller distortion. We therefore propose that Jahn–Teller distortion plays an important role in the OER activities of compounds containing Mn³⁺. The OER performance of Mn³⁺-based compounds can be further improved by minimizing the Jahn–Teller distortion, suggesting their future application as energy saving catalysts for metal-air batteries. Work is currently ongoing in our group synthesizing Ni³⁺-based spinel oxides with suppressed Jahn–Teller distortion (using liquid phase synthesis) and exploring their OER performances.

Acknowledgements

This work was partly supported by a Grant-in-Aid for Scientific Research (B 15H04169) from the Japan Society for the Promotion of Science, the Ministry of Education, Culture, Sports, Science, and Technology of Japan.

References

1. M. Armand and J. M. Tarascon, Nature, 2008, 451, 652.
2. Y. C. Lu, Z. Xu, H. A. Gasteiger, S. Chen, K. Hamas-Schifferli and Y. Shao-Horn, J. Am. Chem. Soc., 2010, 132, 12170.
3. N. S. Lewis and D. G. Nocera, Proc. Natl. Acad. Sci. U. S. A., 2006, 103, 15729.
4. M. G. Walter, E. L. Warren, J. R. McKone, S. W. Boettcher, Q. X. Mi, E. A. Santori and N. S. Lewis, Chem. Rev., 2010, 110, 6446.
5. Y. Lee, J. Suntivich, K. J. May, E. E. Perry and Y. Shao-Horn, J. Phys. Chem. Lett., 2012, 3, 399.
6. J. Suntivich, K. J. May, H. A. Gasteiger, J. B. Goodenough and Y. Shao-Horn, Science, 2011, 334, 1383.
7. U. Maitra, B. S. Naidu, A. Govindaraj and C. N. R. Rao, Proc. Natl. Acad. Sci. U. S. A., 2013, 110, 11704.
8. Y. Zhu, W. Zhou, Z. G. Chen, Y. Chen, C. Su, M. O. Tadé and Z. Shao, Angew. Chem., Int. Ed., 2015, 54, 3897.
9. J. Kim, X. Yin, K. C. Tsao, S. Fang and H. Fang, J. Am. Chem. Soc., 2014, 136, 14646.
10. B. Han, M. Risch, Y. L. Lee, C. Ling, H. Lia and Y. Shao-Horn, Phys. Chem. Chem. Phys., 2015, 17, 22576.
11. V. Baron, J. Gutzmer, H. Rundlof and R. Tellgren, Am. Mineral., 1998, 83, 786.
12. S. Cimino, S. Colonna, S. de Rossi, M. Faticanti, L. Lisi, I. Pettiti and P. Porta, J. Catal., 2002, 205, 309.
13. X. Sun and D. Li, Adv. Mater. Res., 2014, 834–836, 458.
14. G. Z. Aminoff, Kristallografiya, 1926, 64, 475.
15. Y. Xiao, D. E. Wittmer, F. Izumi, S. Mini, T. Graber and P. Viccaro, Appl. Phys. Lett., 2004, 85, 736.
16. A. Ramírez, P. Hillebrand, D. Stellmach, M. M. May, P. Bogdanoff and S. Fiechter, J. Phys. Chem. C, 2014, 118, 14073.
17. H. Bordeneuve, C. Tenailleau, S. Guillemet-Fritsch, R. Smith, E. Suard and A. Rousset, Solid State Sci., 2010, 12, 379.
18. E. Lee, J. H. Janh and Y. U. Kwon, J. Power Sources, 2015, 273, 735.
19. L. Wang, X. Zhao, Y. Lu, M. Xu, D. Zhang, R. S. Ruoff, K. J. Stevenson and J. B. Goodenough, J. Electrochem. Soc., 2011, 158, A1379.
20. C. Li, X. Han, F. Cheng, Y. Hu, C. Chen and J. Chen, Nat. Commun., 2015, 6, 1.
21. F. Cheng, J. Shen, B. Peng, Y. Pan, Z. Tao and J. Chen, Nat. Chem., 2011, 3, 79.
22. E. Rios, J. L. Gautier, G. Poillerat and P. Chartier, Electrochim. Acta, 1998, 44, 1491.
23. A. C. Larson and R. B. von Dreele, Los Alamos National Laboratory Report LAUR, 2000, p. 86.
24. J. Suntivich, H. A. Gasteiger, N. Yabuuchi and Y. Shao-Horn, J. Electrochem. Soc., 2010, 157, B1263.
25. J. I. Jung, H. Y. Jeong, J. S. Lee, M. G. Kim and J. Cho, Angew. Chem., Int. Ed., 2014, 53, 4582.
26. J. Tafel, K. Schmitz, K. Naremann and B. Z. Emmert, Phys. Chem., 1905, 50, 641.
27. G. T. Burstein, Corros. Sci., 2005, 47, 2858.
28. (ESI†) Mn_3−xCo_xO₄ nanoparticles had BET surface areas of 60 m² g⁻¹ (x = 0), 70 m² g⁻¹ (x = 0.3), 65 m² g⁻¹ (x = 0.6), and 74 m² g⁻¹ (x = 0.9).
29. R. Tackett, G. Lawes, B. C. Melot, M. Grossman, E. S. Toberer and R. Seshadri, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 76, 024409.
30. T. Suzuki and T. Katsufuji, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 220402.
31. Y. Nii, H. Sagayama, H. Umetsu, N. Abe, K. Taniguchi and T. Arima, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87, 195115.
32. H. F. McMurdie, B. M. Sullivan and F. A. Mauer, J. Res. Natl. Bur. Stand., 1950, 45, 35.

---

Footnote

† Electronic supplementary information (ESI) available: Powder X-ray diffraction profiles, variation of lattice parameters with composition, specific OER activities, variation of overpotentials, variation of OER specific activities, and linear sweep voltammetry curves of the OER. See DOI: 10.1039/c5ra22873e

---