Evidence of oxygen vacancy-mediated ultrahigh SERS sensitivity of Niobium pentoxide nanoparticles through defect engineering: theoretical and experimental studies†
Sirsendu
Ghosal
a,
Abhilasha
Bora
b and
P. K.
Giri
 *ab
*ab
aDepartment of Physics, Indian Institute of Technology Guwahati, Guwahati 781039, India. E-mail: giri@iitg.ac.in
bCentre for Nanotechnology, Indian Institute of Technology Guwahati, Guwahati 781039, India
First published on 21st November 2023
Abstract
Oxygen vacancy engineering in metal oxide-based semiconductors has emerged as an important area of research for sensing applications, such as Surface-enhanced Raman scattering (SERS), gas sensing, etc. It has the potential to replace high-cost and unstable noble metal-based substrates in the near future. However, improving the SERS enhancement factor in semiconductor-based substrates remains a challenge. In the present study, we demonstrate that oxygen vacancy engineering in Niobium pentoxide (Nb2O5) enables ultrahigh SERS sensitivity. Oxygen vacancies were induced and manipulated in the Nb2O5 nanoparticles via a facile high-energy ball milling method and post-growth oxygen annealing. A high enhancement factor (EF) of 5.15 × 107 was obtained for the Methylene Blue (MeB) molecule on the oxygen-deficient substrate with the lowest detection limit of 10−8 M, which is 2 orders of magnitude lower than the pristine substrate. Through a careful analysis of the experimental data and theoretical calculations, we investigated the underlying mechanism behind the high EF in SERS and showed that the SERS performance is directly proportional to the oxygen vacancy concentration in the Nb2O5 nanoparticles. Density functional theory (DFT) calculation suggests a strong coupling of the vibronic states and an increased charge transfer (CT) efficiency in the Nb2O5–MeB complex mediated through the vacancy-induced trap states in the defective Nb2O5 structure. Finite element method (FEM)-based simulations revealed a field enhancement factor of ∼4.17 × 102 that contributed to the SERS EF, while the remaining is contributed to the oxygen vacancy-mediated charge transfer, i.e., a factor of ∼1.23 × 105 is due to the high CT efficiency, the highest among the reported values. We believe that these findings offer valuable insights into the fabrication of defect-tailored cost-effective semiconductor-based SERS substrates for ensuing applications, such as trace dye detection.
1. Introduction
Surface-enhanced Raman scattering (SERS) is a powerful analytical technique for investigating the chemical fingerprint of a molecule at the nanoscale. However, since its discovery, most of the research studies have been carried out with noble metallic nanostructured substrates, which enhance the Raman signal of any analyte by several thousand-fold, owing to their localized surface plasmon resonance (LSPR) effect.1,2 However, despite the enhancement, there are several bottlenecks such as the high cost of fabrication, high oxidizability, lack of selectivity, etc.,2–4 which have inadvertently led researchers to be on the constant lookout for other alternatives.5Quite recently, semiconductor-based SERS substrates have attracted the attention of researchers worldwide due to their easily tunable electronic structures, high stability, and low fabrication cost.6 However, achieving metal-like enhancement in these substrates still remains a great challenge. Defect engineering is a cost-effective strategy that has been proposed to effectively boost the performance of these SERS active semiconductor substrates.7–9 Defect engineering of materials has been consciously performed to alter the periodic arrangement of atoms, increasing the surface reactivity, promoting electron–hole charge transfer, enhancing light-trapping capabilities, etc., to directly contribute to the SERS activity of the material. However, a better insight into its mechanisms is crucial for further research in this direction.
In this regard, semiconducting oxides as SERS substrates are particularly interesting as oxygen vacancies play an important role in their electrochemical behavior. Cong et al. reported a metal-comparable SERS enhancement in WO3 by creating oxygen vacancies through H2 annealing.10 Zheng et al. reported ion irradiation as an effective method for creating oxygen vacancies for high SERS sensitivity.11 Yang et al. demonstrated a chemical reduction method for synthesizing oxygen-deficient TiO2 nanoparticles for better SERS sensitivity.12 The SERS activities of wide bandgap semiconductors like TiO2, ZnO, etc. have been studied extensively due to their superior physio-chemical properties. Compared to these studies, there are only a few reports on Niobium pentoxide (Nb2O5) on its application as a SERS sensor. In 2017, Shan et al. reported Nb2O5 nanoparticles as promising candidates for SERS application.13 However, following that study, there is hardly any report on exploiting its properties for this particular application.
Herein, high-energy ball milling was carried out on bulk Nb2O5 powder to create surface oxygen vacancy defects along with a reduction in particle size. A boosted SERS performance with an improved detection limit (10−8 M) and a very high enhancement factor of 5.15 × 107 were observed for Methylene Blue (MeB) trace dye detection. Transmission electron microscopy (TEM) and high-resolution TEM (HRTEM) images help us to determine that along with particle size reduction, a significant amount of strain is also induced due to the hard milling process. X-ray photoelectron spectroscopy (XPS) studies confirm the generation of oxygen vacancies with increased milling duration. Photoluminescence (PL) measurements also depict the generation of new defect-induced states near the conduction band edge. Moreover, it was found that oxygen vacancies play a pivotal role in increasing the SERS performance as they create new electronic energy states. These new states effectively promote the charge transfer process and are responsible for the high enhancement. Density functional theory (DFT) calculations corroborate with the generation of new electronic energy states and the increased density of states (DOS) near the conduction band edge after vacancy formation. FEM simulations were also carried out to estimate the field enhancement and to identify the contributions from the electromagnetic enhancement (EM) and chemical enhancement (CM) mechanisms individually. We believe that these findings will be essential for the development of metal-free, low-cost, robust, and biocompatible SERS substrates in the future.
2. Experimental procedure
2.1 Ball milling and the preparation of substrates for SERS measurement
Nb2O5 nanoparticles were synthesized by the dry ball mill method using zirconium balls (diameter 5 mm) with a material-to-ball weight ratio of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 20. 1000 mg of Nb2O5 powder (Sigma-Aldrich, 99.9%) was milled in a planetary ball mill (Retsch, Germany) up to 8 h at 300 rpm with the help of 25 zirconium balls (approx. 20 g) in a zirconia vial of 50 ml volume (Retsch) under dry conditions. After milling, the milled powder was mixed in DI water with the help of ultrasonication for 10 min. The sonicated mixtures were centrifuged for 9 min at 9000 rpm and the supernatant precipitate was separated. Then, the supernatant part was discarded and the precipitate was dried in a vacuum oven for 8 h at 80 °C to remove any residual moisture. The milling time was varied from 2 h to 4 h to 8 h as we investigated the effect of milling duration on their corresponding SERS enhancements. The milled samples with different milling durations are referred to in the text as NbO0, NbO2, NbO4, and NbO8 for 0, 2, 4, and 8 hours of milling, respectively.
20. 1000 mg of Nb2O5 powder (Sigma-Aldrich, 99.9%) was milled in a planetary ball mill (Retsch, Germany) up to 8 h at 300 rpm with the help of 25 zirconium balls (approx. 20 g) in a zirconia vial of 50 ml volume (Retsch) under dry conditions. After milling, the milled powder was mixed in DI water with the help of ultrasonication for 10 min. The sonicated mixtures were centrifuged for 9 min at 9000 rpm and the supernatant precipitate was separated. Then, the supernatant part was discarded and the precipitate was dried in a vacuum oven for 8 h at 80 °C to remove any residual moisture. The milling time was varied from 2 h to 4 h to 8 h as we investigated the effect of milling duration on their corresponding SERS enhancements. The milled samples with different milling durations are referred to in the text as NbO0, NbO2, NbO4, and NbO8 for 0, 2, 4, and 8 hours of milling, respectively.
For SERS substrate preparation, the samples were prepared in the following way. First, 10 mg of the ball-milled sample was dispersed in 10 ml of DI water and ultrasonicated for 15 min. Then Si wafers of size 1 cm × 1 cm were cleaned with acetone, propanol, and DI water, respectively. Then, 10 μL of the sonicated mixture was drop-cast on the cleaned Si wafers and dried for several minutes. Subsequently, 5 μL of Methylene Blue (MeB) dye solution of different concentrations was drop-cast on the as-prepared substrates and dried for several hours. Then, SERS measurements were performed in a micro-Raman spectrometer. For the annealing of the samples, we have used a single-zone tube furnace with a rotary pump connected to one end of it. The annealing temperatures were kept at 300, 500, and 700 °C, respectively. Oxygen gas was sent through a mass flow controller at a flow rate of 15 sccm for 1 h.
2.2 Characterization techniques
The morphology, particle size, crystallinity, and lattice spacing of the milled samples were investigated using a field emission transmission electron microscope (FETEM) (JEOL 2100F operated at 200 kV). The phase and crystal structure of the samples were recorded using an X-ray diffractometer (Rigaku RINT 2500 TRAX-III, Cu Kα radiation). The morphology and distribution of the nanoparticles were characterized using a field emission scanning electron microscope (FESEM) (JEOL JSM-7610F). X-ray photoelectron spectroscopy (XPS) (Ulvac-Phi, Inc.) operated with an Al Kα X-ray beam (1486.6 eV) at 20 kV, 84 W was employed for analyzing the elemental composition of the milled powders. XPS peak fit 4.1 was used for fitting the high-resolution XPS spectra. UV-visible diffused reflectance spectroscopy (DRS) spectra were recorded with a commercial spectrometer (PerkinElmer, Lambda950). For room temperature photoluminescence (PL) measurements of the milled powders, a commercial fluorimeter (Horiba Jobin Yvon, Fluoromax-4) was used. All the Raman and SERS experiments were carried out with a high-resolution micro-Raman spectrometer (LabRam HR800, Jobin Yvon) using 532 and 633 nm laser excitation sources. SERS measurements were performed using a 100× objective lens with a laser power of 1.25 mW for all samples to avoid laser burning of the samples, and the acquisition time was kept 10 s and an 1800 grating per mm was used for all the measurements.2.3 Computational details
Electronic band structure and density of states (DOS) calculations were performed in a DFT framework using a plane wave basis set using Quantum Espresso software.14–16 In our calculations, we used the HSE06 (Heyd–Scuseria–Ernzerhof) screened hybrid functional for the band structure and DOS calculations for both the pristine and vacancy-induced defective Nb2O5 systems. Hybrid functionals generally give more accurate results than the standard functionals, which leads to a good estimation of the bandgap close to the experimental one.17 The plane wave cut-off energy was kept at 60 Ry, and a Monkhorst–Pack k-point sampling of ∼8 × 8 × 8 was used to sample the Brillouin zone. A force convergence cut off of 0.01 eV Å−1 was set and the energy convergence threshold was kept 1 × 10−9 for accuracy.For the molecular adsorption on the Nb2O5 surface, a slab geometry was considered in the (001) direction and a vacuum level of 20 Å was added in the z direction to minimize the interaction with the neighbouring atoms. The charge density difference isosurface plot was generated using VESTA software according to the following formula:
| Δρcdd = ρNb2O5/MeB − ρNb2O5 − ρMeB | (1) |
Electromagnetic FEM simulations were performed in a CST microwave studio design environment. A plane wave source of 532 nm wavelength was made incident on the nanoparticle along the negative Z direction, and PML (perfectly matched layer) boundary conditions were used along the Z direction. The boundary conditions for magnetic and electric fields were taken along the x and y directions, respectively. The dispersive optical characteristics of Nb2O5 were taken from the work of Gao et al.18 A tetrahedral meshing was employed to carry out the simulations.
3. Results and discussion
3.1 Morphology studies
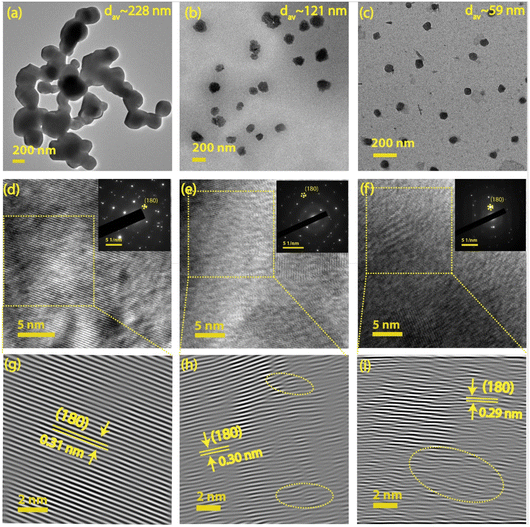
TEM and HRTEM techniques were utilized to evaluate the particle size distribution, morphology, d-spacing, and strain induced in the system. Fig. 1(a–c) illustrates the morphology and size distribution of Nb2O5 nanoparticles for different milling durations, 0, 4, and 8 h, respectively. Fig. 1(d–f) displays the corresponding HRTEM images of the samples along with their corresponding selected area electron diffraction (SAED) patterns (as inset). A magnified view of the inverse fast Fourier transform (IFFT) pattern (as shown in Fig. 1(g–i)) provides a direct visualization of the strain developed in the system after each milling stage. The strained region is indicated by an oval-shaped ring for the NbO4 and NbO8 samples. The pristine sample (NbO0) exhibits clear lattice fringes without any visible distortion, indicating no apparent strain in the system. The calculated d-spacing is approximately 0.31 nm, which corresponds to the (180) plane of the orthorhombic Nb2O5 structure.19 In comparison, the NbO8 sample lattice shows significant distortion and numerous dislocations in the structure. The d-spacing in the strained region is approximately 0.29 nm, suggesting a compressive nature of the strain. Fig. 1(h) reveals a mild distortion in the lattice fringes after 4 hours of milling, showing a gradual increase of strain with an increase in the duration of milling. The SAED pattern also shows a transition from bright, well-defined crystal fringes to a nearly amorphous character after 8 hours of milling. The average particle size of the unmilled sample is ∼228 nm, which reduces to ∼59 nm (Fig. S1(a–c), ESI†) after 8 h of milling, showing a gradual reduction of particle size with increased milling duration. The zeta potential measurements on all these samples revealed that with the reduction of particle sizes, the zeta potential value systematically decreases from −20.6 mV (in NbO0) to −34.6 mV (in NbO8) (Fig. S1(d), ESI†). This high zeta potential value in small-size particles indicates higher colloidal stability or less agglomeration. Furthermore, the samples were ultrasonicated for extended durations to separate out the agglomeration before taking TEM measurements, although the aggregation of particles plays a crucial role in the observed SERS enhancement, which is discussed later. The highly distorted structure may result in intriguing features in terms of band structure and optical properties, which are discussed later. Moreover, the reduced particle size and the presence of defects/dislocations in the milled sample enhance adsorption capabilities for dye molecules, making it beneficial for SERS applications. Previous studies by Gomes et al. have reported that vacancies, such as oxygen vacancies or cation substitutions, can lead to similar distortions and high strain values.20 The evolution of an amorphous structure in the sample from a high crystalline phase greatly helps in boosting its SERS performance by creating more adsorption sites and providing more charge transfer pathways through the formation of defect-induced energy levels in the forbidden energy region. Our results are consistent with the previous reports based on other oxide materials.21,223.2 XRD and Raman analysis
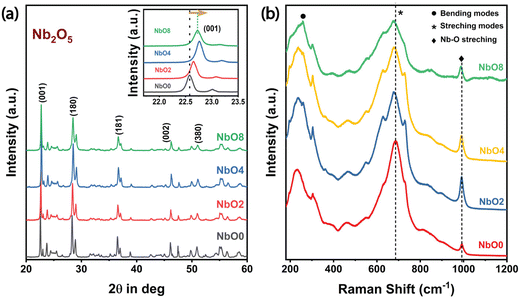
The XRD patterns of the milled samples are depicted in Fig. 2(a). As shown in Fig. 2(a), all of the samples exhibit the T-phase orthorhombic structure of Nb2O5 as all the peaks closely match with the JCPDS card # 27-1003,23 and even after 8 hours of milling, no significant phase change is observed in the material. However, the intensity of the peaks gradually decreases with increasing milling time, indicating a reduction in the crystalline nature of the material during the milling process. Furthermore, a noticeable shift in the peak position towards higher 2θ values is observed (inset of Fig. 2(a)), suggesting the development of compressive strain within the system.24 Concurrently, the full width at half maximum (FWHM) of the peaks gradually increases with longer milling durations, indicating a reduction in the crystallite size and a subsequent loss of crystallinity. These observations are consistent with our TEM analysis. By analyzing the peak position and FWHM data for the (001) plane, the average crystallite size can be estimated. The calculated values are listed in Table T1 (ESI†), showing the evolution of crystallite size during the milling process. To determine the average size of the crystallites and the strain, we employed the Williamson–Hall (W–H) plot utilizing the following equation: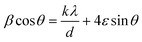 | (2) |
 . By incorporating the strain energy density into the W–H equation, a modified version of the equation is obtained, which takes into account strain anisotropy and provides a more accurate estimation of crystallite size and strain values.
. By incorporating the strain energy density into the W–H equation, a modified version of the equation is obtained, which takes into account strain anisotropy and provides a more accurate estimation of crystallite size and strain values. | (3) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos
cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ vs. 4
θ vs. 4![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin
sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ in the UDM method, now βhkl
θ in the UDM method, now βhkl![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos
cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ is plotted against
θ is plotted against  in the UDEDM method. The lattice strain can be calculated by knowing the Yhkl values of the sample. From the literature,25 the formula for calculating Young's modulus for orthorhombic systems is given as
in the UDEDM method. The lattice strain can be calculated by knowing the Yhkl values of the sample. From the literature,25 the formula for calculating Young's modulus for orthorhombic systems is given as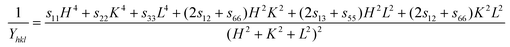 | (4) |
 , and
, and  where a, b, and c are the lattice constants of the orthorhombic crystals and h, k, and l are the Miller indices for each plane. The above parameters have been taken from the JCPDS file (#27-1003), which closely matches with our XRD pattern. In Table T1 (ESI†) we have listed the crystallite size and compared that to what we obtained from the UDM model. From Fig. S2(a) (ESI†), we observed that the crystallite size of the material has reduced from ∼102 nm to ∼52 nm (in the UDEDM model) with increasing milling duration, while the lattice strain did not follow the same trend due to the formation of dislocations in the crystals, leading to strain relaxation. This relaxation in the compressive strain due to the formation of dislocations explains about the observed peak shift of NbO8 in the opposite direction compared to the other samples.26
where a, b, and c are the lattice constants of the orthorhombic crystals and h, k, and l are the Miller indices for each plane. The above parameters have been taken from the JCPDS file (#27-1003), which closely matches with our XRD pattern. In Table T1 (ESI†) we have listed the crystallite size and compared that to what we obtained from the UDM model. From Fig. S2(a) (ESI†), we observed that the crystallite size of the material has reduced from ∼102 nm to ∼52 nm (in the UDEDM model) with increasing milling duration, while the lattice strain did not follow the same trend due to the formation of dislocations in the crystals, leading to strain relaxation. This relaxation in the compressive strain due to the formation of dislocations explains about the observed peak shift of NbO8 in the opposite direction compared to the other samples.26
The Raman spectrum of a material exhibits exceptional sensitivity to subtle changes in the bond order. In the case of the T-Nb2O5 orthorhombic structure, three main Raman modes are observed. The peaks within the range of 200 cm−1–400 cm−1 correspond to the bending modes of Nb–O–Nb linkages (Fig. 2(b)), while a broader peak spanning from 400 cm−1 to 900 cm−1 appears from the symmetric and antisymmetric stretching modes of the NbO6 polyhedra.27 Additionally, a small peak at ∼995 cm−1 is attributed to the stretching modes of the Nb![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O surface groups. The broad Raman bands in Nb2O5, despite being crystalline, can be ascribed to longer bond orders present in the orthorhombic phase. As the milling time increases, notable changes occur in the Raman spectra. The intensities of all Raman bands decrease gradually, and there is a progressive redshift of all the Raman bands, which corresponds to the stretching modes in Nb2O5. This redshift occurs due to the compressive strain generated with prolonged milling time, leading to a more disordered structure. A similar trend was also observed by Joya et al.,27 where gradually increasing the pressure on the samples resulted in reduced peak intensities and a shift towards lower wavenumbers. The peak shift to lower wavenumbers indicates a more amorphous nature of the samples.28 These findings are further supported by HRTEM images and the corresponding SAED pattern. After 8 h of milling, a 10 cm−1 shift towards the lower wavenumber is observed in the Raman band centered at 686 cm−1. Several factors could contribute to this shift, including phonon localization by defects, strain-induced shifting of phonon modes, etc. However, accurately discerning the individual contributions of these mechanisms requires more extensive investigations and complementary characterization studies.29
O surface groups. The broad Raman bands in Nb2O5, despite being crystalline, can be ascribed to longer bond orders present in the orthorhombic phase. As the milling time increases, notable changes occur in the Raman spectra. The intensities of all Raman bands decrease gradually, and there is a progressive redshift of all the Raman bands, which corresponds to the stretching modes in Nb2O5. This redshift occurs due to the compressive strain generated with prolonged milling time, leading to a more disordered structure. A similar trend was also observed by Joya et al.,27 where gradually increasing the pressure on the samples resulted in reduced peak intensities and a shift towards lower wavenumbers. The peak shift to lower wavenumbers indicates a more amorphous nature of the samples.28 These findings are further supported by HRTEM images and the corresponding SAED pattern. After 8 h of milling, a 10 cm−1 shift towards the lower wavenumber is observed in the Raman band centered at 686 cm−1. Several factors could contribute to this shift, including phonon localization by defects, strain-induced shifting of phonon modes, etc. However, accurately discerning the individual contributions of these mechanisms requires more extensive investigations and complementary characterization studies.29
Both the Raman and XRD analyses show a development of compressive strain with prolonged milling duration and a significant reduction of crystallinity, indicating a reduction of its crystal lattice order. The observed trends align with HRTEM images and SAED patterns.
3.3 XPS analysis
XPS is a highly surface-sensitive technique used to analyze surface defects and the chemical composition of materials. In Fig. 3(a–c) and (d–f), we presented the high-resolution XPS spectra of the Nb 3d and O 1s oxidation states, respectively, for different samples. Deconvolution of the O 1s spectra using Gaussian fitting revealed two peaks: one at ∼530.1 eV corresponding to lattice oxygen (OL) and a broader peak at ∼532.0 eV associated with the presence of oxygen vacancies (OV).30 The results indicate that the contribution from oxygen vacancies significantly increases with increasing milling time, and is the highest after 8 h of milling. The ratio of the integrated area of oxygen vacancies to lattice oxygen (OV/OL) increases from 0.21 to 0.64, indicating a significant increase in the oxygen vacancy concentration after 8 h of milling (NbO8). In Fig. 3(a–c), the Nb 3d levels were plotted, showing two distinct sharp peaks corresponding to the Nb5+ state in all cases. The peak at 207.3 eV represents the Nb 3d5/2 level, while the peak at 210.0 eV corresponds to the Nb 3d3/2 level.31 Although no significant changes were observed in the Nb 3d peaks, the FWHM of the peaks slightly increased after milling. This broadening may be attributed to the reduction of Nb5+ states and the generation of Nb4+ states due to the formation of oxygen vacancies.20 The deconvoluted spectra revealed additional peaks at 208.3 eV and 205.8 eV, which are associated with Nb4+ states. As oxygen vacancies are formed within the nanoparticles, Nb5+ ions in close proximity to the vacancies acquire an electron from the oxygen vacancy (OV), leading to a transformation into Nb4+ states. From the XPS peak fit analysis, the calculated Nb![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) O ratio in NbO0 is 1
O ratio in NbO0 is 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2.42, whereas for NbO4 it is 1
2.42, whereas for NbO4 it is 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2.01, and for NbO8 it is 1
2.01, and for NbO8 it is 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1.46. The XPS findings clearly indicate an increase in the oxygen vacancy concentration with higher milling time, which may be due to the rapid rise in temperature during the milling process, causing a partial loss of lattice oxygen, as also seen with other transition metal oxides (TMOs).32,33
1.46. The XPS findings clearly indicate an increase in the oxygen vacancy concentration with higher milling time, which may be due to the rapid rise in temperature during the milling process, causing a partial loss of lattice oxygen, as also seen with other transition metal oxides (TMOs).32,33
3.4 Optical characterization
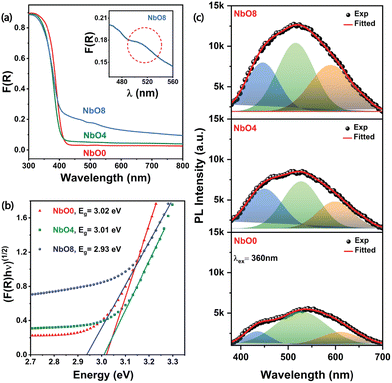
In order to delve into the optical properties and changes in the electronic band structure of the material, we performed UV-visible diffuse reflectance spectroscopy (UV-DRS), which provides a quantitative measurement of the material's absorbance. The bandgap of the material was estimated using the Kubelka–Munk function, and the F(R) values of the milled powders with respect to the wavelength are presented in Fig. 4(a). Notable changes were observed in the DRS spectra with increasing milling time. The sharp band edge becomes diffused and red-shifted, and there is a significant increase in absorption intensity throughout the visible region. In particular, a distinct absorption band is observed at around 510 nm (inset of Fig. 4(a)), after 8 hours of milling, indicating the presence of trap states generated due to the presence of oxygen vacancies. These findings are consistent with similar observations made for other oxide materials, which also exhibit similar trends.26,29 Furthermore, the Tauc plot, constructed using the Kubelka–Munk function for an indirect bandgap semiconductor (Fig. 4(b)), revealed an apparent decrease in the bandgap of the material after milling. The optical band gap of NbO0 estimated from the Tauc plot is 3.02 eV, while the bandgap value of NbO8 has decreased to 2.93 eV (Fig. 4(b)). DFT calculations of the oxygen-deficient Nb2O5 structure also reveal that the generation of shallow trap states associated with Nb4+ states near the conduction band edge can lead to a bandgap narrowing in Nb2O5. Similar observations were made with other oxides too. Wang et al. reported that oxygen vacancy-induced miniband generation near the conduction band minima leads to a bandgap narrowing in ZnO.34 Ücker et al. reported an apparent decrease in the bandgap of Nb2O5 associated with oxygen vacancies induced by heat treatment.35 Overall, these observations from the DRS spectra provide valuable insights into the optical properties of Nb2O5 NPs and changes in their electronic band structure. These results are consistent with the findings from the XPS data, further validating our interpretations of the data.PL emission spectra provide valuable insights into the nature of defects and their impact on the electronic band structure of the material (Fig. 4(c)). As the milling duration was increased, a significant enhancement in the visible PL intensity was observed compared to the pristine sample. Notably, the intensity of the PL peak of sample NbO8 is almost two times higher than that of NbO0. This enhancement can be attributed to the creation of trap states near the conduction band edge due to oxygen deficiency in the sample.36 Since orthorhombic Nb2O5 is an indirect bandgap material, no emission associated with the band edge is expected. Therefore, the observed PL spectra mainly originate from near band-edge emission (NBE) and emissions resulting from the defects present in the sample. To better understand the underlying mechanisms, we deconvoluted each PL spectrum into three Gaussian peaks. The first peak located in the UV region at around 435 nm is attributed to the NBE, primarily associated with the recombination of photo-excited electrons with oxygen vacancies to form excitons.27,37 The second peak, the green emission band at ∼520 nm, is related to defect-induced emission caused by distortions in the NbO6 octahedra, which are also associated with possible oxygen vacancies.27 The third peak in the orange-red emission region at ∼600 nm could be attributed to the F+ centre. It is established that oxygen vacancies can exist in different forms, including neutral, singly ionized, and doubly ionized states, represented as F centres (vacancy occupied by two electrons), F+ centres (vacancy occupied by a single electron), and F2, F2+ centers.38 According to the accepted theory, Nb5+ ions will accept one of the electrons from an F centre, transitioning to the Nb4+ state and leaving behind an F+ centre.20,36
| OV + 2e− → F | (5) |
| F + Nb5+ → F+ + Nb4+ | (6) |
| V0 + e− → F+ | (7) |
| Nb5+ + e− → Nb4+ | (8) |
In addition, defects in the material lead to the generation of intermediate trap states within the forbidden energy gap. These trap states, populated by Nb4+ species, exhibit energy absorption in the lower visible spectral region, which is consistent with our observations from the DRS spectra.
The formation of F+ centres and F centres, along with the transition to lower valence states, has (Nb5+ to Nb4+) also been observed in other oxide materials.36,38 This phenomenon arises mainly due to the presence of defects, such as oxygen vacancies, which introduce localized electronic states within the bandgap. These vacancy-induced intermediate trapped states play a crucial role in absorbing energy in the visible spectral region, contributing to the change in the electronic band structure and the unique PL emission characteristics.
4. SERS performance of the defective Nb2O5 substrate
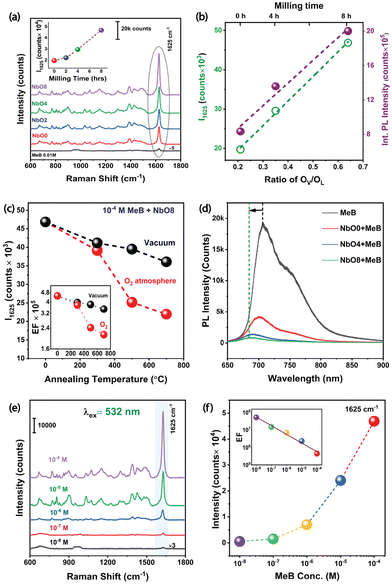
The surface of Nb2O5 is known to possess abundant adsorption sites, comprising intricate Lewis sites consisting of Nb5+ ions, as well as charged Brønsted sites.13 These sites have the potential to establish robust interactions with dye molecules that bear nucleophilic groups or exhibit opposite charges. The successful adsorption of analyte molecules onto the Nb2O5 surface is anticipated to induce modifications in the electron density distribution of these molecules, consequently leading to an amplified Raman scattering cross-section. Methylene Blue (MeB) is a highly useful well-known organic dye used by both chemists and biologists. As a dye, it has its uses in histological and bacteriologic straining due to its distinguishable blue colour. Moreover, it is widely used in the medical sector as a therapeutic drug to treat urinary tract infections, malarial infections, methemoglobinemia, etc. It is also found to inhibit the expression of heat shock response genes in cancer cells.39 Therefore, effective SERS detection of methylene blue dye through more biocompatible substrates is of utmost importance. SERS spectra of 10−4 M of MeB on different substrates are displayed in Fig. 5(a). All the characteristic Raman modes of MeB at 949, 1075, 1297, 1390, and 1625 cm−1 were found in the SERS spectra (Table T2, ESI†).40 Among these peaks, the most intense peak at 1625 cm−1 corresponding to the C–C ring stretching vibration is considered for comparison of the SERS performance on different milled and annealed substrates. In Fig. 5(a), it is observed that the intensity of the Raman modes gradually increases with increasing milling duration. The peak intensity of the 1625 cm−1 mode has increased from 19k counts in the NbO0 sample to almost 46k in the NbO8 sample (inset of Fig. 5(a)) for the same concentration of MeB, which accounts for about ∼2.5-times enhancement. XPS analysis of our samples revealed that the broad peak at 532 eV related to oxygen deficiency is increased substantially in NbO8 compared to NbO0, and a similar trend was also observed in the PL spectra. So, the improved SERS performance is directly correlated with the oxygen vacancy concentration in the sample. In Fig. 5(b), we presented a variation in the intensity of the 1625 cm−1 peak of MeB (10−4 M) with the oxygen vacancy concentration (intensity ratio of OV/OL as obtained from XPS), as well as its variation with the integrated PL intensity, which is essentially proportional to the OV concentration in the Nb2O5 nanoparticles. Interestingly, it demonstrates a linear relationship between the OV concentration and the SERS intensity, suggesting a direct correlation between the SERS activity and oxygen vacancies in the Nb2O5 nanoparticles. To further confirm the role of OV, the NbO8 sample was annealed at 300, 500, and 700 °C under vacuum and an O2 atmosphere, respectively. Interestingly, the oxygen-annealed NbO8 sample showed a decreasing order of SERS performance as the annealing temperature was increased to 700 °C, with the intensity decreased to 22k counts from 46k (Fig. S3(a), ESI†). This can be attributed to a reduction in the oxygen vacancy concentration by oxygen substitution in the sample and increased crystallinity, leading to a less effective adsorption of the molecules. In contrast, with vacuum annealing, the reduction of peak intensity is significantly less compared to the oxygen annealing case (Fig. S3(b), ESI†). This is understood as in the oxygen-deficient environment, the vacancy states remain intact, whereas a little reduction of intensity is due to high crystallinity and less adsorption sites due to the high temperature of annealing (Fig. 5(c)). From energy dispersive X-ray spectroscopy (EDS), the atomic percentage of each of the elements is shown in Fig. S4 (ESI†). As seen in the figure, the atomic percentage of oxygen in NbO8 is 59.4%, which increases to 70.5% in the oxygen-annealed sample, whereas with vacuum annealing, it is 63.7%, the corresponding EFs are listed in Table T5 (ESI†). Experimental evidence of charge transfer in the composite system is inferred from the quenching of PL spectra of MeB dye on different milled substrates (Fig. 5(d)). As shown in Fig. 5(d), the PL emission spectra of MeB with the Nb2O5 substrate is significantly quenched as compared to its emission on a Si substrate. The quenching effect is more prominent as we go from NbO0 to NbO8 in the composite system and with a gradual blue shift in the peak position. This behavior is indicative of a pronounced charge transfer from the MeB molecule to the Nb2O5 substrate in case of the defective system.41 A similar observation was made with the UV-visible absorption spectra too (Fig. S5, ESI†). First, the absorption spectra were taken for MeB 10−5 M aqueous solution, then the MeB molecules were adsorbed on the surface of NbO0, NbO4 and NbO8 samples, respectively, and the corresponding spectra were taken. It was found that the absorption spectra were broadened and blue-shifted systematically from NbO0 to NbO8, suggesting a strong interaction and charge transfer in the MeB–Nb2O5 composite. Moreover, the broadened absorbance peak is close to our excitation wavelength (532 nm) of SERS, facilitating a quasi-CT resonance that contributes to the increased SERS activity in the milled samples. We carried out concentration-dependent SERS experiments with the best-performing sample, NbO8. With this substrate, consisting of a higher defect concentration, we could detect the Raman signal of MeB down to a concentration as low as 10−8 M. As the Raman signal is low at this concentration, we multiplied the intensity with a factor 5 in order to enable comparison, and the broad peak arising at 686 cm−1 here is due to Nb2O5 itself (Fig. 5(e)). The expression for calculating the SERS EF is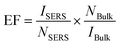 | (9) |
5. Origin of the high enhancement factor
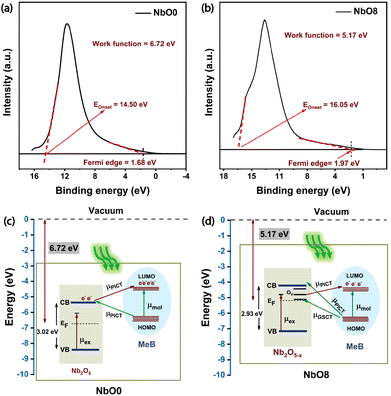
The underlying mechanism of SERS enhancement is still a topic of ongoing debate. However, two widely accepted theories are the electromagnetic (EM) and the chemical enhancement (CM) mechanisms.42 EM enhancement is primarily associated with the generation of a strong local electric field in the vicinity of nanostructures when excited with an appropriate excitation wavelength corresponding to the resonant plasmon frequency. This effect is particularly pronounced in metallic nanostructures where there is a surplus of conduction electrons that oscillate coherently with the incident light and contribute to the enhanced electric field,43 whereas in semiconductor-based systems, the CM mechanism is considered to be more dominant. Chemical enhancement revolves around the concept of photoinduced charge transfer (PICT) resonance between the energy levels of the semiconductor material and the chemisorbed molecule.44 The interaction between the semiconductor and the molecule leads to the modulation of electronic states, resulting in enhanced Raman scattering signals. However, it is important to note that the exact contribution and interplay between these two mechanisms in SERS enhancement are still subjects of ongoing research. Previous studies on Nb2O5 have shown that both EM and CM contribute to the SERS enhancement.13,22 To gain a deeper understanding of these contributions, finite element method (FEM) simulations using the CST microwave studio environment were conducted on the nanoparticles, which will be discussed later.First, we performed ultraviolet photoelectron spectroscopy (UPS) measurements to understand the charge transfer mechanism in the Nb2O5–MeB complex and to find out the position of the valence band edge with respect to the vacuum level in our system. We calculated the work function (φ) using the formula, (φ = hν − EOnset), where hν is the source energy (He I, 21.2 eV) and EOnset is the secondary electron cut-off energy, which is determined by drawing a tangent at higher binding energy. The separation between the Fermi level and the valence band maxima was calculated by extrapolating the linear portion of the spectrum to the energy axis in the low binding energy edge. By analysing the UPS spectra of NbO0 and NbO8 (as shown in Fig. 6(a) and (b)), we calculated the work function (φ) and the valence band edge (VB), and from the bandgap obtained from the DRS spectra, we calculated conduction band (CB) edge positions for both pristine and 8 h milled samples. The CB and VB positions were found to be −5.38 eV and −8.4 eV, respectively, for pristine Nb2O5 and −4.21 eV and −7.14 eV, respectively for the 8 h milled Nb2O5. From the literature, the HOMO and LUMO levels of the MeB molecule were found to be −6.26 eV and −4.55 eV, respectively.13 Defect levels in the NbO8 sample were derived from the PL emission data.
Furthermore, to account for the charge transfer in the Nb2O5–MeB complex, the Herzberg–Teller theory based on the Franck–Condon principle was employed. This, in turn, helped in understanding the coupling between the electronic and the vibronic states of the dye molecule and the semiconductor substrate and its influences on the overall Raman polarizability tensor, thus amplifying the Raman signal of MeB. From the obtained energy band diagram in Fig. 6(c and d), there are several thermodynamically feasible charge transfer pathways possible under excitation with a 532 nm laser, including molecular resonance (μmol) of MeB, exciton resonance of Nb2O5 defect sites, and also the photon-induced charge transfer resonance (μPICT), together with the ground state charge transfer resonance (μGSCT), from the matched energy level between Nb2O5 and the MeB molecule.45 All these can potentially change the Raman polarizability tensor of the molecule and can lead to a significant enhancement of the Raman signal of the molecule. Now, by comparing the CT pathways in Fig. 6(c) and (d), it is observed that there are more feasible CT pathways generated in the defective system (NbO8) due to the generation of several defect energy states in the forbidden energy gap. Electrons from the CB and vacancy-induced trap states (OV) of Nb2O5 can better channel through to the HOMO of the molecule due to the matching of the energy levels, and also the laser excitation energy (2.33 eV) is sufficient for promoting the ground state electrons to the higher conducting energy states (OV) thus increasing the charge carrier population in the conducting states. In contrast, experiments performed with a 633 nm laser did not show much enhancement due to insufficient energy to promote the electrons from the VB of the semiconductor to higher excited states and also due to the presence of fluorescence emission of MeB near that wavelength (Fig. S6(a), ESI†). So, under excitation with a 633 nm laser, only up to 10−6 M concentration of MeB was detected. The concentration-dependent SERS spectra with the NbO0 (pristine) substrate are also depicted in Fig. S6(b) (ESI†). From the work of Lombardi and Wang,46,47 the intensity of a Raman transition is related to the molecular polarizability tensor αρσ with the following expression:
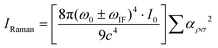 | (10) |
| αρσ = A + B + C | (11) |
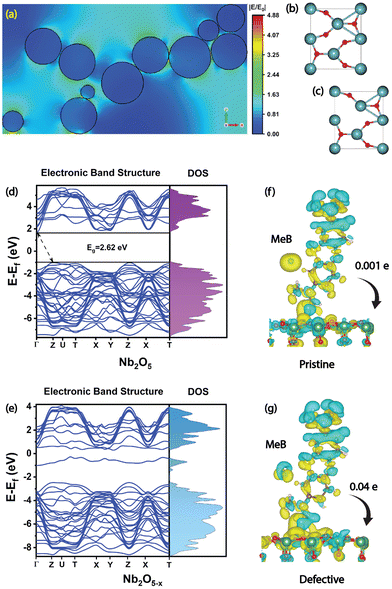
Next, we have conducted FEM simulations to find out the EM contribution of the Nb2O5 nanoparticles. To replicate the observed agglomeration pattern and morphology, large-area FESEM images of all the SERS substrates were taken and are shown in Fig. S8(a–c) (ESI†) and based on the observed morphology and the aggregation pattern (Fig. S8(d), ESI†), we modelled our simulation environment. The results demonstrate that the maximum electric field enhancement occurred in the agglomerated regions of the sample, and multiple hotspots were generated in the sub-nanometer gap between the nanoparticles, as shown in Fig. 7(a). The excitation wavelength used for the simulations was 532 nm, and a maximum |E/E0| value obtained was 4.52 in one of these hotspots. Since the EM enhancement factor is proportional to |E|4, the EM mechanism alone accounted for a factor of 4.17 × 102 enhancement in the SERS signal. The remaining EF is expected to be due to the chemical enhancement (CM). In this case, it comes out to be ∼105 for the CM mechanism, which is expressed by the charge transfer through multiple pathways.
DFT calculations were performed to interpret our experimental results and to acquire an in-depth understanding of the changes in the electronic band structure in the defective system. The unit cell structures of pristine and oxygen-deficient Nb2O5 are depicted in Fig. 7(b and c). The simulated band structure of orthorhombic Nb2O5 exhibits an indirect bandgap of 2.62 eV, which is close to our experimentally measured bandgap (∼3.02 eV) since DFT functionals generally underestimate the bandgap (Fig. 7(d)). In the defective system, oxygen vacancies (10%) were created by removing the oxygen atoms randomly from several locations. Both the density of states (DOS) and the band structure reveal the generation of new energy states near the conduction band edge (Fig. 7(e)) and also a reduction in the bandgap to 1.82 eV. These results match pretty well with our experimental observations.
Next, the charge density difference (CDD), along with Bader charge analysis, was conducted to elucidate the interfacial CT process in the adsorbed system between the molecules and the SERS substrate. The isosurface plot reveals a net charge transfer from the MeB molecule to the Nb2O5 substrate in both the pristine and defective systems (Fig. 7(f) and (g)). Although a stronger interaction is observed in the defective system as there is significant amount of charge accumulation near the interface, Bader charge analysis reveals a net amount of 0.04 times the electronic charge (e) per molecule was depleted from MeB and was gained by the defective Nb2O5 substrate, which enhanced the SERS signal dramatically. In contrast, for a pristine system, a comparatively less charge (0.001e) was transferred in the process. According to the Fermi golden rule, the transition probability is defined by
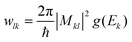 | (12) |
Although it is quite challenging to separate out the contributions of each mechanism, with the help of computation, we attempted to quantitatively separate out the contributions from the calculated enhancement factor. The details are given in Table 1. Here, with the NbO0 sample, i.e., with the pristine material, we have obtained an EF value of 1.86 × 106, and from the FEM simulation, we obtained an EF value of 4.17 × 102 for EM enhancement. So, we expect the remaining contribution of 4.46 × 103 is due to CM enhancement in the pristine sample. Interestingly, with the NbO8 sample, the EF factor has increased to 5.15 × 107, so a factor of 27.6 is increased for the milled sample due to the higher defect concentration. This extra factor can be attributed to the CM enhancement due to the additional vacancies. So, a 27-fold additional enhancement was found with the more defective sample. Note that from Bader charge analysis, we noticed a 40 times higher charge transfer taking place from MeB to Nb2O5−x when the vacancy percentage was just 10%, as also seen with the charge density difference plot. So, here we have a fairly good agreement with the experimentally obtained results. The observed discrepancy is due to the presence of OV defects in the pristine samples, which was not considered for the calculation.
| Enhancement factor (EF) | ||||
|---|---|---|---|---|
| NbO0 | NbO8 | |||
| 1.86 × 106 | 5.15 × 107 | |||
| EM | CM | EM | CM | |
| 4.17 × 102 | 4.46 × 103 | 4.17 × 102 | 1.23 × 105 | |
| Band alignment | Oxygen vacancies | |||
| 4.46 × 103 | 2.76 × 101 | |||
6. Conclusion
We demonstrated a low-cost ball milling technique to fabricate an efficient plasmon-free SERS substrate with Nb2O5 nanoparticles containing abundant oxygen vacancies. The defective Nb2O5 nanoparticles exhibited a high SERS enhancement towards detecting the MeB dye molecule with an improved detection limit of 10−8 M and an enhancement factor reaching as high as 5.15 × 107. Through XPS and PL measurements, we have shown that the oxygen vacancy percentage can be modulated by varying the duration of milling and post-growth annealing. The oxygen vacancies generated in the sample played a crucial role in amplifying the SERS signal by strengthening the charge transfer process at the semiconductor/dye interface. Systematic investigations through different characterization methods and annealing experiments revealed that SERS enhancement is directly proportional to the oxygen vacancy concentration in the Nb2O5 nanoparticles, and charge transfer through these defects primarily contributes to the high EF. The individual contributions from field enhancement and charge transfer mechanisms were identified through different simulations and experiments. Electromagnetic FEM simulations revealed a factor of ∼102 enhancement arising from the field enhancement, and the CM mechanism contributed a factor of >105 in the SERS enhancement, which is fully consistent with the DFT calculation results that additional energy states in the forbidden energy gap provide multiple pathways for charge transfer. We believe that this work will have a significant impact on the future development of cost-effective, plasmon-free, and high-performing SERS substrates.Conflicts of interest
There are no conflicts to declare.Acknowledgements
We acknowledge the financial support from MEITY (Grant No. 5(1)/2022-NANO) and SERB (Grant No. CRG/2021/006397) for carrying out part of this work. The Central Instruments Facility, I.I.T. Guwahati, is acknowledged for providing the XRD, XPS, and TEM facilities. We also thank Prof. Gagan Kumar of IIT Guwahati for his help with the FEM simulations.References
- J. Langer, D. J. de Aberasturi, J. Aizpurua, R. A. Alvarez-Puebla, B. Auguié, J. J. Baumberg, G. C. Bazan, S. E. J. Bell, A. Boisen, A. G. Brolo, J. Choo, D. Cialla-May, V. Deckert, L. Fabris, K. Faulds, F. Javier García de Abajo, R. Goodacre, D. Graham, A. J. Haes, C. L. Haynes, C. Huck, T. Itoh, M. Käll, J. Kneipp, N. A. Kotov, H. Kuang, E. C. Le Ru, H. K. Lee, J. F. Li, X. Y. Ling, S. A. Maier, T. Mayerhöfer, M. Moskovits, K. Murakoshi, J. M. Nam, S. Nie, Y. Ozaki, I. Pastoriza-Santos, J. Perez-Juste, J. Popp, A. Pucci, S. Reich, B. Ren, G. C. Schatz, T. Shegai, S. Schlücker, L. L. Tay, K. George Thomas, Z. Q. Tian, R. P. van Duyne, T. Vo-Dinh, Y. Wang, K. A. Willets, C. Xu, H. Xu, Y. Xu, Y. S. Yamamoto, B. Zhao and L. M. Liz-Marzán, Present and Future of Surface-Enhanced Raman Scattering, ACS Nano, 2020, 14(1), 28–117 CrossRef CAS.
- M. Fleischmann, P. J. Hendra and A. J. McQuillan, Raman Spectra of Pyridine Adsorbed at a Silver Electrode, Chem. Phys. Lett., 1974, 26(2), 163–166, DOI:10.1016/0009-2614(74)85388-1.
- X. Wang, E. Zhang, H. Shi, Y. Tao and X. Ren, Semiconductor-Based Surface Enhanced Raman Scattering (SERS): From Active Materials to Performance Improvement, Analyst, 2022, 147(7), 1257–1272 RSC.
- B. Yang, S. Jin, S. Guo, Y. Park, L. Chen, B. Zhao and Y. M. Jung, Recent Development of SERS Technology: Semiconductor-Based Study, ACS Omega, 2019, 4(23), 20101–20108 CrossRef CAS PubMed.
- L. Fabris, SERS on Semiconductor Substrates Receives an Electromagnetic Boost, Chem, 2023, 9(6), 1349–1352 CAS.
- X. Wang and L. Guo, SERS Activity of Semiconductors: Crystalline and Amorphous Nanomaterials, Angew. Chem., Int. Ed., 2020, 4231–4239 CrossRef.
- G. Song, S. Cong and Z. Zhao, Defect Engineering in Semiconductor-Based SERS, Chem. Sci., 2022, 13(5), 1210–1224 RSC.
- H. Wu, H. Wang and G. Li, Metal Oxide Semiconductor SERS-Active Substrates by Defect Engineering, Analyst, 2017, 142(2), 326–335 RSC.
- Samriti, V. Rajput, R. K. Gupta and J. Prakash, Engineering Metal Oxide Semiconductor Nanostructures for Enhanced Charge Transfer: Fundamentals and Emerging SERS Applications, J. Mater. Chem. C, 2022, 10(1), 73–95 RSC.
- S. Cong, Y. Yuan, Z. Chen, J. Hou, M. Yang, Y. Su, Y. Zhang, L. Li, Q. Li, F. Geng and Z. Zhao, Noble Metal-Comparable SERS Enhancement from Semiconducting Metal Oxides by Making Oxygen Vacancies, Nat. Commun., 2015, 6(1), 7800 CrossRef CAS.
- X. Zheng, F. Ren, S. Zhang, X. Zhang, H. Wu, X. Zhang, Z. Xing, W. Qin, Y. Liu and C. Jiang, A General Method for Large-Scale Fabrication of Semiconducting Oxides with High SERS Sensitivity, ACS Appl. Mater. Interfaces, 2017, 9(16), 14534–14544 CrossRef CAS.
- L. Yang, D. Yin, Y. Shen, M. Yang, X. Li, X. Han, X. Jiang and B. Zhao, Highly-Dispersed TiO2 Nanoparticles with Abundant Active Sites Induced by Surfactants as a Prominent Substrate for SERS: Charge Transfer Contribution, Phys. Chem. Chem. Phys., 2017, 19(33), 22302–22308 RSC.
- Y. Shan, Z. Zheng, J. Liu, Y. Yang, Z. Li, Z. Huang and D. Jiang, Niobium Pentoxide: A Promising Surface-Enhanced Raman Scattering Active Semiconductor Substrate, npj Comput. Mater., 2017, 1–6 Search PubMed.
- G. Kresse and J. Furthmüller, Efficient Iterative Schemes for Ab Initio Total-Energy Calculations Using a Plane-Wave Basis Set, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54(16), 11169–11186 CrossRef CAS PubMed.
- R. G. Parr, Density Functional Theory, Annu. Rev. Phys. Chem., 1983, 34(1), 631–656 CrossRef CAS.
- P. Giannozzi, S. Baroni, N. Bonini, M. Calandra, R. Car, C. Cavazzoni, D. Ceresoli, G. L. Chiarotti, M. Cococcioni, I. Dabo, A. D. Corso, S. de Gironcoli, S. Fabris, G. Fratesi, R. Gebauer, U. Gerstmann, C. Gougoussis, A. Kokalj, M. Lazzeri, L. Martin-Samos, N. Marzari, F. Mauri, R. Mazzarello, S. Paolini, A. Pasquarello, L. Paulatto, C. Sbraccia, S. Scandolo, G. Sclauzero, A. P. Seitsonen, A. Smogunov, P. Umari and R. M. Wentzcovitch, QUANTUM ESPRESSO: A Modular and Open-Source Software Project for Quantum Simulations of Materials, J. Phys.: Condens. Matter, 2009, 21(39), 395502 CrossRef PubMed.
- J. Heyd, G. E. Scuseria and M. Ernzerhof, Hybrid Functionals Based on a Screened Coulomb Potential, J. Chem. Phys., 2003, 118(18), 8207–8215 CrossRef CAS.
- L. Gao, F. Lemarchand and M. Lequime, Exploitation of Multiple Incidences Spectrometric Measurements for Thin Film Reverse Engineering, Opt. Express, 2012, 20(14), 15734–15751 CrossRef.
- G. Liu, S. Liu, H. Chen, X. Liu, X. Luo, X. Li and J. Ma, Highly [001]-Oriented N-Doped Orthorhombic Nb2O5 Microflowers with Intercalation Pseudocapacitance for Lithium-Ion Storage, Nanoscale, 2022, 14(32), 11710–11718 RSC.
- G. H. M. Gomes, J. B. Gabriel, C. G. O. Bruziquesi, H. V. Victoria, K. Krambrock, L. C. A. Oliveira and N. D. S. Mohallem, The Role of Oxygen Vacancies in TT-Nb2O5 Nanoparticles for the Photoconversion of Glycerol into Solketal, Ceram. Int., 2023, 49(9), 14719–14732 CrossRef CAS.
- X. Wang, W. Shi, S. Wang, H. Zhao, J. Lin, Z. Yang, M. Chen and L. Guo, Two-Dimensional Amorphous TiO2 Nanosheets Enabling High-Efficiency Photoinduced Charge Transfer for Excellent SERS Activity, J. Am. Chem. Soc., 2019, 141(14), 5856–5862 CrossRef CAS.
- L. Yang, Y. Wei, Y. Song, Y. Peng, Y. Yang and Z. Huang, Surface-Enhanced Raman Scattering from Amorphous Nanoflower-Structural Nb2O5 Fabricated by Two-Step Hydrothermal Technology, Mater. Des., 2020, 193, 108808 CrossRef CAS.
- A. M. Raba, J. Bautista-Ruíz and M. R. Joya, Synthesis and Structural Properties of Niobium Pentoxide Powders: A Comparative Study of the Growth Process, Mater. Res., 2016, 19(6), 1381–1387 CrossRef CAS.
- Y. B. Hu, J. Deng, C. Zhao, F. S. Pan and J. Peng, Microstructure and Mechanical Properties of Mg-Gd-Zr Alloys with Low Gadolinium Contents, J. Mater. Sci., 2011, 46(17), 5838–5846 CrossRef CAS.
- J.-M. Zhang, Y. Zhang, K.-W. Xu and V. Ji, Young's Modulus Surface and Poisson's Ratio Curve for Orthorhombic Crystals, J. Chem. Crystallogr., 2008, 38(10), 733–741 CrossRef CAS.
- G. Rajender and P. K. Giri, Strain Induced Phase Formation, Microstructural Evolution and Bandgap Narrowing in Strained TiO2 Nanocrystals Grown by Ball Milling, J. Alloys Compd., 2016, 676, 591–600 CrossRef CAS.
- M. R. Joya, J. J. Barba Ortega, A. M. Raba Paez, J. G. Da Silva Filho and P. D. T. Cavalcante Freire, Synthesis and Characterization of Nanoparticles of Niobium Pentoxide with Orthorhombic Symmetry, Metals, 2017, 7(4), 142 CrossRef CAS.
- K. Skrodczky, M. M. Antunes, X. Han, S. Santangelo, A. A. Valente, N. Pinna and P. A. Russo, Niobium pentoxide nanomaterials with distorted Structures as Efficient Acid Catalysts, Commun. Chem., 2019, 1–11 Search PubMed.
- P. K. Giri, S. Bhattacharyya, D. K. Singh, R. Kesavamoorthy, B. K. Panigrahi and K. G. M. Nair, Correlation between Microstructure and Optical Properties of ZnO Nanoparticles Synthesized by Ball Milling, J. Appl. Phys., 2007, 102(9), 93515 CrossRef.
- G. Falk, M. Borlaf, M. J. López-Muñoz, J. C. Fariñas, J. B. Rodrigues Neto and R. Moreno, Microwave-Assisted Synthesis of Nb2O5 for Photocatalytic Application of Nanopowders and Thin Films, J. Mater. Res., 2017, 32(17), 3271–3278 CrossRef CAS.
- L. Kong, C. Zhang, J. Wang, W. Qiao and L. Ling, Nanoarchitectured Nb2O5 Hollow, Nb2O5 @ Carbon and NbO2 @ Carbon Core-Shell Microspheres for Ultrahigh-Rate Intercalation Pseudocapacitors, Sci. Rep., 2016, 1–10 Search PubMed.
- Y. Zou, C. Wang, H. Chen, H. Ji, Q. Zhu and W. Yang, Scalable and Facile Synthesis of V2O5 Nanoparticles via Ball Milling for Improved Aerobic Oxidative Desulfurization, Green Energy Environ., 2021, 6(2), 169–175 CrossRef CAS.
- B. Christian Enger, R. Lødeng and A. Holmen, A Review of Catalytic Partial Oxidation of Methane to Synthesis Gas with Emphasis on Reaction Mechanisms over Transition Metal Catalysts, Appl. Catal., A, 2008, 346(1), 1–27 CrossRef.
- J. Wang, Z. Wang, B. Huang, Y. Ma, Y. Liu, X. Qin, X. Zhang and Y. Dai, Oxygen Vacancy Induced Bandgap Narrowing and Enhanced Visible Light Photocatalytic Activity of ZnO, ACS Appl. Mater. Interfaces, 2012, 4(8), 4024–4030 CrossRef CAS PubMed.
- C. L. Ücker, L. T. Gularte, C. D. Fernandes, V. Goetzke, E. C. Moreira, C. W. Raubach, M. L. Moreira and S. S. Cava, Investigation of the Properties of Niobium Pentoxide for Use in Dye-Sensitized Solar Cells, J. Am. Ceram. Soc., 2019, 102(4), 1884–1892 CrossRef.
- B. Santara, P. K. Giri, K. Imakita and M. Fujii, Evidence of Oxygen Vacancy Induced Room Temperature Ferromagnetism in Solvothermally Synthesized Undoped TiO2 Nanoribbons, Nanoscale, 2013, 5(12), 5476–5488 RSC.
- A. M. Al-Baradi, M. M. El-Nahass, A. M. Hassanien, A. A. Atta, M. S. Alqahtani and A. O. Aldawsari, Influence of RF Sputtering Power on Structural and Optical Properties of Nb2O5 Thin Films, Optik, 2018, 168, 853–863 CrossRef CAS.
- S. S. Raj, S. K. Gupta, N. Pathak, V. Grover and A. K. Tyagi, Origin of Visible Photoluminescence in Combustion Synthesized α-Al2O3: Photoluminescence and EPR Spectroscopy, Adv. Powder Technol., 2017, 28(6), 1505–1510 CrossRef CAS.
- M. Wainwright and K. B. Crossley, Methylene Blue – a Therapeutic Dye for All Seasons?, J. Chemother., 2002, 14(5), 431–443 CrossRef CAS.
- T. Thu, H. Pham, X. Hoa, T. Thu and N. Xuan, Enhance Raman Scattering for Probe Methylene Blue Molecules Adsorbed on ZnO Microstructures Due to Charge Transfer Processes, Opt. Mater., 2021, 120, 111460 CrossRef.
- A. Rakovich, Y. P. Rakovich and J. F. Donegan, Optical Studies of the Methylene Blue-Semiconductor Nanocrystals Hybrid System, e-J. Surf. Sci. Nanotechnol., 2009, 7, 349–353 CrossRef CAS.
- I. Alessandri and J. R. Lombardi, Enhanced Raman Scattering with Dielectrics, Chem. Rev., 2016, 116(24), 14921–14981 CrossRef CAS.
- S.-Y. Ding, E.-M. You, Z.-Q. Tian and M. Moskovits, Electromagnetic Theories of Surface-Enhanced Raman Spectroscopy, Chem. Soc. Rev., 2017, 46(13), 4042–4076 RSC.
- J. R. Lombardi and R. L. Birke, A Unified View of Surface-Enhanced Raman Scattering, Acc. Chem. Res., 2009, 42(6), 734–742 CrossRef CAS.
- Z. Sun, Y. Gao, C. Ban, J. Meng, J. Wang, K. Wang and L. Gan, 3 nm-Wide WO3−x Nanorods with Abundant Oxygen Vacancies as Substrates for High-Sensitivity SERS Detection, ACS Appl. Nano Mater., 2023, 6(10), 8635–8642 CrossRef CAS.
- J. R. Lombardi and R. L. Birke, Theory of Surface-Enhanced Raman Scattering in Semiconductors, J. Phys. Chem. C, 2014, 118(20), 11120–11130 CrossRef CAS.
- X. Wang, W. Shi, G. She and L. Mu, Using Si and Ge Nanostructures as Substrates for Surface-Enhanced Raman Scattering Based on Photoinduced Charge Transfer Mechanism, J. Am. Chem. Soc., 2011, 133(41), 16518–16523 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d3nr04112c |
| This journal is © The Royal Society of Chemistry 2024 |