Is the chemistry of lawrencium peculiar?

Abstract

It is explicitly verified that the atomic 7p¹ ground-state configuration of Lr originates from relativistic effects. Without relativity one has 6d¹. All three ionization potentials IP_1–3 of Lr resemble those of Lu. Simple model studies on mono- and trihydrides, monocarbonyls or trichlorides suggest no major chemical differences between Lr and the lanthanides.

---

1 Introduction

The periodic table is about chemistry. The group is related to the number of valence electrons and the period is related to the number of nodes in the radial functions of these electrons. In lawrencium, ₁₀₃Lr, counting the filled 5f shell as the ‘core’, there are three valence electrons. It had been debated for some time, whether they are 7s²6d¹ or 7s²7p_1/2¹, until both an experiment¹ and also the latest calculations supported the latter alternative. That 7p¹ atomic ground state was first surmised by Brewer² and first calculated by Desclaux and Fricke.³ Large MCDF calculations by Zou and Froese Fischer⁴ support the 5d¹ and 7p¹ ground states for Lu and Lr, respectively, and yield very different oscillator strengths. For Lr, however, they are not yet experimentally confirmed.

This does not yet settle the question on the chemical behaviour. If all three valence electrons are formally ionized away, in an Lr(III) compound, lawrencium clearly belongs to Group 3 in Period 7, and nothing unexpected has happened in its chemistry.

The three first ionization potentials of Lr are compared with those of La–Lu in Fig. 1. They are quite similar, especially with Lu. Therefore the ionic chemistry of Lr could be expected to be similar to that of the lanthanides.

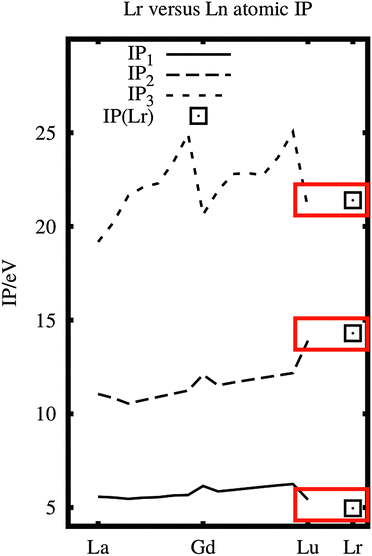Fig. 1 The ionization potentials of La–Lu (experimental data⁵) and Lr (experimental and calculated IP₁;¹ calculated IP_2,3⁶). Note the similarity of Lu and Lr.

Experimentally this is what happened, see Brüchle et al.,⁷ Hoffman et al.⁸ and Scherer et al.,⁹ all in 1988. Brüchle and Hoffman found that Lr(III) had a similar elution behaviour to the latter Ln(III) and Scherer found no evidence for a possible reduction to lower oxidation states than Lr(III) in aqueous solution. Recently, in reductive surroundings, all the divalent lanthanide oxidation states Ln(II) have also been obtained.¹⁰ These divalent lanthanide, Ln(II), compounds are mostly 5d¹. No such experiments exist on Lr.

Calculations suggest that the free-atom Lr(I) and Lr(II) are 7s² and 7s¹, respectively,^5,6,11 in contrast to the quoted 5d¹ for Ln(II) in compounds.¹⁰ The stabilisation of the 7s shell in Group 3 can be compared with that of the 6s shell in Group 13, which contributes to the chemical difference between indium and thallium, having the main oxidation states In(III) and Tl(I), respectively. Similarly, for lead, the relativistic stabilization of the 6s shell favours the divalent Pb(II) state in PbO or PbSO₄ and destabilizes the Pb(IV) state in PbO₂, thereby explaining most of the voltage of the lead battery.¹² In these main-group cases the relativistic stabilization of an ns shell leads to different main oxidation states in Periods 5 and 6. One possibility considered here is whether one could have a similar change between lanthanides and actinides. Recall that the relativistic stabilization of valence s shells down the same column increases as Z², where Z is the nuclear charge.

2 Atomic results

We first verify the relativistic origin of the ground-state change from 6d¹ to 7p¹, see Table S1 in the ESI.† Compared with the non-relativistic results, Dirac–Fock (DF) shifts down the relative energy of (n + 1) ²P to n²D by nearly 3 eV, and changes the ground state configuration. The relativistic effect is so large that already DF-level evidence makes sense. MCDF results were reported by Fritzsche et al.¹³

The calculated orbital energies for Tl and Lr atoms are shown in Fig. 2. It is seen that the relativistic stabilization of the Tl 6s shell is substantial, making its energy comparable to the ligand orbital energy (here H). In contrast, the Lr 7s orbital energy is small, despite a larger Z.

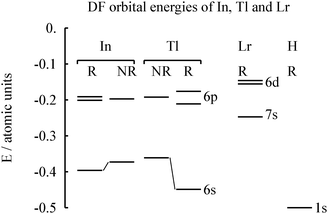Fig. 2 The calculated relativistic (R) and non-relativistic (NR) Dirac–Fock orbital energies of neutral In, Tl and Lr atoms. For lawrencium, the electron configuration 7s²6d¹ is assumed. Values from Desclaux.¹⁵

Does the atomic ground state matter in chemistry? As seen in Fig. 2, the valence orbital energies of the electropositive element Lr are small and hence in compounds these electrons, whether 7s, 6d or 7p, will largely go away, anyway. Group 13 is more electronegative¹⁴ than Group 3.

Why is Lr, like other lanthanides and actinides, so electropositive? A broad-brush explanation could be that they all belong to Group 3 and the electronegativities in the Periodic table increase from left to right (from Group 1 to Group 18), probably due to increasing partial screening by the fellow valence electrons.

3 Molecular results

3.1 Hydrides

We first consider the simple hydride models and calculate the reaction energy, ΔE for the model reaction

MH₃ → MH + H₂ (1)

for M = Lr, Lu, In and Tl. As seen in Table 1, this ΔE is negative for thallium which clearly prefers to be Tl(I), and positive for the other four metals, which prefer being M(III), including Lr(III). Tl(I) is an example of the relativistic 6s² inert pair.

Table 1 Reaction energies ΔE for MH₃ → MH + H₂ (1) (in eV). The In and Tl results are from Vest et al.¹⁶ The other results from the present work. As seen from Table 2, LrH₃ is C_3v

M	HF	MP2	CCSD(T)	DFT
a Triple-zeta basis. Other wave function methods use the double-zeta basis.
Lr	+0.17	−0.07	+0.10	+0.39
Lr^a	+0.14	−0.03	+0.13
Ac	+0.44	+0.10	+0.25	+0.89
Lu	+0.88	+0.66	+0.72	+0.91
In			+0.07
Tl			−0.72

---

The structural parameters are given in Table 2.

Table 2 Geometrical two-component (2c) DFT parameters of MH, MH₂, MH₃, MCO, MCl₃ and [(Cp′)₃M]⁻ (M = Lu, Lr). Lengths in Å, angles in degrees

Molecule	Symmetry	Bond length	Bond angle
a Ref. 17. b Ref. 10. c Shortest M–C.
LuH	Linear	1.895
LrH	Linear	1.960
LuH2	C 2v	1.915	113.5
LrH2	C 2v	1.954	110.6
	C 2v	2.015	117.5
LuH3	C 3v	1.921	112
LrH3	C 3v	1.940	107
LuCO	Linear	2.297 (Lu–C), 1.167	180
LrCO	Linear	2.384 (Lr–C), 1.169	180
LuCl3	D 3h	2.394	120
LrCl3	C 3v	2.424	113
[(Cp′)3Lu]^−	C 1	2.635^b^,^c
[(Cp′)3Lr]^−	C 1	2.697^c

---

3.2 Monocarbonyls

We then compare LrCO with the series LnCO, Ln = La–Lu, studied both experimentally and theoretically by Xu et al.¹⁹ There the three last members Ln = Tm, Yb, and Lu which could not be made, and they had theoretically weak bonds, for Lu with a σ²π¹ valence configuration. We now find that Lr behaves just like Lu, which further supports putting it under Lu in the Periodic table. At the CCSD(T) level, LrCO is 1.0 eV below Lr + CO, while LuCO is 0.77 eV below Lu + CO. The attempts to produce LuCO¹⁹ nevertheless failed.

Population analyses are shown in Tables 3–5. Projection analysis is stable regarding different types of functionals. In this study, Mulliken populations agree well with the projection analysis. There is a high correlation between Lu and Lr electronic configurations in all the hydrides and carbonyls.

Table 3 Population analysis of LrH, LrH₂, LrH₃, LuCO and LrCO. P: projection analysis;¹⁸ M: Mulliken population. G: Four-component Hamiltonian with the DZ Gaussian basis set; S: two-component Hamiltonian with the DZ Slater basis set

Mol.	Type	Functional	Valence population
LuH	P, G	PBE	Lu 6s(1.78)5d(0.71)6p(0.22) H 1s(1.26)
	M, S	PBE	Lu 6s(1.77)5d(0.68)6p(0.20) H 1s(1.34)
LrH	P, G	PBE	Lr 7s(1.82)6d(0.63)7p(0.28) H 1s(1.25)
	P, G	PBE0	Lr 7s(1.80)6d(0.57)7p(0.28) H 1s(1.30)
	P, G	CAMB3LYP	Lr 7s(1.80)6d(0.53)7p(0.27) H 1s(1.34)
	M, S	PBE	Lr 7s(1.83)6d(0.61)7p(0.21) H 1s(1.34)
LuH2	M, S	PBE	Lu 6s(1.03)5d(0.96)6p(0.27) H 1s(1.35)
LrH2	M, S	PBE	Lr 7s(1.19)6d(0.87)7p(0.25) H 1s(1.33)
LuH3	P, G	PBE	Lu 6s(0.74)5d(1.06)6p(0.27) H 1s(1.29)
	M, S	PBE	Lu 6s(0.66)5d(0.97)6p(0.25) H 1s(1.36)
LrH3	P, G	PBE	Lr 7s(0.89)6d(1.05)7p(0.25) H 1s(1.26)
	M, S	PBE	Lr 7s(0.84)6d(0.94)7p(0.17) H 1s(1.34)
LuCO	M, S	PBE	Lu 6s(1.77)5d(0.75)6p(0.18) C 2sp(3.90) O 2sp(6.25)
LrCO	M, S	PBE	Lr 7s(1.82)6d(0.66)7p(0.20) C 2sp(3.91) O 2sp(6.27)

---

Table 4 Natural electron configurations of LrH, LrH₃, LuCO and LrCO. The density matrices are from ZORA1c and PBE calculations. At this level, an NBO was available

Mol.	Natural electron configuration
LrH	[core]7s(1.92) 6d(0.34) 7p(0.04)
LrH3	[core]7s(0.84) 6d(0.67) 7p(0.01)
LrCO	[core]7s(1.93) 6d(0.51) 7p(0.05)
LuCO	[core]6s(1.90) 5d(0.56) 6p(0.06)

---

Table 5 Frontier HF orbitals of LrH, LrH₃, LuCO and LrCO. The first two closed-shell molecules are computed using an X2C Hamiltonian, while the latter two open-shell ones use a scalar relativistic ECP. Energies in eV. Mulliken populations in %. h = HOMO. At the spin–orbit-split two-component level, only Mulliken population analysis was available

Mol.	Orb.	Energy	Populations
LrH	h	−6.31	Lr s(64), Lr p(14), Lr d(13), H s(7)
	h-1	−10.61	Lr s (28), Lr p(5), Lr d(10), H s(56)
LrH3	h	−9.65	Lr p(8), Lr d(26), H s(67)
	h-1	−10.16	Lr p(14), Lr d(18), H s(65)
	h-2	−11.65	Lr s(39), H s(55)
LrCO	h	−6.31	Lr p(10), Lr d(26), C p(47), O p(16)
	h-1	−6.92	Lr s(86), Lr p(7), Lr d(6)
LuCO	h	−6.72	Lu p(11), Lu d(23), C p(44), O p(15)
	h-1	−6.38	Lu s(84), Lu p(8), Lu d(8)

---

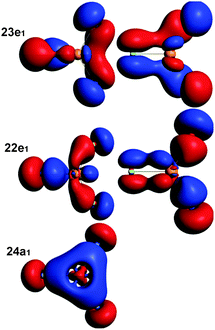
The C–O stretching frequencies are 1897 and 1921 cm⁻¹ for LrCO and LuCO, respectively. The valence orbitals of LuCO and LrCO are compared in Fig. 3 and found to be very similar. We conclude that although the p populations strongly depend on the method of calculation, Mulliken, NBO (Natural Bond Orbital) or projection, the results for Lu 6p and Lr 7p are closely similar.

Fig. 3 The HF π (HOMO), σ (HOMO−1) and σ donation molecular orbitals (from left to right) of the monocarbonyls LuCO and LrCO with ECP. They are fairly similar to each other.¹⁹ Isodensity value = 0.05 a.u.

3.3 Lawrencium trichloride and a divalent complex

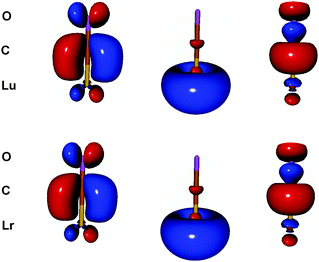
One feature of the bonding in lanthanide chlorides is the pπ–dπ bond. It is also observed in LrCl₃. Note that unlike in D_3h LuCl₃, the geometry of LrCl₃ is C_3v, with an out-of-plane vibrational frequency of only 48 cm⁻¹. For the bonding molecular orbitals, see Fig. 4.

Fig. 4 The molecular orbitals of the C_3v LrCl₃ with 3p(Cl)–6d(Lr) bonds. They are fairly similar to those of LuCl₃. The ‘e’ orbitals are doubly degenerate and both components are shown. Isodensity value = 0.05.

In recent years, one breakthrough in lanthanide chemistry is that divalent complexes were synthesized and characterized for all lanthanides. We now studied an Lr complex with the same ligand as that for Ln, i.e. C₅H₄SiMe₃ (Cp′). Experimentally, a potassium atom in a crown ether¹⁰ functioned as the counterion of [Ln(Cp′)₃]⁻.

A stable geometry was found for this complex anion. The electronic structure is similar to that of Lu. The metal configuration is 6d¹. Spin–orbit effects were included in the calculation. As seen from Fig. 5, this HOMO is a d¹ orbital on Lr.

Fig. 5 The HOMO orbital of [(Cp′)₃Lr]⁻. Isodensity value = 0.05 a.u.

The structures of all systems are given in the ESI.†

4 Relation to the periodic table

Three different choices can be outlined for the f-element rows:

1. Fourteen-element rows, La–Yb and Ac–No. Put Lu and Lr in Group 3. Chosen by Jensen²⁰ and currently Wikipedia.

2. Fourteen-element rows, Ce–Lu and Th–Lr. Put La and Ac in Group 3. Chosen by Lavelle,²¹ the Royal Society of Chemistry and the American Chemical Society.

3. Fifteen-element rows, La–Lu and Ac–Lr. This includes f⁰ among the f¹ to f¹⁴ series. All elements are mostly trivalent. Their ionic and covalent²² radii form a continuous series. Now chosen by IUPAC²³ and by us. To us the atomic ground state is less important than the chemical bonding, in the systems so far considered.

5 Computational details

The geometries were optimized at the ZORA2c²⁴ level, DFT (PBE functional²⁵) with TZ2P²⁶ Slater basis sets. The vibrational frequencies were obtained to confirm the minima. However, [(Cp′)₃Lr]⁻ was optimized with the TPSSh functional^27,28 to compare with the published [(Cp′)₃Lu]⁻ results. Solvent effects were considered by the COSMO model²⁹ with tetrahydrofurane (THF) parameters. For more details, see the computational part of ref. 10. ADF 2016^30,31 and Turbomole 7.02³² packages were used. To calculate more accurate energetics, the two-component (2c)-MP2^33,34 and (2c)-CCSD(T)³⁵ as implemented in Dirac 15.0,³⁶ and CCSD(T) implemented in Molpro 2015.1^37,38 were employed. The basis sets are Dyall all-electron double zeta³⁹ and ECP from the Stuttgart/Cologne group,^40,41 respectively.

6 Conclusion

All three ionization potentials of the lawrencium atom resemble those of the lanthanides, especially lutetium. Despite the different atomic ground states of d¹ and (p*)¹ for Lu and Lr, respectively, their chemical behaviour in the present systems is found to be similar. Nothing prevents one from keeping a fifteen-element trivalent actinide row Ac–Lr, under the trivalent lanthanide row La–Lu. This entirely avoids the issues arising from fourteen-element rows.^20,21

Acknowledgements

This project is supported by grant 275845 from The Academy of Finland to Professor Dage Sundholm. WHX thanks the China Scholarship Council and the Northwest University, Xi'an for a leave of absence and support (No. 13NW24). CSC, Espoo, Finland, provided both software and computer time. PP is grateful to the Magnus Ehrnrooth Foundation for a travel grant.

References

1. T. K. Sato, M. Asai, A. Borschevsky, T. Stora, N. Sato, Y. Kaneya, K. Tsukada, Ch. E. Düllmann, K. Eberhardt, E. Eliav, S. Ichikawa, U. Kaldor, J. V. Kratz, S. Miyashita, Y. Nagame, K. Ooe, A. Osa, D. Renisch, J. Runke, M. Schädel, O. Thörle-Pospiech, A. Toyoshima and N. Trautmann, Nature, 2015, 520, 209–211.
2. L. Brewer, J. Opt. Soc. Am., 1971, 61, 1101–1111.
3. J. P. Desclaux and B. Fricke, J. Phys., 1980, 41, 943–946.
4. Y. Zou and C. Froese Fischer, Phys. Rev. Lett., 2002, 88, 183001.
5. A. Kramida, Yu. Ralchenko, J. Reader and the NIST ASD Team, Atomic Spectra Database (ver. 5.2), 2016, v 5.2.
6. X.-Y. Cao and M. Dolg, Mol. Phys., 2003, 101, 961–969.
7. W. Brüchle, M. Schädel, U. W. Scherer, J. V. Kratz, K. E. Gregorich, D. Lee, M. Nurmia, R. M. Chasteler, H. L. Hall, R. A. Henderson and D. C. Hoffman, Inorg. Chim. Acta, 1988, 146, 267–276.
8. D. C. Hoffman, R. A. Henderson, K. E. Gregorich, D. A. Bennett, R. M. Chasteler, C. M. Chasteler, C. M. Gannett, H. L. Hall, D. M. Lee, M. Nurmia, S. Cai, R. Agarwal, A. W. Charlop, Y. Y. Chu, G. T. Seaborg and R. J. Silva, J. Radioanal. Nucl. Chem., 1988, 124, 135–144.
9. U. W. Scherer, J. V. Kratz, M. Schädel, W. Brüchle, K. E. Grigorich, R. A. Henderson, D. Lee, M. Nurmia and D. C. Hoffman, Inorg. Chim. Acta, 1988, 146, 249–254.
10. M. R. MacDonald, J. E. Bates, J. W. Ziller, F. Furche and W. J. Evans, J. Am. Chem. Soc., 2013, 135, 9857–9868.
11. E. Eliav, U. Kaldor and Y. Ishikawa, Phys. Rev. A: At., Mol., Opt. Phys., 1995, 52, 291–296.
12. R. Ahuja, A. Blomqvist, P. Larsson, P. Pyykkö and P. Zaleski-Ejgierd, Phys. Rev. Lett., 2011, 106, 018301.
13. S. Fritzsche, C. Z. Dong, F. Koike and A. Uvarov, Eur. Phys. J. D, 2007, 45, 107–113.
14. L. Pauling, The Nature of the Chemical Bond, Cornell Univ. Press, Ithaca, NY, 3rd edn, 1960.
15. J. P. Desclaux, At. Data Nucl. Data Tables, 1973, 12, 311–406.
16. B. Vest, K. Klinkhammer, C. Thierfelder, M. Lein and P. Schwerdtfeger, Inorg. Chem., 2009, 48, 7953–7961.
17. K. Balasubramanian, J. Chem. Phys., 2002, 116, 3568–3575.
18. S. Dubillard, J.-B. Rota, T. Saue and K. Faegri, J. Chem. Phys., 2006, 124, 154307.
19. W.-H. Xu, X. Jin, M.-H. Chen, P. Pyykkö, M.-F. Zhou and J. Li, Chem. Sci., 2012, 3, 1548–1554.
20. W. B. Jensen, Found. Chem., 2015, 17, 23–31.
21. L. Lavelle, J. Chem. Educ., 2008, 85, 1482–1483.
22. P. Pyykkö, J. Phys. Chem. A, 2015, 119, 2326–2337.
23. IUPAC, iupac.org/what-we-do/periodic-table-of-elements/, Downloaded 4 May 2016.
24. E. van Lenthe, E. J. Baerends and J. G. Snijders, J. Chem. Phys., 1993, 99, 4597–4610.
25. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868.
26. E. van Lenthe and E. J. Baerends, J. Comput. Chem., 2003, 24, 1142–1156.
27. J. Tao, J. P. Perdew, V. N. Staroverov and G. E. Scuseria, Phys. Rev. Lett., 2003, 91, 146401.
28. V. Staroverov, G. Scuseria, J. Tao and J. Perdew, J. Chem. Phys., 2003, 119, 12129–12137.
29. A. Klamt and G. Schüürmann, J. Chem. Soc., Perkin Trans. 2, 1993, 799–805.
30. ADF2016, SCM, Theoretical Chemistry, Vrije Universiteit, Amsterdam, The Netherlands, http://www.scm.com.
31. G. te Velde, F. M. Bickelhaupt, E. J. Baerends, C. Fonseca Guerra, S. J. A. van Gisbergen, J. G. Snijders and T. Ziegler, J. Comput. Chem., 2001, 22, 931–967.
32. TURBOMOLE V7.02 2015, a development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007, TURBOMOLE GmbH, since 2007; available from http://www.turbomole.com.
33. S. Knecht and T. Saue, X2Cmod: A modular code for Exact-Two-Component Hamiltonian Transformations. 2010–2013 with contributions from M. Ilias, H. J. Aa. Jensen and M. Repisky, 2010.
34. J. K. Laerdahl, T. Saue and K. Faegri Jr, Theor. Chem. Acc., 1997, 97, 177–184.
35. L. Visscher, T. J. Lee and K. G. Dyall, J. Chem. Phys., 1996, 105, 8769–8776.
36. R. Bast, T. Saue, L. Visscher, H. J. A. Jensen et al., DIRAC, a relativistic ab initio electronic structure program, Release DIRAC15 (2015), see http://www.diracprogram.org.
37. H.-J. Werner, P. Knowles, G. Knizia, F. Manby and M. Schütz, et al. MOLPRO, version 2015.1, a package of ab initio programs, 2015, see http://www.molpro.net.
38. H.-J. Werner, P. J. Knowles, G. Knizia, F. R. Manby and M. Schütz, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2012, 2, 242–253.
39. K. G. Dyall, Theor. Chem. Acc., 2012, 131, 1–11.
40. X.-Y. Cao and M. Dolg, J. Chem. Phys., 2001, 115, 7348–7355.
41. X.-Y. Cao, M. Dolg and H. Stoll, J. Chem. Phys., 2003, 118, 487–496.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c6cp02706g

---